Optimization of TSPWM for Common-Mode Voltage Reduction in Vehicular Electric Drive System
Abstract
:1. Introduction
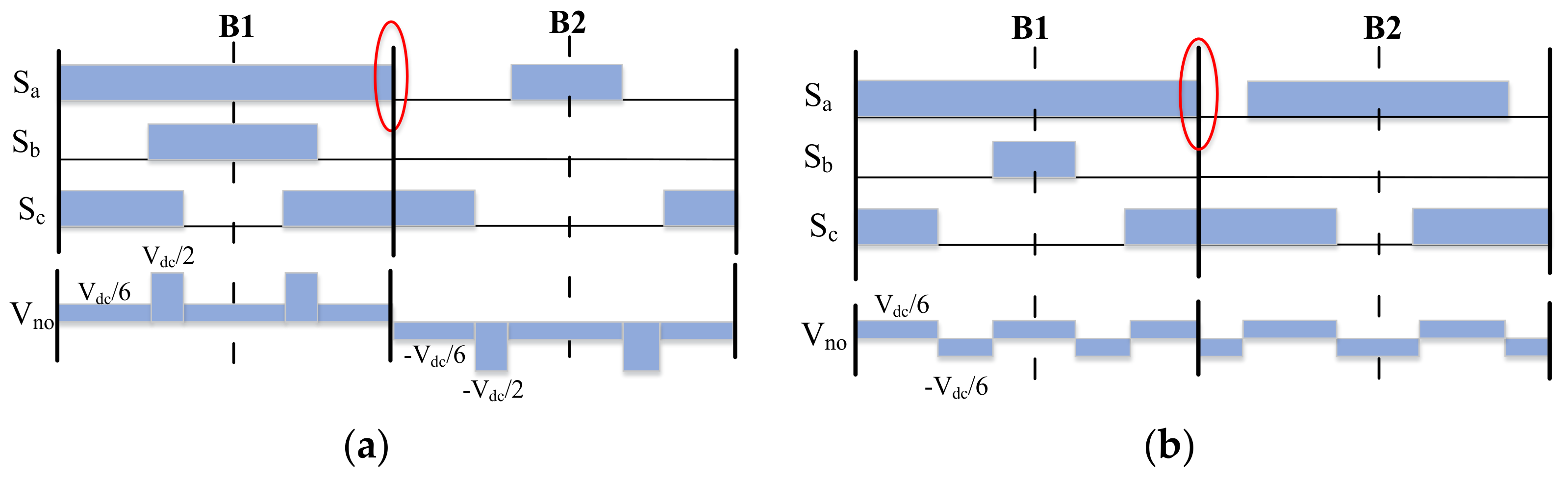
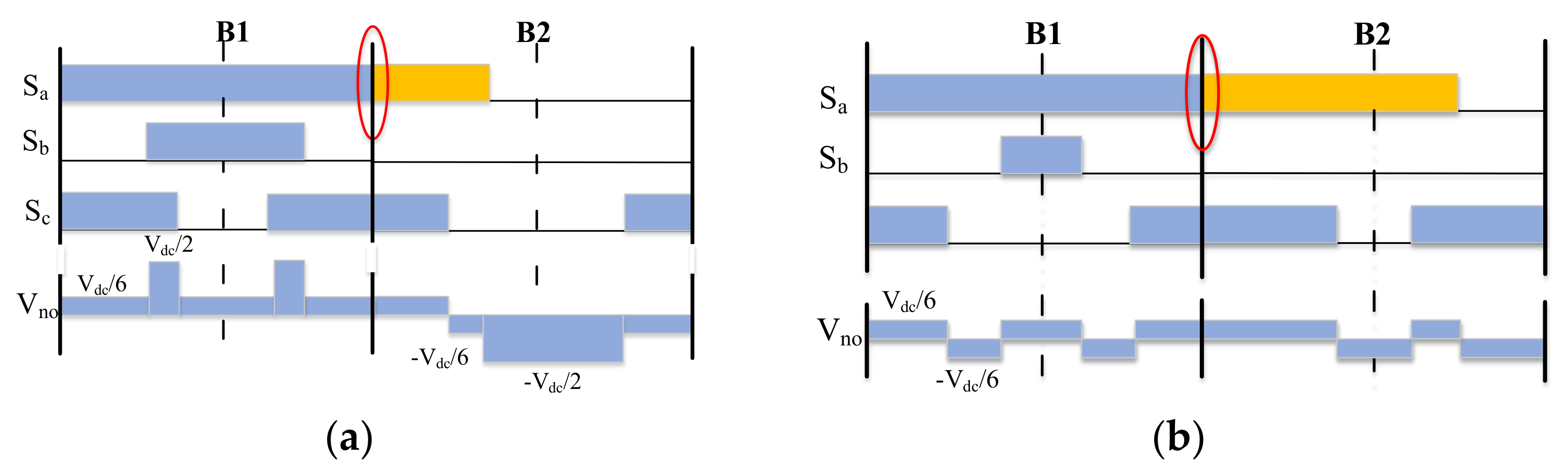
- The standard TSPWM needs to switch three times in one period when the voltage vector transit from one sector to another, which is not suitable for vehicular electric drive applications. This paper combines different alignment technology in the sector transition process. The switch times are reduced to one while the CMV can keep low in the whole control range.
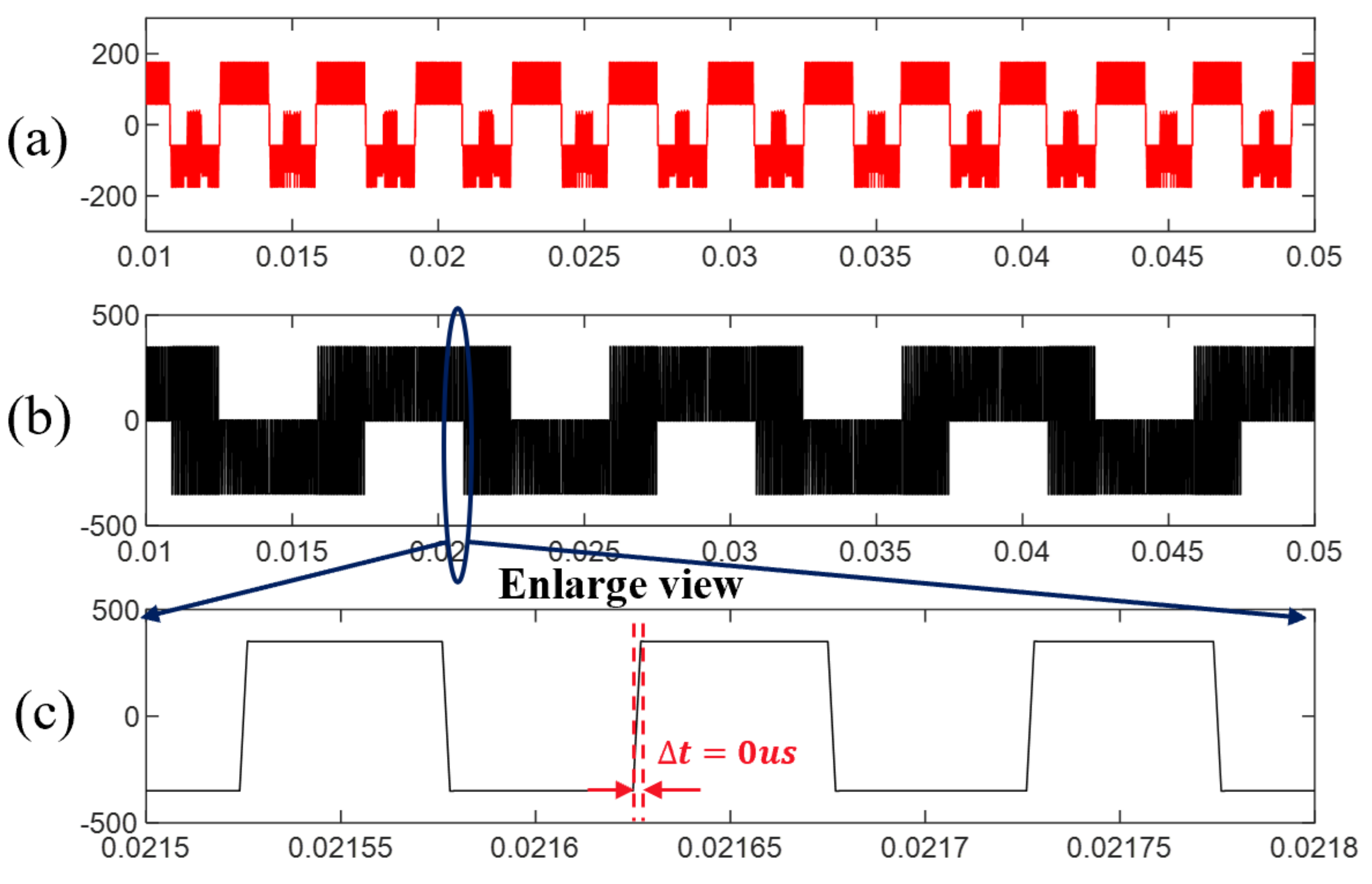
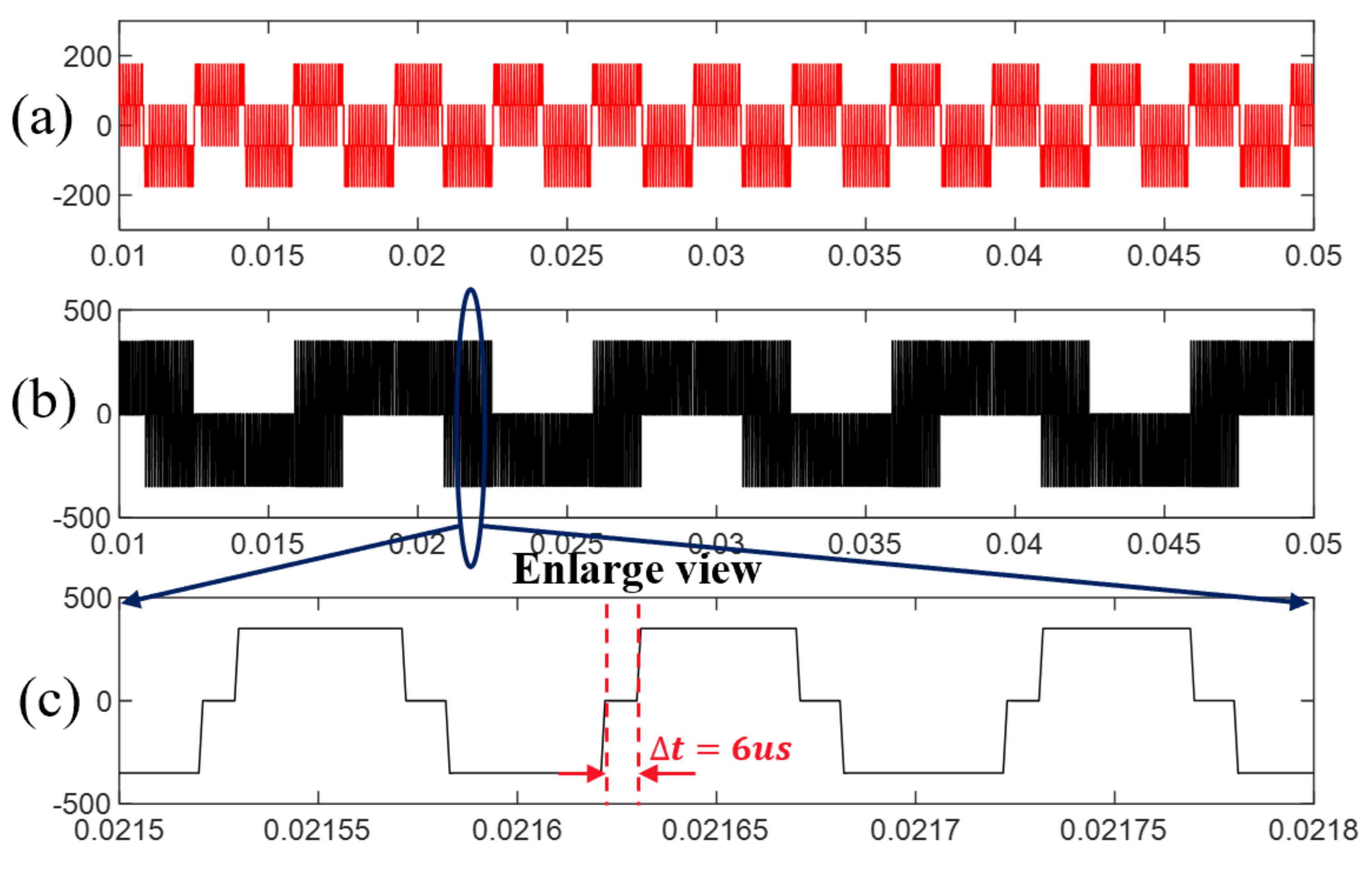
- In the standard TSPWM, the minimum zero-voltage time interval between line-to-line voltage pulse reversals is close to zero in the border between the high and low region of TSPWM, which causes overvoltage and can be dangerous in a vehicular electric drive application. This paper proposes an unsymmetric translation method to reconstruct the voltage vector, and then the minimum zero-voltage time interval is controlled to a safe lower limit.
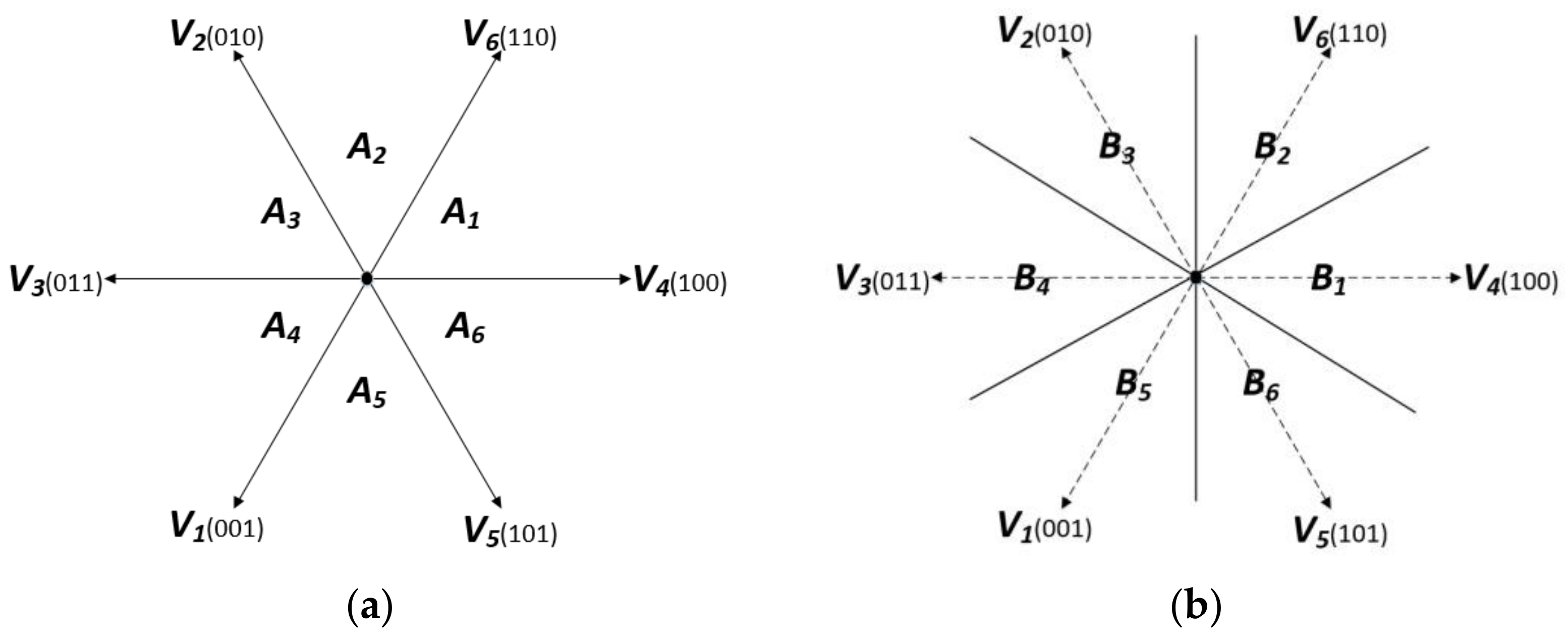
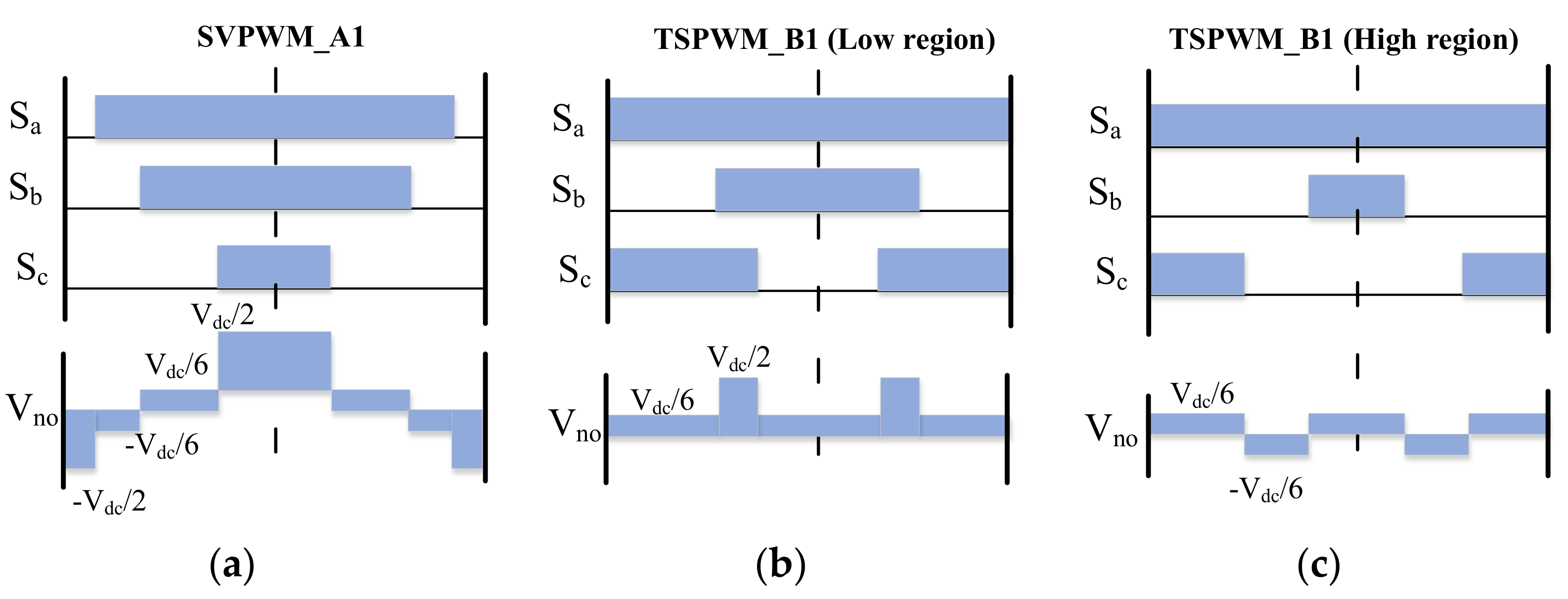
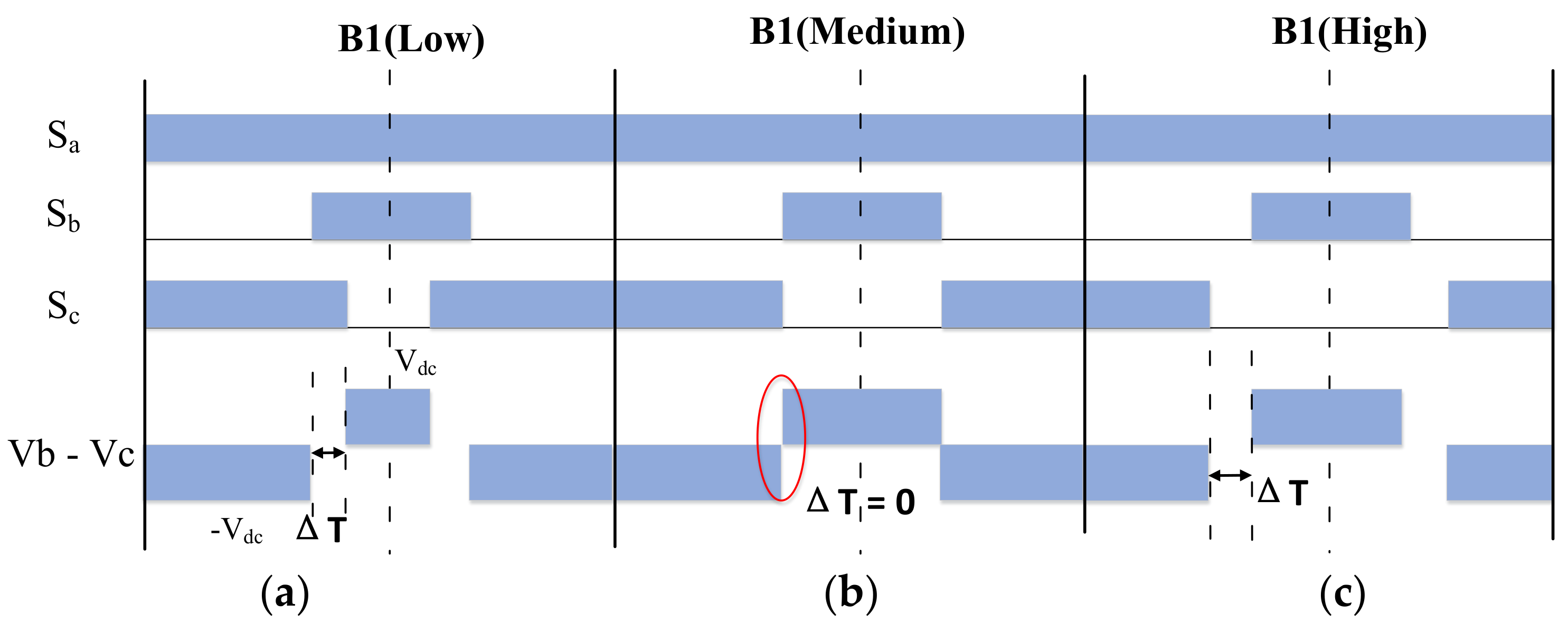
2. Review of the Existing TSPWM Technology
3. Optimization of TSPWM
3.1. Discontinuous Switching between Different Sectors
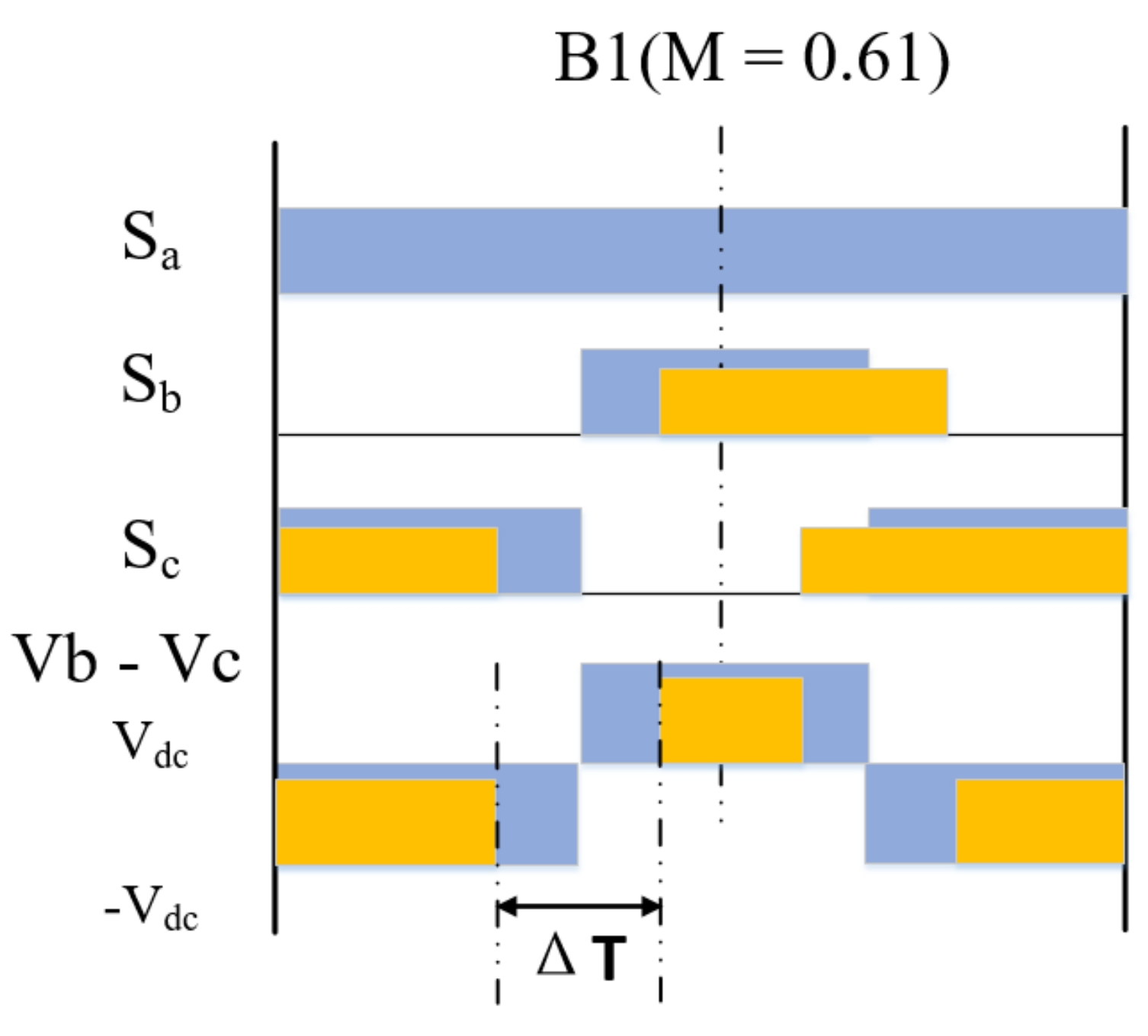
3.2. Increase Zero-Voltage Time Interval
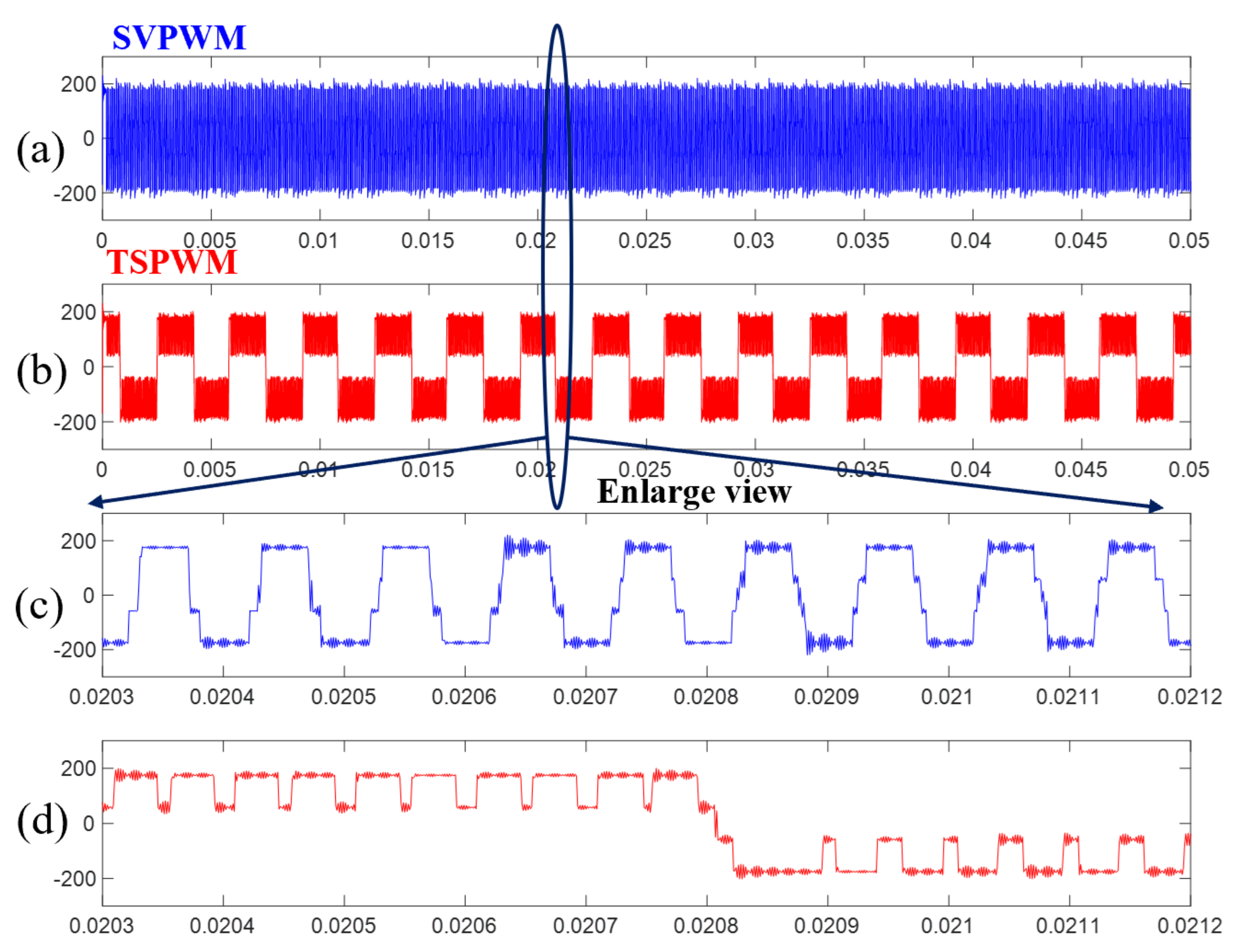
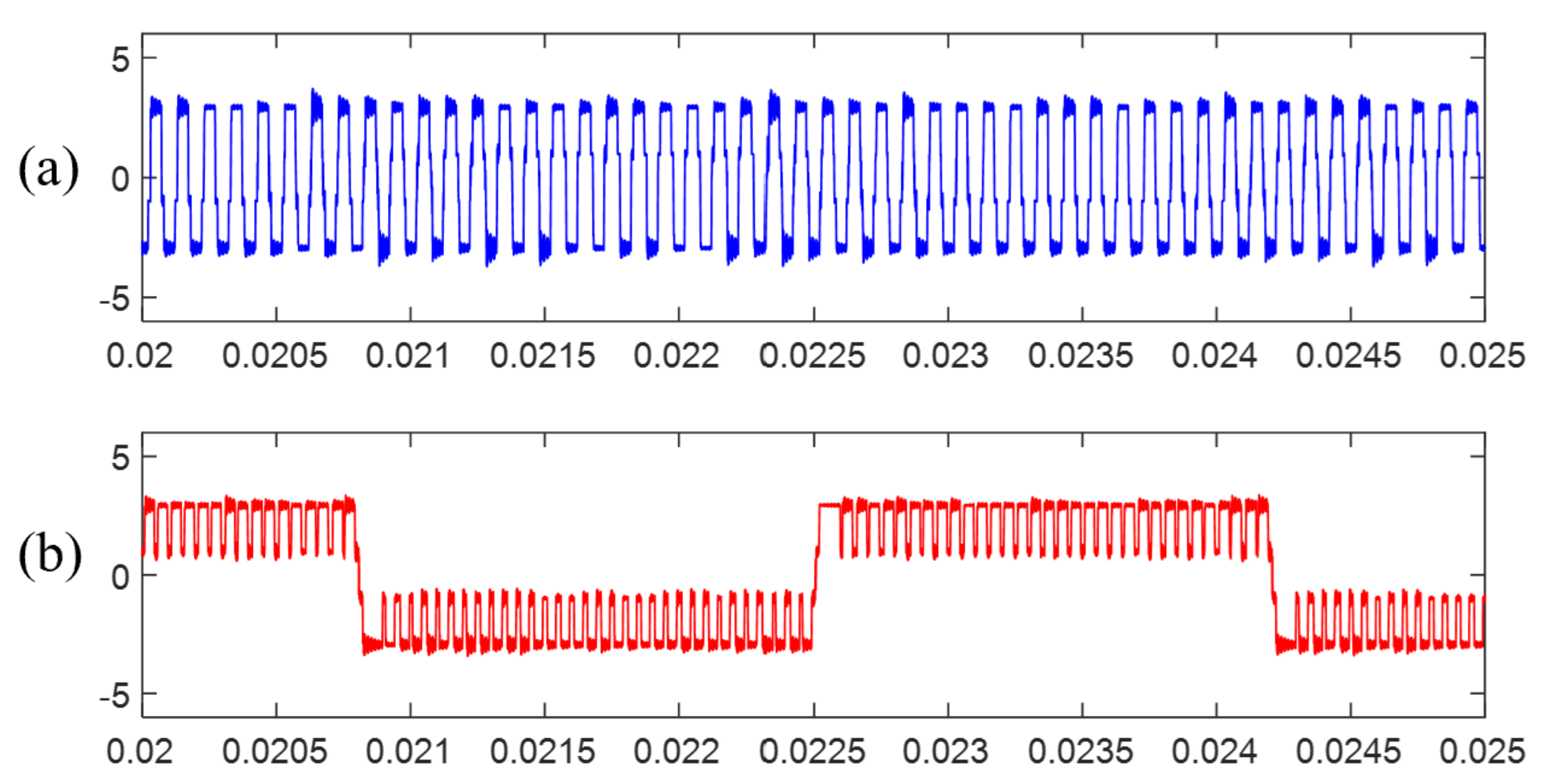
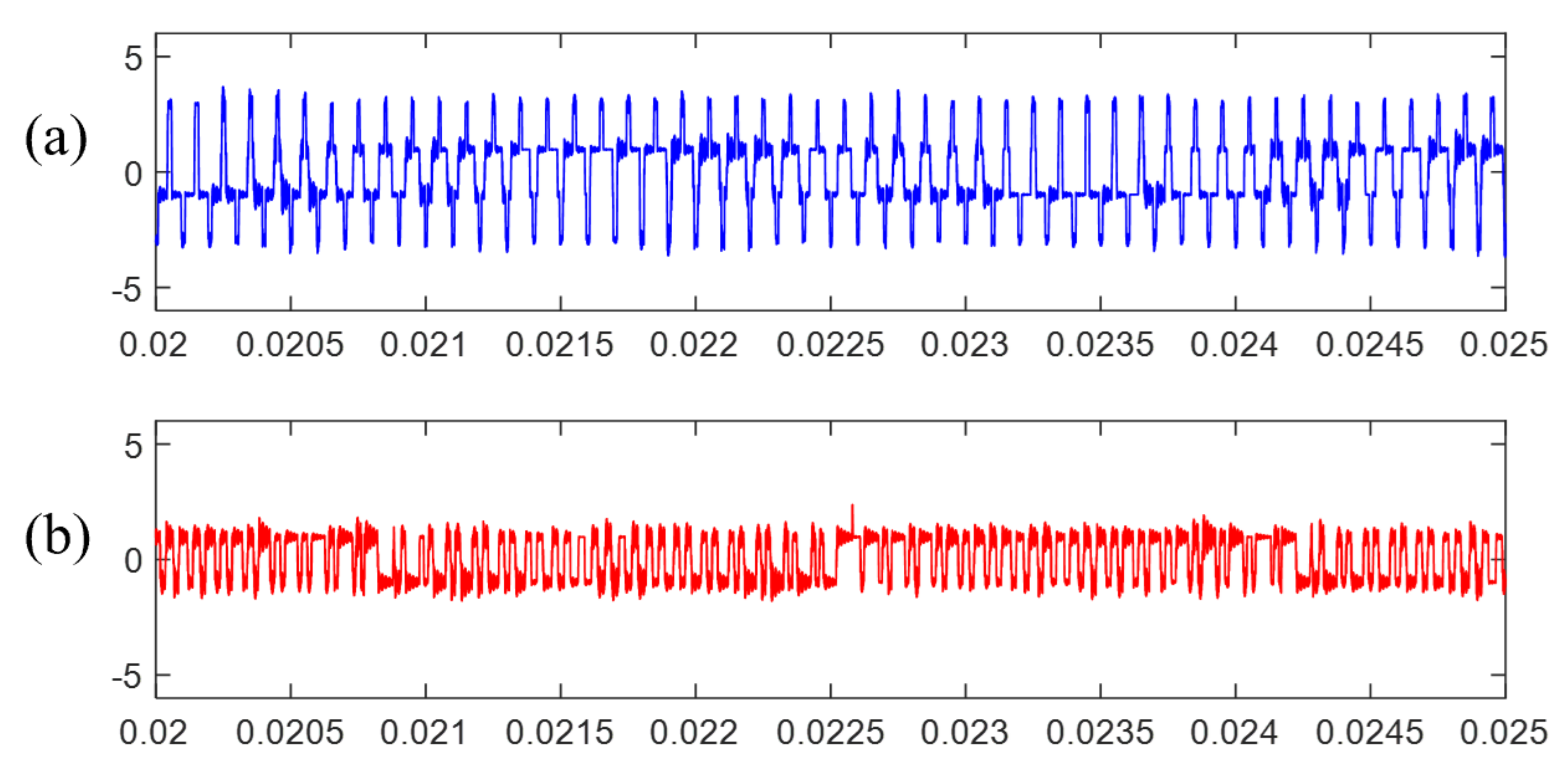
4. Experiment
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- Zhu, Y.; Xiao, M.; Su, X.; Yang, G.; Lu, K.; Wu, Z. Modeling of conduction and switching losses for IGBT and FWD based on SVPWM in automobile electric drives. Appl. Sci. 2020, 10, 4539. [Google Scholar] [CrossRef]
- Kolli, A.; Béthoux, O.; De Bernardinis, A.; Labouré, E.; Coquery, G. Space-vector PWM control synthesis for an H-bridge drive in electric vehicles. IEEE Trans. Veh. Technol. 2013, 62, 2441–2452. [Google Scholar] [CrossRef]
- Jayaraman, K.; Kumar, M. Design of passive common-mode attenuation methods for inverter-fed induction motor drive with reduced common-mode voltage PWM technique. IEEE Trans. Power Electron. 2019, 35, 2861–2870. [Google Scholar] [CrossRef]
- Li, G.; Pommerenke, D.; Min, J. A low frequency electric field probe for near-field measurement in EMC applications. In Proceedings of the 2017 IEEE International Symposium on Electromagnetic Compatibility & Signal/Power Integrity (EMCSI), Washington, DC, USA, 7–11 August 2017; pp. 498–503. [Google Scholar]
- Hu, W.; Ruan, C.; Nian, H.; Sun, D. Zero-sequence current suppression strategy with common-mode voltage control for open-end winding PMSM drives with common DC bus. IEEE Trans. Ind. Electron. 2020, 68, 4691–4702. [Google Scholar] [CrossRef]
- Baik, J.H.; Yun, S.W.; Kim, D.S.; Kwon, C.K.; Yoo, J.Y. EMI noise reduction with new active zero state PWM for integrated dynamic brake systems. J. Power Electron. 2018, 18, 923–930. [Google Scholar]
- Qin, C.; Zhang, C.; Chen, A.; Xing, X.; Zhang, G. A space vector modulation scheme of the quasi-Z-source three-level T-type inverter for common-mode voltage reduction. IEEE Trans. Ind. Electron. 2018, 65, 8340–8350. [Google Scholar] [CrossRef]
- Dabour, S.M.; Abdel-Khalik, A.S.; Massoud, A.M.; Ahmed, S. Analysis of scalar PWM approach with optimal common-mode voltage reduction technique for five-phase inverters. IEEE J. Emerg. Sel. Top. Power Electron. 2018, 7, 1854–1871. [Google Scholar] [CrossRef] [Green Version]
- Hava, A.M.; Ün, E. Performance analysis of reduced common-mode voltage PWM methods and comparison with standard PWM methods for three-phase voltage-source inverters. IEEE Trans. Power Electron. 2009, 24, 241–252. [Google Scholar] [CrossRef]
- Hava, A.M.; Ün, E. A high-performance PWM algorithm for common-mode voltage reduction in three-phase voltage source inverters. IEEE Trans. Power Electron. 2010, 26, 1998–2008. [Google Scholar] [CrossRef]
- Un, E.; Hava, A.M. A near-state PWM method with reduced switching losses and reduced common-mode voltage for three-phase voltage source inverters. IEEE Trans. Ind. Appl. 2009, 45, 782–793. [Google Scholar] [CrossRef]
- Lu, H.; Qu, W.; Cheng, X.; Fan, Y.; Zhang, X. A novel PWM technique with two-phase modulation. IEEE Trans. Power Electron. 2007, 22, 2403–2409. [Google Scholar] [CrossRef]
- Lu, H.; Cheng, X.; Qu, W.; Sheng, S.; Li, Y.; Wang, Z. A three-phase current reconstruction technique using single DC current sensor based on TSPWM. IEEE Trans. Power Electron. 2013, 29, 1542–1550. [Google Scholar]
- Xu, J.; Han, J.; Wang, Y.; Ali, M.; Tang, H. High-frequency SiC three-phase VSIs with common-mode voltage reduction and improved performance using novel tri-state PWM method. IEEE Trans. Power Electron. 2018, 34, 1809–1822. [Google Scholar] [CrossRef]




| Parameters | Value | Parameters | Value |
|---|---|---|---|
| DC link voltage | 360 V | Csf | 11 nf |
| Switching frequency | 10 kHz | Csr | 33 pf |
| Machine speed | 6000 rpm | Crf | 1.65 nf |
| Phase current | 200 A | Cb | 300 pf |
| Simulation step | 1 × 10−6 s | Rb | 3.2 Ω |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jiang, S.; Wang, Y. Optimization of TSPWM for Common-Mode Voltage Reduction in Vehicular Electric Drive System. World Electr. Veh. J. 2022, 13, 5. https://doi.org/10.3390/wevj13010005
Jiang S, Wang Y. Optimization of TSPWM for Common-Mode Voltage Reduction in Vehicular Electric Drive System. World Electric Vehicle Journal. 2022; 13(1):5. https://doi.org/10.3390/wevj13010005
Chicago/Turabian StyleJiang, Shang, and Yuan Wang. 2022. "Optimization of TSPWM for Common-Mode Voltage Reduction in Vehicular Electric Drive System" World Electric Vehicle Journal 13, no. 1: 5. https://doi.org/10.3390/wevj13010005
APA StyleJiang, S., & Wang, Y. (2022). Optimization of TSPWM for Common-Mode Voltage Reduction in Vehicular Electric Drive System. World Electric Vehicle Journal, 13(1), 5. https://doi.org/10.3390/wevj13010005





