Numerical Analysis of Meshing of Loaded Misaligned Straight Bevel Gear Drives of Automobile Differential
Abstract
:1. Introduction
2. Computerized Design of Gear Model
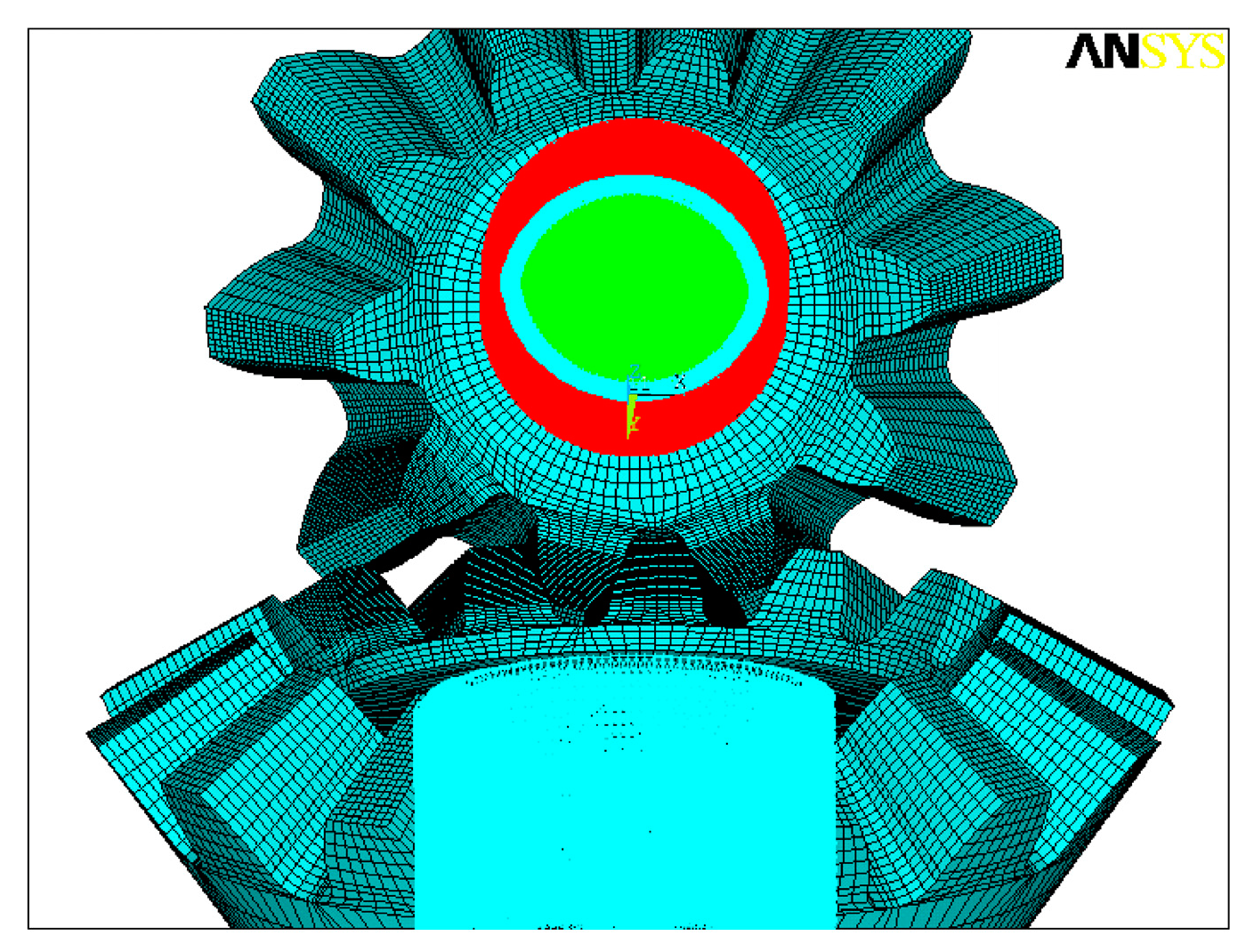
3. Establishment of Finite Element Models
- (i)
- Nodes on the bottom rim of the driven gear are fixed.
- (ii)
- For the nodes on the bottom rim of the driving pinion, the radial and axial degrees of freedom are considered as fixed, and only the rational degree of freedom is set as free and coupled.
- (iii)
- The effect of force F at every node on the bottom rim of the driving pinion in the rotational direction on the pinion is equal to that of working torque T on the pinion. The force F is defined as the following:
4. Determination of the Meshing Interval for Investigation
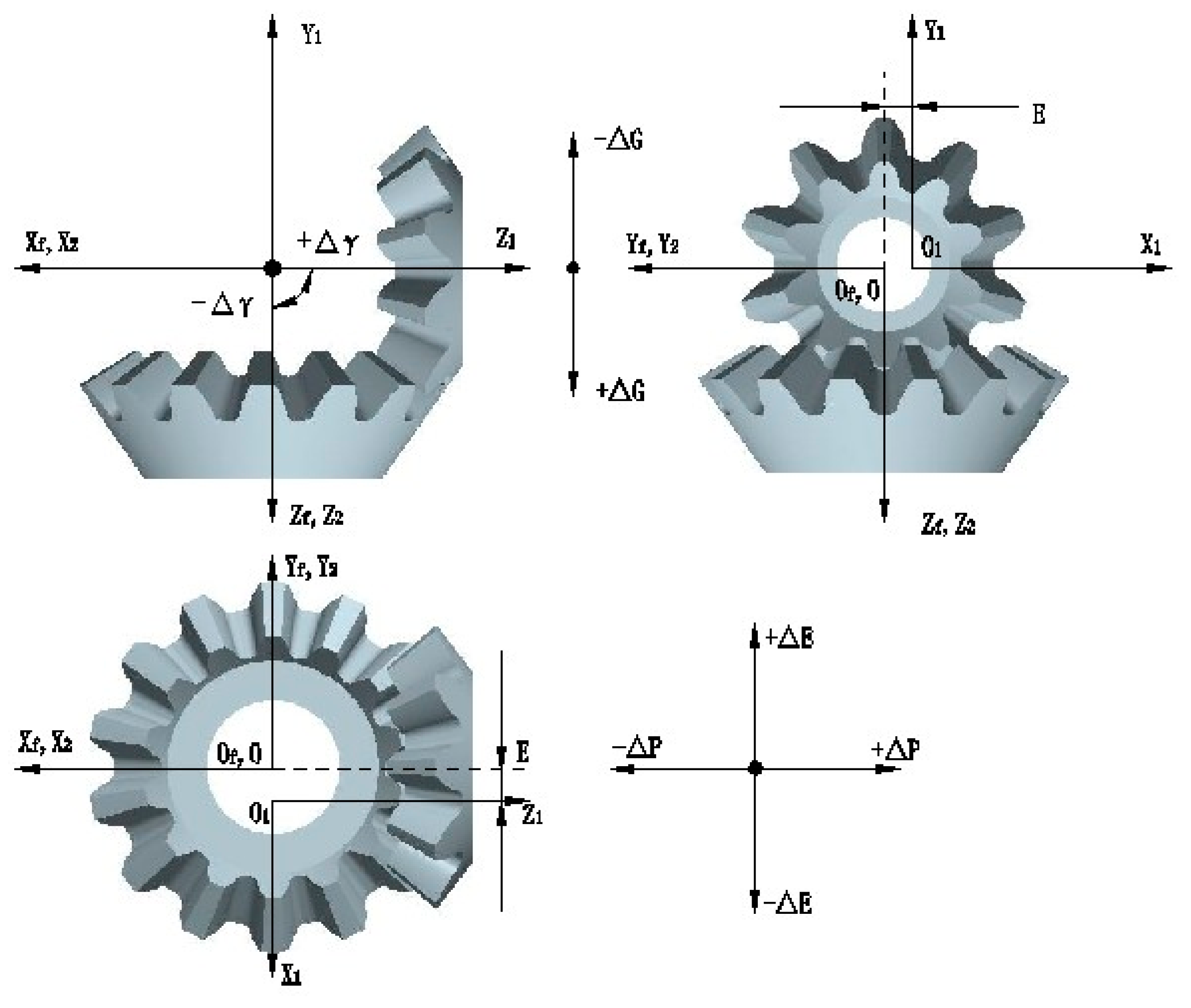
5. Selection of Alignment Errors
- (i)
- Δγ is defined as the change of crossing angle.
- (ii)
- ΔP is defined as the axial displacement of the pinion.
- (iii)
- ΔG is defined as the axial displacement of the gear.
- (iv)
- ΔE is defined as the change center distance of the assembling straight bevel gears.
6. Results and Discussion
6.1. Influence of Single Alignment Error on the Gear Mesh
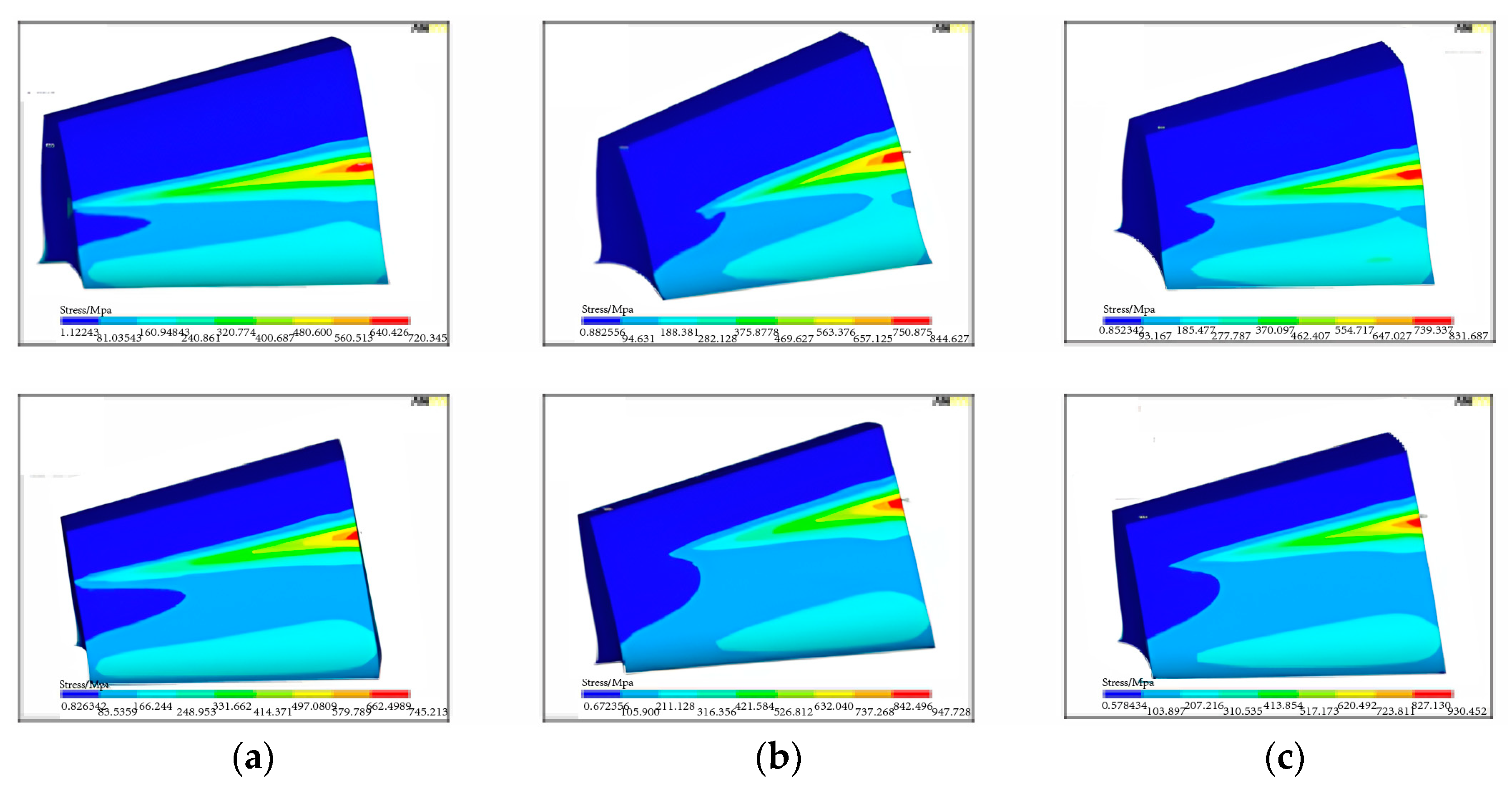
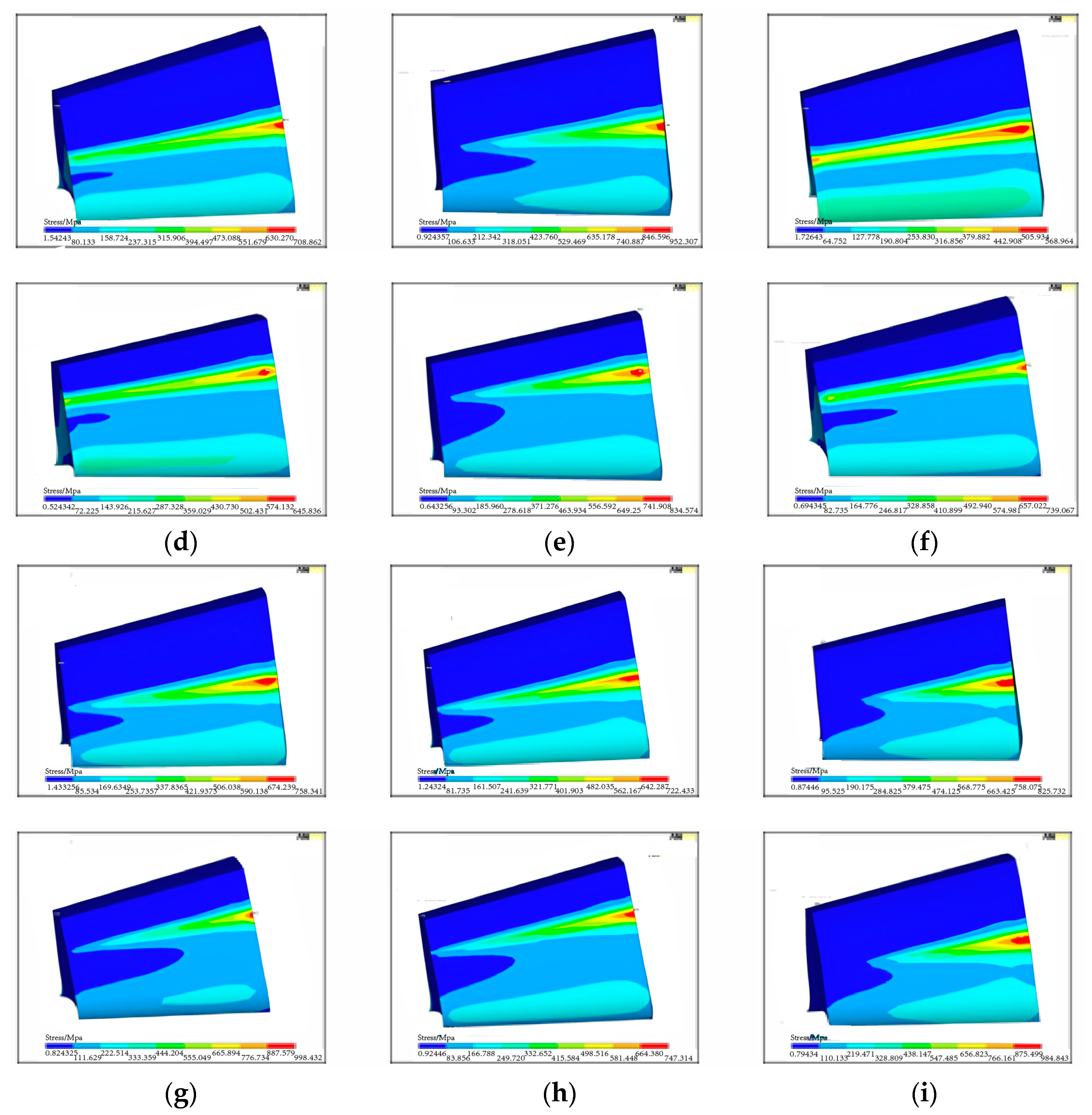
6.1.1. Influence of Single Alignment Error on the Contact Area
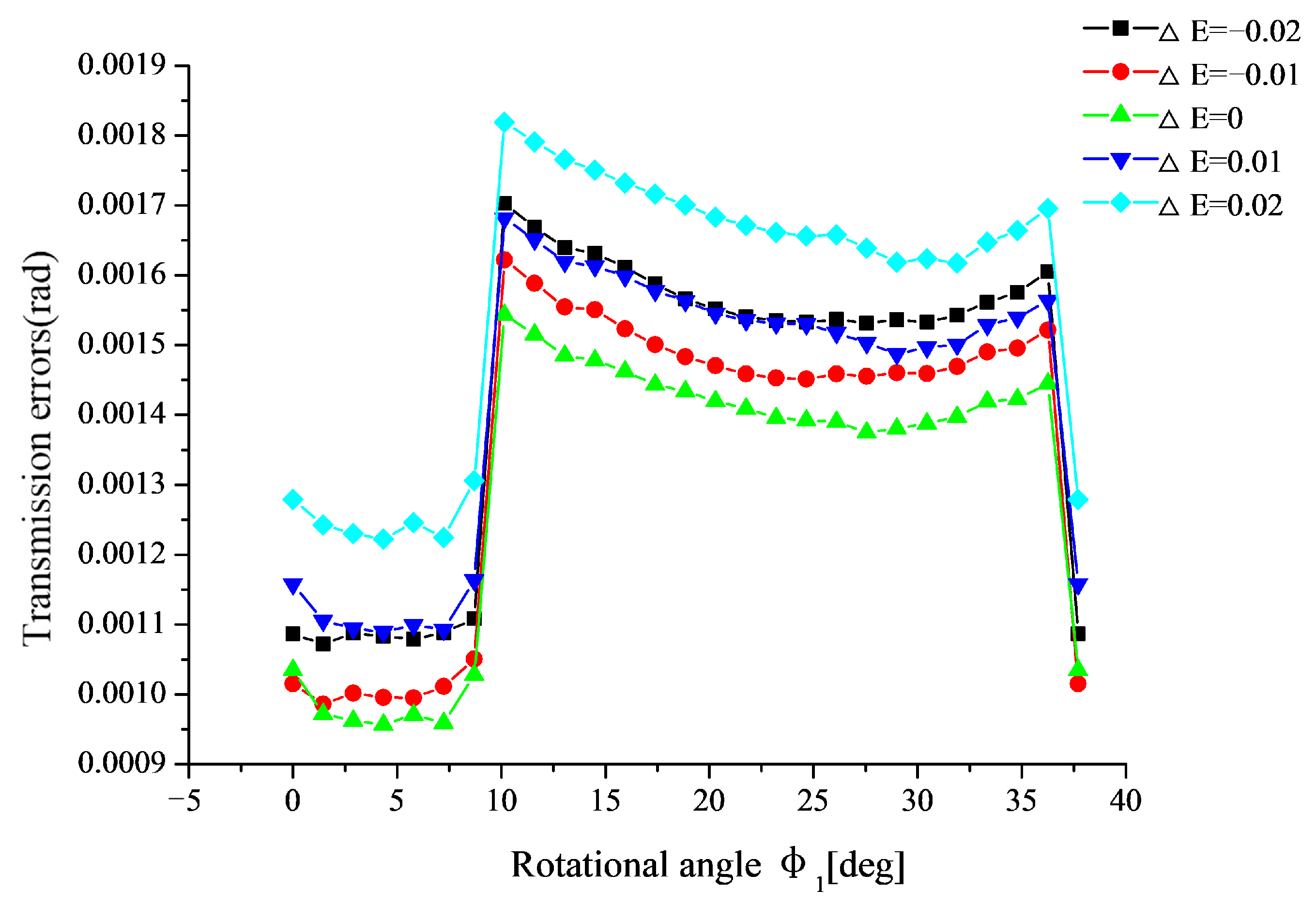
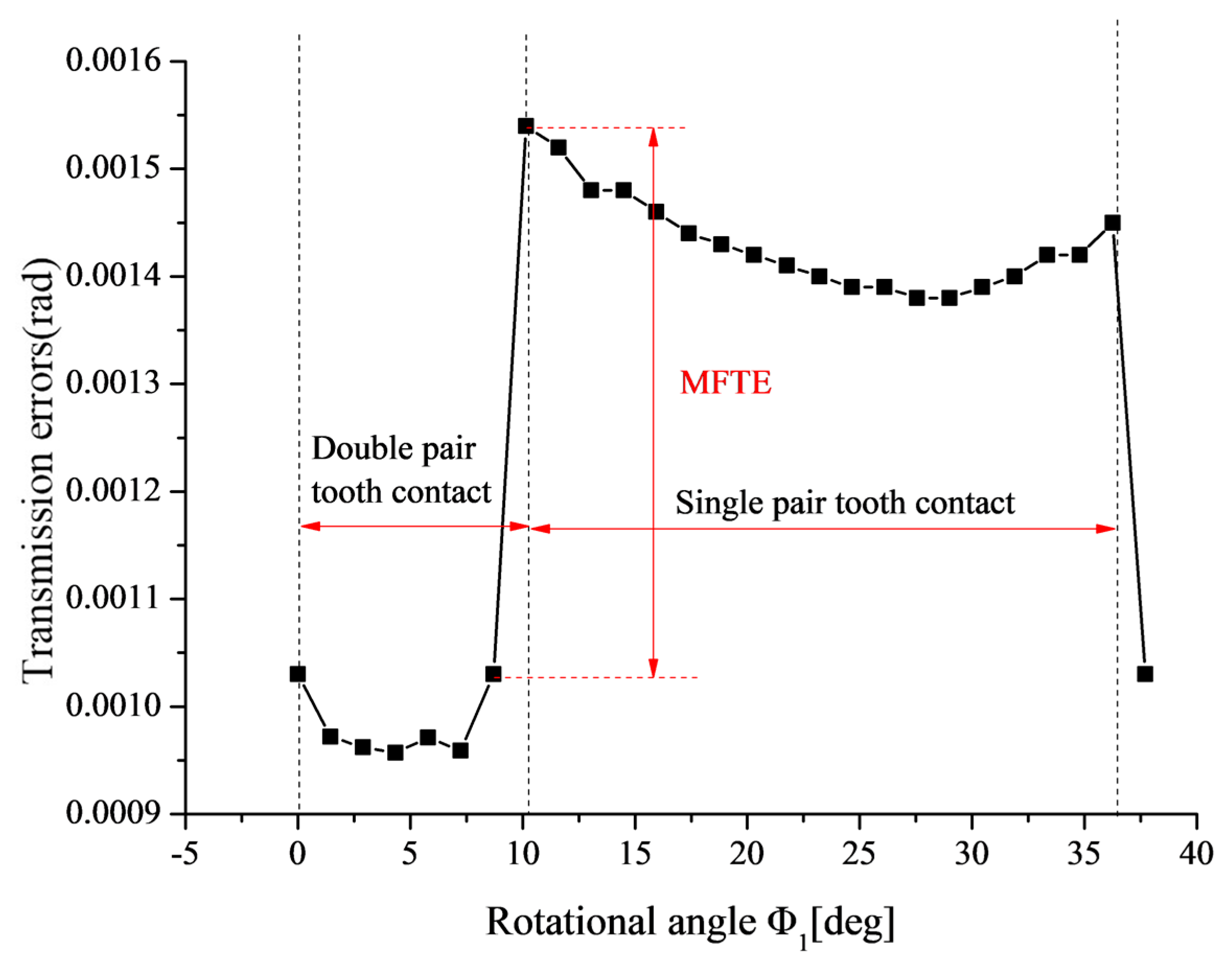
6.1.2. Influence of the Single Alignment Error on the Transmission Error
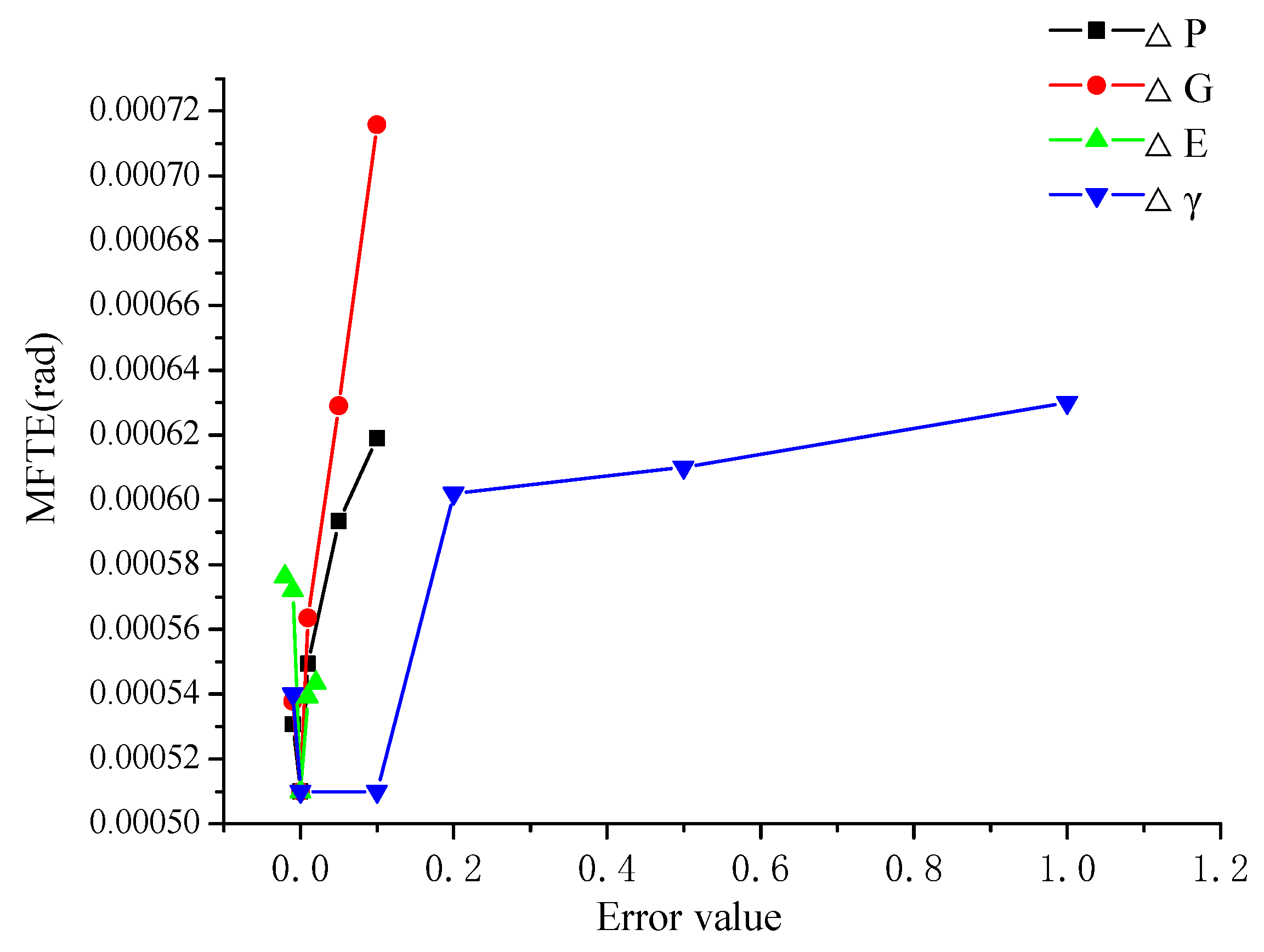
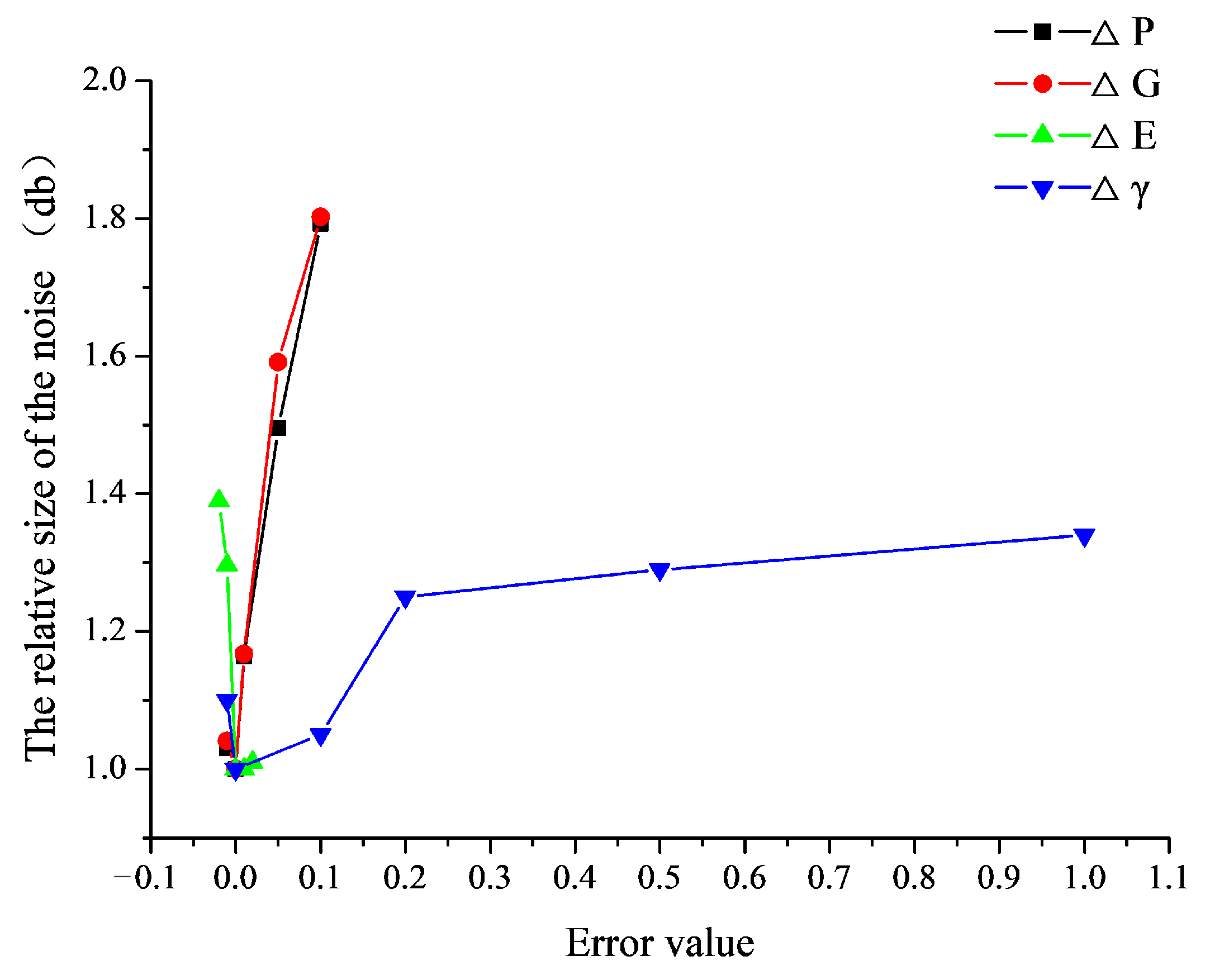
6.1.3. Influence of Single Alignment Error on the Vibration and Noise of Gear Drives
6.2. Influence of Combined Alignment Errors on the Gear Mesh
6.2.1. Influence of Combined Alignment Errors on the Contact Zone
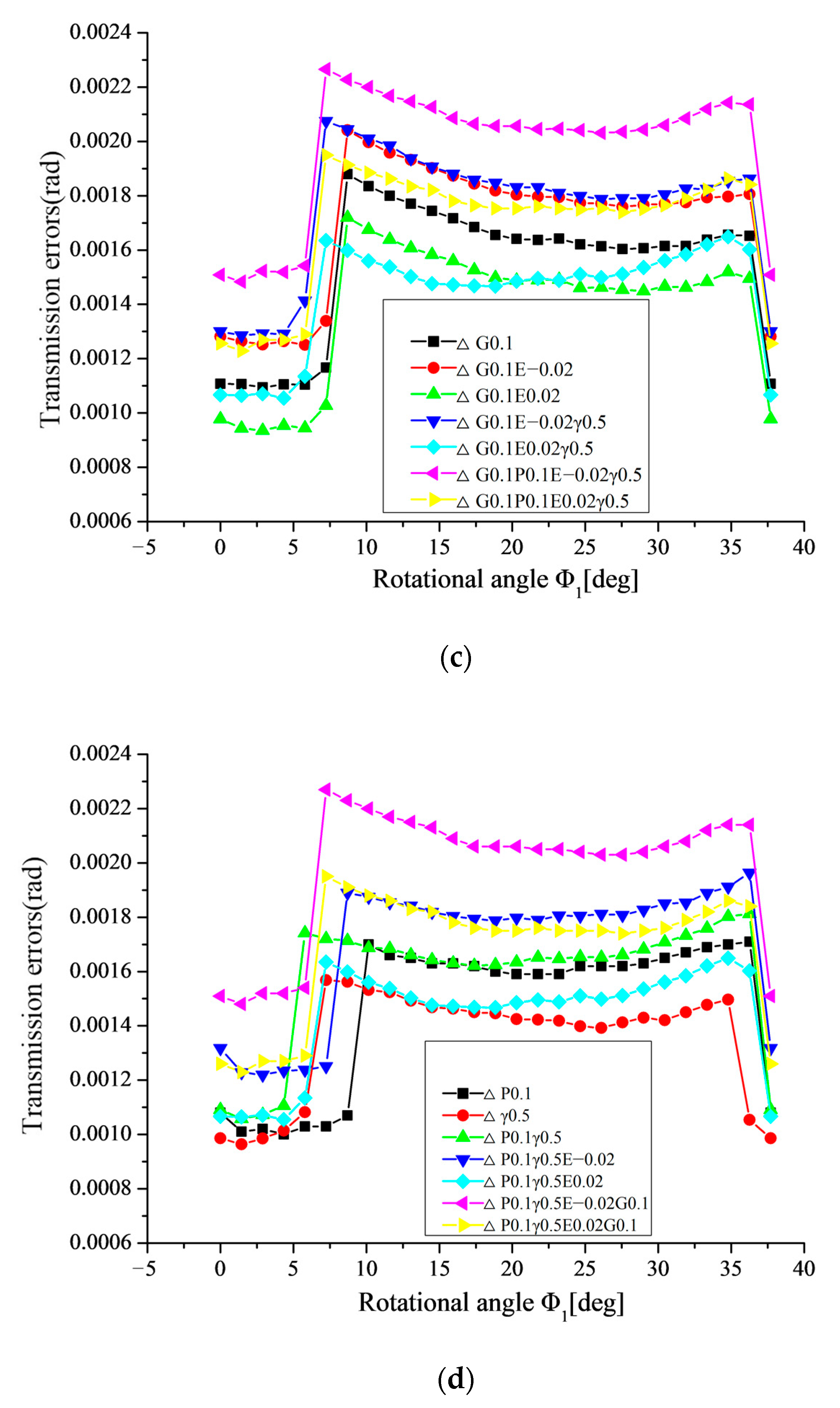
6.2.2. Influence of Combined Alignment Errors on the Transmission Error
- (1)
- As shown in Figure 20a, it can be seen that, when ΔP and ΔG combine, the transmission error increases greatly in the whole meshing circle, compared with that in the single ΔP or ΔG.
- (2)
- As shown in Figure 20b, it can be seen that, when ΔP and ΔE (ΔE < 0) combine, the transmission error increases greatly in the whole meshing circle compared with that in the single ΔP. However, when ΔP and ΔE (ΔE > 0) combine, the transmission error decreases dramatically in the single pair tooth contact zone, compared with that in the single ΔP. However, the transmission error in the double pair tooth contact zone is basically in accordance with the single ΔP.
- (3)
- As shown in Figure 20c, it can be obtained that, when ΔG and ΔE < 0 combine transmission error increases greatly in the whole meshing circle compared with that in single ΔG. However, when ΔG and ΔE > 0 combine, the transmission error decreases dramatically in the whole meshing circle compared with that in single ΔG.
- (4)
- As shown in Figure 20d,e,f, it can be seen that, when Δγ and one of ΔP, ΔG, ΔE combines, the transmission errors are basically consistent compared with that in single ΔP, ΔG or ΔE. Therefore, it can be concluded that the transmission error is not sensitive to Δγ.
- (5)
- It can be seen from Figure 20 that it is the most dangerous type of alignment errors for increasing the transmission error dramatically, when ΔP, ΔG, ΔE (ΔE < 0) and Δγ.
- (6)
- The alignment errors have a cumulative effect on the length of contact line and the contact zone causing the change of gear stiffness. The reduction of the length of contact line will cause the decrease of gear stiffness. The transmission errors increase with the decreasing of gear stiffness.
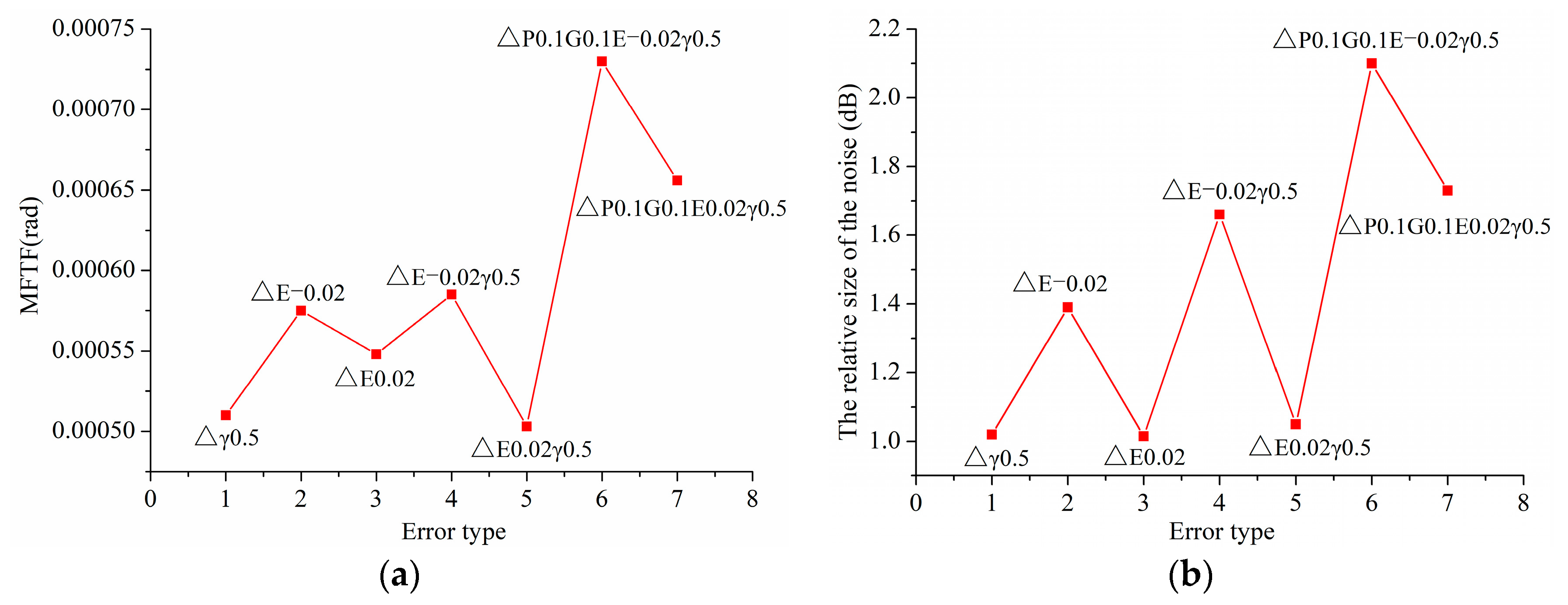
6.2.3. Influence of Combined Alignment Errors on the Vibration and Noise of Gear Drives
7. Conclusions
- (1)
- The alignment errors will cause the straight bevel gear pair contacting at one end and gaping at the other end of the teeth and make the contact zone and the contact line of the straight bevel gear pair change greatly. Furthermore, this change has a direct effect on the transmission error, vibration, and noise of the gear drives.
- (2)
- The contact zone and the contact line of the straight bevel gear pair are sensitive to ΔP, ΔG, and ΔE, resulting in the uneven load distribution and stress concentration on the tooth pair. However, the contact zone and the contact line of the straight bevel gear pair are not sensitive to the alignment error Δγ.
- (3)
- The single alignment error ΔP, ΔG or ΔE has great influence on the transmission error, vibration, and noise of the straight bevel gear drives, and the single alignment error Δγ has great influence on the vibration and noise of the straight bevel gear drives, but it does not have a great effect on the transmission error of the straight bevel gear drives.
- (4)
- Since the combined alignment errors have great influence on the meshing performance of the straight bevel gear, it is important to control the combined alignment errors to ensure the straight bevel gear drives with low vibration and noise. In the practical assembly of the straight bevel gear pair, it is feasible to improve the meshing performance of the straight bevel gear through a better combination of the alignment errors such as the combination of ΔP and ΔE (ΔE > 0) or the combination of ΔG and ΔE (ΔE > 0).
- (5)
- The most dangerous type of alignment error is ΔP, ΔG, ΔE (ΔE < 0) and Δγ. Under this type of alignment error, the length of contact line decreases along longitudinal direction dramatically, leading to a great increase in transmission error, vibration, and noise of the straight bevel gear drives.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Lin, C.F.; Chuang, Y. Energy management strategy and control laws of an inverse differential gear hybrid vehicle. World Electr. Veh. J. 2011, 4, 98–103. [Google Scholar] [CrossRef] [Green Version]
- Tian, H.; Zhu, W.G.; Wang, S.R. Adaptive electronic differential control of vehicle by torque balance. Mob. Netw. Appl. 2020, 25, 1604–1610. [Google Scholar] [CrossRef] [Green Version]
- Litvin, F.L.; Fuentes, A.; Fan, Q.; Handschuh, R.F. Computerized design, simulation of meshing, and contact and stress analysis of face-milled formate generated spiral bevel gears. Mech. Mach. Theory 2002, 37, 441–459. [Google Scholar] [CrossRef]
- Simon, V. Computer simulation of tooth contact analysis of mismatched spiral bevel gears. Mech. Mach. Theory 2007, 42, 365–381. [Google Scholar] [CrossRef]
- Simon, V. Influence of tooth errors and misalignments on tooth contact in spiral bevel gears. Mech. Mach. Theory 2008, 43, 1253–1267. [Google Scholar] [CrossRef]
- Litvin, F.L.; Wang, A.G.; Handschuh, R.F. Computerized generation and simulation of meshing and contact of spiral bevel gears with improved geometry. Comput. Methods Appl. Mech. Eng. 1998, 158, 35–64. [Google Scholar] [CrossRef]
- Tang, J.; Chen, X.; Luo, C. Contact analysis of spur gears based on longitudinal modification and alignment errors. J. Cent. South Univ. 2012, 43, 1703–1709. [Google Scholar]
- Xu, H.; Gan, Z. Influence of alignment errors on tooth surface contact of straight bevel gears. Sci. Tech. Inf. 2008, 29, 95–97. (In Chinese) [Google Scholar]
- Chen, M.Z.; Xiong, X.S.; Zhuang, W.H. Design and simulation of meshing performance of modified straight bevel gears. Metals 2021, 11, 33. [Google Scholar] [CrossRef]
- Litvin, F.L.; Vecchiato, D.; Fuentes, A.; Yukishima, K.; Gonzalez-Perez, I.; Hayasaka, K. Reduction of noise of loaded and unloaded misaligned gear drives. Comput. Methods Appl. Mech. Eng. 2006, 195, 5523–5536. [Google Scholar] [CrossRef]
- Wang, B.; Hua, L. Computerized design and FE simulation of meshing of involute spiral bevel gears with alignment errors. Adv. Mat. Res. 2011, 200, 386–391. [Google Scholar] [CrossRef]
- Litvin, F.L.; Gonzalez-Perez, I.; Yukishima, K.; Fuentes, A.; Hayasaka, K. Design, simulation of meshing, and contact stress analysis for an improved worm gear drive. Mech. Mach. Theory 2007, 42, 940–959. [Google Scholar] [CrossRef]
- Simon, V.V. Influence of tooth errors and shaft misalignments on loaded tooth contact in cylindrical worm gears. Mech. Mach. Theory 2006, 41, 707–724. [Google Scholar] [CrossRef]
- Wang, J. Effect of fitting error on the performance of bevel gear couple. Constr. Mach Equip. 1994, 2, 29–31. (In Chinese) [Google Scholar]
- Chen, X.; Tang, J. Tooth contact analysis of spur face gear drives with alignment errors. In Proceedings of the 2011 Second International Conference on Digital Manufacturing & Automation, Zhangjiajie, China, 5–7 August 2011; pp. 1368–1371. [Google Scholar]
- Shunmugam, M.S.; Subbarao, B.; Jayaprakash, V. Establishing gear tooth surface geometry and normal deviation. Mech. Mach. Theory 1998, 33, 525–534. [Google Scholar] [CrossRef]
- Shuting, L. Effect of addendum on contact strength bending strength and basic performance parameters of a pair of spur gears. Mech. Mach. Theory 2008, 13, 1543–1556. [Google Scholar]
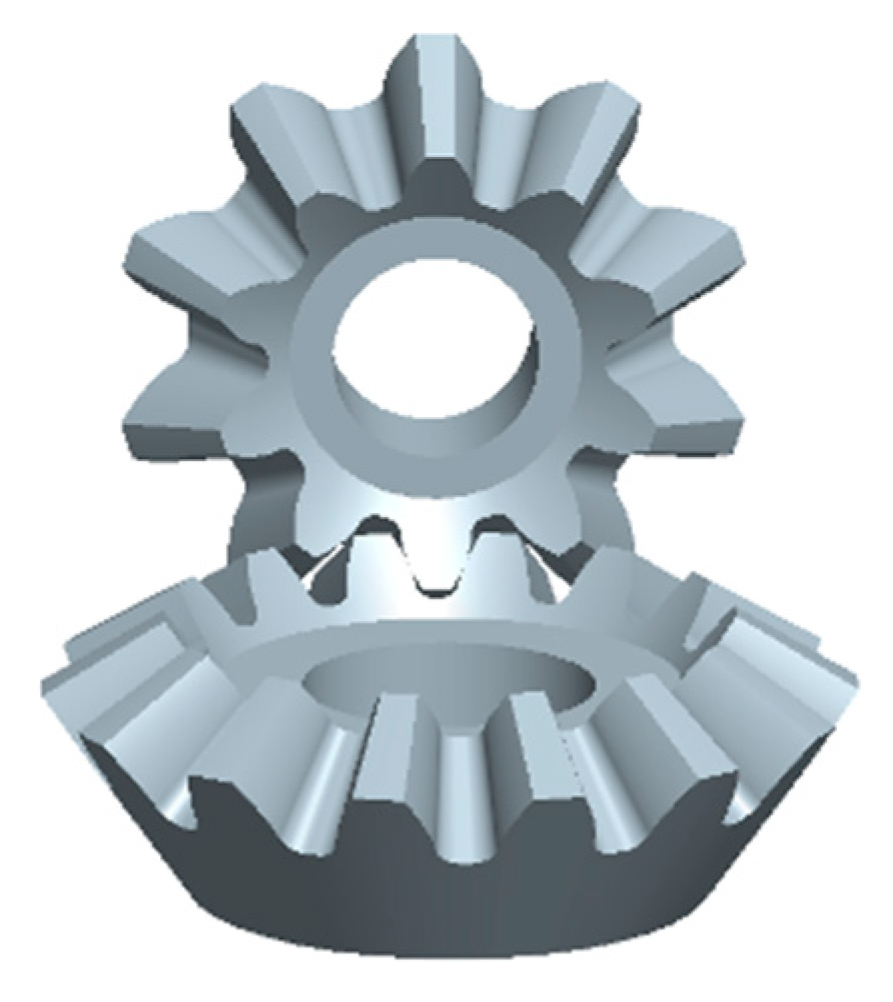


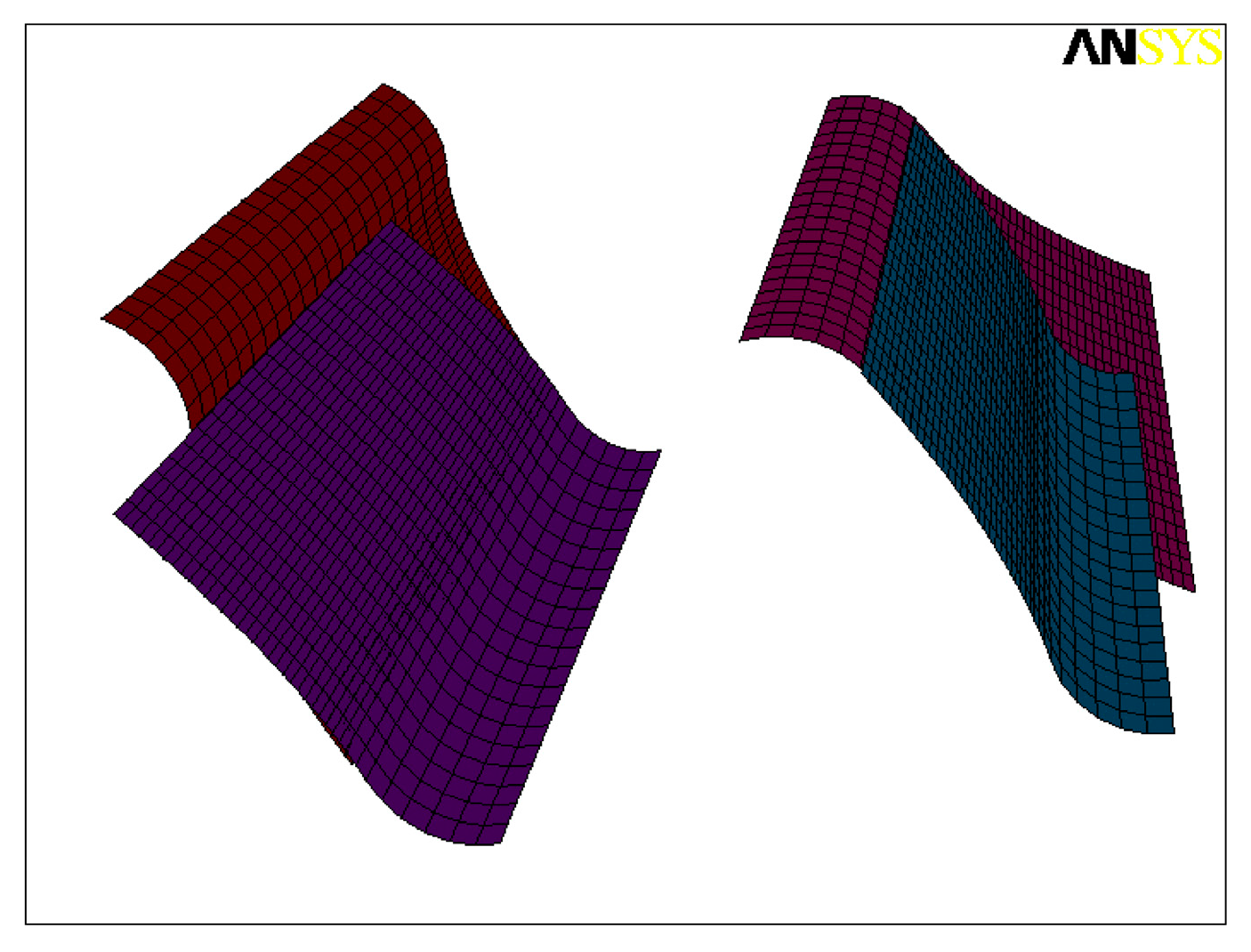












| Parameter | Driving Pinion | Driven Gear |
|---|---|---|
| Module [mm] | 3.7792 | 3.7792 |
| Shaft angle [deg] | 90 | 90 |
| Number of teeth | 10 | 14 |
| Pressure angle [deg] | 22.5 | 22.5 |
| Modification coefficient of height | −0.1812 | −0.1812 |
| Modification coefficient of thickness | 0.05 | −0.05 |
| Addendum coefficient | 0.8 | 0.8 |
| Headspace coefficient | 0.188 | 0.188 |
| Face width [mm] | 9.75 | 9.75 |
| Gear center hole [mm] | 7.0275 | 10 |
| Basic Type of Alignment Error | Δγ (deg) | ΔP (mm) | ΔG (mm) | ΔE (mm) |
|---|---|---|---|---|
| Value | −0.01 | −0.01 | −0.01 | −0.02 |
| 0.1 | 0.01 | 0.01 | −0.01 | |
| 0.2 | 0.05 | 0.05 | 0.01 | |
| 0.5 | 0.1 | 0.1 | 0.02 | |
| 1 |
| Combined Alignment Errors | ΔPΔGΔγ | ΔPΔEΔγ | ΔGΔEΔγ | ΔPΔGΔE | ΔPΔGΔEΔγ | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Value | 0.1 | 0.1 | 0.1 | −0.02 | 0.1 | −0.02 | 0.1 | 0.1 | 0.1 | 0.1 | −0.02 | 0.5 | ||||
| 0.1 | 0.1 | 0.5 | 0.1 | 0.02 | 0.1 | 0.02 | 0.1 | 0.1 | −0.02 | 0.1 | 0.1 | 0.02 | 0.5 | |||
| 0.1 | −0.02 | 0.5 | 0.1 | −0.02 | 0.5 | 0.1 | 0.1 | 0.02 | ||||||||
| 0.1 | 0.02 | 0.5 | 0.1 | 0.02 | 0.5 | |||||||||||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, Q.; Wang, S.; Li, P.; Li, X.; Liu, J.; Hu, D.; Zhao, Z.; Xiong, X. Numerical Analysis of Meshing of Loaded Misaligned Straight Bevel Gear Drives of Automobile Differential. World Electr. Veh. J. 2022, 13, 41. https://doi.org/10.3390/wevj13020041
Chen Q, Wang S, Li P, Li X, Liu J, Hu D, Zhao Z, Xiong X. Numerical Analysis of Meshing of Loaded Misaligned Straight Bevel Gear Drives of Automobile Differential. World Electric Vehicle Journal. 2022; 13(2):41. https://doi.org/10.3390/wevj13020041
Chicago/Turabian StyleChen, Qianjin, Shuiming Wang, Pengfei Li, Xinguang Li, Jianhua Liu, Dewu Hu, Zhigang Zhao, and Xiaoshuang Xiong. 2022. "Numerical Analysis of Meshing of Loaded Misaligned Straight Bevel Gear Drives of Automobile Differential" World Electric Vehicle Journal 13, no. 2: 41. https://doi.org/10.3390/wevj13020041
APA StyleChen, Q., Wang, S., Li, P., Li, X., Liu, J., Hu, D., Zhao, Z., & Xiong, X. (2022). Numerical Analysis of Meshing of Loaded Misaligned Straight Bevel Gear Drives of Automobile Differential. World Electric Vehicle Journal, 13(2), 41. https://doi.org/10.3390/wevj13020041





