Assessment of Exposure to Electric Vehicle Inductive Power Transfer Systems: Experimental Measurements and Numerical Dosimetry
Abstract
:1. Introduction
2. Materials and Methods
2.1. WPT Systems for the Bus: Tested Charging System
2.2. WPT Systems for the Light Vehicle
2.3. Measurements Protocol Adopted at the Bus Station
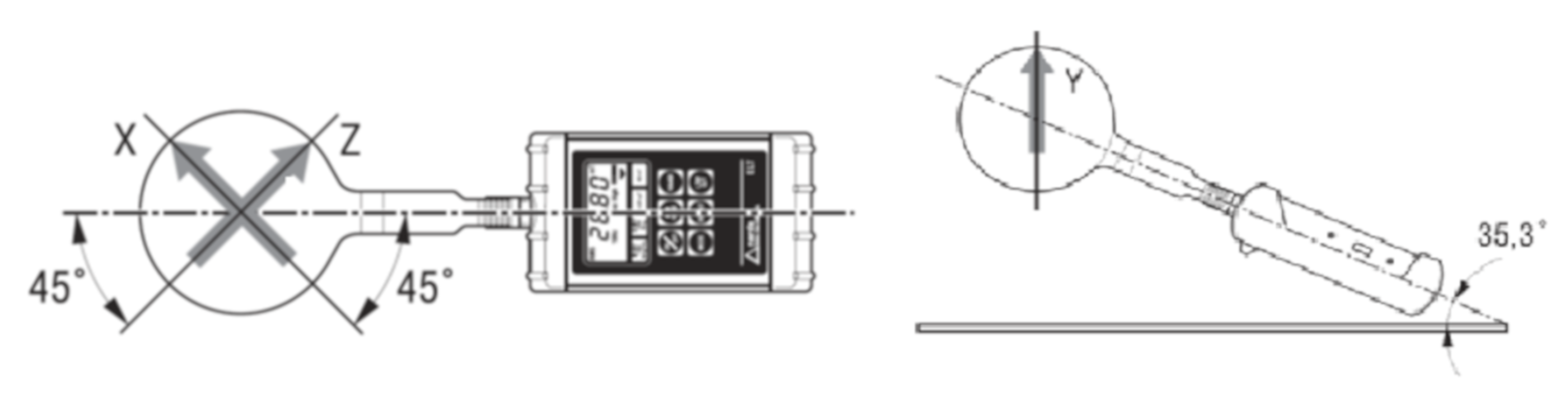
2.3.1. Instrumentation
2.3.2. Positioning System
2.3.3. Measurement Procedure
- Place the probe at one measurement point;
- Adjust the software setting of the measurement time instant for the acquisition and measurement systems, based on the synchronization cards;
- Switch on the charging station power supply. The system starts running in a few seconds;
- Upon the automatic start of the measurement, the measured values were recorded by the acquisition software, including the coil currents and the r.m.s. values of the resulting magnetic induction at the measurement point.
2.3.4. Uncertainty Analysis of On-Site Measurements
- tolerance in dB: the tolerance of each component, expressed in dB in terms of its influence on the quantity of interest;
- probability distribution (distr): the variation in the probability of the true value lying at any particular offset from the measured result. R-(log)rectangular distribution, N-(log)normal distribution;
- divisor (div): either 2 for a normal distribution or (1.7321). A divisor of relates the standard uncertainty of a rectangular distribution to a normal distribution;
- sensitivity coefficient (): the sensitivity coefficient of each uncertainty component that translates the unit of the tolerance to the unit for which the uncertainty is determined. Since all tolerances are already expressed in dB relative to the quantity of interest in this work, the sensitivity coefficient is unity for all contributing components and set to zero for irrelevant components;
- standard uncertainty in dB (std unc): the contribution of the uncertainty component to the combined standard uncertainty.
- ±0.55% at instrument indications of 0.8 µT and above;
- ±1.0% at instrument indications in the range of 0.2–0.8 µT;
- ±5.0% at instrument indications below 0.2 µT.
2.4. Numerical Simulations
2.4.1. Numerical Models of the IPT Systems
2.4.2. Procedure for the Validation of the Numerical Model of the IPT Systems through On-Site Measurements
2.4.3. Simulation Protocol for Numerical Dosimetry
2.4.4. Analyzed Metrics
3. Results
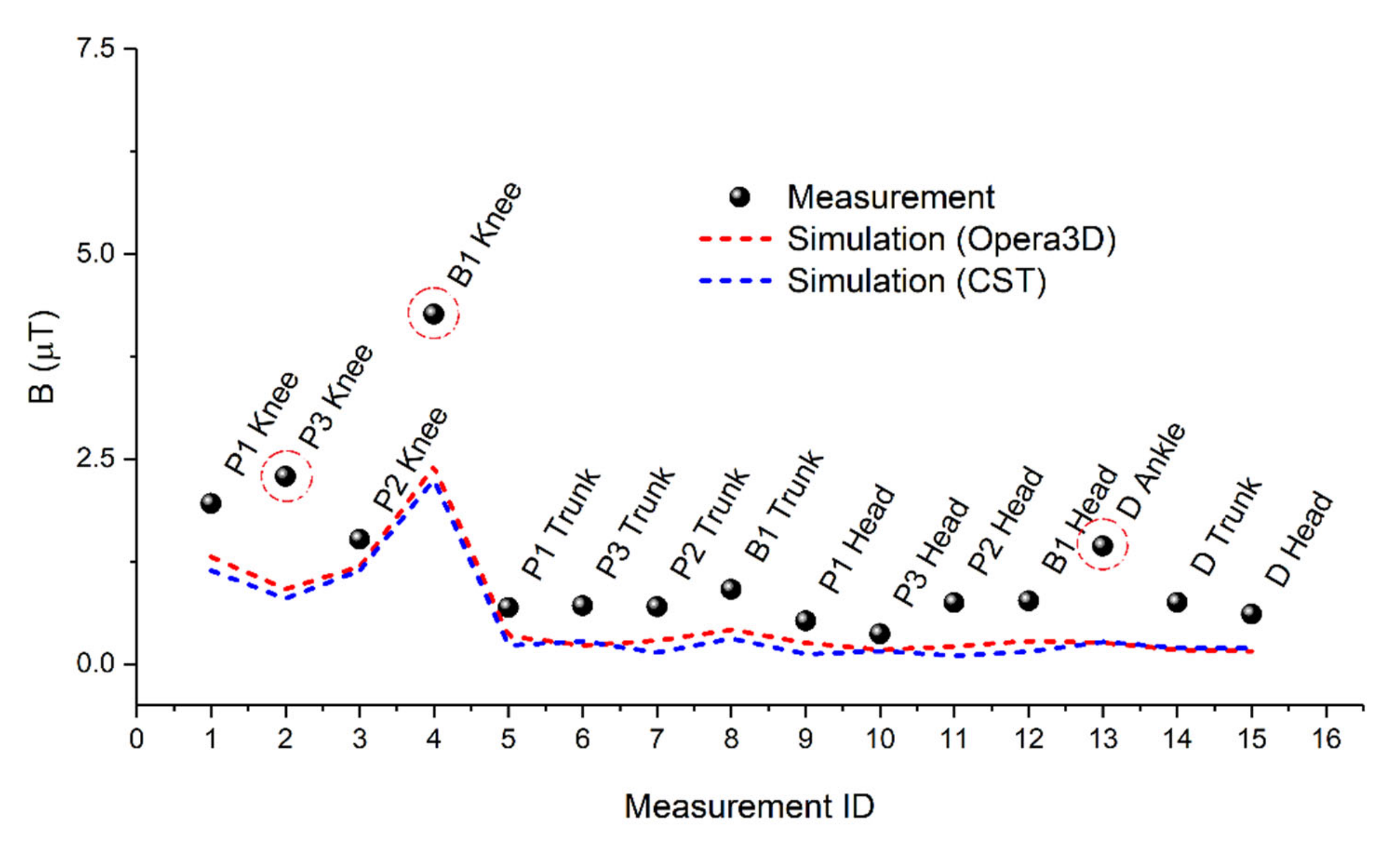
3.1. Comparison between Experiments and Simulations
3.2. Numerical Dosimetry of Human Exposure
3.2.1. Exposure of the Light EV (The Car)
3.2.2. Exposure of the Bus
4. Discussion
4.1. Magnetic Field Distribution
4.2. Dosimetric Results
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Tesla, N. Apparatus for Transmitting Electrical Energy. U.S. Patent 1,119,732, 1 December 1914. [Google Scholar]
- Kurs, A.; Karalis, A.; Moffatt, R.; Joannopoulos, J.D.; Fisher, P.; Soljačić, M. Wireless Power Transfer via Strongly Coupled Magnetic Resonances. Science 2007, 317, 83–86. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Villa, J.L.; Sallán, J.; Llombart, A.; Sanz, J.F. Design of a High Frequency Inductively Coupled Power Transfer System for Electric Vehicle Battery Charge. Appl. Energy 2009, 86, 355–363. [Google Scholar] [CrossRef]
- El-Shahat, A.; Ayisire, E.; Wu, Y.; Rahman, M.; Nelms, D. Electric Vehicles Wireless Power Transfer State-of-The-Art. Energy Procedia 2019, 162, 24–37. [Google Scholar] [CrossRef]
- Beh, T.C.; Imura, T.; Kato, M.; Hori, Y. Basic Study of Improving Efficiency of Wireless Power Transfer via Magnetic Resonance Coupling Based on Impedance Matching. In Proceedings of the 2010 IEEE International Symposium on Industrial Electronics, Bari, Italy, 4–7 July 2010; pp. 2011–2016. [Google Scholar]
- Jang, Y.J. Survey of the Operation and System Study on Wireless Charging Electric Vehicle Systems. Transp. Res. Part C Emerg. Technol. 2018, 95, 844–866. [Google Scholar] [CrossRef]
- Christ, A.; Douglas, M.; Nadakuduti, J.; Kuster, N. Assessing Human Exposure to Electromagnetic Fields from Wireless Power Transmission Systems. Proc. IEEE 2013, 101, 1482–1493. [Google Scholar] [CrossRef]
- Schneider, J. Wireless Power Transfer for Light-Duty Plug-in/Electric Vehicles and Alignment Methodology; SAE Int. J2954 Taskforce; SAE Interanational: Warrendale, PA, USA, 2016. [Google Scholar]
- ICNIRP Guidelines for Limiting Exposure to Time-Varying Electric, Magnetic, and Electromagnetic Fields (up to 300 GHz). Heal. Phys 1998, 74, 494–522.
- ICNIRP Guidelines for Limiting Exposure to Time-Varying Electric and Magnetic Fields (1 Hz to 100 kHz). Health Phys. 2010, 99, 818–836.
- IEEE Standards Coordinating Committee. 28. IEEE Standard for Safety Levels with Respect to Human Exposure to Radio Frequency Electromagnetic Fields, 3kHz to 300GHz; IEEE C95. 1-1991; IEEE Standards Coordinating Committee: Piscataway, NJ, USA, 1992. [Google Scholar]
- Liorni, I.; Lisewski, T.; Capstick, M.H.; Kuehn, S.; Neufeld, E.; Kuster, N. Novel Method and Procedure for Evaluating Compliance of Sources with Strong Gradient Magnetic Fields Such as Wireless Power Transfer Systems. IEEE Trans. Electromagn. Compat. 2019. (Early Access). [Google Scholar] [CrossRef]
- Park, S. Evaluation of Electromagnetic Exposure During 85 kHz Wireless Power Transfer for Electric Vehicles. IEEE Trans. Magn. 2018, 54, 5100208. [Google Scholar] [CrossRef]
- Laakso, I.; Hirata, A. Evaluation of the Induced Electric Field and Compliance Procedure for a Wireless Power Transfer System in an Electrical Vehicle. Phys. Med. Biol. 2013, 58, 7583. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Shimamoto, T.; Laakso, I.; Hirata, A. In-Situ Electric Field in Human Body Model in Different Postures for Wireless Power Transfer System in an Electrical Vehicle. Phys. Med. Biol. 2014, 60, 163. [Google Scholar] [CrossRef] [PubMed]
- De Santis, V.; Campi, T.; Cruciani, S.; Laakso, I.; Feliziani, M. Assessment of the Induced Electric Fields in a Carbon-Fiber Electrical Vehicle Equipped with a Wireless Power Transfer System. Energies 2018, 11, 684. [Google Scholar] [CrossRef] [Green Version]
- Miwa, K.; Takenaka, T.; Hirata, A. Electromagnetic Dosimetry and Compliance for Wireless Power Transfer Systems in Vehicles. IEEE Trans. Electromagn. Compat. 2019, 61, 2024–2030. [Google Scholar] [CrossRef]
- Wang, Q.; Li, W.; Kang, J.; Wang, Y. Electromagnetic Safety Evaluation and Protection Methods for a Wireless Charging System in an Electric Vehicle. IEEE Trans. Electromagn. Compat. 2019, 61, 1913–1925. [Google Scholar] [CrossRef]
- Wen, F.; Huang, X. Human Exposure to Electromagnetic Fields from Parallel Wireless Power Transfer Systems. Int. J. Environ. Res. Public Health 2017, 14, 157. [Google Scholar] [CrossRef]
- Chakarothai, J.; Wake, K.; Arima, T.; Watanabe, S.; Uno, T. Exposure Evaluation of an Actual Wireless Power Transfer System for an Electric Vehicle With Near-Field Measurement. IEEE Trans. Microw. Theory Tech. 2018, 66, 1543–1552. [Google Scholar] [CrossRef]
- Cirimele, V.; Freschi, F.; Giaccone, L.; Pichon, L.; Repetto, M. Human Exposure Assessment in Dynamic Inductive Power Transfer for Automotive Applications. IEEE Trans. Magn. 2017, 53, 1–4. [Google Scholar] [CrossRef]
- Zucca, M.; Bottauscio, O.; Harmon, S.; Guilizzoni, R.; Schilling, F.; Schmidt, M.; Ankarson, P.; Bergsten, T.; Tammi, K.; Sainio, P. Metrology for Inductive Charging of Electric Vehicles (MICEV). In Proceedings of the International Conference of Electrical and Electronic Technologies for Automotive 2019 (Aeit Automotive), Torino, Italy, 2–4 July 2019; pp. 1–4. [Google Scholar]
- Christ, A.; Kainz, W.; Hahn, E.G.; Honegger, K.; Zefferer, M.; Neufeld, E.; Rascher, W.; Janka, R.; Bautz, W.; Chen, J. The Virtual Family—Development of Surface-Based Anatomical Models of Two Adults and Two Children for Dosimetric Simulations. Phys. Med. Biol. 2009, 55, N23. [Google Scholar] [CrossRef]
- Gosselin, M.-C.; Neufeld, E.; Moser, H.; Huber, E.; Farcito, S.; Gerber, L.; Jedensjö, M.; Hilber, I.; Di Gennaro, F.; Lloyd, B. Development of a New Generation of High-Resolution Anatomical Models for Medical Device Evaluation: The Virtual Population 3.0. Phys. Med. Biol. 2014, 59, 5287. [Google Scholar] [CrossRef] [PubMed]
- EN Standard 62233:2008, Measurement Methods for Electromagnetic Fields of Household Appliances and Similar Apparatus with Regard to Human Exposure. Available online: https://webstore.iec.ch/publication/6618 (accessed on 3 June 2020).
- Bottauscio, O.; Chiampi, M.; Crotti, G.; Zucca, M. Probe influence on the measurement accuracy of non-uniform LF magnetic fields. IEEE Trans. Instrum. Meas. 2005, 54, 722–726. [Google Scholar] [CrossRef]
- Chiampi, G.; Crotti, D. Giordano. Set up and characterization of a system for the generation of reference magnetic fields, from 1 to 100 kHz. IEEE Trans. Instrum. Meas. 2007, 564, 300–304. [Google Scholar] [CrossRef]
- Zucca, M.; Squillari, P.; Pogliano, U. A Measurement System for the Characterization of Wireless Charging Stations for Electric Vehicles, accepted for presentation to CPEM Conference; Denver, CO, USA. 2020. [Google Scholar]
- Li, F.; Ferguson, R.; Hart, D.; Guilizzoni, R.; Fatadin, I.; Harmon, S. Characterisation of an optical 2D tracking system. In Proceedings of the of IEEE British and Irish Conference on Optics and Photonics, London, UK, 11–13 December 2019. [Google Scholar]
- Taylor, B.N.; Kuyatt, C.E. Guidelines for Evaluating and Expressing the Uncertainty of NIST Measurement Results; Physics Laboratory National Institute of Standards and Technology Gaithersburg: Gaithersburg, MD, USA, 1994. [Google Scholar]
- JCGM. JCGM 100-2008: Evaluation of Measurement Data–Guide to the Expression of Uncertainty in Measurements; Bureau International des Pois et Mesures (BIPM): Sevres, France, 2008. [Google Scholar]
- Simulia, OPERA. 2020. Available online: https://www.3ds.com/products-services/simulia/products/opera/solutions/ (accessed on 14 April 2020).
- Simulia, CST. Available online: https://www.3ds.com/products-services/simulia/products/cst-studio-suite/electromagnetic-systems/ (accessed on 14 April 2020).
- Hasgall, P.A.; Neufeld, E.; Gosselin, M.C.; Klingenböck, A.; Kuster, N.; Hasgall, P.; Gosselin, M. IT’IS Database for Thermal and Electromagnetic Parameters of Biological Tissues. 2012. Available online: https://www.scienceopen.com/document?vid=a95fbaa4-efd8-429a-a59e-5e208fea2e45 (accessed on 3 June 2020).
- Kuster, N.; Torres, V.B.; Nikoloski, N.; Frauscher, M.; Kainz, W. Methodology of Detailed Dosimetry and Treatment of Uncertainty and Variations for in Vivo Studies. Bioelectromagnetics 2006, 27, 378–391. [Google Scholar] [CrossRef]
- ZMT, Sim4Life-v5.0. Available online: https://zmt.swiss/news-and-events/news/sim4life/sim4life-major-release-v5-0 (accessed on 14 April 2020).
- ICNIRP. Guidelines for limiting exposure to electromagnetic fields (100 kHz to 300 GHz). Health Phys. 2020, 118, 483–524. [Google Scholar] [CrossRef]
- Arduino, A.; Bottauscio, O.; Chiampi, M.; Giaccone, L.; Liorni, I.; Kuster, N.; Zilberti, L.; Zucca, M. Accuracy Assessment of Numerical Dosimetry for the Evaluation of Human Exposure to Electric Vehicle Inductive Charging Systems. IEEE Trans. Electromagn. Compat. 2020. [Google Scholar] [CrossRef]















| Session | TX, RX Coil Position | TX Current (A) | RX Current (A) | Phase Shift (deg) | Measurement Region | |||
|---|---|---|---|---|---|---|---|---|
| Avg. | Std. Dev. | Avg. | Std. Dev. | Avg. | Std. Dev. | |||
| S.1 | Aligned | 427 | 8 | 108 | 23 | 89 | 1.5 | Inside bus at floor level |
| S.2 | Aligned | 485 | 3 | 81 | 0.5 | 82 | 0.3 | In three passenger positions (P1, P2, P3), in bystander position (B) and in driver position (D) |
| S.3 | Misaligned (0.843 m along the y-axis) | 711 | 78 | 83 | 0.4 | 76 | 0.6 | Along three lines at different heights along the bus entrance |
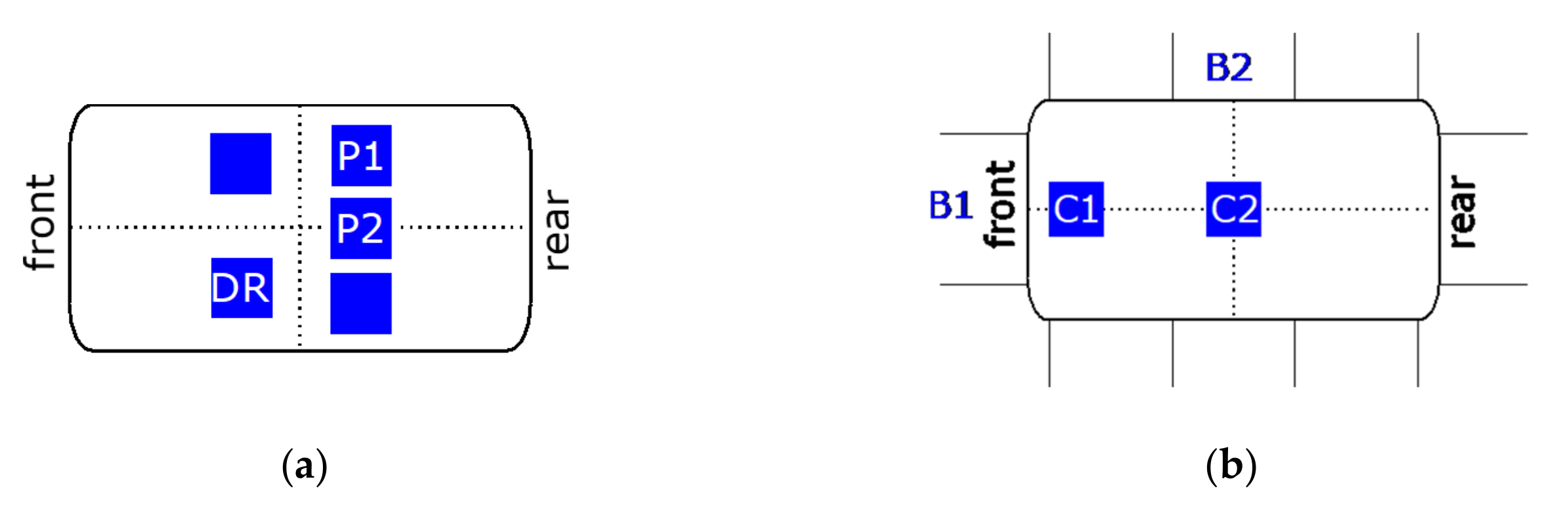
| Position | Outside the Bus | Inside the Bus |
|---|---|---|
| Ankle | Z = 0.1 m | Z = 0.28 m |
| knee | Z = 0.5 m | Z = 0.7 m |
| trunk | Z = 1.4 m | Z = 1.6 m |
| head | Z = 1.7 m | Z = 1.9 m |
| Source of Uncertainty | Value | Probability | Divisor | c1 | u1 |
|---|---|---|---|---|---|
| % | Distribution | % | |||
| Calibration of ELT-400 | 0.55 | Normal | 2 | 1 | 0.275 |
| 3D tracker positional accuracy (automated) | 0.23 | Rectangular | 1.7321 | 1 | 0.133 |
| Spatial uncertainty accounting for probe volume | 0.30 | Normal | 2 | 1 | 0.150 |
| Reproducibility | 0.10 | Normal | 2 | 1 | 0.050 |
| Combined uncertainty for 3D tracked measurements | Normal | 0.344 | |||
| Expanded uncertainty for 3D tracked measurements | Normal | k = 2 | 0.688 | ||
| Source of Uncertainty | Value | Probability | Divisor | c1 | u1 |
|---|---|---|---|---|---|
| % | Distribution | % | |||
| Calibration of ELT-400 | 0.55 | Normal | 2 | 1 | 0.275 |
| Manual positional accuracy | 2.80 | Rectangular | 1.7321 | 1 | 1.617 |
| Spatial uncertainty accounting for probe volume | 0.30 | Normal | 2 | 1 | 0.150 |
| Reproducibility | 0.10 | Normal | 2 | 1 | 0.050 |
| Combined uncertainty for 3D tracked measurements | Normal | 1.647 | |||
| Expanded uncertainty for 3D tracked measurements | Normal | k = 2 | 3.295 | ||
| IPT System Position | ViP Model Position | Posture |
|---|---|---|
| Central/Front | B2/B1 | Duke standing |
| Central/Front | B2/B1 | Duke crouching on knees |
| Front | B1 | Duke lying with arm stretched |
| Central/Front | B2/B1 | Thelonious sitting |
| Central | inside the car | Charlie (P2), Duke (DR) and Thelonious (P3) sitting |
| IPT System Position | ViP Model Position | Posture |
|---|---|---|
| Central | outside/front of the door | Duke standing |
| Central | outside/front of the door | Charlie sitting |
| Central | outside/front of the door | Duke crouching |
| Central | outside/front of the door | Thelonious sitting |
| Central | inside/front of the door | Duke sitting |
| Central | inside/front of the door | Charlie sitting |
| Guideline | Metric | f = 27.8 kHz | f = 85 kHz | Averaging Method |
|---|---|---|---|---|
| ICNIRP 2010 | Electric field (V/m) | 3.75 | 11.47 | 2 mm side cube |
| ICNIRP 1998 | Current density (mA/m2) | 55 | 170 | 1 cm2 disc |
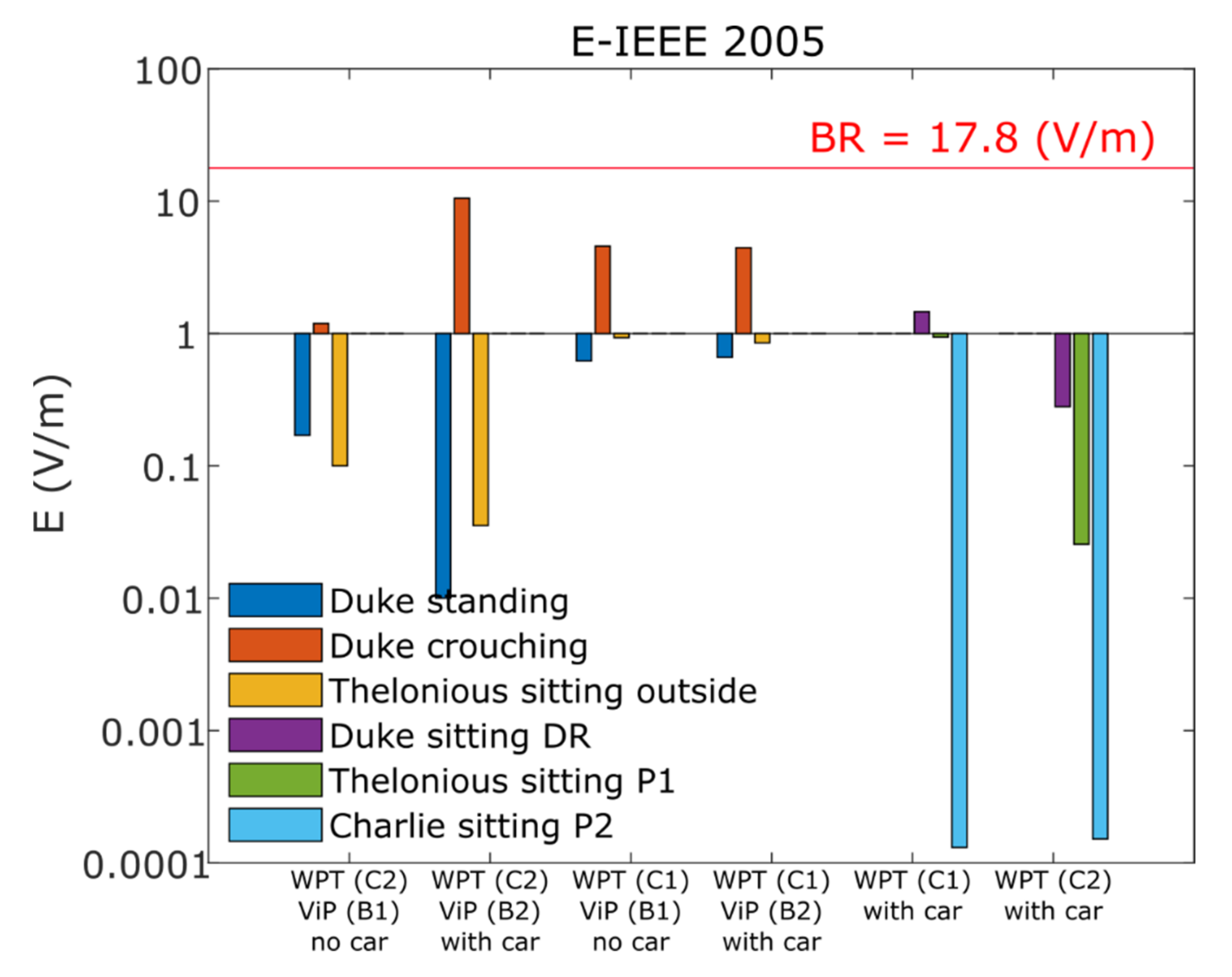
| IEEE 2005 | Electric field (V/m) | 5.81 | 17.76 | 5 mm line segment |
| ViP Model Position | Posture | E-ICNIRP (V/m) (in Bracket the Ratio with Respect to the Limit) | E-IEEE (V/m) (in Bracket the Ratio with Respect to the Limit) | J-ICNIRP (mA/m2) (in Bracket the Ratio with Respect to the Limit) |
|---|---|---|---|---|
| Carbon-steel alloy bus-body | ||||
| outside/central | Duke standing | 0.3 (0.08) | 0.2 (0.03) | 4.7 (0.08) |
| outside/central | Charlie sitting | 0.2 (0.05) | 0.1 (0.02)) | 4.2 (0.07) |
| outside/central | Duke crouching | 4.2 (1.12)) | 3.1 (0.53) | 36.8 (0.66) |
| outside/central | Thelonious sitting | 0.6 (0.16) | 0.4 (0.07) | 76.8 (1.38) |
| Inside | Duke standing | 0.2 (0.05) | 0.1 (0.02) | 4.3 (0.08) |
| Inside | Charlie sitting | 0.2 (0.05) | 0.1 (0.02) | 2.6 (0.05) |
| Fiberglass bus-body | ||||
| outside/central | Duke standing | 0.4 (0.01) | 0.3 (0.05) | 4.4 (0.08) |
| outside/central | Charlie sitting | 0.2 (0.06) | 0.2 (0.03) | 5.5 (0.10) |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liorni, I.; Bottauscio, O.; Guilizzoni, R.; Ankarson, P.; Bruna, J.; Fallahi, A.; Harmon, S.; Zucca, M. Assessment of Exposure to Electric Vehicle Inductive Power Transfer Systems: Experimental Measurements and Numerical Dosimetry. Sustainability 2020, 12, 4573. https://doi.org/10.3390/su12114573
Liorni I, Bottauscio O, Guilizzoni R, Ankarson P, Bruna J, Fallahi A, Harmon S, Zucca M. Assessment of Exposure to Electric Vehicle Inductive Power Transfer Systems: Experimental Measurements and Numerical Dosimetry. Sustainability. 2020; 12(11):4573. https://doi.org/10.3390/su12114573
Chicago/Turabian StyleLiorni, Ilaria, Oriano Bottauscio, Roberta Guilizzoni, Peter Ankarson, Jorge Bruna, Arya Fallahi, Stuart Harmon, and Mauro Zucca. 2020. "Assessment of Exposure to Electric Vehicle Inductive Power Transfer Systems: Experimental Measurements and Numerical Dosimetry" Sustainability 12, no. 11: 4573. https://doi.org/10.3390/su12114573
APA StyleLiorni, I., Bottauscio, O., Guilizzoni, R., Ankarson, P., Bruna, J., Fallahi, A., Harmon, S., & Zucca, M. (2020). Assessment of Exposure to Electric Vehicle Inductive Power Transfer Systems: Experimental Measurements and Numerical Dosimetry. Sustainability, 12(11), 4573. https://doi.org/10.3390/su12114573





