Persistence in Self-Employment Rates before the Great Lockdown: The Case of the UK
Abstract
:1. Introduction
2. A Selective Survey of Previous Literature
3. Methods and Results
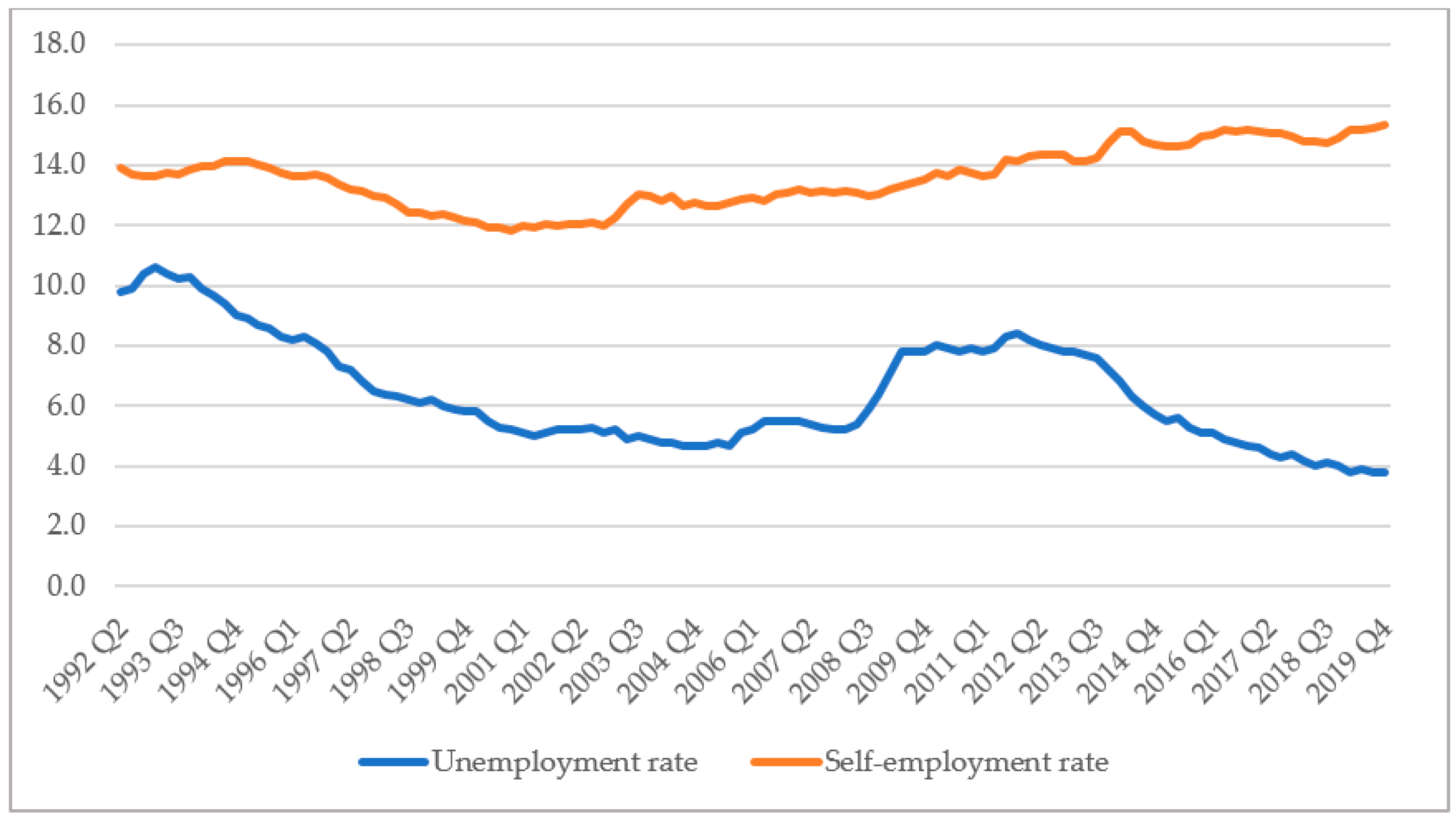
3.1. Data and Measurement Issues
3.2. Methodology
4. Results and Discussion
4.1. Unit Roots
4.2. An Unobserved Component Model
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A
Fractional Integration
| Coefficient | Std. Error | t-Value | t-Prob | |
|---|---|---|---|---|
| d parameter | 0.7762 | 0.05297 | 4.29 | 0.0000 |
| Constant | 11.0493 | 7.849 | 1.41 | 0.1610 |
| 3 Lags | 4 Lags | 6 Lags | ||||
|---|---|---|---|---|---|---|
| Regime | i = 1 | i = 2 | i = 1 | i = 2 | i = 1 | i = 2 |
| 0.037 * | 0.038 * | 0.043 *** | 0.040 ** | 3.146 ** | 1.851 ** | |
| (0.022) | (0.026) | (0.018) | (0.021) | (1.409) | (1.102) | |
| 0.573 *** | 0.592 *** | 0.773 *** | ||||
| (0.149) | (0.146) | (0.061) | ||||
| τ | −0.0040 | 0.0302 | 0.0286 | |||
| % obs. | 54.13% | 45.87% | 46.79% | 53.21% | 47.71% | 52.29% |
References
- Congregado, E.; de Andrés, M.I.; Roman, C. Explorando la heterogeneidad en el empleo mediado por plataformas digitales: Intensidad y localización. Rev. Econ. Labor. 2019, 16, 69–103. [Google Scholar] [CrossRef]
- Broughton, N.; Richards, B. Tough Gig: Low Paid Self-Employment in London and the UK; Social Market Foundation: London, UK, 2016. [Google Scholar]
- Kenney, M.; Zysman, J. The rise of the platform economy. Issues Sci. Technol. 2016, 32, 61. [Google Scholar]
- IPSE. Exploring the Rise of Self-Employment in the Modern Economy. Available online: https://www.ipse.co.uk/uploads/assets/uploaded/59868faa-841d-496d-bba6891d417a7e94.pdf (accessed on 25 May 2020).
- Saridakis, G.; Papaioannou, G. Forecasting self-employment in the UK. Empir. Econ. Lett. 2014, 13, 923–930. [Google Scholar]
- Parker, S.C.; Congregado, E.; Golpe, A.A. Is entrepreneurship a leading or lagging indicator of the business cycle? Evidence from UK self-employment data. Int. Small Bus. J. 2012, 30, 736–753. [Google Scholar] [CrossRef]
- Amankawah, A.; Wales, P. Trends in Self-Employment in the UK: 2001 to 2015; Office for National Statistics: Newport, UK, 2016.
- Tomlison, D.; Corlett, A. A Tough Gig? The Nature of Self-Employment in 21st Century Britain and Policy Implications; Resolution Foundation: London, UK, 2017. [Google Scholar]
- Caliendo, M.; Goethner, M.; Weißenberger, M. Entrepreneurial persistence beyond survival: Measurement and determinants. J. Small Bus. Manag. 2020, 58, 617–647. [Google Scholar] [CrossRef]
- Dvouletý, O.; Lukeš, M. Review of empirical studies on self-employment out of unemployment: Do self-employment policies make a positive impact? Int. Rev. Entrep. 2016, 14, 361–376. [Google Scholar]
- Boeri, T.; Giupponi, G.; Krueger, A.B.; Machin, S. Solo Self-Employment and Alternative Work Arrangements: A Cross-Country Perspective on the Changing Composition of Jobs. J. Econ. Perspect. 2020, 34, 170–195. [Google Scholar] [CrossRef]
- Román, C.; Congregado, E.; Millán, J.M. Dependent self-employment as a way to evade employment protection legislation. Small Bus. Econ. 2011, 37, 363–392. [Google Scholar] [CrossRef]
- Böheim, R.; Muehlberger, U. Dependent Forms of Self-employment in the UK: Identifying Workers on the Border between Employment and Self-employment; Discussion Paper No. 1963; The Institute for the Study of Labor (IZA): Bonn, Germany, 2006; p. 24. [Google Scholar]
- Böheim, R.; Muehlberger, U. Dependent self-employment: Workers between employment and self-employment in the UK. Z. ArbeitsmarktForsch. 2009, 42, 182–195. [Google Scholar] [CrossRef] [Green Version]
- Caliendo, M.; Kühn, S. Start-up subsidies for the unemployed: Long-term evidence and effect heterogeneity. J. Public Econ. 2011, 95, 311–331. [Google Scholar] [CrossRef] [Green Version]
- Caliendo, M.; Künn, S.; Weißenberger, M. Catching up or Lagging behind? The Long-Term Business Potential of Subsidized Start-Ups out of Unemployment; Discussion Paper No. 12690; The Institute for the Study of Labor (IZA): Bonn, Germany, 2019; p. 34. [Google Scholar]
- Román, C.; Congregado, E.; Millán, J.M. The role of labor market institutions on entrepreneurship dynamics in times of crisis: Evidence from European countries. In Entrepreneurship and Global Competitiveness in Regional Economies: Determinants and Policy Implications (Advances in the Study of Entrepreneurship, Innovation and Economic Growth); Libecap, G.D., Hoskinson, S., Eds.; Emerald Group Publishing Limited: Bingley, UK, 2011; Volume 22, pp. 161–183. [Google Scholar] [CrossRef]
- Torrini, R. Cross-country differences in self-employment rates: The role of institutions. Labour Econ. 2005, 12, 661–683. [Google Scholar] [CrossRef]
- Baumann, F.; Brändle, T. Self-employment, educational attainment and employment protection legislation. Labour Econ. 2012, 19, 846–859. [Google Scholar] [CrossRef]
- Robson, M.T. Does stricter employment protection legislation promote self-employment? Small Bus. Econ. 2003, 21, 309–319. [Google Scholar] [CrossRef] [Green Version]
- Acs, Z. How is Entrepreneurship Good for Economic Growth? Innov. Technol. Gov. Glob. 2006, 1, 97–107. [Google Scholar] [CrossRef]
- Lucas, R.E. On the Size Distribution of Business Firms. Bell J. Econ. 1978, 9, 508–523. [Google Scholar] [CrossRef]
- Cowling, M.; Mitchell, P. The Evolution of U.K. Self-Employment: A Study of Government Policy and the Role of the Macroeconomy. Manch. Sch. 1997, 65, 427–442. [Google Scholar] [CrossRef]
- Blanchflower, D.G.; Shadforth, C. Entrepreneurship in the UK. Foundation and Trends in Entrepreneurship; Now Publishers: Hanover, MA, USA, 2007. [Google Scholar]
- Blundell, J.; Machin, S. Self-Employment in the Covid-19 Crisis. A CEP Covid-19 Analysis; no. 003; Centre for Economic Performance, LSE: London, UK, 2020. [Google Scholar]
- Thurik, A.R. Entrepreneurship and Unemployment in the UK. Scott. J. Political Econ. 2003, 50, 264–290. [Google Scholar] [CrossRef]
- Faria, J.R.; Cuestas, J.C.; Gil-Alana, L.A. Unemployment and entrepreneurship: A cyclical relation? Econ. Lett. 2009, 105, 318–320. [Google Scholar] [CrossRef]
- Carmona, M.; Congregado, E.; Golpe, A.A. Co-Movement and Causality between Self-employment, Unemployment and Business Cycle in the EU-12. Int. Rev. Entrep. 2010, 8, 1–35. [Google Scholar]
- Millán, J.M.; Congregado, E.; Román, C. Determinants of self-employment survival in Europe. Small Bus. Econ. 2012, 38, 231–258. [Google Scholar] [CrossRef]
- Millán, J.M.; Congregado, E.; Román, C. Entrepreneurship persistence with and without personnel: The role of human capital and previous unemployment. Int. Entrep. Manag. J. 2014, 10, 187–206. [Google Scholar] [CrossRef]
- Lechmann, D.S.J.; Wunder, C. The dynamics of solo self-employment: Persistence and transition to employership. Labour Econ. 2017, 49, 95–105. [Google Scholar] [CrossRef] [Green Version]
- Baptista, R.; Karaöz, M.; Mendonça, J. The impact of human capital on the early success of necessity versus opportunity-based entrepreneurs. Small Bus. Econon. 2014, 42, 831–847. [Google Scholar] [CrossRef] [Green Version]
- Congregado, E.; Golpe, A.A.; Parker, S.C. The dynamics of entrepreneurship: Hysteresis, business cycles and government policy. Empir. Econ. 2012, 43, 1239–1261. [Google Scholar] [CrossRef] [Green Version]
- Parker, S.C.; Congregado, E.; Golpe, A.A. Testing for hysteresis in entrepreneurship in 23 OECD countries. Appl. Econ. Lett. 2012, 19, 61–66. [Google Scholar] [CrossRef]
- Gil-Alana, L.A.; Payne, J.E. A time-series analysis of US entrepreneurship: Evidence from fractional integration. Appl. Econ. Lett. 2015, 22, 521–524. [Google Scholar] [CrossRef]
- Jaeger, A.; Parkinson, M. Testing for Hysteresis in Unemployment Rates: An unobserved component approach. Empir. Econ. 1990, 15, 185–198. [Google Scholar] [CrossRef] [Green Version]
- Congregado, E.; Golpe, A.; van Stel, A. The recession-push hypothesis reconsidered. Int. Entrep. Manag. J. 2012, 8, 325–342. [Google Scholar] [CrossRef]
- Congregado, E. (Ed.) Measuring Entrepreneurship; Springer: New York, NY, USA, 2008. [Google Scholar]
- Jaeger, A.; Parkinson, M. Some evidence on Hysteresis in Unemployment Rates. Eur. Econ. Rev. 1994, 38, 329–342. [Google Scholar] [CrossRef] [Green Version]
- Pérez-Alonso, A.; Di Sanzo, S. Unemployment and hysteresis: A nonlinear unobserved components approach. Stud. Nonlinear Dyn. Econom. 2010, 15, 1–29. [Google Scholar] [CrossRef] [Green Version]
- Carmona, M.; Congregado, E.; Golpe, A.A.; Iglesias, J. Self-employment and business cycles: Searching for asymmetries in a panel of 23 OECD countries. J. Bus. Econ. Manag. 2016, 17, 1155–1171. [Google Scholar] [CrossRef] [Green Version]
- Gil-Alana, L.A.; Hualde, J. Fractional integration and cointegration: An overview and an empirical application. In Palgrave Handbook of Econometrics; Palgrave Macmillan: London, UK, 2009; pp. 434–469. [Google Scholar]

| I(1) vs. I(0) | |||||||
|---|---|---|---|---|---|---|---|
| ADF | PP | Ng-Perron | |||||
| Variable | Test | Test | MZa | MZt | MSB | MPT | Lags |
| Self-employment rate | −1.6442 (0.7688) | −1.7552 (0.7197) | −2.14730 | −0.95439 | 0.44446 | 38.1818 | 1 |
| GDP | −1.2879 (0.8856) | −1.4225 (0.8492) | −9.36138 | −2.05835 | 0.21988 | 10.1738 | 1 |
| Unemployment rate | −3.2788 * (0.0758) | −1.5470 (0.8073) | −4.51677 | −1.50199 | 0.33254 | 20.1686 | 2 |
| 3 Lags | 4 Lags | |
|---|---|---|
| Natural rate equation | ||
| β | 1.842 *** (0.082) | 1.893 *** (0.040) |
| σN | 0.089 *** (0.030) | 0.000 (0.001) |
| Cyclical rate equation | ||
| ϕ1 | −0.469 *** (0.303) | −0.611 *** (0.079) |
| ϕ2 | 0.391 *** (0.092) | 0.287 *** (0.076) |
| σC | 0.115 *** (0.030) | 0.149 *** (0.012) |
| α | 0.821 *** (0.058) | 0.808 *** (0.060) |
| δ | 1.038 ** (0.504) | 0.490 ** (0.248) |
| σD | 0.467 *** (0.057) | 0.480 *** (0.050) |
| Bootstrap p value | 0.25 |
| 3 Lags | 4 Lags | |
|---|---|---|
| Natural rate equation | ||
| β | 1.945 ** (1.175) | 1.192 *** (0.146) |
| σN | 0.123 *** (0.012) | 0.000 (0.014) |
| Cyclical rate equation | ||
| ϕ1 | 0.520 *** (0.210) | 0.037 (0.162) |
| ϕ2 | −0.068 (0.055) | 0.178 * (0.132) |
| σC | 0.048 (0.040) | 0.148 *** (0.012) |
| α | 0.4812 *** (0.107) | 0.485 *** (0.101) |
| δ | 2.071 (9.393) | 0.519 (2.236) |
| σD | 3.035 *** (0.213) | 3.036 *** (0.213) |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lopez-Perez, E.; Rodriguez-Santiago, A.; Congregado, E. Persistence in Self-Employment Rates before the Great Lockdown: The Case of the UK. Sustainability 2020, 12, 6429. https://doi.org/10.3390/su12166429
Lopez-Perez E, Rodriguez-Santiago A, Congregado E. Persistence in Self-Employment Rates before the Great Lockdown: The Case of the UK. Sustainability. 2020; 12(16):6429. https://doi.org/10.3390/su12166429
Chicago/Turabian StyleLopez-Perez, Elisabeth, Ana Rodriguez-Santiago, and Emilio Congregado. 2020. "Persistence in Self-Employment Rates before the Great Lockdown: The Case of the UK" Sustainability 12, no. 16: 6429. https://doi.org/10.3390/su12166429
APA StyleLopez-Perez, E., Rodriguez-Santiago, A., & Congregado, E. (2020). Persistence in Self-Employment Rates before the Great Lockdown: The Case of the UK. Sustainability, 12(16), 6429. https://doi.org/10.3390/su12166429





