Uncertainty in Intermodal and Synchromodal Transport: Review and Future Research Directions
Abstract
1. Introduction
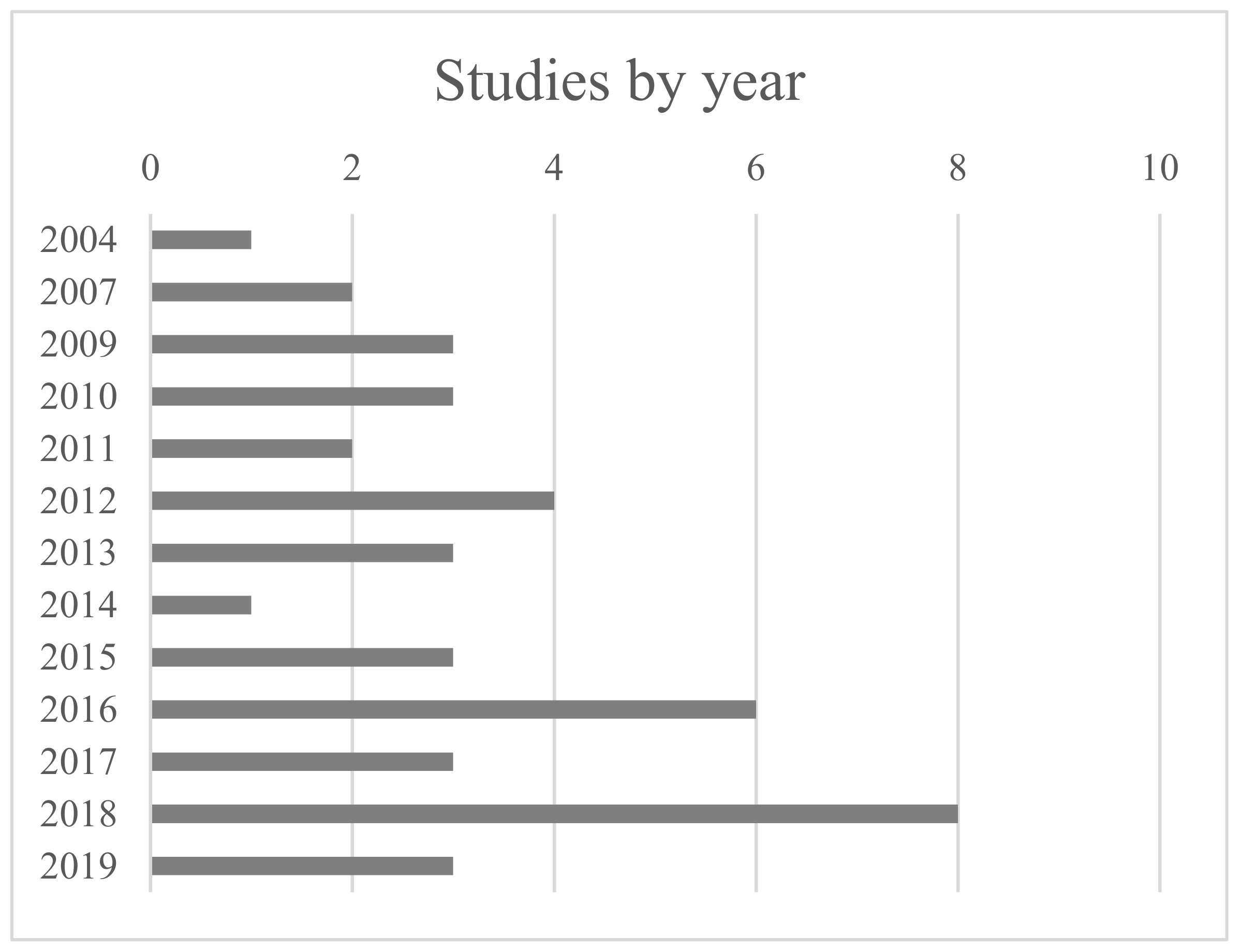
2. Methodology
3. Strategic Decisions
3.1. Planning Problems
3.2. Solution Methods
4. Tactical Decisions
4.1. Planning Problems
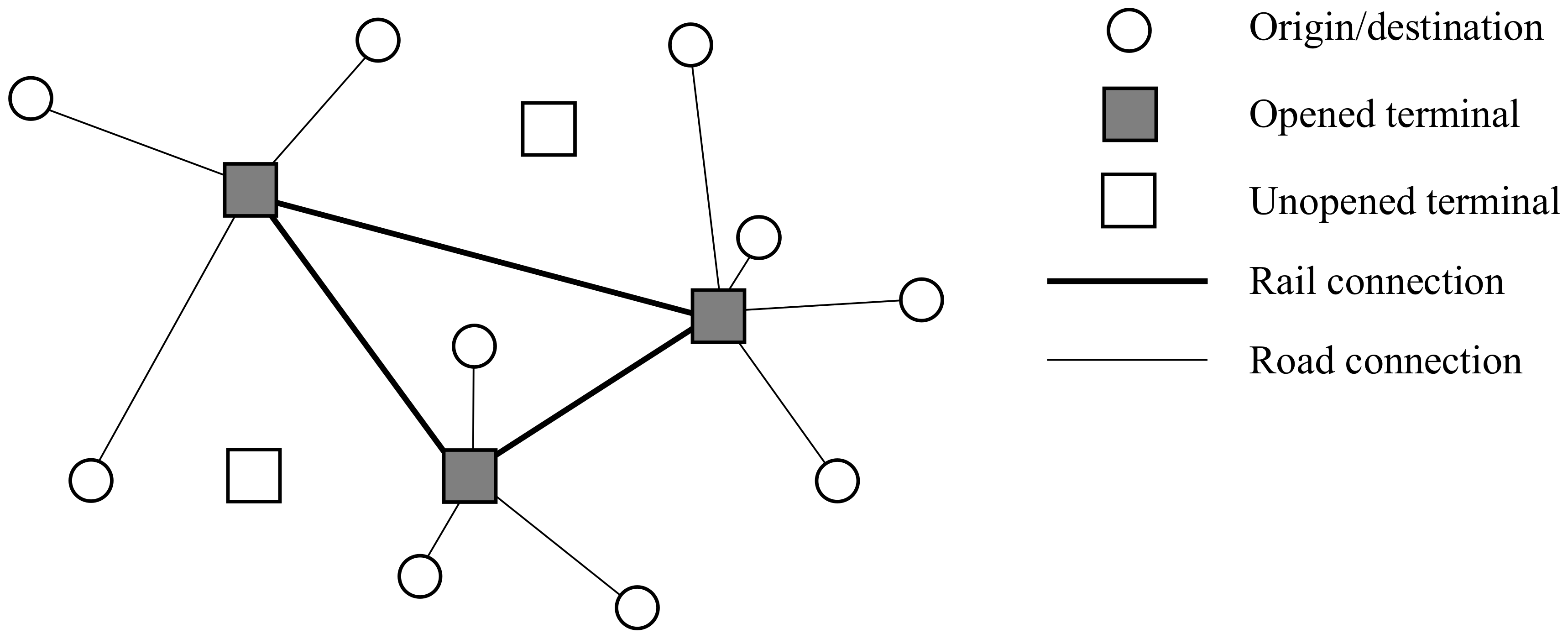
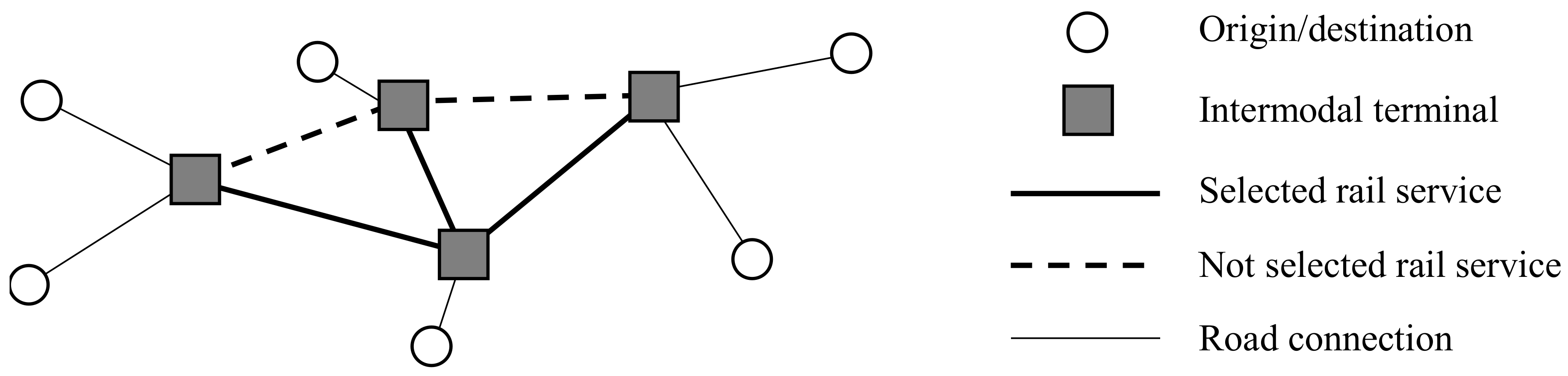
4.1.1. Service Network Design
4.1.2. Network Flow Planning
4.2. Solution Methods
5. Operational Decisions
5.1. Planning Problems
5.1.1. Replanning
5.1.2. Resource Management
5.2. Solution Methods
6. Discussion and Future Research Directions
- The share of road transport is higher when considering stochastic demand, transit times or capacity compared to a deterministic setting.
Author Contributions
Funding
Conflicts of Interest
Appendix A

References
- EUROSTAT. EU Transport in Figures; EUROSTAT: Luxembourg, 2019; p. 138. [Google Scholar]
- Reis, V. Should we keep on renaming a+ 35-year-old baby? J. Transp. Geogr. 2015, 46, 173–179. [Google Scholar] [CrossRef]
- Agamez-Arias, A.-D.-M.; Moyano-Fuentes, J. Intermodal transport in freight distribution: A literature review. Transp. Rev. 2017, 37, 782–807. [Google Scholar] [CrossRef]
- Hanssen, T.-E.S.; Mathisen, T.A.; Jørgensen, F. Generalized transport costs in intermodal freight transport. Procedia Soc. Behav. Sci. 2012, 54, 189–200. [Google Scholar] [CrossRef]
- Liao, C.-H.; Tseng, P.-H.; Lu, C.-S. Comparing carbon dioxide emissions of trucking and intermodal container transport in Taiwan. Transp. Res. Part D Transp. Environ. 2009, 14, 493–496. [Google Scholar] [CrossRef]
- Van Heeswijk, W.; Mes, M.; Schutten, J.; Zijm, W. Freight consolidation in intermodal networks with reloads. Flex. Serv. Manuf. J. 2018, 30, 452–485. [Google Scholar] [CrossRef]
- Pan, S.; Ballot, E.; Fontane, F. The reduction of greenhouse gas emissions from freight transport by pooling supply chains. Int. J. Prod. Econ. 2013, 143, 86–94. [Google Scholar] [CrossRef]
- Craighead, C.W.; Blackhurst, J.; Rungtusanatham, M.J.; Handfield, R.B. The severity of supply chain disruptions: Design characteristics and mitigation capabilities. Decis. Sci. 2007, 38, 131–156. [Google Scholar] [CrossRef]
- Svensson, G. A conceptual framework for the analysis of vulnerability in supply chains. Int. J. Phys. Distrib. Logist. Manag. 2000, 30, 731–750. [Google Scholar] [CrossRef]
- Evans, J.S. Strategic flexibility for high technology manoeuvres: A conceptual framework. J. Manag. Stud. 1991, 28, 69–89. [Google Scholar] [CrossRef]
- Goetz, A.R.; Szyliowicz, J.S. Revisiting transportation planning and decision making theory: The case of Denver International Airport. Transp. Res. Part A Policy Pract. 1997, 31, 263–280. [Google Scholar] [CrossRef]
- Chen, L.; Miller-Hooks, E. Resilience: An indicator of recovery capability in intermodal freight transport. Transp. Sci. 2012, 46, 109–123. [Google Scholar] [CrossRef]
- SteadieSeifi, M.; Dellaert, N.P.; Nuijten, W.; Van Woensel, T.; Raoufi, R. Multimodal freight transportation planning: A literature review. Eur. J. Oper. Res. 2014, 233, 1–15. [Google Scholar] [CrossRef]
- Miller-Hooks, E.; Zhang, X.; Faturechi, R. Measuring and maximizing resilience of freight transportation networks. Comput. Oper. Res. 2012, 39, 1633–1643. [Google Scholar] [CrossRef]
- Flodén, J.; Bärthel, F.; Sorkina, E. Transport buyers choice of transport service—A literature review of empirical results. Res. Transp. Bus. Manag. 2017, 100, 35–45. [Google Scholar] [CrossRef]
- Sommar, R.; Woxenius, J. Time perspectives on intermodal transport of consolidated cargo. Eur. J. Transp. Infrastruct. Res. 2007, 7. [Google Scholar] [CrossRef]
- Uddin, M.; Huynh, N. Routing model for multicommodity freight in an intermodal network under disruptions. Transp. Res. Rec. 2016, 2548, 71–80. [Google Scholar] [CrossRef]
- Uddin, M.; Huynh, N. Reliable Routing of Road-Rail Intermodal Freight under Uncertainty. Netw. Spat. Econ. 2019, 19, 929–952. [Google Scholar] [CrossRef]
- Demir, E.; Burgholzer, W.; Hrušovský, M.; Arıkan, E.; Jammernegg, W.; Van Woensel, T. A green intermodal service network design problem with travel time uncertainty. Transp. Res. Part B Methodol. 2016, 93, 789–807. [Google Scholar] [CrossRef]
- Verweij, K. Synchronic modalities–Critical success factors. In Logistics Handbook Edition; Evofenedex: Zoetermeer, The Netherlands, 2011; pp. 75–88. [Google Scholar]
- Ambra, T.; Caris, A.; Macharis, C. Should I Stay or Should I Go? Assessing Intermodal and Synchromodal Resilience from a Decentralized Perspective. Sustainability 2019, 11, 1765. [Google Scholar] [CrossRef]
- van Riessen, B.; Negenborn, R.R.; Dekker, R. Synchromodal container transportation: An overview of current topics and research opportunities. In Proceedings of the International Conference on Computational Logistics, Barcelona, Spain, 27–29 July 2015; pp. 386–397. [Google Scholar]
- Macharis, C.; Bontekoning, Y.M. Opportunities for OR in intermodal freight transport research: A review. Eur. J. Oper. Res. 2004, 153, 400–416. [Google Scholar] [CrossRef]
- Crainic, T.G. Service network design in freight transportation. Eur. J. Oper. Res. 2000, 122, 272–288. [Google Scholar] [CrossRef]
- Qu, W.; Rezaei, J.; Maknoon, Y.; Tavasszy, L. Hinterland freight transportation replanning model under the framework of synchromodality. Transp. Res. Part E Logist. Transp. Rev. 2019, 131, 308–328. [Google Scholar] [CrossRef]
- Li, L.; Negenborn, R.R.; De Schutter, B. Intermodal freight transport planning–A receding horizon control approach. Transp. Res. Part C Emerg. Technol. 2015, 60, 77–95. [Google Scholar] [CrossRef]
- Elbert, R.; Müller, J.P.; Rentschler, J. Tactical network planning and design in multimodal transportation—A systematic literature review. Res. Transp. Bus. Manag. 2020, 100462. [Google Scholar] [CrossRef]
- Pizzol, M. Deterministic and stochastic carbon footprint of intermodal ferry and truck freight transport across Scandinavian routes. J. Clean. Prod. 2019, 224, 626–636. [Google Scholar] [CrossRef]
- Carlo, H.J.; Vis, I.F.; Roodbergen, K.J. Transport operations in container terminals: Literature overview, trends, research directions and classification scheme. Eur. J. Oper. Res. 2014, 236, 1–13. [Google Scholar] [CrossRef]
- Carlo, H.J.; Vis, I.F.; Roodbergen, K.J. Seaside operations in container terminals: Literature overview, trends, and research directions. Flex. Serv. Manuf. J. 2015, 27, 224–262. [Google Scholar] [CrossRef]
- Carlo, H.J.; Vis, I.F.; Roodbergen, K.J. Storage yard operations in container terminals: Literature overview, trends, and research directions. Eur. J. Oper. Res. 2014, 235, 412–430. [Google Scholar] [CrossRef]
- Alumur, S.; Kara, B.Y. Network hub location problems: The state of the art. Eur. J. Oper. Res. 2008, 190, 1–21. [Google Scholar] [CrossRef]
- Campbell, J.F.; O’Kelly, M.E. Twenty-five years of hub location research. Transp. Sci. 2012, 46, 153–169. [Google Scholar] [CrossRef]
- Farahani, R.Z.; Hekmatfar, M.; Arabani, A.B.; Nikbakhsh, E. Hub location problems: A review of models, classification, solution techniques, and applications. Comput. Ind. Eng. 2013, 64, 1096–1109. [Google Scholar] [CrossRef]
- Sim, T.; Lowe, T.J.; Thomas, B.W. The stochastic p-hub center problem with service-level constraints. Comput. Oper. Res. 2009, 36, 3166–3177. [Google Scholar] [CrossRef]
- Ishfaq, R.; Sox, C.R. Design of intermodal logistics networks with hub delays. Eur. J. Oper. Res. 2012, 220, 629–641. [Google Scholar] [CrossRef]
- Meraklı, M.; Yaman, H. Robust intermodal hub location under polyhedral demand uncertainty. Transp. Res. Part B Methodol. 2016, 86, 66–85. [Google Scholar] [CrossRef]
- Fotuhi, F.; Huynh, N. Reliable intermodal freight network expansion with demand uncertainties and network disruptions. Netw. Spat. Econ. 2017, 17, 405–433. [Google Scholar] [CrossRef]
- Karimi, B.; Bashiri, M.; Nikzad, E. Multi-commodity Multimodal Splittable Logistics Hub Location Problem with Stochastic Demands. Int. J. Eng. 2018, 31, 1935–1942. [Google Scholar] [CrossRef]
- Wang, R.; Yang, K.; Yang, L.; Gao, Z. Modeling and optimization of a road–rail intermodal transport system under uncertain information. Eng. Appl. Artif. Intell. 2018, 72, 423–436. [Google Scholar] [CrossRef]
- Abbassi, A.; El hilali Alaoui, A.; Boukachour, J. Robust optimisation of the intermodal freight transport problem: Modeling and solving with an efficient hybrid approach. J. Comput. Sci. 2019, 30, 127–142. [Google Scholar] [CrossRef]
- Woxenius, J. Generic framework for transport network designs: Applications and treatment in intermodal freight transport literature. Transp. Rev. 2007, 27, 733–749. [Google Scholar] [CrossRef]
- Dyer, M.E.; Frieze, A.M. A simple heuristic for the p-centre problem. Oper. Res. Lett. 1985, 3, 285–288. [Google Scholar] [CrossRef]
- Teitz, M.B.; Bart, P. Heuristic methods for estimating the generalized vertex median of a weighted graph. Oper. Res. 1968, 16, 955–961. [Google Scholar] [CrossRef]
- Wieberneit, N. Service network design for freight transportation: A review. OR Spectr. 2008, 30, 77–112. [Google Scholar] [CrossRef]
- Yang, K.; Yang, L.; Gao, Z. Planning and optimization of intermodal hub-and-spoke network under mixed uncertainty. Transp. Res. Part E Logist. Transp. Rev. 2016, 95, 248–266. [Google Scholar] [CrossRef]
- Sun, Y.; Hrušovský, M.; Zhang, C.; Lang, M. A time-dependent fuzzy programming approach for the green multimodal routing problem with rail service capacity uncertainty and road traffic congestion. Complexity 2018, 2018, 8645793. [Google Scholar] [CrossRef]
- Zhao, Y.; Liu, R.; Zhang, X.; Whiteing, A. A chance-constrained stochastic approach to intermodal container routing problems. PLoS ONE 2018, 13, e0192275. [Google Scholar] [CrossRef]
- Huang, M.; Hu, X.; Zhang, L. A Decision Method for Disruption Management Problems in Intermodal Freight Transport. In Proceedings of the 3rd International Conference on Intelligent Decision Technologies (IDT’2011), Beirut, Lebanon, 11–14 December 2011; p. 13. [Google Scholar]
- Andersen, J.; Christiansen, M. Designing new European rail freight services. J. Oper. Res. Soc. 2009, 60, 348–360. [Google Scholar] [CrossRef]
- Lium, A.-G.; Crainic, T.G.; Wallace, S.W. A study of demand stochasticity in service network design. Transp. Sci. 2009, 43, 144–157. [Google Scholar] [CrossRef]
- Hoff, A.; Lium, A.-G.; Løkketangen, A.; Crainic, T.G. A metaheuristic for stochastic service network design. J. Heuristics 2010, 16, 653–679. [Google Scholar] [CrossRef]
- Crainic, T.G.; Fu, X.; Gendreau, M.; Rei, W.; Wallace, S.W. Progressive hedging-based metaheuristics for stochastic network design. Networks 2011, 58, 114–124. [Google Scholar] [CrossRef]
- Puettmann, C.; Stadtler, H. A collaborative planning approach for intermodal freight transportation. OR Spectr. 2010, 32, 809–830. [Google Scholar] [CrossRef]
- Bai, R.; Wallace, S.W.; Li, J.; Chong, A.Y.-L. Stochastic service network design with rerouting. Transp. Res. Part B Methodol. 2014, 60, 50–65. [Google Scholar] [CrossRef]
- Meng, Q.; Hei, X.; Wang, S.; Mao, H. Carrying capacity procurement of rail and shipping services for automobile delivery with uncertain demand. Transp. Res. Part E Logist. Transp. Rev. 2015, 82, 38–54. [Google Scholar] [CrossRef]
- Hrušovský, M.; Demir, E.; Jammernegg, W.; Van Woensel, T. Hybrid simulation and optimization approach for green intermodal transportation problem with travel time uncertainty. Flex. Serv. Manuf. J. 2018, 30, 486–516. [Google Scholar] [CrossRef]
- Zhao, Y.; Xue, Q.; Cao, Z.; Zhang, X. A two-stage chance constrained approach with application to stochastic intermodal service network design problems. J. Adv. Transp. 2018, 2018. [Google Scholar] [CrossRef]
- Layeb, S.B.; Jaoua, A.; Jbira, A.; Makhlouf, Y. A simulation-optimization approach for scheduling in stochastic freight transportation. Comput. Ind. Eng. 2018, 126, 99–110. [Google Scholar] [CrossRef]
- Sun, Y.; Zhang, G.; Hong, Z.; Dong, K. How uncertain information on service capacity influences the intermodal routing decision: A fuzzy programming perspective. Information 2018, 9, 24. [Google Scholar] [CrossRef]
- Li, Z.; Nachtmann, H.; Rossetti, M.D. WebShipCost-Quantifying Risk in Intermodal Transportation. In Proceedings of the IIE Annual Conference, Fayetteville, AR, USA, 8–10 October 2004. [Google Scholar]
- Meng, Q.; Wang, T.; Wang, S. Short-term liner ship fleet planning with container transshipment and uncertain container shipment demand. Eur. J. Oper. Res. 2012, 223, 96–105. [Google Scholar] [CrossRef]
- Magnanti, T.L.; Wong, R.T. Network design and transportation planning: Models and algorithms. Transp. Sci. 1984, 18, 1–55. [Google Scholar] [CrossRef]
- Shier, D.R. Iterative methods for determining the k shortest paths in a network. Networks 1976, 6, 205–229. [Google Scholar] [CrossRef]
- Kleywegt, A.J.; Shapiro, A.; Homem-de-Mello, T. The sample average approximation method for stochastic discrete optimization. SIAM J. Optim. 2002, 12, 479–502. [Google Scholar] [CrossRef]
- Bock, S. Real-time control of freight forwarder transportation networks by integrating multimodal transport chains. Eur. J. Oper. Res. 2010, 200, 733–746. [Google Scholar] [CrossRef]
- Burgholzer, W.; Bauer, G.; Posset, M.; Jammernegg, W. Analysing the impact of disruptions in intermodal transport networks: A micro simulation-based model. Decis. Support. Syst. 2013, 54, 1580–1586. [Google Scholar] [CrossRef]
- Escudero, A.; Muñuzuri, J.; Guadix, J.; Arango, C. Dynamic approach to solve the daily drayage problem with transit time uncertainty. Comput. Ind. 2013, 64, 165–175. [Google Scholar] [CrossRef]
- van Riessen, B.; Negenborn, R.R.; Lodewijks, G.; Dekker, R. Impact and relevance of transit disturbances on planning in intermodal container networks using disturbance cost analysis. Marit. Econ. Logist. 2015, 17, 440–463. [Google Scholar] [CrossRef]
- Sun, Y.; Schonfeld, P. Holding decisions for correlated vehicle arrivals at intermodal freight transfer terminals. Transp. Res. Part B Methodol. 2016, 90, 218–240. [Google Scholar] [CrossRef]
- Rivera, A.E.P.; Mes, M.R. Anticipatory freight selection in intermodal long-haul round-trips. Transp. Res. Part E Logist. Transp. Rev. 2017, 105, 176–194. [Google Scholar] [CrossRef]
- Rivera, A.E.P.; Mes, M.R. Scheduling drayage operations in synchromodal transport. In Proceedings of the International Conference on Computational Logistics, Southampton, UK, 18–20 October 2017; pp. 404–419. [Google Scholar]
- Topaloglu, H.; Powell, W.B. Dynamic-programming approximations for stochastic time-staged integer multicommodity-flow problems. Inf. J. Comput. 2006, 18, 31–42. [Google Scholar] [CrossRef]
- Topaloglu, H. A parallelizable and approximate dynamic programming-based dynamic fleet management model with random travel times and multiple vehicle types. In Dynamic Fleet Management; Springer: Cham, Switzerland, 2007; pp. 65–93. [Google Scholar] [CrossRef]
- Lam, S.-W.; Lee, L.-H.; Tang, L.-C. An approximate dynamic programming approach for the empty container allocation problem. Transp. Res. Part C Emerg. Technol. 2007, 15, 265–277. [Google Scholar] [CrossRef]
- Di Francesco, M.; Lai, M.; Zuddas, P. Maritime repositioning of empty containers under uncertain port disruptions. Comput. Ind. Eng. 2013, 64, 827–837. [Google Scholar] [CrossRef]
- van Riessen, B.; Negenborn, R.R.; Dekker, R. Real-time container transport planning with decision trees based on offline obtained optimal solutions. Decis. Support. Syst. 2016, 89, 1–16. [Google Scholar] [CrossRef]
- Solomon, M.M. Algorithms for the vehicle routing and scheduling problems with time window constraints. Oper. Res. 1987, 35, 254–265. [Google Scholar] [CrossRef]
| Planning Level | Total | Transit Times | Demand | Capacity | Costs | Hub Failures | Departure Times and Cancellations |
|---|---|---|---|---|---|---|---|
| Strategic | 7 | 3 | 4 | 1 | 2 | 1 | |
| Tactical | 23 | 12 | 11 | 7 | 1 | ||
| Operational | 12 | 6 | 7 | 2 | 1 | 1 |
| Authors (Year) | Reference | Transport Modes | Transit Times | Demand | Capacity | Hub Failures | Costs | Solution Method 1 | Uncertainty Mitigation |
|---|---|---|---|---|---|---|---|---|---|
| Sim et al. (2009) | [35] | Unspecified | x | A: Heuristic | Scenario generation | ||||
| Ishfaq and Sox (2012) | [36] | Rail, road | x | A: Tabu-search metaheuristic | Scenario generation | ||||
| Meraklı and Yaman (2016) | [37] | Unspecified | x | E: Benders decomposition | Robust optimisation | ||||
| Fotuhi and Huynh (2017) | [38] | Rail, road | x | x | A: Genetic algorithm | Robust optimisation | |||
| Karimi et al. (2018) | [39] | Unspecified | x | E: Solver | Scenario generation | ||||
| Wang et al. (2018) | [40] | Rail, road | x | x | x | A: Memetic algorithm | Scenario generation | ||
| Abbassi et al. (2019) | [41] | Ship, road | x | x | A: Simulated annealing metaheuristic | Robust optimisation |
| Authors (Year) | Reference | Modes | Transit Times | Demand | Capacity | Costs | Late Deliveries | Solution Method 1 | Uncertainty Mitigation |
|---|---|---|---|---|---|---|---|---|---|
| Andersen and Christiansen (2009) | [50] | Rail | x | Penalty for delivery time variability | E: Solver | Scenario generation | |||
| Lium et al. (2009) | [51] | Unspecified | x | Ad hoc capacity increase | E | Scenario generation | |||
| Hoff et al. (2010) | [52] | Unspecified | x | Ad hoc capacity increase | A: Metaheuristic | Scenario generation | |||
| Crainic et al. (2011) | [53] | Unspecified | x | Ad hoc capacity increase | A: Tabu-search metaheuristic | Scenario generation, recourse | |||
| Puettmann and Stadtler (2010) | [54] | Road, ship | x | Not allowed | E: Solver | Collaboration | |||
| Bai et al. (2014) | [55] | Unspecified | x | Ad hoc capacity increase, rerouting | E: Solver | Rerouting | |||
| Meng et al. (2015) | [56] | Barge, rail, road | x | Ad hoc capacity increase | A: SAA, matheuristic | Recourse | |||
| Demir et al. (2016) | [19] | Barge, rail, road | x | x | Penalty cost | A: SAA, solver | Recourse | ||
| Yang et al. (2016) | [46] | Air, rail, road | x | x | Travel time in objective | A: Simulated annealing metaheuristic | Scenario generation | ||
| Hrušovský et al. (2018) | [57] | Barge, rail, road | x | Ad hoc capacity increase | A: Simulation–optimisation | Scenario generation | |||
| Zhao et al. (2018) | [48] | Rail, ship | x | Penalty and nonfulfilment cost | A: Genetic algorithm | Scenario generation | |||
| Zhao et al. (2018) | [58] | Rail, Ship | x | x | Penalty cost | A: SAA, ant colony optimisation metaheuristic | Recourse | ||
| Layeb et al. (2018) | [59] | Barge, rail, road | x | x | Penalty cost | A: Simulation–optimisation | Scenario generation | ||
| Sun et al. (2018) | [60] | Rail, road | x | Not applicable | E: Solver | Scenario generation |
| Authors (Year) | Reference | Modes | Transit Times | Demand | Capacity | Late Deliveries | Solution Method 1 | Uncertainty Mitigation |
|---|---|---|---|---|---|---|---|---|
| Li et al. (2004) | [61] | Barge, rail, road | x | Cost and time in objective function | E | Scenario generation | ||
| Huang et al. (2011) | [49] | Rail, road, ship | x | No penalty cost | E: Depth-first search | Rerouting | ||
| Meng et al. (2012) | [62] | Ship | x | Excess demand is lost | A: SAA, Lagrangian relaxation | Scenario generation | ||
| Chen and Miller-Hooks (2012) | [12] | Barge, rail, road | x | x | Minimum service level | E: Benders decomposition, column generation | Scenario generation | |
| Miller-Hooks et al. (2012) | [14] | Unspecified | x | x | Not applicable | E: L-shaped method | Scenario generation | |
| Li et al. (2015) | [26] | Barge, rail, road | x | x | Penalty cost | E: Linear programming | Receding horizon approach | |
| Uddin and Huynh (2016) | [17] | Rail, road | x | No delivery and penalty cost | A: SAA, solver | Scenario generation | ||
| Sun et al. (2018) | [47] | Rail, road | x | x | Not allowed | E: Solver | Scenario generation | |
| Uddin and Huynh (2019) | [18] | Rail, road | x | No delivery and penalty cost | E: Solver | Scenario generation |
| Authors (Year) | Reference | Transport Modes | Transit Times | Demand | Capacity | Other | Solution Method 1 | Uncertainty Mitigation |
|---|---|---|---|---|---|---|---|---|
| Bock (2010) | [66] | Road | x | x | A: Metaheuristic | Rerouting | ||
| Burgholzer et al. (2013) | [67] | Barge, rail, road | x | x | A: Simulation | Rerouting | ||
| Escudero et al. (2013) | [68] | Unspecified | x | A: Genetic algorithm | Rerouting | |||
| van Riessen et al. (2015) | [69] | Barge, rail, road | departure times and cancellations | E: Linear programming | Rerouting | |||
| Sun and Schonfeld (2016) | [70] | Unspecified | x | A: Direct search | Holding decisions | |||
| Rivera and Mes (2017) | [71] | Barge, road | x | A: Approximate dynamic programming | Replanning | |||
| Rivera and Mes (2017) | [72] | Road | x | x | A: Matheuristic | Replanning | ||
| Qu et al. (2019) | [25] | Barge, rail, road | x | x | E: Solver | Replanning |
| Authors (Year) | Reference | Problem Type | Transport Modes | Transit Times | Demand | Other | Solution Method 1 | Uncertainty Mitigation |
|---|---|---|---|---|---|---|---|---|
| Lam et al. (2007) | [75] | empty container repositioning | Ship | x | A: Approximate dynamic programming | Scenario generation | ||
| Topaloglu (2007) | [74] | empty vehicle repositioning | Rail | x | x | A: Approximate dynamic programming | Replanning | |
| Di Francesco et al. (2013) | [76] | empty container repositioning | Ship | hub failures | E: Stochastic integer programming | Scenario generation | ||
| van Riessen et al. (2016) | [77] | container allocation | Barge, rail, road | x | A: Heuristic | Machine learning |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Delbart, T.; Molenbruch, Y.; Braekers, K.; Caris, A. Uncertainty in Intermodal and Synchromodal Transport: Review and Future Research Directions. Sustainability 2021, 13, 3980. https://doi.org/10.3390/su13073980
Delbart T, Molenbruch Y, Braekers K, Caris A. Uncertainty in Intermodal and Synchromodal Transport: Review and Future Research Directions. Sustainability. 2021; 13(7):3980. https://doi.org/10.3390/su13073980
Chicago/Turabian StyleDelbart, Thibault, Yves Molenbruch, Kris Braekers, and An Caris. 2021. "Uncertainty in Intermodal and Synchromodal Transport: Review and Future Research Directions" Sustainability 13, no. 7: 3980. https://doi.org/10.3390/su13073980
APA StyleDelbart, T., Molenbruch, Y., Braekers, K., & Caris, A. (2021). Uncertainty in Intermodal and Synchromodal Transport: Review and Future Research Directions. Sustainability, 13(7), 3980. https://doi.org/10.3390/su13073980






