A Mathematical Model of Value Co-Creation Dynamics Using a Leverage Mechanism
Abstract
:1. Introduction
2. Materials and Methods
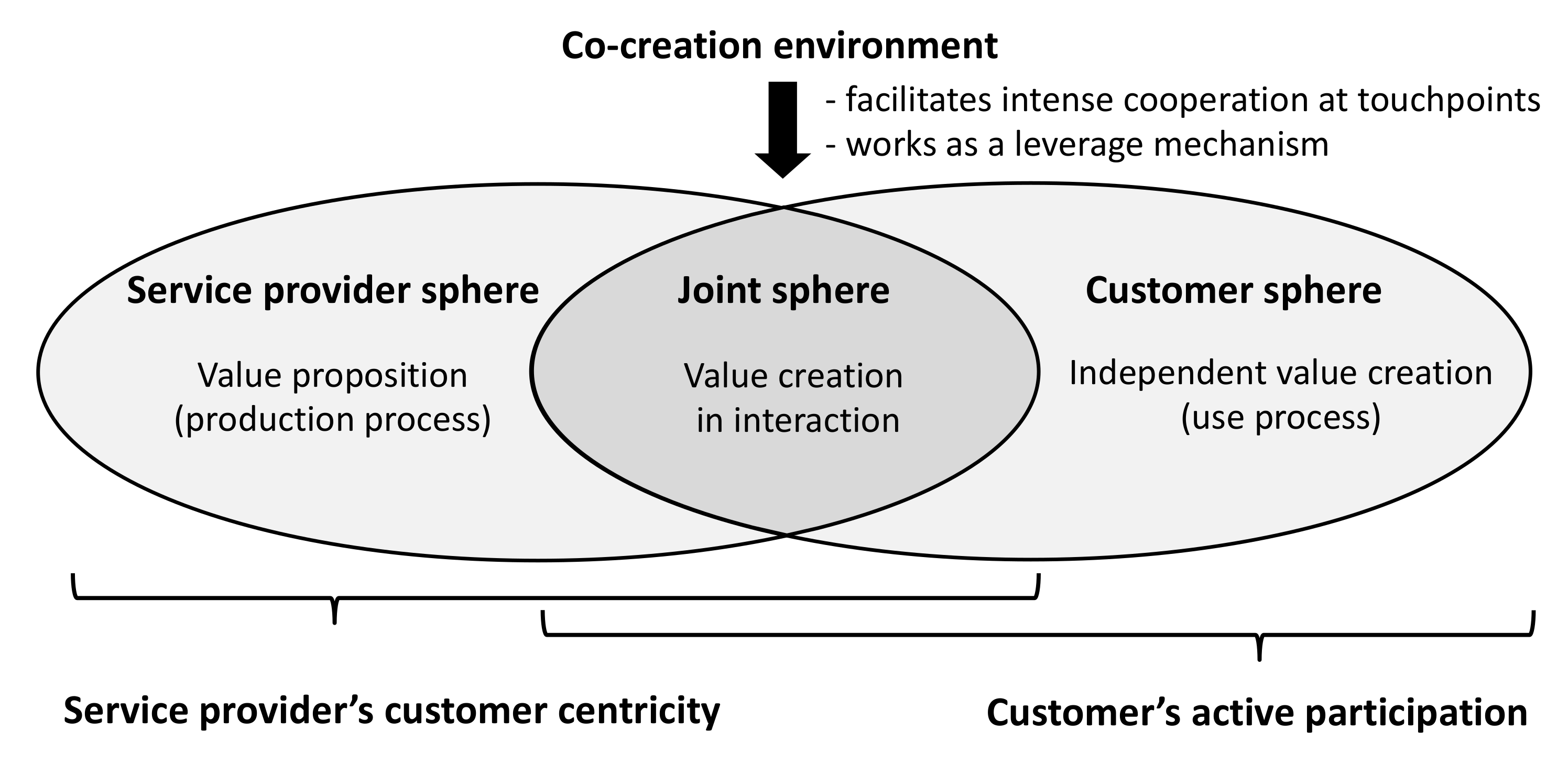
2.1. Basic Idea of the Seesaw Catapult System
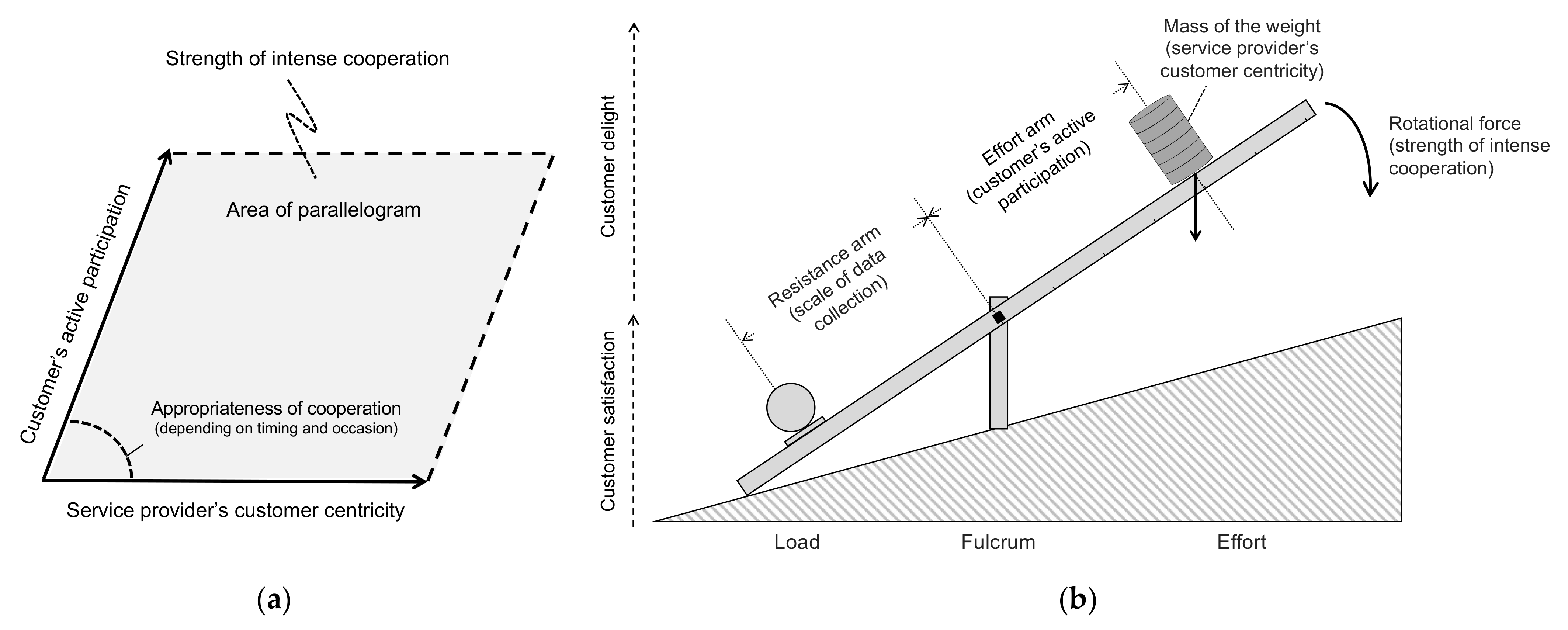
2.1.1. Service Provider’s Customer Centricity and Customer’s Active Participation
2.1.2. Rotational Force of the Lever System
2.1.3. Trade-Off Relationship and the Scale of Data Collection
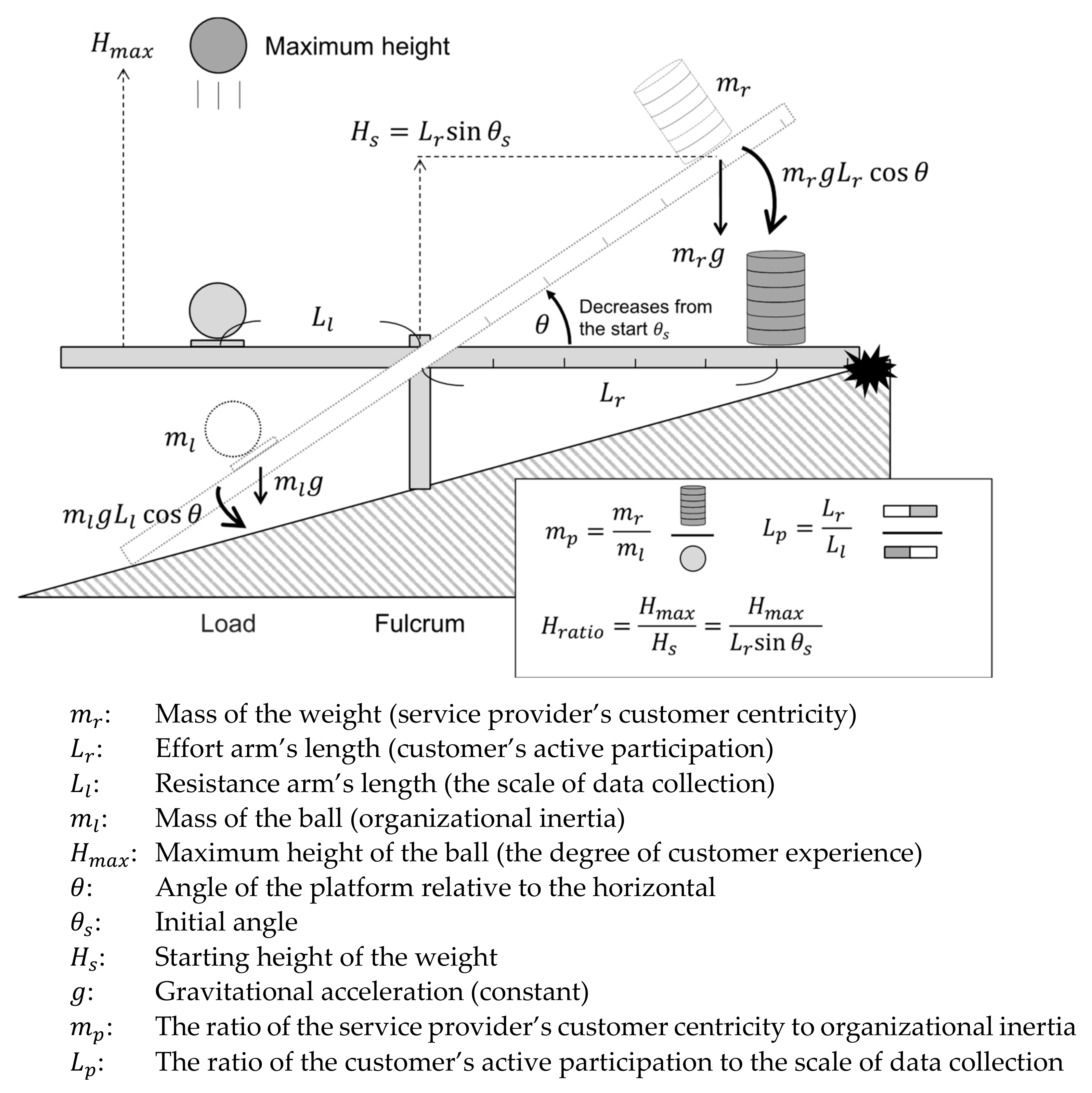
2.2. Dynamics of Rotational Motion
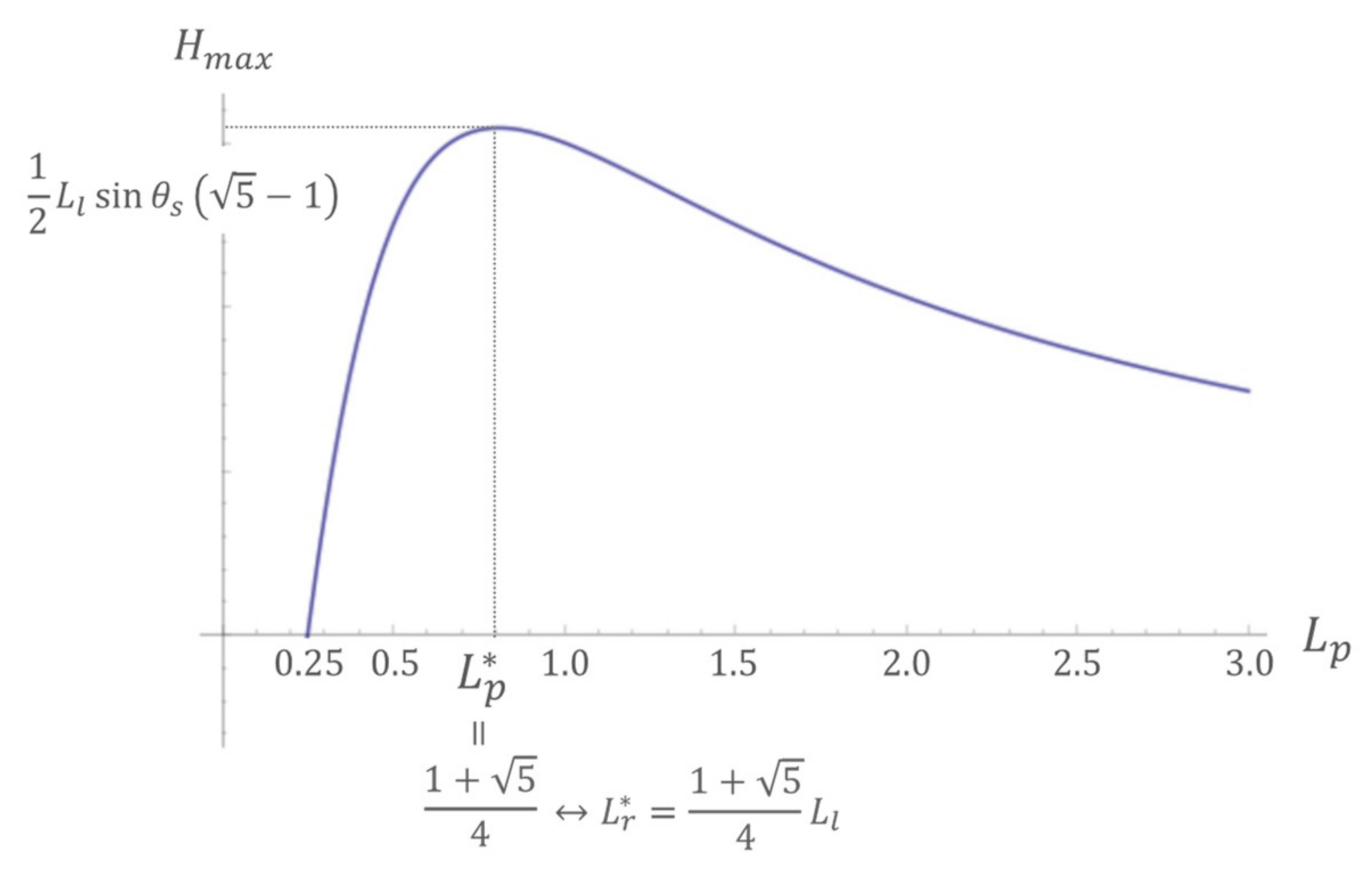
2.3. Ball’s Maximum Height: Objective Function
2.4. Design Variables and Delight Criterion
3. Results
3.1. Case 1: Changing the Design Variable “Customer’s Active Participation”
3.1.1. Maximizing the Ball’s Height
3.1.2. Additional Delight Condition
3.1.3. Process of Service Development Leading to Customer Delight
- An organization X had insufficient organizational capability, indicating reduced service provider’s customer centricity. Thus, we supposed that .
- The organization strived to enhance the customer experience (), even under the current condition of , by encouraging more appropriate active participation of the customer. However, this did not achieve delight, which satisfied Equation (10).
- The organization worked on improving customer centricity, and was realized. Accordingly, the most appropriate level of active participation was recalculated and pursued by encouraging and moderating its current level.
3.2. Case 2: Changing the Design Variable “the Scale of Data Collection”
3.2.1. Maximizing the Ball’s Height
3.2.2. Additional Delight Condition
3.2.3. Process of Service Development Leading to Customer Delight
- An organization X had insufficient organizational capability, resulting in reduced customer centricity of service providers. Thus, we suppose that holds.
- The organization strived to enhance the customer experience (), even under the current condition of , by encouraging a more appropriate scale of data collection. However, delight was not achieved, which satisfied Equation (17).
- The organization worked on improving customer centricity, and was realized. Accordingly, the most appropriate scale for data collection was updated and pursued by expanding and reducing its current level.As a result, the possibility of customer delight via co-creation increased.
3.3. Switchback Co-Creation Process for Customer Delight
4. Discussion
5. Implications
5.1. Theoretical Implications
5.2. Practical Implications
6. Limitation
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
| Level | Service Provider’s Customer Centricity | Customer’s Active Participation |
|---|---|---|
| 1 | Rewards | Acceptance |
| 2 | Regulations | Express needs clearly |
| 3 | Requests from customers | Use efficiently and effectively |
| 4 | Observation from the customer’s point of view | Provide feedback |
| 5 | Empathy for customers | Recommend to others |
| 6 | Social interest (or community feeling) | Feel psychological ownership [43] |
References
- Anderson, L.; Ostrom, A.L. Transformative Service Research: Advancing Our Knowledge About Service and Well-Being. J. Serv. Res. 2015, 18, 243–249. [Google Scholar] [CrossRef] [Green Version]
- Barnes, D.C.; Mesmer-Magnus, J.; Scribner, L.L.; Krallman, A.; Guidice, R.M. Customer Delight during a Crisis: Understanding Delight through the Lens of Transformative Service Research. J. Serv. Manag. 2021, 32, 129–141. [Google Scholar] [CrossRef]
- Vargo, S.L.; Maglio, P.P.; Akaka, M.A. On Value and Value Co-Creation: A Service Systems and Service Logic Perspective. Eur. Manag. J. 2008, 26, 145–152. [Google Scholar] [CrossRef]
- Barile, S.; Pels, J.; Polese, F.; Saviano, M. An Introduction to the Viable Systems Approach and Its Contribution to Marketing. J. Bus. Mark. Manag. 2012, 5, 54–78. [Google Scholar]
- Meynhardt, T.; Chandler, J.D.; Strathoff, P. Systemic Principles of Value Co-Creation: Synergetics of Value and Service Ecosystems. J. Bus. Res. 2016, 69, 2981–2989. [Google Scholar] [CrossRef]
- ISO 23592:2021; Service Excellence—Principles and Model. International Standardization Organization: Geneva, Switzerland, 2021.
- Finn, A. Customer Delight: Distinct Construct or Zone of Nonlinear Response to Customer Satisfaction? J. Serv. Res. 2012, 15, 99–110. [Google Scholar] [CrossRef]
- Grönroos, C.; Voima, P. Critical Service Logic: Making Sense of Value Creation and Co-Creation. J. Acad. Mark. Sci. 2013, 41, 133–150. [Google Scholar] [CrossRef]
- Wang, X.; Wong, Y.D.; Teo, C.C.; Yuen, K.F. A Critical Review on Value Co-Creation: Towards a Contingency Framework and Research Agenda. J. Serv. Theory Pract. 2019, 29, 165–188. [Google Scholar] [CrossRef]
- Qiu, R.G. Computational Thinking of Service Systems: Dynamics and Adaptiveness Modeling. Serv. Sci. 2009, 1, 42–55. [Google Scholar] [CrossRef] [Green Version]
- Grissemann, U.S.; Stokburger-Sauer, N.E. Customer Co-Creation of Travel Services: The Role of Company Support and Customer Satisfaction with the Co-Creation Performance. Tour. Manag. 2012, 33, 1483–1492. [Google Scholar] [CrossRef]
- Ho, B.Q.; Murae, Y.; Hara, T.; Okada, Y. Consumer Experience as Suppliers on Value Co-Creation Behavior. J. Serv. 2019, 4, 1–7. [Google Scholar]
- Nadeem, W.; Juntunen, M.; Shirazi, F.; Hajli, N. Consumers’ Value Co-Creation in Sharing Economy: The Role of Social Support, Consumers’ Ethical Perceptions and Relationship Quality. Technol. Forecast. Soc. Chang. 2020, 151, 119786. [Google Scholar] [CrossRef]
- Cheung, M.L.; Pires, G.D.; Rosenberger, P.J.; Leung, W.K.S.; Ting, H. Investigating the Role of Social Media Marketing on Value Co-Creation and Engagement: An Empirical Study in China and Hong Kong. Australas. Mark. J. 2020, 29, 118–131. [Google Scholar] [CrossRef]
- Yang, J.; Xia, J.; Sun, D.; Wei, Y.; Qiao, Z.; Wang, E.; Zhou, Y.; Shi, S.; Wang, S. A Multi-Agent Model-Based Evolutionary Model of Port Service Value Network and Decision Preferences. Sustainability 2022, 14, 3565. [Google Scholar]
- Trinh, T.H.; Liem, N.T.; Kachitvichyanukul, V. A Game Theory Approach for Value Co-Creation Systems. Prod. Manuf. Res. 2014, 2, 253–265. [Google Scholar] [CrossRef] [Green Version]
- Ueda, K.; Takenaka, T.; Fujita, K. Toward Value Co-Creation in Manufacturing and Servicing. CIRP J. Manuf. Sci. Technol. 2008, 1, 53–58. [Google Scholar] [CrossRef]
- Kaihara, T.; Nishino, N.; Ueda, K.; Tseng, M.; Váncza, J.; Schönsleben, P.; Teti, R.; Takenaka, T. Value Creation in Production: Reconsideration from Interdisciplinary Approaches. CIRP Ann. 2018, 67, 791–813. [Google Scholar] [CrossRef] [Green Version]
- Durugbo, C.; Pawar, K. A Unified Model of the Co-Creation Process. Expert Syst. Appl. 2014, 41, 4373–4387. [Google Scholar] [CrossRef]
- Hara, T.; Sato, K.; Arai, T. Modeling the Transition to a Provider–Customer Relationship in Servitization for Expansion of Customer Activity Cycles. CIRP Ann. 2016, 65, 173–176. [Google Scholar] [CrossRef]
- Ho, B.Q.; Shirahada, K. Actor Transformation in Service: A Process Model for Vulnerable Consumers. J. Serv. Theory Pract. 2021, 31, 534–562. [Google Scholar] [CrossRef]
- ISO/TS 24082:2021; Service Excellence—Designing Excellent Service to Achieve Outstanding Customer Experiences. International Standardization Organization: Geneva, Switzerland, 2021.
- Verleye, K. The Co-Creation Experience from the Customer Perspective: Its Measurement and Determinants. J. Serv. Manag. 2015, 26, 321–342. [Google Scholar] [CrossRef] [Green Version]
- Hara, T.; Tsuru, S.; Yasui, S. Models for Designing Excellent Service through Co-Creation Environment. Commun. Comput. Inf. Sci. 2020, 1189 CCIS, 73–83. [Google Scholar]
- Guliyev, F.A.O. Metaphor as an Object of the Synergy Paradigm Study. Linguist. Cult. Rev. 2021, 5, 1–10. [Google Scholar] [CrossRef]
- Puerari, E.; de Koning, J.I.J.C.; von Wirth, T.; Karré, P.M.; Mulder, I.J.; Loorbach, D.A. Co-Creation Dynamics in Urban Living Labs. Sustainability 2018, 10, 1893. [Google Scholar] [CrossRef] [Green Version]
- Chen, T.; Ou Yang, S.; Leo, C. The Beginning of Value Co-Creation: Understanding Dynamics, Efforts and Betterment. J. Serv. Theory Pract. 2017, 27, 1145–1166. [Google Scholar] [CrossRef]
- Siano, D. Trebuchet Mechanics. Available online: http://faculty.weber.edu/kharward/MET1000/trebmath35.pdf (accessed on 2 April 2022).
- Arnold, A.S.; Richards, C.T.; Ros, I.G.; Biewener, A.A. There Is Always a Trade-off between Speed and Force in a Lever System: Comment on McHenry. Biol. Lett. 2011, 7, 878–879. [Google Scholar] [CrossRef] [Green Version]
- Tural, G. Amazing Physics: Learning about Work, Energy and Projectile Motion in a Historical Context. Phys. Educ. 2013, 48, 336. [Google Scholar] [CrossRef]
- Johnston, R. The Zone of Tolerance Exploring the Relationship between Service Transactions and Satisfaction with the Overall Service. Int. J. Serv. Ind. Manag. 1995, 6, 46–61. [Google Scholar] [CrossRef] [Green Version]
- Zeithaml, V.A.; Berry, L.L.; Parasuraman, A. The Nature and Determinants of Customer Expectations of Service. J. Acad. Mark. Sci. 1993, 21, 1–12. [Google Scholar] [CrossRef] [Green Version]
- Gilbert, C.G. Unbundling the Structure of Inertia: Resource versus Routine Rigidity. Acad. Manag. J. 2005, 48, 741–763. [Google Scholar] [CrossRef] [Green Version]
- Gummesson, E. Service Research Methodology: From Case Study Research to Case Theory. Rev. Ibero-Am. Estratégia 2014, 13, 8–17. [Google Scholar] [CrossRef]
- Thomassen, J.-P.; de Haan, E. How to Create a Service Excellence Organization. 2016. Available online: https://www.salesgids.com/wp-content/uploads/2017/02/Whitepaper_Service-Excellence.pdf (accessed on 2 April 2022).
- Sanders, E.B.-N.; Stappers, P.J. Co-Creation and the New Landscapes of Design. CoDesign 2008, 4, 5–18. [Google Scholar] [CrossRef] [Green Version]
- Botero, A.; Hyysalo, S. Ageing Together: Steps towards Evolutionary Co-Design in Everyday Practices. CoDesign 2013, 9, 37–54. [Google Scholar] [CrossRef]
- Arai, T.; Shimomura, Y. Proposal of Service CAD System—A Tool for Service Engineering. CIRP Ann. 2004, 53, 397–400. [Google Scholar] [CrossRef]
- Hara, T.; Arai, T.; Shimomura, Y. A CAD System for Service Innovation: Integrated Representation of Function, Service Activity, and Product Behaviour. J. Eng. Des. 2009, 20, 367–388. [Google Scholar] [CrossRef]
- Sakao, T.; Hara, T.; Fukushima, R. Using Product/Service-System Family Design for Efficient Customization with Lean Principles: Model, Method, and Tool. Sustainability 2020, 12, 5779. [Google Scholar] [CrossRef]
- ISO/PRF TS 23686:2022; Service Excellence—Measuring Service Excellence Performance. International Standardization Organization: Geneva, Switzerland, 2022.
- Van Ackere, A.; Larsen, E.R.; Morecroft, J.D.W. Systems Thinking and Business Process Redesign: An Application to the Beer Game. Eur. Manag. J. 1993, 11, 412–423. [Google Scholar] [CrossRef]
- Chen, T.; Dodds, S.; Finsterwalder, J.; Witell, L.; Cheung, L.; Falter, M.; Garry, T.; Snyder, H.; McColl-Kennedy, J.R. Dynamics of Wellbeing Co-Creation: A Psychological Ownership Perspective. J. Serv. Manag. 2020, 32, 383–406. [Google Scholar] [CrossRef]
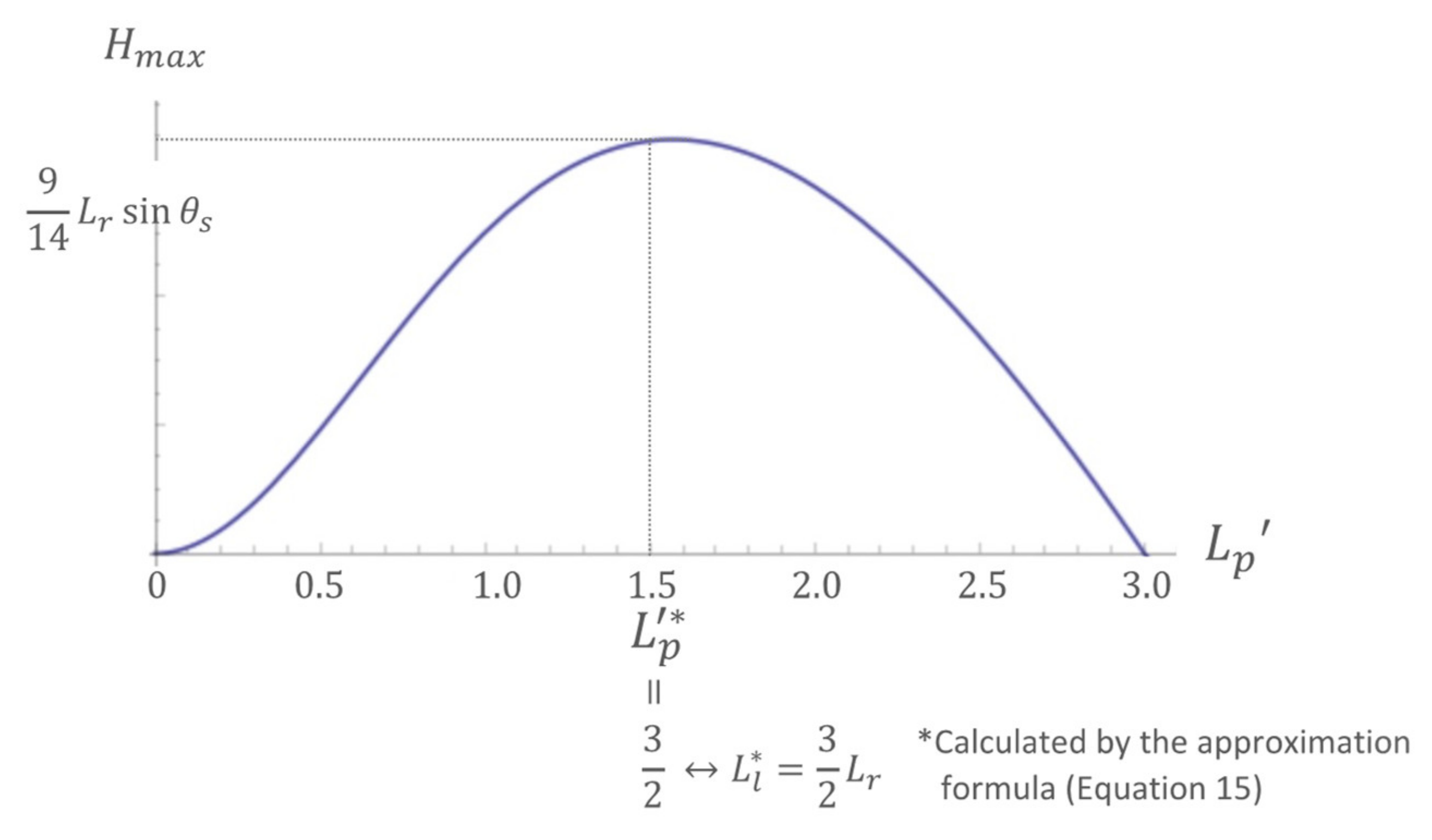

| Case 1: Designing the Customer’s Active Participation | Case 2: Designing the Scale of Data Collection | |
|---|---|---|
| Maximum of | ||
| Optimal design variable | ||
| Additional condition to exceed the delight criterion | 5 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hara, T.; Tsuru, S.; Yasui, S. A Mathematical Model of Value Co-Creation Dynamics Using a Leverage Mechanism. Sustainability 2022, 14, 6531. https://doi.org/10.3390/su14116531
Hara T, Tsuru S, Yasui S. A Mathematical Model of Value Co-Creation Dynamics Using a Leverage Mechanism. Sustainability. 2022; 14(11):6531. https://doi.org/10.3390/su14116531
Chicago/Turabian StyleHara, Tatsunori, Satoko Tsuru, and Seiichi Yasui. 2022. "A Mathematical Model of Value Co-Creation Dynamics Using a Leverage Mechanism" Sustainability 14, no. 11: 6531. https://doi.org/10.3390/su14116531
APA StyleHara, T., Tsuru, S., & Yasui, S. (2022). A Mathematical Model of Value Co-Creation Dynamics Using a Leverage Mechanism. Sustainability, 14(11), 6531. https://doi.org/10.3390/su14116531







