Pricing Problems in the Pharmaceutical Supply Chain with Mixed Channel: A Power Perspective
Abstract
:1. Introduction
- (1)
- What effects do power structures have on pricing, performance and social welfare in the pharmaceutical supply chain where the pharmaceutical manufacturer is regulated?
- (2)
- How does the restricted wholesale price cap affect the financial performance and social welfare in three different power structures?
2. Literature
- Price cap regulation in pharmaceutical supply chain
- Power structure in the supply chain
- Social welfare in the supply chain
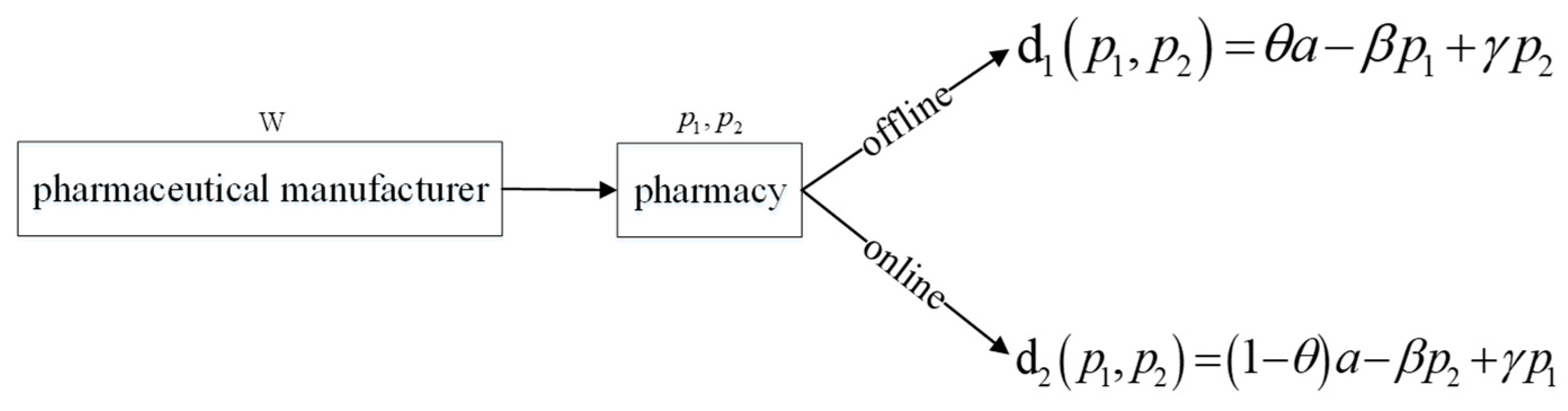
3. Model Descriptions
4. Mathematical Models and Equilibrium Analysis
4.1. Base Models
4.1.1. Pharmaceutical Manufacturer Stackelberg Model
4.1.2. Pharmacy Stackelberg Model
4.1.3. Vertical Nash Model
4.2. Price Cap Regulation Models
- (1)
- If the wholesale price cap is higher than the optimal wholesale price in the MS model (), the price cap regulation has no effect on the pharmaceutical supply chain under three power structures.
- (2)
- If the wholesale price cap is higher than the optimal wholesale price in the VN model and lower than that in the MS model (), the price cap regulation only has effects on the pharmaceutical supply chain when the pharmaceutical manufacturer is dominant in the market.
- (3)
- If the wholesale price cap is higher than the optimal wholesale price in the PS model and lower than that in the VN model (), the price cap regulation affects the pharmaceutical supply chain when the pharmaceutical manufacturer is dominant in the market or the pharmaceutical manufacturer and pharmacy are in a balanced power structure.
- (4)
- If the wholesale price cap is lower than the optimal wholesale price in the PS model (), the pharmaceutical supply chain under three different power structures will be affected.
5. Effects of the Power Structures
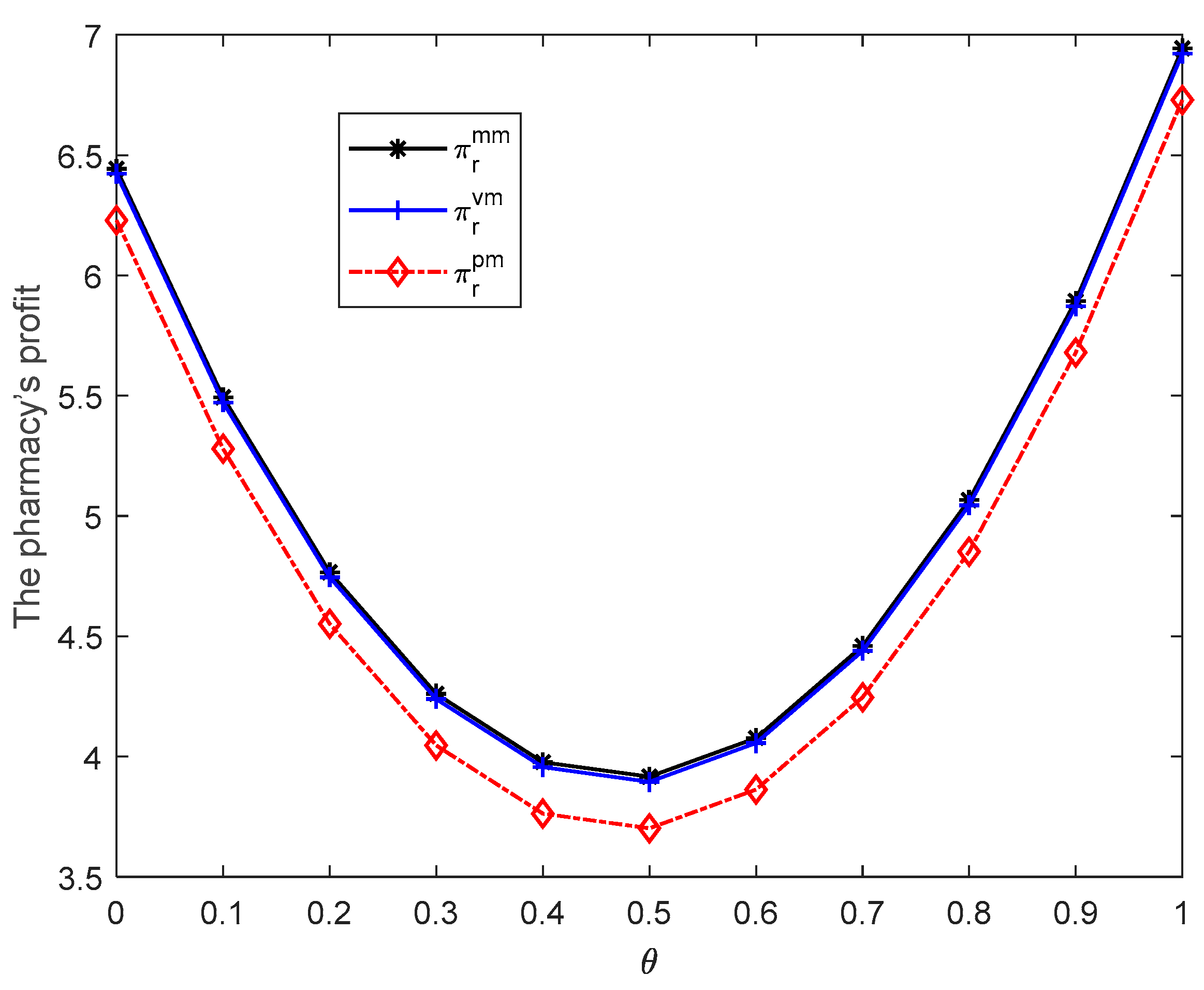
5.1. Effects of Power Structures on the Pricing and Performance
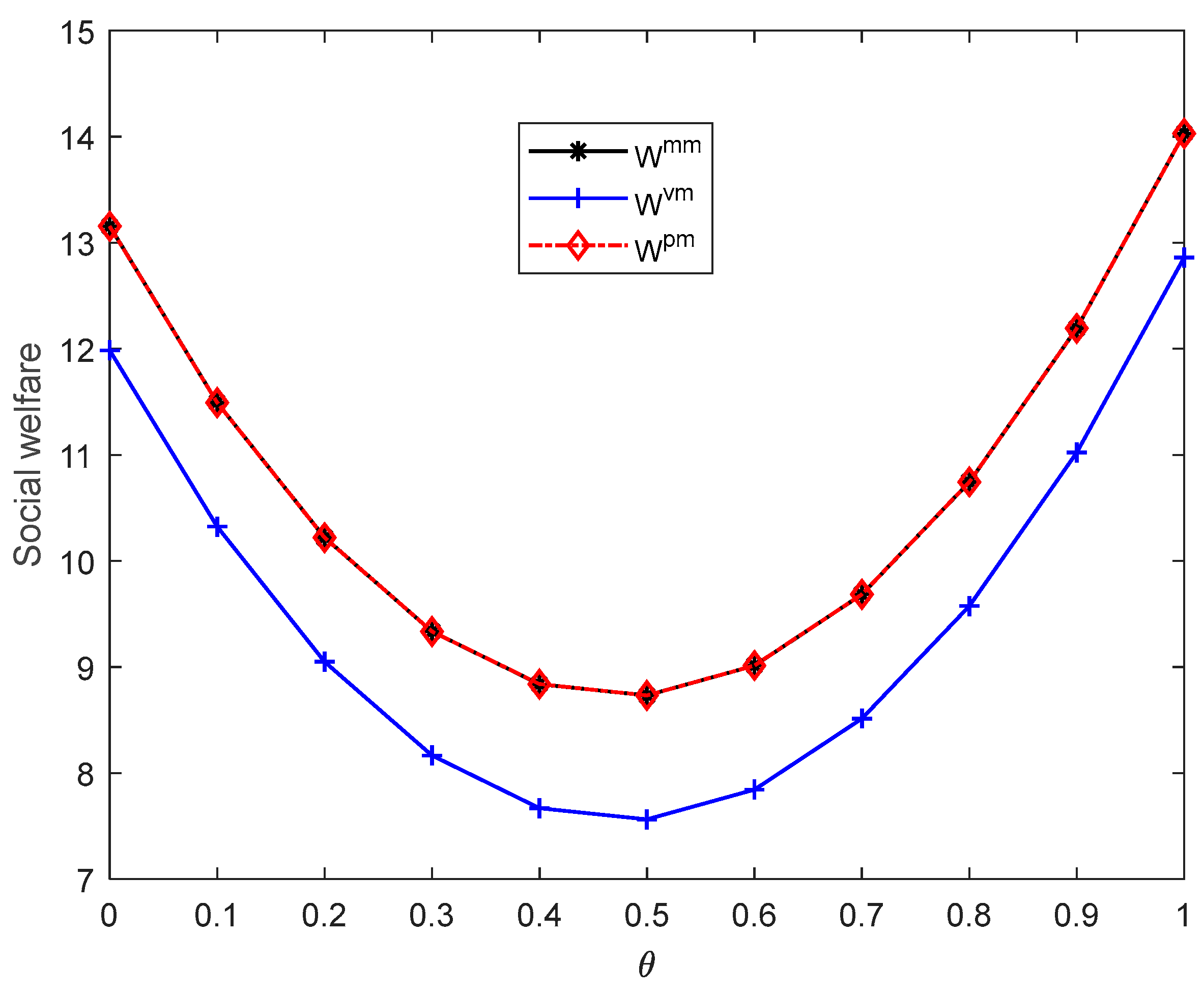
5.2. Effects of Power Structure on Social Welfare
6. Effects of the Price Cap Regulation
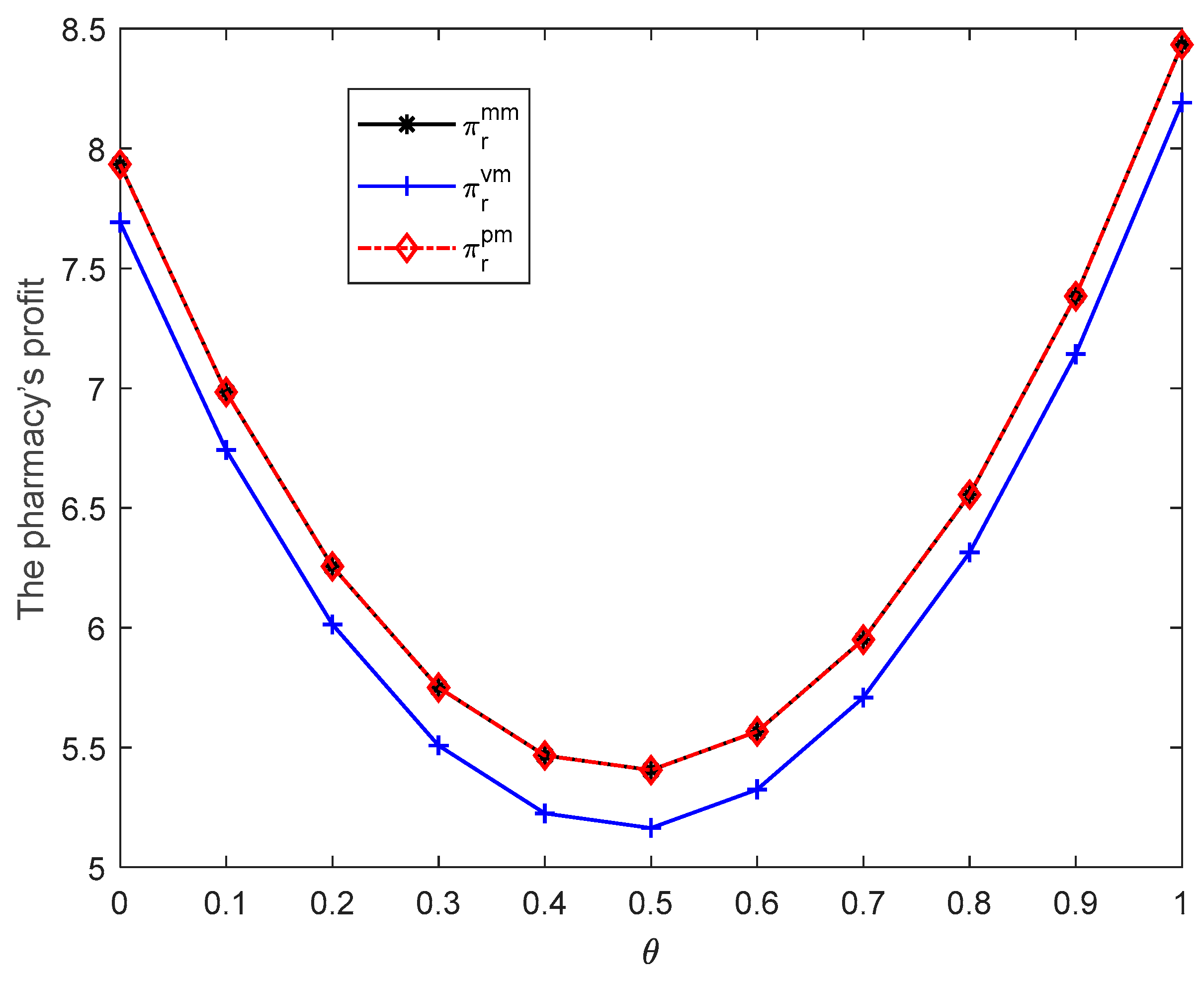
6.1. Effects of Price Cap Regulation on Performance
6.2. Effects of Price Cap Regulation on Social Welfare
7. Conclusions and Future Research
- (1)
- When the price cap is in different ranges, the influence of the power structure on the economic and social benefits of the pharmaceutical supply chain is different. When the wholesale price cap is higher than the optimal wholesale price in the MS model, the price cap regulation will not work in the supply chain in three different power structures. The pharmaceutical manufacturer and pharmacy can gain more profits when they dominate the market, whereas the balanced power relationship between supply chain members can improve the total profit and social welfare. When the wholesale price cap is lower than the optimal price cap, the pharmaceutical manufacturer will still gain higher profits when it has more market power over other supply chain members. However, for pharmacies, market power cannot ensure that they can make more profits with the influence of the restricted wholesale price cap. Therefore, leading pharmacies may resist the price cap regulation; as a result, this increases the risk of policy failure. The government may consider subsidizing dominated pharmacies when implementing price cap regulation. When the price cap is very low, an interesting discovery is presented. Price cap regulation might harm financial performance and social welfare when pharmaceutical firms are in a balanced power structure. Thus, when the wholesale price cap is lower than a certain threshold, price cap regulation might be detrimental to creating a fair market environment for enterprises.
- (2)
- We find the wholesale price cap is an important parameter that affects financial performance and social welfare of the pharmaceutical supply chain. Regardless of the power structures, the pharmaceutical manufacturer’s profit is positively correlated with restricted wholesale price. The pharmacy’s profit is negatively correlated with the restricted wholesale price. In addition, social welfare in pharmaceutical manufacturer Stackelberg and pharmacy Stackelberg markets is negatively correlated with the restricted wholesale price, and social welfare in the balanced market is dependent on the restricted wholesale price.
- (3)
- Overall, this paper provides insights into the pricing decisions of pharmaceutical companies under different power structures. In addition, it also provides a decision-making basis for the government to make price limit policies in different power markets.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
Appendix A.1. Proof of the Table 1
Appendix A.1.1. MS Model
Appendix A.1.2. PS Model
Appendix A.1.3. VN Model
Appendix A.2. Proof of Table 2
Appendix A.2.1. MM Model
- (i)
- If , the manufacturer price cap regulation has no effect on the medical supply chain, so the optimal prices are , and .
- (ii)
- If , the price cap regulation has effects on the pharmaceutical manufacturer and pharmacy, so the optimal wholesale price is , , .
Appendix A.2.2. PM Model
- (i)
- If , then the manufacturer price cap regulation has no effect on the pharmaceutical manufacturer and pharmacy, so the optimal prices are , and .
- (ii)
- If , then the manufacturer price cap regulation has effects on the pharmaceutical manufacturer and pharmacy, so the optimal prices are , Replace in Formula (5), we need solve . , , .
Appendix A.2.3. VM Model
- (i)
- If , then the manufacturer price cap regulation has no effect on the pharmaceutical manufacturer and pharmacy, so the optimal prices are , and .
- (ii)
- If , then the manufacturer price cap regulation has effects on the pharmaceutical manufacturer, so the optimal prices are , and .
Appendix A.3. Proof of Proposition 1
Appendix A.4. Proof of Proposition 2
Appendix A.5. Proof of Proposition 3
Appendix A.6. Proof of Proposition 4
Appendix A.7. Proof of Proposition 5
Appendix A.8. Proof of Proposition 6
Appendix A.9. Proof of Proposition 7
References
- Abbott, T.A., III. Price regulation in the pharmaceutical industry: Prescription or placebo? J. Health Econ. 1995, 14, 551–565. [Google Scholar] [CrossRef]
- Brekke, K.R.; Grasdal, A.L.; Holmås, T.H. Regulation and pricing of pharmaceuticals: Reference pricing or price cap regulation? Eur. Econ. Rev. 2006, 53, 170–185. [Google Scholar] [CrossRef]
- Cai, G.; Zhang, Z.G.; Zhang, M. Game theoretical perspectives on dual-channel supply chain competition with price discounts and pricing schemes. Int. J. Prod. Econ. 2009, 117, 80–96. [Google Scholar] [CrossRef]
- Chen, J.; Zhang, H.; Sun, Y. Implementing coordination contracts in a manufacturer Stackelberg dual-channel supply chain. Omega 2012, 40, 571–583. [Google Scholar] [CrossRef]
- Chen, X.; Li, L.; Zhou, M. Manufacturer’s pricing strategy for supply chain with warranty period-dependent demand. Omega 2012, 40, 807–816. [Google Scholar] [CrossRef]
- Chen, X.; Li, S.S.; Wang, X. Evaluating the effects of quality regulations on the pharmaceutical supply chain. Int. J. Prod. Econ. 2020, 230, 107770. [Google Scholar] [CrossRef]
- Chen, X.; Luo, Z.; Wang, X.J. Impact of efficiency, investment, and competition on low carbon manufacturing. J. Clean. Prod. 2017, 143, 388–400. [Google Scholar] [CrossRef] [Green Version]
- Chen, X.; Wang, X. Free or bundled: Channel selection decisions under different power structures. Omega 2015, 53, 11–20. [Google Scholar] [CrossRef]
- Chen, X.; Wang, X.; Chan, H.K. Manufacturer and retailer coordination for environmental and economic competitiveness: A power perspective. Transp. Res. Part E Logist. Transp. Rev. 2017, 97, 268–281. [Google Scholar] [CrossRef] [Green Version]
- Chen, X.; Wang, X.J.; Gong, K. The effect of bidimensional power structure on supply chain decisions and performance. IEEE Trans. Syst. Man Cybern. Syst. 2020, 50, 1095–1110. [Google Scholar] [CrossRef] [Green Version]
- Chen, X.; Wang, X.J.; Jiang, X.K. The impact of power structure on the retail service supply chain with an O2O mixed channel. J. Oper. Res. Soc. 2016, 67, 294–301. [Google Scholar] [CrossRef]
- Chen, X.; Yang, H.; Wang, X.J. Effects of price cap regulation on the pharmaceutical supply chain. J. Bus. Res. 2019, 97, 281–290. [Google Scholar] [CrossRef]
- Choi, S.C. Price Competition in a Channel Structure with a Common Retailer. Mark. Sci. 1991, 10, 271–296. [Google Scholar] [CrossRef]
- Choi, S.C. Price competition in a duopoly common retailer channel. J. Retail. 1996, 72, 117–134. [Google Scholar] [CrossRef]
- Cowan, S. Welfare Consequences of Tight Price-Cap Regulation. Bull. Econ. Res. 1998, 50, 105–116. [Google Scholar] [CrossRef]
- Danzon, P.; Towse, A.; Mestre-Ferrandiz, J. Value-Based Differential Pricing: Efficient Prices for Drugs in a Global Context. Health Econ. 2015, 24, 294–301. [Google Scholar] [CrossRef] [Green Version]
- Danzon, P.M.; Chao, L.W. Does regulation drive out competition in pharmaceutical markets? J. Law Econ. 2000, 43, 311–358. [Google Scholar] [CrossRef]
- Danzon, P.M.; Mulcahy, A.W.; Towse, A.K. Pharmaceutical pricing in emerging markets: Effects of income, competition, and procurement. Health Econ. 2015, 24, 238–252. [Google Scholar] [CrossRef] [Green Version]
- Ekelund, M.; Persson, B. Pharmaceutical pricing in a regulated market. Rev. Econ. Stat. 2003, 85, 298–306. [Google Scholar] [CrossRef]
- Han, S.; Liang, H.; Su, W.; Xue, Y.; Shi, L. Can price controls reduce pharmaceutical expenses? A case study of antibacterial expenditures in 12 Chinese hospitals from 1996 to 2005. Int. J. Health Serv. 2013, 43, 91–103. [Google Scholar] [CrossRef]
- Hou, Y.H.; Wang, F.; Chen, Z.T.; Shi, V. Coordination of a Dual-Channel Pharmaceutical Supply Chain Based on the Susceptible-Infected-Susceptible Epidemic Model. Int. J. Environ. Res. Public Health 2020, 17, 3292. [Google Scholar] [CrossRef] [PubMed]
- Jin, Y.N.; Wang, S.J.; Hu, Q.Y. Contract type and decision right of sales promotion in supply chain management with a capital constrained retailer. Eur. J. Oper. Res. 2015, 240, 415–424. [Google Scholar] [CrossRef]
- Li, S.; Dan, B.; Li, H.; Zhang, H. Power Structure for Pharmaceutical Dual-channel Supply Chain under Price Cap Policy and Public Welfare. Manag. Rev. 2019, 31, 266–277. [Google Scholar] [CrossRef]
- Li, S.Y.; Dan, B.; Zhou, M.S.; Wang, D. Pricing and coordination strategies in pharmaceutical dual-channel supply chain under the influence of price cap policy and public welfare. J. Ind. Eng. Eng. Manag. 2019, 33, 196–204. [Google Scholar] [CrossRef]
- Luiza, V.L.; Chaves, L.A.; Silva, R.M.; Emmerick, I.C.M.; Chaves, G.C.; Araujo, S.C.F.; Moraes, E.L.; Oxman, A.D. Pharmaceutical policies: Effects of cap and co-payment on rational use of medicines. Cochrane Database Syst. Rev. 2015, 5, CD007017. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Luo, Z.; Chen, X.; Chen, J.; Wang, X. Optimal pricing policies for differentiated brands under different supply chain power structures. Eur. J. Oper. Res. 2016, 259, 437–451. [Google Scholar] [CrossRef] [Green Version]
- Mukhopadhyay, S.K.; Yao, D.Q.; Yue, X.H. Information sharing of value-adding retailer in a mixed channel hi-tech supply chain. J. Bus. Res. 2008, 61, 950–958. [Google Scholar] [CrossRef]
- Pan, K.W.; Lai, K.K.; Leung, S.C.H.; Xiao, D. Revenue-sharing versus wholesale price mechanisms under different channel power structures. Eur. J. Oper. Res. 2010, 203, 532–538. [Google Scholar] [CrossRef]
- Puig-Junoy, J. Impact of European Pharmaceutical Price Regulation on Generic Price Competition A Review. PharmacoEconomics 2010, 28, 649–663. [Google Scholar] [CrossRef]
- Sana, S.S.; Chedid, J.A.; Navarro, K.S. A three layer supply chain model with multiple suppliers, manufacturers and retailers for multiple items. Appl. Math. Comput. 2014, 229, 139–150. [Google Scholar] [CrossRef]
- Shi, R.X.; Zhang, J.; Ru, J. Impacts of power structure on supply chains with uncertain demand. Prod. Oper. Manag. 2013, 22, 1232–1249. [Google Scholar] [CrossRef]
- Vogler, S. The impact of pharmaceutical pricing and reimbursement policies on generics uptake: Implementation of policy options on generics in 29 European countries—An overview. Gabi J.-Generics Biosimilars Initiat. J. 2012, 1, 93–100. [Google Scholar] [CrossRef] [Green Version]
- Wang, C.; Chen, X. Option pricing and coordination in the fresh produce supply chain with portfolio contracts. Ann. Oper. Res. 2017, 248, 471–491. [Google Scholar] [CrossRef]
- Yang, C.J.; Wu, L.N.; Cai, W.F.; Zhu, W.W.; Shen, Q.; Li, Z.J.; Fang, Y. Current Situation, Determinants, and Solutions to Drug Shortages in Shaanxi Province, China: A Qualitative Study. PLoS ONE 2016, 11, e0165783. [Google Scholar] [CrossRef] [PubMed]
- Zhang, W.; Guh, D.; Sun, H.; Marra, C.A.; Lynd, L.D.; Anis, A.H. The Impact of Price-cap Regulations on Exit by Generic Pharmaceutical Firms. Med. Care 2016, 54, 884–890. [Google Scholar] [CrossRef] [PubMed]
- Zheng, Y.; Liu, L.; Shi, V.; Liu, B.; Huang, W.X. Price Cap Models in Pharmaceutical Online-to-Offline Supply Chains. Complexity 2020, 2020, 7471948. [Google Scholar] [CrossRef]
- Baron, D.P.; Roger, B.M. Regulating a Monopolist with Unknown Costs. Econometrica 1982, 50, 911–930. [Google Scholar] [CrossRef] [Green Version]
- Xue, K.; Sun, G. Impacts of Supply Chain Competition on Firms’ Carbon Emission Reduction and Social Welfare under Cap-and-Trade Regulation. Int. J. Environ. Res. Public Health 2022, 19, 3226. [Google Scholar] [CrossRef]
- Chen, S.; Su, J.; Wu, Y.; Zhou, F. Optimal production and subsidy rate considering dynamic consumer green perception under different government subsidy orientations. Comput. Ind. Eng. 2022, 168, 108073. [Google Scholar] [CrossRef]
- Wang, M.; Liu, K.; Choi, T.-M.; Yue, X. Effects of Carbon Emission Taxes on Transportation Mode Selections and Social Welfare. IEEE Trans. Syst. Man Cybern. Syst. 2015, 45, 1413–1423. [Google Scholar] [CrossRef]
- Wang, W.; Zhang, S.; Zhang, L.; Liu, Q. Government Subsidy Policies and Corporate Social Responsibility. IEEE Access 2020, 8, 112814–112826. [Google Scholar] [CrossRef]



| MS Model | PS Model | VN Model | |
|---|---|---|---|
| Models | ||||
|---|---|---|---|---|
| MM model | ||||
| PM model | ||||
| VM model | ||||
| Models | |||
|---|---|---|---|
| MM model | |||
| PM model | |||
| VM model | |||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, X.; Liu, L.; Zheng, Y.; Yang, X.; Sun, S. Pricing Problems in the Pharmaceutical Supply Chain with Mixed Channel: A Power Perspective. Sustainability 2022, 14, 7420. https://doi.org/10.3390/su14127420
Yang X, Liu L, Zheng Y, Yang X, Sun S. Pricing Problems in the Pharmaceutical Supply Chain with Mixed Channel: A Power Perspective. Sustainability. 2022; 14(12):7420. https://doi.org/10.3390/su14127420
Chicago/Turabian StyleYang, Xiaojie, Li Liu, Yi Zheng, Xue Yang, and Shanlin Sun. 2022. "Pricing Problems in the Pharmaceutical Supply Chain with Mixed Channel: A Power Perspective" Sustainability 14, no. 12: 7420. https://doi.org/10.3390/su14127420
APA StyleYang, X., Liu, L., Zheng, Y., Yang, X., & Sun, S. (2022). Pricing Problems in the Pharmaceutical Supply Chain with Mixed Channel: A Power Perspective. Sustainability, 14(12), 7420. https://doi.org/10.3390/su14127420





