Inclined Layer Method-Based Theoretical Calculation of Active Earth Pressure of a Finite-Width Soil for a Rotating-Base Retaining Wall
Abstract
:1. Introduction
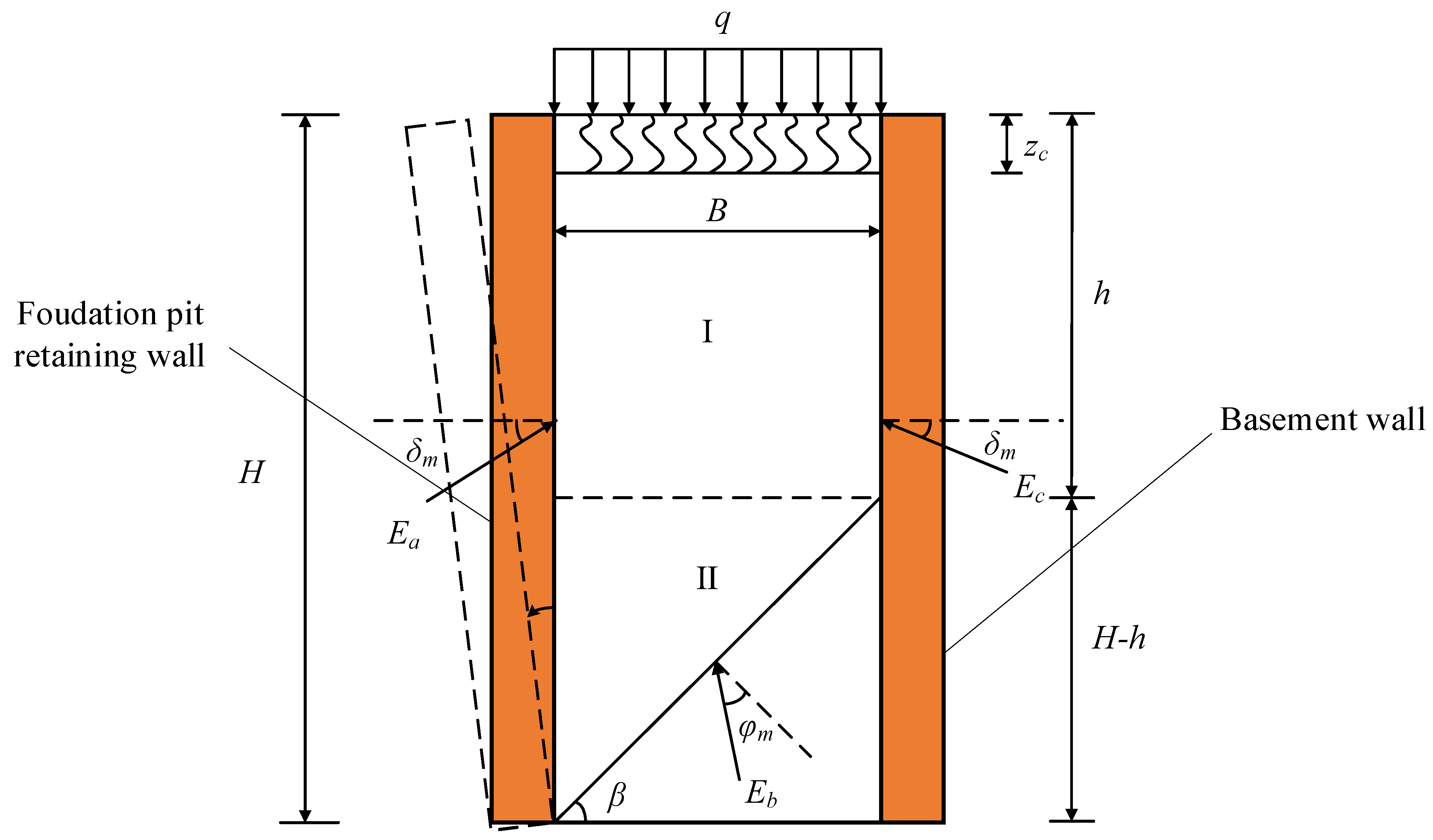
2. Basic Hypothesis
- (1)
- The clay behind the wall is of the same material, the soil’s cohesion is c, and the friction angle of the soil is φ. In addition, the developed value of φ is φm.
- (2)
- The basement wall does not move, and the foundation-pit retaining wall rotates outward around the wall base.
- (3)
- The Mohr–Coulomb criterion governs the shear strength of the soil with a finite width.
- (4)
- The roughness of the wall back is taken into account, and the wall–soil friction angles of both the foundation-pit retaining wall and the basement exterior wall are δ. Therefore, the effective value of δ is δm.
- (5)
- The wall–soil cohesion of the retaining-wall foundation pit and that of the basement wall is cw and cd, respectively.
- (6)
- The soil on the ground surface has a uniform load q (unit: kN/m).
- (7)
- A straight slip surface is assumed across the wall heel.
3. Determination of Friction Angle and Soil’s Cohesion in the Nonlimit State
3.1. Qualitative Analysis of Nonlimit State
3.2. Calculation of Shear Strength Parameters in Nonlimit State
3.2.1. Calculation of Friction Angle Parameters
3.2.2. Calculation of Cohesion Parameters
4. Derivation of Active Earth Pressure of a Finite-Width Soil
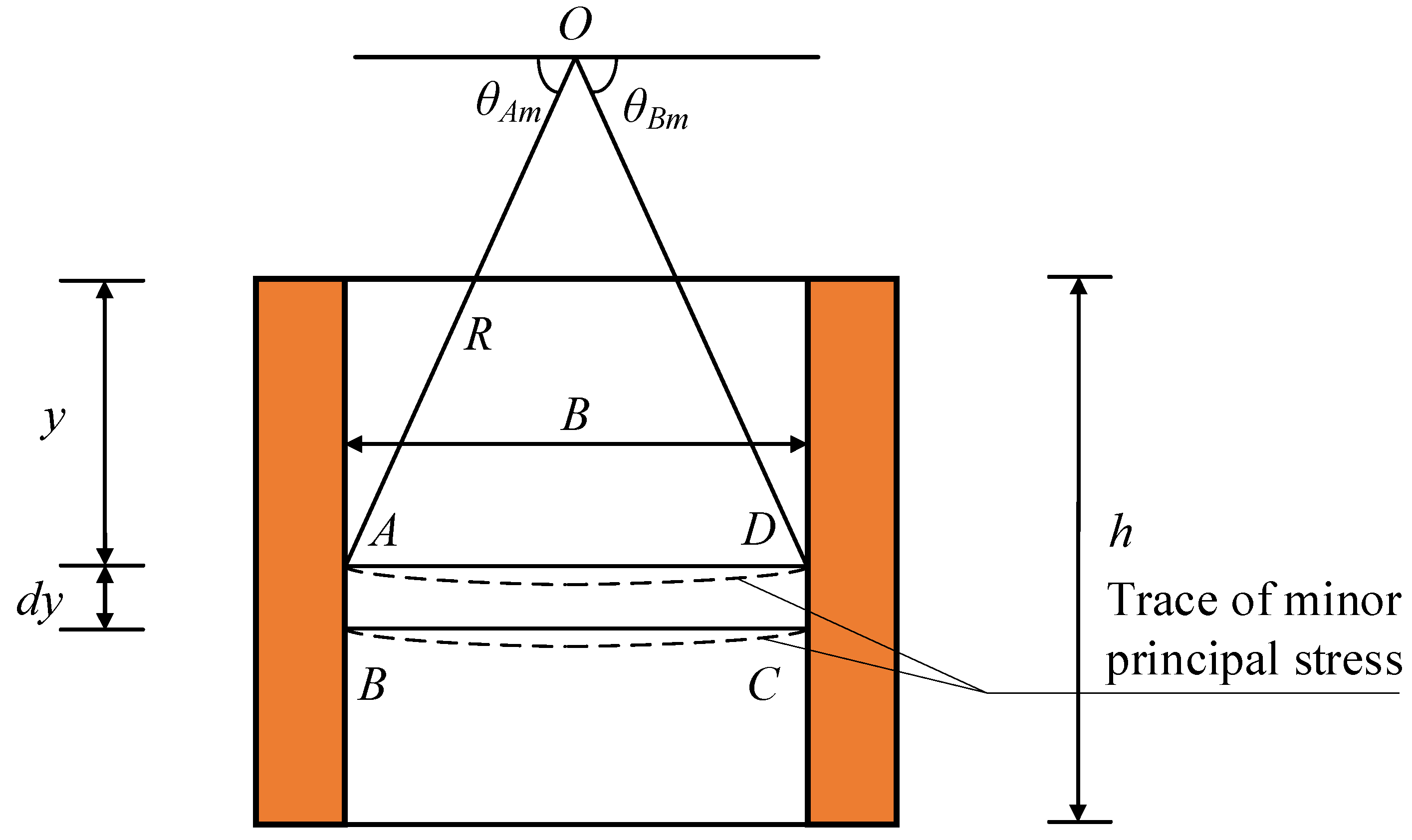
4.1. Principal Stress Trajectory
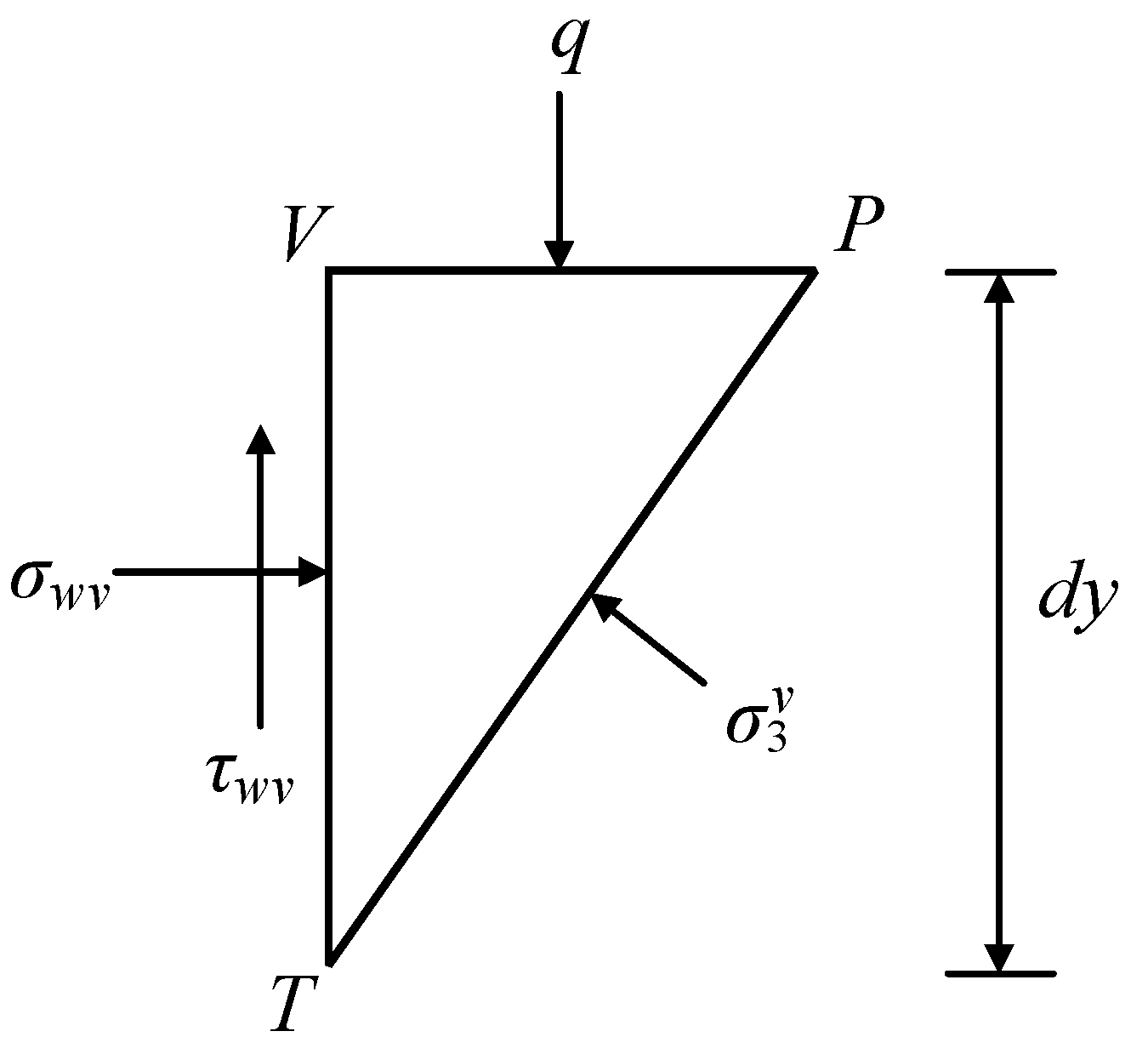
4.2. Derivation of Active Earth Pressure in Rectangular Area
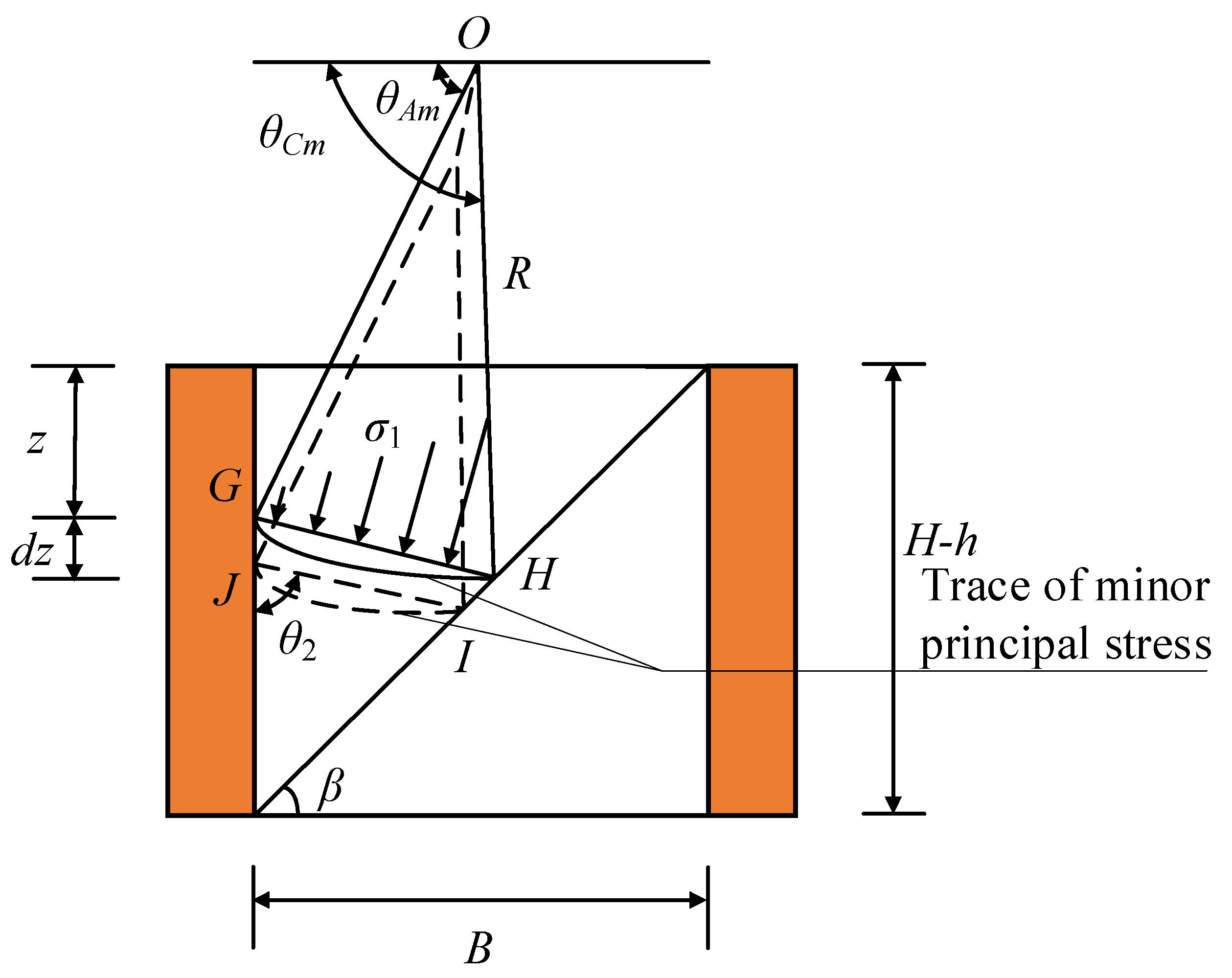
4.3. Derivation of Active Earth Pressure in Triangular Area
5. Verification by Comparison
6. Parameter Sensitivity Analysis
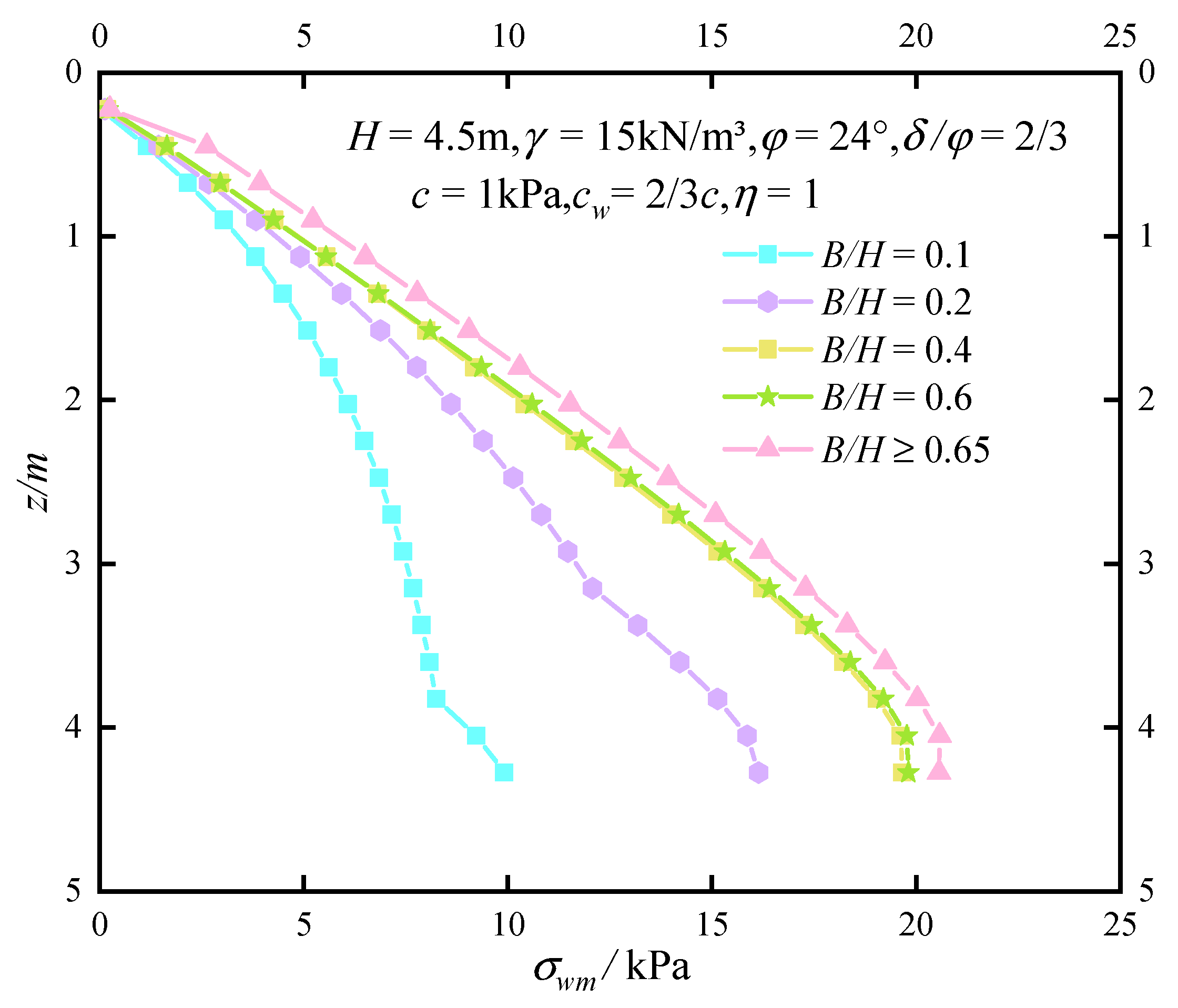
6.1. Analysis of the Soil Pressure Distribution Parameters
6.1.1. Effect of B/H on the Earth Pressure Distribution
6.1.2. Effect of φ on Soil Pressure Distribution
6.1.3. Effect of c on the Earth Pressure Distribution
6.2. Analysing the Height of the Soil-Pressure Application Point
6.2.1. Effect of B/H and φ on the Height of the Resultant Point
6.2.2. Effect of δ/φ = 2/3 on the Height of the Resultant Point
6.2.3. Effect of c on the Height of the Resultant Point
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| β | rupture angle of the backfill (); |
| h | height of zone I (m); |
| H | height of the retaining wall (m); |
| B | width of the backfill (m); |
| φ | soil’s friction angle (); |
| developed value of soil’s friction angle (); | |
| δ | wall–soil friction angle (); |
| developed value of wall–soil friction angle (); | |
| interface friction coefficient; | |
| c | cohesion of the backfill (kPa); |
| wall–soil cohesion of the retaining wall (kPa); | |
| wall–soil cohesion of the the basement wall (kPa); | |
| developed value of the wall–soil cohesion of the retaining wall (kPa); | |
| developed value of the wall–soil cohesion of the the basement wall (kPa); | |
| q | overload of the ground surface (kN/m); |
| horizontal displacement of the wall top (m); | |
| critical horizontal displacement of the retaining wall reaching the active limit state (m); | |
| failure ratio; | |
| initial value of soil friction angle (); | |
| initial value of wall–soil friction angle (); | |
| static earth-pressure coefficient; | |
| horizontal displacement of the wall at a certain depth (m); | |
| η | displacement ratio of the retaining wall; |
| normal stress on the left interface of the thin-layer element ABCD (kPa); | |
| shear stress on the left interface of the thin-layer element ABCD (kPa); | |
| normal stress on the right interface of the thin-layer element ABCD (kPa); | |
| shear stress on the right interface of the thin-layer element ABCD (kPa); | |
| major stress of the soil at the contact between the soil and the retaining wall of zone I (kPa); | |
| minor stress of the soil at the contact between the soil and the retaining wall of zone I (kPa); | |
| major stress of the soil at the interface between the soil and the basement wall of zone I (kPa); | |
| minor stress of the soil at the interface between the soil and the basement wall of zone I (kPa); | |
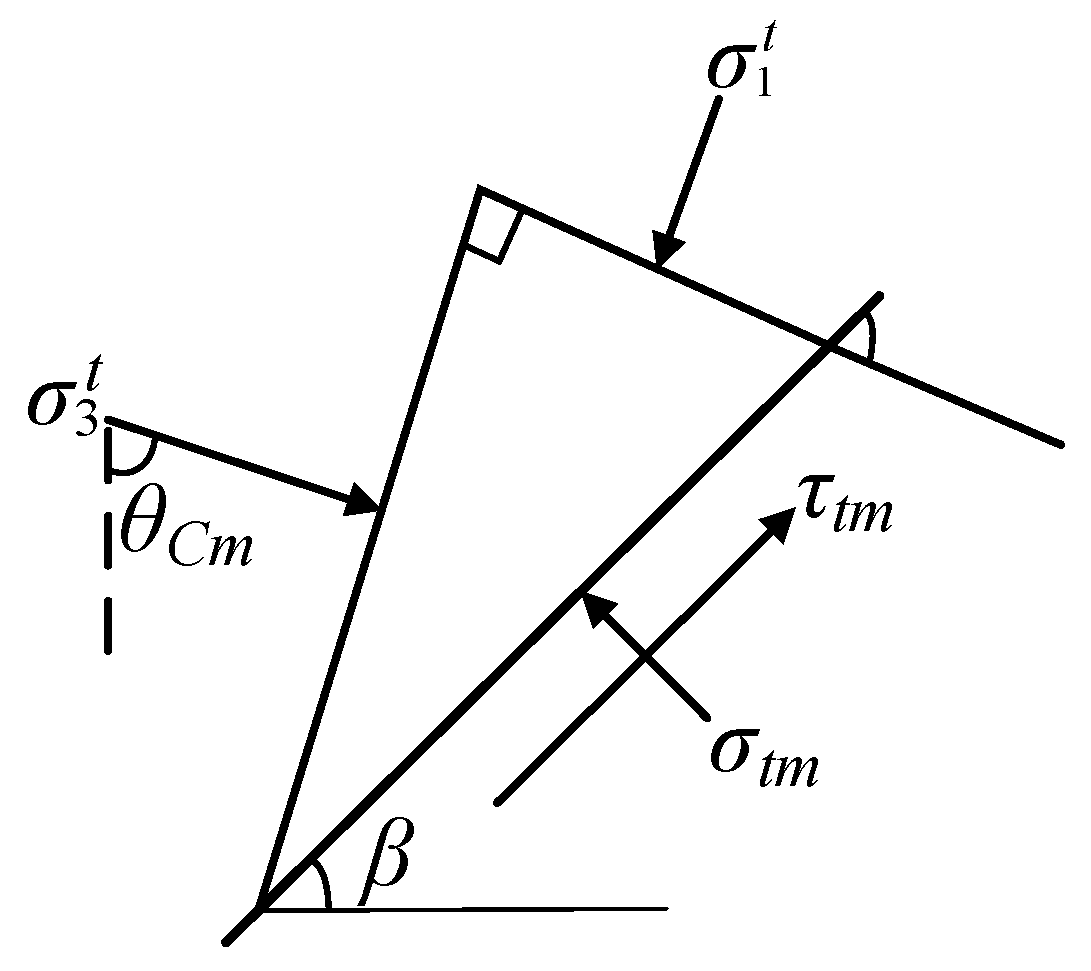
| cut angle between the maximum principal stress and the horizontal direction at the interface between the retaining wall and the soil (); | |
| included angle between the maximum principal stress and the horizontal direction at the interface between the basement wall and the soil (); | |
| N | ratio of major to minor principal stress; |
| cut angle between the major principal stress and the vertical direction (); | |
| R | radius of the Mohr stress circle (m); |
| length of left interface of the unit of zone I (m); | |
| length of right interface of the unit of zone I (m); | |
| length of upper interface of the unit of zone I (m); | |
| length of lower interface of the unit of zone I(m); | |
| average principal stress acting on (kPa); | |
| y | vertical distance between thin layer unit of zone I and surface (m); |
| dy | thickness of thin layer unit of zone I (m); |
| tangential force on the left interface of the thin-layer element ABCD (kN); | |
| tangential force on the right interface of the element ABCD (kN); | |
| force on the upper interface of the element ABCD (kN); | |
| force on the lower interface of the element ABCD (kN); | |
| gravity of the thin-layer unit ABCD (kN); | |
| unit weight (kPa); | |
| minor principal stress at point V (kPa); | |
| normal stress on the left interface of ΔVPT (kPa); | |
| shear stress on the left interface of ΔVPT (kPa); | |
| length of the line VT (m); | |
| length of the line PT (m); | |
| length of the line PV (m); | |
| depth of the fractured space (m); | |
| active earth pressure resultant force in zone I (kN); | |
| tilting moment of the retaining wall in zone I (kN·m); | |
| z | vertical distance between the upper interface of unit GHIJ and the top of zone II (m); |
| dz | thickness of thin layer unit of zone II (m); |
| normal stress on the left interface of the thin-layer element GHIJ (kPa); | |
| shear stress on the left interface of the thin-layer element GHIJ (kPa); | |
| normal stress on the right interface of the thin-layer element GHIJ (kPa); | |
| shear stress on the right interface of the thin-layer element GHIJ (kPa); | |
| cut angle between the maximum principal stress and the horizontal direction at the interface between the slip surface and the soil in zone II (); | |
| included angle between the inclined thin-layer element and the vertical direction (); | |
| major stress of the soil at the contact between the soil and the retaining wall of zone II (kPa); | |
| minor stress of the soil at the contact between the soil and the retaining wall of zone II (kPa); | |
| major stress of the soil at the interface between the soil and the slip surface (kPa); | |
| minor stress of the soil at the interface between the soil and the slip surface (kPa); | |
| length of the line GH (m); | |
| length of the line HI (m); | |
| length of the line IJ (m); | |
| length of the line GJ (m); | |
| length of the line KJ (m); | |
| length of the line GK (m); | |
| length of the line IL (m); | |
| altitude difference between points G and H (m); | |
| average principal stress acting on (kPa); | |
| force on the upper interface of the element GHIJ (kN); | |
| force on the lower interface of the element GHIJ (kN); | |
| normal force on the left interface of the element GHIJ (kN); | |
| tangential force on the left interface of the element GHIJ (kN); | |
| normal force on the right interface of the element GHIJ (kN); | |
| tangential force on the right interface of the element GHIJ (kN); | |
| gravity of the thin-layer unit GHIJ (kN); | |
| gravity of the triangular element (kN); | |
| t | minimum distance between the upper and lower interfaces of the element GHIJ (m); |
| active earth pressure resultant force in zone II (kN); | |
| tilting moment of the retaining wall in zone II (kN·m); | |
| active earth pressure resultant force (kN); | |
| M | tilting moment of the retaining wall (kN·m); |
| height of the resultant-force application point (m); |
References
- Rankine, W.J.M. On the stability of loose earth. Philos. Trans. R. Soc. Lond. 1857, 147, 9–27. [Google Scholar] [CrossRef] [Green Version]
- Coulomb, C.A. Essai sur Une Application des Règles de Maximis et Minimis Aquelques Problèmes de Statique, Relatifs a l’architecture; Imprimerie Royale; Royal Printing Office: Paris, France, 1776. (In French) [Google Scholar]
- Nian, T.; Han, J. Analytical solution for Rankine’s seismic active earth pressure in c-ϕ soil with infinite slope. J. Geotech. Geoenviron. Eng. 2013, 139, 1611–1616. [Google Scholar] [CrossRef]
- Yang, M.; Dai, X.; Zhao, M.; Luo, H. Experimental study on active earth pressure of cohesionless soil with limited width behind retaining wall. Chin. J. Geotech. Eng. 2016, 38, 131–137. (In Chinese) [Google Scholar]
- Zhang, H.; Liu, M.; Zhou, P.; Zhao, Z.; Li, X.; Xu, X.; Song, X. Analysis of earth pressure variation for partial displacement of retaining wall. Appl. Sci. 2021, 11, 4152. [Google Scholar] [CrossRef]
- Kingsley, H.W. Geostatic wall pressures. Geotech Eng. 1989, 115, 1321–1325. [Google Scholar]
- Yang, T.; He, H.J. Calculation of unlimited passive earth pressure in the model of RBT movement. Adv. Mat. Res. 2011, 250–253, 1572–1577. [Google Scholar] [CrossRef]
- Dou, G.; Xia, J.; Yu, W.; Yuan, F.; Bai, W.G. Nonlimit passive soil pressure on rigid retaining walls. Int. J. Min. Sci. Technol. 2017, 27, 581–587. [Google Scholar] [CrossRef]
- Li, D.; Wang, W.; Zhang, Q. Lateral Earth Pressure behind Walls Rotating about Base considering Arching Effects. Math. Probl. Eng. 2014, 2014, 715891. [Google Scholar] [CrossRef] [Green Version]
- Chen, F.; Lin, Y.; Li, D. Solution to active earth pressure of narrow cohesionless backfill against rigid retaining walls under translation mode. Soils Found. 2018, 59, 151–161. [Google Scholar] [CrossRef]
- Xie, M.; Zheng, J.; Zhang, R.; Cui, L.; Miao, C. Active earth pressure on rigid retaining walls built near rock faces. Int. J. Geomech. 2020, 20, 04020061. [Google Scholar] [CrossRef]
- Terzaghi, K. Theoretical Soil Mechanics; Wiley: New York, NY, USA, 1943; pp. 40–43. [Google Scholar]
- Frydman, S.; Keissar, I. Earth pressure on retaining walls near rock faces. J. Geotech. Eng. 1987, 113, 586–599. [Google Scholar] [CrossRef]
- Tsagareli, Z.V. Experimental investigation of the pressure of a loose medium on re-taining walls with a vertical back face and horizontal backfill surface. Soil Mech. Found. Eng. 1965, 2, 197–200. [Google Scholar] [CrossRef]
- James, R.G.; Bransby, P.L. Experimental and theoretical investigations of a passive earth pressure problem. Geotechnique 1970, 20, 17–37. [Google Scholar] [CrossRef]
- Yang, X.L.; Zhang, S. Seismic active earth pressure for soils with tension cracks. Int. J. Geomech. 2019, 19, 06019009. [Google Scholar] [CrossRef]
- Yang, M.; Tang, X. Rigid retaining walls with narrow cohesionless backfills under various wall movement modes. Int. J. Geomech. 2017, 17, 04017098. [Google Scholar] [CrossRef]
- Goel, S.; Patra, N.R. Effect of arching on active earth pressure for rigid retaining walls considering translation mode. Int. J. Geomech. 2008, 8, 123–133. [Google Scholar] [CrossRef]
- Rao, P.; Chen, Q.; Zhou, Y.; Nimbalkar, S.; Chiaro, G. Determination of active earth pressure on rigid retaining wall considering arching effect in cohesive backfill soil. Int. J. Geomech. 2016, 16, 04015082. [Google Scholar] [CrossRef]
- Cai, Y.; Chen, Q.; Zhou, Y.; Nimbalkar, S.; Yu, J. Estimation of passive earth pressure against rigid retaining wall considering arching effect in cohesive-frictional backfill under translation mode. Int. J. Geomech. 2017, 17, 04016093. [Google Scholar] [CrossRef]
- Pain, A.; Chen, Q.; Nimbalkar, S.; Zhou, Y. Evaluation of seismic passive earth pressure of inclined rigid retaining wall considering soil arching effect. Soil Dyn. Earthq. Eng. 2017, 100, 286–295. [Google Scholar] [CrossRef]
- Zhou, Y.T. Active earth pressure against rigid retaining wall considering effects of wall-soil friction and inclinations. KSCE J. Civ. Eng. 2018, 22, 4901–4908. [Google Scholar] [CrossRef]
- Cao, H.Y.; Liu, J.F.; Wu, C.F.; Du, L. Active earth pressures and tensile crack of the fill in a Nonlimit State. China J. Highw. Transp. 2020, 33, 51–62. (In Chinese) [Google Scholar] [CrossRef]
- Fang, Y.; Ishibashi, I. Static earth pressures with various wall movements. Geotech. Eng. 1986, 112, 317–333. [Google Scholar] [CrossRef]
- Chang, M.F. Lateral earth pressures behind rotating walls. Can. Geotech. J. 1997, 34, 498–509. [Google Scholar] [CrossRef]
- Greco, V. Active thrust on retaining walls of narrow backfill width. Comput. Geotech. 2013, 50, 66–78. [Google Scholar] [CrossRef]
- Lin, Y.-J.; Chen, F.-Q.; Yang, J.-T.; Li, D. Active earth pressure of narrow cohesionless backfill on inclined rigid retaining walls rotating about the bottom. Int. J. Geomech. 2020, 20, 04020102. [Google Scholar] [CrossRef]
- Cao, W.; Zhang, H.; Liu, T.; Tan, X. Analytical solution for the active earth pressure of cohesionless soil behind an inclined retaining wall based on the curved thin-layer element method. Comput. Geotech. 2020, 128, 103851. [Google Scholar] [CrossRef]
- Cao, W.; Liu, T.; Xu, Z. Estimation of active earth pressure on inclined retaining wall based on simplified principal stress trajectory method. Int. J. Geomech. 2019, 19, 06019011. [Google Scholar] [CrossRef]
- Matsuo, M.; Kenmochi, S.; Yagi, H. Experimental study on earth pressure of retaining wall by field tests. Soils Found. 1978, 18, 27–41. [Google Scholar] [CrossRef]
- Gong, C.; Yu, J.; Xu, R.; Wei, G. Calculation of earth pressure against rigid retaining wall rotating outward about base. J. -Zhejiang Univ. Eng. Sci. 2005, 39, 1690–1694. (In Chinese) [Google Scholar]
- Bang, S.C. Active earth pressure behind retaining walls. J. Geotech. Eng. 1985, 111, 407–412. [Google Scholar] [CrossRef]
- Niedostatkiewicz, M.; Lesniewska, D.; Tejchman, J. Experimental Analysis of Shear Zone Patterns in Cohesionless for Earth Pressure Problems Using Particle Image Velocimetry. Strain 2011, 47, 218–231. [Google Scholar] [CrossRef]
- Bourgeois, E.; Soyez, L.; Kouby, A.L. Experimental and numerical study of the behavior of a reinforced-earth wall subjected to a local load. Comput. Geotech. 2011, 38, 515–525. [Google Scholar] [CrossRef]
- Hu, J.; Zhang, Y.; Chen, L. Study on active earth pressure on retaining wall in nonlimit state. Chin. J. Geotech. Eng. 2013, 35, 381–387. (In Chinese) [Google Scholar]
- Sherif, M.; Fang, Y.; Sheriffri, R.I. Ka and Ko behind rotating and non-yielding walls. J. Geotech. Eng. 1984, 110, 41–56. [Google Scholar] [CrossRef]
- Xu, R.Q. Methods of earth pressure calculation for excavation. J. Zhejiang Univ. Eng. Sci. 2000, 34, 370–375. (In Chinese) [Google Scholar]
- Matsuzawa, H.; Hararika, H. Analyses of active earth pressure against rigid retaining walls subjected to different modes of movement. Soils Found. 1996, 36, 51–65. [Google Scholar] [CrossRef] [Green Version]
- Naikai, T. Finite element computations for active and passive earth pressure problems of retaining wall. Soils Found. 1985, 25, 98–112. [Google Scholar] [CrossRef] [Green Version]
- Xu, R.; Liao, B.; Wu, J.; Chang, S. Computational method for active earth pressure of cohesive under nonlimit state. Rock Soil Mech. 2013, 34, 148–154. (In Chinese) [Google Scholar]
- Qian, J.; Yin, Z.Z. Geotechnical Principle and Calculation; Water & Power Press: Beijing, China, 1996. [Google Scholar]
- Liu, J.; Hou, X.Y. Foundation Pit Engineering Manual; China Architecture and Building Press: Beijing, China, 1977. (In Chinese) [Google Scholar]
- Zhao, H.H. A calculation of earth pressure of cohesive fill behind retaining wall. Chin. J. Geotech. Eng. 1983, 5, 134–146. (In Chinese) [Google Scholar]
- Xu, R.; Xu, Y.; Cheng, K.; Feng, S.; Shen, S. Method to calculate active earth pressure considering soil arching effect under nonlimit state of clay. Chin. J. Geotech. Eng. 2020, 42, 362–371. (In Chinese) [Google Scholar]
- Smita, P.; Kousik, D. Study of Active Earth Pressure behind a Vertical Retaining Wall Subjected to Rotation about the Base. Int. J. Geomech. 2020, 20, 04020028. [Google Scholar] [CrossRef]
- Lai, F.; Yang, D.; Liu, S.; Zhang, H.; Cheng, Y. Towards an improved analytical framework to estimate active earth pressure in narrow c–ϕ soils behind rotating walls about the base. Comput. Geotech. 2022, 141, 1–16. [Google Scholar] [CrossRef]
- Yang, D.; Lai, F.; Liu, S.Y. Earth pressure in narrow cohesive-frictional soils behind retaining walls rotated about the top: An analytical approach. Comput. Geotech. 2022, 149, 104849. [Google Scholar] [CrossRef]
- Chen, F.-Q.; Lin, C.; Lin, L.-B.; Huang, M. Active earth pressure of narrow cohesive backfill on rigid retaining wall of rotation about the bottom. Soils Found. 2021, 61, 95–112. [Google Scholar] [CrossRef]
- Zhou, Y.; Ren, M.L. An experimental study on active earth pressure behind rigid retaining wall. Chin. J. Geotech. Eng. 1990, 12, 19–26. (In Chinese) [Google Scholar]
- Liu, H.; Kong, D.Z. Active Earth Pressure of Finite Width Soil Considering Intermediate Principal Stress and Soil Arching Effects. Int. J. Geomech. 2022, 22, 04021294. [Google Scholar] [CrossRef]
- Anderson, D.G. Seismic Analysis and Design of Retaining Walls, Buried Structures, Slopes and Embankments; Transportation Research Board: Washington, DC, USA, 2009. [Google Scholar]









Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Z.; Liu, X.; Wang, W.; Tao, Z.; Li, S. Inclined Layer Method-Based Theoretical Calculation of Active Earth Pressure of a Finite-Width Soil for a Rotating-Base Retaining Wall. Sustainability 2022, 14, 9772. https://doi.org/10.3390/su14159772
Wang Z, Liu X, Wang W, Tao Z, Li S. Inclined Layer Method-Based Theoretical Calculation of Active Earth Pressure of a Finite-Width Soil for a Rotating-Base Retaining Wall. Sustainability. 2022; 14(15):9772. https://doi.org/10.3390/su14159772
Chicago/Turabian StyleWang, Zeyue, Xinxi Liu, Weiwei Wang, Ziyu Tao, and Song Li. 2022. "Inclined Layer Method-Based Theoretical Calculation of Active Earth Pressure of a Finite-Width Soil for a Rotating-Base Retaining Wall" Sustainability 14, no. 15: 9772. https://doi.org/10.3390/su14159772
APA StyleWang, Z., Liu, X., Wang, W., Tao, Z., & Li, S. (2022). Inclined Layer Method-Based Theoretical Calculation of Active Earth Pressure of a Finite-Width Soil for a Rotating-Base Retaining Wall. Sustainability, 14(15), 9772. https://doi.org/10.3390/su14159772





