Marine Construction Waste Recycling Mechanism Considering Public Participation and Carbon Trading: A Study on Dynamic Modeling and Simulation Based on Sustainability Policy
Abstract
:1. Introduction
2. Literature Review
2.1. Recycling and Resourcing of Construction Waste
2.2. Carbon Trading Game
3. Problem Description and Model Framework
3.1. Problem Description
3.2. Problem Explanation
3.3. Model Establishment
4. Model Analysis
4.1. Stability Analysis of DER’s Strategies
4.2. Stability Analysis of CWR’s Strategies
4.3. Stability Analysis of Construction Waste Generators’ Strategies
4.4. Stability Analysis of the Equilibrium Point of the Game Model System
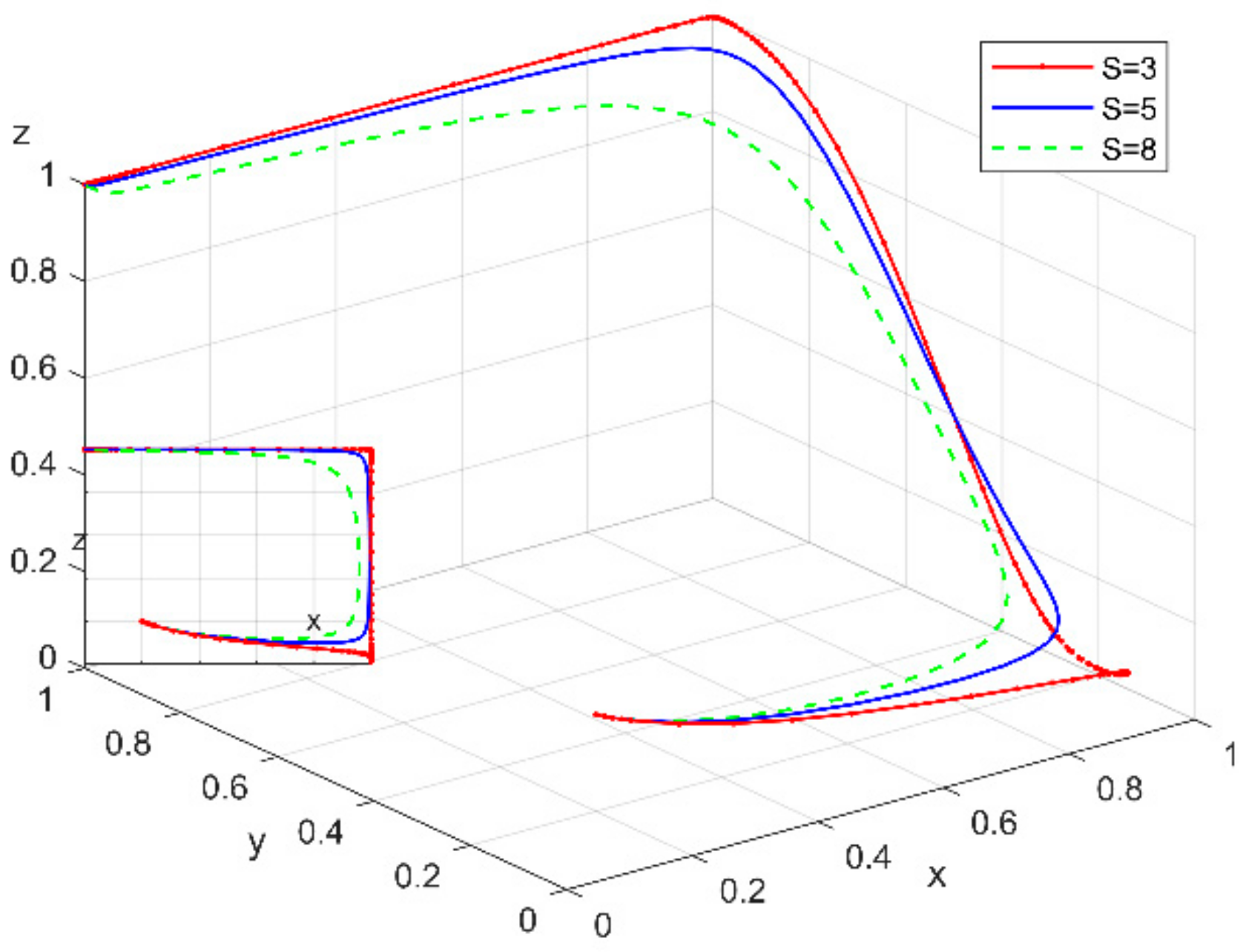
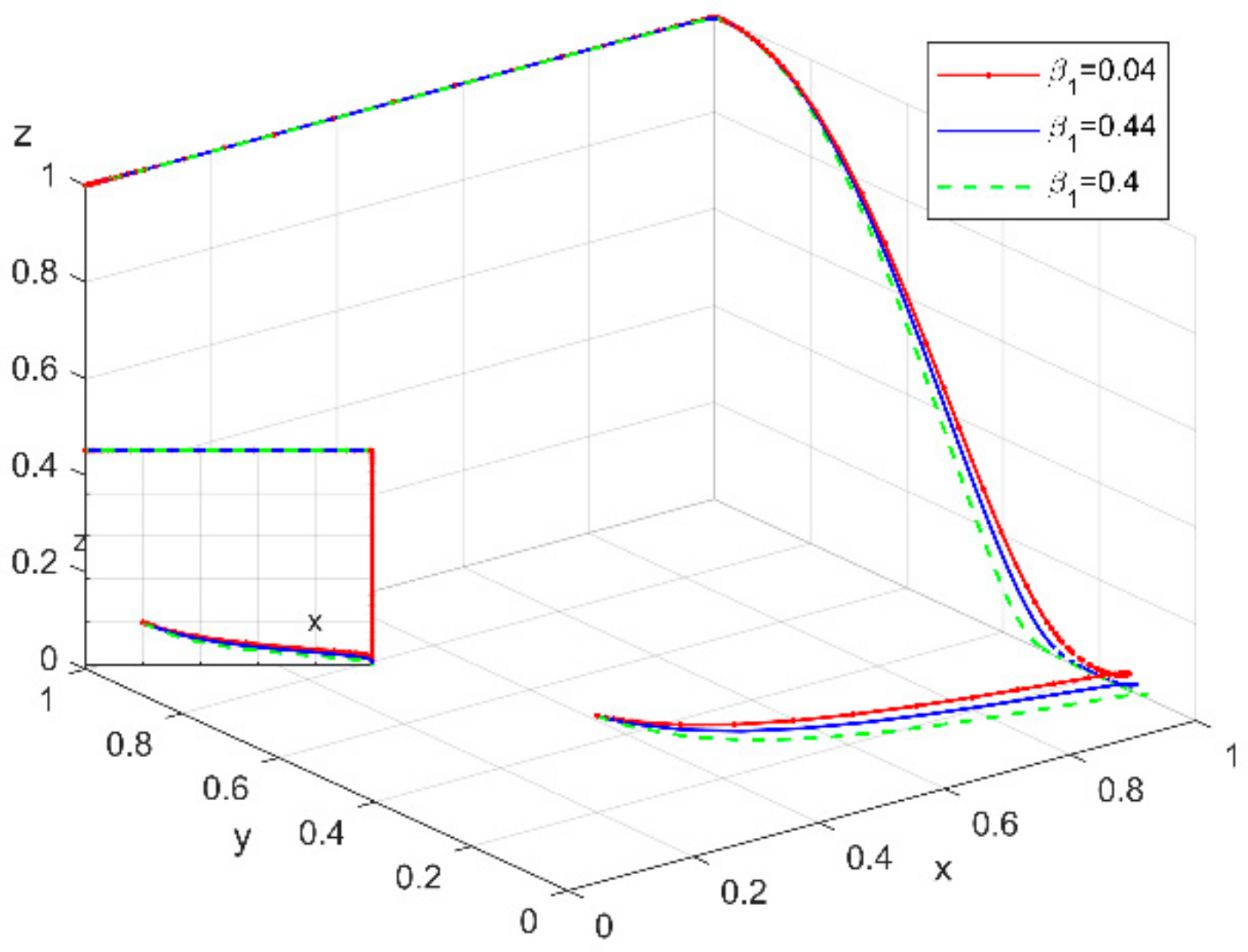
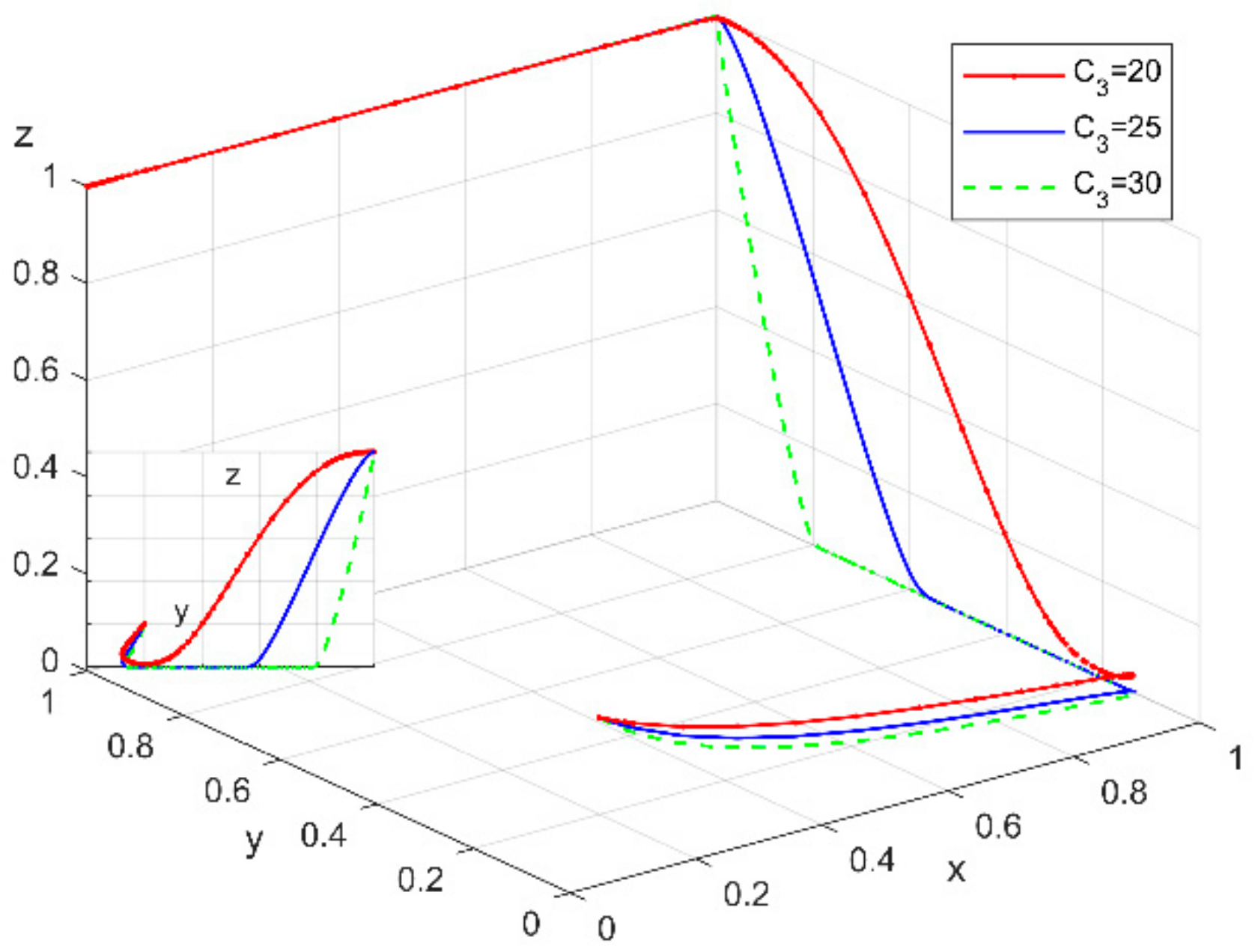
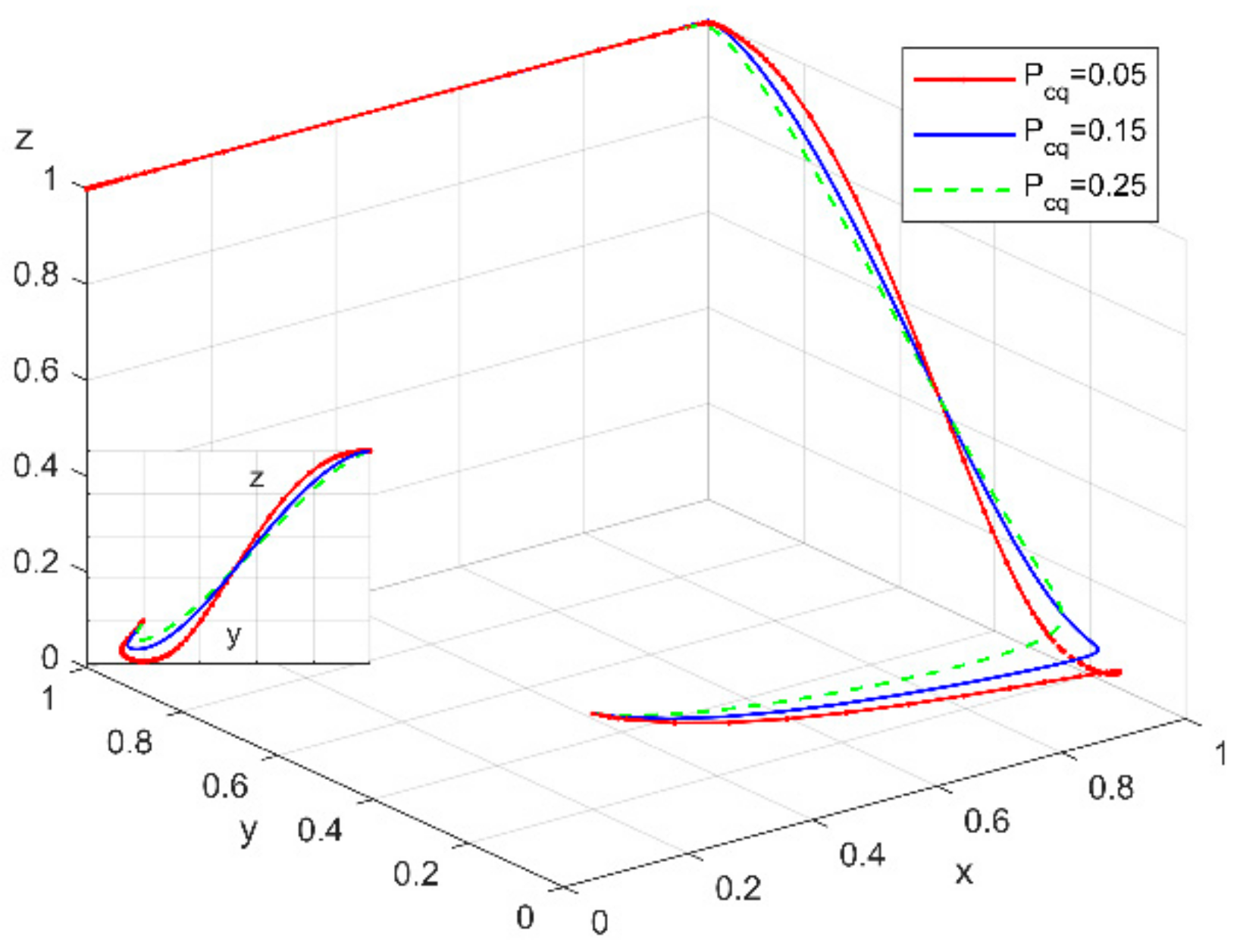
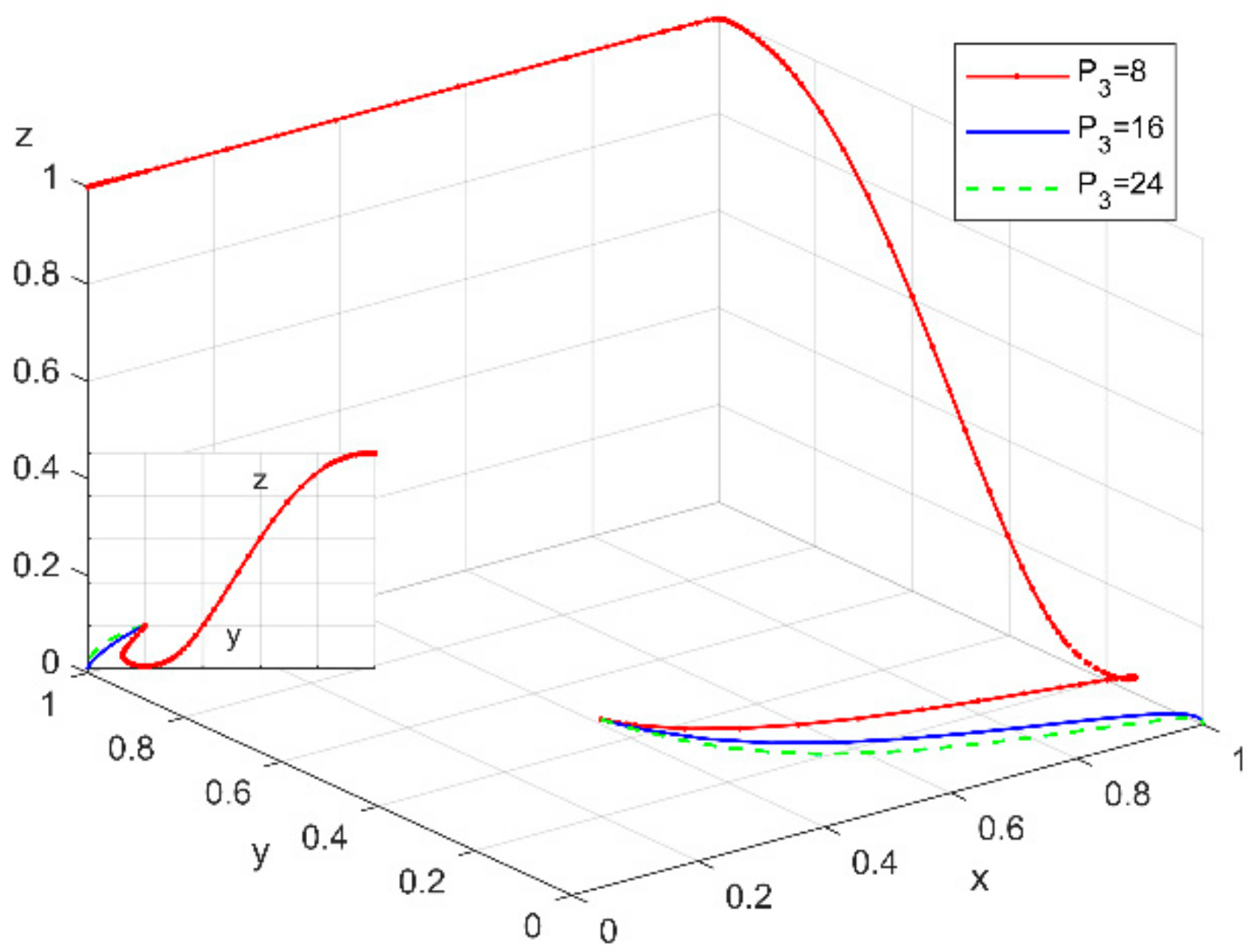
5. Numerical Simulation Analysis
6. Discussion and Limitation
6.1. Discussion
- (1)
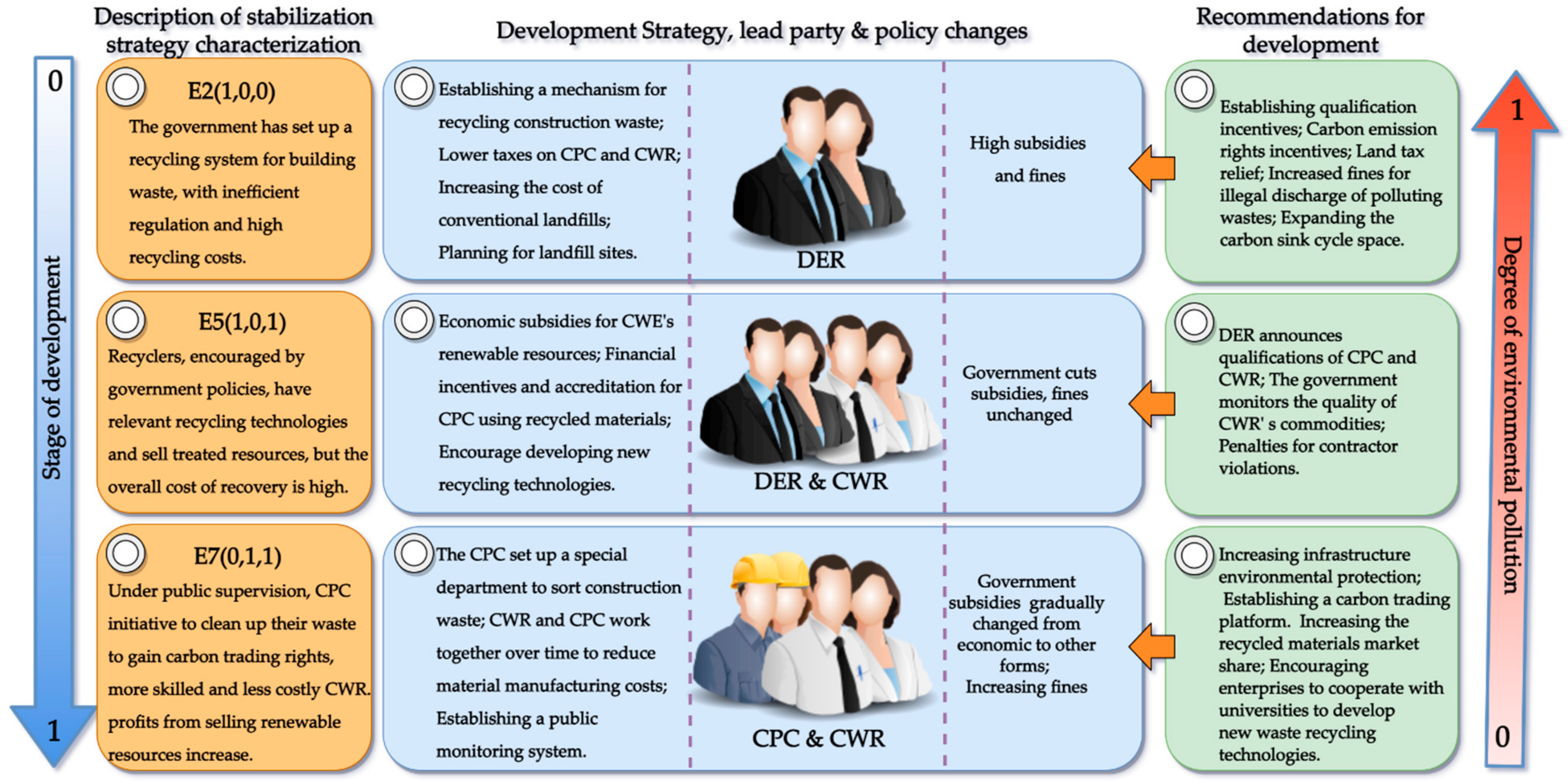
- Government chooses to specify positive incentive rules to promote marine environmental construction waste disposal and carbon trading. Under this strategy choice, it is generally the government that uses coercive measures to build the market framework and encourages all parties to participate in the policy construction by adopting the development of incentive regulations to promote the construction waste treatment and carbon sink cycle. For the other two parties, since they are all capitalists, they tend to choose the strategy that is favorable to them. With various forms of incentives from the government, such as construction qualification incentives, land tax exemptions, environmental protection qualification ratings [42], etc. The whole industry will be stimulated to generate internal healthy competition, and more project contractors and construction waste recyclers will participate in the process of construction waste sorting and recycling, which will greatly stimulate the market share of carbon trading. This will greatly stimulate the market share of carbon trading and increase the carbon sink space [12], which is the early development stage of the whole construction waste disposal system.
- (2)
- The second one is a scenario in which the government chooses incentive policies and construction waste recyclers can take the initiative to recycle construction waste. This scenario, according to the simulated values of this study, will exist after the policy is formulated, and the government’s stimulus incentive behavior has been carried out for some time [44]. At this point, after a period of development of the construction waste disposal and carbon trading platform in the marine environment, each entity has already built the relevant responsible department or institution. In particular, the construction waste recyclers will gradually move towards the industrialized and mature stage under the development of the large market of carbon trading for waste recycling, with industrial assembly lines and skilled workers with relevant skills, which can greatly reduce the cost of recycling. After the cost is reduced, construction waste recyclers will compete within the industry to improve technical productivity to expand profit margins. They will also optimize the disposal methods and technologies of non-recyclable waste [36] and expand the business of land reclamation for the disposal of non-polluting building materials waste, earning more room for development of their industry.
6.2. Limitations
7. Conclusions
- In the early stages, the planning policies of DER were crucial in increasing the proportion of enterprises recycling construction waste. Appropriate incentive policies should be formulated to establish the processing and recycling system of waste in the marine environment and the platform of carbon sink reserve and carbon trading.
- With the gradual maturation of the construction waste recycling industry, it is profitable for enterprises to recycle such waste. When a high percentage of enterprises do this, DER will gradually reduce their intervention in them, eventually evolving into no regulation. Setting a reasonable reward and punishment mechanism is conducive to promoting enterprises’ construction waste recycling and motivating DER to fulfill its responsibilities, however, excessive incentive money could discourage it from actively planning.
- The public is a significant participant in the construction waste recycling system, and whether to classify and recycle such waste could affect the living environment of the public. It is an effective method to promote construction waste recycling to mobilize public participation and increase informants’ protection and rewards. Certain profits could be brought by the current carbon emissions trading market for enterprises producing renewable construction materials. DER could take the lead to accelerate the cooperation between enterprises and universities to develop more efficient and cleaner construction waste sorting equipment to bring more carbon emission trading indexes for enterprises, which can also promote enterprises to recycle construction waste.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- UNE (UN Environment); IEA (International Energy Agency). Towards a Zero Emission. Efficient, and Resilient Buildings and Construction Sector. Global Status REPORT 2017; UN Environment and International Energy Agency: Paris, France, 2017. [Google Scholar]
- Sang, X.; Leng, X.; Xue, L.; Ran, X. Based on the Time-Spatial Power-Based Cryptocurrency Miner Driving Force Model, Establish a Global CO2 Emission Prediction Framework after China Bans Cryptocurrency. Sustainability 2022, 14, 5332. [Google Scholar] [CrossRef]
- Chen, W.; Hu, Z.-H. Analysis of Multi-Stakeholders’ Behavioral Strategies Considering Public Participation under Carbon Taxes and Subsidies: An Evolutionary Game Approach. Sustainability 2020, 12, 1023. [Google Scholar] [CrossRef]
- Bajare, D.; Bumanis, G.; Korjakins, A.; Sele, L. (Eds.) Reuse of non-metallic residues from aluminium recycling industry in production of porous building materials. In Proceedings of the 1st International Conference on Construction Materials and Structures, Johannesburg, South Africa, 24–26 November 2014. [Google Scholar] [CrossRef]
- Chen, W.; Yi, S.; Zou, S.; Zhang, S.C. Three party asymmetrical evolutionary game of construction waste resource management. J. Civ. Eng. Manag. 2019, 36, 54–59. [Google Scholar]
- Guo, F.; Wang, J.; Song, Y. How to promote sustainable development of construction and demolition waste recycling systems: Production subsidies or consumption subsidies? Sustain. Pro. Cons. 2022, 7, 407–423. [Google Scholar] [CrossRef]
- Thipwong, P.; Ting, C.T.; Huang, Y.S.; Chen, Y.Z.; Huang, W.T. Estimating the Value of Cultural Heritage Creativity from the Viewpoint of Tourists. In Proceedings of the 11th International Conference of the Thailand-Econometric-Society (TES), Chiang Mai, Thailand, 10–12 January 2018. [Google Scholar] [CrossRef]
- Zhao, W.; Leeftink, R.B.; Rotter, V.S. Evaluation of the economic feasibility for the recycling of construction and demolition waste in China-the case of ChongQing. Resour. Conserv. Recycl. 2010, 54, 377–389. [Google Scholar] [CrossRef]
- Ghaffar, S.H.; Burman, M.; Braimah, N. Pathways to circular construction: An integrated management of construction and demolition waste for resource recovery. J. Clean. Prod. 2020, 244, 118710. [Google Scholar] [CrossRef]
- Ding, Y.; Wang, Y. The research on control mechanism to environmental regulation and high-tech enterprises technology innovation. In Proceedings of the 2014 International Conference on Management Science & Engineering 21th Annual Conference Proceedings, Helsinki, Finland, 17–19 August 2014; IEEE: Piscataway, NJ, USA, 2014; pp. 1720–1724. [Google Scholar] [CrossRef]
- Lei, D.; Yingbin, F.; Wei, L.; Lingkai, K.; Zhi, Y. Evolutionary game analysis of stakeholders’ decision-making behaviours in construction and demolition waste management. Environ. Impact Assess. 2020, 84, 106408. [Google Scholar] [CrossRef]
- Li, D.; Peng, Y.; Guo, C.X.; Tan, R.W. Pricing Strategy of Construction and Demolition Waste Considering Retailer Fairness Concerns under a Governmental Regulation Environment. Int. J. Environ. Res. Public Health 2019, 16, 3896. [Google Scholar] [CrossRef]
- Hafezalkotob, A. Competition, cooperation, and coopetition of green supply chains under regulations on energy saving levels. Transp. Res. Pt. e-Logist. Transp. Rev. 2017, 97, 228–250. [Google Scholar] [CrossRef]
- Long, H.Y.; Liu, H.Y.; Li, X.W.; Chen, L.J. An Evolutionary Game Theory Study for Construction and Demolition Waste Recycling Considering Green Development Performance under the Chinese Government’s Reward-Penalty Mechanism. Int. J. Environ. Res. Public Health 2020, 17, 6303. [Google Scholar] [CrossRef]
- Tang, K.; Zhou, Y.; Liang, X.Y.; Zhou, D. The effectiveness and heterogeneity of carbon emissions trading scheme in China. Environ. Sci. Pollut. Res. 2021, 28, 17306–17318. [Google Scholar] [CrossRef] [PubMed]
- Tam, V.W.; Tam, C.M. A review on the viable technology for construction waste recycling. Res. Conserv. Recy. 2006, 47, 209–221. [Google Scholar] [CrossRef]
- Tirth, V.; Singh, R.K.; Tirth, A.; Islam, S.; Bhatkulkar, M. Sustainable Construction Practices for Residential Buildings to Reduce the Water Footprint. In Wastewater Assessment, Treatment, Reuse and Development in India; Springer: Cham, Switzerland, 2022; pp. 263–277. [Google Scholar] [CrossRef]
- Liu, J.K.; Teng, Y.; Wang, D.; Gong, E.Q. System dynamic analysis of construction waste recycling industry chain in China. Environ. Sci. Pollut. Res. 2020, 27, 37260–37277. [Google Scholar] [CrossRef] [PubMed]
- Liu, B.; Fan, J.; Hu, H.; Fu, L. (Eds.) Recycled Technology of Urban Road Construction Waste and Miscellaneous Fill Used as Subgrade Fillings. In Proceedings of the 1st International Conference on Civil Engineering, Architecture and Building Materials (CEABM 2011), Haikou, China, 18–20 June 2011. [Google Scholar] [CrossRef]
- Ulsen, C.; Kahn, H.; Hawlitschek, G.; Masini, E.A.; Angulo, S.C.; John, V.M. Production of recycled sand from construction and demolition waste. Constr. Build. Mater. 2013, 40, 1168–1173. [Google Scholar] [CrossRef]
- Qiu, L.; Zhu, Y.; Song, D.; He, X.; Wang, W.; Liu, Y.; Liu, Q. Study on the Nonlinear Characteristics of EMR and AE during Coal Splitting Tests. Minerals 2022, 12, 108. [Google Scholar] [CrossRef]
- Qiu, L.; Liu, Z.; Wang, E.; He, X.; Feng, J.; Li, B. Early-warning of rock burst in coal mine by low-frequency electromagnetic radiation. Eng. Geol. 2020, 279, 105755. [Google Scholar] [CrossRef]
- Wang, Z.; Li, H.; Zhang, X. Construction waste recycling robot for nails and screws: Computer vision technology and neural network approach. Autom. Constr. 2019, 97, 220–228. [Google Scholar] [CrossRef]
- Rautkoski, H.; Vaha-Nissi, M.; Kataja, K.; Gestranius, M.; Liukkonen, S.; Maattanen, M.; Liukkonen, J.; Kouko, J.; Asikainen, S. Recycling of Contaminated Construction and Demolition Wood Waste. Waste Biomass Valoris. 2016, 7, 615–624. [Google Scholar] [CrossRef]
- Waskow, R.P.; dos Santos, V.L.G.; Ambros, W.M.; Hoffmann Sampaio, C.; Passuello, A.; Tubino, R.M.C. Optimization and dust emissions analysis of the air jigging technology applied to the recycling of construction and demolition waste. J. Environ. Manag. 2020, 266, 110614. [Google Scholar] [CrossRef]
- Vegas, I.; Broos, K.; Nielsen, P.; Lambertz, O.; Lisbona, A. Upgrading the quality of mixed recycled aggregates from construction and demolition waste by using near-infrared sorting technology. Constr. Build. Mater. 2015, 75, 121–128. [Google Scholar] [CrossRef]
- Sarkar, M.; Maiti, M.; Malik, M.A.; Xu, S.; Li, Q.; Mandal, S. Influence of metal oxide (V2O5) in recycled waste materials for advanced durable construction technology. Constr. Build. Mater. 2018, 171, 770–778. [Google Scholar] [CrossRef]
- Tirth, V. Nexus of water footprint with energy and GDP of Saudi Arabia and solution for sustainable water usage. Adv. Water Resour. Eng. Manag. 2020, 39, 43–57. [Google Scholar] [CrossRef]
- Du, Q.; Yan, Y.Q.; Huang, Y.D.; Hao, C.C.; Wu, J. Evolutionary Games of Low-Carbon Behaviors of Construction Stakeholders under Carbon Taxes. Int. J. Environ. Res. Public Health 2021, 18, 508. [Google Scholar] [CrossRef] [PubMed]
- Xia, X.Q.; Li, C.Y.; Zhu, Q.H. Game analysis for the impact of carbon trading on low-carbon supply chain. J. Clean. Prod. 2020, 276, 123220. [Google Scholar] [CrossRef]
- Sun, C.Y.; Wei, J.; Zhao, X.L.; Yang, F. Impact of Carbon Tax and Carbon Emission Trading on Wind Power in China: Based on the Evolutionary Game Theory. Front. Energy. Res. 2022, 9, 811234. [Google Scholar] [CrossRef]
- Liu, L.; Zhang, Z.S.; Wang, Z. Two-sided matching and game on investing in carbon emission reduction technology under a cap-and-trade system. J. Clean. Prod. 2021, 282, 124436. [Google Scholar] [CrossRef]
- Wang, M.; Hou, S.H.; Qiu, R. (Eds.) Non-cooperative Game Based Carbon Emission Reduction for Supply Chain Enterprises with a Cap and Trade Mechanism. In Proceedings of the 13th International Conference on Management Science and Engineering Management (ICMSEM), St. Catharines, ON, Canada, 5–8 August 2019. [Google Scholar] [CrossRef]
- Yu, S.; Hou, Q.; Sun, J.Y. Investment Game Model Analysis of Emission-Reduction Technology Based on Cost Sharing and Coordination under Cost Subsidy Policy. Sustainability 2020, 12, 2203. [Google Scholar] [CrossRef]
- Zhang, S.Y.; Wang, C.X.; Yu, C. The evolutionary game analysis and simulation with system dynamics of manufacturer’s emissions abatement behavior under cap-and-trade regulation. Appl. Math. Comput. 2019, 355, 343–355. [Google Scholar] [CrossRef]
- Maria, A.D.; Eyckmans, J.; Acker, K.V. Downcycling versus recycling of construction and demolition waste: Combining LCA and LCC to support sustainable policy making. Waste Manag. 2018, 75, 3–21. [Google Scholar] [CrossRef]
- Yongbo, S. Multi-agent evolutionary game in the recycling utilization of construction waste. Sci. Total Environ. 2020, 738, 139826. [Google Scholar] [CrossRef]
- Zhang, F.; Ju, Y.B.; Gonzalez, E.; Wang, A.H.; Dong, P.W.; Giannakis, M. Evaluation of construction and demolition waste utilization schemes under uncertain environment: A fuzzy heterogeneous multi-criteria decision-making approach. J. Clean. Prod. 2021, 313, 127907. [Google Scholar] [CrossRef]
- Wang, J.J.; Teng, Y.; Chen, Z.; Bai, J.; Niu, Y.N.; Duan, H.B. Assessment of carbon emissions of building interior decoration and renovation waste disposal in the fast-growing Greater Bay Area, China. Sci. Total Environ. 2021, 798, 149158. [Google Scholar] [CrossRef] [PubMed]
- Wang, S.S.; Chen, G.; Han, X. An Analysis of the Impact of the Emissions Trading System on the Green Total Factor Productivity Based on the Spatial Difference-in-Differences Approach: The Case of China. Int. J. Environ. Res. Public Health 2021, 18, 9040. [Google Scholar] [CrossRef] [PubMed]
- Chen, L.Q.; Gao, M. Predictive modeling for behavioral evo Formal or informal lution of municipal household waste classification and recycling. Suatain. Cities Soc. 2022, 78, 9040. [Google Scholar] [CrossRef]
- Guo, F.; Wang, J.; Liu, D.; Song, Y. Evolutionary Process of Promoting Construction Safety Education to Avoid Construction Safety Accidents in China. Int. J. Environ. Res. Public Health 2021, 18, 10392. [Google Scholar] [CrossRef]
- Song, Y.; Wang, J.; Liu, D.; Huangfu, Y.; Guo, F.; Liu, Y. The Influence of Government’s Economic Management Strategies on the Prefabricated Buildings Promoting Policies: Analysis of Quadripartite Evolutionary Game. Buildings 2021, 11, 444. [Google Scholar] [CrossRef]
- Chen, J.G.; Hua, C.X.; Liu, C.Y. Considerations for better construction and demolition waste management: Identifying the decision behaviors of contractors and government departments through a game theory decision-making model. J. Clean. Prod. 2019, 212, 190–199. [Google Scholar] [CrossRef]


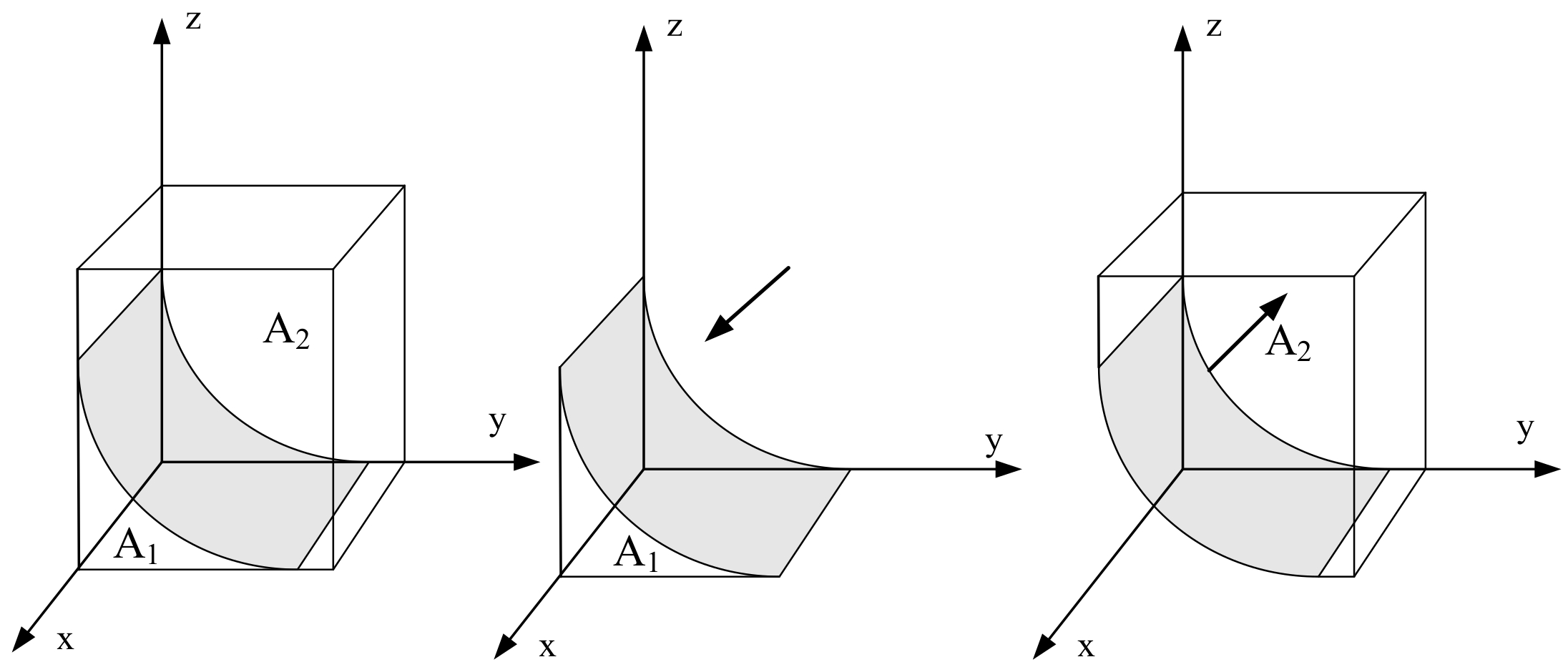
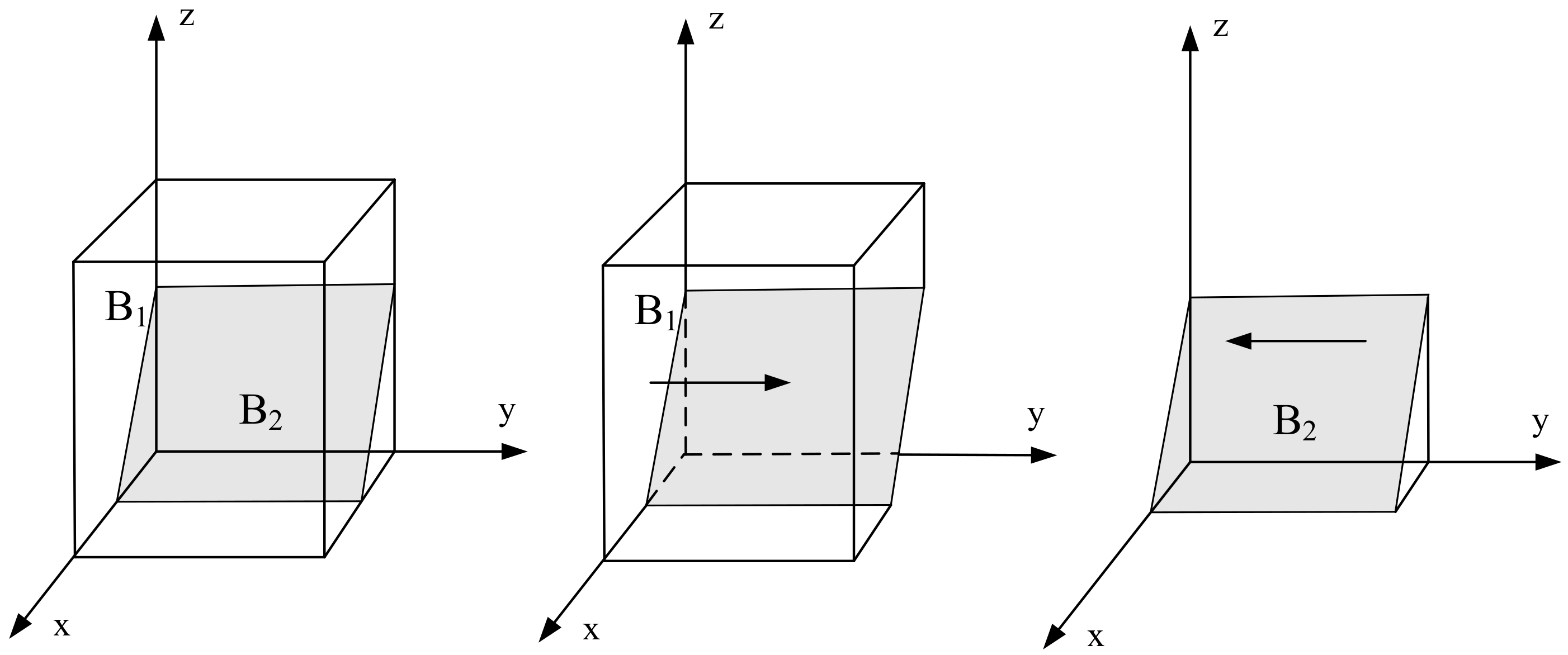
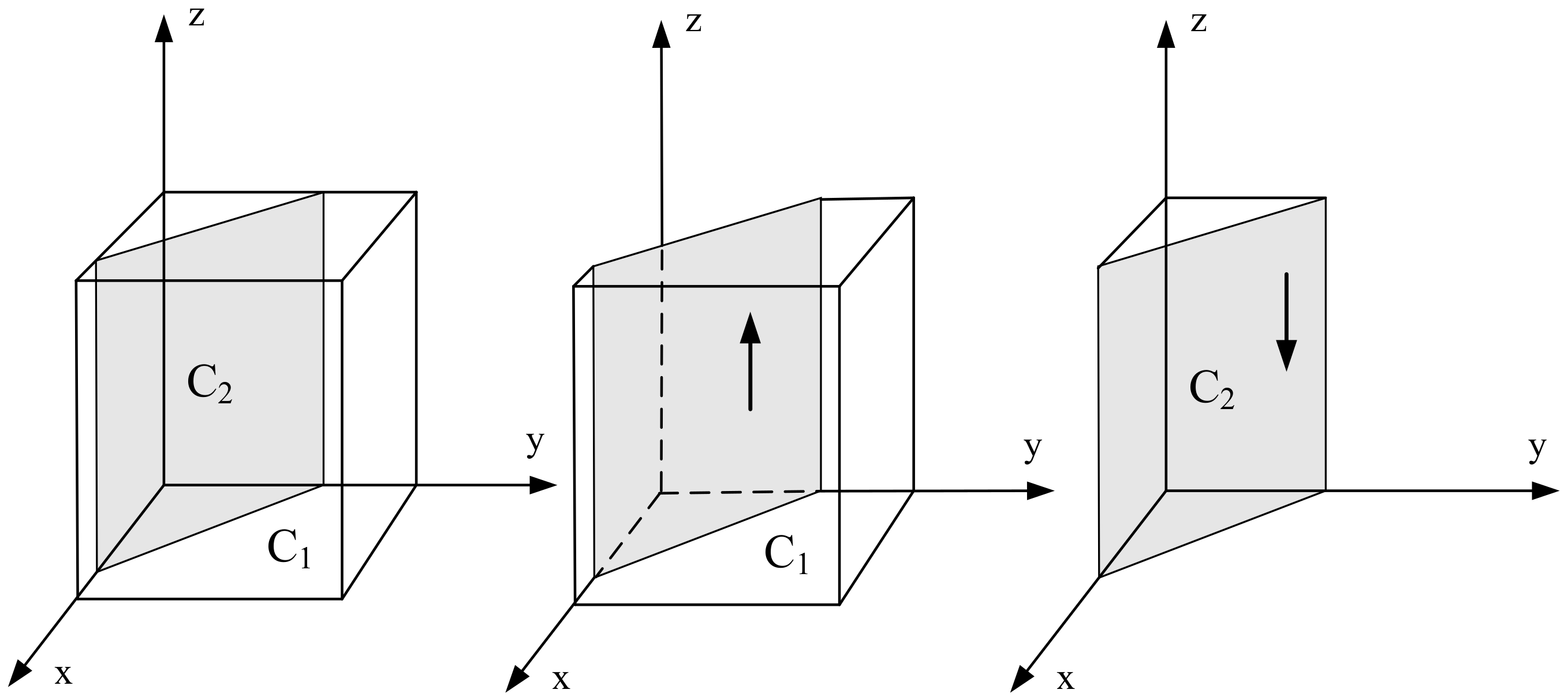



| CPC | DER’s Active Regulation(x) | DER’s Negative Regulation(1 − x) | ||
|---|---|---|---|---|
| Recycle of CWR(y) | Non-Recycle of CWR(1 − y) | Recycle of CWR(y) | Non-Recycle of CWR(1 − y) | |
| recycling(z) | ||||
| Non- recycling (1 − z) | ||||
| Equilibrium Points | Jacobian Matrix Eigenvalues | Stability Conclusions | Conditions | |
| Symbols | ||||
| , , | (+,−,−) | IP | ||
| , , | (−,−,−) | ESS | ➀ | |
| , , | (+,+,+) | IP | ||
| , | (+,−,+) | IP | ||
| , , | (−,−,−) | ESS | ➁ | |
| , , | (−,−,+) | IP | ||
| , , | (−,−,−) | ESS | ||
| , | (+,−,−) | IP | ||
| ) | ,0,0 | (−,,0) | IP | ➂ |
| (, ,−) | IP | ➃ ➄ | ||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, J.; Song, Y.; Wang, W.; Wang, S.; Guo, F.; Lu, J. Marine Construction Waste Recycling Mechanism Considering Public Participation and Carbon Trading: A Study on Dynamic Modeling and Simulation Based on Sustainability Policy. Sustainability 2022, 14, 10027. https://doi.org/10.3390/su141610027
Wang J, Song Y, Wang W, Wang S, Guo F, Lu J. Marine Construction Waste Recycling Mechanism Considering Public Participation and Carbon Trading: A Study on Dynamic Modeling and Simulation Based on Sustainability Policy. Sustainability. 2022; 14(16):10027. https://doi.org/10.3390/su141610027
Chicago/Turabian StyleWang, Junwu, Yinghui Song, Wei Wang, Suikuan Wang, Feng Guo, and Jiequn Lu. 2022. "Marine Construction Waste Recycling Mechanism Considering Public Participation and Carbon Trading: A Study on Dynamic Modeling and Simulation Based on Sustainability Policy" Sustainability 14, no. 16: 10027. https://doi.org/10.3390/su141610027
APA StyleWang, J., Song, Y., Wang, W., Wang, S., Guo, F., & Lu, J. (2022). Marine Construction Waste Recycling Mechanism Considering Public Participation and Carbon Trading: A Study on Dynamic Modeling and Simulation Based on Sustainability Policy. Sustainability, 14(16), 10027. https://doi.org/10.3390/su141610027







