Research on Closed-Loop Supply Chain Decision Making and Recycling Channel Selection under Carbon Allowance and Carbon Trading

Abstract

The massive emission of carbon dioxide and other greenhouse gases has begun to threaten the survival of human beings and the balance of the Earth’s ecology. If carbon emission is left unchecked, we will face terrible consequences. Closed-loop supply chain operation is an effective way to improve economic and environmental benefits at the same time. This paper attempts to study the decision-making and recycling channel selection of CLSC under carbon allowance and carbon trading policies. This paper constructs a closed-loop supply chain consisting of a manufacturer, a retailer and a third-party recycler, and uses the Stackelberg game-theoretic method to decide variables such as the product price and recycling rate of CLSC under three recycling models. Through the analysis and comparison of the manufacturer’s profit of and the carbon emissions in different models, the following conclusions are drawn. (1) When the manufacturer recycles WEEE, the supply chain obtains the most profit. (2) When the retailer recycles WEEE, the carbon emissions of the supply chain are lowest. (3) The manufacturer tends to choose the M model, which is the manufacturer recycling model. The T model and the R model have little difference in carbon emissions. For easier management, the government prefers to choose the T model, which is the third-party recycler recycling model.

1. Introduction

In recent years, global warming and environmental pollution have posed a serious threat to the ecological balance and human survival, which has attracted the attention of many countries and organizations in the world. Academics generally agree that the emission of greenhouse gases such as carbon dioxide is an important cause of these issues. Therefore, reducing carbon emissions has become a consensus in the international community. Since 1997, most countries in the world have successively signed the Kyoto Protocol to stabilize the greenhouse gas content in the atmosphere at an appropriate level, so as to prevent serious climate change from causing harm to human beings [1]. The EU ETS, the world’s first multi-country carbon emissions trading system, was officially launched in January 2005. It allocated the emission reduction targets stipulated in the Kyoto Protocol to member countries, so as to achieve the carbon emission reduction targets more effectively [2]. In 2015, nearly 200 countries jointly adopted the Paris Agreement, which aims to significantly reduce global greenhouse gas emissions and seek measures to limit the temperature rise to no more than 1.5 degrees Celsius higher than the pre-industrial levels [3].

In 2021, global carbon dioxide emissions reached 34.9 billion tons, up 4.8% over the previous year. If this trend continues, the temperature of the Earth may rise by 1.5 degrees Celsius in 9.5 years [4]. In order to avoid catastrophic climate change, governments and enterprises should take joint action to implement more extensive and effective mitigation measures. At present, the government’s emission reduction policies are mainly concentrated in manufacturing enterprises, such as formulating industrial emission standards, allocating carbon allowances, developing Emission Trading Schemes (ETS) and so on [5]. Among the existing policies, carbon allowance and carbon trading are among the most effective policies implemented by many countries [6]. According to these policies, the government allocates limited carbon allowances to enterprises, which are called carbon emission caps. When an enterprise’s carbon emissions exceed its quota, it should use greener technologies to reduce emissions or buy more allowances from the carbon trading market. Those who have excess carbon allowances can sell in the same market [7]. Enterprises that emit more carbon emissions than the quota will be severely punished by the government. Therefore, this policy can not only encourage enterprises to reduce carbon emissions, but also punish those enterprises that pollute heavily.

With the progress of technology and the increase of customers’ demand, the replacement cycle of electronic products is becoming shorter and shorter, and more and more customers stop using them before the end of the product life cycle. A large volume of waste electronic and electrical equipment (WEEE) has been produced, which brings great pressure on the government and enterprises. On the one hand, manufacturing enterprises need to purchase more carbon allowances to produce more products. On the other hand, the government needs to spend resources to deal with more and more WEEE, which brings serious pollution to the air and soil. The closed-loop supply chain (CLSC) is considered to be an effective means to deal with WEEE. It can reduce the environmental pollution and carbon emissions of WEEE, and bring economic benefits to enterprises. CLSC adds recycling and remanufacturing to the traditional supply chain. In recent years, many enterprises have introduced it into manufacturing. Relying on advanced technology and management methods, CLSC can effectively meet the demand of sustainable development. Saberi et al. [8], Gayialis et al. [9], Yadav et al. [10], Manavalan et al. [11] and Konstantakopoulos et al. [12] integrate various advanced technologies into supply chain management, which significantly enhances the development of a sustainable economy and effectively curbs carbon emissions.

It is estimated that CLSC can help reduce 10 million tons of carbon dioxide per year in the UK [13]. Some international enterprises, such as Canon and Xerox, have achieved significant cost savings by implementing closed-loop supply chain management. Canon can save millions of dollars every year by remanufacturing recycled toner cartridges as raw material [14]. By implementing closed-loop supply chain management, Xerox has realized the effective recycling and remanufacturing of more than 65% of ink cartridges, which not only reduces the generation of waste, but also saves manufacturing costs [15]. Apple has improved the WEEE recovery rate by setting up an online recycling platform, and CLSC has helped it reduce its carbon emissions by 15.4% [16]. Huawei has integrated the concept of carbon emission reduction into product planning, design, R&D, manufacturing, recycling and services to provide customers with leading low-carbon and environmentally friendly products and solutions [17]. The academic circles have discussed CLSC management under various carbon policies. Some scholars (Li et al. [18], De and Giri [19], Handayani et al. [20]) have constructed emission reduction or low-carbon CLSC under carbon policies. Other scholars have optimized CLSC based on carbon policies, balancing economic development and low-carbon environmental protection.

This paper studies CLSC decision making under carbon cap and carbon trading policies. We will explore the following questions:

(1) How do manufacturing enterprises make decisions about CLSC under carbon allowance and carbon trading policy? How is a recycling channel chosen?

(2) Can carbon allowance and carbon trading policies effectively reduce the carbon emissions of enterprises? Do they reduce the economic benefits of the enterprises?

(3) How can the government achieve the maximum effect of carbon emission reduction?

In order to answer these three questions, three game theoretic models are developed to evaluate the impact of carbon allowance and carbon trading policies on the decision making and recycling channel selection of a CLSC consisting of a manufacturer, a retailer and a third-party recycler. The manufacturer, the retailer and the third-party recycler make their own decisions separately to maximize profits, with the manufacturer as the leader of the Stackelberg game. In each of the three models, the manufacturer, the retailer and the third-party recycler are responsible for WEEE recycling separately. The models and results provide optimal strategies for member enterprises in CLSC.

The rest of this article is organized as follows. Section 2 provides a review of the related literature. Section 3 puts forward the research questions and basic assumptions. In Section 4, three models are constructed and the optimal solutions are pointed out. Section 5 compares the results of the different models. Section 6 is the numerical analysis. The results are discussed in Section 7. Section 8 summarizes the main findings, and puts forward the future research directions.

2. Literature Review

In this section, we review the research related to this article. These studies can be divided into three directions: CLSC decision-making research, CLSC recycling channel selection research and supply chain management research under carbon policies.

2.1. CLSC Decision-Making Research

CLSC decision-making research refers to the study of the pricing strategy and the corresponding coordination mechanism of each node enterprise in the CLSC system. He et al. [21] designed a dual-channel CLSC, in which the manufacturer can sell new products through the retailer and remanufactured products through the third-party platform with government subsidies. They deduced the manufacturer’s optimal channel structure and pricing decisions, and found that the government can encourage the manufacturer to adopt the desired channel structure by setting appropriate subsidy levels. Masudin et al. [22] modeled a reverse logistics network for battery recycling considering environmental and manufacturing costs, solving the problem of determining optimal orders of recycled batteries, lead alloy and plastics. Some scholars have introduced government subsidies into the decision making of CLSC: Li et al. [23] believed that when the government rewards or punishes enterprises according to the recovery rate of WEEE, the joint decision making of the manufacturer, the retailer and the third-party recycler can maximize the total profit of CLSC, but this will lead to the emergence of a monopoly, so the government usually intervenes through other means. Ma et al. [24] considered the impact of government consumption subsidies from the perspectives of consumers, enterprises, and the scale of CLSC. They found that consumer subsidies are conducive to the expansion of CLSC, and both the manufacturer and the retailer are beneficiaries of consumer subsidies. Some scholars also discussed the decision-making behavior of CLSC from the perspective of consumer preference. Su et al. [25] explored CLSC decision making considering consumers’ green preferences, and provided useful decision support and guidance for enterprises and governments in decisions related to waste product recycling. Guo et al. [26], Bell et al. [27], Zhang et al. [28], Panda et al. [29] and Wang et al. [30] respectively considered the influence of dual-channel, natural resource scarcity, equity crowdfunding, social responsibility and consumer value considerations on the closed-loop supply chain. They explored the decision making of CLSC based on different scenarios.

2.2. CLSC Recycling Channel Selection Research

For a CLSC composed of a monopoly manufacturer and a retailer, Savaskan [31] studied pricing decisions and the selection of recycling channels. Based on the game-theoretic method, he concluded that in CLSC with decentralized decision making, selecting the retailer as the recycling channel can maximize the profit of CLSC. Modak [32] considered the quality differences of WEEE in different recycling channels, and studied the influence of quality and recycling channels on the recycling pricing strategy of enterprises in CLSC. Liu et al. [33] used the revenue-sharing and cost-sharing contracts as the coordination mechanism to study the WEEE recycling problem under the third-party recycling mode. The results showed that when the retailer sells both new products and remanufactured products, consumers are more sensitive to the recovery price, and the profits of the manufacturer, the retailer and the third-party recycler all increase. Considering the uncertainty of second-hand product collection, Giri et al. [34] developed different recycling models under the different power structures or interactions of CLSC. Huang and Liang [35] discussed the CLSC models of three recycling modes (single online recycling, single offline recycling, online and offline dual recycling channels), and found that the relationship between the recycling volumes of the three modes depends on consumers’ preference for online recycling. Choi et al. [36] studied the power structure of CLSC, compared the influence of different power structures on the selection of CLSC recycling channels, and found that no matter what the power structure is, using the retailer as a recycling channel can make the whole supply chain achieve the greatest profit. Based on game theory, Ranjbar et al. [37] analyzed the influence of two competitive recycling channels, the retailer recycling channel and the third-party recycler recycling channel, on CLSC decision making.

2.3. Supply Chain Management Research under Carbon Policies

In recent years, decision making under carbon policy has become one of the most critical areas of research. Handayani et al. [20] proposed the Production–Distribution model with high traceability and low carbon emissions, and it produces the minimum total production and distribution cost. Li et al. [38] analyzed the impact of carbon subsidies on CLSC. They discussed when and how the government would implement carbon subsidies to encourage enterprises to reduce carbon emissions most effectively. Wang et al. [39] explored the willingness of manufacturers to participate in WEEE recycling in the CLSC under the carbon trading mechanism. They found that the unit carbon transaction price affects the profits of supply chain members according to the amount of carbon emission reduction. Zhang and Li [40] found that under different carbon regulatory policies, corporate social responsibility activities have different impacts on the environmental benefits of the supply chain and corporate carbon emission reduction decisions. Mohammed et al. [41] extended the closed-loop supply chain model to three models considering different carbon policies: carbon cap policy, carbon cap-trade policy and carbon offset policy. By comparing them, they concluded that the carbon cap-trade mechanism is the most effective and flexible policy. Xu et al. [42] studied the impact of different carbon policies on CLSC decision making, and the results of their proposed model and numerical experiments showed that CLSC produces fewer emissions and is more cost-effective under the carbon cap-trade policy. Samuel et al. [43] explored the operation of CLSC under three different carbon strategies. They found that the carbon cap policy had the most significant impact on the network structure, and as the penalty increased, the carbon cap policy had a reverse impact on profits. To sum up, scholars generally believe that the CLSC under the carbon cap-and-trade policy can obtain the best profits and has the best emission reduction effect.

3. Problem Description and Model Assumption

This paper designs a CLSC network consisting of a manufacturer and a retailer or a manufacturer, a retailer and a third-party recycler. The manufacturer uses new raw materials to manufacture new products at unit price ${\mathrm{c}}_n$, and uses recycled WEEE to remanufacture products at unit price ${\mathrm{c}}_r$. The manufacturer wholesales the product to the retailer at the unit price of $\mathsf{\omega }$, and the retailer sells the product to the consumers at the unit price of $p$. In different models, a different member is responsible for recycling WEEE, and no matter who is recycling, they all recycle waste products from the market at the unit price of A. When the retailer or the third-party recycler is responsible for recycling WEEE, they transfer it to the manufacturer at a unit price of $b$. According to Yang et al. [44], the carbon allowance allocated to the manufacturer is $Q$, and the unit price of carbon trading is $p_c$. The initial unit carbon emission from manufacturing new products is $e$, the unit carbon intensity of remanufactured products relative to newly manufactured products is $\lambda$, $0<\lambda <1$. Therefore, the unit carbon emission of the remanufactured products is $\lambda e$.

In order to make the research more practical, this paper makes the following assumptions:

Hypothesis 1.

The manufacturer is in the leading position in CLSC because of its unique technology or patent, followed by the retailer and the third-party recycler.

Hypothesis 2.

The quality and function of new products manufactured from the original raw materials are the same as those remanufactured from recycled WEEE [45].

Hypothesis 3.

The cost of products remanufactured from recycled WEEE is less than the cost of products manufactured from virgin raw materials, that is,$c_n>c_r+b$. Let$∆=c_n-c_r$, obviously,$∆>b$.

Hypothesis 4.

In order to ensure that the profit of the enterprise participating in recycling is positive, it is assumed that$b>A$, so as to ensure that relevant enterprises actively participate in recycling WEEE.

Hypothesis 5.

The market demand$D$decreases with the increase of the product price p, and the demand price function is$D=\varnothing -\beta p$ [31,46], where $\varnothing$ is the potential market size, and $\beta$ is the price elasticity coefficient of demand.

Hypothesis 6.

In the process of recycling WEEE, in addition to the cost of recycling WEEE, the recycler also needs to pay an additional recycling effort cost, which is$I=C_L{\tau }^2$ [47,48]. $C_L$ is the recycling effort coefficient, and the recovery rate $\tau$ is the ratio of the output of remanufacturing with recycled WEEE to the total output. Under this assumption, the recovery effort cost $I$ is a quadratic function of $\tau$, and the larger the recovery rate, the higher the recovery effort cost.

Hypothesis 7.

The carbon trading market is a completely free market with sufficient market capacity. Carbon allowance and carbon trading prices are set entirely by the government.

Hypothesis 8.

To make the results meaningful, it is assumed that$8C_L>\beta {\left(A-∆-e_0{p}_e+\lambda e_0{p}_e\right)}^2$and$\varnothing \ge \beta c_n+\beta e{p}_c$.

The parameter description is shown in back part.

When the solved variables and expressions are marked with a “*” in the upper right corner, they represent the optimal solution.

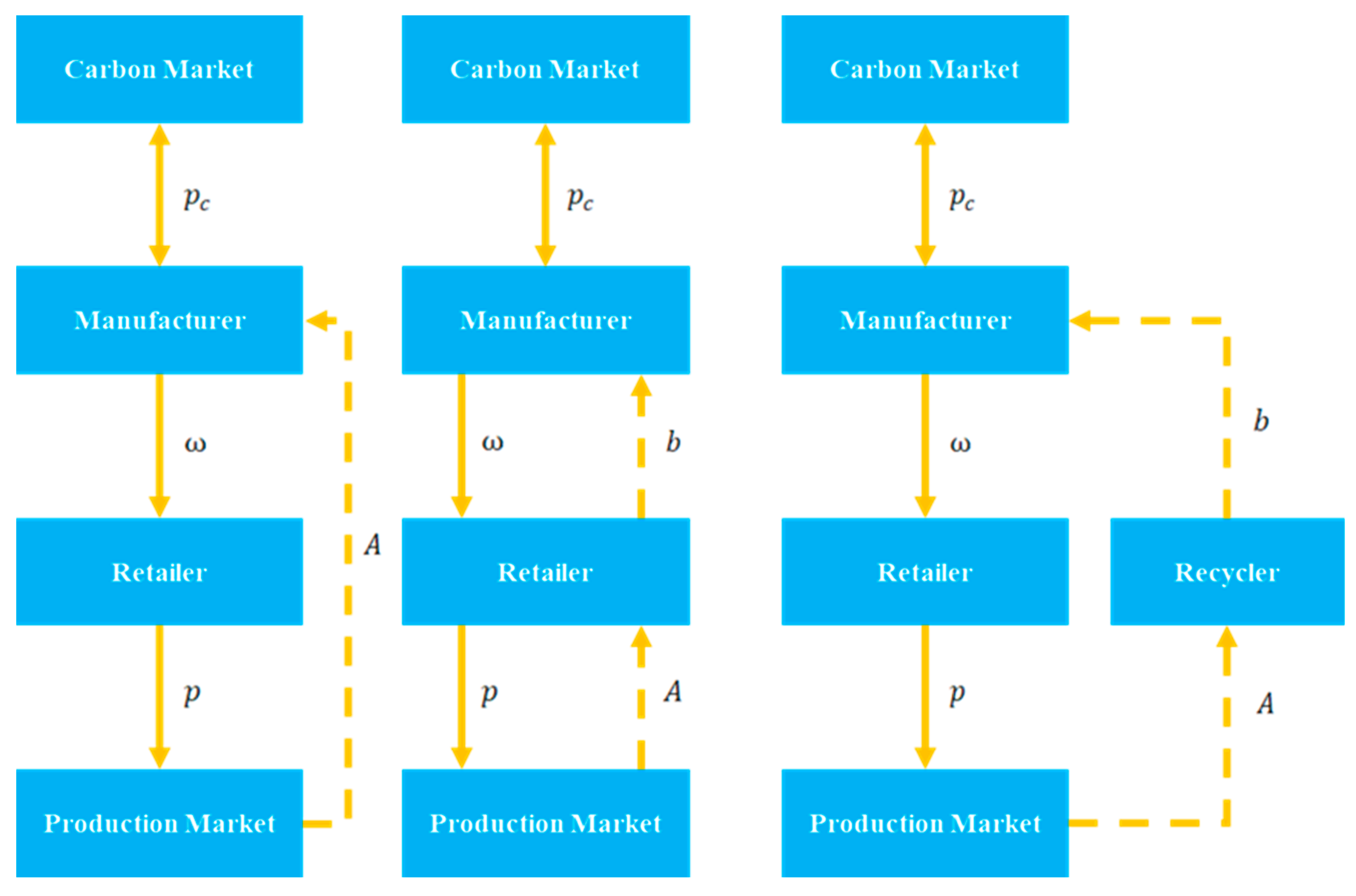
This paper studies closed-loop supply chain decision making under carbon allowance and carbon trading policies. Let ${\pi }_i^j$ denote the profit of enterprise $i$ under model $j$, max ${\pi }_i^j$ denote the optimal profit, and $x_j{}^{*}$ denote the optimal solution of parameter $x$ under the model $j$. This paper designs three models, namely, the manufacturer recycling model (M model), the retailer recycling model (R model) and the third-party recycler recycling model (T model). The schematic diagrams of the three models are shown in Figure 1.

4. Model Analysis

This paper develops a Stackelberg game-theoretic model to study the behavior of CLSC under carbon allowance and carbon trading policies, and to decide the choice of WEEE recycling channels. The Stackelberg game is a two-stage dynamic game with complete information. The main idea is that both sides choose their own strategies according to each other’s possible strategies, and maximize their own interests under each other’s strategies, so as to reach Nash equilibrium. In the game model, the party that makes the decision first is called the leader. After the leader, the remaining players decide according to the leader’s decision, which is called the follower. Then, the leader adjusts his decision according to that of the followers. The players repeat the preceding operations until a Nash equilibrium is reached. In this paper, the manufacturer is the leader, while the retailer and the third-party recycler are the followers. In this section, the three recycling models are discussed respectively. No matter which model is used, the carbon emissions are

$$C{E}^j=q\left(1-\tau \right)e+q\tau \lambda e$$

4.1. M Model

In the M model, the manufacturer is responsible for recycling WEEE. The decision order is that the manufacturer decides the wholesale price $\omega$ and the recovery rate $\tau$, and then the retailer decides the retail price $p$.

The manufacturer’s profit function is

$${\pi }_M^M=q\omega -q\left(1-\tau \right){\mathrm{c}}_n-q\tau {\mathrm{c}}_r-\left(C_L{\tau }^2+q\tau A\right)+p_c\left[Q-q\tau l\lambda e-\left(1-\tau \right)qe\right]$$

(1)

In this formula, the first item to the right of the equal sign is the benefit of wholesaling products, the second item is the cost of manufacturing new products, the third item is the cost of remanufacturing products, the fourth item is the cost of recycling WEEE from the market and the last item is the carbon trading volume. When $Q>q\tau l\lambda e+\left(1-\tau \right)qe$, the manufacturer has remaining carbon credits, and the carbon trading revenue is positive. On the contrary, the manufacturer needs to purchase additional carbon credits from the carbon trading market, and the carbon trading revenue is negative.

The retailer’s profit function is

$${\pi }_R^M=q\left(p-\omega \right)$$

(2)

In this formula, the item to the right of the equal sign is the profit of retailing products to consumers. The relevant proof is shown in Appendix A.

Proposition 1.

In the M model, when$8C_L>\beta {\left(A-∆-e_0{p}_e+\lambda e_0{p}_e\right)}^2$, Equation (1) has a unique optimal solution; the optimal solution is

$${\mathsf{\omega }}_M^{*}=\frac{(-\mathsf{\beta }\varnothing {\left(\mathrm{A}-∆-\mathrm{e}{p}_c+\mathsf{\lambda }\mathrm{e}{p}_c\right)}^2+4{\mathsf{\beta }\mathrm{C}}_L{c}_n+4{\mathsf{\beta }\mathrm{C}}_L\mathrm{e}{p}_c+4\varnothing {\mathrm{C}}_L)}{\mathsf{\beta }[8{\mathrm{C}}_L-\mathsf{\beta }{\left(\mathrm{A}-∆-\mathrm{e}{p}_c+\mathsf{\lambda }\mathrm{e}{p}_c\right)}^2]}$$

$$p_M^{*}=\frac{(-\beta \varnothing {\left(A-∆-e{p}_c+\lambda e{p}_c\right)}^2+2\beta C_L{c}_n+2\beta C_{L}e{p}_c+6\varnothing C_L)}{\beta [8C_L-\beta {\left(A-∆-e{p}_c+\lambda e{p}_c\right)}^2]}$$

$${\tau }_M^{*}=\frac{\left(\beta c_n+\beta e{p}_c-\varnothing \right)\left(A-∆-e{p}_c+\lambda e{p}_c\right)}{8{\mathrm{C}}_L-\beta {\left(A-∆-e{p}_c+\lambda e{p}_c\right)}^2}$$

The optimal profits of the manufacturer and the retailer are respectively

$$max{\pi }_M^M=\frac{-{\beta }^2\varnothing p_c{\left(A-∆-e{p}_c+\lambda e{p}_c\right)}^2+\beta C_L\left(c_n+e{p}_c\right)\left(\beta c_n-2\varnothing +\beta e{p}_c\right)+8Q\beta C_L{p}_c+C_L{\varnothing }^2}{\beta [8C_L-\beta {\left(A-∆-e{p}_c+\lambda e{p}_c\right)}^2]}$$

$$max{\mathsf{\pi }}_R^M=\frac{4{\mathrm{C}}_L{}^2{\left(\mathsf{\beta }{c}_n-\varnothing +\mathsf{\beta }\mathrm{e}{p}_c\right)}^2}{\mathsf{\beta }{[8{\mathrm{C}}_L-\mathsf{\beta }{\left(\mathrm{A}-∆-\mathrm{e}{p}_c+\mathsf{\lambda }\mathrm{e}{p}_c\right)}^2]}^2}$$

The carbon emissions in M model are

$$C{E}^M=\frac{2{\mathrm{eC}}_L\left(\mathsf{\beta }{c}_n-\varnothing +\mathsf{\beta }\mathrm{e}{p}_c\right)\left[\left(A-∆-e{p}_c+\lambda e{p}_c\right)\left(\mathsf{\beta }\mathrm{A}-\varnothing +\mathsf{\beta }{c}_r+\varnothing \mathsf{\lambda }-\mathsf{\beta }{c}_n\mathsf{\lambda }\right)-8{\mathrm{C}}_L\right]}{{(8{\mathrm{C}}_L-\mathsf{\beta }{\left(\mathrm{A}-∆-\mathrm{e}{p}_c+\mathsf{\lambda }\mathrm{e}{p}_c\right)}^2)}^2}$$

Corollary 1.

In the M model, when the recycling rate of WEEE${\tau }_M^{*}<0.5$, the wholesale price${\omega }_M^{*}$, the retail price$p_M^{*}$, the manufacturer’s profit$max{\pi }_M^M$and the retailer’s profit$max{\pi }_R^M$increase with the increase of the carbon trading price$p_c$or unit carbon emission$e$.

Corollary 2.

In the M model, when${\tau }_M^{*}\ge 0.5$, if$\lambda >1-\frac{1}{2{\tau }_M^{*}}$, with the increase of$p_c$or$e$,${\omega }_M^{*}$,$p_M^{*}$,$max{\pi }_M^M$and$max{\pi }_R^M$increase accordingly.

Corollary 1 shows that when the recycling rate is low, the manufacturer can only carry out remanufacturing activities in a low proportion, and the contribution to cost savings is limited. With the growth of carbon price or carbon emissions per unit of product, if the manufacturer adopts a strategy of small profits, but quick turnover, they will inevitably spend too much on purchasing extra carbon quotas. Therefore, with the increase of the carbon cost, the manufacturer will raise the wholesale price, increase the profit of individual products, reduce the market size, and sell abundant carbon credits to obtain more extra profits. At this time, the marginal revenue of carbon trading is greater than that of the products. In the M model, carbon trading and recycling activities are not directly related to the retailer. With the increase in the wholesale price, the retailer will also raise the retail price to maintain profits. When the price increase is too high, customers’ sensitivity to the price decreases, so the retail price increase will be higher than the wholesale price increase, and the retailer’s profit will be further improved. Corollary 2 shows that when the carbon emission reduction intensity coefficient of remanufacturing is greater than the threshold, the contribution of remanufacturing to carbon emission reduction is low. Under the condition of limited carbon allowance, the manufacturer cannot produce more products, and with the increase in carbon cost, the wholesale price will also be raised to maintain its profit.

4.2. R Model

In the R model, the retailer is responsible for recycling WEEE. The decision order is that the manufacturer decides the wholesale price $\mathsf{\omega }$, and then the retailer decides the retail price $p$ and the recovery rate $\tau$.

The manufacturer’s profit function is

$${\pi }_M^R=q\omega -q\left(1-\tau \right){\mathrm{c}}_n-q\tau {\mathrm{c}}_r-q\tau b+p_c\left[Q-q\tau l\lambda e-\left(1-\tau \right)qe\right]$$

(3)

In this formula, the first item to the right of the equal sign is the benefit of wholesaling products, the second item is the cost of manufacturing new products, the third item is the cost of remanufacturing products, the fourth item is the cost of buying WEEE from the retailer and the last item is the carbon trading volume. When $Q>q\tau l\lambda e+\left(1-\tau \right)qe$, the manufacturer has remaining carbon credits and the carbon trading revenue is positive. On the contrary, the manufacturer needs to purchase additional carbon credits from the carbon trading market, and the carbon trading revenue is negative.

The retailer’s profit function is

$${\pi }_R^R=q\left(p-\omega \right)-\left(C_L{\tau }^2+q\tau A\right)+q\tau b$$

(4)

In this formula, the first item to the right of the equal sign is the profit of retailing products to consumers, the second item is the cost of recycling WEEE from the customers and the third item is the retailer’s benefit of transferring WEEE to the manufacturer.

Proposition 2.

In the R model, when$4C_L>\beta \left(A-b\right)\left(A-∆-e{p}_c+\lambda e{p}_c\right)$, Equation (3) has a unique optimal solution; the optimal solution is

$${\mathsf{\omega }}_R^{*}=\frac{-{\beta }^2{\left(A-b\right)}^2\left(c_n+e{p}_c\right)-\beta \varnothing \left(A-b\right)\left(A+b-2∆-2e{p}_c+2\lambda e{p}_c\right)+4C_L\left(\beta c_n+\beta e{p}_c+\varnothing \right)}{2\mathsf{\beta }\left[4C_L-\mathsf{\beta }\left(A-b\right)\left(A-∆-e{p}_c+\lambda e{p}_c\right)\right]}$$

$$p_R^{*}=\frac{-\beta \varnothing \left(A-b\right)\left(A-∆-e{p}_c+\lambda e{p}_c\right)+C_L\left(\beta c_n+\beta e{p}_c+3\varnothing \right)}{\beta \left[4C_L-\mathsf{\beta }\left(A-b\right)\left(A-∆-e{p}_c+\lambda e{p}_c\right)\right]}$$

$${\tau }_R^{*}=\frac{\left(\beta c_n+\beta e{p}_c-\varnothing \right)\left(A-b\right)}{2\left[4C_L-\mathsf{\beta }\left(A-b\right)\left(A-∆-e{p}_c+\lambda e{p}_c\right)\right]}$$

The optimal profits of the manufacturer and the retailer are, respectively,

$$max{\pi }_M^R=\frac{2Q\beta p_c\left[4C_L-\mathsf{\beta }\left(A-b\right)\left(A-∆-e{p}_c+\lambda e{p}_c\right)\right]+C_L{\left(\beta c_n+\beta e{p}_c-\varnothing \right)}^2}{2\beta \left[4C_L-\mathsf{\beta }\left(A-b\right)\left(A-∆-e{p}_c+\lambda e{p}_c\right)\right]}$$

$$max{\mathsf{\pi }}_R^R=\frac{{\mathrm{C}}_L{\left(\mathsf{\beta }{c}_n-\varnothing +\mathsf{\beta }\mathrm{e}{p}_c\right)}^2\left(4{\mathrm{C}}_L-\mathsf{\beta }{\left(A-b\right)}^2\right)}{4\mathsf{\beta }{[4C_L-\mathsf{\beta }\left(A-b\right)\left(A-∆-e{p}_c+\lambda e{p}_c\right)]}^2}$$

The carbon emissions in the R model are

$$C{E}^R=\frac{{\mathrm{C}}_{L}e[\left(-\mathsf{\beta }{c}_n+\varnothing -\mathsf{\beta }\mathrm{e}{p}_c\right)\left(8C_L-\varnothing \left(\mathrm{A}-\mathrm{b}\right)\left(\lambda -1\right)-\mathsf{\beta }\left(\mathrm{A}-\mathrm{b}\right)\left(2A-∆+c_r-c_n\lambda -e{p}_c+\lambda e{p}_c\right)\right]}{2{\left[4C_L-\mathsf{\beta }\left(A-b\right)\left(A-∆-e{p}_c+\lambda e{p}_c\right)\right]}^2}$$

Corollary 3.

In the R model, as the carbon trading price$p_c$increases, the recycling rate of WEEE${\tau }_R^{*}$decreases, and the wholesale price${\omega }_R^{*}$and retail price$p_R^{*}$of the products increase.

Corollary 4.

In the R model, as the carbon trading price$p_c$increases, the profit of manufacturer$max{\pi }_M^R$and the profit of retailer$max{\pi }_R^R$increase.

Corollaries 3 and 4 show that with the increase in carbon trading price, the manufacturer is reluctant to purchase quotas in the carbon market to increase production, so they will reduce market demand by increasing the wholesale price of the products. Although the cost of carbon trading increases, the marginal profit of selling the product is greater than the marginal cost of carbon trading, so the profit of the manufacturer increases. As the manufacturer increases the wholesale price, the retailer will also increase the retail price in order to maintain profit. At the same time, for the retailer, the market demand is low, and excessive recycling of WEEE will result in excessive recycling effort costs, but the recycling volume is deficient, so the recovery rate will decrease. When the retail price is too high, customers are less sensitive to the price, so the increase in the retail price will be higher than the increase in the wholesale price, and the retailer’s profit will further increase.

4.3. T Model

In the T model, the third-party recycler is responsible for recycling WEEE. The decision order is that the manufacturer decides the wholesale price $\mathsf{\omega }$, the retailer decides the retail price $p$, and the third-party recycler decides the recovery rate $\tau$.

The manufacturer’s profit function is

$${\pi }_M^T=q\omega -q\left(1-\tau \right){\mathrm{c}}_n-q\tau {\mathrm{c}}_r-q\tau b+p_c\left[Q-q\tau l\lambda e-\left(1-\tau \right)qe\right]$$

(5)

In this formula, the first item to the right of the equal sign is the benefit of wholesaling products, the second item is the cost of manufacturing new products, the third item is the cost of remanufacturing products, the fourth item is the cost of buying WEEE from the retailer and the last item is the carbon trading volume. When $Q>q\tau l\lambda e+\left(1-\tau \right)qe$, the manufacturer has remaining carbon credits, and the carbon trading revenue is positive. On the contrary, the manufacturer needs to purchase additional carbon credits from the carbon trading market, and the carbon trading revenue is negative.

The retailer’s profit function is

$${\pi }_R^T=q\left(p-\omega \right)$$

(6)

In this formula, the item to the right of the equal sign is the profit of retailing products to consumers.

The third-party recycler’s profit function is

$${\pi }_T^T=q\tau b-q\tau A-C_L{\tau }^2$$

(7)

In this formula, the first item to the right of the equal sign is the cost of recycling WEEE from the customers, the second item is the retailer’s benefit of transferring WEEE to the manufacturer and the third item is the recovery effort cost.

Proposition 3.

In the T model, the optimal solution is

$${\mathsf{\omega }}_T^{*}=\frac{2C_L\left(c_n+\beta e{p}_c+\varnothing \right)-\beta \varnothing \left(A-b\right)\left(b-∆-e{p}_c+\lambda e{p}_c\right)}{\mathsf{\beta }\left[4C_L-\mathsf{\beta }\left(A-b\right)\left(b-∆-e{p}_c+\lambda e{p}_c\right)\right]}$$

$$p_T^{*}=\frac{C_L\left(c_n+\beta e{p}_c+3\varnothing \right)-\beta \varnothing \left(A-b\right)\left(b-∆-e{p}_c+\lambda e{p}_c\right)}{\beta \left[4C_L-\mathsf{\beta }\left(A-b\right)\left(b-∆-e{p}_c+\lambda e{p}_c\right)\right]}$$

$${\tau }_T^{*}=\frac{\left(\beta c_n+\beta e{p}_c-\varnothing \right)\left(A-b\right)}{2\left[4C_L-\mathsf{\beta }\left(A-b\right)\left(b-∆-e{p}_c+\lambda e{p}_c\right)\right]}$$

The optimal profits of the manufacturer, the retailer and the third-party recycler are, respectively,

$$max{\pi }_M^T=\frac{2Q\beta p_c\left[4C_L-\mathsf{\beta }\left(A-b\right)\left(b-∆-e{p}_c+\lambda e{p}_c\right)\right]+C_L{\left(\beta c_n+\beta e{p}_c-\varnothing \right)}^2}{2\beta \left[4C_L-\mathsf{\beta }\left(A-b\right)\left(b-∆-e{p}_c+\lambda e{p}_c\right)\right]}$$

$$max{\mathsf{\pi }}_R^T=\frac{{\mathrm{C}}_L{}^2{\left(\mathsf{\beta }{c}_n-\varnothing +\mathsf{\beta }\mathrm{e}{p}_c\right)}^2}{\mathsf{\beta }{[4C_L-\mathsf{\beta }\left(A-b\right)\left(b-∆-e{p}_c+\lambda e{p}_c\right)]}^2}$$

$$max{\mathsf{\pi }}_T^T=\frac{{\mathrm{C}}_L{\left(\mathsf{\beta }{c}_n-\varnothing +\mathsf{\beta }\mathrm{e}{p}_c\right)}^2{\left(A-b\right)}^2}{4{[4C_L-\mathsf{\beta }\left(A-b\right)\left(b-∆-e{p}_c+\lambda e{p}_c\right)]}^2}$$

The carbon emissions in the T model are

$$C{E}^T=\frac{{\mathrm{C}}_{L}e\left[-\mathsf{\beta }{c}_n+\varnothing -\mathsf{\beta }\mathrm{e}{p}_c\right)\left(8C_L-\varnothing \left(\mathrm{A}-\mathrm{b}\right)\left(\lambda -1\right)-\mathsf{\beta }\left(\mathrm{A}-\mathrm{b}\right)\left(2b-∆+c_r-c_n\lambda -e{p}_c+\lambda e{p}_c\right)\right]}{2{\left[4C_L-\mathsf{\beta }\left(A-b\right)\left(b-∆-e{p}_c+\lambda e{p}_c\right)\right]}^2}$$

Corollary 5.

In the T model, with the increase of carbon trading price$p_c$, the recycling rate of WEEE${\tau }_R^{*}$decreases, and the wholesale price${\omega }_R^{*}$and retail price$p_R^{*}$of the products increase.

Corollary 6.

In the T model, with the increase of carbon trading price$p_c$, the profit of the third-party recycler$max{\pi }_T^T$decreases, and the profit of manufacturer$max{\pi }_M^T$and the profit of retailer$max{\pi }_R^T$increase.

Corollaries 5 and 6 show that with the increase in the carbon trading price, the manufacturer is reluctant to purchase quotas in the carbon market to increase production, so they will reduce market demand by increasing the wholesale price of products. Although the cost of carbon trading increases, the marginal profit of selling the product is greater than the marginal cost of carbon trading, so the profit of the manufacturer increases. As the manufacturer increases the wholesale price, the retailer will also increase the retail price in order to maintain profit. When the retail price is too high, customers are less sensitive to the price, so the increase in the retail price will be higher than the increase in the wholesale price, and the retailer’s profit will further increase. At the same time, for the third-party recycler, the market demand is low, and excessive recycling of WEEE will result in excessive recycling effort costs, but the recycling volume is deficient, so the recovery rate will decrease. As the recycling rate of WEEE decreases, the third-party recycler’s profit decreases.

5. Comparison of Economic Benefit and Carbon Reduction Benefit

In Section 4, we made decisions on CLSC using three different models. In this section, we compare the economic benefit and carbon reduction benefits of the optimal decision results.

Proposition 4.

The manufacturer is the dominant player in CLSC. In the M model, its profit is the highest, and in the T model, its profit is the lowest. That is$max{\pi }_M^M>max{\pi }_M^R>max{\pi }_M^T$.

Proposition 5.

In the M model, the manufacturer’s carbon emission is the largest, in the R model the manufacturer’s carbon emission is the lowest. That is$C{E}^M>C{E}^T>C{E}^R$.

Proposition 4 indicates that the manufacturer controls the recycling right in his own hands, and it will obtain the maximum profit. This also shows that the more variables the manufacturer can make decisions with, the more beneficial it is to increase its profit. Proposition 5 shows that the manufacturer will not pursue carbon reduction in pursuit of maximum profit. In the M model, the manufacturer makes the most profit at the cost of the most significant carbon emissions. This is not what the government wants to see, and it is bound to intervene in its recycling activities. In reality, will the government choose the R model with the smallest carbon footprint? It is unlikely. Generally speaking, in order to expand its profit, the manufacturer usually builds their own retail systems or directly participates in the decision-making activities of the retailer, such as Apple or Huawei. It is difficult for the government to intervene in the decision-making behavior of the retailer. Therefore, the government should choose the T model to control the recycling channel of WEEE, so as to encourage the manufacturers to maximize the reduction of carbon emissions.

6. Numerical Example Simulation

This section discusses the manufacturer’s profit and carbon emissions through a numerical case. It is assumed that the relevant parameters are as follows: $\varnothing =200$, $c_n=30$, $c_r=10$, $\beta =0.5$, $A=5$, $b=7$, $C_L=100$, $Q=700$, $e=5$, and $\lambda =0.6$. After the assignment, we can visually compare the profit of the manufacturer and carbon emissions under different recycling scenarios under the carbon allowance and carbon trading policies.

Figure 2 shows that with the carbon trading price increases, the manufacturer’s maximum profit also increases. In the M model, the profit of the manufacturer is the largest, and its slope with the increase in carbon price is also the largest. The manufacturer’s profit increase slope is the same in the R and T models, but the profit is slightly higher in the R model.

Figure 3 shows the profit comparison of CLSC under the three models. It can be seen from the figure that no matter which model is used, the profit of CLSC increases due to the increase of the carbon trading price. The profit of CLSC in the M model is the largest, and the profit of CLSC in the R model and the T model is very close.

Figure 4 shows a comparison of the manufacturer’s carbon emissions in the three models. As can be seen from the figure, in the M model, carbon emissions increase with the increase of the carbon trading price, while in the R model and the T model, carbon emissions decrease with the increase of the carbon trading price. The carbon emission in the M model is the largest, and the carbon emission in the R model is the smallest, which is consistent with the above conclusions. Compared with the R model, the carbon emission of the T model is similar, but far smaller than of the M model. It can be seen that the government’s choice of the T model can achieve a significant reduction in carbon emissions.

In Figure 2, Figure 3 and Figure 4, the horizontal axis represents the carbon trading price p, and the vertical axis represents the manufacturer’s optimal profit, CLSC’s most profitable, and carbon emissions.

From Figure 2 and Figure 3, we can see that when the manufacturer recycles WEEE, with the increase of the carbon trading price $p_c$, the profit and carbon emissions increase simultaneously. It can be considered that the manufacturer always puts the profit first in the balance between profit and carbon emissions. It can be seen from Figure 4 that when the retailer or the third-party recycler recycles WEEE, as the carbon trading price $p_c$ increases, carbon emissions decrease significantly, which indicates that the retailer and the third-party recycler act as checks and balances on the manufacturer, by reducing the manufacturer’s decision-making variables to let it give up some opportunity costs, thereby reducing carbon emissions.

7. Discussions

This paper considers the decision-making problem of CLSC when WEEE is recycled by the manufacturer, the retailer and the third-party recycler, respectively. By solving the three models, this paper draws a series of conclusions. Some of them can be corroborated by previous related research. Chen et al. [49] believe that under the conditions of carbon cap-and-trade supervision and recycling supervision, the introduction of remanufacturing links can effectively reduce carbon emissions in the production process and realize the recycling of resources. Li et al. proposed that under the carbon tax policy, enterprises are increasingly aware of incorporating corporate social responsibility (CSR) into their supply chain strategies, contributing to carbon reduction and the development of a circular economy. Modak and Kelle [50] proposed that under the carbon tax policy, enterprises are increasingly aware of incorporating corporate social responsibility (CSR) into their supply chain strategies, contributing to carbon reduction and the development of a circular economy. These studies are consistent with the findings of this paper, but they do not make concrete proposals for the government to further reduce carbon emissions. Based on the comparison of carbon emissions under the optimal decision making of various recycling models, this paper combines with the actual operating conditions of enterprises, proposing that the government should vigorously build third-party recycling channels to recycle WEEE.

From Propositions 1–3, it can be seen that the amount of carbon allowance does not affect the decision-making results. This is because businesses are profit-seeking by nature and tend to delve into government policies to find ways to make more profits. In order to achieve carbon neutrality, the government has formulated various related policies, among which carbon allowance and carbon trading are representative policies. In the process of business decision making, enterprises seek opportunities to obtain more profits. When the carbon allowance allocated to the enterprise by the government is high, the enterprise will trade the rich quotas as new income. When the carbon allowance allocated to the enterprise is low, the enterprise will not limit the production capacity to the maximum; instead, it will obtain more production opportunities through lower carbon trading prices, and pursue profit growth in the product trading market.

8. Conclusions

Carbon allowance and carbon trading policies affect the profits, decision making and recycling channel choices of enterprises in CLSC. Although scholars have previously studied the recycling model of CLSC in different scenarios, and carbon allowance and carbon trading policies have been proven to be effective in reducing carbon emissions, few studies have considered the roles of both in CLSC.

This paper studied the effects of different recycling models, carbon emission allowance and carbon trading policies on CLSC. Firstly, three CLSC models under different recycling channels were established. Then, the influence of carbon allowance and carbon trading policies on the wholesale price, selling price and recovery rate of CLSC was studied. In addition, we compared and analyzed the profit and the carbon emissions of CLSC under three recycling models. In summary, the following conclusions are drawn. (1) When the manufacturer is responsible for recycling WEEE, both it and CLSC can obtain the maximum profit. At this time, the pursuit of profit is the primary goal of the manufacturer. The manufacturer will not overly consider reducing carbon emissions, and carbon trading is only a means to balance profits. As the trading price of carbon rises, the profit of the manufacturer will also increase. (2) Carbon emissions are the lowest when the retailer is responsible for recycling WEEE. In the R model, the manufacturer cannot interfere with the recovery rate of WEEE, and carbon trading changes from active to passive, which is more conducive to reducing carbon emissions. (3) The manufacturer is more inclined to opt for the M model, but this model is not as effective in reducing carbon emissions. As most manufacturers usually set up their own retail systems, the retailer’s decisions will be influenced by the manufacturer. The T model can also effectively reduce carbon emissions. Compared with the R model, the improvement is not significant, so the government is more inclined to choose the T model.

This paper can obtain the following management inspiration and theoretical significance. Considering the actual remanufacturing cost savings and recycling benefits, the supply chain can choose appropriate WEEE for recycling to achieve carbon emission reduction and maximize the profit of CLSC. When the retailer or the third-party recycler is responsible for recycling WEEE, carbon emission levels can be significantly reduced. However, considering that the retailer usually receives interference or constraints from the manufacturer in the decision-making process, the government should build third-party recycling channels, while regulating and controlling carbon trading prices to stimulate CLSC to recycle more WEEE and reduce more carbon emissions.

Further research can be conducted on the following aspects. Carbon emission reduction can usually be carried out from the management level and the technical perspective. This paper discusses the decision-making research of CLSC under carbon allowance and carbon trading policies, and seeks the optimal recycling channel for carbon emission reduction from a management perspective. Future research can introduce the technical perspective to explore the improvement of CLSC recycling technology, that is, to further control the unit carbon emission reduction intensity coefficient, so as to explore the optimal carbon emission reduction CLSC from the perspective of technology management. In addition, recycling WEEE and remanufacturing can not only reduce manufacturing costs, but also contribute to reducing carbon emissions. This is mainly due to two aspects: firstly, if WEEE is treated as garbage, it will emit carbon. If left it unchecked, it will pollute resources such as land, water and atmosphere. Secondly, manufacturing enterprises make full use of the residual value of WEEE, extract materials from WEEE that can be reused in the manufacture of new products, and turn waste into valuable raw materials. Improving the processing level of WEEE in CLSC is an effective way to increase profits and reduce carbon emissions. In this paper, recycled WEEE is considered to be indistinguishable, they have the same residual value and can produce the same carbon reduction effect for manufacturing, which is a serious deviation from practice. Therefore, in future research, we will further focus on the processing of WEEE from both technical and management perspectives. From a technical standpoint, enterprises should improve the level of WEEE recycling and remanufacture in order to maximize the utilization of WEEE. From the management perspective, the residual value and residual carbon emissions of WEEE should be classified and different recovery methods used for the remaining WEEE with different residual values and carbon emissions.