Cyclic Direct Shear Testing of a Sand with Waste Tires
Abstract
:1. Introduction
2. Experimental Study
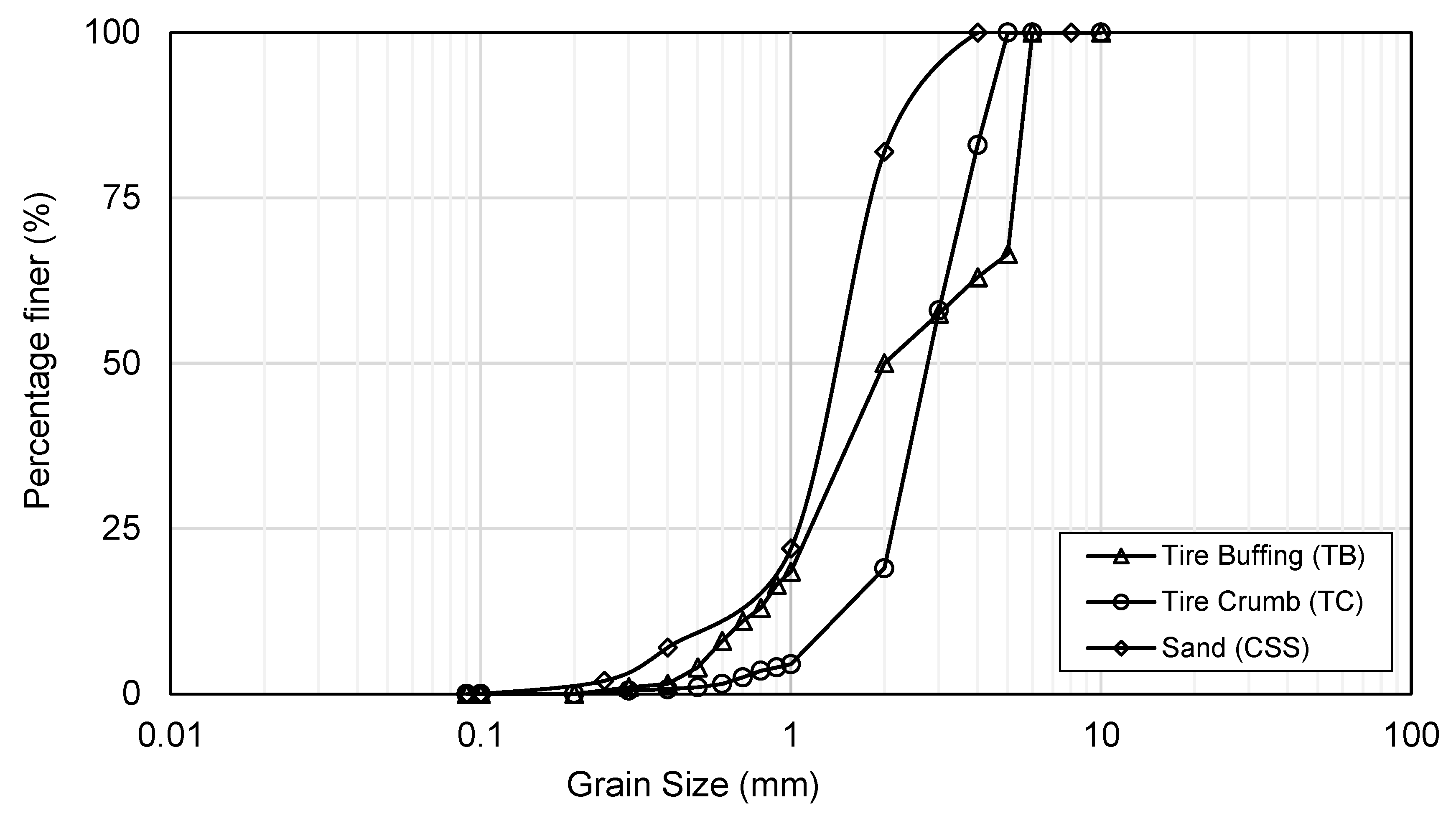
2.1. Materials
2.2. Specimen Preparation and Testing Program
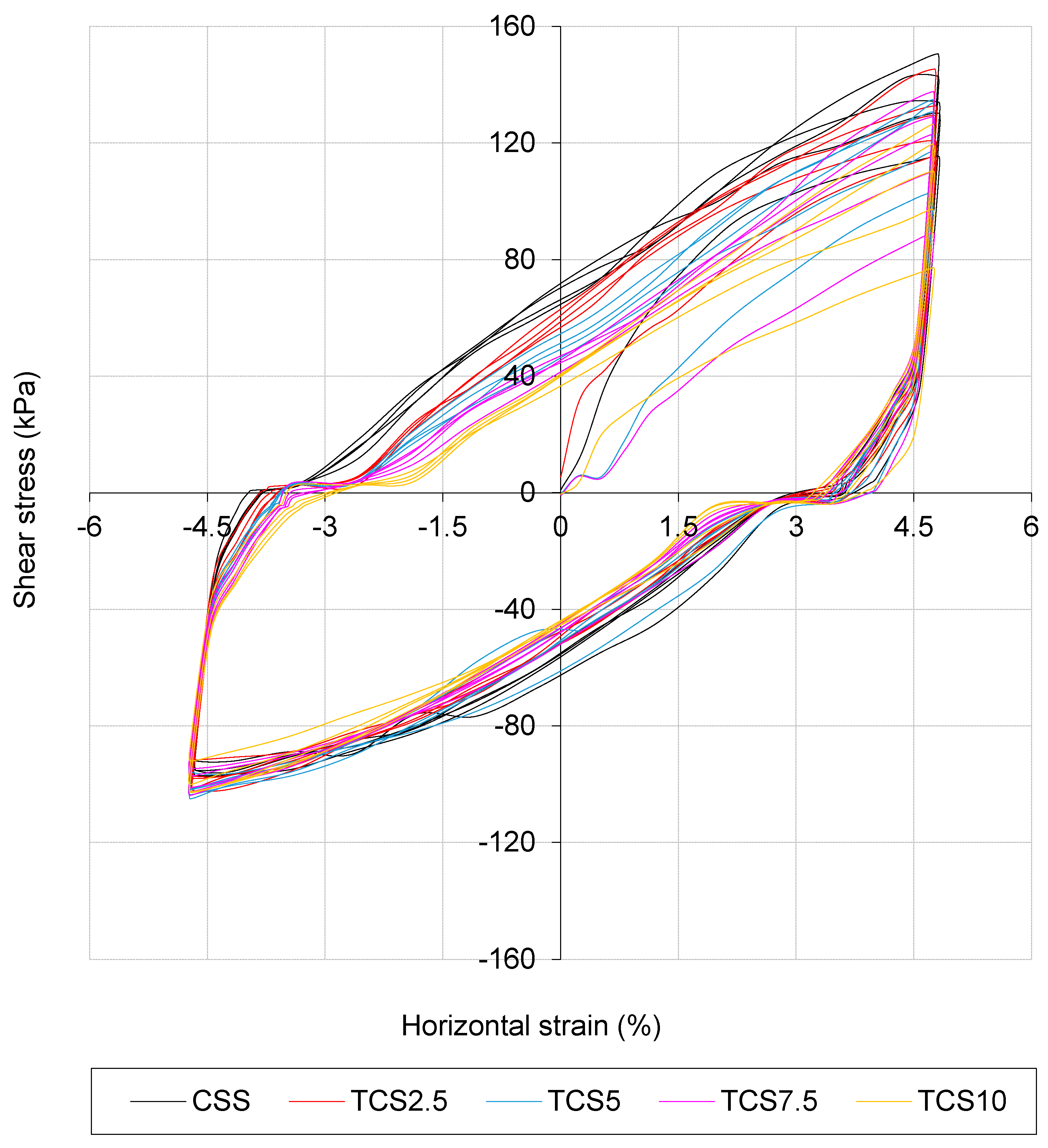
3. Results and Discussion
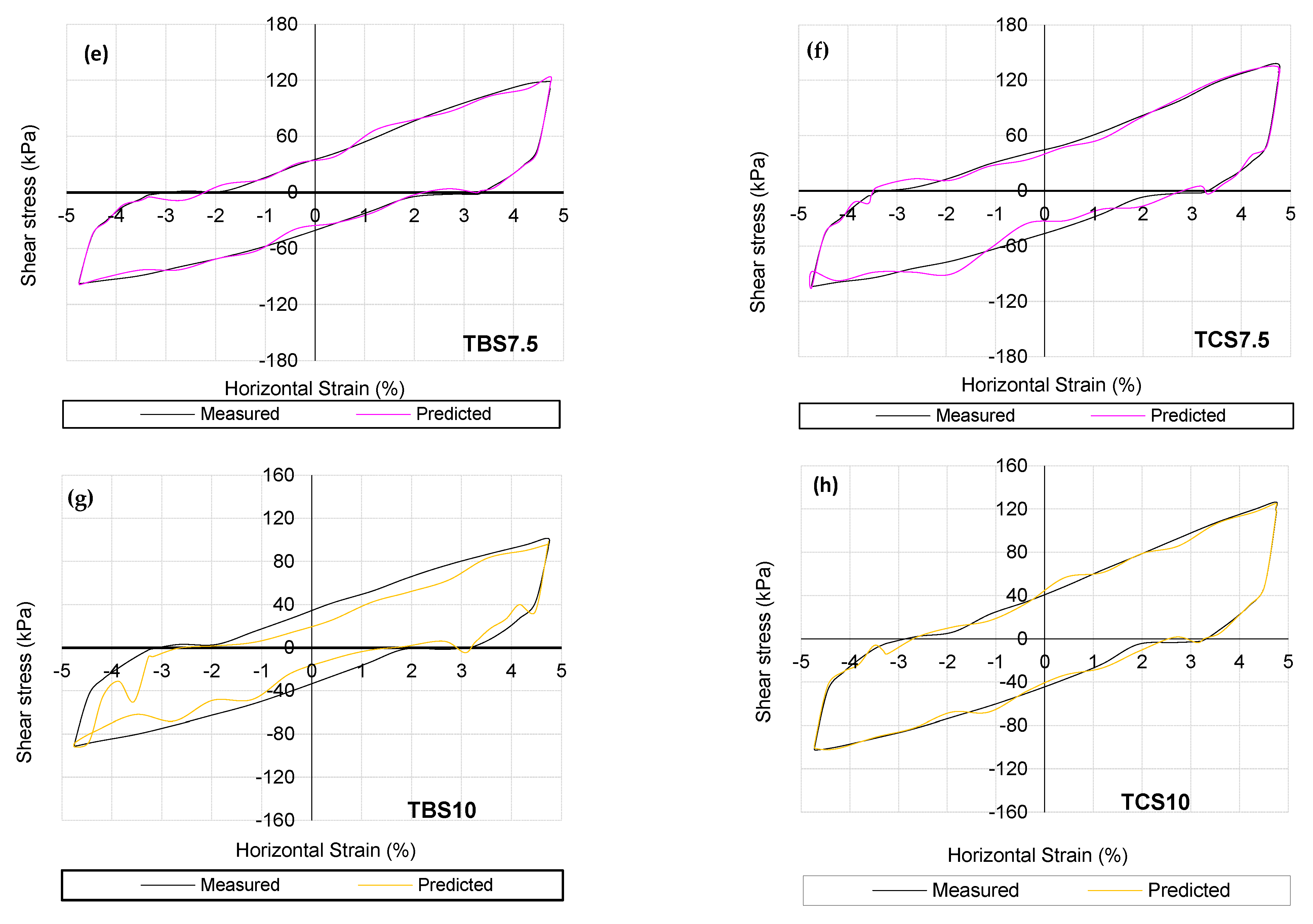
4. Prediction Model
5. Conclusions
- -
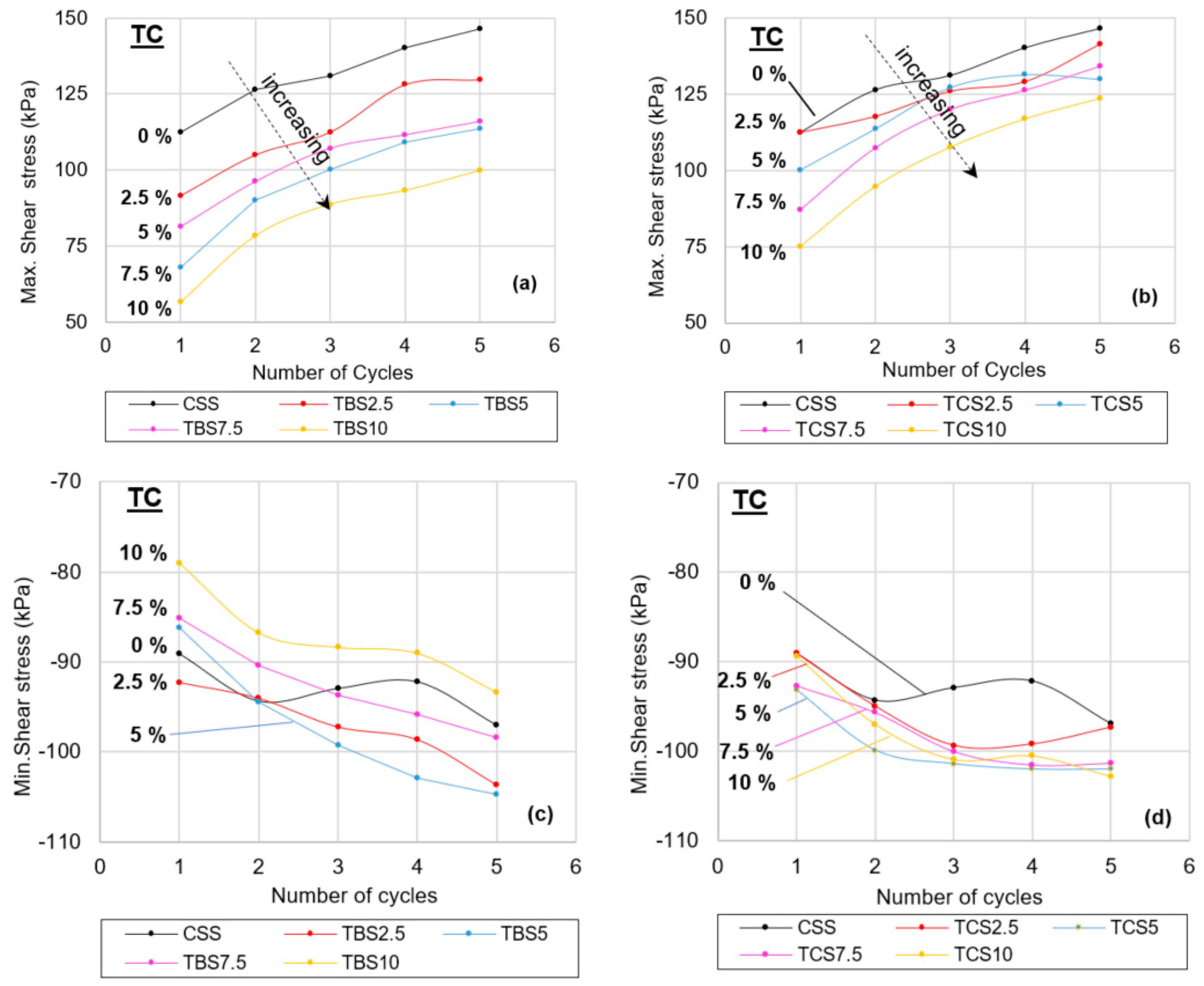
- The addition of waste tire to sand decreased the horizontal stress amplitudes, i.e., higher tire content in the mixture induced lower shear strength. Thereby, waste tire-sand mixtures have smaller values of shear modulus than the clean sand specimen.
- -
- At each additive content, the reduction effect of tire buffing inclusion into sand is higher than that of tire crumb, which is attributed to different grain size and shape properties of the waste tires.
- -
- The cyclic shear test with different processed tires showed that the behavior of waste tire-sand mixtures is significantly affected by the content, grain shape, and aspect ratio of waste tire grains.
- -
- With the developed prediction model, it is deduced that the artificial intelligence-based prediction models can be used conveniently in the prediction of cyclic behavior of waste tire-sand mixtures. This is highly dependent on the quality of the data used.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Potyondy, J.G. Skin Friction between Various Soils and Construction Materials. Géotechnique 1961, 11, 339–353. [Google Scholar] [CrossRef]
- Knodel, P.; Al-Douri, R.; Poulos, H. Static and Cyclic Direct Shear Tests on Carbonate Sands. Geotech. Test. J. 1992, 15, 138–157. [Google Scholar] [CrossRef]
- Desai, C.S.; Drumm, E.C.; Zaman, M.M. Cyclic Testing and Modeling of Interfaces. J. Geotech. Eng. 1985, 111, 793–815. [Google Scholar] [CrossRef]
- Tsubakihara, Y.; Kishida, H. Frictional Behaviour between Normally Consolidated Clay and Steel by Two Direct Shear Type Apparatuses. Soils Found. 1993, 33, 1–13. [Google Scholar] [CrossRef] [Green Version]
- Fakharian, K.; Evgin, E. Simple Shear Versus Direct Shear Tests on Interfaces during Cyclic Loading. In Proceedings of the Third International Conference on Recent Advances in Geotechnical Earthquake Engineering and Soil Dynamics, St. Louis, MO, USA, 2–7 April 1995; Volume 4, pp. 13–16. [Google Scholar]
- Mortara, G.; Ferrara, D.; Fotia, G. Simple Model for the Cyclic Behavior of Smooth Sand-Steel Interfaces. J. Geotech. Geoenvironmental Eng. 2010, 136, 1004–1009. [Google Scholar] [CrossRef]
- Cabalar, A.F. Stress fluctuations in granular material response during cyclic direct shear test. Granul. Matter 2015, 17, 439–446. [Google Scholar] [CrossRef]
- Cabalar, A.F. Cyclic behavior of various sands and structural materials interfaces. Géoméch. Eng. 2016, 10, 1–19. [Google Scholar] [CrossRef]
- Ying, M.; Liu, F.; Wang, J.; Wang, C.; Li, M. Coupling effects of particle shape and cyclic shear history on shear properties of coarse-grained soil–geogrid interface. Transp. Geotech. 2021, 27, 100504. [Google Scholar] [CrossRef]
- Pra-Ai, S.; Boulon, M. Soil–structure cyclic direct shear tests: A new interpretation of the direct shear experiment and its application to a series of cyclic tests. Acta Geotech. 2017, 12, 107–127. [Google Scholar] [CrossRef]
- Fakharian, K.; Evgin, E. A Three-Dimensional Apparatus for Cyclic Testing of Interfaces. In Proceedings of the 46th Annual Canadian Geotechnical Conference, Saskatoon, SK, Canada, 27–29 September 1993; pp. 485–493. [Google Scholar]
- Stark, T.D.; Williamson, T.A.; Eid, H.T. HDPE Geomembrane/Geotextile Interface Shear Strength. J. Geotech. Eng. 1996, 122, 197–203. [Google Scholar] [CrossRef]
- Rui, S.; Wang, L.; Guo, Z.; Zhou, W.; Li, Y. Cyclic behavior of interface shear between carbonate sand and steel. Acta Geotech. 2021, 16, 189–209. [Google Scholar] [CrossRef]
- Hazarika, H.; Pasha, S.M.K.; Ishibashi, I.; Yoshimoto, N.; Kinoshita, T.; Endo, S.; Karmokar, A.K.; Hitosugi, T. Tire-chip reinforced foundation as liquefaction countermeasure for residential buildings. Soils Found. 2020, 60, 315–326. [Google Scholar] [CrossRef]
- Edincliler, A. Utilization of Waste Tires for Geotechnical Applications as Lightweight Materials. In Proceedings of the 9th International Symposium on Environmental Geotechnology and Global Sustainable Development, Hong Kong, China, 25–28 June 2008. [Google Scholar]
- Pincus, H.; Edil, T.; Bosscher, P. Engineering Properties of Tire Chips and Soil Mixtures. Geotech. Test. J. 1994, 17, 453. [Google Scholar] [CrossRef]
- Edinçliler, A.; Ayhan, V. Influence of tire fiber inclusions on shear strength of sand. Geosynth. Int. 2010, 17, 183–192. [Google Scholar] [CrossRef]
- Edinçliler, A.; Yildiz, O. Effects of processing type on shear modulus and damping ratio of waste tire-sand mixtures. Geosynth. Int. 2022, 29, 389–408. [Google Scholar] [CrossRef]
- Pistolas, G.A.; Anastasiadis, A.; Pitilakis, K.; Winter, M.G.; Smith, D.M.; Eldred, P.J.L.; Toll, D.G. Dynamic Properties of Gravel—Recycled Rubber Mixtures: Resonant Column and Cyclic Triaxial Tests. In Proceedings of the XVI ECSMGE Geotechnical Engineering for Infrastructure and Development, Edinburgh, UK, 13–17 September 2015; pp. 2613–2618. [Google Scholar]
- Bosscher, P.J.; Edil, T.B.; Kuraoka, S. Design of Highway Embankments Using Tire Chips. J. Geotech. Geoenvironmental Eng. 1997, 123, 295–304. [Google Scholar] [CrossRef]
- Tweedie, J.J.; Humphrey, D.N.; Sandford, T.C. Tire Shreds as Lightweight Retaining Wall Backfill: Active Conditions. J. Geotech. Geoenvironmental Eng. 1998, 124, 1061–1070. [Google Scholar] [CrossRef]
- Humphrey, D.N.; Whetten, N.; Weaver, J.; Recker, K.; Cosgrove, T.A. Tire TDA as Lightweight Fill for Embankments and Retaining Walls. In Proceedings of the Conference on Recycled Materials in Geotechnical Application, ASCE, Boston, MA, USA, 18–21 October 1998. [Google Scholar]
- Lee, J.H.; Salgado, R.; Bernal, A.; Lovell, C.W. Shredded Tires and Rubber-Sand as Lightweight Backfill. J. Geotech. Geoenvironmental Eng. 1999, 125, 132–141. [Google Scholar] [CrossRef]
- Dickson, T.H.; Dwyer, D.F.; Humphrey, D.N. Prototype Tire-Shred Embankment Construction. Transp. Res. Rec. J. Transp. Res. Board 2001, 1755, 160–167. [Google Scholar] [CrossRef]
- Zornberg, J.G.; Cabral, A.R.; Viratjandr, C. Behaviour of tire shred-sand mixtures. Can. Geotech. J. 2004, 41, 227–241. [Google Scholar] [CrossRef]
- Hazarika, H.; Yasuhara, K.; Hyodo, M.; Karmokar, A.K.; Mitarai, Y. Shaking Table Test on Liquefaction Prevention Using tire Chips and Sand Mixture. In Proceedings of the International Workshop on Scrap Tire Derived Geomaterials—Opportunities and Challenges, Yokosuka, Japan, 23–24 March 2007. [Google Scholar]
- Hazarika, H.; Yasuhara, K.; Hyodo, M.; Karmokar, A.K.; Mitarai, Y. Mitigation of Earthquake Induced Geotechnical Disasters Using a Smart and Novel Geomaterial. In Proceedings of the 14th World Conference on Earthquake Engineering, Beijing, China, 12–17 October 2008; The International Association for Earthquake Engineering (IAEE): Tokyo, Japan, 2008. [Google Scholar]
- Tsang, H.-H. Seismic isolation by rubber–soil mixtures for developing countries. Earthq. Eng. Struct. Dyn. 2007, 37, 283–303. [Google Scholar] [CrossRef]
- Senetakis, K.; Anastasiadis, A.; Trevlopoulos, K.; Souli, A. Dynamic Response of SDOF Systems on Soil Replaced with Sand/Rubber Mixture. In Proceedings of the ECOMAS Thematic Conference on Computation Methods in Structural Dynamics and Earthquake Engineering, Island of Rhodes, Greece, 17–19 June 2009; pp. 22–24. [Google Scholar]
- Senetakis, K.; Anastasiadis, A.; Pitilakis, K. Dynamic properties of dry sand/rubber (SRM) and gravel/rubber (GRM) mixtures in a wide range of shearing strain amplitudes. Soil Dyn. Earthq. Eng. 2012, 33, 38–53. [Google Scholar] [CrossRef]
- Senetakis, K.; Anastasiadis, A.; Pitilakis, K.; Souli, A.; Edil, T.; Dean, S.W. Dynamic Behavior of Sand/Rubber Mixtures, Part II: Effect of Rubber Content on G/GO-γ-DT Curves and Volumetric Threshold Strain. J. ASTM Int. 2012, 9, 1–12. [Google Scholar] [CrossRef]
- Senetakis, K.; Anastasiadis, A. Effects of state of test sample, specimen geometry and sample preparation on dynamic properties of rubber–sand mixtures. Geosynth. Int. 2015, 22, 301–310. [Google Scholar] [CrossRef] [Green Version]
- Mavronicola, E.; Komodromos, P.; Charmpis, D.C. Numerical Investigation of Potential Usage of Rubber-Soil Mixtures as a Distributed Seismic Isolation Approach. In Proceedings of the 10th International Conference on Computational Structures Technology, Stirlingshire, UK, 14–17 September 2010. [Google Scholar] [CrossRef]
- Perez, J.C.L.; Kwok, C.Y.; Senetakis, K. Investigation of the micro-mechanics of sand–rubber mixtures at very small strains. Geosynth. Int. 2017, 24, 30–44. [Google Scholar] [CrossRef] [Green Version]
- Tsang, H.-H.; Lo, S.H.; Xu, X.; Sheikh, M.N. Seismic isolation for low-to-medium-rise buildings using granulated rubber-soil mixtures: Numerical study. Earthq. Eng. Struct. Dyn. 2012, 41, 2009–2024. [Google Scholar] [CrossRef]
- Edincliler, A.; Yildiz, O. Numerical Study on Seismic Isolation for Medium-rise Buildings Using Rubber-Sand Mixtures. In Proceedings of the 4th Conference on Smart Monitoring, Assesment and Rehabilitation of Civil Structures, Zurich, Switzerland, 13–15 September 2017. [Google Scholar]
- Edincliler, A.; Yıldız, Ö. Numerical Study on Effects of Geotechnical Isolation Material on Seismic Performance of Medium Rise Buildings. In Proceedings of the 4th Eurasian Conference on Civil and Environmental Engineering, Istanbul, Turkey, 17–18 June 2019. [Google Scholar]
- Edincliler, A.; Yıldız, Ö. Seismic Behavior of Tire Waste-Sand Mixtures for Transporation Infrastructure in Cold Regions. Sci. Cold Arid. Reg. 2015, 7, 626–631. Available online: http://www.scar.ac.cn/EN/article/advancedSearchResult.do (accessed on 1 November 2022).
- Yildiz, Ö. Numerical Analysis of Geotechnical Seismic Isolation System for High-Rise Buildings. Naturengs 2021, 2, 34–43. [Google Scholar] [CrossRef]
- Humphrey, D.N. Civil Engineering Applications Using Tire Derived Aggregate (TDA); CIWMB: Sacramento, CA, USA, 2003. [Google Scholar]
- Aydilek, A.H.; Madden, E.T.; Demirkan, M.M. Field Evaluation of a Leachate Collection System Constructed with Scrap Tires. J. Geotech. Geoenvironmental Eng. 2006, 132, 990–1000. [Google Scholar] [CrossRef] [Green Version]
- Chaney, R.; Demars, K.; Feng, Z.-Y.; Sutter, K. Dynamic Properties of Granulated Rubber/Sand Mixtures. Geotech. Test. J. 2000, 23, 338–344. [Google Scholar] [CrossRef]
- Pamukcu, S.; Akbulut, S. Thermoelastic Enhancement of Damping of Sand Using Synthetic Ground Rubber. J. Geotech. Geoenvironmental Eng. 2006, 132, 501–510. [Google Scholar] [CrossRef]
- Kim, H.-K.; Santamarina, J.C. Sand–rubber mixtures (large rubber chips). Can. Geotech. J. 2008, 45, 1457–1466. [Google Scholar] [CrossRef] [Green Version]
- Ehsani, M.; Shariatmadari, N.; Mirhosseini, S.M. Shear modulus and damping ratio of sand-granulated rubber mixtures. J. Central South Univ. 2015, 22, 3159–3167. [Google Scholar] [CrossRef]
- Anastasiadis, A.; Pitilakis, K.; Senetakis, K.; Souli, A. Dynamic Shear Modulus and Damping Ratio Curves of Sand/Rubber Mixtures. In Proceedings of the Earthquake Geotechnical Engineering Satellite Conference, XVIIth International Conference on Soil Mechanics & Geotechnical Engineering, Alexandria, Egypt, 2–3 October 2009. [Google Scholar]
- Anastasiadis, A.; Senetakis, K.; Pitilakis, K.; Gargala, C.; Karakasi, I. Dynamic Behavior of Sand/Rubber Mixtures. Part I: Effect of Rubber Content and Duration of Confinement on Small-Strain Shear Modulus and Damping Ratio; ASTM International: West Conshohocken, PA, USA, 2012; Volume 9, pp. 221–247. [Google Scholar] [CrossRef] [Green Version]
- Anastasiadis, A.; Senetakis, K.; Pitilakis, K. Small-Strain Shear Modulus and Damping Ratio of Sand-Rubber and Gravel-Rubber Mixtures. Geotech. Geol. Eng. 2011, 30, 363–382. [Google Scholar] [CrossRef]
- Nakhaei, A.; Marandi, S.; Kermani, S.S.; Bagheripour, M. Dynamic properties of granular soils mixed with granulated rubber. Soil Dyn. Earthq. Eng. 2012, 43, 124–132. [Google Scholar] [CrossRef]
- Li, B.; Huang, M.; Zeng, X. Dynamic Behavior and Liquefaction Analysis of Recycled-Rubber Sand Mixtures. J. Mater. Civ. Eng. 2016, 28, 1–14. [Google Scholar] [CrossRef]
- Mashiri, M.S.; Vinod, J.S.; Sheikh, M.N.; Carraro, J.A.H. Shear modulus of sand–tyre chip mixtures. Environ. Geotech. 2018, 5, 336–344. [Google Scholar] [CrossRef] [Green Version]
- Okur, D.V.; Umu, S.U. Dynamic Properties of Clean Sand Modified with Granulated Rubber. Adv. Civ. Eng. 2018, 2018, 5209494. [Google Scholar] [CrossRef]
- Sarajpoor, S.; Kavand, A.; Zogh, P.; Ghalandarzadeh, A. Dynamic behavior of sand-rubber mixtures based on hollow cylinder tests. Constr. Build. Mater. 2020, 251, 118948. [Google Scholar] [CrossRef]
- Humphrey, D.; Sandford, T.; Cribbs, M.; Manison, W. Shear Strength and Compressibility of Tire Chips for Use as Retaining Wall Backfill. Transp. Res. Rec. 1993, 1422, 29–35. [Google Scholar]
- Foose, G.J.; Benson, C.H.; Bosscher, P.J. Sand Reinforced with Shredded Waste Tires. J. Geotech. Eng. 1996, 122, 760–767. [Google Scholar] [CrossRef]
- Tatlisoz, N.; Edil, T.B.; Benson, C.H. Interaction between Reinforcing Geosynthetics and Soil-Tire Chip Mixtures. J. Geotech. Geoenvironmental Eng. 1998, 124, 1109–1119. [Google Scholar] [CrossRef]
- Ghazavi, M.; Sakhi, M.A. Influence of Optimized Tire Shreds on Shear Strength Parameters of Sand. Int. J. Géoméch. 2005, 5, 58–65. [Google Scholar] [CrossRef]
- Attom, M.F. The use of shredded waste tires to improve the geotechnical engineering properties of sands. Environ. Geol. 2006, 49, 497–503. [Google Scholar] [CrossRef]
- Edinçliler, A.; Baykal, G.; Dengili, K. Determination of static and dynamic behavior of recycled materials for highways. Resour. Conserv. Recycl. 2004, 42, 223–237. [Google Scholar] [CrossRef]
- Edinçliler, A. Using Waste Tire–Soil Mixtures for Embankment Construction. In Scrap Tire Derived Geomaterials—Opportunities and Challenges; Kanto Branch of Japanese Geotechnical Society: Tokyo, Japan, 2007; pp. 319–328. [Google Scholar] [CrossRef]
- Christ, M.; Park, J.-B. Laboratory determination of strength properties of frozen rubber–sand mixtures. Cold Reg. Sci. Technol. 2010, 60, 169–175. [Google Scholar] [CrossRef]
- Cabalar, A.F. Direct Shear Tests on Waste Tires-Sand Mixtures. Geotech. Geol. Eng. 2011, 29, 411–418. [Google Scholar] [CrossRef]
- Marto, A.; Latifi, N.; Moradi, R.; Oghabi, M.; Zolfeghari, S. Shear Properties of Sand—Tire Chips Mixtures. Electron. J. Geotech. Eng. 2013, 18, 325–334. [Google Scholar]
- Anvari, S.M.; Shooshpasha, I.; Kutanaei, S.S. Effect of granulated rubber on shear strength of fine-grained sand. J. Rock Mech. Geotech. Eng. 2017, 9, 936–944. [Google Scholar] [CrossRef]
- Yıldız, O. Investigation on the Mitigation of Earthquake Hazards with Inclusion of Tire Wastes into the Sand. Master’s Thesis, Boğaziçi University, Istanbul, Turkey, 2012. [Google Scholar]
- Chenari, R.J.; Poursalimi, N.; Sosahab, J.S. Dynamic properties of sand-tire crumb mixtures with large cyclic direct shear apparatus. Electron. J. Geotech. Eng. 2017, 22, 5085–5106. [Google Scholar]
- Moussa, A.; El-Naggar, H.; Sadrekarimi, A. Dynamic Properties of Granulated Rubber Using Different Laboratory Tests. Buildings 2021, 11, 186. [Google Scholar] [CrossRef]
- Youwai, S.; Bergado, D.T. Strength and deformation characteristics of shredded rubber tire-sand mixtures. Can. Geotech. J. 2003, 40, 254–264. [Google Scholar] [CrossRef]
- Chaney, R.; Demars, K.; Masad, E.; Taha, R.; Ho, C.; Papagiannakis, T. Engineering Properties of Tire/Soil Mixtures as a Lightweight Fill Material. Geotech. Test. J. 1996, 19, 297–304. [Google Scholar] [CrossRef]
- Tasalloti, A.; Chiaro, G.; Murali, A.; Banasiak, L. Physical and Mechanical Properties of Granulated Rubber Mixed with Granular Soils—A Literature Review. Sustainability 2021, 13, 4309. [Google Scholar] [CrossRef]
- Cabalar, A.F.; Dulundu, K.; Tuncay, K. Strength of various sands in triaxial and cyclic direct shear tests. Eng. Geol. 2013, 156, 92–102. [Google Scholar] [CrossRef]
- ASTM D422-63; Standard Test Method for Particle-Size Analysis of Soils. ASTM International: West Conshohocken, PA, USA, 1998; Volume I, pp. 1–8.
- ASTM D6270-08; Standard Practice of for Use Scrap Tires in Civil Engineering Applications. ASTM International: West Conshohocken, PA, USA, 2020.
- ASTM D8296-19; Standard Test Method for Consolidated Undrained Cyclic Direct Simple Shear Test Under Constant Volume with Load Control or Displacement Control. ASTM International: West Conshohocken, PA, USA, 2019.
- Klar, A.; Roed, M.; Rocchi, I.; Paegle, I. Evaluation of Horizontal Stresses in Soil during Direct Simple Shear by High-Resolution Distributed Fiber Optic Sensing. Sensors 2019, 19, 3684. [Google Scholar] [CrossRef] [Green Version]
- Kutanaei, S.S.; Choobbasti, A.J. Effects of nanosilica particles and randomly distributed fibers on the ultrasonic pulse velocity and mechanical properties of cemented sand. J. Mater. Civ. Eng. 2016, 29, 04016230. [Google Scholar] [CrossRef]
- Kutanaei, S.S.; Choobbasti, A.J. Experimental study of combined effects of fibers and nanosilica on mechanical properties of cemented sand. J. Mater. Civ. Eng. 2016, 28, 06016001. [Google Scholar] [CrossRef]
- Ghazavi, M. Shear strength characteristics of sand-mixed with granular rubber. Geotech. Geol. Eng. 2004, 22, 401–416. [Google Scholar] [CrossRef]
- Shewbridge, S.E.; Sitar, N. Deformation Characteristics of Reinforced Sand in Direct Shear. J. Geotech. Eng. 1989, 115, 1134–1147. [Google Scholar] [CrossRef]
- Choobbasti, A.J.; Kutanaei, S.S. Effect of fiber reinforcement on deformability properties of cemented sand. J. Adhes. Sci. Technol. 2016, 31, 1576–1590. [Google Scholar] [CrossRef]
- Benson, C.H.; Khire, M.V. Soil reinforcement with strips of reclaimed HDPE. J. Geotech. Eng. 1994, 120, 838–855. [Google Scholar] [CrossRef]
- Rummelhart, D.E. The Architecture of Mind: A Connectionist Approach. In Foundations of Cognitive Science; Posner, M.I., Ed.; MIT Press: Cambridge, MA, USA, 1989; pp. 133–159. [Google Scholar] [CrossRef]










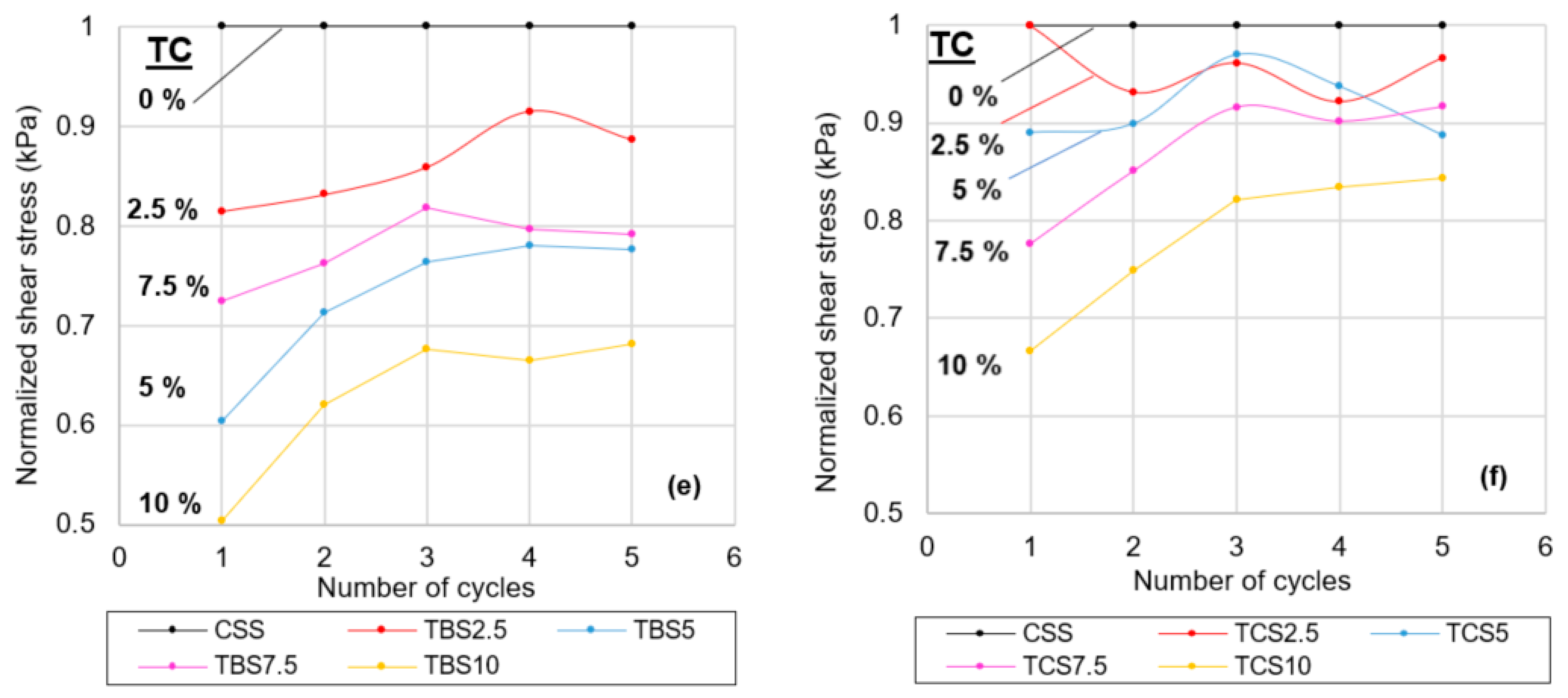
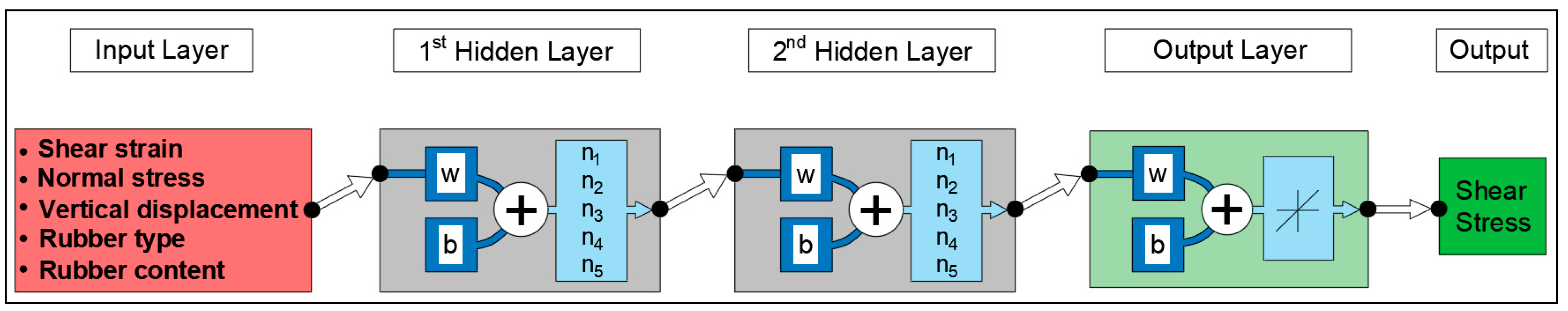
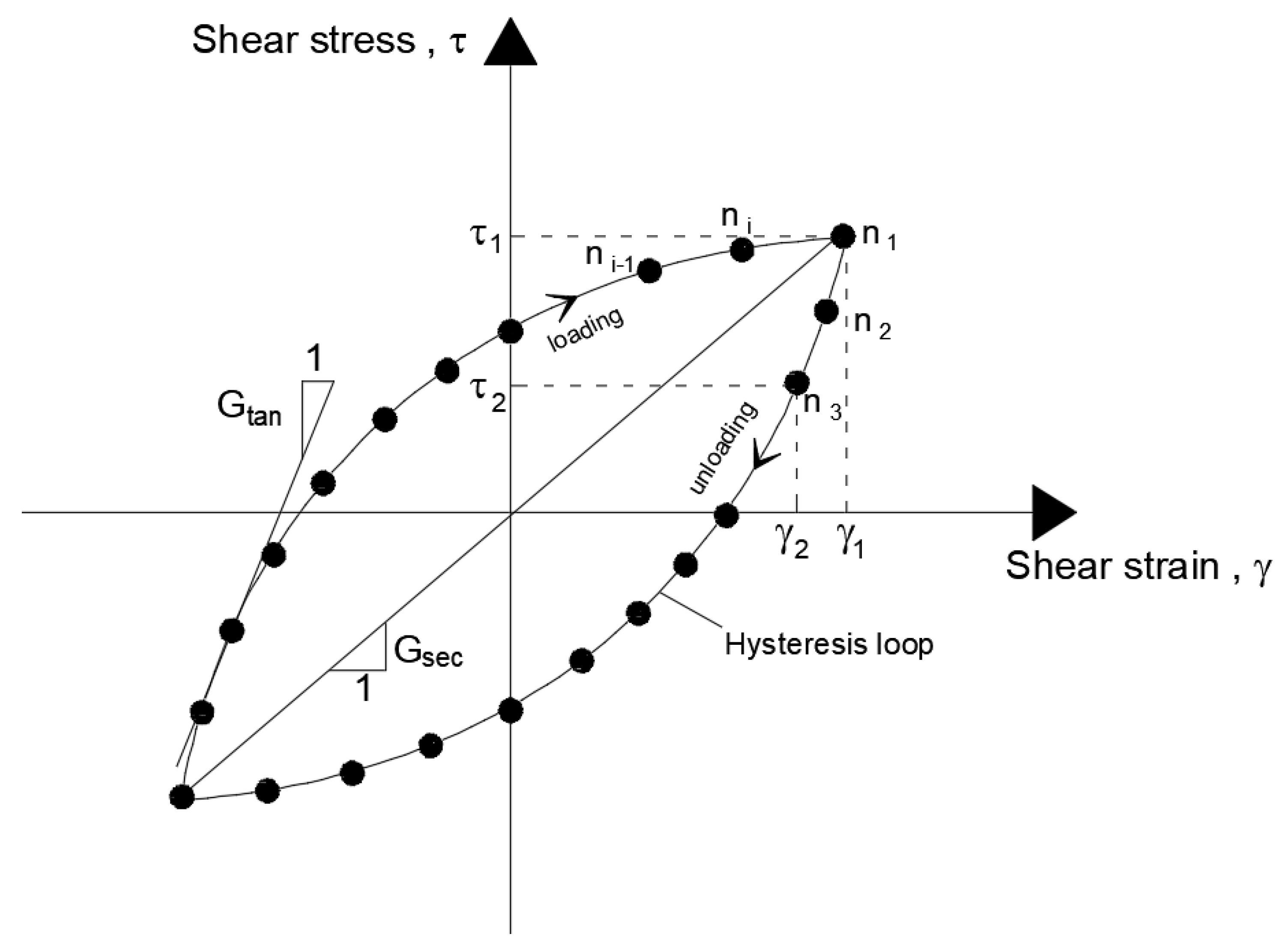

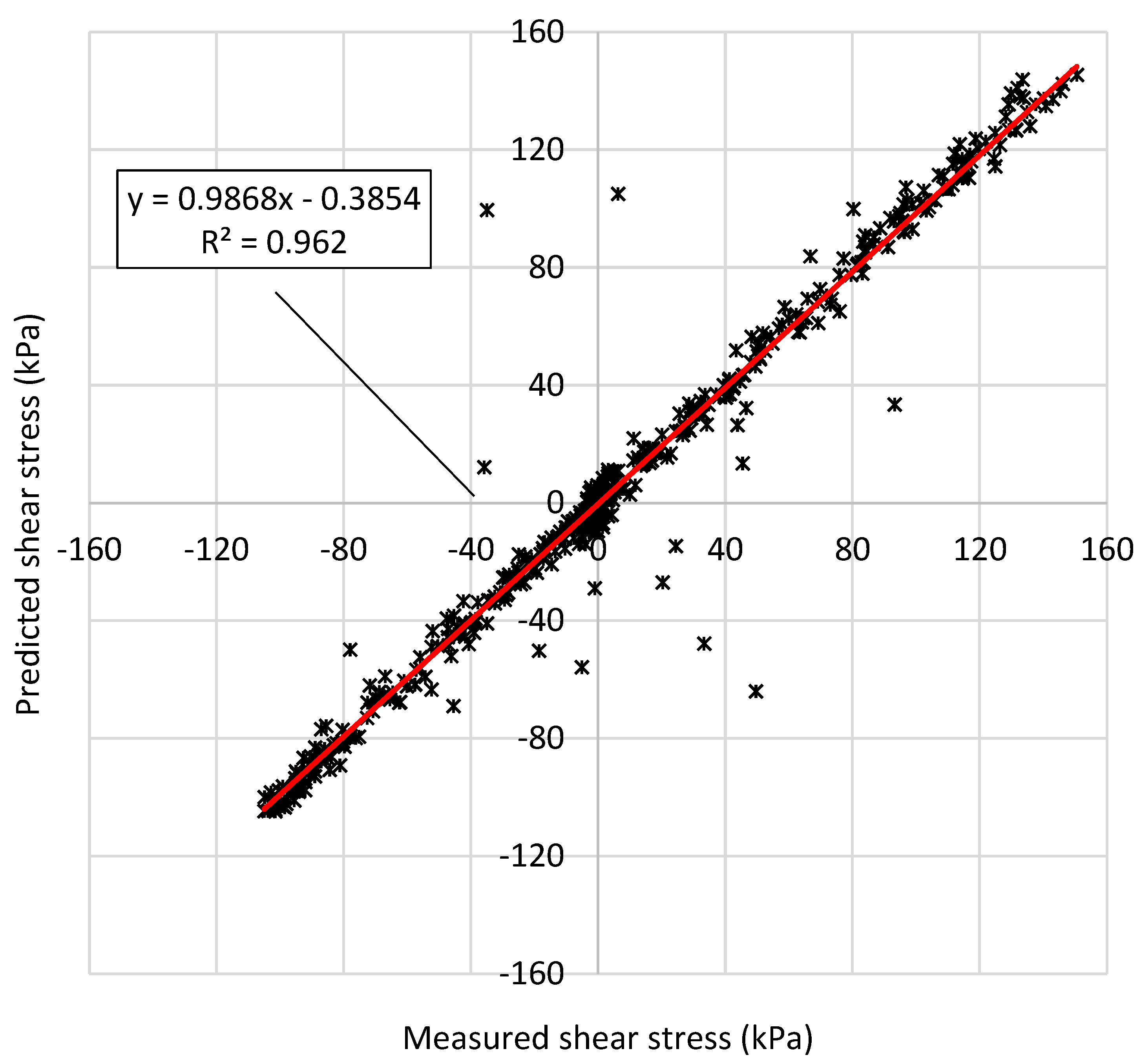

| Type of Waste Tires | Unit Weight (kN/m3) | ||||
|---|---|---|---|---|---|
| Waste Tires Content (%) | |||||
| 0 | 2.5 | 5 | 7.5 | 10 | |
| TB | 18.8 | 18.5 | 17.9 | 16.3 | 16.1 |
| TC | 18.8 | 18.6 | 18.3 | 18.0 | 17.7 |
| Number of Cycle | Max. ShearStress (kPa) | Min. Horizontal Stress (kPa) | Difference (%) |
|---|---|---|---|
| 1 | 115.5 | −92.1 | 25 |
| 2 | 130.3 | −96.9 | 35 |
| 3 | 134.0 | −95.2 | 41 |
| 4 | 143.0 | −95.7 | 49 |
| 5 | 150.6 | −97.0 | 55 |
| Horizontal Stress (kPa) | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Number of Cycle | CSS | TBS2.5 | TBS5 | TBS7.5 | TBS10 | |||||
| Max. | Min. | Max. | Min. | Max. | Min. | Max. | Min. | Max. | Min. | |
| 1 | 115.5 | −92.1 | 94.6 | −95.1 | 69.9 | −88.5 | 84.0 | −87.4 | 58.4 | −80.7 |
| 2 | 130.3 | −96.9 | 108.2 | −97.9 | 92.3 | −97.1 | 98.8 | −92.2 | 80.0 | −88.9 |
| 3 | 134.0 | −95.2 | 115.4 | −101.0 | 102.9 | −102.1 | 110.2 | −95.7 | 90.4 | −90.3 |
| 4 | 143.0 | −95.7 | 131.9 | −102.6 | 112.0 | −104.9 | 111.6 | −97.6 | 95.0 | −91.1 |
| 5 | 150.6 | −97.0 | 133.5 | −103.6 | 116.6 | −105.0 | 118.7 | −98.4 | 101.5 | −93.3 |
| Horizontal Stress (kPa) | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Number of Cycle | CSS | TCS2.5 | TCS5 | TCS7.5 | TCS10 | |||||
| Max. | Min. | Max. | Min. | Max. | Min. | Max. | Min. | Max. | Min. | |
| 1 | 115.5 | 92.1 | 115.6 | −91.5 | 103.0 | −96.4 | 89.4 | −94.6 | 77.2 | −92.0 |
| 2 | 130.3 | −96.9 | 120.8 | −98.0 | 117.0 | −102.0 | 110.2 | −97.2 | 97.4 | −100.0 |
| 3 | 134.0 | −95.2 | 129.5 | −101.5 | 130.7 | −103.6 | 123.1 | −102.6 | 110.4 | −102.9 |
| 4 | 143.0 | −95.7 | 132.7 | −102.1 | 133.9 | −105.0 | 129.1 | −103.8 | 119.7 | −102.6 |
| 5 | 150.6 | −97.0 | 145.4 | −97.0 | 134.8 | −102.0 | 137.7 | −101.3 | 126.5 | −102.8 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yıldız, Ö.; Cabalar, A.F. Cyclic Direct Shear Testing of a Sand with Waste Tires. Sustainability 2022, 14, 16850. https://doi.org/10.3390/su142416850
Yıldız Ö, Cabalar AF. Cyclic Direct Shear Testing of a Sand with Waste Tires. Sustainability. 2022; 14(24):16850. https://doi.org/10.3390/su142416850
Chicago/Turabian StyleYıldız, Özgür, and Ali Firat Cabalar. 2022. "Cyclic Direct Shear Testing of a Sand with Waste Tires" Sustainability 14, no. 24: 16850. https://doi.org/10.3390/su142416850
APA StyleYıldız, Ö., & Cabalar, A. F. (2022). Cyclic Direct Shear Testing of a Sand with Waste Tires. Sustainability, 14(24), 16850. https://doi.org/10.3390/su142416850







