A Game-Theoretic Approach for CSR Emergency Medical Supply Chain during COVID-19 Crisis
Abstract
:1. Introduction
- What are the effects of the retailer’s implementation level of social responsibility on profits of emergency medical member enterprises and systems?
- Will the retailer’s implementation level of social responsibility affect government subsidy and consumer surplus?
- What are the effects of the difficulty factor of manufacturer’s technological innovation ε on profits of emergency medical member enterprises and systems?
- Can the decentralized supply chain be coordinated and how is it coordinated?
2. Literature Review
3. Model Descriptions and Assumptions
4. Decision Model of Emergency Medical Supply Chain during the Pandemic
4.1. Manufacturer-Led Game Model in Decentralized Decision Scenario without Government Subsidies
4.2. Manufacturer-Led Game Model in Decentralized Decision Scenario with Government Subsidies
4.3. Manufacturer-Led Centralized Decision Game Model
4.4. Supply Chain Coordination Model under the Wholesale Price–Cost Sharing Joint Contract
5. Numerical Comparison of Different Cases and Parameters Sensitivity Analysis
5.1. Numerical Analysis of Equilibrium Results
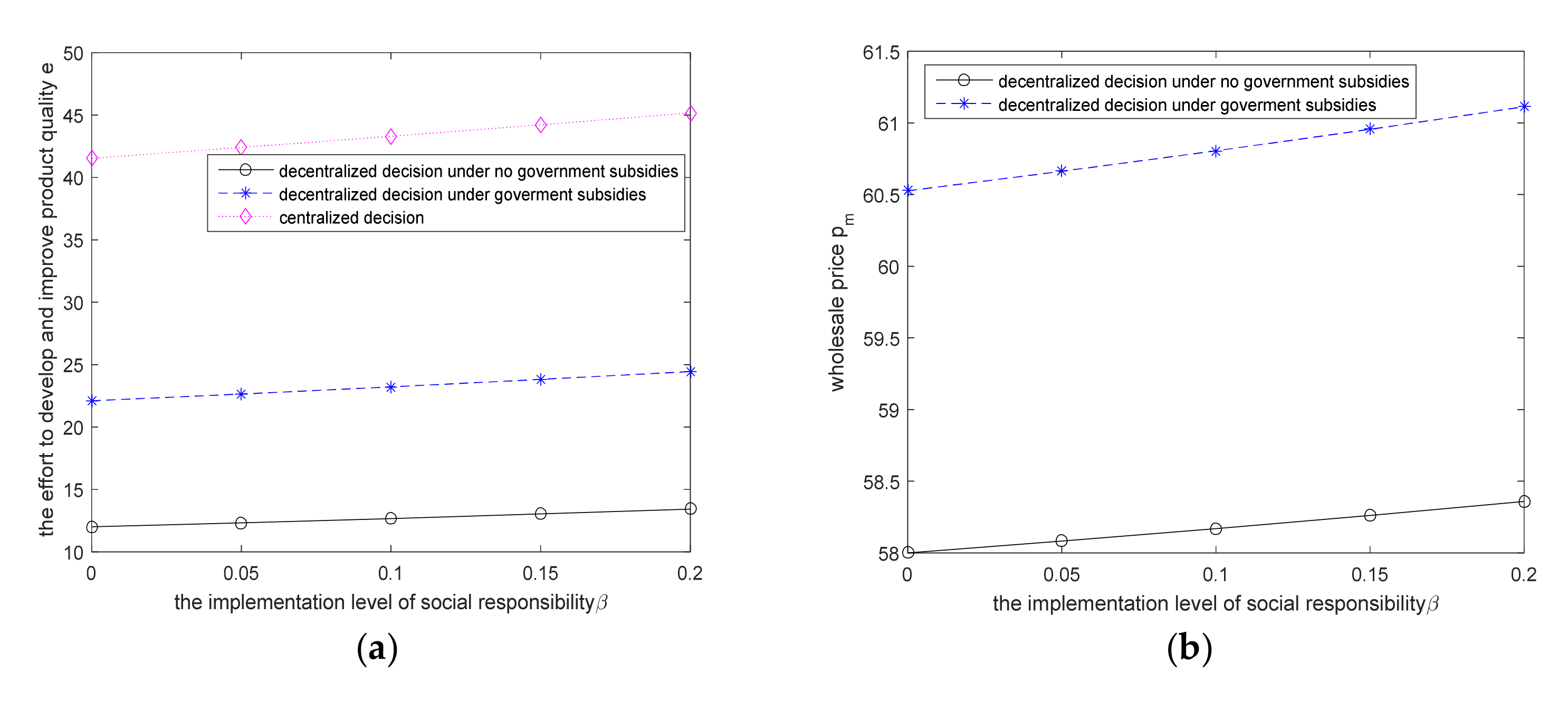
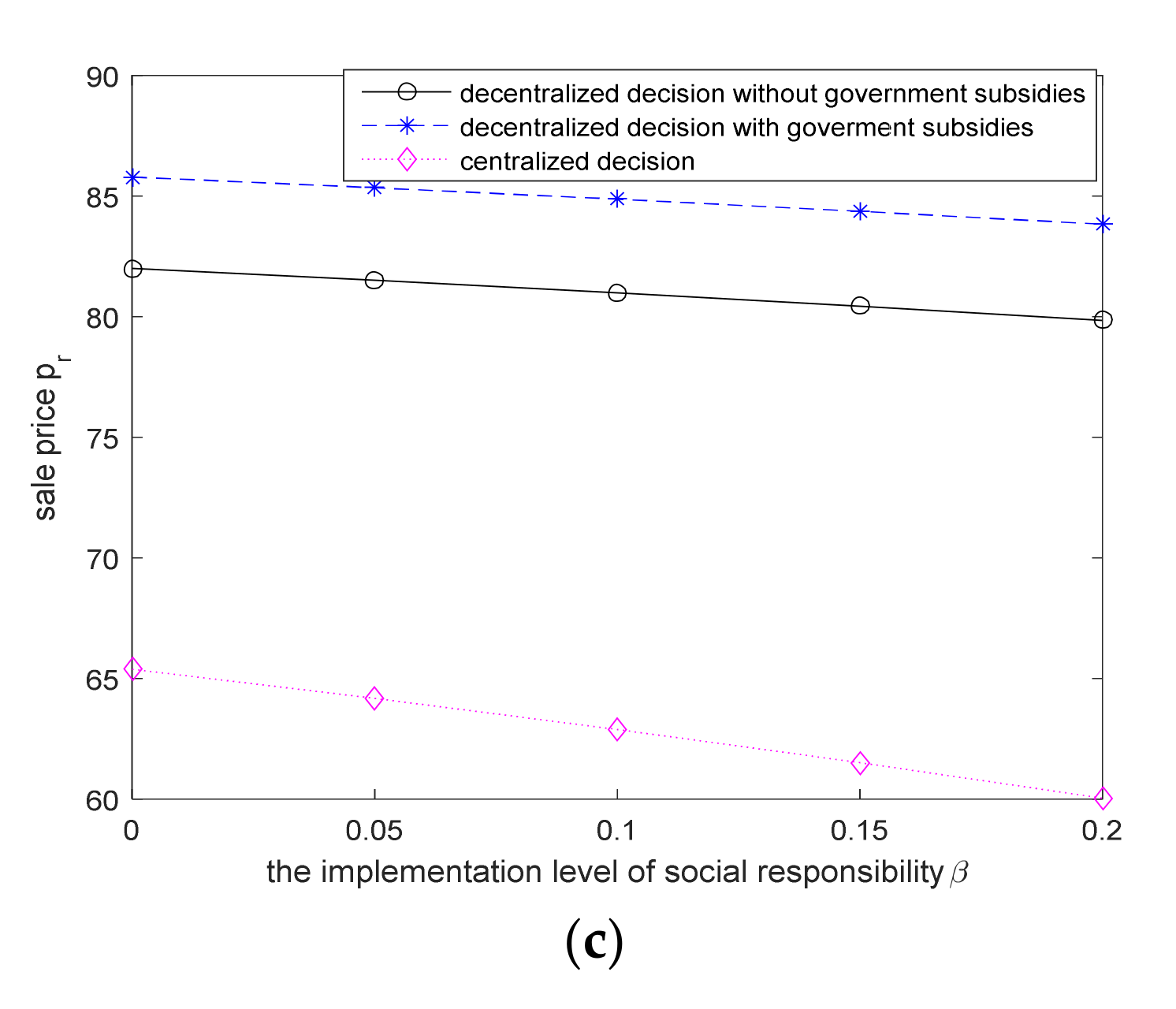
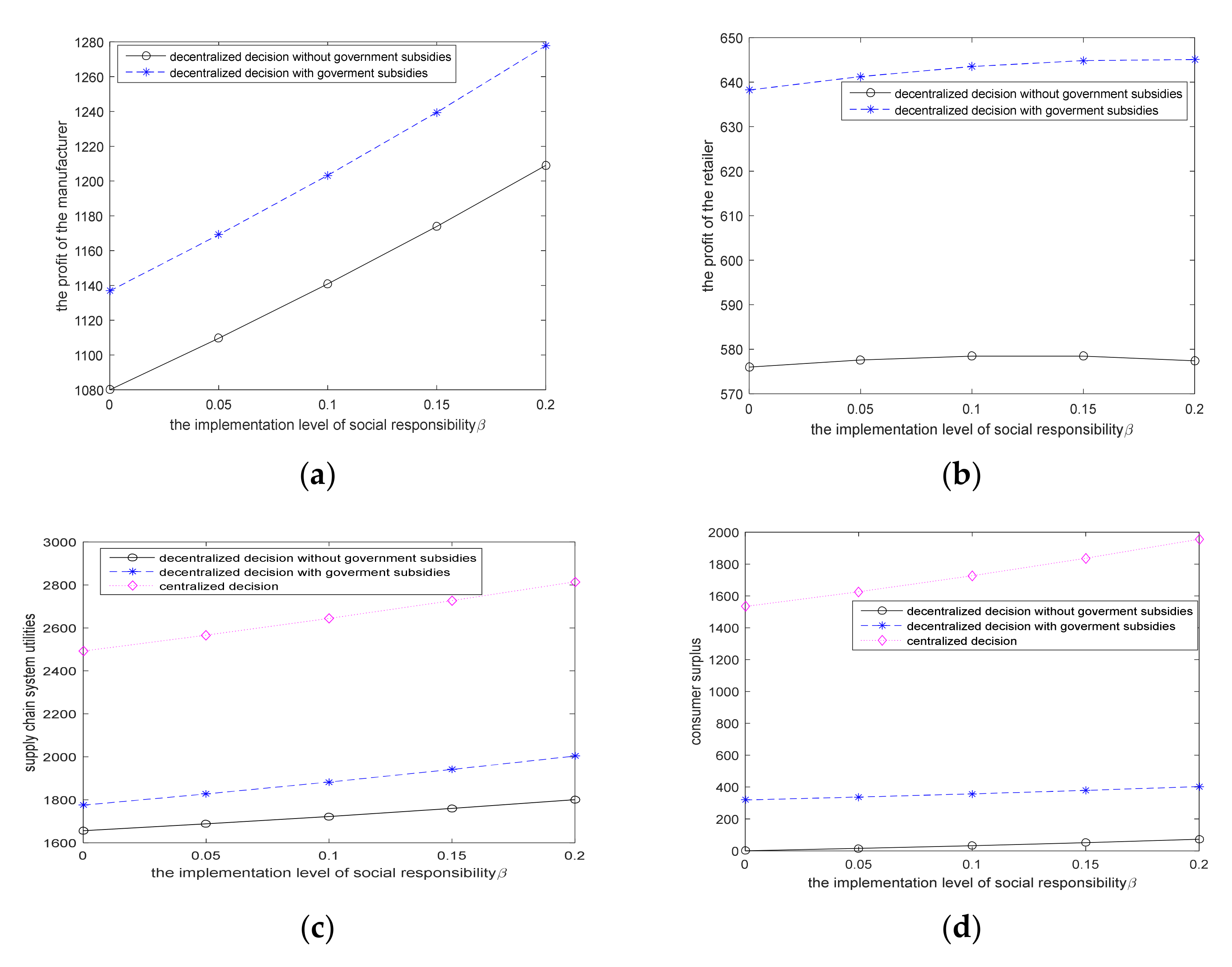
5.2. Impact of Retailer’s CSR Level on Equilibrium Results
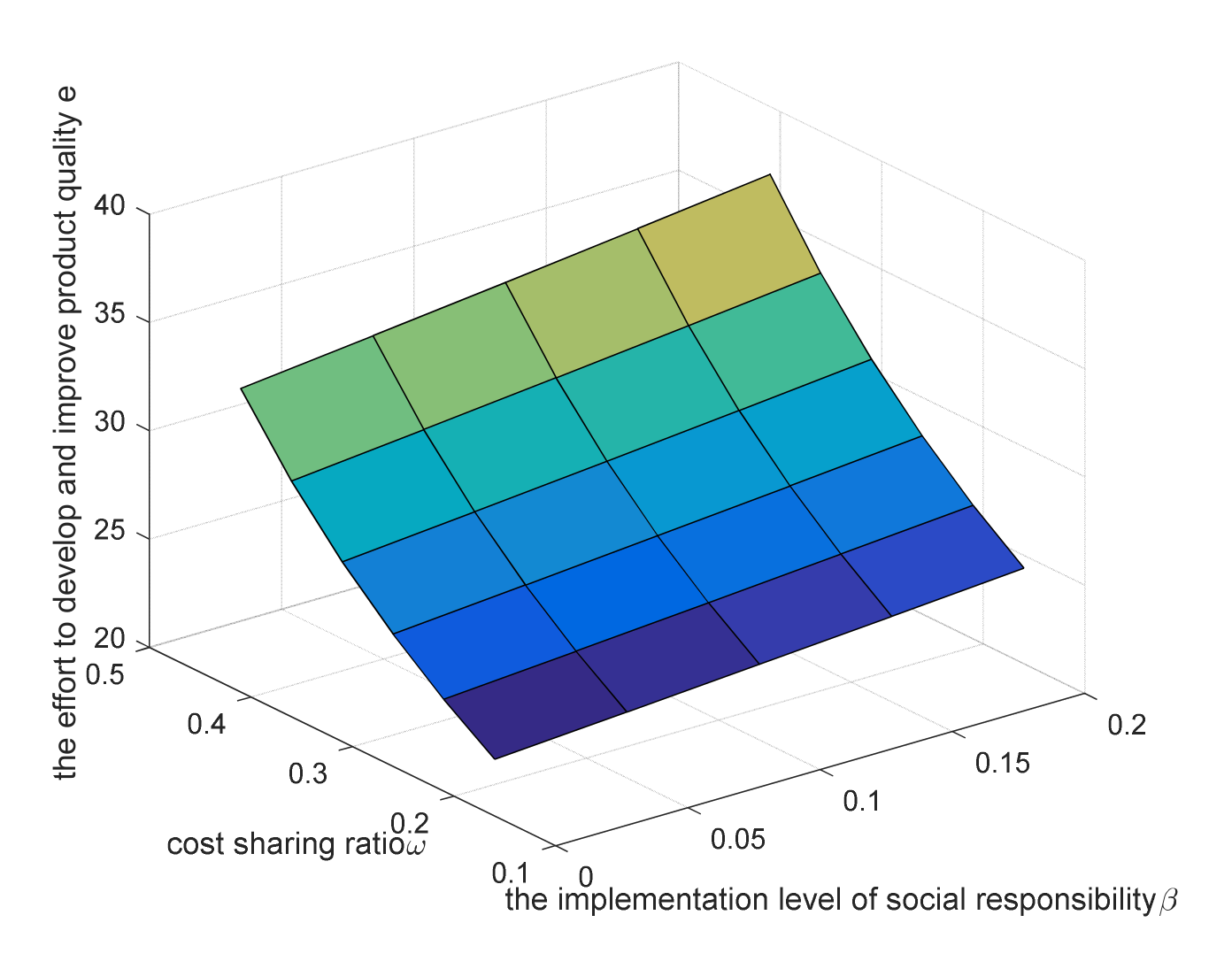
5.3. Coordination Effect Analysis of Wholesale Price–Cost Sharing Joint Contract
6. Concluding Remarks with Future Scopes
6.1. Concluding Remarks
- Compared to equilibrium results without government subsidies, the manufacturer’s effort to develop and improve product quality, the manufacturer’s profit, the retailer’s profit, and the total social welfare under the government subsidy situation are greater.
- The government subsidy coefficient to the manufacturer is not affected by the sensitivity of demand to manufacturer’s effort to improve product design and supply efficiency λ, but λ has increased the effort of the manufacturer to develop and improve products, and the sale price of emergency medical supplies, thereby boosting the profits and social welfare of emergency medical member enterprises.
- Under the decentralized decision scenario, with the enhancement of implementation level of social responsibility, the government subsidy coefficient decreases, the manufacturer’s effort to develop and improve product quality, the wholesale price, and the manufacturer’s profit increases. The impacts of the retailer’s implementation level of social responsibility on the sales price, retailer’s profit, and the overall profit of the supply chain also depend on the manufacturer’s effort to develop and improve product quality. Second, the government does not blindly subsidize the manufacturer, and its subsidy coefficient is directly related to the retailer’s implementation level of social responsibility so that the total social welfare can be optimized.
- The technological innovation difficulty coefficient ε directly affects the emergency medical supplies supply chain members and the optimal value of the system and the retailer’s implementation of social responsibility. Therefore, to accomplish the coordination of the emergency medical supplies supply chain, the government can provide the subsidy to the manufacturer.
- When the fraction of cost-sharing is in a certain range, the profits of emergency medical member enterprises are higher than the corresponding values under decentralized decision making, and the joint contract can encourage emergency medical member enterprises to improve product design and fulfill social responsibilities, thereby boosting the profit of emergency medical member enterprises, realizing the reasonable allocation of supply chain profits.
6.2. Managerial Implications
- For the government: The government plays a vital role in the emergency medical supply chain during the COVID-19 pandemic situation. How to establish an effective incentive mechanism and encourage enterprises to assume social responsibility is a particularly important issue. From the perspective of the manufacturer, the government should provide different incentives, such as production or cost subsidies and various support policies, alleviating the uncertainty of manufacturing enterprises during the epidemic. Compared with no government subsidies, the utility of supply chain members under government subsidies has improved. Therefore, proper government subsidies not only help to maintain the balance of emergency medical supply chain enterprises but also to achieve unified management and save expenses. In terms of coordination models, government subsidy is positively correlated with the level of retailer’s CSR implementation , indicating that the government should focus on raising enterprises’ CSR awareness.
- For the manufacturer: It is necessary to promote technological innovation level in terms of product quality, production efficiency, and material supply during the COVID-19 pandemic. However, technology investment will increase production costs and decrease the enterprise utility and the enthusiasm for social responsibility. In the process of fulfilling technological innovation to assume CSR, the manufacturer should pay attention to costs and the government needs to subsidize the manufacturer’s technological innovation costs to reduce the burden and allow the manufacturer to assume more social responsibilities during the pandemic. Therefore, the manufacturer’s technological innovation difficulty coefficient ε should be relatively small to enhance awareness of social responsibility and obtain more profit.
- For the retailer: The retailer should enhance the CSR awareness and capability for cost-sharing for the manufacturer’s technological innovation under the guidance of the government. Despite the higher CSR awareness and capability for cost-sharing , the profit of the retailer is higher. However, the level of CSR implementation has a negative influence on government subsidies and directly affects the manufacturer’s production investment decision. In addition, when the retailer has a range of cost-sharing capabilities () instead of a random range, the retailer will get more profits. Therefore, the retailer should not only pursue its economic interest but also undertake some CSR responsibilities by actively cooperating with its supply chain partners to maintain a certain level of supply chain CSR. Meanwhile, from Corollary 5, we can observe that centralized decision making is the best cooperation state. Therefore, emergency medical supply chain enterprises should balance technological innovation investments and CSR investments rather than blindly invest and strive to achieve the level of centralized decision making.
6.3. Limitations and Future Scopes
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Goel, R.K.; Saunoris, J.W.; Goel, S.S. Supply chain performance and economic growth: The impact of COVID-19 disruptions. J. Policy Modeling 2021, 43, 298–316. [Google Scholar] [CrossRef]
- Sajid, Z. A dynamic risk assessment model to assess the impact of the coronavirus (COVID-19) on the sustainability of the biomass supply chain: A case study of a U.S. biofuel industry. Renew. Sustain. Energy Rev. 2021, 151, 111574. [Google Scholar] [CrossRef]
- Palouj, M.; Lavaei Adaryani, R.; Alambeigi, A.; Movarej, M.; Safi Sis, Y. Surveying the impact of the coronavirus (COVID-19) on the poultry supply chain: A mixed methods study. Food Control 2021, 126, 108084. [Google Scholar] [CrossRef]
- Paul, S.K.; Chowdhury, P.; Moktadir, M.A.; Lau, K.H. Supply chain recovery challenges in the wake of COVID-19 pandemic. J. Bus. Res. 2021, 136, 316–329. [Google Scholar] [CrossRef]
- Alam, S.T.; Ahmed, S.; Ali, S.M.; Sarker, S.; Kabir, G.; Ul-Islam, A. Challenges to COVID-19 vaccine supply chain: Implications for sustainable development goals. Int. J. Prod. Econ. 2021, 239, 108193. [Google Scholar] [CrossRef]
- Rowan, N.J.; Laffey, J.G. Challenges and solutions for addressing critical shortage of supply chain for personal and protective equipment (PPE) arising from Coronavirus disease (COVID19) pandemic—Case study from the Republic of Ireland. Sci. Total Environ. 2020, 725, 138532. [Google Scholar] [CrossRef]
- Shokrani, A.; Loukaides, E.G.; Elias, E.; Lunt, A.J.G. Exploration of alternative supply chains and distributed manufacturing in response to COVID-19—A case study of medical face shields. Mater. Des. 2020, 192, 108749. [Google Scholar] [CrossRef]
- Lin, Y.; Fan, D.; Shi, X.; Fu, M. The effects of supply chain diversification during the COVID-19 crisis: Evidence from Chinese manufacturers. Transp. Res. Part E Logist. Transp. Rev. 2021, 155, 102493. [Google Scholar] [CrossRef]
- Achmad, A.L.H.; Chaerani, D.; Perdana, T. Designing a food supply chain strategy during COVID-19 pandemic using an integrated Agent-Based Modelling and Robust Optimization. Heliyon 2021, 7, e08448. [Google Scholar] [CrossRef]
- Barman, A.; Das, R.; De, P.K. Impact of COVID-19 in food supply chain: Disruptions and recovery strategy. Curr. Res. Behav. Sci. 2021, 2, 100017. [Google Scholar] [CrossRef]
- Shaw, R.; Kim, Y.K.; Hua, J. Governance, technology and citizen behavior in pandemic: Lessons from COVID-19 in East Asia. Prog. Disaster Sci. 2020, 6, 100090. [Google Scholar] [CrossRef]
- Kang, K.; Luan, X.; Shen, W.; Ma, Y.; Wei, X. The Strategies of the Poverty-Alleviation Supply Chain with Government Subsidies and Cost Sharing: Government-Led or Market-Oriented? Sustainability 2020, 12, 4050. [Google Scholar] [CrossRef]
- Orlando, B.; Tortora, D.; Pezzi, A.; Bitbol-Saba, N. The disruption of the international supply chain: Firm resilience and knowledge preparedness to tackle the COVID-19 outbreak. J. Int. Manag. 2021, 100876. [Google Scholar] [CrossRef]
- Shu, T.; Liu, Q.; Chen, S.; Wang, S.; Lai, K. Pricing Decisions of CSR Closed-Loop Supply Chains with Carbon Emission Constraints. Sustainability 2018, 10, 4430. [Google Scholar] [CrossRef] [Green Version]
- Li, Z.; Pan, Y.; Yang, W.; Ma, J.; Zhou, M. Effects of government subsidies on green technology investment and green marketing coordination of supply chain under the cap-and-trade mechanism. Energy Econ. 2021, 101, 105426. [Google Scholar] [CrossRef]
- Heydari, J. Supply chain coordination using time-based temporary price discounts. Comput. Ind. Eng. 2014, 75, 96–101. [Google Scholar] [CrossRef]
- Narulidea, W.; Lathifah, A. Revenue sharing contract as coordination mechanism with the implementation of logistics outsourcing in decentralized supply chain. J. Eng. Manag. Ind. Syst. 2018, 5, 80–86. [Google Scholar] [CrossRef] [Green Version]
- Heydari, J.; Ghasemi, M. A revenue sharing contract for reverse supply chain coordination under stochastic quality of returned products and uncertain remanufacturing capacity. J. Clean. Prod. 2018, 197, 607–615. [Google Scholar] [CrossRef]
- Heydari, J.; Govindan, K.; Ebrahimi Nasab, H.R.; Taleizadeh, A.A. Coordination by quantity flexibility contract in a two-echelon supply chain system: Effect of outsourcing decisions. Int. J. Prod. Econ. 2020, 225, 107586. [Google Scholar] [CrossRef]
- Li, J.; Luo, X.; Wang, Q.; Zhou, W. Supply chain coordination through capacity reservation contract and quantity flexibility contract. Omega 2021, 99, 102195. [Google Scholar] [CrossRef]
- Aghajani, M.; Torabi, S.A.; Heydari, J. A novel option contract integrated with supplier selection and inventory prepositioning for humanitarian relief supply chains. Socio-Econ. Plan. Sci. 2020, 71, 100780. [Google Scholar] [CrossRef]
- Glock, C.H.; Rekik, Y.; Ries, J.M. A coordination mechanism for supply chains with capacity expansions and order-dependent lead times. Eur. J. Oper. Res. 2020, 285, 247–262. [Google Scholar] [CrossRef]
- Li, X.; Chen, J.; Ai, X. Contract design in a cross-sales supply chain with demand information asymmetry. Eur. J. Oper. Res. 2019, 275, 939–956. [Google Scholar] [CrossRef]
- Hosseini-Motlagh, S.-M.; Nouri-Harzvili, M.; Choi, T.-M.; Ebrahimi, S. Reverse supply chain systems optimization with dual channel and demand disruptions: Sustainability, CSR investment and pricing coordination. Inf. Sci. 2019, 503, 606–634. [Google Scholar] [CrossRef]
- Asl-Najafi, J.; Yaghoubi, S.; Zand, F. Dual-channel supply chain coordination considering targeted capacity allocation under uncertainty. Math. Comput. Simul. 2021, 187, 566–585. [Google Scholar] [CrossRef]
- Qiu, R.; Hou, L.; Sun, Y.; Sun, M.; Sun, Y. Joint pricing, ordering and order fulfillment decisions for a dual-channel supply chain with demand uncertainties: A distribution-free approach. Comput. Ind. Eng. 2021, 160, 107546. [Google Scholar] [CrossRef]
- Hong, I.H.; Dang, J.-F.; Lin, K.-W. The equilibrium contract rent and reward money under incomplete information in reverse supply chains: A game theoretical approach and computational study. Comput. Ind. Eng. 2016, 102, 69–77. [Google Scholar] [CrossRef]
- Mondal, A.; Roy, S.K. Multi-objective sustainable opened- and closed-loop supply chain under mixed uncertainty during COVID-19 pandemic situation. Comput. Ind. Eng. 2021, 159, 107453. [Google Scholar] [CrossRef]
- Dehghan-Bonari, M.; Bakhshi, A.; Aghsami, A.; Jolai, F. Green supply chain management through call option contract and revenue-sharing contract to cope with demand uncertainty. Clean. Logist. Supply Chain 2021, 2, 100010. [Google Scholar] [CrossRef]
- Zou, T.; Zou, Q.; Hu, L. Joint decision of financing and ordering in an emission-dependent supply chain with yield uncertainty. Comput. Ind. Eng. 2021, 152, 106994. [Google Scholar] [CrossRef]
- Jiang, Z.-Z.; He, N.; Huang, S. Government penalty provision and contracting with asymmetric quality information in a bioenergy supply chain. Transp. Res. Part E Logist. Transp. Rev. 2021, 154, 102481. [Google Scholar] [CrossRef]
- Bazyar, A.; Zarrinpoor, N.; Safavian, A. Optimal design of a sustainable natural gas supply chain network under uncertainty. Chem. Eng. Res. Des. 2021, 176, 60–88. [Google Scholar] [CrossRef]
- RezaHoseini, A.; Noori, S.; Ghannadpour, S.F. Integrated scheduling of suppliers and multi-project activities for green construction supply chains under uncertainty. Autom. Constr. 2021, 122, 103485. [Google Scholar] [CrossRef]
- Chen, Z.; Ivan Su, S.-I. Social welfare maximization with the least subsidy: Photovoltaic supply chain equilibrium and coordination with fairness concern. Renew. Energy 2019, 132, 1332–1347. [Google Scholar] [CrossRef]
- Chen, Z.; Su, S.-I.I.; Wang, H. Inter-Basin Water Transfer Supply Chain Equilibrium and Coordination: A Social Welfare Maximization Perspective. Water Resour. Manag. 2019, 33, 2577–2598. [Google Scholar] [CrossRef]
- Ma, P. Optimal generic and brand advertising efforts in a decentralized supply chain considering customer surplus. J. Retail. Consum. Serv. 2021, 60, 102502. [Google Scholar] [CrossRef]
- Zhou, Y.; Hu, F.; Zhou, Z. Pricing decisions and social welfare in a supply chain with multiple competing retailers and carbon tax policy. J. Clean. Prod. 2018, 190, 752–777. [Google Scholar] [CrossRef]
- Bai, Q.; Chen, M.; Nikolaidis, Y.; Xu, J. Improving sustainability and social responsibility of a two-tier supply chain investing in emission reduction technology. Appl. Math. Model. 2021, 95, 688–714. [Google Scholar] [CrossRef]
- Su, R.-H.; Weng, M.-W.; Yang, C.-T. Effects of corporate social responsibility activities in a two-stage assembly production system with multiple components and imperfect processes. Eur. J. Oper. Res. 2021, 293, 469–480. [Google Scholar] [CrossRef]
- Wang, Y.; Su, M.; Shen, L.; Tang, R. Decision-making of closed-loop supply chain under Corporate Social Responsibility and fairness concerns. J. Clean. Prod. 2021, 284, 125373. [Google Scholar] [CrossRef]
- Liu, S.; Yao, F.; Chen, D. CSR investment decision and coordination strategy for closed-loop supply chain with two competing retailers. J. Clean. Prod. 2021, 310, 127378. [Google Scholar] [CrossRef]
- Cheng, H.; Ding, H. Dynamic game of corporate social responsibility in a supply chain with competition. J. Clean. Prod. 2021, 317, 128398. [Google Scholar] [CrossRef]
- Mondal, C.; Giri, B.C. Optimizing price, quality and CSR investment under competing dual recycling channels in a sustainable closed-loop supply chain. CIRP J. Manuf. Sci. Technol. 2021, 35, 193–208. [Google Scholar] [CrossRef]
- Khosroshahi, H.; Dimitrov, S.; Hejazi, S.R. Pricing, greening, and transparency decisions considering the impact of government subsidies and CSR behavior in supply chain decisions. J. Retail. Consum. Serv. 2021, 60, 102485. [Google Scholar] [CrossRef]
- Fander, A.; Yaghoubi, S. Impact of fuel-efficient technology on automotive and fuel supply chain under government intervention: A case study. Appl. Math. Model. 2021, 97, 771–802. [Google Scholar] [CrossRef]
- Meng, Q.; Li, M.; Liu, W.; Li, Z.; Zhang, J. Pricing policies of dual-channel green supply chain: Considering government subsidies and consumers’ dual preferences. Sustain. Prod. Consum. 2021, 26, 1021–1030. [Google Scholar] [CrossRef]
- Rezayat, M.R.; Yaghoubi, S.; Fander, A. The impact of government intervention in competitive electronic closed-loop supply chain to support internal industry. Resour. Policy 2021, 74, 102257. [Google Scholar] [CrossRef]
- Feng, D.; Shen, C.; Pei, Z. Production decisions of a closed-loop supply chain considering remanufacturing and refurbishing under government subsidy. Sustain. Prod. Consum. 2021, 27, 2058–2074. [Google Scholar] [CrossRef]
- Wu, C.-H. A dynamic perspective of government intervention in a competitive closed-loop supply chain. Eur. J. Oper. Res. 2021, 294, 122–137. [Google Scholar] [CrossRef]
- Zhang, X.-m.; Li, Q.-w.; Liu, Z.; Chang, C.-T. Optimal pricing and remanufacturing mode in a closed-loop supply chain of WEEE under government fund policy. Comput. Ind. Eng. 2021, 151, 106951. [Google Scholar] [CrossRef]
- Liu, Y.; Wang, D.-d.; Xu, Q. A supply chain coordination mechanism with suppliers’ effort performance level and fairness concern. J. Retail. Consum. Serv. 2020, 53, 101950. [Google Scholar] [CrossRef]
- Zhou, Y.; Bao, M.; Chen, X.; Xu, X. Co-op advertising and emission reduction cost sharing contracts and coordination in low-carbon supply chain based on fairness concerns. J. Clean. Prod. 2016, 133, 402–413. [Google Scholar] [CrossRef]
- He, P.; He, Y.; Shi, C.; Xu, H.; Zhou, L. Cost-sharing contract design in a low-carbon service supply chain. Comput. Ind. Eng. 2020, 139, 106160. [Google Scholar] [CrossRef]
- Fan, J.; Ni, D.; Fang, X. Liability cost sharing, product quality choice, and coordination in two-echelon supply chains. Eur. J. Oper. Res. 2020, 284, 514–537. [Google Scholar] [CrossRef]
- Bai, Q.; Chen, M.; Xu, L. Revenue and promotional cost-sharing contract versus two-part tariff contract in coordinating sustainable supply chain systems with deteriorating items. Int. J. Prod. Econ. 2017, 187, 85–101. [Google Scholar] [CrossRef]
- Xie, J.; Zhang, W.; Liang, L.; Xia, Y.; Yin, J.; Yang, G. The revenue and cost sharing contract of pricing and servicing policies in a dual-channel closed-loop supply chain. J. Clean. Prod. 2018, 191, 361–383. [Google Scholar] [CrossRef]
- Zhang, Z.; Xu, H.; Liu, Z.; Fang, Y. Implications for pure profit, environmental impact and social welfare in a socially responsible supply chain. Kybernetes 2020, 50, 757–784. [Google Scholar] [CrossRef]
- Peng, Q.; Wang, C.; Xu, L. Emission abatement and procurement strategies in a low-carbon supply chain with option contracts under stochastic demand. Comput. Ind. Eng. 2020, 144, 106502. [Google Scholar] [CrossRef]
- Lin, J.; Fan, R.; Tan, X.; Zhu, K. Dynamic decision and coordination in a low-carbon supply chain considering the retailer’s social preference. Socio-Econ. Plan. Sci. 2021, 77, 101010. [Google Scholar] [CrossRef]
- Tian, G.; Liu, J.; Gong, Y.; Wang, Q.; Sun, H.; Chen, H. Multinational companies’ coordination mechanism for extending corporate social responsibility to Chinese suppliers. J. Clean. Prod. 2020, 267, 121896. [Google Scholar] [CrossRef]
- Li, S.; Li, M.; Zhou, N. Pricing and coordination in a dual-channel supply chain with a socially responsible manufacturer. PLoS ONE 2020, 15, e0236099. [Google Scholar] [CrossRef] [PubMed]
- Kumar, P.; Baraiya, R.; Das, D.; Jakhar, S.K.; Xu, L.; Mangla, S.K. Social responsibility and cost-learning in dyadic supply chain coordination. Transp. Res. Part E Logist. Transp. Rev. 2021, 156, 102549. [Google Scholar] [CrossRef]
- Shang, M.; Li, H.; Wang, Y.-p.; Qin, Y.-y.; Liu, Y.; Tan, Y. Optimal decisions in a closed-loop supply chain under different policies of government intervention. Sustain. Energy Technol. Assess. 2021, 47, 101283. [Google Scholar] [CrossRef]
- Mahmoudi, A.; Govindan, K.; Shishebori, D.; Mahmoudi, R. Product pricing problem in green and non-green multi-channel supply chains under government intervention and in the presence of third-party logistics companies. Comput. Ind. Eng. 2021, 159, 107490. [Google Scholar] [CrossRef]
- Ma, S.; He, Y.; Gu, R.; Li, S. Sustainable supply chain management considering technology investments and government intervention. Transp. Res. Part E Logist. Transp. Rev. 2021, 149, 102290. [Google Scholar] [CrossRef]
- Liu, Y.; Ren, W.; Xu, Q.; Liu, Z. A coordination mechanism through cost sharing of corporate social responsibility and government subsidy in a two-echelon supply chain. J. Bus. Ind. Mark. 2021, 37, 29–46. [Google Scholar] [CrossRef]


| Reference | SC Structure | CSR | Demand Influence Factor | Game Approach | Coordination Mechanism | Governments Policies | Social Welfare |
|---|---|---|---|---|---|---|---|
| Chen and Su (2019) | 1M + 1PA | Price | MS-led PA-led | RS contract | √ | √ | |
| Zhou et al., (2018) | 1M + nR | Price, carbon emissions, and substitutability degree | M-led | √ | √ | ||
| Bai et al., (2021) | 1M + 1R | √ | Price, CSR level, and emission technology level | R-led | RCS contract | ||
| Wang et al., (2021) | 1M + 1R | √ | Price | M-led | GSCS contract | √ | |
| Liu et al., (2021) | 1M + 1R | √ | Price, CSR investment level, and competition coefficient between two retailers | M-led | RCS contract | ||
| Cheng et al., (2021) | 1M + 1R | √ | Price, corporate reputation | M-led | |||
| Shu et al., (2018) | 1M + 1R | √ | Price | M-led | √ | ||
| Asl-Najaf et al., (2021) | 1M + 1R | Price and product amount | TT contract | ||||
| Hong et al., (2016) | 1M + 1Re | M-led | |||||
| Mondal et al., (2021) | Random, COVID-19 | ||||||
| Jiang et al., (2021) | 1PP + nF | Random | WP and QP contract | √ | |||
| Reza Rezayat et al., (2021) | 2M + 2R | Price and quality | M-led | √ | |||
| Feng et al., (2021) | OEM + IR | Random | √ | ||||
| Current study | 1M + 1R | √ | Price, CSR investment level | M-led | WPCS contract | √ | √ |
| Variable | Without Government Subsidy | Decentralized Decision | Centralized Decision |
|---|---|---|---|
| Parameters | |||||
|---|---|---|---|---|---|
| First example | 100 | 1 | 0.5 | 10 | 1 |
| Second example | 10,000 | 12 | 10 | 200 | 16 |
| Decision Variables | Profits and Social Welfare | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| First example | 0.1 | 60.81 | 84.87 | 23.22 | 0.424 | 1203.3 | 643.51 | 679.26 | 1846.8 | 357.50 | 228.76 | 163.40 |
| 0.2 | 61.11 | 83.83 | 24.45 | 0.42 | 1277.8 | 645.07 | 725.71 | 1922.9 | 403.17 | 250.74 | 192.87 | |
| 0.3 | 61.45 | 82.64 | 25.82 | 0.41 | 1362.0 | 641.27 | 778.68 | 2003.3 | 458.05 | 275.78 | 229.82 | |
| 0.4 | 61.83 | 81.27 | 27.33 | 0.407 | 1457.8 | 629.70 | 839.60 | 2087.5 | 524.75 | 276.72 | 304.40 | |
| 0.5 | 62.26 | 79.68 | 29.03 | 0.40 | 1567.7 | 606.87 | 910.30 | 2174.6 | 606.87 | 337.15 | 337.15 | |
| 0.6 | 62.73 | 77.80 | 30.94 | 0.39 | 1695.1 | 567.56 | 993.22 | 2262.6 | 709.45 | 374.63 | 416.26 | |
| 0.7 | 63.28 | 75.57 | 33.10 | 0.38 | 1844.1 | 503.83 | 1091.6 | 2348.0 | 839.71 | 417.37 | 521.71 | |
| 0.8 | 63.89 | 72.87 | 35.55 | 0.37 | 2020.8 | 403.31 | 1210.0 | 2424.1 | 1008.3 | 465.64 | 665.19 | |
| 0.9 | 64.59 | 69.55 | 38.35 | 0.35 | 2233.1 | 246.25 | 1354.4 | 2479.3 | 1231.3 | 518.97 | 864.94 | |
| Second example | 0.1 | 615.60 | 812.47 | 237.45 | 0.42 | 831,210 | 516,740 | 545,450 | 1,347,900 | 287,080 | 19,135 | 683.40 |
| 0.3 | 628.69 | 805.21 | 268.86 | 0.41 | 958,250 | 534,160 | 648,620 | 1,492,400 | 381,540 | 23,929 | 997.04 | |
| 0.5 | 645.60 | 794.14 | 309.44 | 0.40 | 1,128,900 | 529,500 | 794,250 | 1,658,400 | 529,500 | 30,642 | 1532.1 | |
| 0.7 | 668.16 | 776.20 | 363.59 | 0.38 | 1,368,500 | 466,880 | 1,011,600 | 1,835,400 | 778,140 | 40,288 | 2518.0 | |
| 0.9 | 699.38 | 744.77 | 438.50 | 0.35 | 1,725,100 | 247,310 | 1,360,200 | 1,972,400 | 1,236,600 | 54,292 | 4524.3 | |
| Decision Variables | Profits and Social Welfare | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| First example | 0.4 | 74.85 | 102.65 | 79.41 | 0.417 | 1667.6 | 1030 | 1201.7 | 2697.7 | 686.69 | 1051.0 | 840.83 |
| 0.6 | 66.54 | 90.77 | 46.15 | 0.417 | 1453.8 | 782.84 | 913.31 | 2236.7 | 521.89 | 532.54 | 426.04 | |
| 0.8 | 63.13 | 85.90 | 32.53 | 0.417 | 1366.3 | 691.36 | 806.59 | 2057.6 | 460.91 | 352.74 | 282.19 | |
| 1 | 61.28 | 83.26 | 25.12 | 0.417 | 1318.6 | 643.97 | 751.30 | 1962.6 | 429.31 | 262.85 | 210.28 | |
| 1.2 | 60.11 | 81.59 | 20.45 | 0.417 | 1288.6 | 615.03 | 717.53 | 1903.7 | 410.02 | 209.19 | 167.36 | |
| 1.4 | 59.31 | 80.45 | 17.25 | 0.417 | 1268.1 | 595.54 | 694.79 | 1863.6 | 397.03 | 173.63 | 138.90 | |
| 1.6 | 58.73 | 79.61 | 14.92 | 0.417 | 1253.0 | 581.52 | 678.44 | 1834.6 | 387.68 | 148.35 | 118.68 | |
| Second example | 12 | 679.90 | 885.57 | 391.75 | 0.417 | 1,042,100 | 676,800 | 789,600 | 1,718,900 | 451,200 | 38,368 | 1534.7 |
| 14 | 646.98 | 838.55 | 312.76 | 0.417 | 970,590 | 587,150 | 685,000 | 1,557,700 | 391,430 | 28,530 | 1141.2 | |
| 16 | 625.11 | 807.31 | 260.27 | 0.417 | 923,110 | 531,100 | 619,620 | 1,454,200 | 354,070 | 22,581 | 903.23 | |
| 18 | 609.53 | 785.04 | 222.87 | 0.417 | 889,270 | 492,880 | 575,020 | 1,382,100 | 328,590 | 18,627 | 745.09 | |
| 20 | 597.86 | 768.38 | 194.87 | 0.417 | 863,930 | 465,190 | 542,730 | 1,329,100 | 310,130 | 15,823 | 632.92 | |
| 0.17 | 61.31 | 83.30 | 25.23 | 2638.7 | 1213.6 | 1428.5 | 3852.3 | 859.59 | 3876.2 |
| 0.22 | 61.77 | 83.96 | 27.09 | 2662.6 | 1199.8 | 1418.7 | 3862.5 | 875.25 | 3861.1 |
| 0.27 | 62.31 | 84.73 | 29.25 | 2690.3 | 1178.7 | 1402.1 | 3869.0 | 893.58 | 3835.8 |
| 0.32 | 62.94 | 85.64 | 31.78 | 2722.9 | 1146.8 | 1375.6 | 3869.6 | 915.32 | 3795.5 |
| 0.37 | 63.70 | 86.71 | 34.79 | 2761.6 | 1098.8 | 1334.2 | 3860.4 | 941.52 | 3732.7 |
| 0.42 | 64.61 | 88.01 | 38.43 | 2808.4 | 1026.4 | 1269.8 | 3834.7 | 973.70 | 3635.1 |
| 0.45 | 65.25 | 88.93 | 41.00 | 2841.5 | 965.59 | 1214.8 | 3807.0 | 996.78 | 3551.9 |
| Without Government Subsidies | With Government Subsidies | |
|---|---|---|
| Variables | Decentralized Scenario | Decentralized Scenario |
| 58.46 | 61.28 | |
| 79.23 | 83.26 | |
| 13.85 | 25.12 | |
| 1246.2 | 1318.6 | |
| 671.01 | 751.30 | |
| 1917.2 | 2069.9 | |
| 383.43 | 429.31 | |
| 2204.7 | 2260.5 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xie, K.; Zhu, S.; Gui, P. A Game-Theoretic Approach for CSR Emergency Medical Supply Chain during COVID-19 Crisis. Sustainability 2022, 14, 1315. https://doi.org/10.3390/su14031315
Xie K, Zhu S, Gui P. A Game-Theoretic Approach for CSR Emergency Medical Supply Chain during COVID-19 Crisis. Sustainability. 2022; 14(3):1315. https://doi.org/10.3390/su14031315
Chicago/Turabian StyleXie, Kefan, Shufan Zhu, and Ping Gui. 2022. "A Game-Theoretic Approach for CSR Emergency Medical Supply Chain during COVID-19 Crisis" Sustainability 14, no. 3: 1315. https://doi.org/10.3390/su14031315
APA StyleXie, K., Zhu, S., & Gui, P. (2022). A Game-Theoretic Approach for CSR Emergency Medical Supply Chain during COVID-19 Crisis. Sustainability, 14(3), 1315. https://doi.org/10.3390/su14031315







