Annual Evaluation of Natural Ventilation Induction in Solar Chimneys under Tropical, Dry, and Temperate Climates of Mexico: A Case Study †
Abstract
:1. Introduction
2. Materials and Methods
2.1. The Proposed GEB Approach for SC-SoCh and TC-SoCh
2.1.1. GEB Model for SC-SoCh
2.1.2. GEB Model for TC-SoCh
2.1.3. Coefficients for and Properties of Convective Heat Transfer
2.1.4. Algorithm of SC-SoCh and TC-SoCh Models
| Algorithm 1 Method for solving SC-SoCh and TC-SoCh |
|
2.2. GEB Model Validation
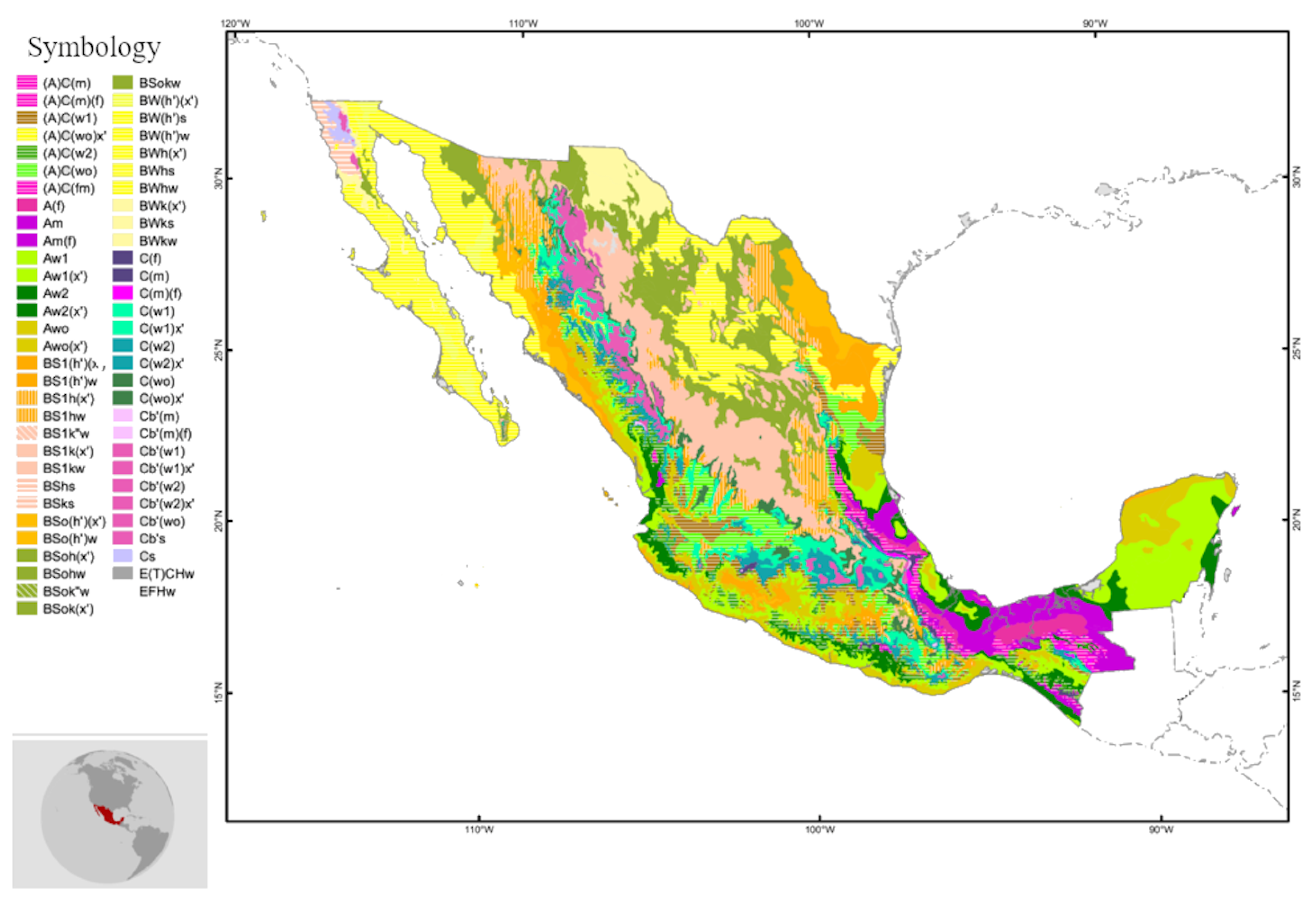
2.3. Parameters of Study: Weather Conditions of Mexico and Considerations for Numerical Modeling
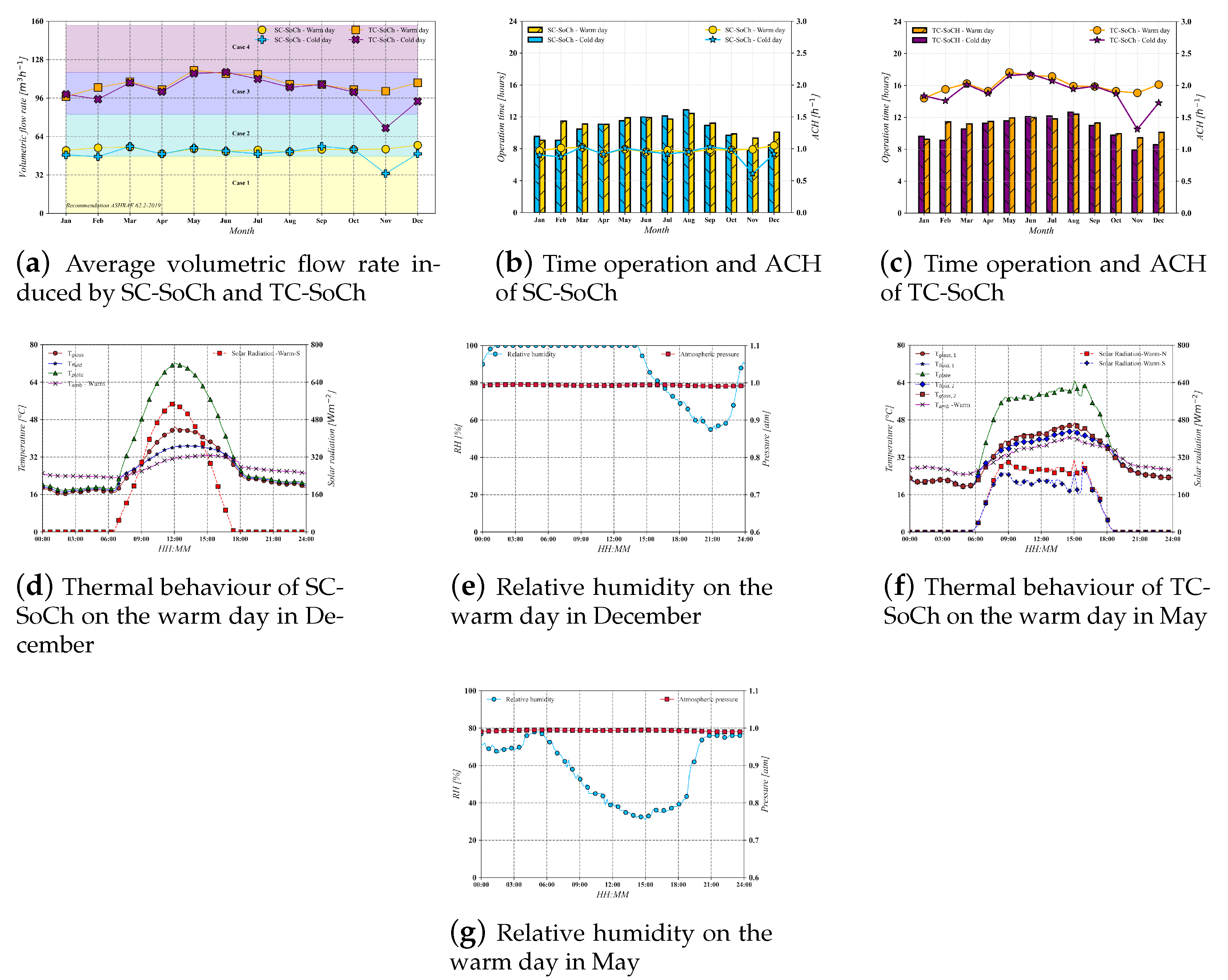
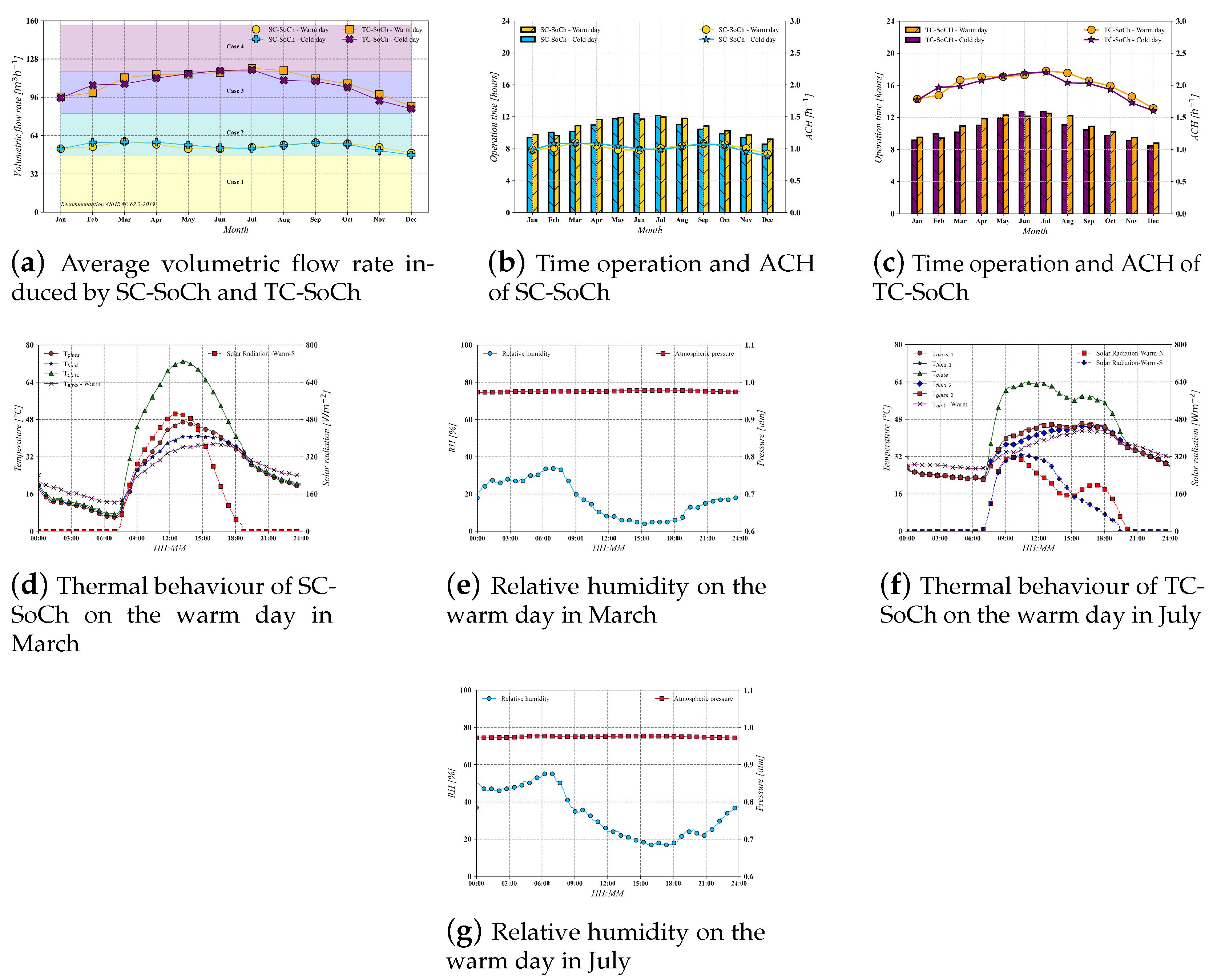
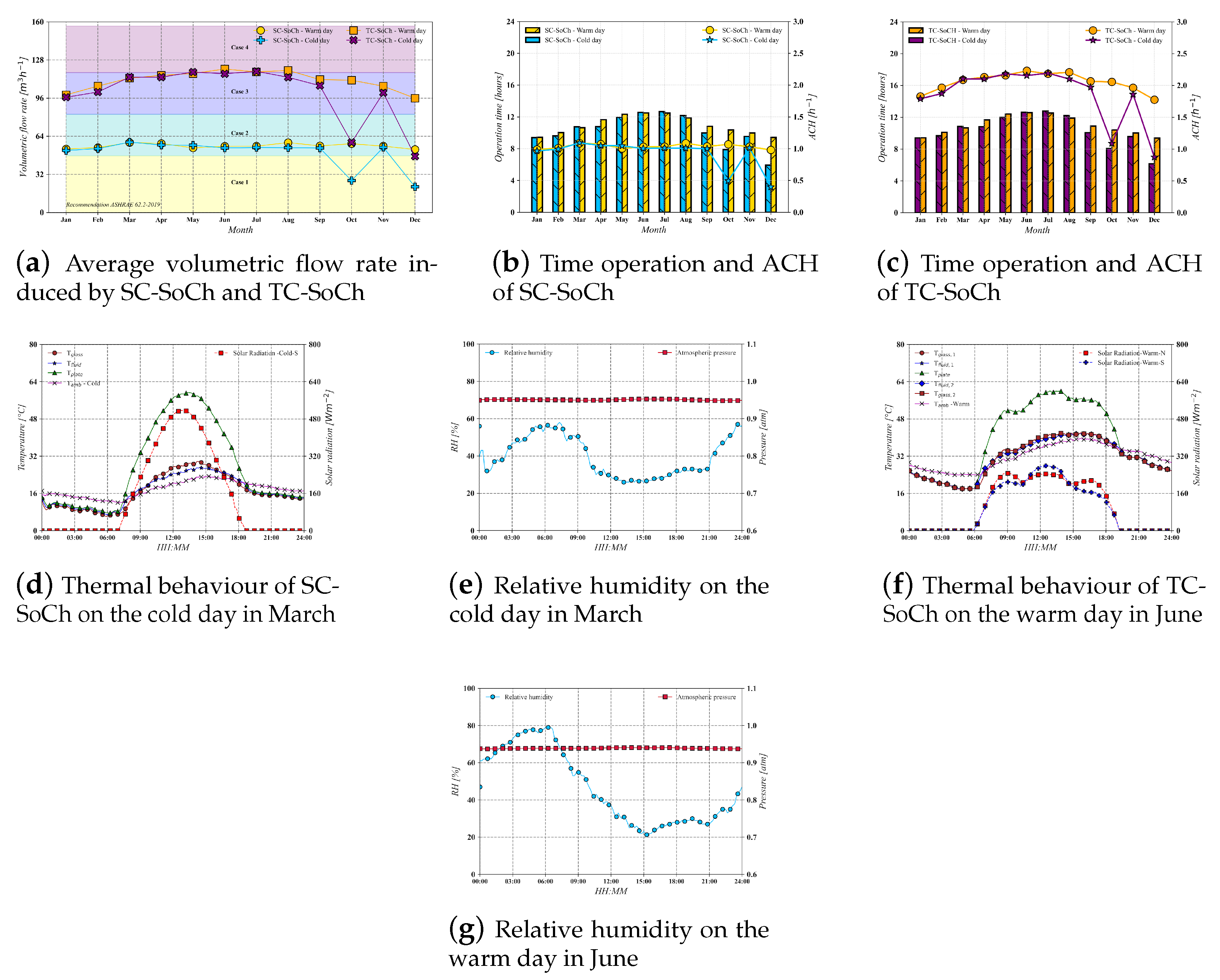
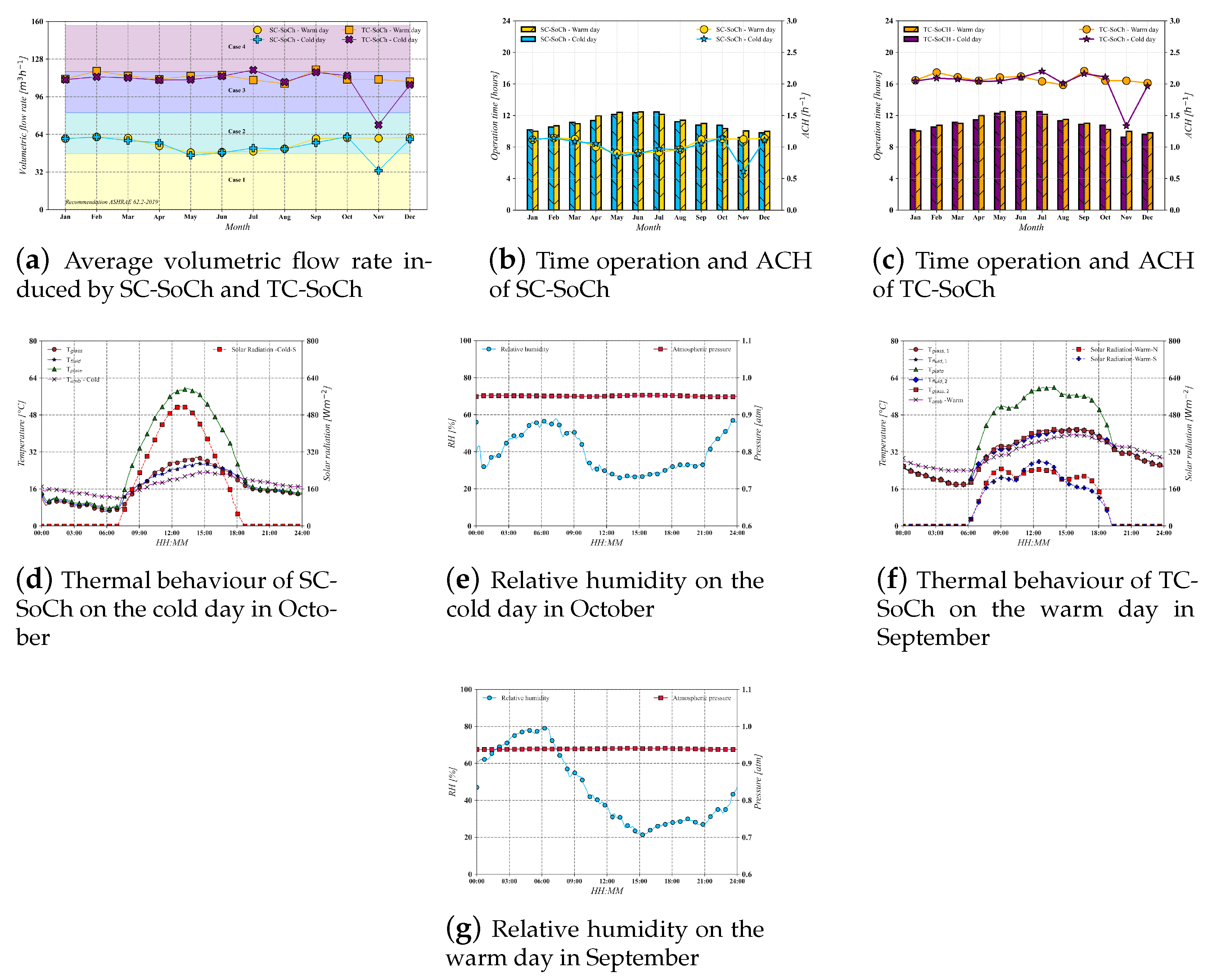
3. Results and Discussion
3.1. Am: Villahermosa
3.2. Aw: Mérida
3.3. BWh: Hermosillo
3.4. BSh: Monterrey
3.5. Cwb: Mexico City
3.6. Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| A | Area (m) | Mean temperature weighting factor (-) | |
| Air change per hour (h) | Thermal conductivity (WmK) | ||
| Specific heat (WkgK) | Material density (kgm) | ||
| Coefficient of discharge (-) | Transmissibility (-) | ||
| f | Mole fraction of water vapor (-) | Kinematic viscosity (ms) | |
| g | Gravitational constant (ms) | Dynamic viscosity (Pa s) | |
| Solar radiation (Wm) | Subscripts | ||
| Grashof number (-) | a | Air | |
| h | Convective heat transfer coefficient (WmK) | Ambient | |
| x-axis distance (m) | Convection | ||
| y-axis distance (m) | External | ||
| z-axis distance (m) | f | Fluid | |
| Mass flow rate (kg s) | g | Glass | |
| Nusselt number (-) | Indoor | ||
| Prandtl number (-) | Inlet | ||
| Atmospheric pressure (atm) | x and y nodes | ||
| Saturation vapor pressure (Pa) | Outdoor | ||
| q | Heat flux (Wm) | Outlet | |
| Rayleigh number (-) | p | Plate | |
| Relative humidity (-) | Radiation | ||
| T | Temperature (°C, K) | Room | |
| t | Time (s) | Sky | |
| Volumetric flow rate (mh) | Solar | ||
| Wind speed (ms) | Solar radiation | ||
| x | x-axis | Saturation vapor | |
| y | y-axis | Thermal insulation | |
| z | z-axis | v | Vapor |
| Z | Compressibility (-) | Wind | |
| Absorptivity (-) | Acronyms | ||
| Thermal expansion coefficient (K) | CFD | Computational fluid dynamics | |
| Difference | GEB | Global energy balance | |
| Layer thickness (m) | IAQ | Indoor air quality | |
| Emissivity (-) | INEGI | Institute of Statistics and Geography | |
| SC | Solar chimney | ||
| SC-SoCh | Single-channel solar chimney | ||
| TC-SoCh | Double-air-channel solar chimney | ||
References
- ANSI/ASHRAE 62.2-2019. Ventilation for Acceptable Indoor Air Quality in Residential Buildings. 2019. Available online: https://www.ashrae.org/about/news/2019/ashrae-releases-updated-versions-of-standard-62-1-and-62-2 (accessed on 15 November 2023).
- Roh, T.; Moreno-Rangel, A.; Baek, J.; Obeng, A.; Hasan, N.T.; Carrillo, G. Indoor Air Quality and Health Outcomes in Employees Working from Home during the COVID-19 Pandemic: A Pilot Study. Atmosphere 2021, 12, 1665. [Google Scholar] [CrossRef]
- Kumar, A.; Moreno-Rangel, A.; Khan, M.A.I.; Piasecki, M. Ventilation and Indoor Air Quality. Atmosphere 2022, 13, 1730. [Google Scholar] [CrossRef]
- Mata, T.M.; Martins, A.A.; Calheiros, C.S.C.; Villanueva, F.; Alonso-Cuevilla, N.P.; Gabriel, M.F.; Silva, G.V. Indoor Air Quality: A Review of Cleaning Technologies. Environments 2022, 9, 118. [Google Scholar] [CrossRef]
- Lavtižar, K.; Fikfak, A.; Fink, R. Overlooked Impacts of Urban Environments on the Air Quality in Naturally Ventilated Schools Amid the COVID-19 Pandemic. Sustainability 2023, 15, 2796. [Google Scholar] [CrossRef]
- Awbi, H. Ventilation of Buildings. 2003. Available online: https://www.taylorfrancis.com/books/mono/10.4324/9780203634479/ventilation-buildings-awbi (accessed on 15 November 2023).
- Wang, Z.; Wang, Y.; Zeng, R.; Srinivasan, R.S.; Ahrentzen, S. Random Forest based hourly building energy prediction. Energy Build. 2018, 171, 11–25. [Google Scholar] [CrossRef]
- International Energy Agency. Key World Energy Statistics; International Energy Agency: Paris, France, 2019. [Google Scholar]
- International Energy Agency. Energy Efficiency; International Energy Agency: Paris, France, 2020. [Google Scholar]
- International Energy Agency. Energy Efficiency; International Energy Agency: Paris, France, 2021. [Google Scholar]
- Secretaría de Energía. Programa para el Desarrollo del Sistema Eléctrico Nacional 2022–2036; Secretaría de Energía: Mexico City, Mexico, 2022. [Google Scholar]
- Instituto Nacional de Estadística y Geografía. Primera Encuesta Nacional Sobre Consumo de Energéticos en Viviendas Particulares (ENCEVI); Instituto Nacional de Estadística y Geografía: Mexico City, Mexico, 2018. [Google Scholar]
- Abdeen, A.; Serageldin, A.A.; Ibrahim, M.G.; El-Zafarany, A.; Ookawara, S.; Murata, R. Solar chimney optimization for enhancing thermal comfort in Egypt: An experimental and numerical study. Sol. Energy 2019, 180, 524–536. [Google Scholar] [CrossRef]
- Li, Y.; Long, T.; Bai, X.; Wang, L.; Li, W.; Liu, S.; Lu, J.; Cheng, Y.; Ye, K.; Huang, S. An experimental investigation on the passive ventilation and cooling performance of an integrated solar chimney and earth–air heat exchanger. Renew. Energy 2021, 175, 486–500. [Google Scholar] [CrossRef]
- Ke, W.; Ji, J.; Xu, L.; Yu, B.; Tian, X.; Wang, J. Numerical study and experimental validation of a multi-functional dual-air-channel solar wall system with PCM. Energy 2021, 227, 120434. [Google Scholar] [CrossRef]
- Correia-da Silva, J.; Silva, A.; De Oliveira Fernandes, E. Passive Cooling in Livestock Buildings. In Proceedings of the Seventh International IBPSA Conference, IBPSA, Rio de Janeiro, Brazil, 13–15 August 2001; pp. 215–218. [Google Scholar]
- Raman, P.; Mande, S.; Kishore, V. A passive solar system for thermal comfort conditioning of buildings in composite climates. Sol. Energy 2001, 70, 319–329. [Google Scholar] [CrossRef]
- Chungloo, S.; Limmeechokchai, B. A field study of free convection in an inclined-roof solar chimney. ScienceAsia 2009, 35, 189–195. [Google Scholar] [CrossRef]
- Salata, F.; Alippi, C.; Tarsitano, A.; Golasi, I.; Coppi, M. A First Approach to Natural Thermoventilation of Residential Buildings through Ventilation Chimneys Supplied by Solar Ponds. Sustainability 2015, 7, 9649–9663. [Google Scholar] [CrossRef]
- Yuan, P.; Fang, Z.; Wang, W.; Chen, Y.; Li, K. Numerical Simulation Analysis and Full-Scale Experimental Validation of a Lower Wall-Mounted Solar Chimney with Different Radiation Models. Sustainability 2023, 15, 11974. [Google Scholar] [CrossRef]
- Arce, J.; Xamán, J.; Alvarez, G.; Jiménez, M.; Guzmán, J.; Heras, M. Theoretical study on a diurnal solar chimney with double air flow. In Proceedings of the 1st International Conference on Solar Heating, Cooling and Buildings (EUROSUN). Sociedade Portuguesa de Energia Solar (SPES), Lisbon, Portugal, 7–10 October 2008; pp. 1–8. [Google Scholar]
- Tlatelpa-Becerra, A. Estudio de la Transferencia de Calor en una Chimenea Solar para Uso Diurno con Doble Canal de Aire. Master’s Thesis, Cenidet, Cuernavaca, Mexico, 2011. [Google Scholar]
- Zavala-Guillén, I.; Xamán, J.; Hernández-Pérez, I.; Hernández-Lopéz, I.; Gijón-Rivera, M.; Chávez, Y. Numerical study of the optimum width of 2a diurnal double air-channel solar chimney. Energy 2018, 147, 403–417. [Google Scholar] [CrossRef]
- Jiménez-Xamán, C.; Xamán, J.; Gijón-Rivera, M.; Zavala-Guillén, I.; Noh-Pat, F.; Simá, E. Assessing the thermal performance of a rooftop solar chimney attached to a single room. J. Build. Eng. 2020, 31, 101380. [Google Scholar] [CrossRef]
- Torres-Aguilar, C.; Moreno-Bernal, P.; Xamán, J.; Nesmachnow, S.; Cisneros-Villalobos, L. Global energy balances for energy analysis in buildings. In Proceedings of the V Ibero-American Congress of Smart Cities, Cuenca, Ecuador, 28–30 November 2022; pp. 306–320. [Google Scholar]
- Tariq, R.; Torres-Aguilar, C.; Sheikh, N.A.; Ahmad, T.; Xamán, J.; Bassam, A. Data engineering for digital twining and optimization of naturally ventilated solar façade with phase changing material under global projection scenarios. Renew. Energy 2022, 187, 1184–1203. [Google Scholar] [CrossRef]
- Cardenas, M.; Tlatelpa-Becerro, A.; Rico-Martínez, R.; Urquiza, G.; Alarcón Hernández, F.; Fuentes-Albarran, M. Prediction of the dynamic behavior of a solar chimney by means of artificial neural networks. Rev. Mex. Ing. Quim. 2022, 21, 1–16. [Google Scholar]
- Awbi, H.; Gan, G. Simulation of solar-induced ventilation. Dep. Constr. Manag. Eng. 1992, 1, 2016–2030. [Google Scholar]
- Bansal, N.; Mathur, R.; Bhandari, M. Solar Chimney for Enhanced Stack Ventilation. Build. Environ. 1993, 28, 373–377. [Google Scholar] [CrossRef]
- Ong, K. Thermal performance of solar air heaters: Mathematical model and solution procedure. Sol. Energy 1995, 55, 93–109. [Google Scholar] [CrossRef]
- Torres-Aguilar, C.E.; Arce, J.; Xamán, J.; Macias-Melo, E. Experimental study and numerical analysis of radiative losses of single-channel solar chimney. J. Build. Phys. 2022, 46, 340–371. [Google Scholar] [CrossRef]
- Ong, K. A mathematical model of a solar chimney. Energy 2003, 28, 1047–1060. [Google Scholar] [CrossRef]
- Duffie, J.; Beckman, W. Solar Engineering of Thermal Processes, 4th ed.; Wiley: Hoboken, NJ, USA, 2013. [Google Scholar]
- Kalogirou, S. Solar Energy Engineering: Processes and Systems, 4th ed.; Academic Press: Cambridge, MA, USA, 2009. [Google Scholar]
- Chantawong, P.; Hirunlabh, J.; Zeghmati, B.; Khedari, J.; Teekasap, S.; Win, M.M. Investigation on thermal performance of glazed solar chimney walls. Sol. Energy 2006, 80, 288–297. [Google Scholar] [CrossRef]
- Swinbank, W. Long-wave radiation from clear skies. Q. J. R. Meteorol. Soc. 1963, 89, 339–348. [Google Scholar] [CrossRef]
- McAdams, W. Heat Transmission, 1st ed.; McGraw-Hill: New York, NY, USA, 1954. [Google Scholar]
- Churchill, S.W.; Chu, H. Correlating equations for laminar and turbulent free convection from a horizontal cylinder. Int. J. Heat Mass Transf. 1975, 18, 1049–1053. [Google Scholar] [CrossRef]
- Bergman, T.L.; Lavine, A.S.; Incropera, F.P.; DeWitt, D.P. Fundamentals of Heat and Mass Transfer, 8th ed.; Wiley: Hoboken, NJ, USA, 2018. [Google Scholar]
- Martí, J.; Heras-Celemin. Dynamic physical model for a solar chimney. Sol. Energy 2007, 81, 614–622. [Google Scholar] [CrossRef]
- Bansal, N.; Mathur, J.; Mathur, S.; Jain, M. Modeling of window-sized solar chimneys for ventilation. Build. Environ. 2005, 40, 1302–1308. [Google Scholar] [CrossRef]
- Mathur, J.; Bansal, N.; Jain, M. Experimental investigations on solar chimney for room ventilation. Sol. Energy 2006, 80, 927–935. [Google Scholar] [CrossRef]
- Arce, J.; Xamán, J.; Alvarez, G.; Jiménez, M.; Enríquez, R.; Heras, M. A Simulation of the Thermal Performance of a Small Solar Chimney Already Installed in a Building. J. Sol. Energy Eng. 2013, 135, 011005-1–011005–10. [Google Scholar] [CrossRef]
- Giacomo, P. Equation for the determination of the density of moist air. Metrologia 1982, 18, 33–40. [Google Scholar] [CrossRef]
- Davis, R. Equation for the determination of the density of moist air. Metrologia 1992, 29, 67–70. [Google Scholar] [CrossRef]
- Andersen, K. Theoretical considerations on natural ventilation by thermal buoyancy. Ashrae 1995, 101, 1103–1117. [Google Scholar]
- Ke, W.; Ji, J.; Wang, C.; Zhang, C.; Xie, H.; Tang, Y.; Lin, Y. Comparative analysis on the electrical and thermal performance of two CdTe multi-layer ventilated windows with and without a middle PCM layer: A preliminary numerical study. Renew. Energy 2022, 189, 1306–1323. [Google Scholar] [CrossRef]
- Duan, S. A predictive model for airflow in a typical solar chimney based on solar radiation. J. Build. Eng. 2019, 26, 100916. [Google Scholar] [CrossRef]
- INEGI. Guía para la Interpretación de Cartografía Climatológica; Instituto Nacional de Estadistica, Geografia e Informatica: Mexico City, Mexico, 2005. [Google Scholar]
- CONABIO. Sistema Nacional de Información sobre Biodiversidad (SNIB); Comision Nacional para el Conocimiento y Uso de la Biodiversidad: Mexico City, Mexico, 2023. [Google Scholar]
- García, E. Modificaciones al Sistema de Clasificación Climática de Köppen, 1st ed.; Universidad Nacional Autónoma de México: Mexico City, Mexico, 2004. [Google Scholar]
- CONAGUA. Estaciones Meteorológicas Automáticas (EMAS); Comision Nacional del Agua: Mexico City, Mexico, 2023. [Google Scholar]
- INEGI. Censo de Población y Vivienda 2020; Instituto Nacional de Estadistica, Geografia e Informatica: Aguascalientes, Mexico, 2020. [Google Scholar]
- Jianliu, X.; Weihua, L. Study on solar chimney used for room natural ventilation in Nanjing. Energy Build. 2013, 66, 467–469. [Google Scholar] [CrossRef]
- ASTM C578-01; Standard Specification for Rigid, Cellular Polystyrene Thermal Insulation. ASTM International: West Conshohocken, PA, USA, 2002.
- Li, Y.; Liu, S.; Lu, J. Effects of various parameters of a PCM on thermal performance of a solar chimney. Appl. Therm. Eng. 2017, 127, 1119–1131. [Google Scholar] [CrossRef]


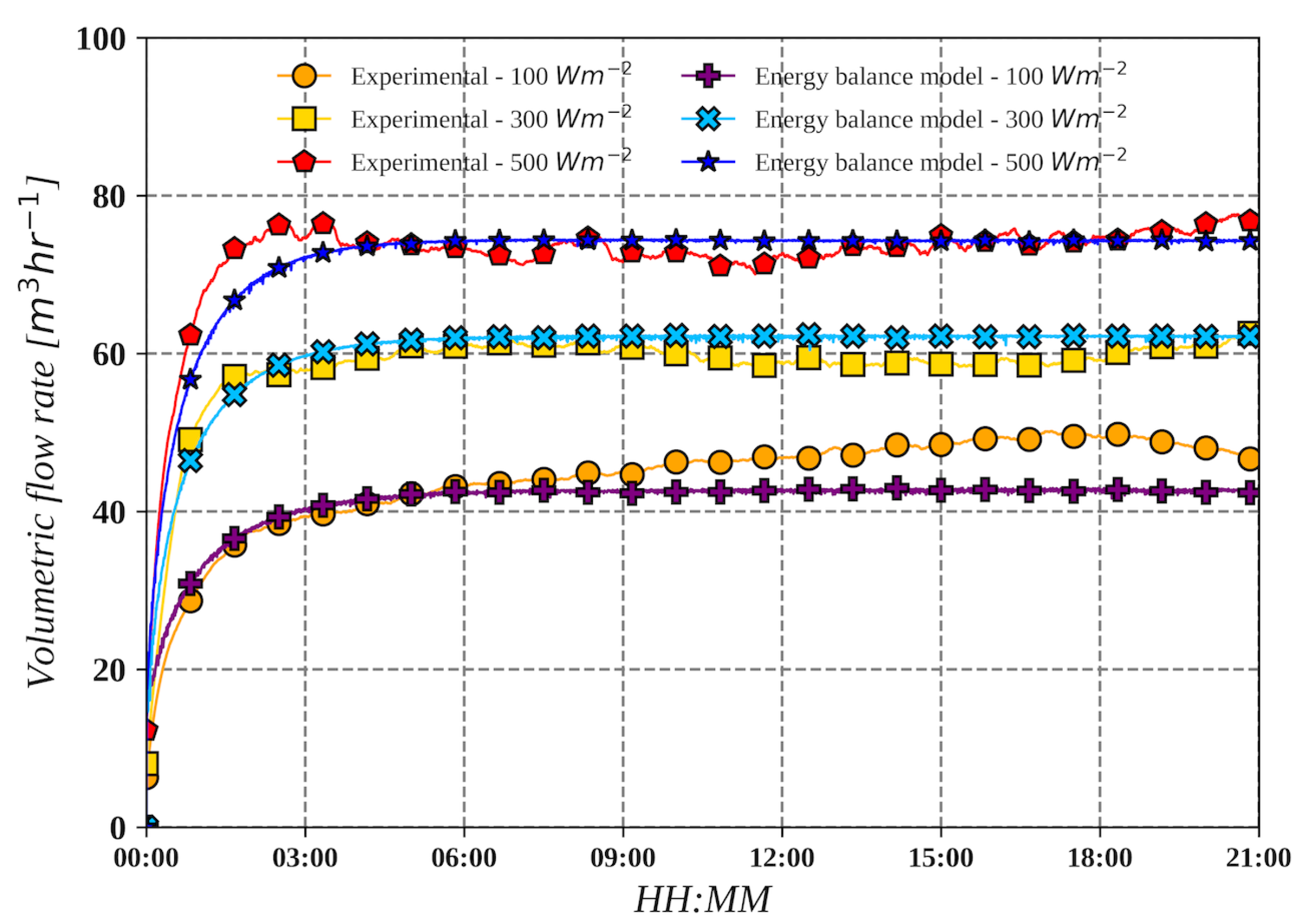

| Parameter | Experimental Evaluation: 100 Wm−2 | Experimental Evaluation: 300 Wm−2 | Experimental Evaluation: 500 Wm−2 |
|---|---|---|---|
| Mass flow rate | 11.79% | 5.46% | 5.04% |
| Volumetric flow rate | 11.25% | 6.19% | 3.69% |
| Temperature of fluid | 5.11% | 8.75% | 10.13% |
| Temperature of absorber plate | 3.20% | 7.12% | 9.67% |
| SC-SoCh and TC-SoCh | ||
|---|---|---|
| Height = = 2.0 m Width = = 1.0 m | Air gap = = = = 0.15 m | |
| Glass cover | Absorber plate (Aluminum with a cover of matte black paint) | Thermal insulation (extruded polystyrene) |
| = 0.003 m = 2500 kg m = 750 J kg K = 1.4 W m K = 0.085 = 0.838 = 0.840 | = 0.0015 m = 2770 kg m = 875 J kg K = 177 W m K = 0.97 = 0.90 | = 0.0508 m = 21 kg m = 1210 J kg K = 0.0001 + 0.0262 W m K = 0.94 |
| Cases evaluated according to ANSI/ASHRAE 62.2-2019 | ||
| Configurations | Parameters | Dwelling-unit ventilation |
| Reference Case | Volume = 27 m Area of floor = 9 m Number of bedrooms = 1 | = 30.06 mh |
| Case 1 | Volume = 54 m Area of floor = 18 m Number of bedrooms = 2 | = 47.52 mh |
| Case 2 | Volume = 108 m Area of floor = 36 m Number of bedrooms = 4 | = 82.44 mh |
| Case 3 | Volume = 162 m Area of floor = 54 m Number of bedrooms = 6 | = 117.36 mh |
| Case 4 | Volume = 216 m Area of floor = 72 m Number of bedrooms = 7 | = 152.28 mh |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Torres-Aguilar, C.E.; Moreno-Bernal, P.; Nesmachnow, S.; Aguilar-Castro, K.M.; Cisneros-Villalobos, L.; Arce, J. Annual Evaluation of Natural Ventilation Induction in Solar Chimneys under Tropical, Dry, and Temperate Climates of Mexico: A Case Study. Sustainability 2023, 15, 16399. https://doi.org/10.3390/su152316399
Torres-Aguilar CE, Moreno-Bernal P, Nesmachnow S, Aguilar-Castro KM, Cisneros-Villalobos L, Arce J. Annual Evaluation of Natural Ventilation Induction in Solar Chimneys under Tropical, Dry, and Temperate Climates of Mexico: A Case Study. Sustainability. 2023; 15(23):16399. https://doi.org/10.3390/su152316399
Chicago/Turabian StyleTorres-Aguilar, Carlos E., Pedro Moreno-Bernal, Sergio Nesmachnow, Karla M. Aguilar-Castro, Luis Cisneros-Villalobos, and Jesús Arce. 2023. "Annual Evaluation of Natural Ventilation Induction in Solar Chimneys under Tropical, Dry, and Temperate Climates of Mexico: A Case Study" Sustainability 15, no. 23: 16399. https://doi.org/10.3390/su152316399
APA StyleTorres-Aguilar, C. E., Moreno-Bernal, P., Nesmachnow, S., Aguilar-Castro, K. M., Cisneros-Villalobos, L., & Arce, J. (2023). Annual Evaluation of Natural Ventilation Induction in Solar Chimneys under Tropical, Dry, and Temperate Climates of Mexico: A Case Study. Sustainability, 15(23), 16399. https://doi.org/10.3390/su152316399








