Reliability Assessment of Reinforced Concrete Beams under Elevated Temperatures: A Probabilistic Approach Using Finite Element and Physical Models
Abstract
1. Introduction
2. Reliability Analysis
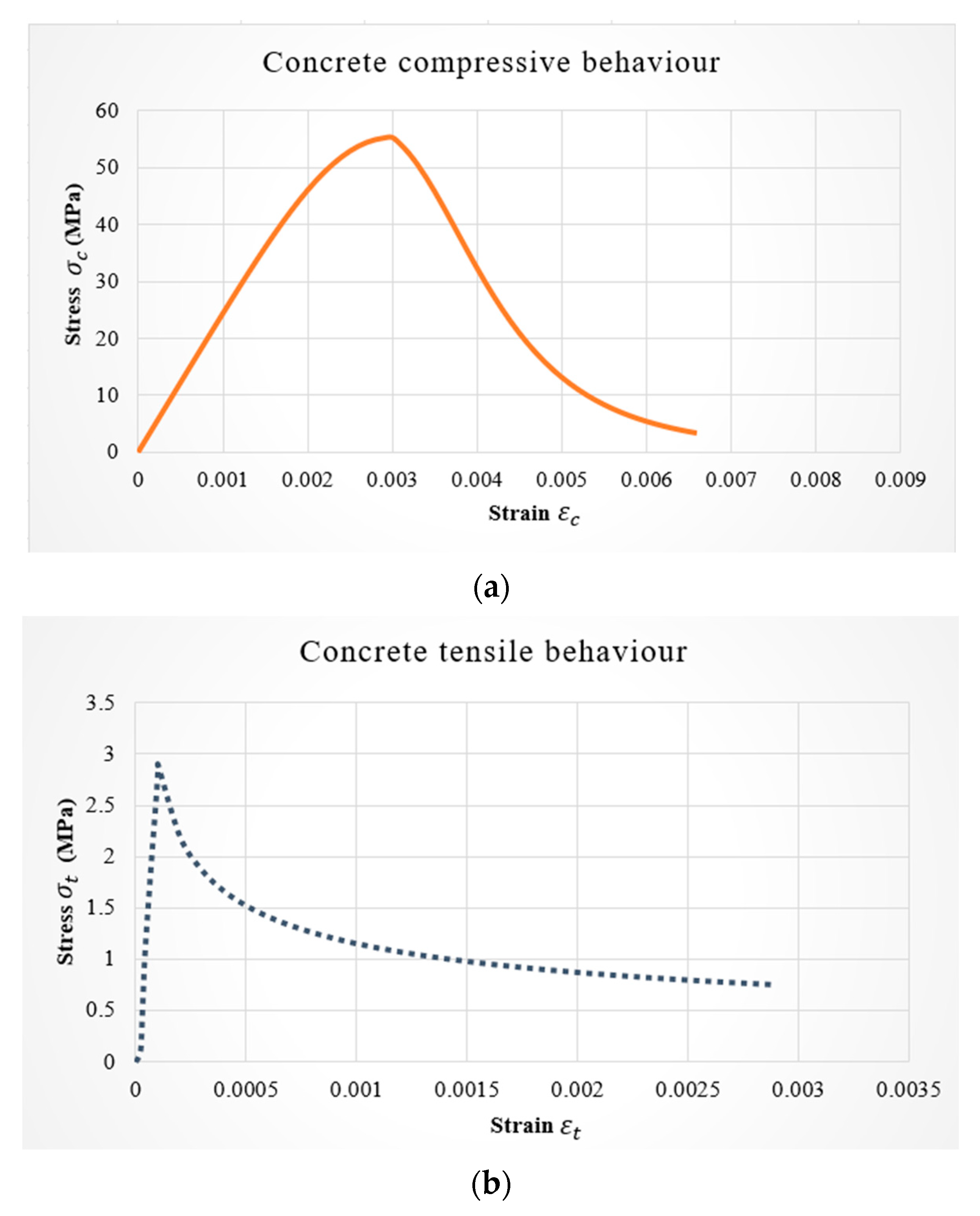
3. The Adopted Constitutive Model
4. Experimental Tests
5. Numerical Modelling
5.1. Finite Element Model
5.2. Model Validation
5.3. Reinforced Concrete at Elevated Temperatures
5.4. Introducing Reliability Analysis
6. Conclusions
- In cases of deterministic design, when temperature is increased, the damage pattern, and stress intensity distributions are extended away from the middle area of the model.
- For all models with different temperature cases, it was shown that by considering β, the corresponding loads and displacements were changed from the resulting values in deterministic designs due to considering concrete properties as random variables.
- The intensity of the tensile damage pattern and intensity of stresses in cases of reliability-based design are less than was observed in cases of deterministic models for each temperature case.
- The results showed that as β increases, the corresponding load and displacement values decrease for each temperature case in the case of probabilistic analysis.
- The pattern of tensile damage and the stress intensities become less intensive as β increases for each temperature case in the case of probabilistic approach. Therefore, β can work as a controlling bound for producing a safe plastic design.
- Design codes and guidelines for reinforced concrete structures should incorporate probabilistic approaches to account for the uncertainties in material properties and loading conditions. This can help to ensure more reliable and safer designs.
- The proposed approach can be extended to other types of reinforced concrete structures, such as columns and slabs, to investigate their behavior under elevated temperatures.
- Future research can focus on investigating the effect of other parameters on the reliability of reinforced concrete structures at high temperatures, such as the effect of different types of reinforcements, different loading conditions, and the effect of cooling methods.
- The developed approach can be combined with other methods, such as fire resistance tests, to validate and improve the accuracy of the numerical models.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| Variable | Full Variable Description |
| The effective compression internal force | |
| The effective uniaxial compressive stress | |
| Effective internal force | |
| Internal force | |
| The effective tension internal force | |
| The effective uniaxial tensile stress | |
| Elastic stiffness of the material | |
| Failure domain | |
| Degraded elastic stiffness | |
| The initial Young’s modulus | |
| Probability of failure | |
| Independent random vectors | |
| Variable of compression damage | |
| Variable of tension damage | |
| Probability density function | |
| Compressive strain | |
| The equivalent compression plastic strains | |
| Strain tensor | |
| Elastic part of strain tensor | |
| Plastic part of strain tensor | |
| Tensile strain | |
| The equivalent tension plastic strain | |
| d | Stiffness degradation |
| fb0/fc0 | The ratio of initial equi-biaxial compressive yield stress to initial uniaxial compressive yield stress |
| K | Softening parameter |
| Number of sample points | |
| Mean value | |
| Variance | |
| Reliability index |
References
- Demir, A.; Caglar, N.; Ozturk, H.; Sumer, Y. Nonlinear Finite Element Study on the Improvement of Shear Capacity in Reinforced Concrete T-Section Beams by an Alternative Diagonal Shear Reinforcement. Eng. Struct. 2016, 120, 158–165. [Google Scholar] [CrossRef]
- Salih, R.; Zhou, F.Y. Numerical Investigation of the Behavior of Reinforced Concrete Beam Reinforced with FRP Bars. Civ. Eng. J. 2019, 5, 2296–2308. [Google Scholar]
- Kytinou, V.K.; Chalioris, C.E.; Karayannis, C.G. Analysis of Residual Flexural Stiffness of Steel Fiber-Reinforced Concrete Beams with Steel Reinforcement. Materials 2020, 13, 2698. [Google Scholar] [CrossRef] [PubMed]
- Chalioris, C.E.; Kytinou, V.K.; Voutetaki, M.E.; Karayannis, C.G. Flexural Damage Diagnosis in Reinforced Concrete Beams Using a Wireless Admittance Monitoring System—Tests and Finite Element Analysis. Sensors 2021, 21, 679. [Google Scholar] [CrossRef] [PubMed]
- Megarsa, E.; Kenea, G. Numerical Investigation on Shear Performance of Reinforced Concrete Beam by Using Ferrocement Composite. Math. Probl. Eng. 2022, 2022, 5984177. [Google Scholar] [CrossRef]
- Kytinou, V.K.; Kosmidou, P.-M.K.; Chalioris, C.E. Numerical Analysis Exterior RC Beam-Column Joints with CFRP Bars as Beam’s Tensional Reinforcement under Cyclic Reversal Deformations. Appl. Sci. 2022, 12, 7419. [Google Scholar] [CrossRef]
- Georgali, B.; Tsakiridis, P.E. Microstructure of Fire-Damaged Concrete. A Case Study. Cem. Concr. Compos. 2005, 27, 255–259. [Google Scholar] [CrossRef]
- Janotka, I.; Nürnbergerová, T. Effect of Temperature on Structural Quality of the Cement Paste and High-Strength Concrete with Silica Fume. Nucl. Eng. Des. 2005, 235, 2019–2032. [Google Scholar] [CrossRef]
- Ali, F.; Nadjai, A.; Silcock, G.; Abu-Tair, A. Outcomes of a Major Research on Fire Resistance of Concrete Columns. Fire Saf. J. 2004, 39, 433–445. [Google Scholar] [CrossRef]
- Sakr, K.; El-Hakim, E. Effect of High Temperature or Fire on Heavy Weight Concrete Properties. Cem. Concr. Res. 2005, 35, 590–596. [Google Scholar] [CrossRef]
- Luccioni, B.M.; Figueroa, M.I.; Danesi, R.F. Thermo-Mechanic Model for Concrete Exposed to Elevated Temperatures. Eng. Struct. 2003, 25, 729–742. [Google Scholar] [CrossRef]
- Arioz, O. Effects of Elevated Temperatures on Properties of Concrete. Fire Saf. J. 2007, 42, 516–522. [Google Scholar] [CrossRef]
- Hertz, K.D. Concrete Strength for Fire Safety Design. Mag. Concr. Res. 2015, 57, 445–453. [Google Scholar] [CrossRef]
- Bazant, Z.P.; Kaplan, M.F.; Haslach, H.W. Concrete at High Temperatures: Material Properties and Mathematical Models. Appl. Mech. Rev. 1997, 50, B75. [Google Scholar]
- Zhang, B.; Bicanic, N.; Pearce, C.J.; Balabanic, G. Residual Fracture Properties of Normal- and High-Strength Concrete Subject to Elevated Temperatures. Mag. Concr. Res. 2015, 52, 123–136. [Google Scholar] [CrossRef]
- Ismail Ahmed Ali, S.; Lublóy, E. Effect of Elevated Temperature on the Magnetite and Quartz Concrete at Different W/C Ratios as Nuclear Shielding Concretes. Nucl. Mater. Energy 2022, 33, 101234. [Google Scholar] [CrossRef]
- Esfahani, M.; Hoseinzade, M.; Shakiba, M.; Arbab, F.; Yekrangnia, M.; Pachideh, G. Experimental Investigation of Residual Flexural Capacity of Damaged Reinforced Concrete Beams Exposed to Elevated Temperatures. Eng. Struct. 2021, 240, 112388. [Google Scholar] [CrossRef]
- Kodur, V.K.R.; Dwaikat, M.B.; Fike, R.S. An Approach for Evaluating the Residual Strength of Fire-Exposed RC Beams. Mag. Concr. Res. 2010, 62, 479–488. [Google Scholar] [CrossRef]
- Abid, S.R.; Abbass, A.A.; Murali, G.; Al-Sarray, M.L.J.; Nader, I.A.; Ali, S.H. Repeated Impact Response of Normal-and High-Strength Concrete Subjected to Temperatures up to 600 C. Materials 2022, 15, 5283. [Google Scholar] [CrossRef]
- Cho, H.-C.; Han, S.-J.; Heo, I.; Kang, H.; Kang, W.-H.; Kim, K.S. Heating Temperature Prediction of Concrete Structure Damaged by Fire Using a Bayesian Approach. Sustainability 2020, 12, 4225. [Google Scholar] [CrossRef]
- Ilango, S.; Mahato, S. Behaviour of Polypropylene Fibre-Reinforced Concrete Beam with CFRP Reinforcement under Elevated Temperature. Asian J. Civ. Eng. 2020, 21, 677–694. [Google Scholar] [CrossRef]
- Kakae, N.; Miyamoto, K.; Momma, T.; Sawada, S.; Kumagai, H.; Ohga, Y.; Hirai, H.; Abiru, T. Physical and Thermal Properties of Concrete Subjected to High Temperature. J. Adv. Concr. Technol. 2017, 15, 190–212. [Google Scholar] [CrossRef]
- Xiao, J.; König, G. Study on Concrete at High Temperature in China—An Overview. Fire Saf. J. 2004, 39, 89–103. [Google Scholar] [CrossRef]
- Cioni, P.; Croce, P.; Salvatore, W. Assessing Fire Damage to r.c. Elements. Fire Saf. J. 2001, 36, 181–199. [Google Scholar] [CrossRef]
- Demirel, B.; Keleştemur, O. Effect of Elevated Temperature on the Mechanical Properties of Concrete Produced with Finely Ground Pumice and Silica Fume. Fire Saf. J. 2010, 45, 385–391. [Google Scholar] [CrossRef]
- Savva, A.; Manita, P.; Sideris, K.K. Influence of Elevated Temperatures on the Mechanical Properties of Blended Cement Concretes Prepared with Limestone and Siliceous Aggregates. Cem. Concr. Compos. 2005, 27, 239–248. [Google Scholar] [CrossRef]
- Hlavička, V.; Hlavicka-Laczák, L.E.; Lublóy, É. Residual Fracture Mechanical Properties of Quartz and Expanded Clay Aggregate Concrete Subjected to Elevated Temperature. Constr. Build. Mater. 2022, 328, 126845. [Google Scholar] [CrossRef]
- Song, Y.; Fu, C.; Liang, S.; Yin, A.; Dang, L. Fire Resistance Investigation of Simple Supported RC Beams with Varying Reinforcement Configurations. Adv. Civ. Eng. 2019, 2019, 8625360. [Google Scholar] [CrossRef]
- Cai, B.; Li, B.; Fu, F. Finite Element Analysis and Calculation Method of Residual Flexural Capacity of Post-Fire RC Beams. Int. J. Concr. Struct. Mater. 2020, 14, 58. [Google Scholar] [CrossRef]
- Agrawal, A.; Kodur, V. Residual Response of Fire-Damaged High-Strength Concrete Beams. Fire Mater. 2019, 43, 310–322. [Google Scholar] [CrossRef]
- Yuye, X.; Bo, W.; Ronghui, W.; Ming, J.; Yi, L.U.O. Experimental Study on Residual Performance of Reinforced Concrete Beams after Fire. J. Build. Struct. 2013, 34, 20. [Google Scholar]
- Li, L.Y.; Purkiss, J. Stress–Strain Constitutive Equations of Concrete Material at Elevated Temperatures. Fire Saf. J. 2005, 40, 669–686. [Google Scholar] [CrossRef]
- European Committee for Standardization. Eurocode 2: Design of Concrete Structures-Part 1.2 General Rules-Structural Fire Design; European Committee for Standardization: Brussels, Belgium, 2004. [Google Scholar]
- Kim, J.K.; Han, S.H.; Song, Y.C. Effect of Temperature and Aging on the Mechanical Properties of Concrete: Part I. Experimental Results. Cem. Concr. Res. 2002, 32, 1087–1094. [Google Scholar] [CrossRef]
- ACI Committee 318. Building Code Requirements for Reinforced Concrete; American Concrete Institute: Farmington Hills, MI, USA, 1995. [Google Scholar]
- Felicetti, R.; Gambarova, P.G.; Meda, A. Residual Behavior of Steel Rebars and R/C Sections after a Fire. Constr. Build. Mater. 2009, 23, 3546–3555. [Google Scholar] [CrossRef]
- Dotreppe, J.C. Mechanical Properties of Quenched and Self-Tempered Reinforcing Steel at Elevated Temperatures Compared with Recommendations of Eurocode 2-Part 1–2. Mater. Struct. 1997, 30, 430–438. [Google Scholar] [CrossRef]
- Qiu, Z.; Huang, R.; Wang, X.; Qi, W. Structural Reliability Analysis and Reliability-Based Design Optimization: Recent Advances. Sci. China Phys. Mech. Astron. 2013, 56, 1611–1618. [Google Scholar] [CrossRef]
- Arangio, S. Reliability Based Approach for Structural Design and Assessment: Performance Criteria and Indicators in Current European Codes and Guidelines. Int. J. Lifecycle Perform. Eng. 2012, 1, 64–91. [Google Scholar] [CrossRef]
- Van Cao, V. Reliability-Based Moment Capacity Assessment of Reinforced Concrete Beams in Fire. Int. J. Civ. Eng. 2022, 20, 1291–1308. [Google Scholar] [CrossRef]
- Van Dao, D.; Adeli, H.; Ly, H.-B.; Le, L.M.; Le, V.M.; Le, T.-T.; Pham, B.T. A Sensitivity and Robustness Analysis of GPR and ANN for High-Performance Concrete Compressive Strength Prediction Using a Monte Carlo Simulation. Sustainability 2020, 12, 830. [Google Scholar] [CrossRef]
- Benyahi, K.; Kachi, M.S.; Bouafia, Y.; Barboura, S.; Li, J. Reliability Assessment of the Behavior of Reinforced and/or Prestressed Concrete Beams Sections in Shear Failure. Frat. Integr. Strutt. 2021, 15, 195–222. [Google Scholar] [CrossRef]
- Roy, A.; Robuschi, S.; Hendriks, M.A.N.; Belletti, B. Safety Assessment of Existing Reinforced Concrete Beams Using Probabilistic Methods at Different Levels. In Key Engineering Materials; Trans Tech Publications Ltd.: Stafa-Zurich, Switzerland, 2016; Volume 711, pp. 958–965. [Google Scholar]
- Rakoczy, A.M.; Nowak, A.S. Reliability-Based Sensitivity Analysis for Prestressed Concrete Girder Bridges. PCI J. 2013, 58, 81–92. [Google Scholar] [CrossRef]
- Słowik, M.; Skrzypczak, I.; Kotynia, R.; Kaszubska, M. The Application of a Probabilistic Method to the Reliability Analysis of Longitudinally Reinforced Concrete Beams. Procedia Eng. 2017, 193, 273–280. [Google Scholar] [CrossRef]
- Olmati, P.; Sagaseta, J.; Cormie, D.; Jones, A.E.K. Simplified Reliability Analysis of Punching in Reinforced Concrete Flat Slab Buildings under Accidental Actions. Eng. Struct. 2017, 130, 83–98. [Google Scholar] [CrossRef]
- Schlune, H.; PLoS, M.; Gylltoft, K. Safety Formats for Non-Linear Analysis of Concrete Structures. Mag. Concr. Res. 2012, 64, 563–574. [Google Scholar] [CrossRef]
- Eamon, C.D.; Jensen, E. Reliability Analysis of RC Beams Exposed to Fire. J. Struct. Eng. 2013, 139, 212–220. [Google Scholar] [CrossRef]
- Li, Z.; Xiao, J.; Xie, Q. Reliability Analysis of the Residual Moment Capacity of High-Strength Concrete Beams after Elevated Temperatures. Struct. Concr. 2021, 22, 1586–1599. [Google Scholar] [CrossRef]
- Haldar, A.; Mahadevan, S. Probability, Reliability, and Statistical Methods in Engineering Design; John Wiley & Sons Incorporated: New York, NY, USA, 2000. [Google Scholar]
- ICS 91.010.30; Others Eurocode—Basis of Structural Design. CEN: Brussels, Belgium, 2002.
- Smith, M. ABAQUS/Standard User’s Manual, Version 6.9; Dassault Systèmes Simulia Corp: Mayfield Heights, OH, USA, 2009. [Google Scholar]
- Schneider, U. Concrete at High Temperatures—A General Review. Fire Saf. J. 1988, 13, 55–68. [Google Scholar] [CrossRef]
- Meda, A.; Gambarova, P.G.; Bonomi, M. High-Performance Concrete in Fire-Exposed Reinforced Concrete Sections. Struct. J. 2002, 99, 277–287. [Google Scholar] [CrossRef]
- Narayanan, R.S. EN1992 Eurocode 2: Design of Concrete Structures. In Institution of Civil Engineers-Civil Engineering; Thomas Telford Ltd.: London, UK, 2001; Volume 144, pp. 23–28. [Google Scholar]
- Wiśniewski, D.F.; Cruz, P.J.S.; Henriques, A.A.R.; Simões, R.A.D. Probabilistic Models for Mechanical Properties of Concrete, Reinforcing Steel and Pre-Stressing Steel. Struct. Infrastruct. Eng. 2009, 8, 111–123. [Google Scholar] [CrossRef]







| Diameter (mm) | Yield Strength (MPa) | Ultimate Tensile Strength (MPa) | |
|---|---|---|---|
| ϕ = 12 mm | 540 | 662 | 200,000 |
| ϕ = 8 mm | 583 | 687 | 200,000 |
| Dilation Angle | Eccentricity | fb0/fc0 | K |
| 30 | 0.1 | 1.16 | 0.667 |
| Concrete Tensile Behavior | Concrete Tension Damage | ||
| Yield stress (MPa) | Cracking Strain | Damage Parameter T | Cracking Strain |
| 2.9 | 0 | 0 | 0 |
| 0.754896741 | 0.0029 | 0.739959615 | 0.002878401 |
| Temperature (°C) | Compressive Strength—f’c (MPa) | Young’s Modulus (GPa) |
|---|---|---|
| 20 | 55.30 | 34.95 |
| 150 | 48.60 | 32.70 |
| 350 | 45.30 | 31.60 |
| 450 | 42.50 | 30.60 |
| 750 | 13.82 | 17.40 |
| Temperature (°C) | Fultimate (kN) | U (mm) | Stress and Tensile Damage Intensity | Percentage of the Tensile Damaged Elements (%) |
|---|---|---|---|---|
| 20 | 74 | 33 | 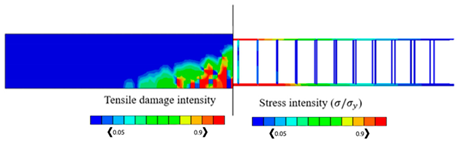 | 3.16 |
| 150 | 57 | 15.60 |  | 4.36 |
| 350 | 48.6 | 10.15 | 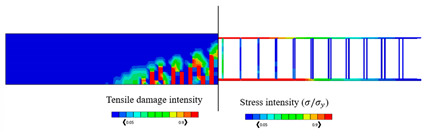 | 4.61 |
| 450 | 36.8 | 12 |  | 6.58 |
| 750 | 36 | 8.18 |  | 41 |
| Parameter | Unit | Distribution | Mean Values | Coefficient of Variation | Source |
|---|---|---|---|---|---|
| Compressive strength (f’c) | MPa | Normal | Table 3 | [56] | |
| Young’s modulus (E0) | GPa | Normal | Table 3 | [56] |
| F (kN) | U (mm) | Stress and Tensile Damage Intensity | Percentage of the Tensile Damaged Elements (%) | |
|---|---|---|---|---|
| 3.40 | 67.4 | 10.67 |  | 2.53 |
| 3.05 | 69.8 | 13.00 |  | 3.47 |
| F (kN) | U (mm) | Stress and Tensile Damage Intensity | Percentage of the Tensile Damaged Elements (%) | |
|---|---|---|---|---|
| 3.40 | 52.6 | 5.09 | 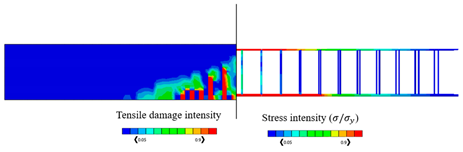 | 2.83 |
| 3.05 | 54.3 | 5.96 |  | 3.00 |
| F (kN) | U (mm) | Stress and Tensile Damage Intensity | Percentage of the Tensile Damaged Elements (%) | |
|---|---|---|---|---|
| 3.40 | 44.7 | 5.11 |  | 3.28 |
| 3.05 | 46.5 | 5.56 |  | 3.44 |
| F (kN) | U (mm) | Stress and Tensile Damage Intensity | Percentage of the Tensile Damaged Elements (%) | |
|---|---|---|---|---|
| 3.40 | 32.1 | 4.94 | 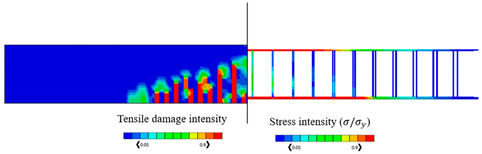 | 5.64 |
| 3.05 | 33.8 | 6.02 | 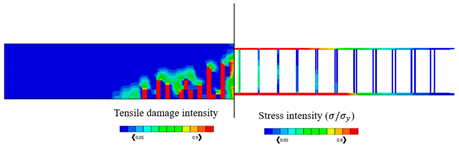 | 6.08 |
| F (kN) | U (mm) | Stress and Tensile Damage Intensity | Percentage of the Tensile Damaged Elements (%) | |
|---|---|---|---|---|
| 3.40 | 31 | 6.57 |  | 26.53 |
| 3.05 | 32.4 | 7.41 |  | 28.69 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Szép, J.; Habashneh, M.; Lógó, J.; Movahedi Rad, M. Reliability Assessment of Reinforced Concrete Beams under Elevated Temperatures: A Probabilistic Approach Using Finite Element and Physical Models. Sustainability 2023, 15, 6077. https://doi.org/10.3390/su15076077
Szép J, Habashneh M, Lógó J, Movahedi Rad M. Reliability Assessment of Reinforced Concrete Beams under Elevated Temperatures: A Probabilistic Approach Using Finite Element and Physical Models. Sustainability. 2023; 15(7):6077. https://doi.org/10.3390/su15076077
Chicago/Turabian StyleSzép, János, Muayad Habashneh, János Lógó, and Majid Movahedi Rad. 2023. "Reliability Assessment of Reinforced Concrete Beams under Elevated Temperatures: A Probabilistic Approach Using Finite Element and Physical Models" Sustainability 15, no. 7: 6077. https://doi.org/10.3390/su15076077
APA StyleSzép, J., Habashneh, M., Lógó, J., & Movahedi Rad, M. (2023). Reliability Assessment of Reinforced Concrete Beams under Elevated Temperatures: A Probabilistic Approach Using Finite Element and Physical Models. Sustainability, 15(7), 6077. https://doi.org/10.3390/su15076077








