Monitoring of Location Parameters with a Measurement Error under the Bayesian Approach Using Ranked-Based Sampling Designs with Applications in Industrial Engineering
Abstract
1. Introduction
2. Bayesian Approach
2.1. Squared Error Loss Function
2.2. Linex Loss Function
3. Ranked Set Sampling
3.1. Median Ranked Set Sampling
3.2. Extreme Ranked Set Sampling
3.3. Measurement Error
3.3.1. Covariate Model
3.3.2. Multiple Measurements Method
4. Suggested Bayesian-AEWMA CC with Different RSS Schemes Using LF under ME
4.1. Proposed Bayesian-AEWMA CC under an ME for Posterior and Posterior Predictive Distribution Using Different RSS Schemes under SELF for Covariate Model
4.2. Proposed Bayesian-AEWMA CC with an ME for Posterior and Posterior Predictive Distribution Using Different RSS Schemes under SELF for Multiple Measurements Method
5. Simulation Study
- The standard normal distribution is selected for both the prior and sampling distribution, and the mean and variance are calculated using distinct LFs, i.e., and .
- For the specific value of the smoothing constant , the value of (threshold) is selected.
- To simulate an in-control process, a random sample of size n is generated from a normal distribution., i.e., .
- Calculate the suggested Bayesian-AEWMA statistic under the Bayesian approach and appraise the design-based procedure;
- If initially, the process is declared in-control, repeat the above steps until it is determined to be out of control, and then write down the frequency of the run-lengths for the in control process.
- For a shifted process, the random samples are selected from the normal distribution, given as follows: .
- Calculate the Bayesian-AEWMA plotting statistic and analyze the process based on the recommended design.
- The aforementioned two steps are repeated until the process shows an out-of-control signal; write down the run length for the in-control process, if it is initially declared to be in-control.
- Compute the ARLs and SDRLs after repeating the above steps 100,000 times.
6. Results and Discussion
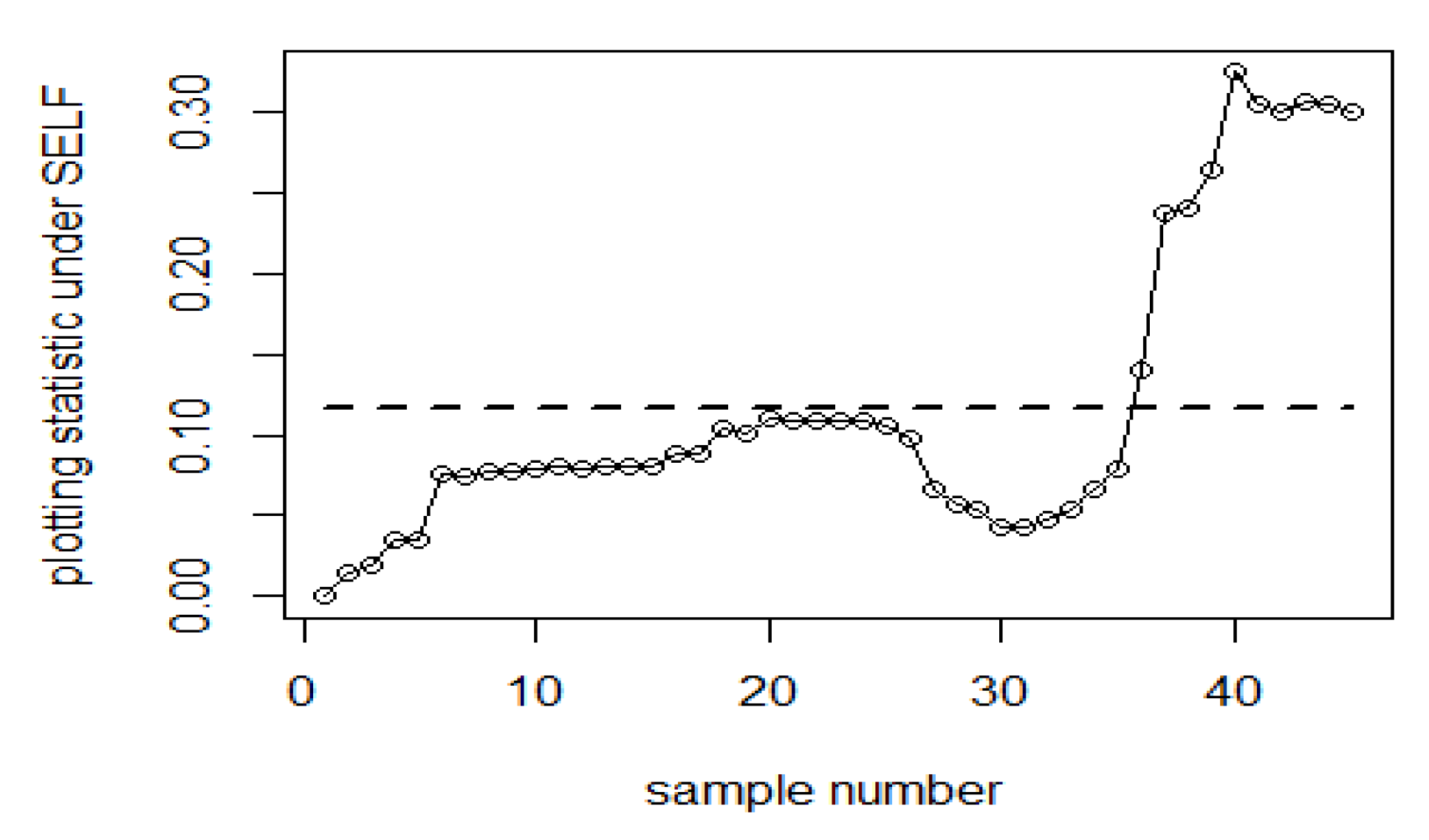
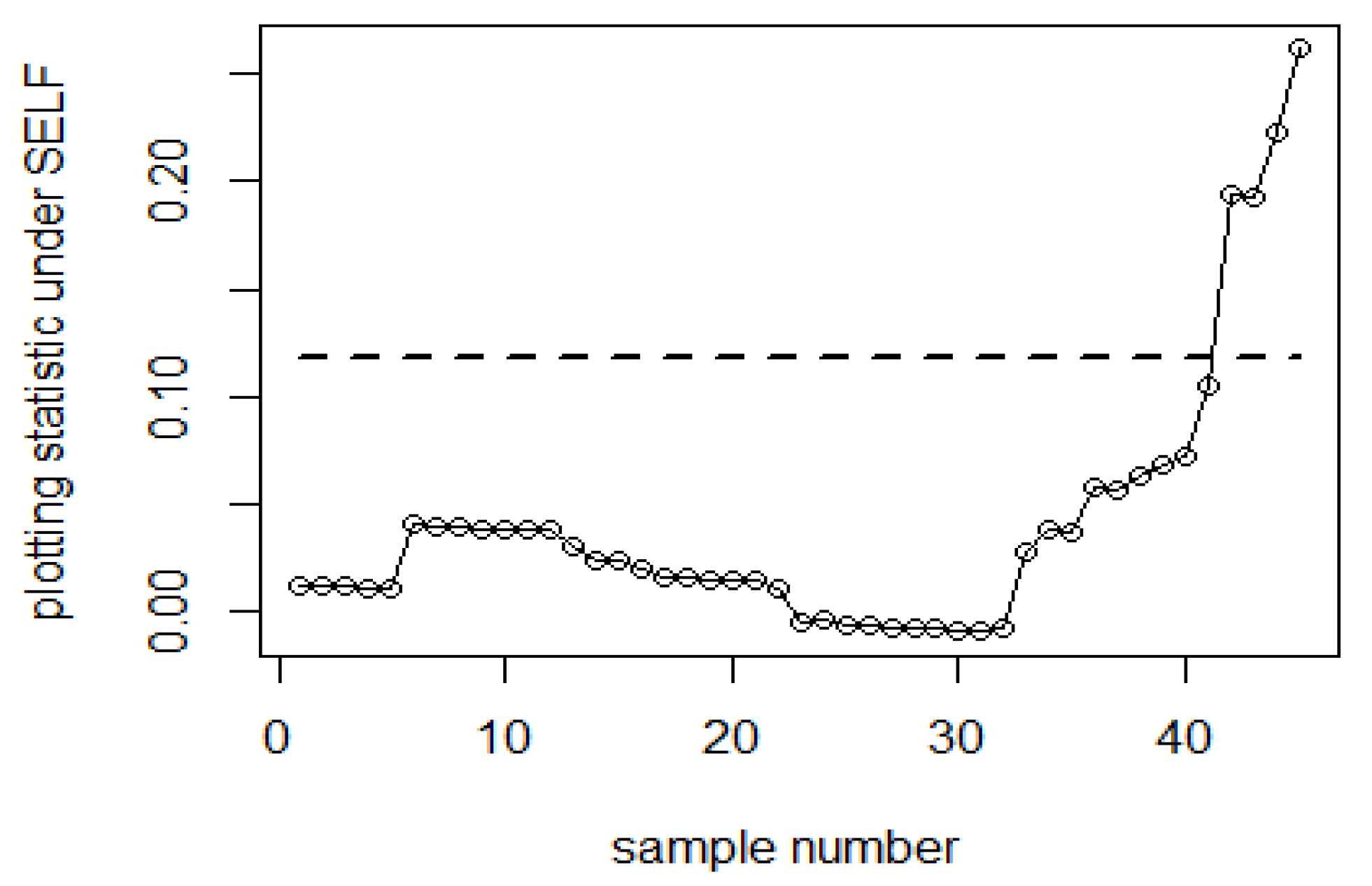
7. Real Data Applications
8. Conclusions
Author Contributions
Funding
Conflicts of Interest
Appendix A
Appendix A.1
Appendix A.2
Appendix A.3
Appendix A.4
References
- Shewhart, W.A. The application of statistics as an aid in maintaining quality of a manufactured product. J. Am. Stat. Assoc. 1925, 20, 546–548. [Google Scholar] [CrossRef]
- Page, E.S. Continuous inspection schemes. Biometrika 1954, 41, 100–115. [Google Scholar] [CrossRef]
- Roberts, S. Control chart tests based on geometric moving averages. Technometrics 1959, 42, 97–101. [Google Scholar] [CrossRef]
- Yoon, S.; Yoon, S.; Shin, Y. A study on the design of adaptive EWMA control chart using Kalman gain recursive average. J. Korean Soc. Qual. Manag. 1996, 24, 73–86. [Google Scholar]
- Capizzi, G.; Masarotto, G. An adaptive exponentially weighted moving average control chart. Technometrics 2003, 45, 199–207. [Google Scholar] [CrossRef]
- Haq, A.; Gulzar, R.; Khoo, M.B. An efficient adaptive EWMA control chart for monitoring the process mean. Qual. Reliab. Eng. Int. 2018, 34, 563–571. [Google Scholar] [CrossRef]
- Mittag, H.-J.; Stemann, D. Gauge imprecision effect on the performance of the XS control chart. J. Appl. Stat. 1998, 25, 307–317. [Google Scholar]
- Stemann, D.; Weihs, C. The EWMA-XS-control chart and its performance in the case of precise and imprecise data. Stat. Pap. 2001, 42, 207–224. [Google Scholar] [CrossRef]
- Linna, K.W.; Woodall, W.H. Effect of measurement error on Shewhart control charts. J. Qual. Technol. 2001, 33, 213–222. [Google Scholar] [CrossRef]
- Huwang, L.; Hung, Y. Effect of measurement error on monitoring multivariate process variability. Stat. Sin. 2007, 17, 749–760. [Google Scholar]
- Noor-ul-Amin, M.; Javaid, A.; Hanif, M.; Dogu, E. Performance of maximum EWMA control chart in the presence of measurement error using auxiliary information. Commun. Stat.-Simul. Comput. 2022, 51, 5482–5506. [Google Scholar] [CrossRef]
- Menzefricke, U. Control charts for the generalized variance based on its predictive distribution. Commun. Stat.—Theory Methods 2007, 36, 1031–1038. [Google Scholar] [CrossRef]
- Wu, Z.; Jiao, J.; Yang, M.; Liu, Y.; Wang, Z. An enhanced adaptive CUSUM control chart. IIE Trans. 2009, 41, 642–653. [Google Scholar] [CrossRef]
- Walden, C.T. An Analysis of Variables Control Charts in the Presence of Measurement Errors. Master’s Thesis, Mississippi State University, Starkville, MI, USA, 1991. [Google Scholar]
- Noor-ul-Amin, M.; Noor, S. Bayesian EWMA control chart with measurement error under different loss functions. Qual. Reliab. Eng. Int. 2021, 37, 3362–3380. [Google Scholar] [CrossRef]
- Khan, I.; Khan, D.M.; Noor-ul-Amin, M.; Khalil, U.; Alshanbari, H.M.; Ahmad, Z. Hybrid EWMA Control Chart under Bayesian Approach Using Ranked Set Sampling Schemes with Applications to Hard-Bake Process. Appl. Sci. 2023, 13, 2837. [Google Scholar] [CrossRef]
- Gauss, C. Methods Moindres Carres Memoire sur la Combination des Observations; Bertrand, J., Translator; Mallet-Bachelier: Paris, France, 1955. [Google Scholar]
- Varian, H.R. A Bayesian approach to real estate assessment. In Studies in Bayesian Econometric and Statistics in Honor of Leonard J. Savage; North-Holland Pub. Co.: Amsterdam, The Netherlands, 1975; pp. 195–208. [Google Scholar]
- McIntyre, G. A method for unbiased selective sampling, using ranked sets. Aust. J. Agric. Res. 1952, 3, 385–390. [Google Scholar] [CrossRef]
- Muttlak, H. Median ranked set sampling. J. Appl. Stat. Sci. 1997, 6, 245–255. [Google Scholar]
- Samawi, H.M.; Ahmed, M.S.; Abu-Dayyeh, W. Estimating the population mean using extreme ranked set sampling. Biom. J. 1996, 38, 577–586. [Google Scholar] [CrossRef]
- Bennett, C.A. Effect of measurement error on chemical process control. Ind. Qual. Control. 1954, 10, 17–20. [Google Scholar]
- Sarwar, M.A.; Noor-ul-Amin, M. Design of a new adaptive EWMA control chart. Qual. Reliab. Eng. Int. 2022, 38, 3422–3436. [Google Scholar] [CrossRef]
- Montgomery, D.C. Introduction to Statistical Quality Control; John Wiley & Sons: Hoboken, NJ, USA, 2009. [Google Scholar]
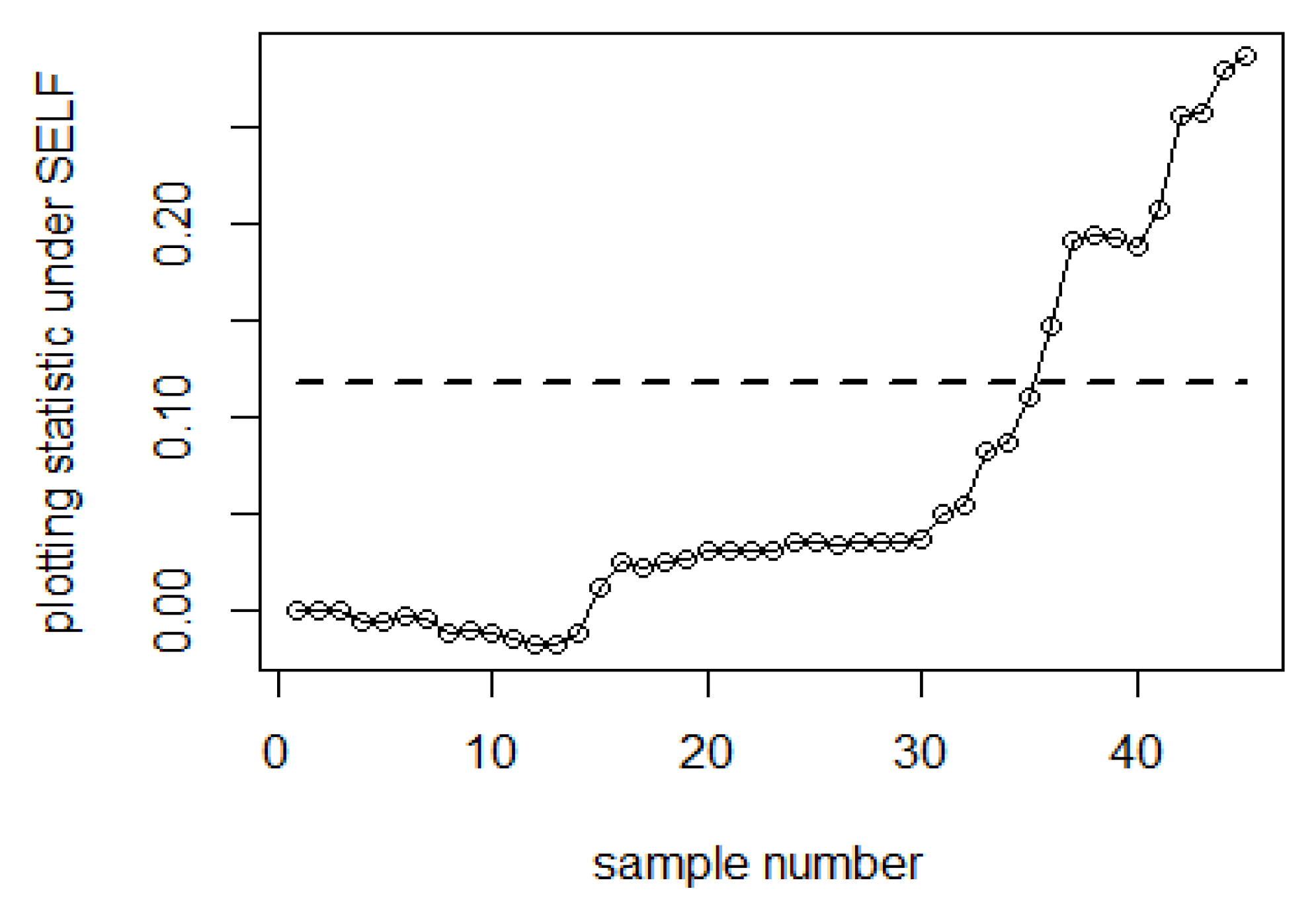
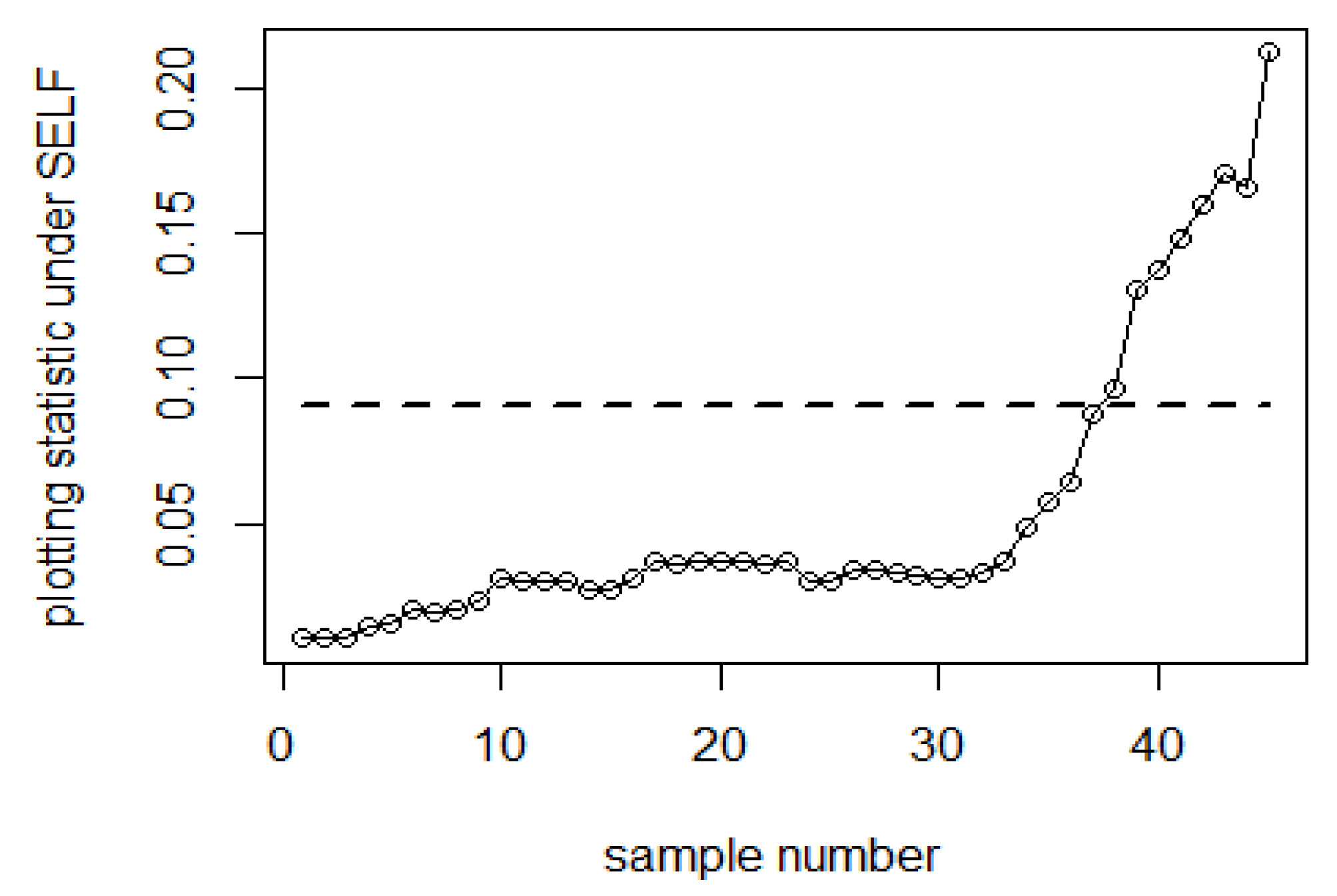


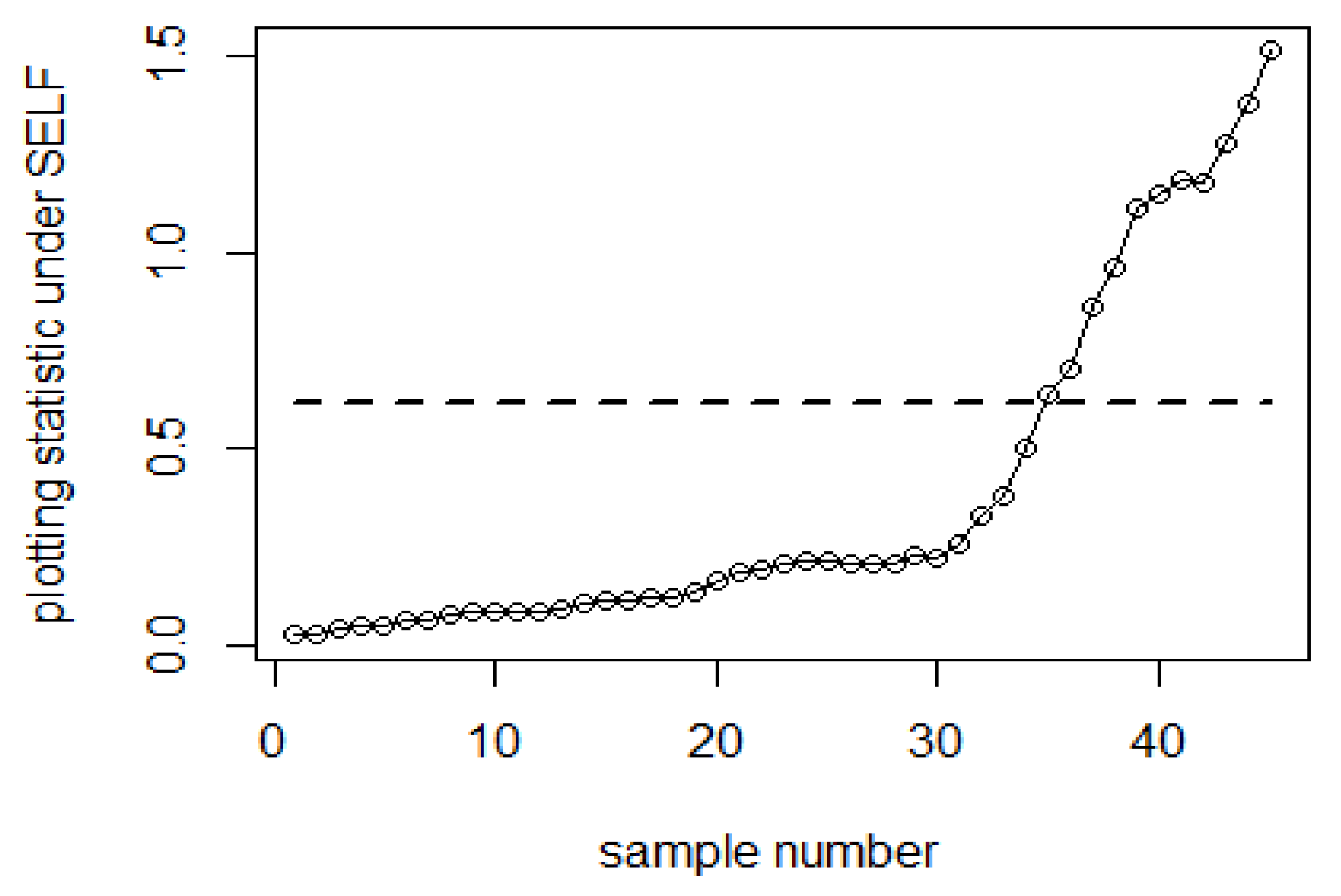
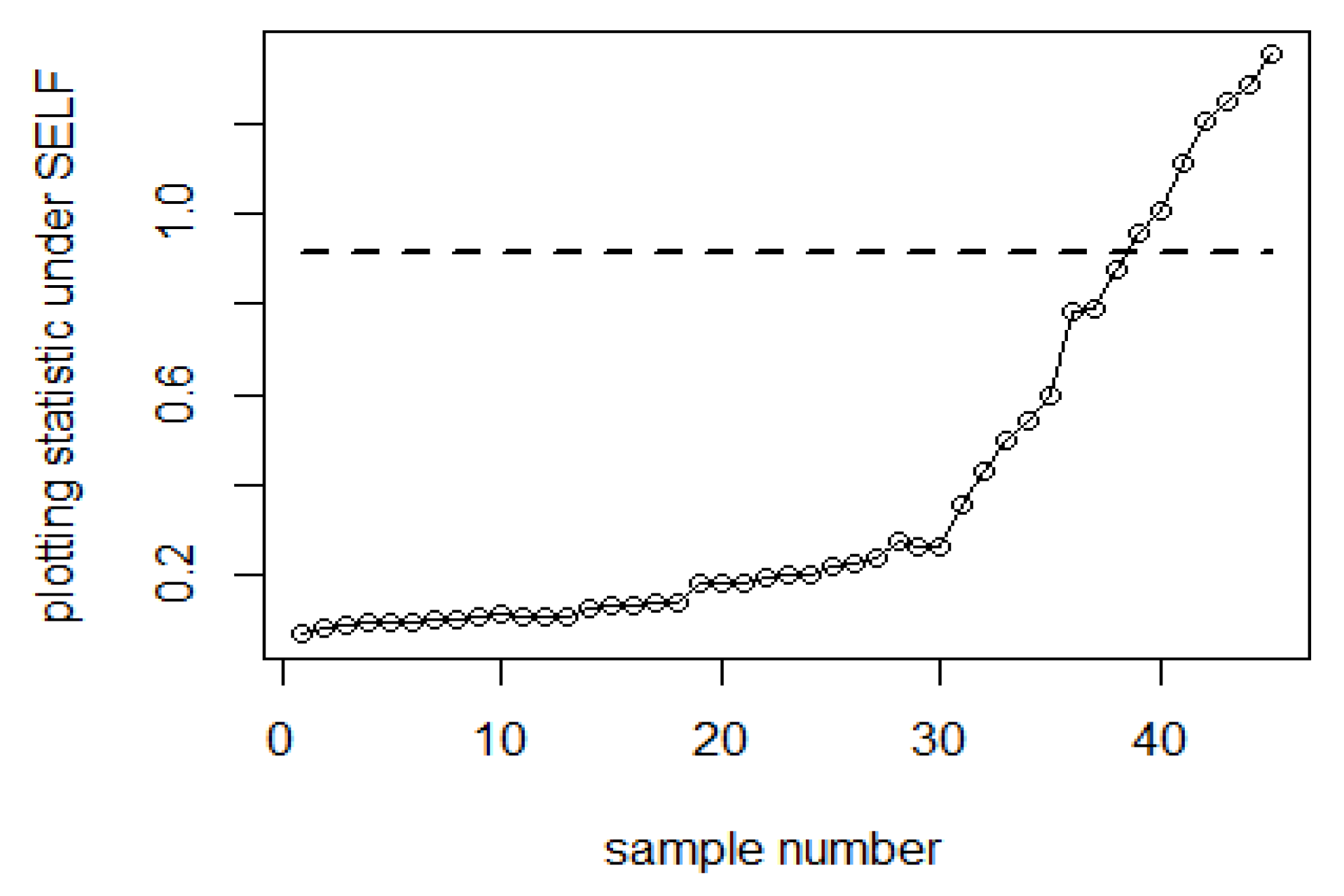

| Bayesian-AEWMA-RSS | Bayesian-AEWMA-MRSS | Bayesian-AEWMA-ERSS | |||||||
|---|---|---|---|---|---|---|---|---|---|
| Shift | No Error | 0.5 | 1 | No Error | 0.5 | 1 | No Error | 0.5 | 1 |
| 0.0 | 369.23 (360.00) | 370.01 (365.31) | 370.78 (362.21) | 370.03 (364.48) | 370.17 (366.78) | 370.21 (367.34) | 370.13 (457.80) | 369.89 (366.24) | 370.90 (367.54) |
| 0.10 | 113.64 (86.20) | 148.48 (93.90) | 187.09 (103.81) | 99.97 (71.51) | 87.96 (76.96) | 78.26 (72.67) | 104.84 (102.39) | 61.35 (56.61) | 78.30 (67.88) |
| 0.20 | 49.08 (32.79) | 66.37 (37.73) | 80.60 (42.11) | 44.00 (28.28) | 46.41 (30.54) | 51.67 (48.67) | 41.80 (39.45) | 30.45 (25.94) | 36.92 (28.78) |
| 0.30 | 27.63 (18.49) | 37.30 (21.79) | 46.01 (24.44) | 15.65 (11.14) | 23.58 (17.70) | 24.34 (19.49) | 21.96 (21.07) | 11.83 (9.90) | 21.42 (16.76) |
| 0.40 | 17.42 (11.91) | 23.14 (14.11) | 28.39 (16.05) | 14.99 (10.30) | 15.39 (11.55) | 16.21 (12.94) | 12.87 (12.40) | 18.03 (15.03) | 28.00 (10.92) |
| 0.50 | 11.95 (8.36) | 15.54 (9.84) | 19.18 (11.30) | 9.77 (6.89) | 10.62 (8.16) | 11.56 (9.40) | 8.30 (7.93) | 8.07 (6.64) | 9.68 (7.72) |
| 0.60 | 8.51 (6.03) | 10.93 (7.18) | 13.19 (8.10) | 7.01 (4.93) | 7.68 (5.88) | 8.56 (6.89) | 5.94 (5.52) | 6.06 (4.94) | 7.06 (5.47) |
| 0.70 | 6.26 (4.45) | 8.04 (5.32) | 9.72 (6.09) | 5.21 (3.68) | 5.86 (4.46) | 6.61 (5.28) | 4.43 (3.92) | 4.64 (3.69) | 5.39 (4.17) |
| 0.80 | 4.88 (3.41) | 6.20 (4.14) | 7.28 (4.56) | 4.05 (2.80) | 4.59 (3.42) | 5.19 (4.07) | 3.47 (2.93) | 3.68 (2.86) | 4.27 (3.20) |
| 0.90 | 3.92 (2.71) | 4.81 (3.12) | 5.69 (3.53) | 3.22 (2.15) | 3.76 (2.76) | 4.28 (3.31) | 2.86 (2.27) | 3.06 (2.26) | 3.48 (2.57) |
| 1.0 | 3.22 (2.17) | 4.00 (2.58) | 4.67 (2.91) | 2.66 (1.72) | 3.15 (2.24) | 3.56 (2.70) | 2.39 (1.80) | 2.56 (1.79) | 2.91 (2.07) |
| 1.5 | 1.66 (0.89) | 1.94 (1.06) | 2.14 (1.19) | 1.43 (0.69) | 1.66 (0.93) | 1.86 (1.13) | 1.37 (0.67) | 1.46 (0.76) | 1.56 (0.83) |
| 2.0 | 1.18 (0.42) | 1.28 (0.52) | 1.38 (0.59) | 1.08 (0.28) | 1.20 (0.45) | 1.32 (0.61) | 1.09 (0.30) | 1.12 (0.36) | 1.03 (0.18) |
| 2.5 | 1.03 (0.19) | 1.07 (0.26) | 1.10 (0.32) | 1 (0) | 1.04 (0.21) | 1.09 (0.30) | 1.01 (0.12) | 1.02 (0.15) | 1.16 (0.40) |
| 3 | 1 (0) | 1 (0) | 1.01 (0.12) | 1 (0) | 1 (0) | 1.02 (0.16) | 1 (0) | 1 (0) | 1 (0) |
| 4 | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1 (0) |
| Bayesian-AEWMA-RSS | Bayesian-AEWMA-MRSS | Bayesian-AEWMA-ERSS | |||||||
|---|---|---|---|---|---|---|---|---|---|
| Shift | No Error | 0.5 | 1 | No Error | 0.5 | 1 | No Error | 0.5 | 1 |
| 0.0 | 370.92 (332.68) | 370.89 (361.23) | 370.12 (366.12) | 369.44 (344.38) | 370.04 (365.35) | 369.89 (361.21) | 371.12 (316.71) | 370.67 (365.18) | 370.33 (362.12) |
| 0.10 | 113.62 (77.72) | 148.72 (105.97) | 183.96 (105.88) | 102.05 (68.97) | 127.41 (80.67) | 156.53 (89.05) | 125.03 (90.94) | 156.37 (99.83) | 200.20 (126.88) |
| 0.20 | 50.89 (32.73) | 63.47 (37.12) | 78.87 (41.18) | 43.63 (28.65) | 53.21 (31.96) | 66.43 (35.53) | 52.45 (35.89) | 69.43 (41.00) | 86.21 (45.99) |
| 0.30 | 29.15 (19.09) | 35.89 (21.62) | 44.15 (24.07) | 23.39 (15.70) | 29.57 (17.96) | 35.84 (20.00) | 31.99 (20.90) | 39.87 (23.48) | 48.63 (25.97) |
| 0.40 | 18.11 (12.29) | 22.54 (14.11) | 27.36 (15.72) | 14.72 (10.26) | 17.86 (11.53) | 21.73 (13.00) | 20.49 (13.77) | 25.17 (15.54) | 31.09 (17.32) |
| 0.50 | 12.37 (8.59) | 14.94 (9.79) | 18.38 (11.05) | 9.81 (6.98) | 11.73 (7.87) | 14.33 (8.88) | 13.79 (9.59) | 17.03 (10.98) | 20.57 (12.12) |
| 0.60 | 8.64 (6.10) | 10.56 (6.97) | 12.71 (7.86) | 6.91 (4.87) | 8.31 (5.63) | 9.98 (6.35) | 10.00 (6.95) | 12.06 (7.99) | 14.57 (9.04) |
| 0.70 | 6.56 (4.59) | 7.90 (5.21) | 9.37 (5.96) | 5.13 (3.66) | 6.10 (4.14) | 7.29 (4.68) | 7.52 (5.28) | 8.99 (6.02) | 10.89 (6.82) |
| 0.80 | 5.10 (3.58) | 6.05 (4.00) | 7.22 (4.52) | 4.03 (2.77) | 4.68 (3.15) | 5.49 (3.55) | 5.74 (4.04) | 6.96 (4.64) | 8.20 (5.17) |
| 0.90 | 4.01 (2.72) | 4.76 (3.16) | 5.60 (3.54) | 3.23 (2.17) | 3.71 (2.42) | 4.22 (2.67) | 4.62 (3.19) | 5.47 (3.57) | 6.43 (4.15) |
| 1.0 | 3.36 (2.20) | 3.86 (2.51) | 4.45 (2.82) | 2.65 (1.74) | 3.02 (1.93) | 3.52 (2.17) | 3.81 (2.56) | 4.44 (2.89) | 5.19 (3.22) |
| 1.5 | 1.69 (0.91) | 1.06 (0.25) | 2.10 (1.16) | 1.42 (0.67) | 1.54 (0.76) | 1.68 (0.84) | 1.88 (1.06) | 2.12 (1.21) | 2.40 (1.35) |
| 2.0 | 1.19 (0.43) | 1.27 (0.51) | 1.35 (0.57) | 1.09 (0.30) | 1.13 (0.35) | 1.18 (0.41) | 1.28 (0.53) | 1.38 (0.61) | 1.50 (0.69) |
| 2.5 | 1.04 (0.20) | 1.06 (0.24) | 1.08 (0.29) | 1 (0) | 1.01 (0.13) | 1.02 (0.16) | 1.07 (0.27) | 1.11 (0.33) | 1.15 (0.38) |
| 3 | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1.02 (0.14) | 1.03 (0.18) |
| 4 | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1 (0) |
| Bayesian-AEWMA-RSS | Bayesian-AEWMA-MRSS | Bayesian-AEWMA-ERSS | |||||||
|---|---|---|---|---|---|---|---|---|---|
| Shift | No Error | 0.5 | 1 | No Error | 0.5 | 1 | No Error | 0.5 | 1 |
| 0.0 | 370.18 (351.20) | 370.89 (364.22) | 370.98 (363.68) | 370.56 (348.97) | 369.78 (361.23) | 370.08 (363.51) | 369.23 (351.25) | 370.65 (359.90) | 370.05 (362.10) |
| 0.10 | 115.82 (81.46) | 149.01 (94.02) | 187.52 (106.10) | 99.75 (69.58) | 128.24 (80.58) | 160.78 (99.38) | 119.83 (85.48) | 154.45 (102.45) | 191.77 (115.74) |
| 0.20 | 51.42 (33.50) | 64.85 (37.57) | 79.72 (41.78) | 36.48 (26.34) | 54.27 (32.37) | 67.07 (36.79) | 54.59 (35.50) | 69.51 (40.81) | 84.63 (45.39) |
| 0.30 | 29.24 (19.01) | 36.13 (21.60) | 44.50 (24.16) | 23.96 (16.06) | 29.93 (18.37) | 36.66 (20.25) | 31.48 (20.70) | 39.46 (23.42) | 48.12 (26.27) |
| 0.40 | 18.34 (12.30) | 22.65 (14.13) | 27.64 (15.99) | 14.77 (10.17) | 18.06 (11.86) | 22.03 (13.36) | 20.16 (13.69) | 24.94 (15.36) | 30.33 (17.41) |
| 0.50 | 12.42 (8.66) | 15.17 (9.78) | 18.43 (11.00) | 9.87 (7.01) | 12.05 (8.01) | 14.32 (8.94) | 13.82 (9.46) | 16.81 (10.70) | 20.42 (12.12) |
| 0.60 | 8.87 (6.27) | 10.70 (7.05) | 12.91 (8.08) | 7.00 (4.93) | 8.18 (5.57) | 9.97 (6.33) | 9.83 (6.88) | 11.91 (7.87) | 14.38 (8.90) |
| 0.70 | 6.56 (4.63) | 7.90 (5.26) | 9.38 (5.96) | 5.20 (3.64) | 6.15 (4.17) | 7.33 (4.67) | 7.40 (5.21) | 6.85 (4.58) | 10.59 (6.65) |
| 0.80 | 5.13 (3.55) | 5.99 (3.98) | 7.20 (4.52) | 3.99 (2.74) | 4.72 (3.15) | 5.50 (3.52) | 5.80 (4.08) | 8.89 (5.86) | 8.06 (5.18) |
| 0.90 | 4.10 (2.82) | 4.79 (3.14) | 5.64 (3.55) | 3.22 (2.16) | 3.75 (2.47) | 4.35 (2.73) | 4.65 (3.22) | 5.44 (3.60) | 6.45 (4.07) |
| 1.0 | 3.34 (2.24) | 3.92 (2.53) | 4.52 (2.77) | 2.69 (1.73) | 3.09 (1.96) | 3.57 (2.20) | 3.77 (2.54) | 4.43 (2.87) | 5.15 (3.25) |
| 1.5 | 1.71 (0.91) | 1.90 (1.03) | 2.14 (1.17) | 1.43 (0.69) | 1.56 (0.78) | 1.69 (0.87) | 1.88 (1.05) | 2.08 (1.19) | 2.37 (1.33) |
| 2.0 | 1.20 (0.44) | 1.27 (0.51) | 1.36 (0.58) | 1.09 (0.29) | 1.12 (0.34) | 1.17 (0.41) | 1.29 (0.54) | 1.40 (0.63) | 1.49 (0.69) |
| 2.5 | 1.04 (0.20) | 1.06 (0.24) | 1.09 (0.30) | 1 (0) | 1.01 (0.13) | 1.03 (0.17) | 1.07 (0.27) | 1.11 (0.32) | 1.15 (0.38) |
| 3 | 1 (0) | 1 (0) | 1.01 (0.12) | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1.02 (0.14) | 1.03 (0.18) |
| 4 | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1 (0) |
| Bayesian-AEWMA-RSS | Bayesian-AEWMA-MRSS | Bayesian-AEWMA-ERSS | |||||||
|---|---|---|---|---|---|---|---|---|---|
| Shift | No Error | 0.5 | 1 | No Error | 0.5 | 1 | No Error | 0.5 | 1 |
| 0.0 | 369.23 (360.00) | 370.32 (363.10) | 370.02 (365.18) | 370.03 (364.48) | 370.77 (359.98) | 370.32 (357.48) | 370.13 (457.80) | 370.53 (355.48) | 370.90 (346.48) |
| 0.10 | 113.64 (86.20) | 127.67 (86.57) | 133.57 (90.78) | 99.97 (71.51) | 108.27 (75.50) | 114.93 (75.01) | 104.84 (102.39) | 94.46 (76.67) | 97.85 (77.40) |
| 0.20 | 49.08 (32.79) | 55.78 (34.57) | 57.57 (34.93) | 44.00 (28.28) | 46.30 (29.34) | 48.07 (30.06) | 41.80 (39.45) | 42.67 (31.28) | 45.80 (32.30) |
| 0.30 | 27.63 (18.49) | 31.55 (20.01) | 32.97 (20.47) | 15.65 (11.14) | 25.29 (16.46) | 26.49 (17.11) | 21.96 (21.07) | 24.73 (18.20) | 26.08 (18.77) |
| 0.40 | 17.42 (11.91) | 19.69 (12.91) | 20.58 (13.41) | 14.99 (10.30) | 15.73 (10.80) | 16.30 (10.93) | 12.87 (12.40) | 16.12 (11.93) | 16.38 (12.06) |
| 0.50 | 11.95 (8.36) | 13.27 (8.98) | 13.90 (9.13) | 9.77 (6.89) | 10.27 (7.18) | 10.64 (7.31) | 8.30 (7.93) | 11.09 (8.32) | 11.37 (8.54) |
| 0.60 | 8.51 (6.03) | 9.35 (6.49) | 9.82 (6.65) | 7.01 (4.93) | 7.25 (5.07) | 7.55 (5.22) | 5.94 (5.52) | 8.04 (5.99) | 8.36 (6.15) |
| 0.70 | 6.26 (4.45) | 7.12 (4.87) | 7.17 (4.96) | 5.21 (3.68) | 5.38 (3.79) | 5.59 (3.87) | 4.43 (3.92) | 6.08 (4.56) | 6.32 (4.69) |
| 0.80 | 4.88 (3.41) | 5.41 (3.70) | 5.61 (3.83) | 4.05 (2.80) | 4.20 (2.85) | 4.30 (2.95) | 3.47 (2.93) | 4.77 (3.47) | 4.93 (3.56) |
| 0.90 | 3.92 (2.71) | 4.29 (2.88) | 4.39 (2.92) | 3.22 (2.15) | 3.36 (2.27) | 3.45 (2.28) | 2.86 (2.27) | 3.97 (2.81) | 4.01 (2.85) |
| 1.0 | 3.22 (2.17) | 3.50 (2.29) | 3.60 (2.35) | 2.66 (1.72) | 2.77 (1.77) | 2.81 (1.78) | 2.39 (1.80) | 3.20 (2.22) | 3.30 (2.30) |
| 1.5 | 1.66 (0.89) | 1.76 (0.96) | 1.79 (0.96) | 1.43 (0.69) | 1.48 (0.72) | 1.48 (0.72) | 1.37 (0.67) | 1.67 (0.93) | 1.72 (0.97) |
| 2.0 | 1.18 (0.42) | 1.21 (0.45) | 1.24 (0.49) | 1.08 (0.28) | 1.10 (0.31) | 1.10 (0.32) | 1.09 (0.30) | 1.20 (0.45) | 1.21 (0.47) |
| 2.5 | 1.03 (0.19) | 1.04 (0.22) | 1.05 (0.22) | 1 (0) | 1.01 (0.11) | 1.01 (0.11) | 1.01 (0.12) | 1.04 (0.21) | 1.04 (0.21) |
| 3 | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1 (0) |
| 4 | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1 (0) |
| Bayesian-AEWMA-RSS | Bayesian-AEWMA-MRSS | Bayesian-AEWMA-ERSS | |||||||
|---|---|---|---|---|---|---|---|---|---|
| Shift | No Error | 0.5 | 1 | No Error | 0.5 | 1 | No Error | 0.5 | 1 |
| 0.0 | 370.92 (332.68) | 370.43 (346.68) | 370.23 (357.18) | 369.44 (344.38) | 370.62 (354.38) | 370.22 (351.38) | 371.12 (316.71) | 370.62 (356.51) | 370.02 (355.51) |
| 0.10 | 113.62 (77.72) | 119.61 (84.33) | 131.04 (90.83) | 102.05 (68.97) | 106.40 (72.90) | 115.91 (77.29) | 125.03 (90.94) | 128.81 (95.38) | 139.0 (96.08) |
| 0.20 | 50.89 (32.73) | 52.54 (33.62) | 55.90 (34.64) | 43.63 (28.65) | 44.68 (28.72) | 48.96 (30.06) | 52.45 (35.89) | 59.08 (37.64) | 62.26 (38.06) |
| 0.30 | 29.15 (19.09) | 30.24 (19.58) | 31.57 (19.73) | 23.39 (15.70) | 24.87 (16.39) | 26.98 (17.14) | 31.99 (20.90) | 33.44 (21.58) | 34.99 (21.86) |
| 0.40 | 18.11 (12.29) | 18.97 (12.67) | 19.80 (12.89) | 14.72 (10.26) | 15.41 (10.52) | 16.38 (11.04) | 20.49 (13.77) | 21.46 (14.11) | 30.95 (7.39) |
| 0.50 | 12.37 (8.59) | 12.69 (8.68) | 13.76 (9.06) | 9.81 (6.98) | 10.00 (7.04) | 10.78 (7.30) | 13.79 (9.59) | 14.53 (9.84) | 22.23 (14.57) |
| 0.60 | 8.64 (6.10) | 9.10 (6.36) | 9.41 (6.52) | 6.91 (4.87) | 7.09 (4.98) | 7.70 (5.33) | 10.00 (6.95) | 10.31 (7.04) | 10.95 (7.39) |
| 0.70 | 6.56 (4.59) | 6.75 (4.69) | 7.00 (4.81) | 5.13 (3.66) | 5.31 (3.74) | 5.48 (3.80) | 7.52 (5.28) | 7.81 (5.42) | 8.02 (5.53) |
| 0.80 | 5.10 (3.58) | 5.27 (3.66) | 5.40 (3.68) | 4.03 (2.77) | 4.11 (2.83) | 4.25 (2.90) | 5.74 (4.04) | 6.05 (4.18) | 6.24 (4.28) |
| 0.90 | 4.01 (2.72) | 4.17 (2.81) | 4.32 (2.90) | 3.23 (2.17) | 3.28 (2.20) | 3.38 (2.21) | 4.62 (3.19) | 4.82 (3.25) | 5.01 (3.40) |
| 1.0 | 3.36 (2.20) | 3.44 (2.29) | 3.54 (2.35) | 2.65 (1.74) | 2.71 (1.76) | 2.85 (1.82) | 3.81 (2.56) | 3.91 (2.60) | 4.06 (2.72) |
| 1.5 | 1.69 (0.91) | 1.72 (0.92) | 1.76 (0.94) | 1.42 (0.67) | 1.46 (0.70) | 1.49 (0.73) | 1.88 (1.06) | 1.92 (1.07) | 1.99 (1.11) |
| 2.0 | 1.19 (0.43) | 1.21 (0.46) | 1.22 (0.46) | 1.09 (0.30) | 1.09 (0.29) | 1.11 (0.33) | 1.28 (0.53) | 1.30 (0.55) | 1.32 (0.57) |
| 2.5 | 1.04 (0.20) | 1.04 (0.21) | 1.04 (0.22) | 1 (0) | 1.01 (0.10) | 1.01 (0.13) | 1.07 (0.27) | 1.08 (0.28) | 1.08 (0.29) |
| 3 | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1 (0) |
| 4 | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1 (0) |
| Bayesian-AEWMA-RSS | Bayesian-AEWMA-MRSS | Bayesian-AEWMA-ERSS | |||||||
|---|---|---|---|---|---|---|---|---|---|
| Shift | No Error | 0.5 | 1 | No Error | 0.5 | 1 | No Error | 0.5 | 1 |
| 0.0 | 370.18 (351.20) | 370.56 (348.97) | 370.16 (335.97) | 370.25 (331.27) | 370.25 (341.27) | 370.51 (348.21) | 369.23 (351.25) | 370.03 (343.15) | 370.10 (342.22) |
| 0.10 | 115.82 (81.46) | 123.05 (85.57) | 123.50 (98.55) | 99.75 (69.58) | 106.68 (71.96) | 110.95 (74.69) | 119.83 (85.48) | 129.26 (93.16) | 133.78 (93.15) |
| 0.20 | 51.42 (33.50) | 53.32 (33.95) | 56.36 (34.50) | 36.48 (26.34) | 45.96 (28.81) | 47.69 (29.80) | 54.59 (35.50) | 57.03 (36.74) | 60.09 (37.49) |
| 0.30 | 29.24 (19.01) | 30.27 (19.43) | 31.71 (20.01) | 23.96 (16.06) | 25.11 (16.41) | 26.16 (17.01) | 31.48 (20.70) | 32.78 (21.00) | 34.33 (21.84) |
| 0.40 | 18.34 (12.30) | 19.19 (12.71) | 19.62 (13.02) | 14.77 (10.17) | 15.31 (10.29) | 16.12 (10.84) | 20.16 (13.69) | 20.79 (13.95) | 21.77 (14.23) |
| 0.50 | 12.42 (8.66) | 12.78 (8.70) | 13.38 (8.94) | 9.87 (7.01) | 10.18 (7.10) | 10.57 (7.44) | 13.82 (9.46) | 14.07 (11.20) | 14.78 (9.87) |
| 0.60 | 8.87 (6.27) | 9.13 (6.33) | 56.36 (34.50) | 7.00 (4.93) | 7.20 (5.11) | 7.48 (5.21) | 9.83 (6.88) | 10.09 (6.93) | 10.60 (7.28) |
| 0.70 | 6.56 (4.63) | 6.77 (4.68) | 7.06 (4.83) | 5.20 (3.64) | 5.32 (3.69) | 5.56 (3.80) | 7.40 (5.21) | 7.66 (5.28) | 7.97 (5.55) |
| 0.80 | 5.13 (3.55) | 5.20 (3.56) | 5.34 (3.66) | 3.99 (2.74) | 4.15 (2.87) | 4.30 (2.92) | 5.80 (4.08) | 5.93 (4.13) | 6.23 (4.27) |
| 0.90 | 4.10 (2.82) | 4.16 (2.85) | 4.36 (2.94) | 3.22 (2.16) | 3.30 (2.18) | 3.47 (2.29) | 4.65 (3.22) | 4.70 (3.24) | 4.98 (3.33) |
| 1.0 | 3.34 (2.24) | 3.43 (2.26) | 3.52 (2.30) | 2.69 (1.73) | 2.75 (1.79) | 2.85 (1.82) | 3.77 (2.54) | 3.85 (2.58) | 3.99 (2.65) |
| 1.5 | 1.71 (0.91) | 1.73 (0.92) | 1.76 (0.95) | 1.43 (0.69) | 1.47 (0.71) | 1.49 (0.72) | 1.88 (1.05) | 1.91 (1.08) | 1.97 (1.12) |
| 2.0 | 1.20 (0.44) | 1.21 (0.45) | 1.22 (0.47) | 1.09 (0.29) | 1.09 (0.30) | 1.11 (0.32) | 1.29 (0.54) | 1.31 (0.55) | 1.33 (0.58) |
| 2.5 | 1.04 (0.20) | 1.05 (0.22) | 1.04 (0.21) | 1 (0) | 1.01 (0.11) | 1.01 (0.12) | 1.07 (0.27) | 1.08 (0.28) | 1.09 (0.29) |
| 3 | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1 (0) |
| 4 | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1 (0) | 1 (0) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Khan, I.; Noor-ul-Amin, M.; Khan, D.M.; AlQahtani, S.A.; Dahshan, M.; Khalil, U. Monitoring of Location Parameters with a Measurement Error under the Bayesian Approach Using Ranked-Based Sampling Designs with Applications in Industrial Engineering. Sustainability 2023, 15, 6675. https://doi.org/10.3390/su15086675
Khan I, Noor-ul-Amin M, Khan DM, AlQahtani SA, Dahshan M, Khalil U. Monitoring of Location Parameters with a Measurement Error under the Bayesian Approach Using Ranked-Based Sampling Designs with Applications in Industrial Engineering. Sustainability. 2023; 15(8):6675. https://doi.org/10.3390/su15086675
Chicago/Turabian StyleKhan, Imad, Muhammad Noor-ul-Amin, Dost Muhammad Khan, Salman A. AlQahtani, Mostafa Dahshan, and Umair Khalil. 2023. "Monitoring of Location Parameters with a Measurement Error under the Bayesian Approach Using Ranked-Based Sampling Designs with Applications in Industrial Engineering" Sustainability 15, no. 8: 6675. https://doi.org/10.3390/su15086675
APA StyleKhan, I., Noor-ul-Amin, M., Khan, D. M., AlQahtani, S. A., Dahshan, M., & Khalil, U. (2023). Monitoring of Location Parameters with a Measurement Error under the Bayesian Approach Using Ranked-Based Sampling Designs with Applications in Industrial Engineering. Sustainability, 15(8), 6675. https://doi.org/10.3390/su15086675






