Energy, Exergy, Exergoeconomic Analysis, and Optimization in a Natural Gas Decompression Station with a Vortex Tube and Geothermal Preheating
Abstract
:1. Introduction
- Perform the energy analysis to obtain the energy performance, energy flow rates, and energy interactions of the equipment that makes up the decompression station. This analysis is to evaluate the energy feasibility and obtain the nominal capacities of the equipment, and in this way, establish the thermal design of some components, such as the geothermal heat exchanger;
- Perform the exergy analysis to determine the maximum theoretical potential of the decompression station and evaluate the magnitude of the thermodynamic inefficiencies that occurred in the equipment during the natural gas decompression process. The above is to identify the critical components that have the most significant influence on the thermodynamic performance of the decompression station;
- Perform the exergoeconomic analysis to estimate the station’s operating costs and, through thermoeconomic indicators, determine the equipment with the most significant area of opportunity for the reduction in operating costs and costs related to the thermodynamic inefficiencies of the components;
- Carry out a parametric optimization of the decompression station to reduce natural gas costs and reduce exergy destruction, modifying some variables and input parameters of the station through a process of iterations, simulating different operating conditions and their effect on the costs.
2. System Description
3. Materials and Methods
- Estimation of general properties: This initial stage involves general property calculations taking into account the influence of natural gas composition. Also, the inlet and outlet pressure and temperature conditions of the station are selected;
- Energy analysis: In this stage, mass and energy balances are realized in the equipment to obtain the required heat flows in the heat exchangers. The importance of the geothermal exchanger design is highlighted particularly by analyzing the influence of the U-tube diameter relative to its length;
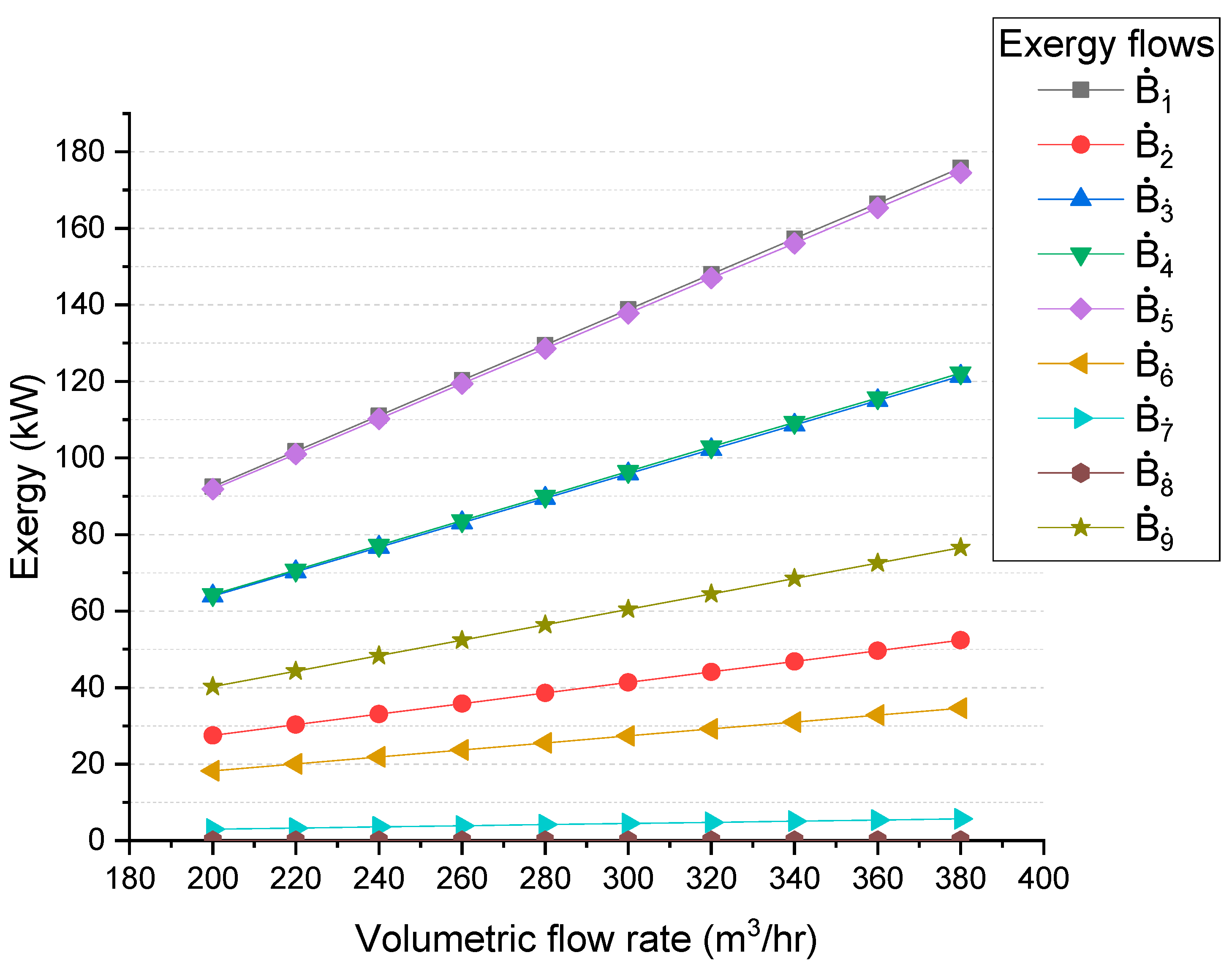
- Exergetic analysis: For this stage, it is necessary to calculate all exergy flows of the system, as well as exergy destruction, and define the fuel and product of each equipment. Subsequently, changes in exergy flows are analyzed by varying the volumetric flow rate;
- Exergoeconomic analysis: All equipment cost equations, considering inflation, as well as the cost rate of each one, are obtained. Similar to the previous stage, the influence of volumetric flow rate variation on costs is considered;
- System optimization: This is the last stage of the analysis, where a parametric analysis is performed to obtain the most appropriate decision variables for carrying out two optimization processes of two single objective functions: natural gas cost and system exergy destruction.
3.1. Natural Gas Properties and Initial Considerations
3.1.1. Initial Considerations
- Natural gas flow is considered stationary and stable;
- Natural gas flow is compressible;
- Kinetic energy changes (ΔKc) and potential energy (ΔEp) from all equipment are negligible;
- System pipelines are considered adiabatic, so the heat losses are negligible;
- The pressure in the system equipment is considered constant. The pressure only decreases in the expansion stage inside the heating vat [35];
- Natural gas composition is considered with all the hydrocarbons that conform to it according to Table 1;
- Natural gas is analyzed as a real gas mixture;
- The natural gas arrives at the station with an ambient temperature (20 °C) and a 250 bar pressure [36];
- The water in the heating vat remains constant at a temperature of 62 °C;
- The natural gas output pressure is 3 bar, and the output temperature is 20 °C;
- Natural gas flow output from the station is rated at 300 m3/h [37].
3.1.2. Natural Gas Composition
3.1.3. Estimation of General Properties
3.2. Energetic Modeling
3.2.1. Vortex Tube
3.2.2. Geothermal Heat Input
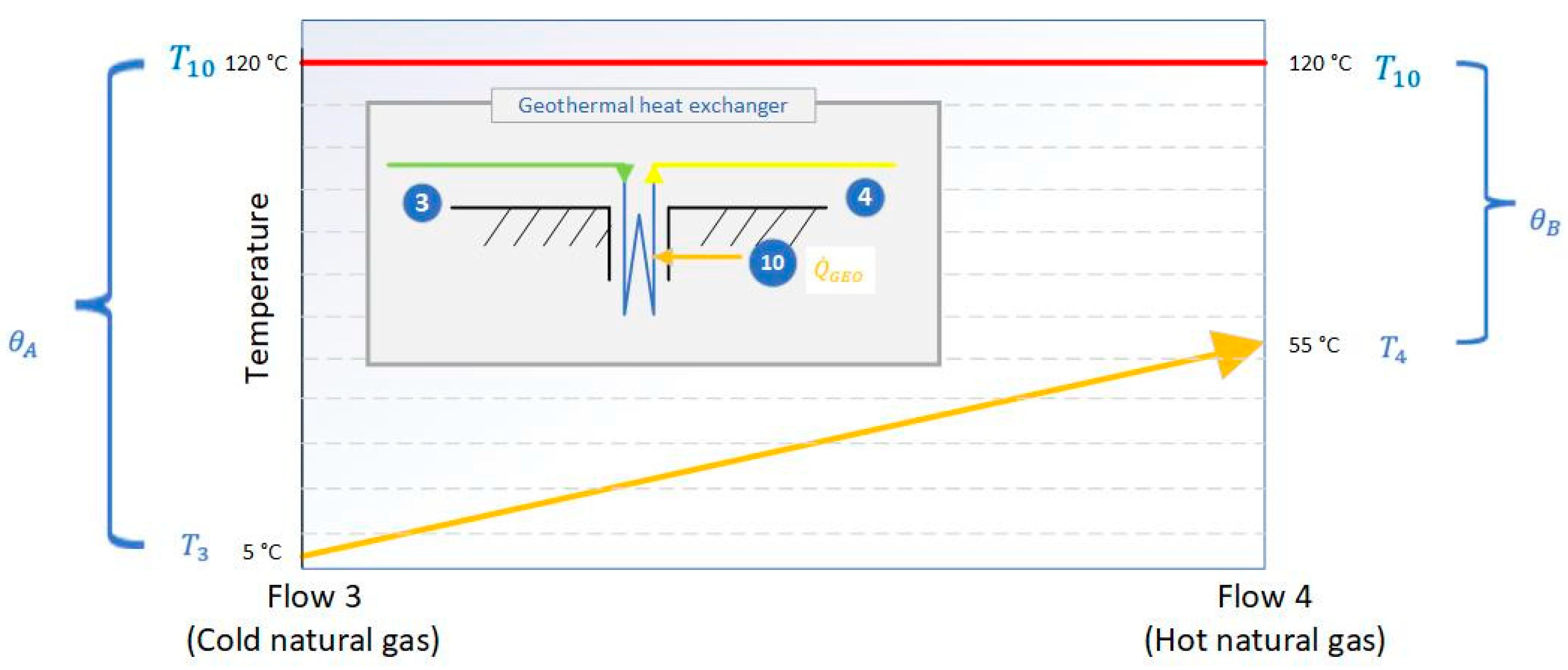
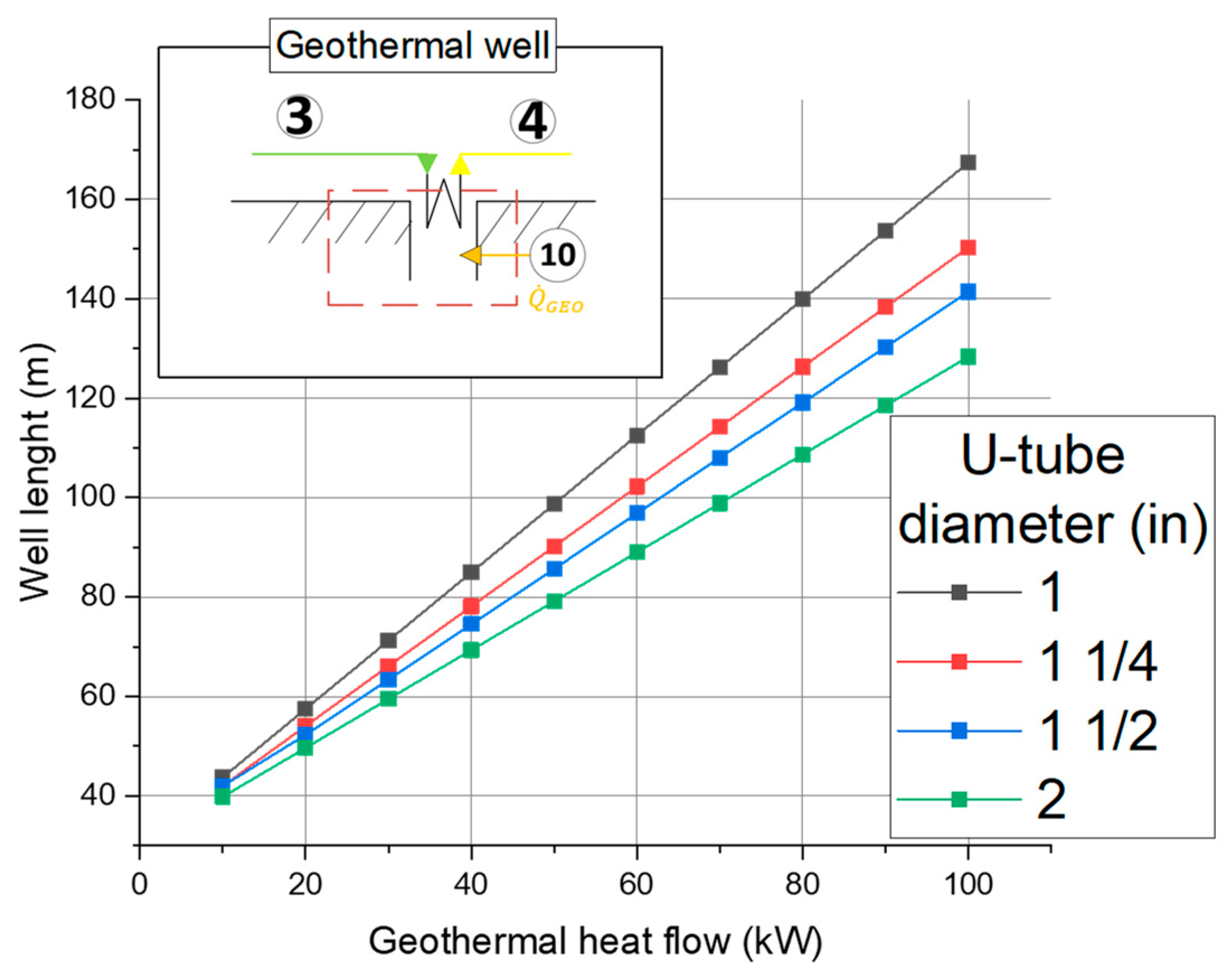
3.2.3. Geothermal Heat Exchanger Design
3.2.4. Water Bath Heater (Heating Vat)
3.3. Exergetic Modeling
3.3.1. Exergy Flows
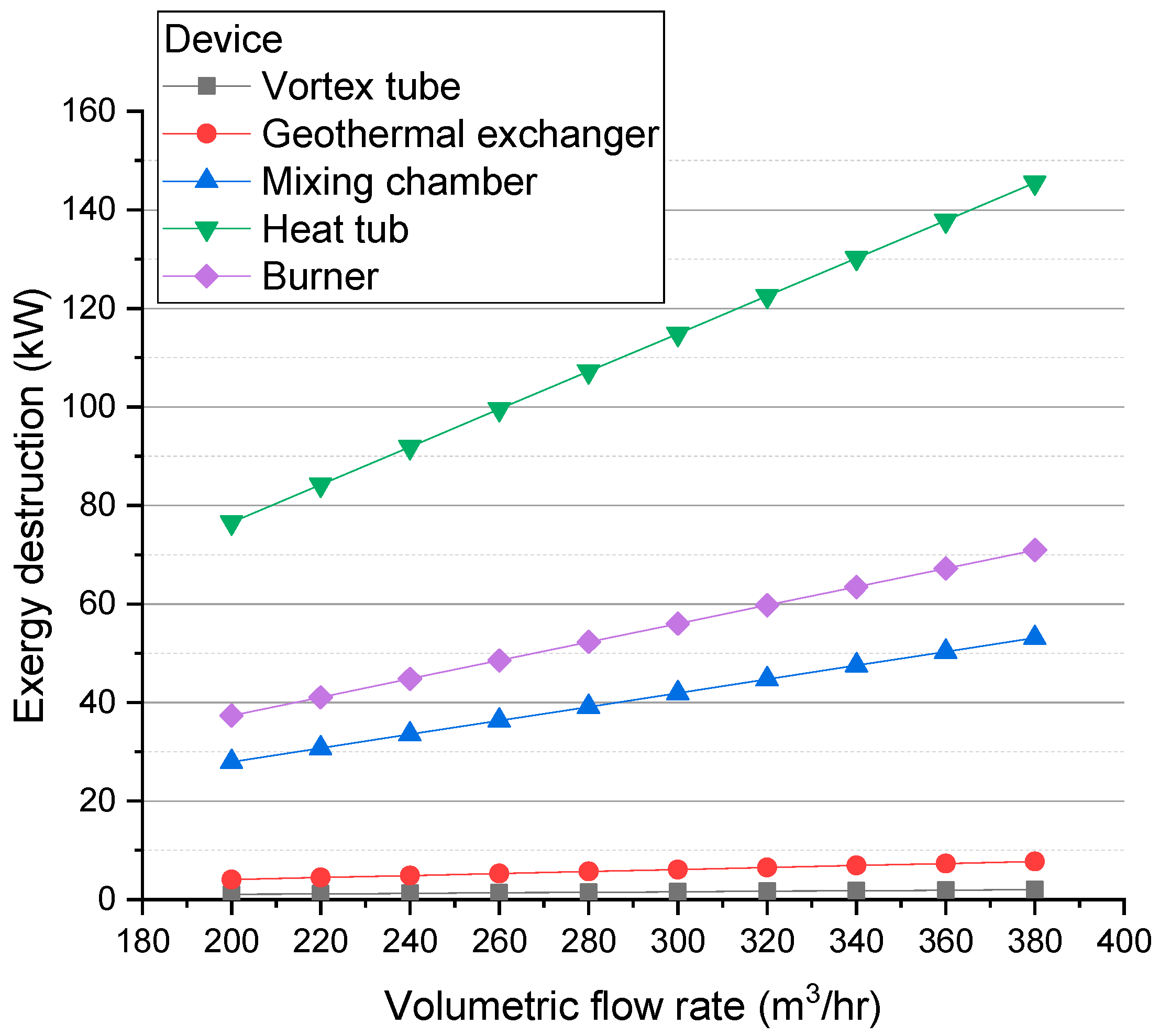
3.3.2. Exergy Destruction
3.4. Exergoeconomic Modeling
3.4.1. System Equipment Costs
3.4.2. Capital Recovery Factor
3.4.3. System Equipment Cost Rate
3.4.4. Costs Balance
3.4.5. Thermoeconomics Evaluation
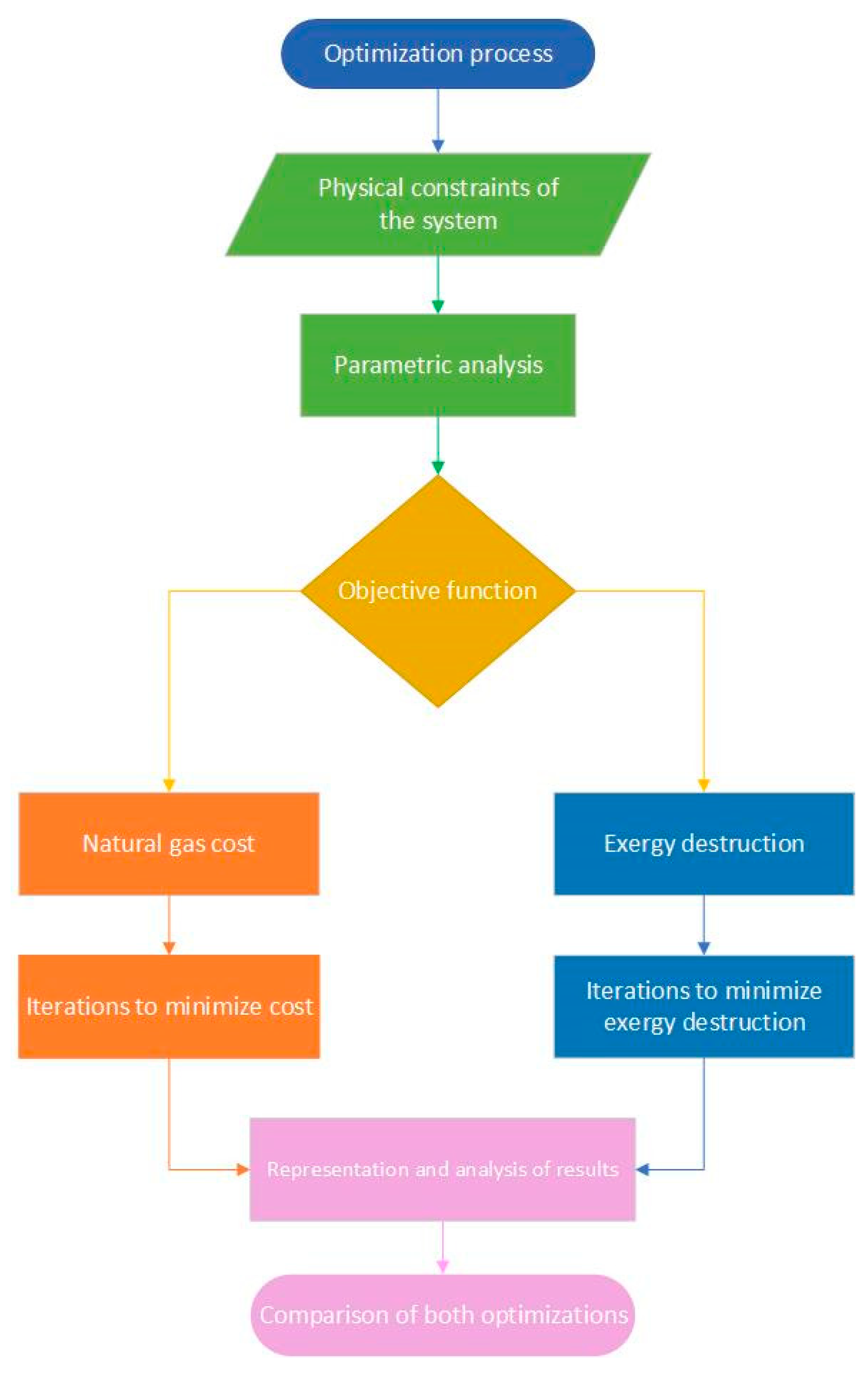
3.5. System Optimization
3.5.1. Parametric Analysis
3.5.2. Objective Function Optimization
4. Results and Discussion
4.1. Energy Analysis Results
4.1.1. Vortex Tube
4.1.2. Geothermal Heat Input
4.1.3. Geothermal Heat Exchanger Design
4.1.4. Heating Vat
4.2. Exergetic Analysis Results
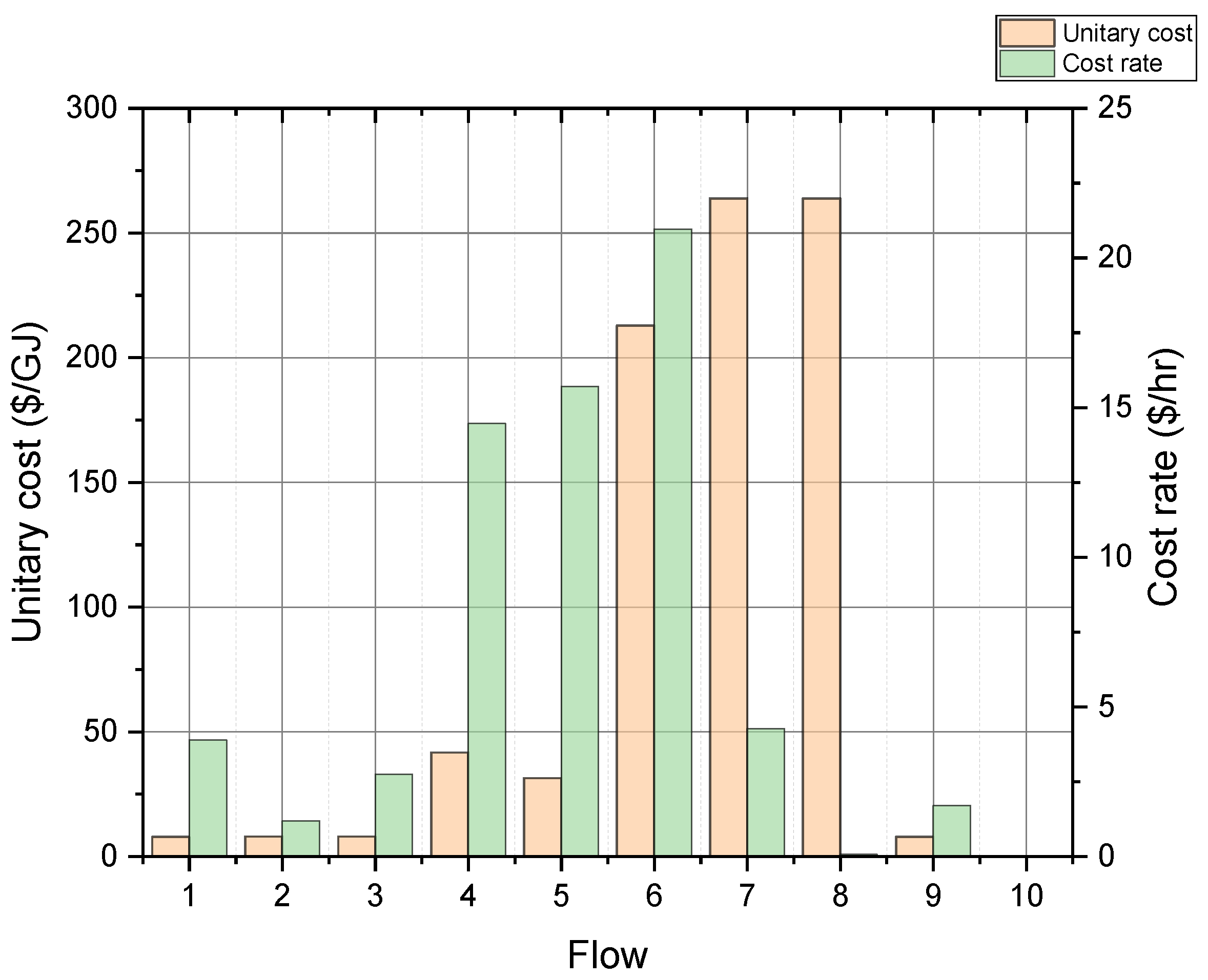
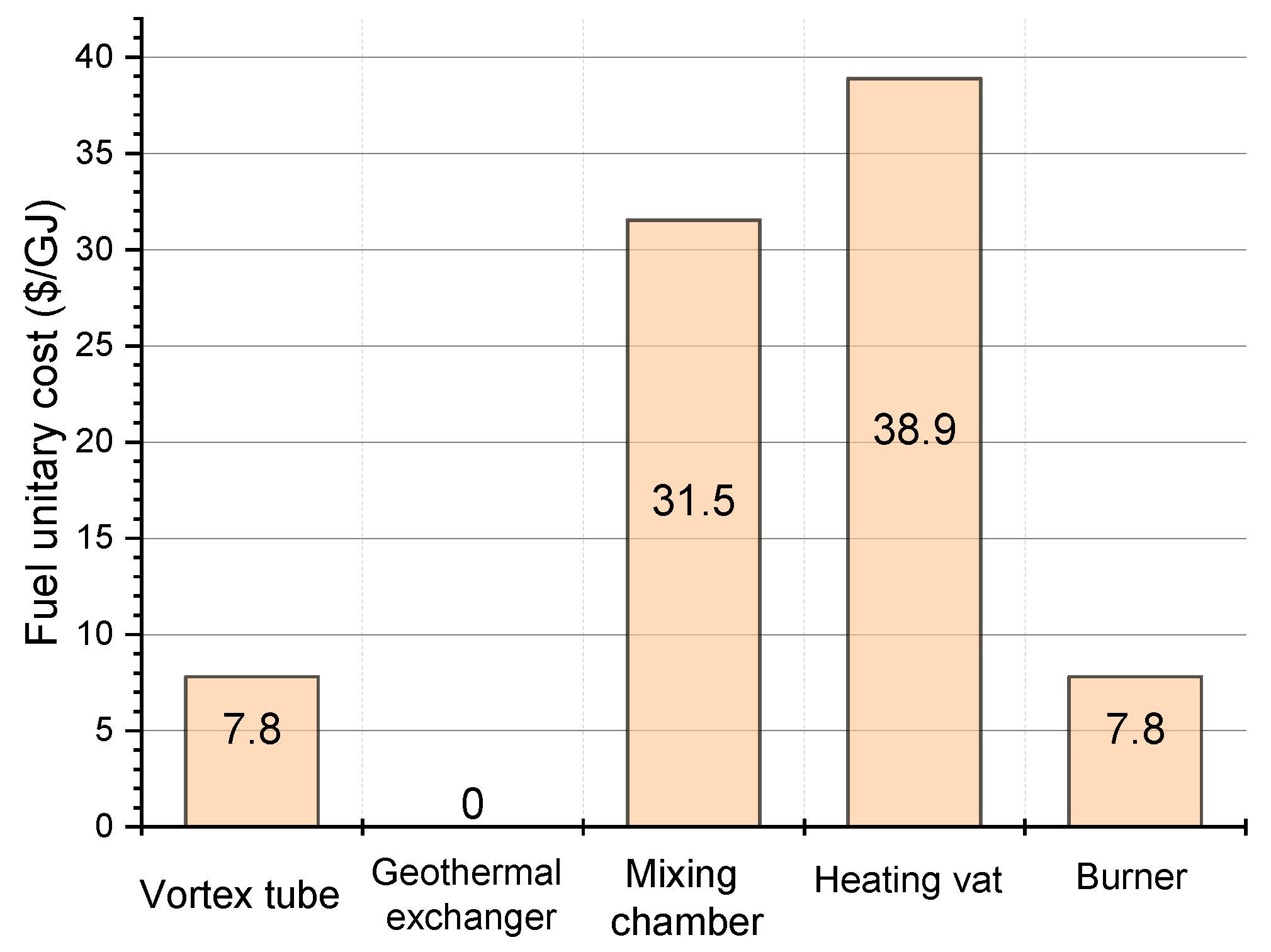
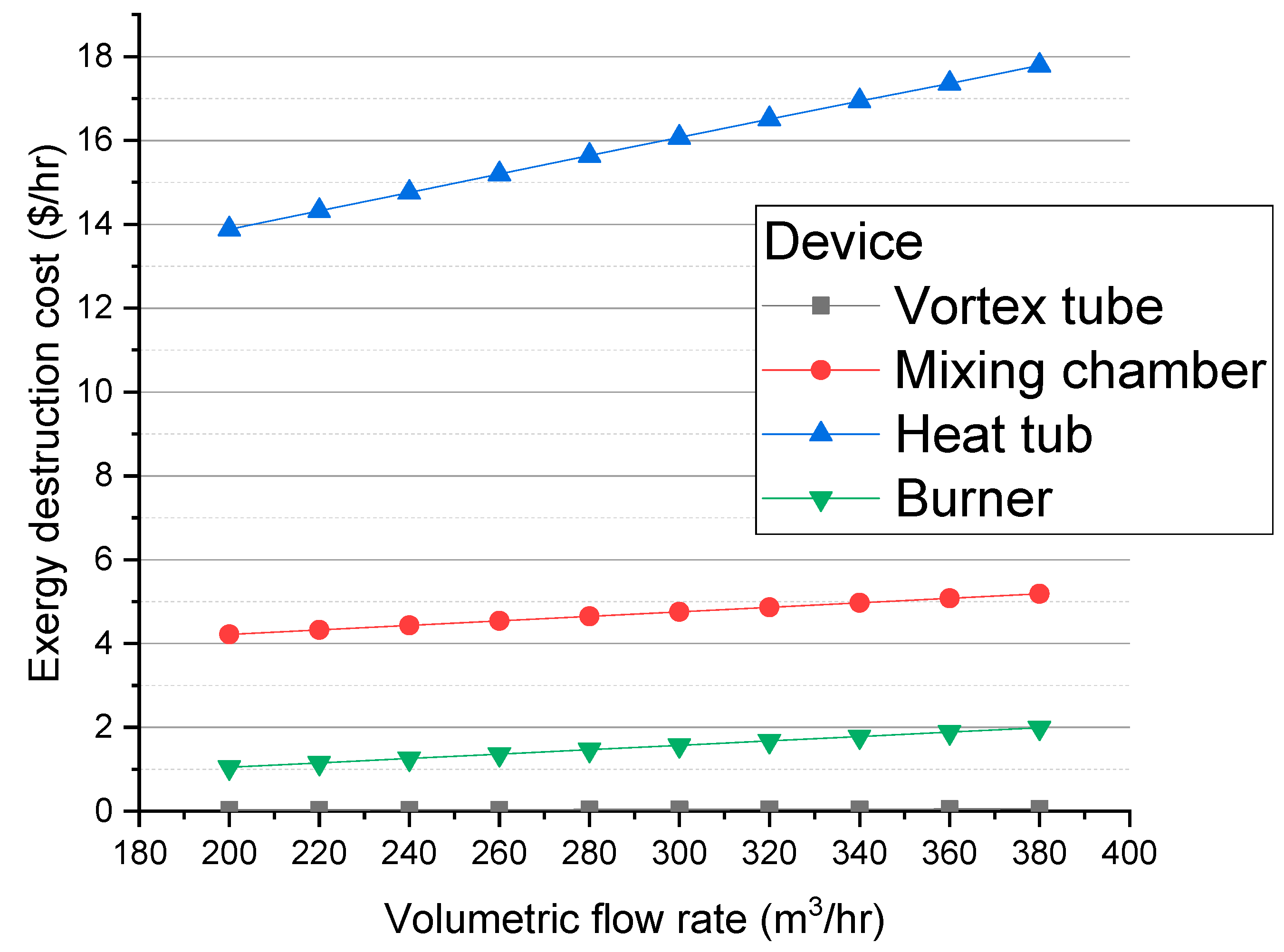
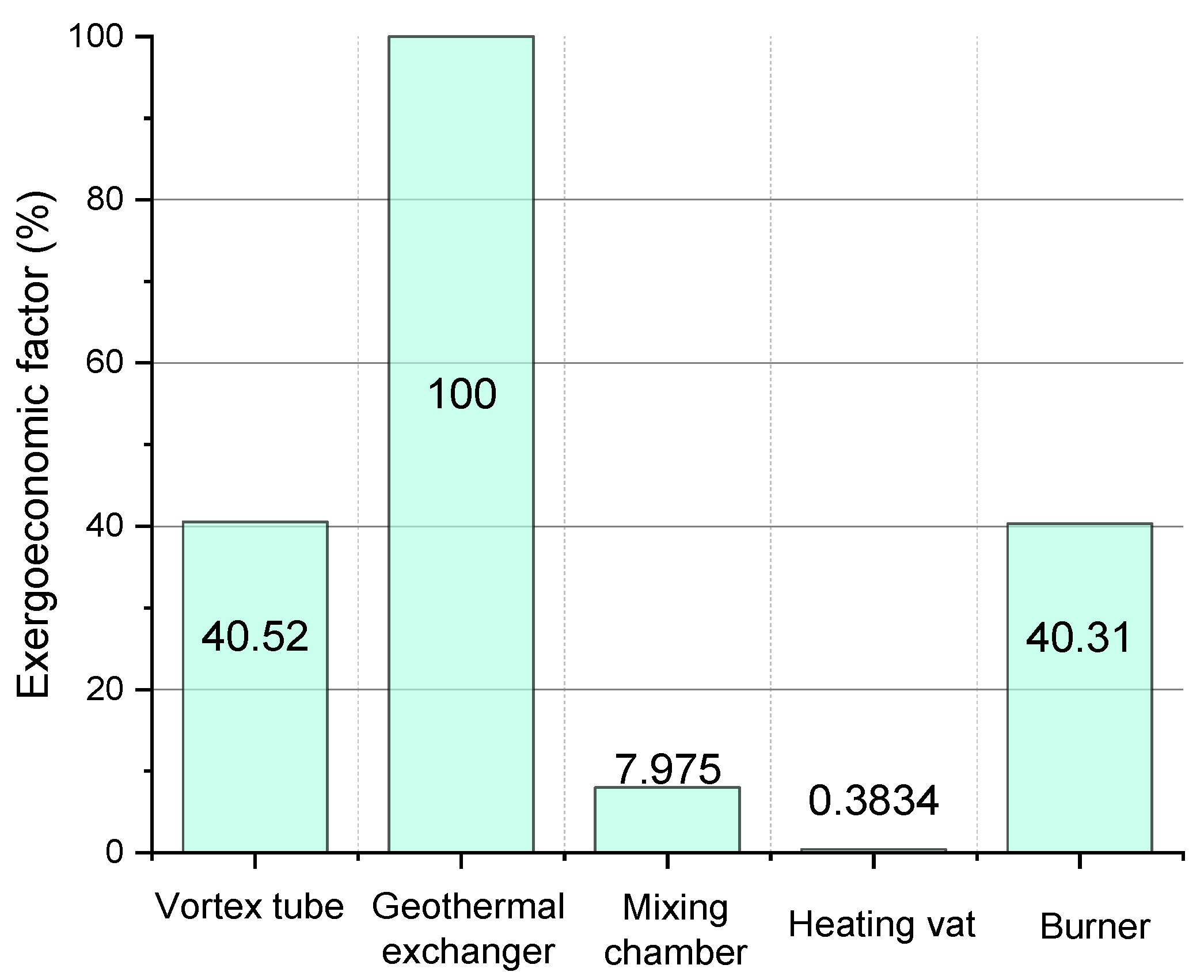
4.3. Exergoeconomic Analysis Results
Deviations Considering Real Conditions
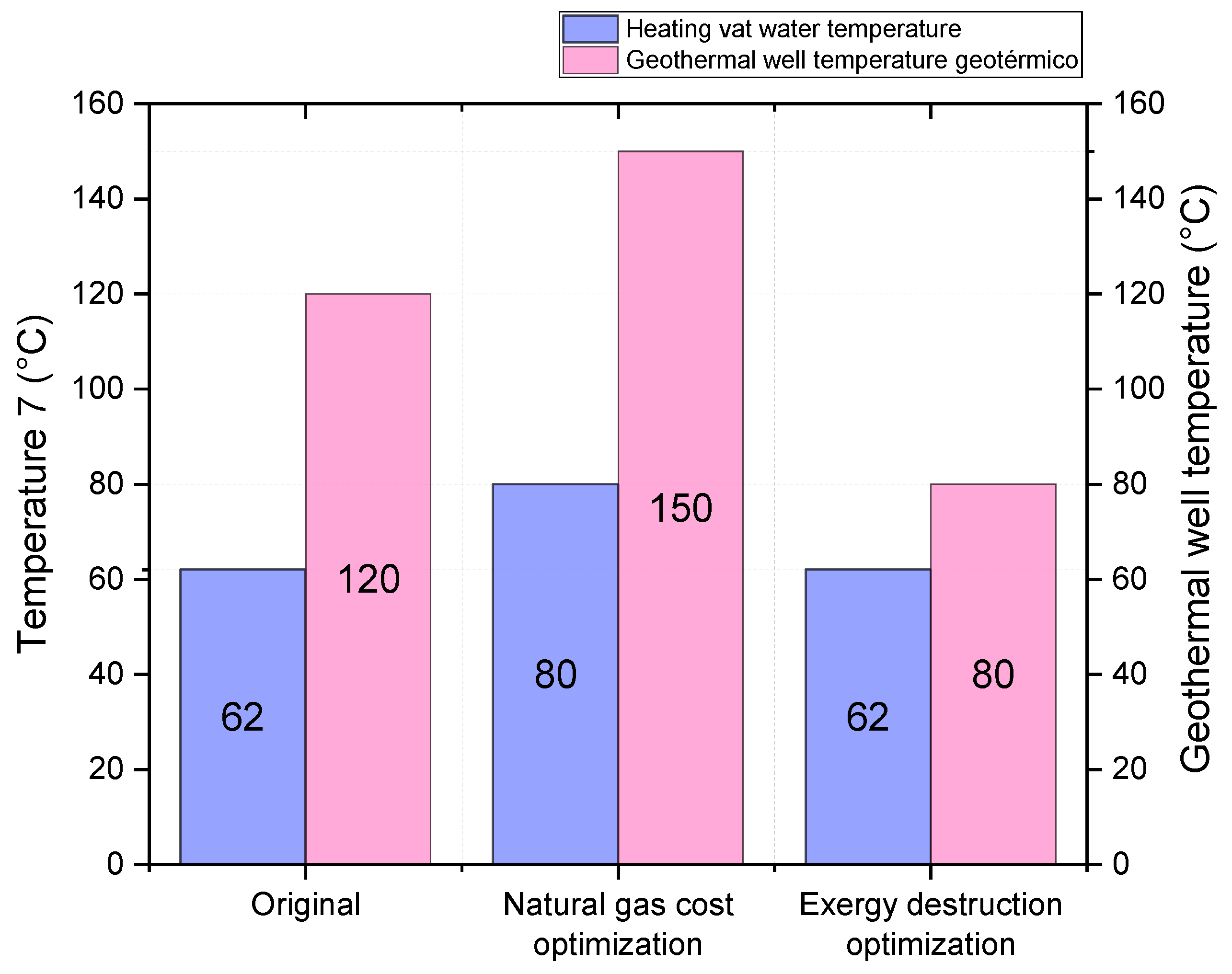
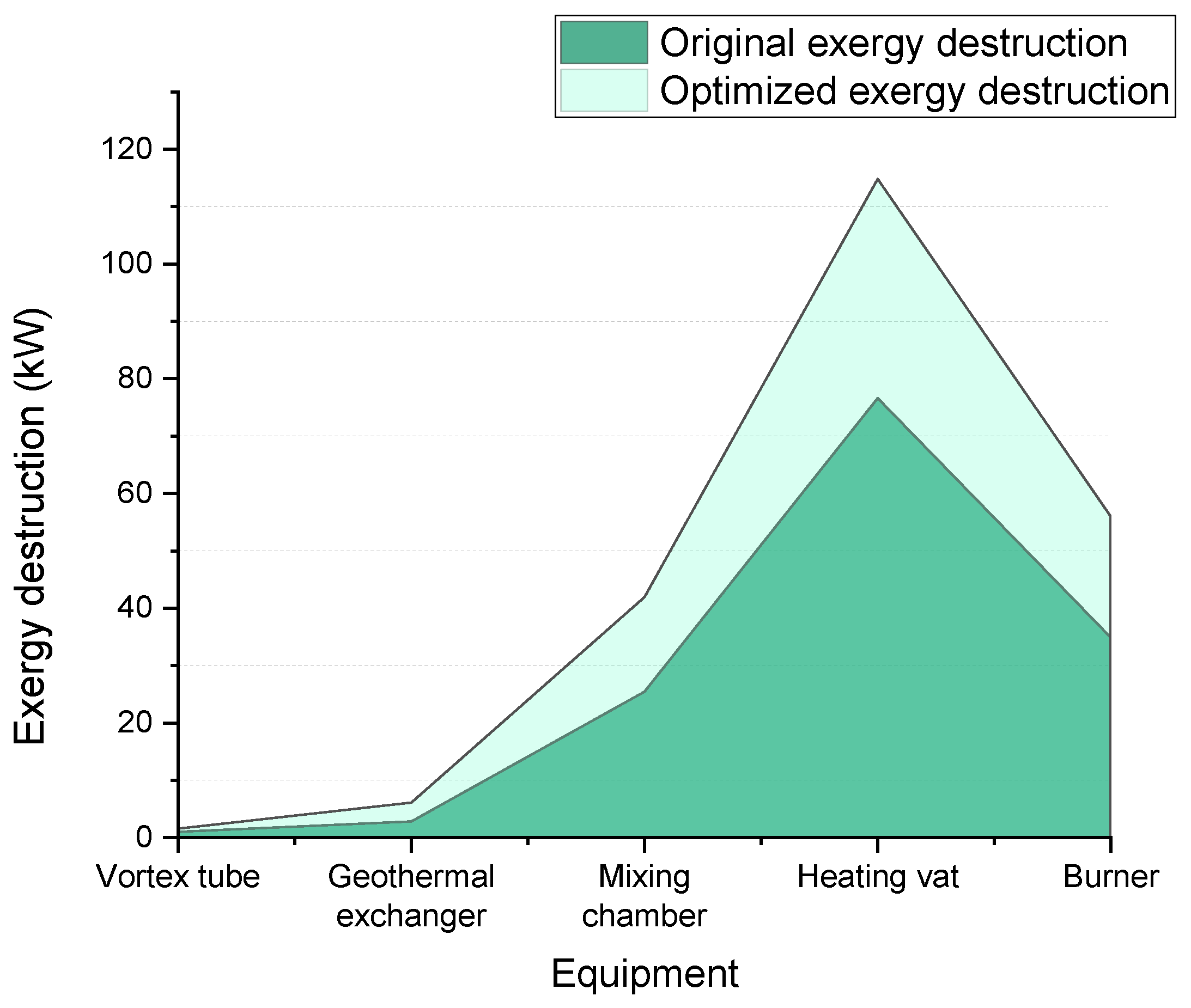
4.4. System Optimization Results
5. Conclusions
- From the energy analysis: A 69.07 kW heat flow in the heating vat and 26.28 kW heat flow in the geothermal heat exchanger are obtained. Also, the water flow needed in the heating vat is 0.3905 kg/s, meaning that 2.046% of the station’s natural gas is used for the preheating process compared to other systems that use up to 5%;
- From the exergy and exergoeconomic analysis: All component and flow costs in the system, as well as the exergoeconomics performance and the exergy destruction, are obtained, highlighting that the component with the most fuel cost is the heating vat (39.9 USD/GJ), and a natural gas exit cost of 212.9 USD/GJ. The component with the highest cost rate is the geothermal exchanger (11.7 USD/h). The vortex tube is the equipment with the lowest cost rate (0.03 USD/h). The heating vat is the equipment with the most significant amount of exergy destruction, with a cost of 16.7 USD/h which increases to USD 17.85 when the volumetric flow of the station augments from 300 to 380 . Also, it is remarked that the heating vat and the mixing chamber are the components with the smaller exergoeconomic factor, with a value of 0.3834% and 7.976%, respectively;
- From the optimization: It is possible to reduce natural gas costs by raising the heating vat water temperature by 29.03% and the volumetric flow of natural gas at the entrance by 26.66% and modifying the hot and cold flow percentage at the vortex tube outlets from a 70/30 relation to a 65/25 relation. A final cost of 179.4 USD/GJ for the natural gas was obtained, which means a cost reduction of 15.73%.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Area | |
| Exergy (kW) | |
| Cost (USD) | |
| Exergy cost (USD/s, USD/h) | |
| Specific exergy (USD/kJ, USD/GJ) | |
| Specific heat | |
| Maintenance cost (USD) | |
| Capital recovery factor | |
| Calorific value (kJ/kg) | |
| Diameter | |
| Exergy destruction (kW), | |
| Design factor | |
| Pressure factor | |
| Material factor | |
| Darcy factor | |
| Exergoeconomic factor (%) | |
| Specific material factor | |
| Convection heat transfer coefficient , enthalpy | |
| Interest rate | |
| Actual cost index | |
| Base cost index | |
| Thermal conductivity | |
| Length | |
| Logarithmic mean temperature difference | |
| Molar mass | |
| Mass (kg) | |
| Mass Flow rate | |
| Mass fraction | |
| Number of moles (mole) | |
| Period | |
| Nusselt number | |
| Pressure , power (kW) | |
| Prandtl number | |
| Heat flow | |
| Radius | |
| Relative cost difference (%) | |
| Gas constant , radius (m, in) | |
| Universal gas constant | |
| Reynolds number | |
| Temperature | |
| Overall heat transfer coefficient | |
| Volume | |
| Specific volume | |
| Volumetric flow | |
| Molar fraction | |
| Compressibility factor | |
| Cost rate (USD/s, USD/h) | |
| Natural gas percentage | |
| Greek letters | |
| Form factor | |
| Temperature change | |
| Maintenance factor | |
| Temperature difference | |
| Pressure difference | |
| Viscosity | |
| Hot fraction | |
| Cold fraction | |
| Joule–Thomson coefficient | |
| Density | |
| Subscripts | |
| Hot | |
| Exergy destruction | |
| Fuel | |
| Cold | |
| Geothermal preheating | |
| Natural gas | |
| Water | |
| Iteration | |
| Joule–Thomson | |
| Component | |
| m | Mixture |
| Product | |
| Perforation | |
| Vortex tube | |
| Reference condition | |
| Flow | |
Appendix A
Appendix A.1
Appendix A.2
References
- Riva, P.J.; Atwater, G.I.; Solomon, L.H.; Carruthers, J.E.; Waddams, A.L. Natural Gas Types, discovery, Reserves, & Facts. Encyclopedia Britannica. 2023. Available online: https://www.britannica.com/science/natural-gas/Composition-and-properties-of-natural-gas (accessed on 15 November 2023).
- Alternative Fuels Data Center: Natural Gas Fuel Basics. Office of Energy Efficiency and Renewable Energy, U.S. Department of Energy. Available online: https://afdc.energy.gov/fuels/natural_gas_basics.html (accessed on 13 October 2023).
- Mokhatab, S.; Poe, W.A.; Speight, J.G. Handbook of Natural Gas Transmission and Processing; Gulf Professional Publishing: Burlington, VT, USA, 2006; pp. 1–26. ISBN 0128158174. [Google Scholar]
- Wang, X.; Economides, M.J. Advanced Natural Gas Engineering; Gulf Professional Publishing: Houston, TX, USA, 2009; ISBN 9781933762388. [Google Scholar]
- Aroussi, A.; Benyahia, F. Proceedings of the 3rd International Gas Processing Symposium: Qatar, March 2012, 2nd ed.; Elsevier: Oxford, UK, 2012; ISBN 9780444594969. [Google Scholar]
- Olfati, M.; Bahiraei, M.; Veysi, F. A novel modification on preheating process of natural gas in pressure reduction stations to improve energy consumption, exergy destruction and CO2 emission: Preheating based on real demand. Energy 2019, 173, 598–609. [Google Scholar] [CrossRef]
- Romanov, D.; Leiss, B. Geothermal energy at different depths for district heating and cooling of existing and future building stock. Renew. Sustain. Energy Rev. 2022, 167, 112727. [Google Scholar] [CrossRef]
- DiPippo, R. Geothermal Power Plants, 4th ed.; Butterworth-Heinemann: Oxford, UK, 2015; ISBN 9780081008799. [Google Scholar]
- Llopis Trillo, G.; Angulo, V. Guía de la Energía Geotérmica, Fundación de la Energía de la Comunidad de Madrid. 2008. Available online: https://www.fenercom.com/publicacion/guia-de-la-energia-geotermica-2008/ (accessed on 14 June 2023).
- Ma, Y. Deep geothermal resources in China: Potential, distribution, exploitation, and utilization. Energy Geosci. 2023, 4, 100209. [Google Scholar] [CrossRef]
- Bamisile, O.; Cai, D.; Adun, H.; Taiwo, M.; Li, J.; Hu, Y.; Huang, Q. Geothermal energy prospect for decarbonization, EWF nexus and energy poverty mitigation in East Africa; the role of hydrogen production. Energy Strategy Rev. 2023, 49, 101157. [Google Scholar] [CrossRef]
- Diaz Molina, O.P. Valoración del Potencial Energético Basado en el Recurso Geotérmico de Baja Entalpía en la Isla de Gran Canaria. Master’s Thesis, Universidad de las Palmas de Gran Canaria, Las Palmas de Gran Canaria, Gran Canaria, 2017. [Google Scholar]
- Ouyangm, T.; Xie, S.; Tan, J.; Wu, W.; Zhang, M. Optimization of energy-exergy efficiencies of an advanced cold energy utilization system in liquefied natural gas filling station. J. Nat. Gas Sci. Eng. 2021, 95, 104235. [Google Scholar] [CrossRef]
- Gajanayake, S.M.; Gamage, R.P.; Li, X.S.; Huppert, H. Natural gas hydrates—Insights into a paradigm-shifting energy resource. Energy Rev. 2023, 2, 100013. [Google Scholar] [CrossRef]
- Esmaeilpour, M.; Korzani, M.G.; Kohl, T. Stochastic performance assessment on long-term behavior of multilateral closed deep geothermal systems. Renew. Energy 2023, 208, 26–35. [Google Scholar] [CrossRef]
- McClean, A.; Pedersen, O.W. The role of regulation in geothermal energy in the UK. Energy Policy 2023, 173, 113378. [Google Scholar] [CrossRef]
- García Contreras, O.; Muñoz Bravo, J.E.; Fajardo, F. Construcción y caracterización de un tubo Ranque-Hilsch. Bras. Ensino Física 2008, 30, 4305.1–4305.5. [Google Scholar] [CrossRef]
- Sungur, M.; Elnajjar, E.; Hamdan, M.O.; Al-Omari, S.A.B. A numerical analysis investigation to optimize the performance of the Ranque–Hilsch Vortex tube by changing the internal tapering angle. Int. J. Thermofluids 2023, 20, 100467. [Google Scholar] [CrossRef]
- Ghezelbash, R.; Farzaneh-Gord, M.; Sadi, M. Performance assessment of vortex tube and vertical ground heat exchanger in reducing fuel consumption of conventional pressure drop stations. Appl. Therm. Eng. 2016, 102, 213–226. [Google Scholar] [CrossRef]
- Bianchi, M.; Branchini, L.; De Pascale, A.; Melino, F.; Orlandini, V.; Peretto, A.; Archetti, D.; Campana, F.; Ferrari, T.; Rossetti, N. Techno-Economic Analysis of ORC in Gas Compression Stations Taking Into Account Actual Operating Conditions. Energy Procedia 2017, 129, 543–550. [Google Scholar] [CrossRef]
- Rahman, M.M. Power generation from pressure reduction in the natural. J. Mech. Eng. 2011, 41, 89–95. [Google Scholar] [CrossRef]
- Ashouri, E.; Veysi, F.; Schojaeizadeh, E.; Asadi, M. The minimum gas temperature at the inlet of regulators in natural gas pressure reduction stations (CGS) for energy saving in water bath heaters. J. Nat. Gas Sci. Eng. 2014, 21, 230–240. [Google Scholar] [CrossRef]
- Arabkoohsar, A.; Farzaneh-Gord, M.; Deymi-Dashtebayaz, M.; Machado, L.; Koury, R.N.N. A new design for natural gas pressure reduction points by employing a turbo expander and a solar heating set. Renew. Energy 2015, 81, 239–250. [Google Scholar] [CrossRef]
- Kostowski, W.J.; Usón, S. Thermoeconomic assessment of a natural gas expansion system integrated with a cogeneration unit. Appl. Energy 2013, 101, 58–66. [Google Scholar] [CrossRef]
- Ghorbani, B.; Mehrpooya, M.; Hamedi, M.; Amidpour, M. Exergoeconomic analysis of integrated natural gas liquids (NGL) and liquefied natural gas (LNG) processes. Appl. Therm. Eng. 2017, 113, 1483–1495. [Google Scholar] [CrossRef]
- Barone, G.; Buonomano, A.; Calise, F.; Forzano, C.; Palombo, A. Energy recovery through natural gas turboexpander and solar collectors: Modelling and thermoeconomic optimization. Energy 2019, 183, 1211–1232. [Google Scholar] [CrossRef]
- Deymi-Dashtebayaz, M.; Dadpour, D.; Khadem, J. Using the potential of energy losses in gas pressure reduction stations for producing power and fresh water. Desalination 2021, 497, 114763. [Google Scholar] [CrossRef]
- Sanaye, S.; Nasab, A.M. Modeling and optimizing a CHP system for natural gas pressure reduction plant. Energy 2012, 40, 358–369. [Google Scholar] [CrossRef]
- Kagiri, C.; Zhang, L.; Xia, X. Optimization of a compressed natural gas station operation to minimize energy cost. Energy Procedia 2017, 142, 2003–2008. [Google Scholar] [CrossRef]
- Deymi-Dashtebayaz, M.; Khorsand, M.; Rahbari, H. Optimization of fuel consumption in natural gas city gate station based on gas hydrate temperature (Case study: Abbas Abad station). Energy Environ. 2018, 30, 408–426. [Google Scholar] [CrossRef]
- Mohammad, N.E.; Aly, S.; Hussein, M.H. A Multi objective Optimization Method for Simulating the Operation of Natural gas Transport System. Korean J. Chem. Eng 2023.
- Cervantes Garcia, A.; Velázquez Aguilar, J.G.; Montejo Alvaro, F.; Rubio Maya, C.; Solorio Díaz, G.; Gutiérrez Sánchez, H.C. Estudio experimental del fenómeno ranque-hilsch en un tubo vórtice. In Proceedings of the XVI Congreso Internacional Anual De La Somim, Monterrey, Mexico, 22–24 September 2010; ISBN 9786079530938. [Google Scholar]
- Okolie, S.T.A.; Ozuor, O.; Fakehinde, O.; Ongbali, S.O.; Fayomi, O.S.I.; Agu, F.A. Study of Nigeria Geothermal Energy resources Viability, Brief Production Techniques and Transportation. Energy Procedia 2019, 157, 1475–1485. [Google Scholar] [CrossRef]
- Afanaseva, O.; Bezyukov, O.; Pervukhin, D.; Tukeev, D. Experimental Study Results Processing Method for the Marine Diesel Engines Vibration Activity Caused by the Cylinder-Piston Group Operations. Inventions 2023, 8, 71. [Google Scholar] [CrossRef]
- Li, C.; Zheng, S.; Li, J.; Zeng, Z. Optimal design and thermo-economic analysis of an integrated power generation system in natural gas pressure reduction stations. Energy Convers. Manag. 2019, 200, 112079. [Google Scholar] [CrossRef]
- Saryazdi, S.M.E.; Rezaei, F.; Saboohi, Y. Optimal detailed design and performance assessment of natural gas pressure reduction stations system equipped with variable inlet guide vane radial turboexpander for energy recovery. J. Nat. Gas Sci. Eng. 2021, 96, 104222. [Google Scholar] [CrossRef]
- Khoshvaght-Aliabadi, M.; Soleimani, P.; Rehman, S.; Alimoradi, A. Employing enhanced geometries in water bath heating system of natural gas pressure drop stations: Comparative study. J. Nat. Gas Sci. Eng. 2021, 87, 103775. [Google Scholar] [CrossRef]
- Waraporn, R.; Krairin, T. Improvement Vortex Cooling Capacity by Reducing Hot Tube Surface Temperature: Experiment. Energy Procedia 2014, 52, 1–9. [Google Scholar] [CrossRef]
- Ganjehsarabi, H.; Gungor, A.; Dincer, I. Exergoeconomic evaluation of a geothermal power plant. Int. J. Exergy 2014, 14, 303–319. [Google Scholar] [CrossRef]
- Maestre-Cambronel, D.; Guzmán Barros, J.; Gonzalez-Quiroga, A.; Bula, A.; Duarte-Forero, J. Thermoeconomic analysis of improved exhaust waste heat recovery system for natural gas engine based on Vortex Tube heat booster and supercritical CO2 Brayton cycle. Sustain. Energy Technol. Assess. 2021, 47, 101355. [Google Scholar] [CrossRef]
- Islam, A.; Roy, H.; Rahman, M. Energy efficiency study of household natural gas burner using pot-bottom shield and modified pot arrangement. Energy Rep. 2022, 8, 12871–12885. [Google Scholar] [CrossRef]
- NOM-001-SECRE-2010; Especificaciones Del Gas Natural. Comisión Reguladora de Energía, Diario Oficial de la Federación: Mexico City, Mexico, 2010. Available online: https://www.dof.gob.mx/normasOficiales/3997/sener/sener.htm (accessed on 3 October 2023).
- RES/596/2014; Resolución por la que la Comisión Reguladora de Energía Determina las Medidas que Deberán Implementar los Suministradores y Permisionarios de Sistemas de Transporte, Distribución y Almacenamiento de Gas Natural para dar Cumplimiento a lo Dispuesto en la Norma Oficial Mexicana NOM-001-SECRE-2010, Especificaciones del Gas Natural, así como el Sistema de Alertas para Informar oportunamente a sus Usuarios Sobre la Entrega de Gas Natural Fuera de Especificaciones. Comisión Reguladora de Energía, Diario Oficial de la Federación: Mexico City, Mexico, 2014. Available online: https://www.dof.gob.mx/nota_detalle.php?codigo=5383381&fecha=25/02/2015 (accessed on 8 February 2024).
- Çengel, Y.A.; Boles, M.A. Thermodynamics: An Engineering Approach, 7th ed.; McGraw-Hill: Boston, MA, USA, 2010; ISBN 007352932x. [Google Scholar]
- Xiong, W.; Zhang, L.; Zhao, Y.; Hu, Q.; Tian, T.; He, X.; Zhang, R.; Zhang, T. Prediction of the viscosity of natural gas at high temperature and high pressure using free-volume theory and entropy scaling. Pet. Sci. 2023, 20, 3210–3222. [Google Scholar] [CrossRef]
- Alvarado, D.A.; Bánzer, S.C. Recuperación Térmica de Petróleo, Caracas, Venezuela, 2002. Available online: https://1library.co/document/zwre527y-alvarado-d-and-banzer-c-recuperacion-termica-petroleo.html(accessed on 6 January 2024).
- Samuel, O.D.; Aigba, P.A.; Tran, T.K.; Fayaz, H.; Pastore, C.; Der, O.; Erçetin, A.; Enweremadu, C.C.; Mustafa, A. Comparison of the Techno-Economic and Environmental Assessment of Hydrodynamic Cavitation and Mechanical Stirring Reactors for the Production of Sustainable Hevea brasiliensis Ethyl Ester. Sustainability 2023, 15, 16287. [Google Scholar] [CrossRef]
- Cartlidge, J.; Chowdhury, N.; Povey, T. Performance characteristics of a divergent vortex tube. Int. J. Heat Mass Transf. 2022, 186, 122497. [Google Scholar] [CrossRef]
- Fuchs, S.; Norden, B.; Neumann, F.; Kaul, N.; Tanaka, A.; Kukkonen, I.T.; Pascal, C.; Christiansen, R.; Gola, G.; Šafanda, J.; et al. Quality-assurance of heat-flow data: The new structure and evaluation scheme of the IHFC Global Heat Flow Database. Tectonophysics 2023, 863, 229976. [Google Scholar] [CrossRef]
- Ham, J.; Kim, E.; You, N.; Cho, H. Comparison of thermal performance in solution heat exchangers with different chevron angles in absorption system. Case Stud. Therm. Eng. 2023, 51, 103598. [Google Scholar] [CrossRef]
- Gao, Q.; Zhao, S.; Zhang, Z.; Zhang, J.; Zhao, Y.; Sun, Y.; Li, D.; Yuan, H. Performance Analysis and Multi-Objective Optimization of a Cooling-Power-Desalination Combined Cycle for Shipboard Diesel Exhaust Heat Recovery. Sustainability 2023, 15, 16942. [Google Scholar] [CrossRef]
- Li, H.; Huang, H.; Xu, G.; Wen, J.; Wu, H. Performance analysis of a novel compact air-air heat exchanger for aircraft gas turbine engine using LMTD method. Appl. Therm. Eng. 2017, 116, 445–455. [Google Scholar] [CrossRef]
- Noori, N.R.; Saeed, A.F. A study of finding empirical correlation for the Nusselt number and the factors influencing it for perforated fin array of different shapes. Ain Shams Eng. J. 2024, 15, 102320. [Google Scholar] [CrossRef]
- Zhao, Q.; Mao, B.; Zhao, J.; Li, H.; Wei, S.; Bai, X.; Zhang, X.; Zhang, Y. Experimental study on the forced convection heat transfer characteristics of airflow with variable thermophysical parameters in a circular tube. Case Stud. Therm. Eng. 2022, 40, 102495. [Google Scholar] [CrossRef]
- Çengel, Y.A.; Ghajar, A.J. Heat and Mass Transfer. A Practical Approach, 3rd ed.; McGraw Hill: New York, NY, USA, 2009; ISBN 9780073250359. [Google Scholar]
- Liang, M.; Tu, J.; Zeng, L.; Zhang, Z.; Cheng, N.; Luo, Y. Thermal Performance Analysis and Multi-Factor Optimization of Middle–Deep Coaxial Borehole Heat Exchanger System for Low-Carbon Building Heating. Sustainability 2023, 15, 15215. [Google Scholar] [CrossRef]
- Lozano, M.A.; Valero, A. Theory of the exergetic cost. Energy 1993, 18, 939–960. [Google Scholar] [CrossRef]
- Wan, T.; Bai, B.; Zhou, W. Exergy destruction analysis of a power generation system utilizing the cold energy of LNG. Heliyon 2023, 9, e19393. [Google Scholar] [CrossRef] [PubMed]
- Ambriz-Díaz, V.M.; Rubio-Maya, C.; Ruiz-Casanova, E.; Martínez-Patiño, E.; Pastor-Martínez, E. Advanced exergy and exergoeconomic analysis for a polygeneration plant operating in geothermal cascade. Energy Convers. Manag. 2020, 203, 112227. [Google Scholar] [CrossRef]
- GeothermEX, Inc. New Geothermal Site Identification and Qualification [Technical Report]; Energy Commission: Sacramento, CA, USA, 2004. [Google Scholar]
- Acar, M.S.; Arslan, O. Exergo-economic Evaluation of a New Drying System Boosted by Ranque-Hilsch Vortex Tube. Appl. Therm. Eng. 2017, 124, 1–16. [Google Scholar] [CrossRef]
- Corripio, A.B.; Chrien, K.S.; Evans, L.B. Estimate costs of heat exchangers and storage tanks via correlations. Chem. Eng. 1995, 89, 125–127. [Google Scholar]
- Corripio, A.B.; Chrien, K.S.; Evans, L.B. Shell and tube Heat Exchanger Cost Estimation. Chem. Eng. 1982, 89, 125–127. [Google Scholar]
- PEDCo Environmental Inc. Cost Equations for Industrial Boilers; US Environmental Protection Agency: Washington, DC, USA, 1980.
- Fiaschi, D.; Manfrida, G.; Rogai, E.; Talluri, L. Exergoeconomic analysis and comparison between ORC and Kalina cycles to exploit low and medium-high temperature heat from two different geothermal sites. Energy Convers. Manag. 2017, 154, 503–516. [Google Scholar] [CrossRef]
- Mohammadkhani, F.; Shokati, N.; Mahmoudi, S.M.S.; Yari, M.; Rosen, M.A. Exergoeconomic assessment and parametric study of a Gas Turbine-Modular Helium Reactor combined with two Organic Rankine Cycles. Energy 2014, 65, 533–543. [Google Scholar] [CrossRef]
- Comisión Reguladora de Energía. Índices de Referencia de Precios de Gas Natural. 2023. Available online: https://www.cre.gob.mx/IPGN/ (accessed on 6 January 2024).
- NOM-002-SECRE-2010; Instalaciones de Aprovechamiento de Gas Natural. Comisión Reguladora de Energía, Diario Oficial de la Federación: Mexico City, Mexico, 2010. Available online: https://dof.gob.mx/normasOficiales/4290/sener/sener.htm (accessed on 15 October 2023).
- F-Chart Software. DIRECT Optimization Algorithm. Available online: https://www.fchart.com/ees/eeshelp/hs637.htm (accessed on 11 October 2023).
- Aviña, H.; Pérez, E. Deshidratador Geotérmico de Alimentos (DGA). Instituto de Ingeniería UNAM. Available online: https://www.iingen.unam.mx/es-mx/AlmacenDigital/Gaceta/GacetaMarzo-Abril2020/Paginas/dga-iidea.aspx (accessed on 23 October 2023).
- Saripudin; Ariadji, T.; Adisasmito, S.; Mucharam, L.; Abdassah, D. Improved Joule Thomson equation of supercritical CO2-rich natural gas in separation system. Nat. Gas Ind. B 2023, 10, 245–253. [Google Scholar] [CrossRef]






| Component | Chemical Formula | Composition (%) |
|---|---|---|
| Methane | 92 | |
| Etane | 3.5 | |
| Propane | 2.5 | |
| Butane | 0.5 | |
| Nitrogen | 0.8 | |
| Carbon dioxide | 0.5 | |
| Oxygen | 0.2 |
| Property | Equations | Units |
|---|---|---|
| Mass fraction | -- | |
| Molar mass | kg/kmol | |
| Molar fraction | -- | |
| Gas constant | ||
| Compressibility factor | P [kPa] T [K] Z [--] | |
| Density | ||
| Mass flow | kg/s | |
| Specific heat capacity | ||
| Viscosity | ||
| Thermal conductivity |
| Flow | Equation |
|---|---|
| 1–6 | |
| 7,8 | |
| 9 (Burner) | |
| 10 (Geothermal well) | |
| 11 (Heating vat) |
| Equipment | Fuel (kW) | Product (kW) | Exergy Destruction (kW) |
|---|---|---|---|
| Vortex tube | |||
| Geothermal heat exchanger | |||
| Mixing chamber | |||
| Heating vat | |||
| Burner |
| Equipment | Cost Equations | Units |
|---|---|---|
| Vortex tube | [kW] [USD] | |
| Geothermal heat exchanger | L [ft] A [] [USD] [USD] | |
| Mixing chamber | [USD] | |
| Heating vat | [USD] | |
| Burner | W] [USD] |
| Equipment | Balance | Auxiliary Equations |
|---|---|---|
| Vortex tube | ||
| Geothermal heat exchanger | ||
| Mixing chamber | -- | |
| Heating vat | ||
| Burner |
| Flow | Equation |
|---|---|
| Heating vat temperature | |
| Vortex tube cold flow outlet percentage | |
| Geothermal well temperature | |
| Station volumetric flow |
| Property | Variable | Value | Units |
|---|---|---|---|
| Logarithmic mean temperature difference | 87.64 | K | |
| Reynolds number | Re | 351,882 | -- |
| Prandtl number | Pr | 0.8496 | -- |
| Darcy factor | f | 0.01401 | -- |
| Nusselt number | Nuss | 552.2 | -- |
| Convective heat transfer coefficient | 4.429 | ||
| Overall heat transfer coefficient | U | 0.04449 | |
| Heat exchange area | A | 6.773 | |
| Additional exchanger length | 30 | m | |
| Total exchanger length | L | 72.44 | m |
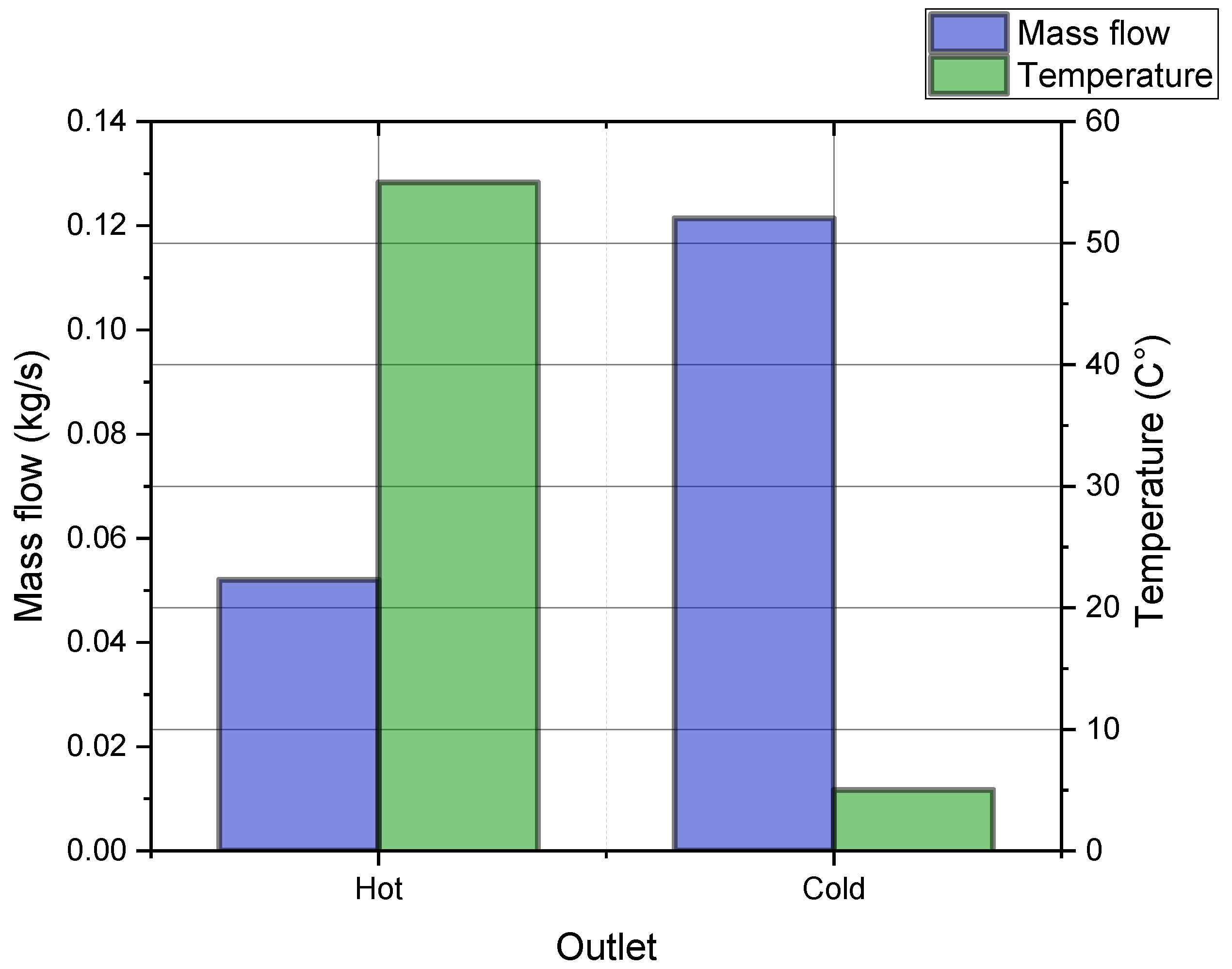
| Flow | T (°C) | (kg/s) | (kW) |
|---|---|---|---|
| 1 | 20 | 0.1734 | 138.8 |
| 2 | 55 | 0.05201 | 41.33 |
| 3 | 5 | 0.1214 | 95.85 |
| 4 | 55 | 0.1214 | 96.44 |
| 5 | 55 | 0.1734 | 137.8 |
| 6 | 20 | 0.1734 | 27.35 |
| 7 | 62 | 0.3905 | 4.49 |
| 8 | 25 | 0.3905 | 0.06886 |
| 9 | -- | 0.003547 | 60.43 |
| 10 | 120 | -- | 6.709 |
| Equipment | Exergy Destruction (kW) |
|---|---|
| Vortex tube | 1.595 |
| Geothermal heat exchanger | 6.116 |
| Mixing chamber | 41.93 |
| Heating vat | 114.8 |
| Burner | 56.01 |
| Total | 220.5 |
| Equipment | ||
|---|---|---|
| Vortex tube | 0.04478 | 1.955 |
| Geothermal heat exchanger | -- | -- |
| Mixing chamber | 4.759 | 0.3955 |
| Heating vat | 16.07 | 447.7 |
| Burner | 1.572 | 3285 |
| Variable | Ideal | Real | Variation (%) |
|---|---|---|---|
| Exergy destruction (kW) | 220.5 | 261.7 | 18.68 |
| Natural gas cost (USD/GJ) | 212.9 | 219.9 | 3.29 |
| Exergy efficiency (%) | 21.42 | 21.11 | 1.45 |
| Variable | Original Value | Natural Gas Cost Optimization | Exergy Destruction Optimization |
|---|---|---|---|
| Cold flow fraction () | 0.70 | 0.65 | 0.73 |
| Volumetric flow () [] | 300 | 380 | 200 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Villalón-López, L.F.; Ambriz-Díaz, V.M.; Rubio-Maya, C.; Chávez, O.; Rosas, I.Y. Energy, Exergy, Exergoeconomic Analysis, and Optimization in a Natural Gas Decompression Station with a Vortex Tube and Geothermal Preheating. Sustainability 2024, 16, 1669. https://doi.org/10.3390/su16041669
Villalón-López LF, Ambriz-Díaz VM, Rubio-Maya C, Chávez O, Rosas IY. Energy, Exergy, Exergoeconomic Analysis, and Optimization in a Natural Gas Decompression Station with a Vortex Tube and Geothermal Preheating. Sustainability. 2024; 16(4):1669. https://doi.org/10.3390/su16041669
Chicago/Turabian StyleVillalón-López, Luis F., Víctor M. Ambriz-Díaz, Carlos Rubio-Maya, Oscar Chávez, and Israel Y. Rosas. 2024. "Energy, Exergy, Exergoeconomic Analysis, and Optimization in a Natural Gas Decompression Station with a Vortex Tube and Geothermal Preheating" Sustainability 16, no. 4: 1669. https://doi.org/10.3390/su16041669
APA StyleVillalón-López, L. F., Ambriz-Díaz, V. M., Rubio-Maya, C., Chávez, O., & Rosas, I. Y. (2024). Energy, Exergy, Exergoeconomic Analysis, and Optimization in a Natural Gas Decompression Station with a Vortex Tube and Geothermal Preheating. Sustainability, 16(4), 1669. https://doi.org/10.3390/su16041669






