Development of an Environmentally Friendly Steel Structural Framework: Evaluation of Bending Stiffness and Yield Bending Moment of Cross-Laminated Timber Slab–H-Shaped Steel Composite Beams for Component Reuse

Abstract

The building and construction sector accounts for nearly 40% of global greenhouse gas emissions, with steel-framed buildings being a significant contributor due to high CO₂ emissions during production. To mitigate this issue, integrating Cross-Laminated Timber (CLT) into structural systems has emerged as a sustainable alternative. CLT, known for its carbon sequestration properties, offers an environmentally friendly replacement for reinforced-concrete slabs, particularly when paired with steel structures to enhance material reuse and reduce lifecycle impacts. This study focuses on hybrid systems combining H-shaped steel beams and CLT floor panels connected using high-strength friction bolts. A four-point bending test, simulating a secondary beam, was conducted, demonstrating that the composite effect significantly enhances flexural stiffness and strength. Additionally, a simplified method for evaluating the flexural stiffness and yielding strength of these composite beams, based on material and joint properties, was shown to successfully evaluate the test results.

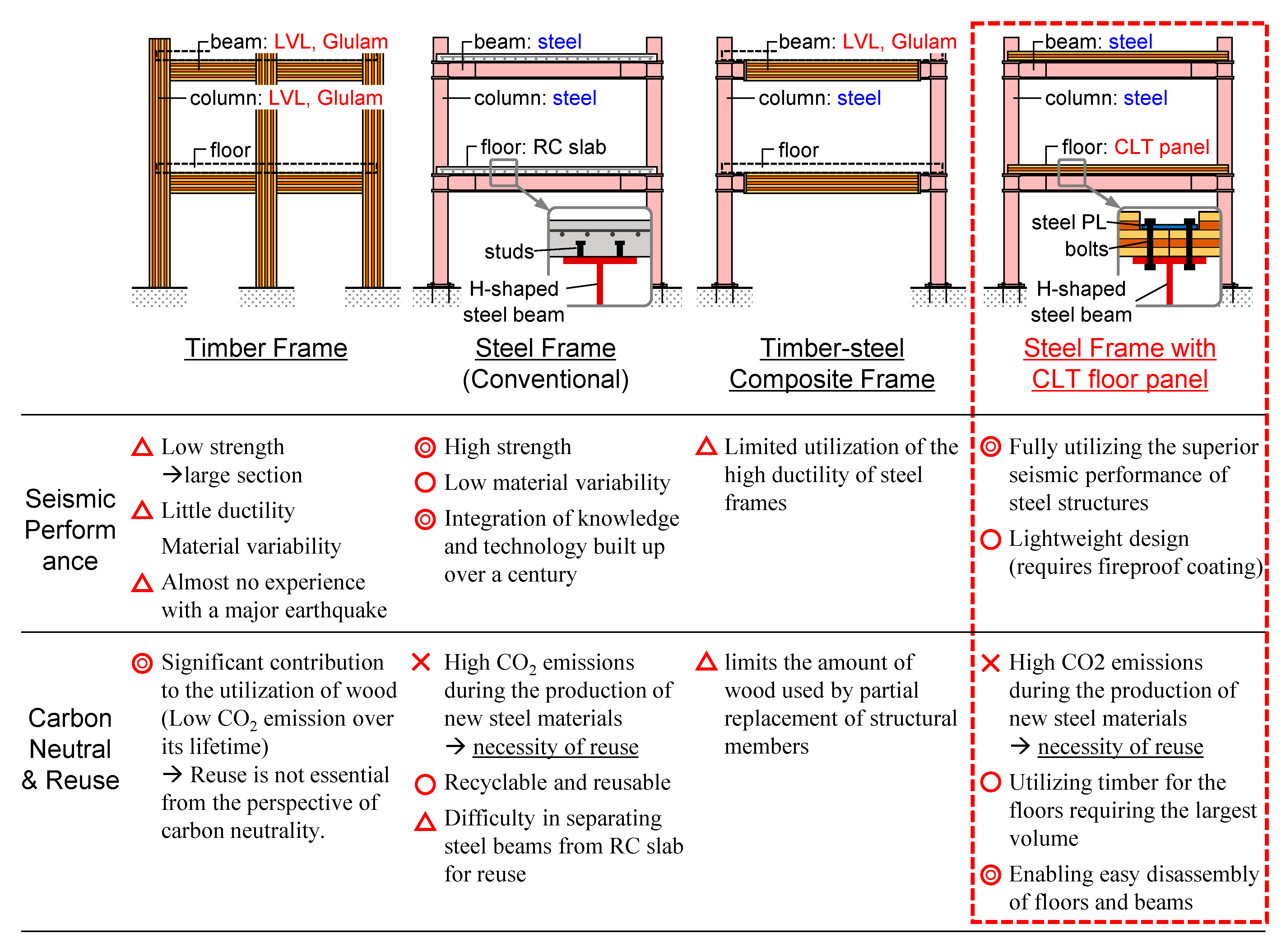
1. Introduction

The building and construction sector is widely recognized as a significant contributor to global greenhouse gas (GHG) emissions, accounting for nearly 40% of the total [1]. Efforts to mitigate emissions in this sector present a substantial opportunity to advance global sustainability objectives. A large share of these emissions arises from the production of building materials and the construction process itself [2,3,4]. Therefore, the selection of structural systems during the construction phase is crucial in shaping a building’s total lifetime emissions.

Steel-framed buildings, which have significantly contributed to the development of mid- to high-rise buildings due to their high strength and resilience, now face the need for fundamental transformation due to their high CO₂ emissions, particularly during the steel manufacturing phase. Efforts to reduce CO₂ emissions during the production of steel are actively underway. For instance, studies show that steelmaking using electric arc furnaces with scrap materials can reduce CO₂ emissions by 25%, with further reductions achievable through the use of renewable energy sources [5,6]. Recycling and reuse are also considered promising methods for minimizing life cycle energy consumption and CO₂ emissions in steel structures. Notably, reuse has been reported to reduce CO₂ emissions by nearly 90% compared to recycling in certain cases [7]. Therefore, reusing steel has emerged as a critical approach for achieving environmentally and economically sustainable construction practices [7,8,9,10]. However, several challenges remain. These include the lack of a database to determine the feasibility of reusing dismantled members, as well as higher storage and transportation costs compared to new production [7,11,12]. Additionally, buildings designed with reused members may have greater weight than those built with new materials due to the limited availability of suitable member cross-sections [9,13]. Proposed solutions include the establishment of industry-wide regulations and unified standards for reuse, simplification of inspection and certification processes, and the adoption of digital technologies for inventory and demand management of reusable members [7,11,12,13,14,15]. To promote a circular economy through the reuse of structural components, adopting modular floor plans is a viable approach [16,17]. Furthermore, flexibility in floor plans, high load-bearing capacity, and the removability of non-structural components are essential factors for facilitating reuse [18].

In Japan—a country frequently affected by major earthquakes such as the 2011 Great East Japan Earthquake, the 2016 Kumamoto Earthquake, and the 2024 Noto Peninsula Earthquake—seismic resilience is the highest priority. Ensuring seismic resilience while considering the ultimate failure mechanism is essential. To address both seismic resilience and the reduction in CO₂ emissions, Figure 1 summarizes the characteristics of modern timber buildings, steel structures, and steel–wood hybrid structures. It should be noted that the evaluations shown in Figure 1, such as double circles or triangles, are simplified for clarity and do not always have data-based evidence. Here, reinforced-concrete structures, one of the most representative forms of modern architecture, are excluded from consideration due to their integrated construction of structural and non-structural components, which inherently makes reuse difficult.

As summarized in the figure, modern timber structures, which have seen significant development in recent years, are often considered symbolic of the “carbon-neutral” era [2,3,4,19,20,21]. In Japan, the Act for Promotion of Use of Wood in Public Buildings was enacted in 2010 [22], introducing initiatives such as government subsidies to encourage the construction of wooden buildings. Nakano et al. calculated the GHG emissions of buildings using CLT (Cross-Laminated Timber) panels that meet Japan’s seismic standards, concluding that CLT is a promising building material capable of reducing environmental impact even in earthquake-prone regions [23]. However, in this disaster-prone country, uncertainties arising from variations in material quality cannot be overlooked, and the mechanical properties of connections remain less explored compared to steel structures. In particular, studies examining the ultimate failure state of timber buildings during major earthquakes remain insufficient. On the other hand, steel structures, known for their high strength and toughness, have continuously evolved over the past century in response to damage caused by major earthquakes in Japan. Their design and construction technologies have been refined to achieve a high level of seismic performance today. Although the production of new steel materials results in significant CO₂ emissions, steel structures offer the advantage of being relatively easy to reuse. This is due to their high strength, which allows for large spans with minimal cross-sections, enabling flexibility in floor plans. Additionally, as prefabricated structures, they are easy to disassemble, making the efficient reuse of structural members highly feasible. A major challenge lies in the difficulty of dismantling concrete slabs and steel members that are integrally connected through studs. Steel–timber composite systems have been proposed as a means to reduce CO₂ emissions in steel construction, such as by partially replacing steel members with timber [24]. However, the use of timber is limited, and challenges remain regarding the seismic reliability of connection methods between the different structural materials. In response, a “steel frame structure with CLT floor panels”, which retains the steel frame as the structural skeleton while using CLT floor panels as a substitute for RC slabs, could be one of the most promising solutions. While floor materials require the largest volume of material, they are not primary seismic structural elements. This makes it possible to extensively use timber without relying on its variable properties for seismic safety. Reference [25] reports that replacing reinforced-concrete floors in steel structures with CLT can achieve up to a 20% reduction in global warming potential (GWP) compared to steel–concrete floors, even when designed to meet 1 h and 2 h fire resistance ratings. Furthermore, if the connection between the CLT floor panels and H-shaped steel beams is designed as a detachable joint, construction efficiency can be improved compared to RC slabs. At the same time, the complete reuse of all major structural components becomes feasible.

Figure 2 illustrates the connection methods between CLT floor panels and H-shaped steel beams proposed in previous studies [26,27,28,29,30,31,32,33,34,35,36,37,38,39,40,41], for example. Reference [26] proposed various methods for connections using screws and shear plates, while References [28,29,30] investigated the mechanical characteristics of connections using screws and bolts through push-out tests and four-point bending tests, supported by numerical simulations. References [31,32,33] examined cases where CLT floor panels are connected to aluminum members. Reference [34] specifically analyzed the effect of the number of bolts and their spacing on composite action. References [35,36,37,38,39,41] proposed improved connections using screws and bolts with mortar and epoxy, and reference [40] developed connections using studs on steel. The joining methods can be broadly categorized as follows: (a) using screws, (b) using bolts, (c) bonding the interfaces, and (d) using studs and adhesives. Method (c) is commonly combined with (a) or (b). Method (a), fixing CLT floor panels with screws, offers the advantage of easy construction. However, the reuse of CLT panels with pre-drilled screw holes is likely to reduce the rigidity of the connections. Reference [41] proposes a method in which lag screws are pre-inserted into CLT floor panels, enabling a detachable screw-fastening system. However, this approach presents challenges in separating the lag screws embedded in the CLT panels during final disposal. On the other hand, bolt-based connections (method (b)) make it easier to disassemble CLT panels and steel beams, facilitating the reuse of components. Additionally, studies [27,37] have shown that bolt-based connections exhibit higher stiffness and load-bearing capacity compared to screw-based connections. These issues can be mitigated by combining method (c), bonding the interfaces, as noted in [27,28]. However, from the perspective of component reuse, the use of adhesives should be avoided unless absolutely necessary. This consideration also applies to method (d) [40].

Among the joining methods proposed in previous studies for connecting CLT floor panels and steel beams, bolt-based connections are the most advantageous in terms of component reuse. Figure 3 illustrates a conceptual diagram of a steel-framed moment-resisting structure using this construction method. In this method, bolts are inserted through pre-drilled holes at the edges of the CLT floor panels and matching holes in the H-shaped steel beams, with a specified clamping force applied to secure them. To address issues with bearing pressure, a plate is used to distribute the pressure more evenly. The initial tension applied to the high-strength bolts generates static friction between the CLT panels and the upper flange of the H-shaped steel beam, thereby achieving a composite effect between the two. For the steel plate washers and bolt heads protruding from the upper surface of the CLT panels, Figure 3 shows a layered installation approach. This approach accommodates these protrusions within the bottom lamina of the second layer of CLT panels.

Fixing steel members to the slab is essential for enhancing the flexural stiffness and strength of beams through composite action, as well as providing restraint against lateral–torsional buckling [42,43,44,45]. In studies employing bolt connections [27,28,29,30], it has been shown that the composite effect can be sufficiently achieved through the bolted connection between H-shaped steel beams and CLT floor panels, and the failure mechanisms have also been presented. Additionally, analytical models capable of reproducing the mechanical behavior have been proposed. However, the relationship between the bolt clamping force and frictional force, which determines the mechanical properties of the connection, has not been fully clarified. In addition, the method for simply estimating the composite effect of CLT floor panels and H-shaped steel beams during design has not yet been presented.

This study focuses on the secondary beam and evaluates whether the flexural stiffness and flexural strength of composite beams, comprising CLT floor panels and H-shaped steel beams connected with high-strength friction bolts, can be simply estimated for design purposes based on the properties of the members and joints, without relying on advanced analysis. Establishing a simplified evaluation model that mechanically clarifies the key factors controlling the composite effect is expected to facilitate the future adoption of the proposed structural system. In Section 2, two-surface friction tests between steel plates and CLT, connected by high-strength friction bolts, were conducted with bolt clamping force as a variable. In Section 3, four-point bending tests were performed on composite beams. These tests clarified the flexural stiffness and yield flexural strength achieved through the composite effect. Additionally, the effective width of the CLT floor panels in the composite beams was identified. Finally, using the experimentally determined effective width of the CLT floor panels and the static friction force of the high-strength friction bolt connections, it was verified whether the flexural stiffness and yield flexural strength due to the composite effect of the CLT floor panels and H-shaped steel beams in the four-point bending tests could be evaluated.

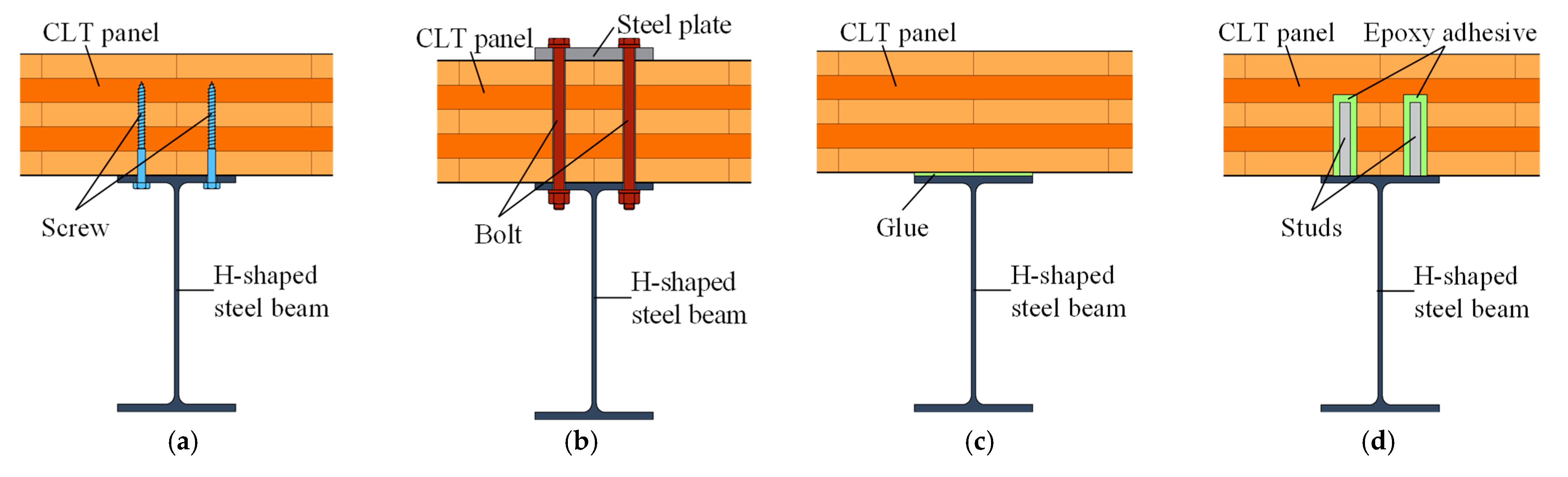
2. Double-Shear Tests of CLT–Steel Plate Friction Joints Using High-Strength Bolts

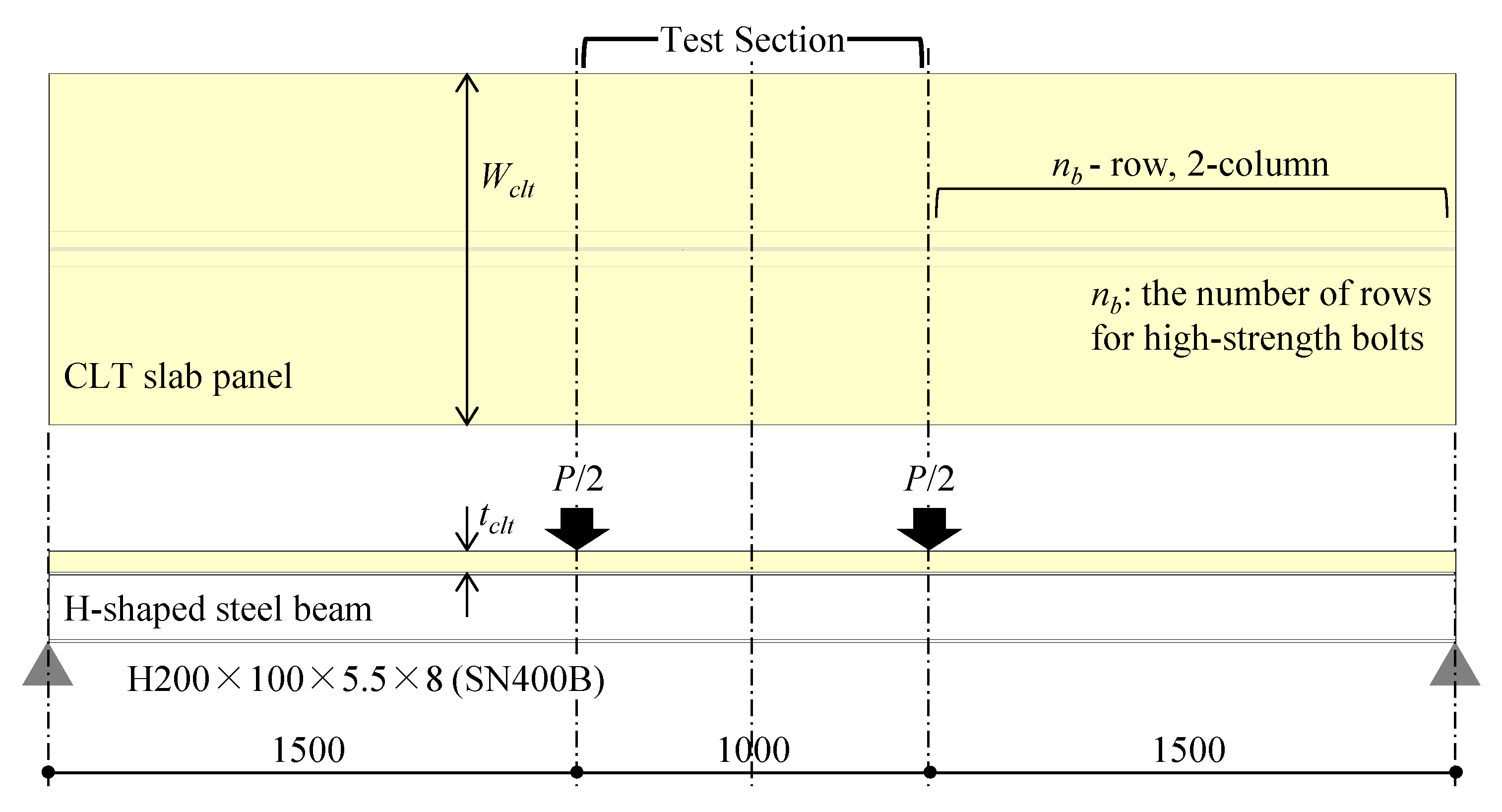
2.1. Test Setup and Test Parameters

The CLT panels used in the experiments in Section 2 and Section 3 are made of sugi (Japanese cedar) and consist of three-layer Cross-Laminated Timber panels (S60A-3-3), with each layer having the same grade composition as specified in the Japanese Agricultural Standard (JAS) 3079 [45]. According to this specification, the CLT panels consist of three layers: the grain directions of the top and bottom layers are aligned, while the grain direction of the middle layer is arranged perpendicular to them. The material grade for the CLT panels was specified as M60B [45]. The material standards defined in JAS 3079 are summarized in

Table 1. Material property standards for timber layers used in CLT panels [45].

Grade	Young’s Modulus in Bending [GPa]			Flexural Strength [MPa]		Tensile Strength [MPa]
	Average	Min.	Max.	Average	Min.	Average	Min.
M60B	6.0	5.0	9.0	27.0	20.0	16.0	12.0

. All laminations used in the CLT panels were subjected to 100% testing following the method specified in Standard [45], and only those that satisfied the Young’s modulus standards defined in Table 1 were used in panel production. The average and the standard deviation of Young’s modulus, moisture content and the density of laminations was 7.3 N/mm² with 0.99, 9.8% with 1.3 and 0.35 g/cm³ with 0.03. Water-based polymeric isocyanate resin was used for bonding lamina during CLT production.

The static friction force between the CLT panels tightened with high-strength bolts M16 (F10T) [46] and the steel plates was evaluated using two-surface friction tests conducted with an Amsler testing machine.

Table 2. List of specimens for two-surface friction tests of bolted CLT panels and steel plates.

Specimen	Bolt Size	Steel Plate Washer Size	Thickness of CLT Panel tclt [mm]	Bolt Rotation Angle ϕ [Degree]
t45-ϕ **1	M16	50 × 80 × 10	45	60, 120, 180, 270, 360, 450
t45-ϕ **1			60	60, 120, 180, 270, 360, 450, 540
t45-ϕ **1			75	60, 120, 180, 270, 360, 450, 540

¹ The ** in the specimen names indicates the bolt tightening angle for each specimen.

lists the test variables, and Figure 4 shows (a) the details of the test specimens and (b) the experimental setup for the two-surface friction tests. As shown in Figure 4, CLT panels with thicknesses of t_clt = 45, 60, and 75 mm were each cut in half at the middle layer, with the cut surfaces placed outward. The steel plates were sandwiched between the pair of half-cut panels and secured with bolts. The CLT panels were arranged such that the direction of frictional force from the steel plates aligned with the grain direction of the wood. As shown in Figure 4, steel plate washers were used to tighten the CLT panels in order to avoid excessive stress concentration on the CLT due to the bolt clamping force. The dimensions of the steel plate washers were set to a depth of 50 mm and a width of 80 mm. This was based on the setup in the four-point bending tests described in Section 3, where the CLT floor panels were bolted to H-shaped steel beams with a floor width of 100 mm, using two bolts per row. Rust-inducing treatment was applied to both the steel plates and the steel plate washers to increase the friction coefficient. The loading rate is set to 0.4 mm/s. Since the purpose was to evaluate the static friction force, the loading was terminated once the displacement measured by the Amsler testing machine reached 4–6 mm, before the slipping bolts came into contact with the holes in the CLT.

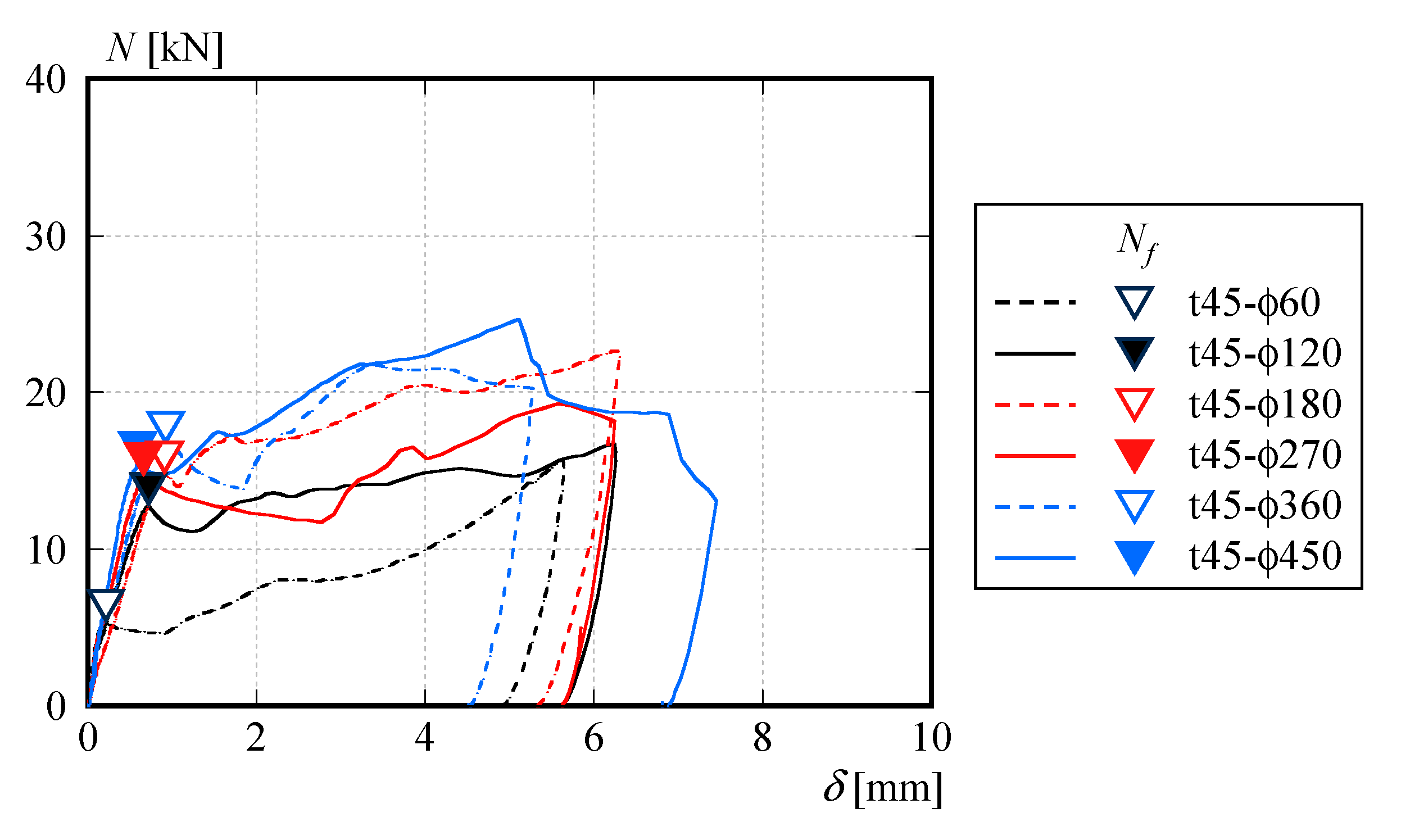
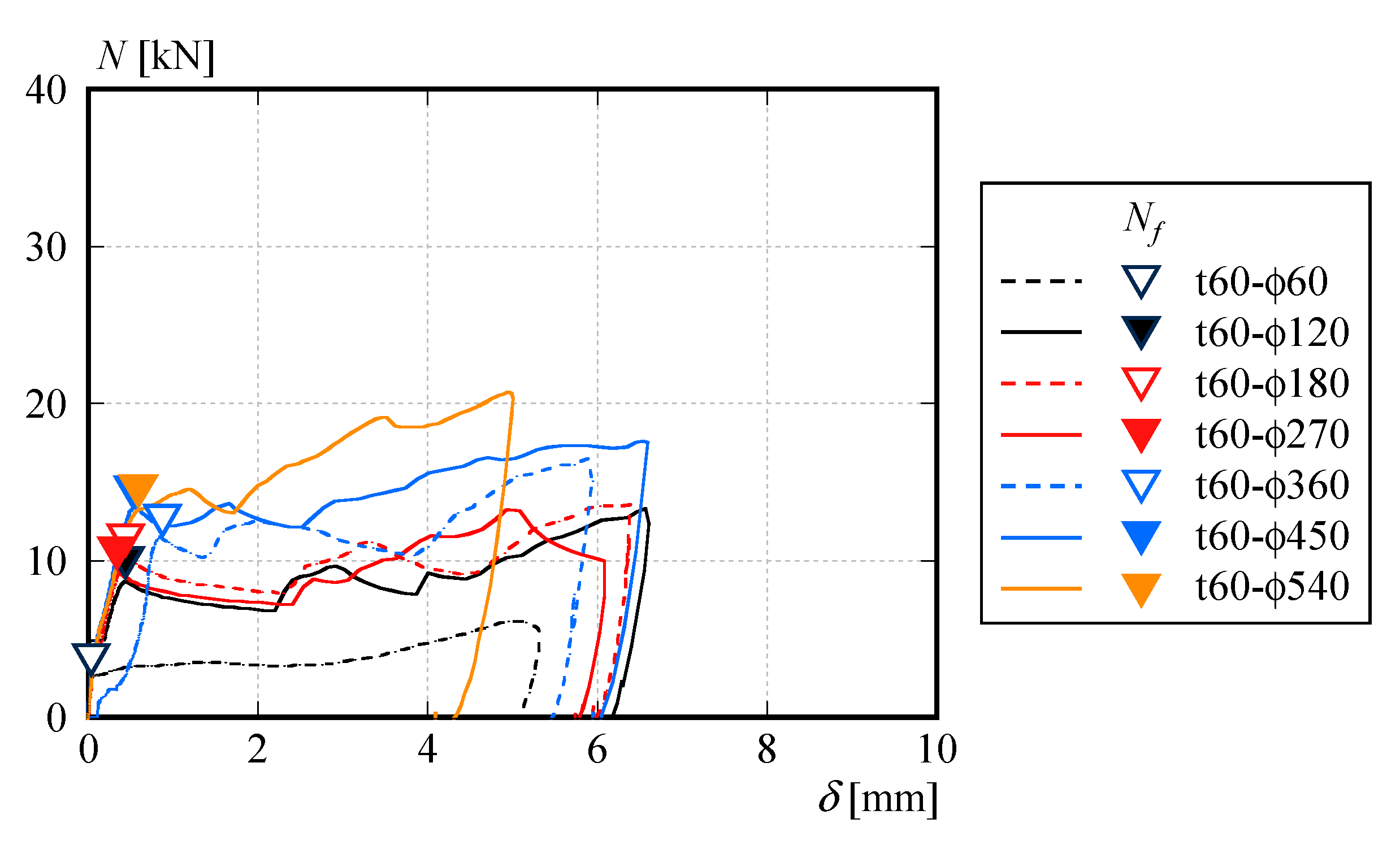
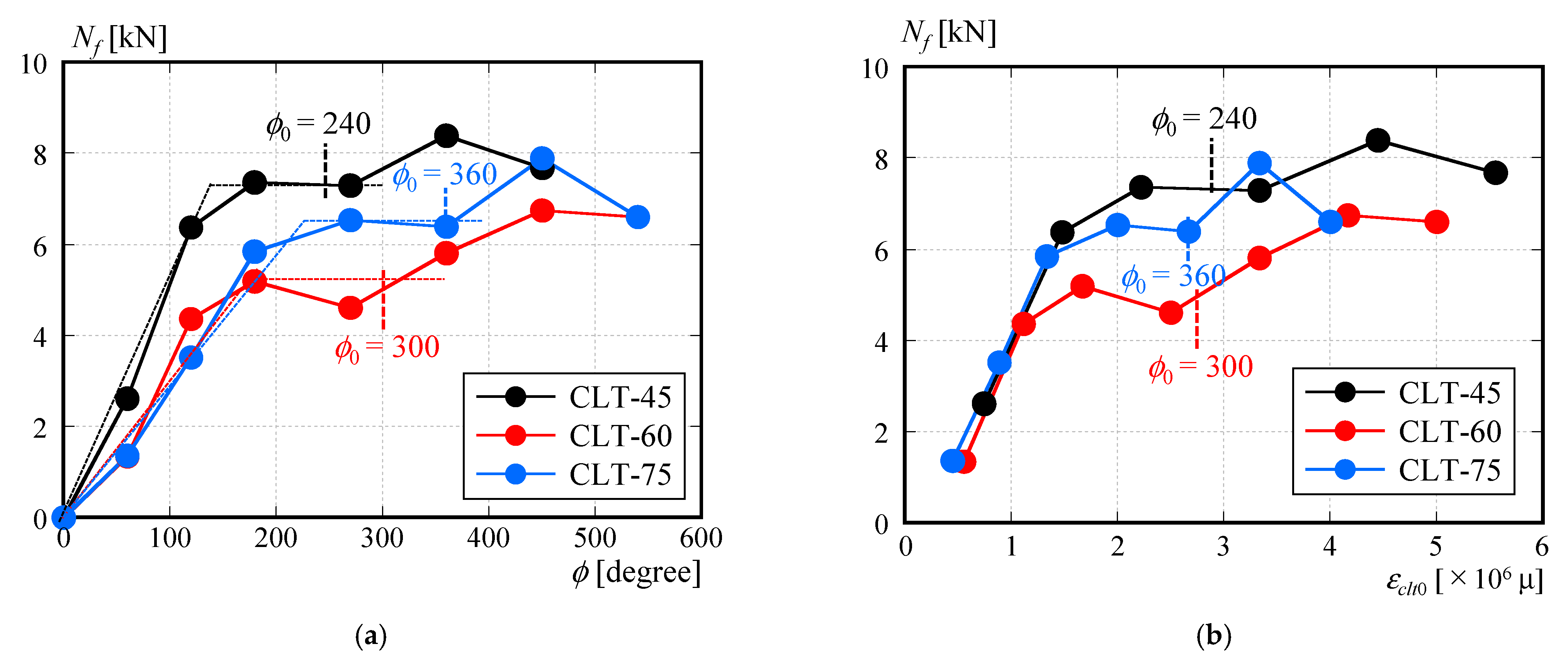
2.2. Static Friction Force with Respect to Bolt Tightening Angle

Figure 5, Figure 6 and Figure 7 show the results of two-surface shear tests for CLT panel thicknesses of t_clt = 45, 60 mm, and 75 mm. The vertical axis represents the applied shear force N, while the horizontal axis represents the displacement δ measured by the Amsler testing machine. From the figure, the displacement increases linearly at low shear forces. However, upon reaching a certain load, the shear force momentarily decreases slightly, followed by a region of increasing displacement. The point at which the shear force reaches its maximum within the range of linear increase and prior to the slight drop is defined as the point where static friction force N_f is achieved, indicated by inverted triangles in the figure.

Figure 8 shows the condition of the CLT after removing the steel plate washers tightened with high-strength bolts following the two-surface shear test. From the figure, no tightening marks are observed at a tightening angle of 120° for any of the samples, while tightening marks are observed at a tightening angle of 180°. This indicates that, within the range of CLT panel thicknesses tested in the current study, the CLT begins to experience bearing yield at tightening angles between 120° and 180°.

Figure 9a summarizes the relationship between the static friction force N_f between the CLT and steel plate and the nut tightening angle ϕ, as obtained from Figure 5, Figure 6 and Figure 7. The static friction force N_f was calculated as half of the shear force at the points indicated by inverted triangles in the figures. From Figure 9, for all CLT thicknesses, the static friction force N_f increases linearly at tightening angles ϕ = 60° and 120°, but tends to remain constant at ϕ ≥ 180°. In Figure 8, tightening marks on the CLT from the steel plate washer are observed starting at ϕ = 180°, indicating that the CLT likely experienced bearing yield. To generalize the relationship between the thickness of the CLT panels and the bolt rotation angle, Figure 9b illustrates the relationship between the static friction force N_f and the equivalent compressive strain of the CLT ε_clt, plotted on the horizontal axis. The compressive strain of the CLT ε_clt was calculated by determining the tightening displacement from the bolt rotation angle ϕ, based on the thread pitch of 2 mm for the M16 bolt, assuming that all compressive deformation occurs within the CLT. From Figure 9b, it can be observed that the initial stiffness is consistent across all CLT panel thicknesses, indicating that the axial deformation caused by bolt tightening occurs almost entirely within the CLT. In this study, the optimal bolt rotation angle ϕ₀ for tightening the CLT floor panels in the four-point loading test described in Section 3 was determined by referring to the standard practice of tightening high-strength bolts in steel-to-steel friction joints with a specified torque, followed by an additional 120° rotation. The dashed line in the figure represents the experimental results modeled using a bilinear approximation to determine the tightening angle at which bearing yield occurs. Here, the optimal rotation angle was set as the angle at which the CLT undergoes bearing yield, plus an additional 120°. Accordingly, the bolt rotation angle ϕ₀ was set to 240°, 300°, and 360° for CLT panel thicknesses of t = 45, 60, and 75 mm, respectively.

Since the bearing yield strength of sugi (Japanese cedar) has not been sufficiently investigated, this paper attempts to predict the results of the composite beam tests in Section 3 using the static friction force N_f obtained from the two-surface shear tests. The investigation of the optimal tightening angle based on the bearing yield strength of sugi (Japanese cedar) is left as a subject for future work.

3. Four-Point Bending Test of CLT-Panel—H-Shaped-Steel Composite Beam

This section presents four-point bending tests on H-shaped steel beams with CLT panels and evaluates the flexural stiffness and flexural strength of composite beams connected with friction joints using high-strength bolts.

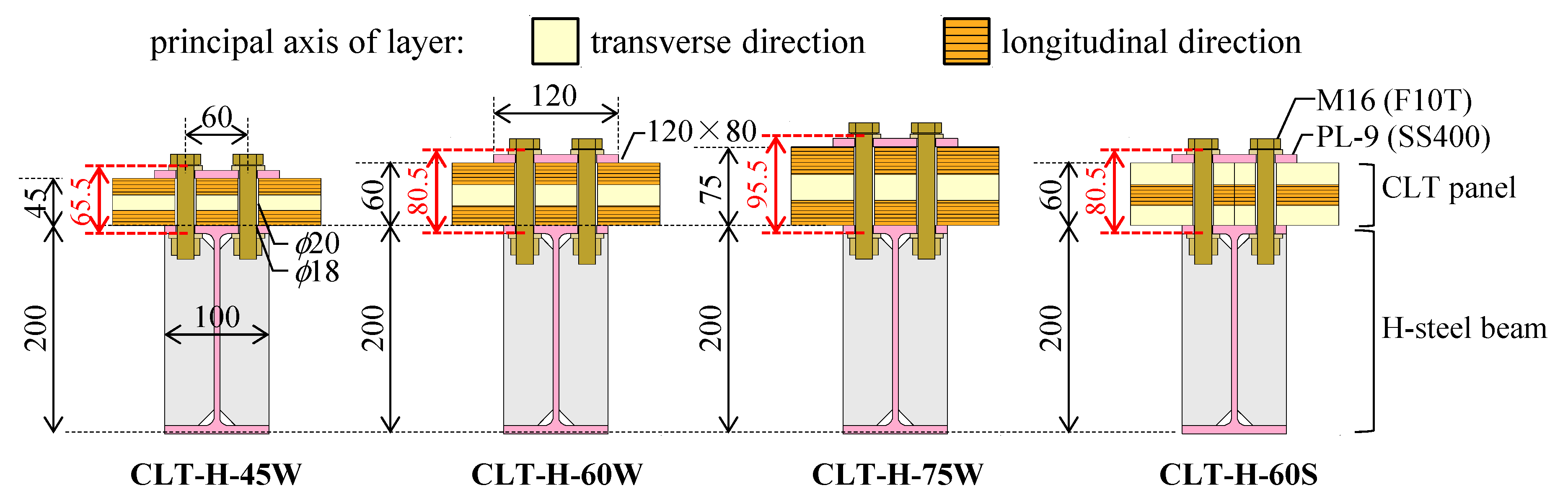
3.1. Specimen Design

In the four-point bending test, an H-shaped steel beam with dimensions H200 × 100 × 5.5 × 8 (SN400B [47]) was used. This selection was based on reference [48], which evaluated the lateral buckling strength of H-shaped steel beams with folded plates. Similarly, reference [49] utilized a beam of the same size, HN200 × 100 × 5.5 × 8, to conduct a four-point bending test on a steel–timber composite beam. Figure 10 illustrates the variables to be determined for conducting a four-point bending test. In reference [38], a four-point bending test was performed on a specimen consisting of a CLT floor panel with a thickness of 80 mm and a width of 250 mm, fixed to an H200 × 100 × 5.5 × 8 steel beam. Likewise, reference [40] conducted a four-point bending test on a specimen with a CLT floor panel 90 mm thick and 500 mm wide, fixed to an H350 × 175 × 7 × 11 steel beam. However, these references and other related research [27,29,31,33,38] do not provide sufficient information on how the thickness and width of the CLT panels were determined. Therefore, in this study, the dimensions (t_clt, W_clt) of the CLT panels for the test specimens were determined by referencing actual cross-sections of steel-framed rigid structures, as described below.

The thickness of the CLT floor panel corresponding to a reduced-scale H-beam (H200 × 100 × 5.5 × 8) was selected so that the flexural stiffness and flexural strength ratios of the composite beam would approximately match those in a full-scale model building. Figure 11 shows the structural diagram of a building model described in the textbook on the design of steel structures provided by the Japan Society of Steel Construction [49]. The structure represents a four-story moment-resisting frame designed for office use. The office space consists of girders with a 9 m span and secondary beams placed at 3 m intervals. In the original example building, a 120 mm-thick reinforced-concrete slab with a deck plate was used. Here, it is assumed that CLT floor panels are applied to this building. Figure 11 indicates the strong-axis direction of the CLT panels. According to the Design Guidebook of CLT Buildings [50], the required thickness of the structural floor to meet the deflection limit is about 150 mm for a span of approximately 3 m. A project commissioned by the Japan Forestry Agency, which examined the use of CLT for flooring in steel-structured office buildings [51], found that while the adoption of CLT can reduce the weight of the structural slab itself, the need for fireproof coating limits the weight reduction to approximately 20% compared to conventional reinforced-concrete slabs with deck plates. Therefore, adopting CLT floor panels is not expected to fundamentally alter the structural design of the building. Accordingly, the building model shown in Figure 11 will be used as is.

According to Japanese Design Recommendations for Composite Constructions [52], the flexural stiffness and flexural strength of the composite beam are determined as follows. First, the effective floor width of the floor panel W_clt,e is calculated using Equations (1) and (2). In these equations, a represents the distance between the edges of the upper flanges of adjacent beams, b is the floor width of the beam, and l is the length of the beam. In the model building shown in Figure 11, the effective floor width W_clt,e becomes 2000 mm for the main beams with a 9 m span and 1275 mm for the secondary beams with a 5.5 m span. AISC Steel Construction Manual [53] gives equivalent but slightly wider effective floor widths, with W_clt,e = 2250 mm for girders and 1375 mm for secondary beams. For calculating the effective width of CLT floor panels connected to timber beams, reference [54] proposes an approximate formula. While those formula are intended for relatively stiff beams with spans exceeding 6 m and are not necessarily applicable in all cases, assuming a Young’s modulus of 6 GPa for the CLT floor panel, a lamina width of 120 mm, and a thickness of 20 mm, the effective floor width W_clt,e is approximately 1100 mm regardless of the beam span. Since no information was found for cases where the beam is made of steel, and the differences in the estimated effective floor widths are not significant, the values calculated using Equation (1) are adopted for design purposes. The width of the CLT floor panel in the test specimen is set to 1800 mm (>1275 mm). This is to enable a re-evaluation of the effective floor width in the CLT floor panel–H-shaped composite beam using high-strength bolts, based on strain gauge measurements attached to the CLT floor panel, as discussed in Section 3.4.

$$W_{clt,e}=2b_a+b$$

(1)

$$\left\{\begin{array}{cc}{b}_a=\left(0.5-0.6a/l\right)a& a<0.5l\\ b_a=0.1l& a\ge 0.5l\end{array}\right.$$

(2)

Next, the stiffness of the composite beam I_cf of a CLT floor panel–H-shaped composite beam is calculated by Equations (3)–(8) based on the Bernoulli–Navier assumption, as illustrated in Figure 12. I_cf, calculated using Equation (3) represents the flexural stiffness of the fully composite beam. In the equation, I_s, A_s, D and E_s represent the second moment of area, cross-sectional area, depth of the steel beam and Young’s modulus of steel, respectively. I_clt, t_clt and E_clt represent the second moment of area, thickness of the CLT slab and Young’s modulus of the CLT panel. The term x_n denotes the neutral axis of the composite beam, as illustrated in Figure 12. In Equations (6) and (7), ε_clt,t represents the strain at the top surface of the slab, while ε_{s_n} and ε_{clt_n} denote the axial strains occurring in the steel beam and the CLT slab, respectively.

$$I_{cf}=I_s+A_s{\left\{x_n-\left(D/2+t_{clt}\right)\right\}}^2+I_{clt}+W_{clt,e}{t}_{clt}{\left\{x_n-t_{clt}/2\right\}}^2$$

(3)

$$x_n={\displaystyle \frac{dn{A}_s+\frac{{t_{clt}}^2}{2}{W}_{clt,e}}{n{A}_s+t_{clt}{W}_{clt,e}}}$$

(4)

$$N_s=N_{clt}$$

(5)

$$N_{clt}=\left(t_{clt}{W}_{clt,e}\right)E_{clt}{\epsilon }_{clt\_n}=\left(t_{clt}{W}_{clt,e}\right)E_{clt}{\epsilon }_{clt,t}{\displaystyle \frac{x_n-\frac{t_{clt}}{2}}{x_n}}$$

(6)

$$N_s=A_s{E}_s{\epsilon }_{s\_n}=A_s{E}_s{\epsilon }_{clt,t}{\displaystyle \frac{d-x_n}{x_n}}$$

(7)

$$n={\displaystyle \frac{E_s}{E_{clt}}}$$

(8)

When the floor panel and the steel beam are not fully connected, slippage occurs at the interface under a certain load, causing the Bernoulli–Navier assumption to no longer hold for the composite beam. As a result, the stiffness of the partially composite beam I_cp is reduced compared to I_cf. Equations (9) and (10) are the formulas adopted in reference [52]. Here, the incremental stiffness resulting from the composite action with the floor panel, compared to the flexural stiffness of the steel beam alone, is calculated by multiplying the difference between the flexural stiffness of the fully composite beam I_cf and that of the steel beam alone I_s by a coefficient α. This approach is based on the method originally adopted by AISC in 1978 [55,56]. The coefficient α is determined as the square root of the ratio of the number of studs n_p connecting the reinforced-concrete slab and the steel beam to the number of studs n_f required for a fully composite beam. To enable application to cases where CLT floor panels are connected using high-strength bolts, α is defined using Equation (11), where Q_f represents the shear force occurring between the steel beam and the CLT floor panels of a fully composite beam when the tensile strain at the bottom of the steel beam ε_s,b (Figure 12) reaches the yield strain. Q_p indicates the yield strength of the connection between the floor panel and the steel beam.

$$I_{cp}=I_s+\alpha \left(I_{cf}-I_s\right)$$

(9)

$$\alpha =\sqrt{{\displaystyle \frac{n_p}{n_f}}}$$

(10)

$$\alpha =\sqrt{{\displaystyle \frac{Q_p}{Q_f}}}$$

(11)

Finally, to determine the bending yield strength of the composite beam, the section modulus of the composite beam is calculated using Equations (12) and (13), where Z_s is the section modulus of the H-shaped steel beam. The section modulus of the fully composite beam, with respect to the bottom of the steel beam, can be calculated using Equation (3) for the fully composite beam, as expressed in Equation (13). The section modulus used to calculate the bending yield strength of a partially composite beam is determined by Equation (12), following a method similar to that used for the flexural stiffness shown in Equation (9).

$$Z_{cp}=Z_s+\alpha \left(Z_{cf}-Z_s\right)$$

(12)

$$Z_{cf}={\displaystyle \frac{I_{cf}}{\left\{x_n-\left(D+t_{clt}\right)\right\}}}$$

(13)

Figure 13 shows the ratio of the composite flexural stiffness and flexural yielding strength to those of the steel beam, with α as the variable, when the CLT floor panel is oriented in the weak-axis direction. The figure illustrates the ratio of the flexural yielding strength of the composite beam to the flexural yielding strength of the steel beam alone, assuming lateral–torsional buckling is fully restrained, as expressed in Equation (14). Here, σ_σs,y represents the yield stress of the steel beam. The effective floor width W_clt,e of the reduced-scale H-section steel beam with CLT floor panels (specimen) were assumed to be equivalent to those of a secondary beam, with an effective floor width of 1275 mm, corresponding to an H350 × 175 × 7 × 11. The Young’s modulus of the steel is E_s = 205,000 N/mm², and for the weak-axis direction of the CLT floor panel, a value of E_clt = 5000 N/mm² was adopted based on reference [40], which used CLT composed of three layers of cedar, the same as the test specimens. From the figure, the flexural stiffness and flexural yielding strength of the composite beam for the assumed secondary beam H350 × 175 × 7 × 11 correspond to those of the scaled test specimen H200 × 100 × 5.5 × 8 when it is equipped with a CLT floor panel of approximately t_clt = 45 to 60 mm. Therefore, in the test specimens used for the four-point bending tests described in Section 3.2, t_clt = 60 mm was set as the standard, with t_clt = 45 mm and t_clt = 75 mm also considered.

$$M_{s,y}=Z_s{\sigma }_{s,y}$$

(14)

The shear force Q_f acting on the interface between the upper flange of the steel beam and the CLT floor panel is calculated by Equations (15) and (16) when the bottom edge of the steel beam reaches its yield stress σ_s,y. Figure 14 illustrates Q_f for a composite beam consisting of a steel beam (H200 × 100 × 5.5 × 8) and CLT floor panels with thicknesses of 45 mm, 60 mm, and 75 mm. The design yield strength of SN400 steel is 235 N/mm² [57]. From Section 2, since the static friction force per high-strength bolt N_f was approximately 5 kN, it is determined that having n_b = 3 rows at least for t_clt = 45 mm, having n_b = 4 or more rows for t_clt = 60 mm and having n_b = 6 rows for t_clt = 75 mm would enable the achievement of a fully composite beam up to the point where the steel reaches its yield strength.

$$Q_f=A_s{\sigma }_{s\_n,y}$$

(15)

$${\sigma }_{s\_n,y}={\sigma }_{s,y}{\displaystyle \frac{\left(D+t_{clt}\right)-x_n}{D/2}}$$

(16)

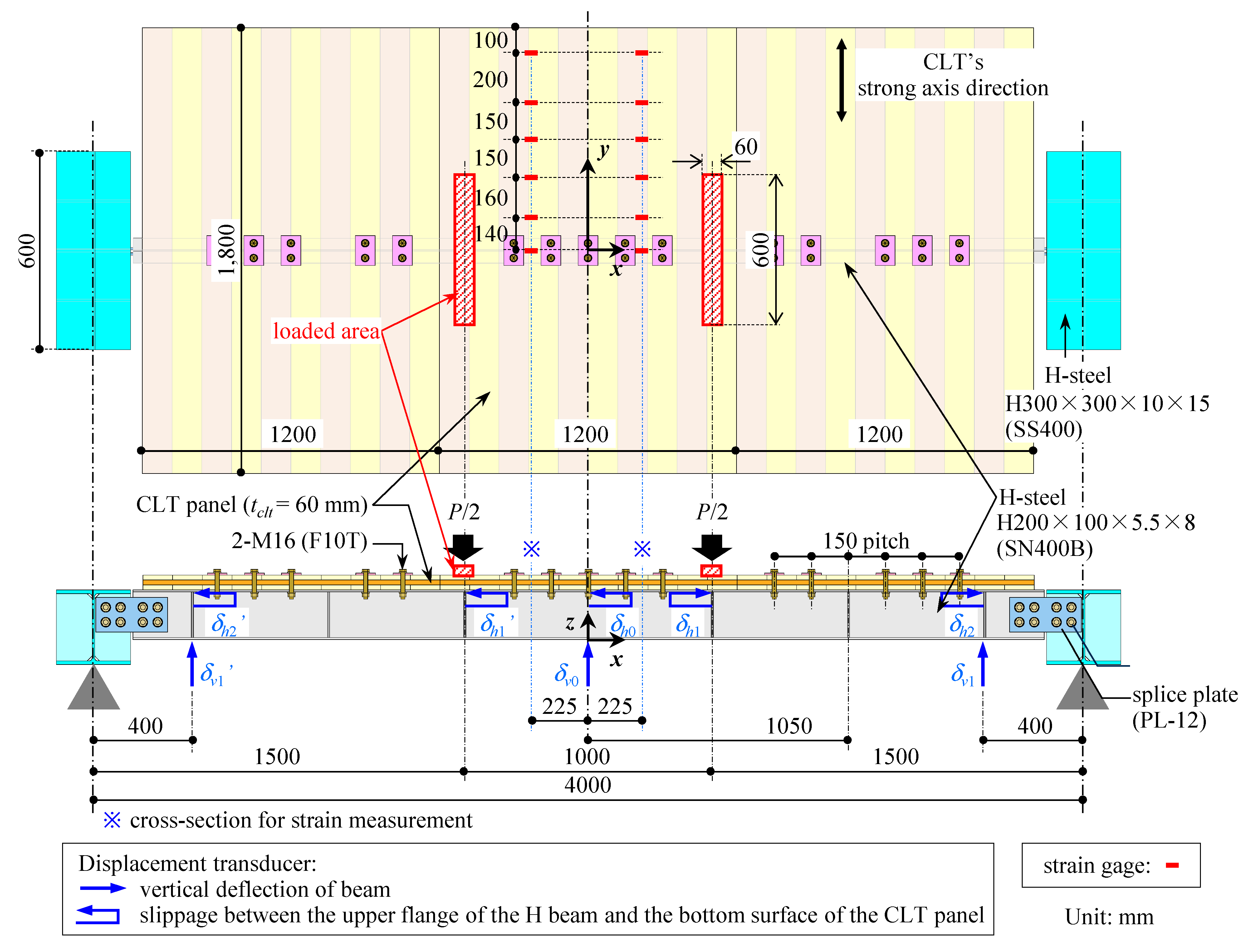
3.2. Test Setup and Test Parameters

Table 3. Specimen list for four-point bending test.

Specimen	Steel Beam	CLT Panel		Bolt Tightening Angle ϕ0
	Section	Section (Material Type)	Thickness (Direction)
H	H200 × 100 × 5.5 × 8 (SN400B)	-	-	-
CLT-H-45W		S60-3-3 (M60B)	45 (weak axis)	240°
CLT-H-60W			60 (weak axis)	300°
CLT-H-75W			75 (weak axis)	360°
CLT-H-60S			60 (strong axis)	300°

shows the parameters of the test specimens for the four-point bending test. The thickness of the CLT floor panel t_clt and the axial direction of the CLT were set as variables. One specimen consisted only of an H-shaped steel beam, while the other four were composite beams with CLT floor panels. The tightening force of the high-strength bolts was controlled by the bolt rotation angle, as shown in Table 3, based on the results from Section 2. Figure 15 illustrates the connection details between CLT floor panels and H-shaped steel beam. The section of the H-shaped steel beam was H200 × 100 × 5.5 × 8 (SN400B [46]). The CLT floor panels used were S60-3-3 (M60B), which had the same specifications and were manufactured simultaneously with the specimens used in the two-surface shear test described in Section 2.

Table 4. Material properties of H-shaped beam.

	Young’s Modulus Es [N/mm2]	Yielding Stress σs,y [N/mm2]
Web	202,300	397
flange	211,800	324

and

Table 5. Young’s modulus of CLT panel.

	Young’s Modulus Eclt,b
	In Bending Eclt,b [N/mm2]	In Compression Eclt,c [N/mm2]
Weak axis	700	2500
Strong axis	6100	4500

summarize material properties of an H-shaped beam, which were obtained from tensile coupon tests, and CLT floor panel, which were obtained from a three-point bending test of CLT floor panels. The Young’s modulus E_clt,c related to the compressive stiffness of the CLT floor panel, shown in Table 5, was estimated from the Young’s modulus E_clt,b related to the flexural stiffness, obtained through the three-point bending test, following the procedure outlined in Figure 16.

Figure 17 shows the test specimen and a schematic diagram of loading conditions for the four-point bending test. The beam span is 4000 mm, and the two-point loads are applied at a distance of 1000 mm apart. Both ends of the beam are connected to perpendicular end members (H300 × 300 × 10 × 15), assumed to represent girders, using M20 high-strength bolts [46] through splice plates. Stiffeners were installed at several locations on the H-shaped steel beam, including directly beneath the loading points, to prevent local deformation during the loading tests. Since the test specimen beam assumes a secondary beam, the CLT floor panels were arranged perpendicular to the H-shaped steel beam, as illustrated in Figure 11. The CLT floor panels measure 1200 mm × 1800 mm. Two high-strength bolts (M16, F10T) per cross-section were arranged with a 150 mm pitch, avoiding stiffeners and loading areas. The reason for adopting a 150 mm pitch is that the relationship between bolt tightening force and static friction force at the interface has not yet been examined for closely spaced bolts. Therefore, even when the CLT floor panel had a thickness of 75 mm, the pitch was chosen to avoid overlap in the influence range of the tightening force of adjacent bolts. Consequently, two high-strength bolts per cross-section were arranged in seven rows on each side symmetrically. Here, the upper surface of the upper flange of the H-shaped steel beam and the steel washer, which form the friction interface with the CLT floor panel, were all pre-rusted before connecting the CLT floor panel.

Figure 18 provides an overall view of the loading device. The end members at both ends are placed on hinged supports, and the load is applied using a jack at the center. The concentrated load from the jack is distributed into two points (spaced 1000 mm apart) through a loading jig and applied to the test specimen via hinge mechanisms. The loading jig is independent of the loading device (weighing 8.76 kN); it was first carefully placed using a chain block before starting the loading process. A 60 mm × 600 mm bearing steel plate was placed on the CLT floor panel directly beneath the hinge points to avoid local bearing failure. The loading area is indicated in red diagonal lines in Figure 17. In reference [54], which evaluates the effective floor width of CLT floor panels in composite beams of CLT floor panels and timber beams, a three-point bending test was conducted with a 2000 mm wide CLT floor panel subjected to a concentrated load at the center. While the loading width may affect the range of bending moments generated in the CLT floor panels, its impact on the composite action between the CLT floor panel and the H-shaped steel beam is considered minimal. The loading was applied in one direction, with the target termination deflection set at δ/L = 1/100 (δ = 40 mm) based on the span L. For the standalone H-steel beam specimen (Specimen H), the load had completely plateaued at δ = 40 mm, so loading was terminated at that point. However, for the CLT–H-steel composite beams, the load continued to increase gradually even at δ = 40 mm, so unloading was performed only after exceeding δ = 50 mm.

Measurement data were collected as follows: The load applied by the central jack was measured using a load cell. The vertical displacements of the beam, measured at the lower flange of the H-shaped steel beam, were recorded at three points with a displacement transducer fixed to the ground, as illustrated in Figure 17 and Figure 18. The relative displacements between the H-shaped steel beam and the CLT floor panel were measured at five points, as illustrated in Figure 17 using displacement transducers. As shown in Figure 19, a displacement transducer was fixed to a magnetic base attached to the upper flange of the H-shaped steel beam, targeting a wooden block fixed to the CLT.

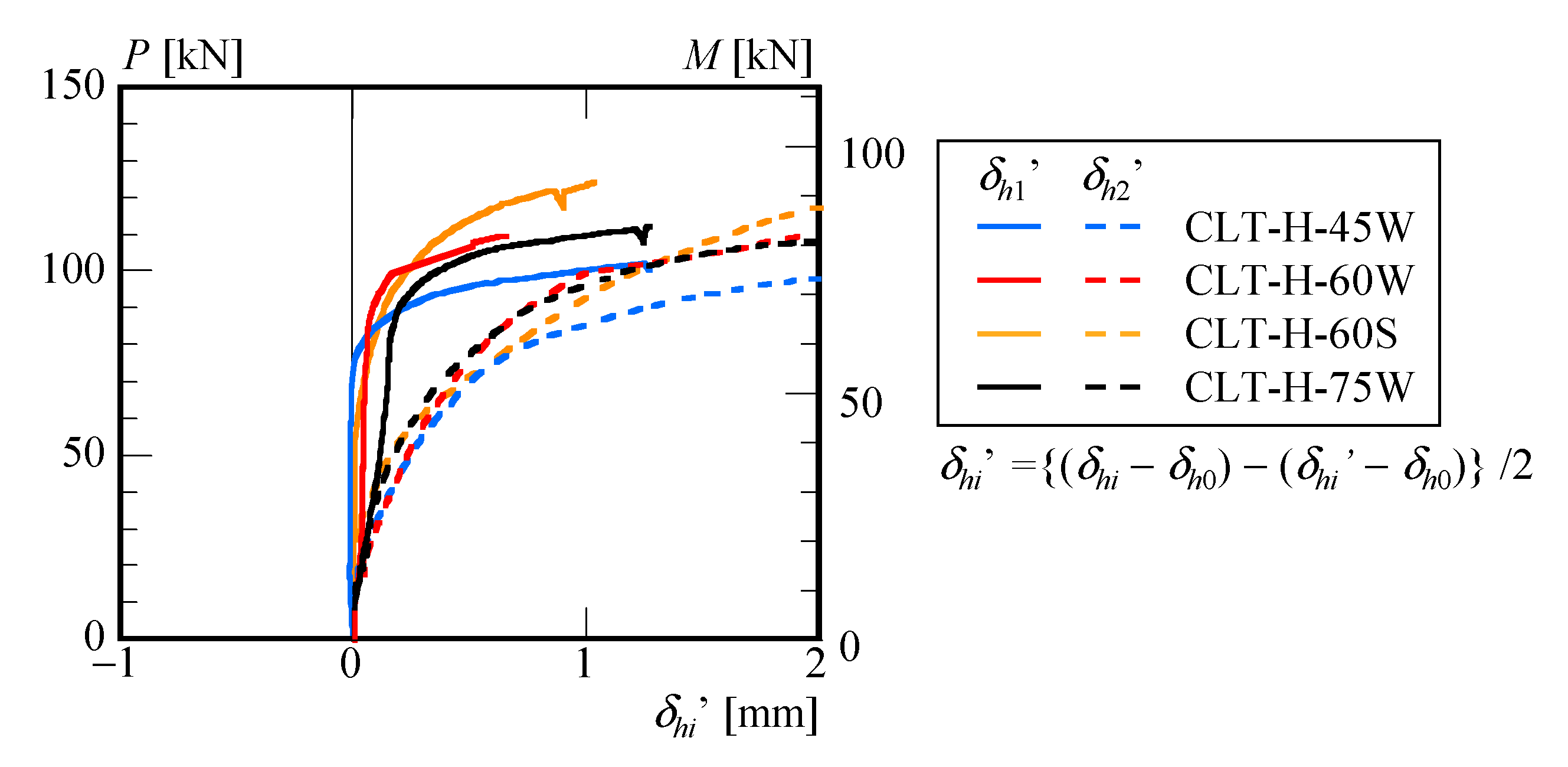
3.3. Test Results of Four-Point Bending Test

Figure 20 shows the relationship between the applied load P (as indicated in Figure 17) and the deflection at the center δ of the H-shaped steel beam for all test specimens. The right-side axis of the figure represents the acting bending moment M on the test section. Here, the central deflection δ is determined as the incremental value of δ_v0 measured at the center relative to the average of δ_v1 and δ_v1′, which were measured at both ends, as shown in Figure 17. The figure indicates P, corresponding to the yield moment M_s,y and the fully plastic moment M_s,p of the H-shaped steel beam under the assumption of no lateral–torsional buckling, using horizontal dashed and solid lines, respectively. In the figure, the inverted triangle indicates the point at which the strain value measured at the bottom flange of the H-shaped steel beam for each test specimen reached the yield strain. The yield strain was calculated by dividing the yield strength of the H-shaped steel σ_s,y by its Young’s modulus E_s, both of which are given in Table 4. From Figure 20, it can be observed that fixing the CLT floor panels to the H-shaped steel beam using high-strength bolts improved both the initial stiffness and flexural yielding strength. The effect of CLT panel thickness (t_clt = 45, 60, 75 mm) on the initial stiffness was not significant, with an approximate 20% increase compared to the test specimen consisting of only the H-shaped steel beam. On the other hand, changing the principal direction of the CLT panels from the weak axis to the strong axis resulted in an almost 40% increase in initial stiffness. Focusing on the yield points indicated by the inverted triangles for each test specimen, the flexural yielding strength exhibited a trend similar to that of the initial stiffness. Figure 21 shows the deflection shape of Specimen CLT-H-60S at δ = 40 mm.

Figure 22 shows the progression of slippage between the CLT floor panel and the H-shaped steel beam with respect to the applied load P. As shown in Figure 17, displacement transducers were symmetrically arranged to measure the slippage. The horizontal axis values, δ_h1′ and δ_h2′, were calculated by averaging the measurements from transducers positioned equidistantly from the center and expressing them as increments from the central measurement. From the figure, δ_h2′, measured outside the bolt positions, exhibited slippage from the very beginning of loading. In contrast, δ_h1′ remained nearly zero under low loads. However, as the bottom flange of the H-shaped steel beam approached yielding, δ_h1′ also began to increase, showing a tendency for slippage displacement to progress at loads similar to those corresponding to yielding.

Figure 23 shows the strain distribution measured on the upper and lower surfaces of the CLT floor panels and the H-shaped steel beam at P = 20, 40, 80 kN. The vertical dashed lines indicate the yield strain of the H-shaped steel beam flanges. The yield strain ε_s,y was calculated by dividing the yield strength σ_s,y by the Young’s modulus E_s, defined in Table 4. The strains were measured at the cross-section indicated by an asterisk (※) in Figure 17, and the values represent the averages of measurements taken at the same height. In the figure, the strain on the bottom surface of the CLT directly above the H-shaped steel beam could not be measured. Therefore, the strain values measured on the top and bottom surfaces of the CLT floor panel at y = 140 mm (Figure 17) are indicated with dashed lines with hollow plots. (a) In the case of the H-shaped steel beam alone, it can be observed that the strain increases while maintaining a neutral axis at the centroidal height of the H-shaped steel (z = 100 mm). (b–d) When the CLT floor panels are installed, the position of the neutral axis, as inferred from the strain distribution, shifts by approximately 10–20 mm towards the CLT side. This indicates that tensile forces are generated in the H-shaped steel beam as a result of its composite action with the CLT floor panels. From the figure, it can be observed that the strain distribution of the composite beam does not strictly adhere to the Bernoulli–Navier assumption. In the method where studs welded to the H-shaped steel beam are fixed to the CLT with epoxy (Figure 2d), it has been reported that the assumption of plane sections remaining plane holds relatively well within the elastic range [41]. On the other hand, when screws or bolts are used for fixation, it has been reported that, as shown in Figure 23, the strain generated in the CLT tends to remain smaller than when the assumption of plane sections is applied [29,38].

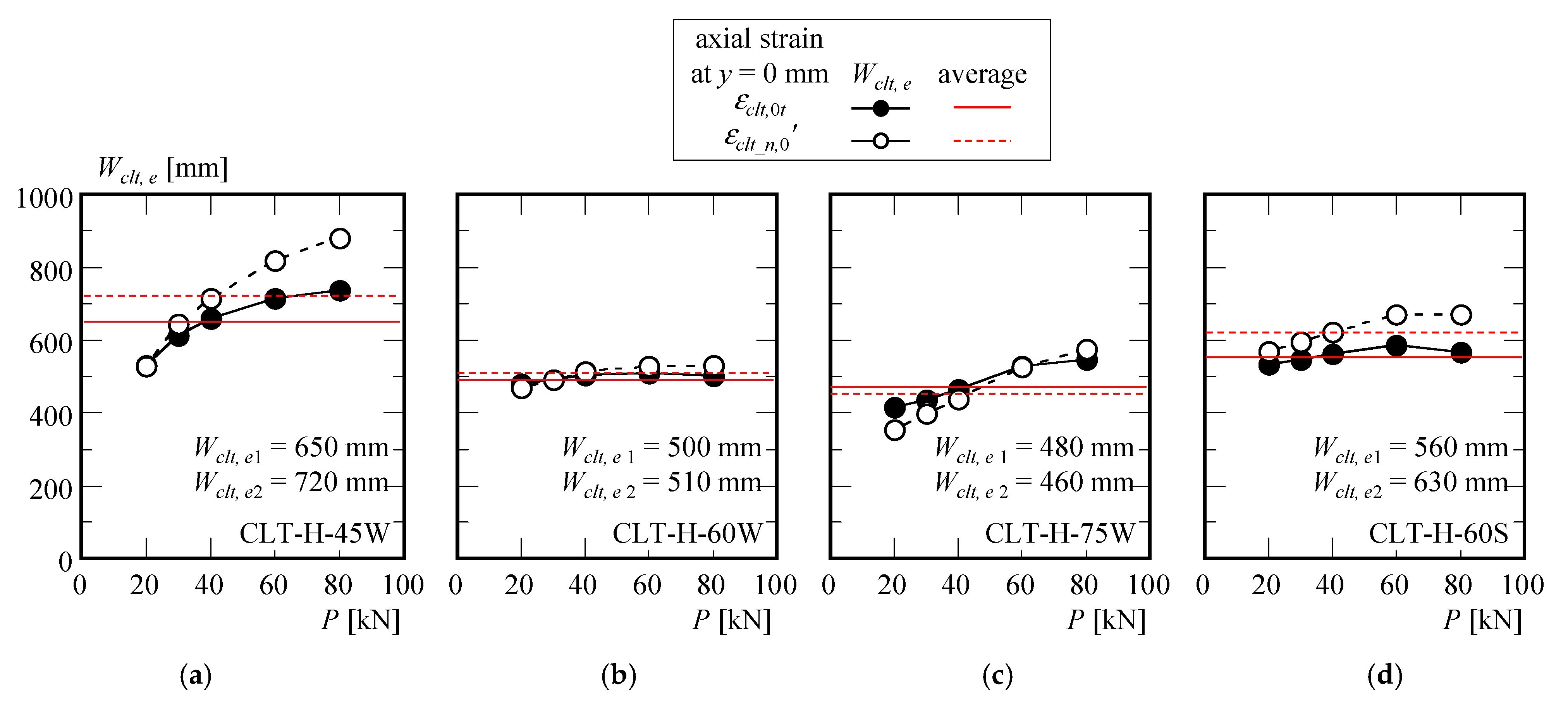
3.4. Effective CLT Slab Width

The effective width of the CLT floor panel in the composite beam consisting of the CLT floor panel and the H-shaped steel beam, joined by high-strength bolts, is estimated using the lateral distribution of strain values measured on the CLT floor panel, as shown in Figure 24. The y-axis in the figure is the axis in the CLT width direction, as defined in Figure 17. In the figure, the black plots represent the average strain measured on the upper and lower surfaces (ε_clt,it and ε_clt,ib), as expressed by Equation (17) where i denotes the location of a strain gauge, indicating the axial strain of the CLT floor panel ε_{clt_n}. At y = 0 mm (where the xyz-axis is defined in Figure 17), strain measurements were not conducted because the bottom surface of the CLT panel was joined to the upper flange of the H-shaped steel beam. In Figure 24, the black plots at y = 0 mm represent the strain measured on the upper surface of the CLT panel ε_clt,0t. The hollow plots represent the estimated axial strain ε_{clt_n0}′ using the difference between the upper and lower strains at y = 140 mm, calculated by Equations (18) and (19). The light blue shaded region in the figure will be explained later.

$${\epsilon }_{clt\_n,i}=\left({\epsilon }_{clt,it}+{\epsilon }_{clt,ib}\right)/2$$

(17)

$${\epsilon }_{clt\_n,0}\prime =\left({\epsilon }_{clt,0t}+{\epsilon }_{clt,0b}\prime \right)/2$$

(18)

$${\epsilon }_{clt,0b}\prime ={\epsilon }_{clt,0t}+\left({\epsilon }_{clt,1b}-{\epsilon }_{clt,1t}\right)$$

(19)

Figure 25 shows the distribution of axial strain measured in the H-shaped composite beam member with CLT floor panels at p = 20, 40, 80 kN. Here, since strains were measured both at x = ±225 mm (Figure 17), those values at the same y-coordinate are averaged. From the figure, in the specimens where the direction of the CLT floor panel aligned with the weak axis, the axial strain exhibited a relatively linear distribution toward the edge of the CLT panel for a panel thickness of t_clt = 45 mm. In contrast, for thicknesses of t_clt = 60 mm and 75 mm, the axial strain tended to decrease significantly as soon as the panel moved away from the H-shaped steel beam. When the CLT floor panel was aligned with the strong axis, a relatively linear distribution of axial strain was observed even for a panel thickness of t_clt = 60 mm.

Figure 26 shows the effective floor width estimated from the strain distribution of the CLT floor panel at P = 20, 30, 40, 60 and 80 kN. As explained in Figure 24, the effective floor width W_clt,e is estimated using Equation (20) as the width equivalent to the area of axial strain occurring in the CLT floor panel (the light blue shaded region in Figure 24), assuming that the axial strain occurring directly above the H-shaped steel beam remains constant across the effective floor width (the area enclosed by the red dashed line). As the axial strain occurring directly above the H-shaped steel beam, ε_clt,0t and ε_{clt_n0}′ are selected. From the figure, except for (b), the effective floor width showed a tendency to increase with the applied load p. Therefore, the average effective floor widths, W_clt,e1 (solid red line) and W_clt,e2 (dashed red line), are shown as the averages of the values for P ≤ 80 kN, which can be considered within the elastic range. In this test specimen, the effective floor width measured for the CLT floor panels ranged between 500 and 700 mm. This is approximately half of the 1275 mm value derived in Section 3.1 based on the referenced literature.

$$W_{clt,e}=2{\displaystyle \frac{{\displaystyle {\sum }_0^5{\epsilon }_{clt\_n,i}{w}_i}}{{\epsilon }_{clt\_n,0}}}$$

(20)

3.5. Prediction of Composite Stiffness and Strength

In this section, the flexural stiffness I_cp of the composite beam is estimated using Equation (9). In Section 3.1, for design purposes, the yield strength of the steel material was assumed to be σ_s,y = 235 N/mm². Under this assumption, the required number of rows of high-strength bolts for a fully composite beam was n_b =3 for t_clt = 45 mm, n_b = 4~5 for t_clt = 45 mm, and n_b = 6 for t_clt = 75 mm. However, since the actual yield strength of the steel material was σ_s,y = 324 N/mm² (Table 4), a fully composite beam was not achieved in any of the test specimens until the lower flange of the H-shaped steel beam yielded. As shown in Figure 17, the number of bolts bearing the shear force at the interface between the CLT floor panel and the H-shaped steel beam differs between the edges and the center of the region where the bending moment remains constant, with n_b =5 rows at the edges and n_b = 8 rows at the center. Therefore, in this study, the value of α in Equation (9) was calculated for both cases, and the average value was adopted. Figure 27 shows the rate of increase in the flexural stiffness of the composite beam using material properties listed in Table 4 and Table 5 and the static friction force obtained from Figure 9, where the horizontal axis represents the effective floor width W_clt,e. The red arrows in the figure illustrates the rate of increase in flexural stiffness due to composite action for each case: t_clt = 45 mm (weak axis), t_clt = 60 mm (weak axis), t_clt = 75 mm (weak axis), and t_clt = 60 mm (strong axis), assuming effective floor widths of 700 mm, 500 mm, 475 mm, and 600 mm, respectively.

Figure 28 shows the load–vertical displacement relationships for the CLT floor panel and H-shaped composite beam joined with high-strength friction bolts, with the estimated initial stiffness of the composite beam represented by black dashed lines. The figures also include the experimental results for the reference case of the H-shaped steel beam alone. Additionally, the yield strength of the composite beam, calculated using Equation (12), is shown as horizontal dashed lines. From the figure, the initial stiffness of the composite beam corresponds well with the evaluated value obtained using Equation (9). Figure 29 summarizes the experimental results for the initial stiffness and yield bending moment of the five specimens using bar graphs, while the estimated values for each specimen based on Equations (9) and (12) are represented by horizontal lines. Here, the initial stiffness was calculated using the least squares method within the range of P = 20–60 kN for Specimen H and P = 20–70 kN for the specimens with CLT floor slabs. From the figure, it can be concluded that the flexural rigidity and flexural strength of a partially composite beam, as used in Equations (9) and (12), can be reasonably well evaluated, provided that the static friction force at the interface and the effective width of the CLT slab are given.

4. Conclusions

With the urgent need to reduce GHG emissions in the building and construction industry, it is essential to propose structural systems that balance seismic performance and environmental considerations, particularly in earthquake-prone countries like Japan. In response, this study focuses on steel structures, known for their high strength, ductility, and prefabricated construction, and explores the development of a hybrid system using detachable CLT floor panels as an alternative to conventional concrete floor slabs, which pose challenges in terms of reuse. High-strength bolt friction joints were employed for the connections, introducing initial tension to achieve a compressive connection between the CLT floor panels and H-shaped steel beams. The main findings obtained from this study are as follows.

(1)

From the results of the double-shear tests conducted on CLT panels and steel plates connected using high-strength bolt friction joints, it was observed that when using a 50 mm × 80 mm × 10 mm steel washer, the static friction force N_f was approximately 5 kN when the axial force causing bearing yield of the CLT panel was applied to the bolt.

(2)

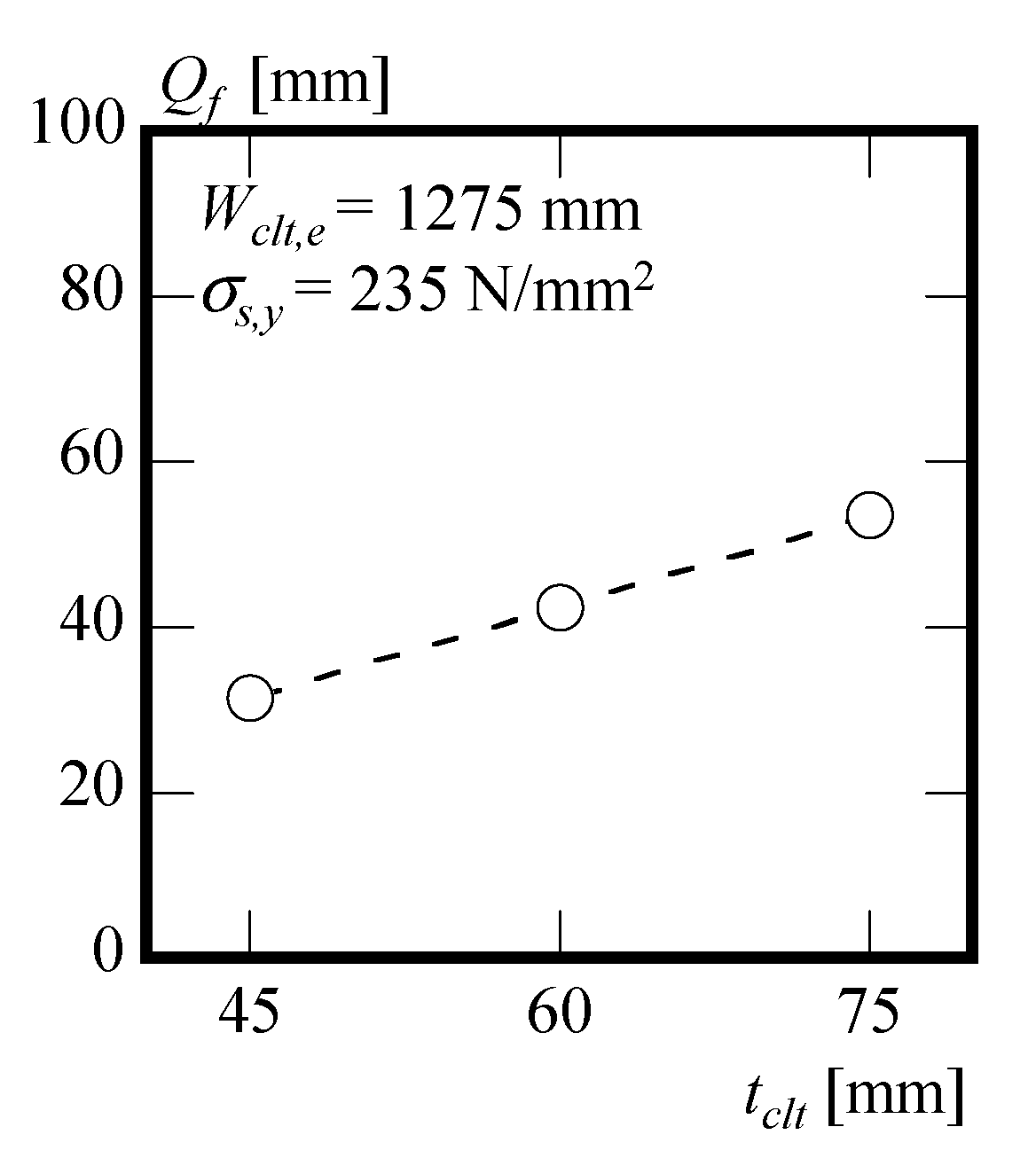
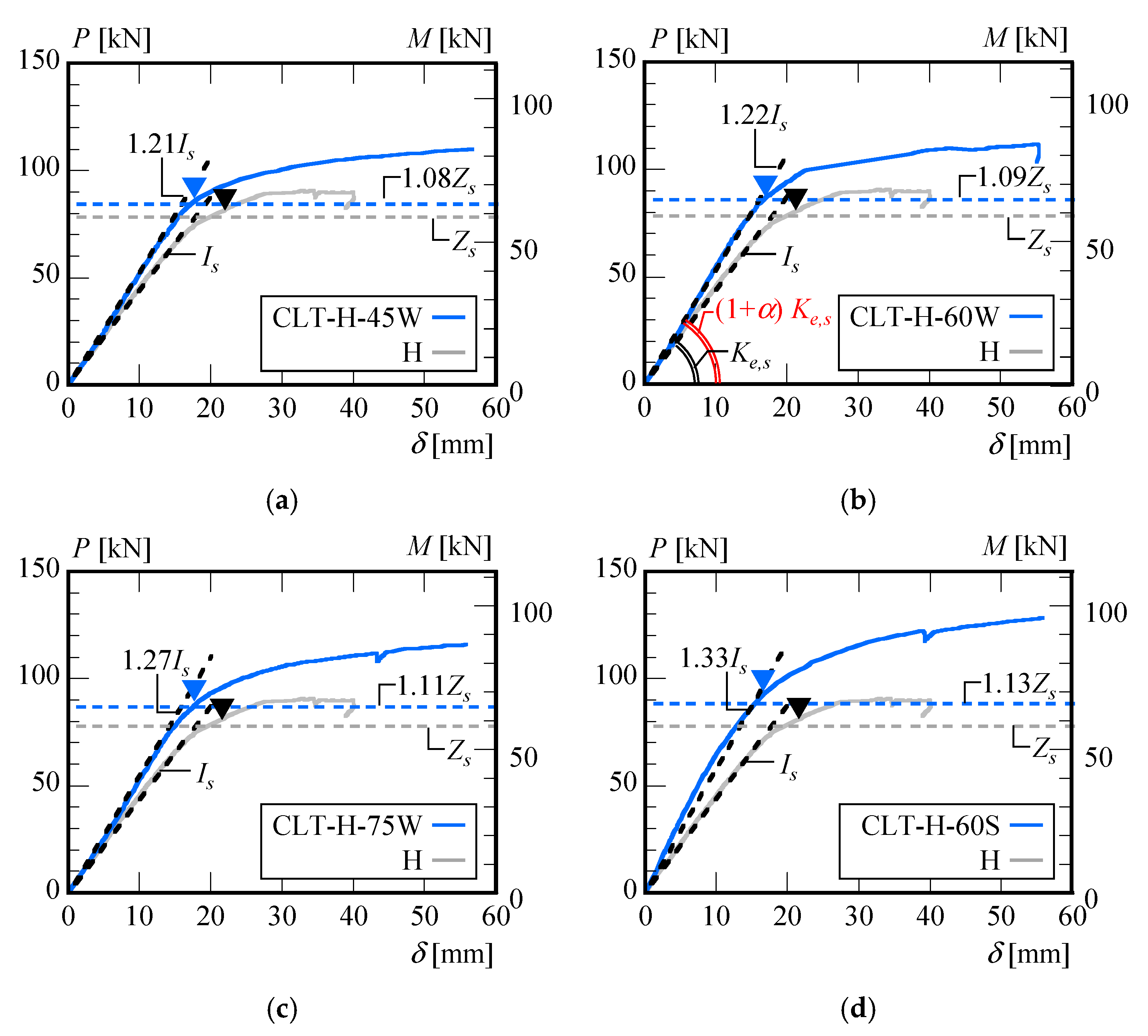
It was confirmed through four-point bending tests that when CLT floor panels with thicknesses of t_clt = 45, 60, and 75 mm were fixed to an H-shaped steel beam (H200 × 100 × 5.5 × 8) in the weak-axis direction, the initial flexural stiffness improved by 20% due to the composite effect. Similarly, when a t_clt = 60 mm CLT floor panel was fixed in the strong-axis direction, the initial flexural stiffness improved by 40% compared to the H-shaped steel beam alone.

(3)

In the four-point bending tests, it was found that the effective width of the CLT floor panel connected to the composite beam using high-strength bolt friction joints was approximately 500 to 700 mm.

(4)

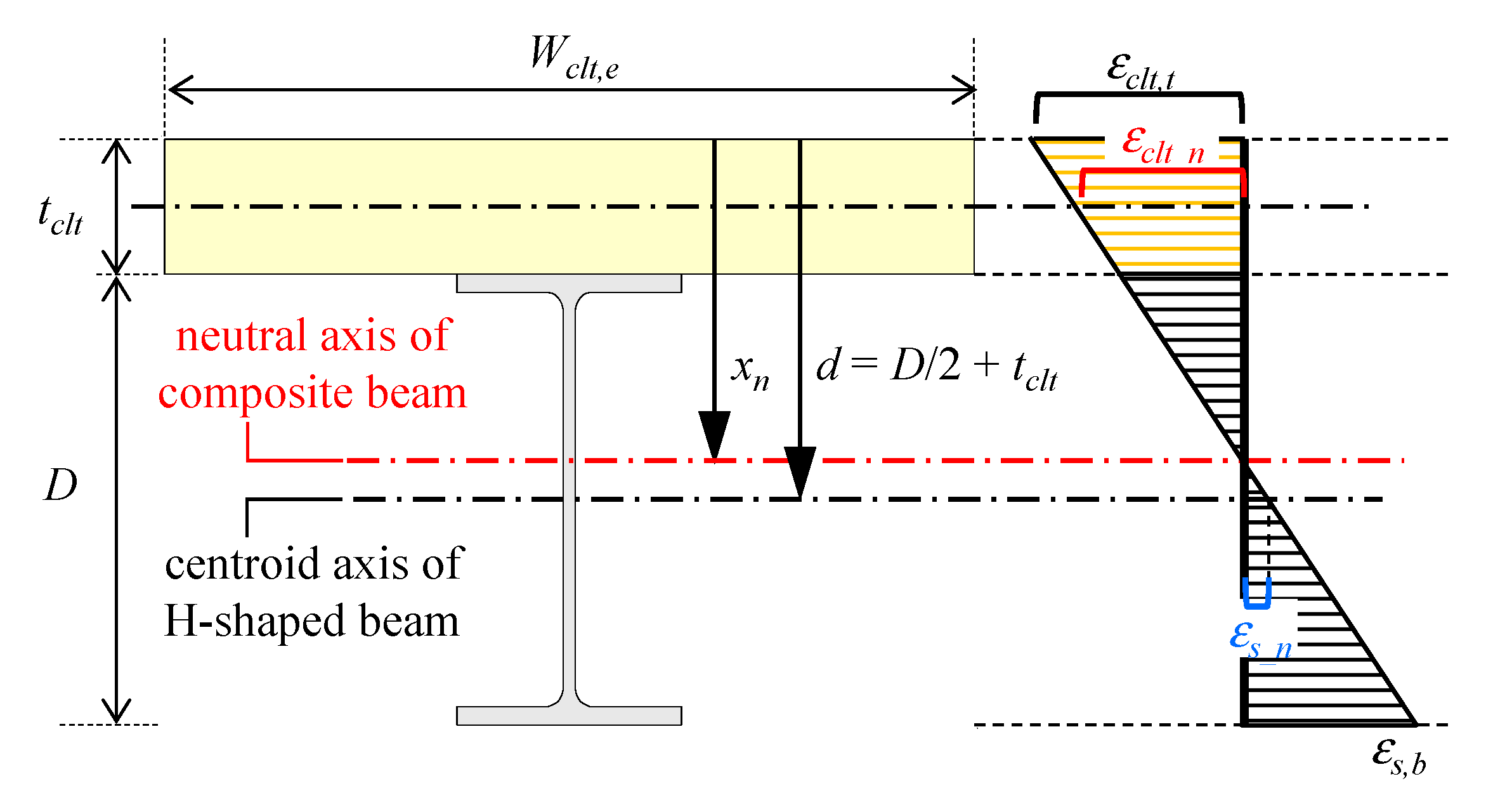
Using the static friction force of the high-strength bolt friction joint obtained in (1), the effective width of the CLT floor panel estimated in (3), and the material properties, it was demonstrated that the initial flexural stiffness and yield bending moment can be estimated by applying the plane-section assumption to the composite beam cross-section, as described in Figure 12 and Equations (3), (9) and (12).

It should be noted that the findings of this study are limited to the experimental conditions used. However, in this paper, unlike References [27,29,31,34,38,40], four-point bending tests were conducted using specimens without restrictions on the effective width of the CLT to clarify the flexural rigidity and yield bending moment of composite beams consisting of CLT floor panels and H-shaped steel beams. Furthermore, by presenting a simplified method for evaluating the relationship between static friction force at the CLT–steel interface and the bending stiffness and yield bending strength of composite beams, this study provides a basis for generalizing the required number of bolts needed to achieve the composite effect.

To reduce GHG emissions in the construction industry and transition toward environmentally friendly structures, it is essential to develop structural systems that enable these goals and establish appropriate design methodologies. However, achieving these objectives still presents several challenges. In the future, to promote the adoption of the connection method using high-strength bolt friction joints for CLT floor panels and H-shaped steel beams in building frames, the following investigations must be continued.

(i)

Clarification of the relationship between optimal bolt axial preload and static friction force, considering the number of bolts and their spacing, as well as the effects of environmental factors and aging on the static friction force at the CLT–steel interface. This includes examining the influence of temperature, humidity, usage conditions, CLT thickness, stiffness, fiber direction, and thermal expansion on both structural performance and building serviceability.

(ii)

Determination of the effective width of the CLT floor panels at the full-scale cross-section level.

(iii)

Evaluation of the serviceability and seismic performance of CLT floor panel–H-steel composite beams, including the applicability range of the simplified evaluation method for bending stiffness and yield bending strength based on Equations (3)–(13) and the assessment of their ultimate strength.

(iv)

Analysis of the seismic response characteristics of a building due to the use of low-axial-stiffness CLT floor panels.

(v)

Assessment of the advantages of steel structures incorporating CLT floor panels from both environmental and disaster resilience perspectives, including the estimation of total costs from construction to demolition. The assessment should also consider the environmental impact of the adhesives used in CLT panels [57].