Degradation of Kinematic PPP of GNSS Stations in Central Europe Caused by Medium-Scale Traveling Ionospheric Disturbances During the St. Patrick’s Day 2015 Geomagnetic Storm
Abstract
1. Introduction
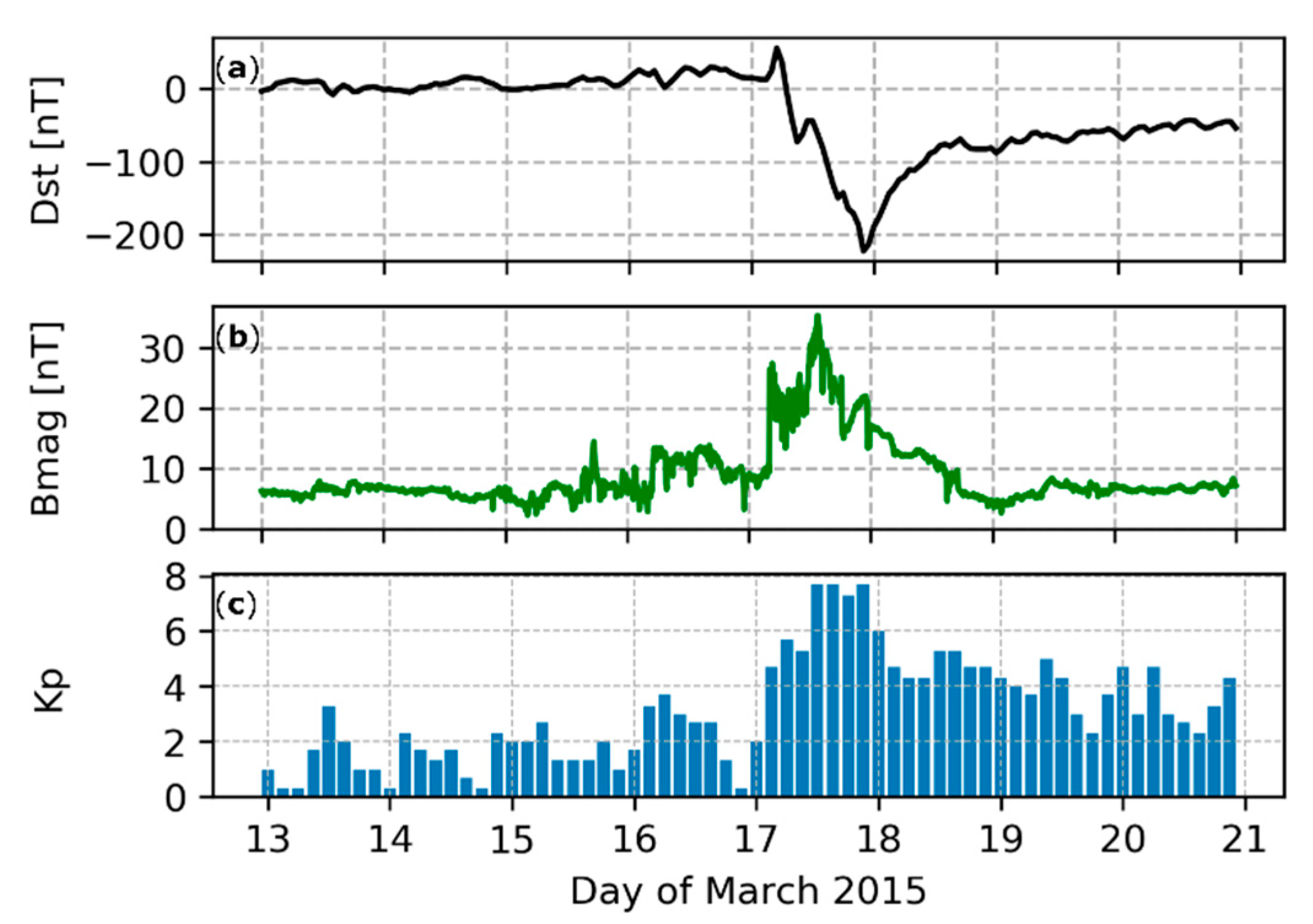
2. The St. Patrick’s Day 2015 Geomagnetic Storm
3. Methodology and Data
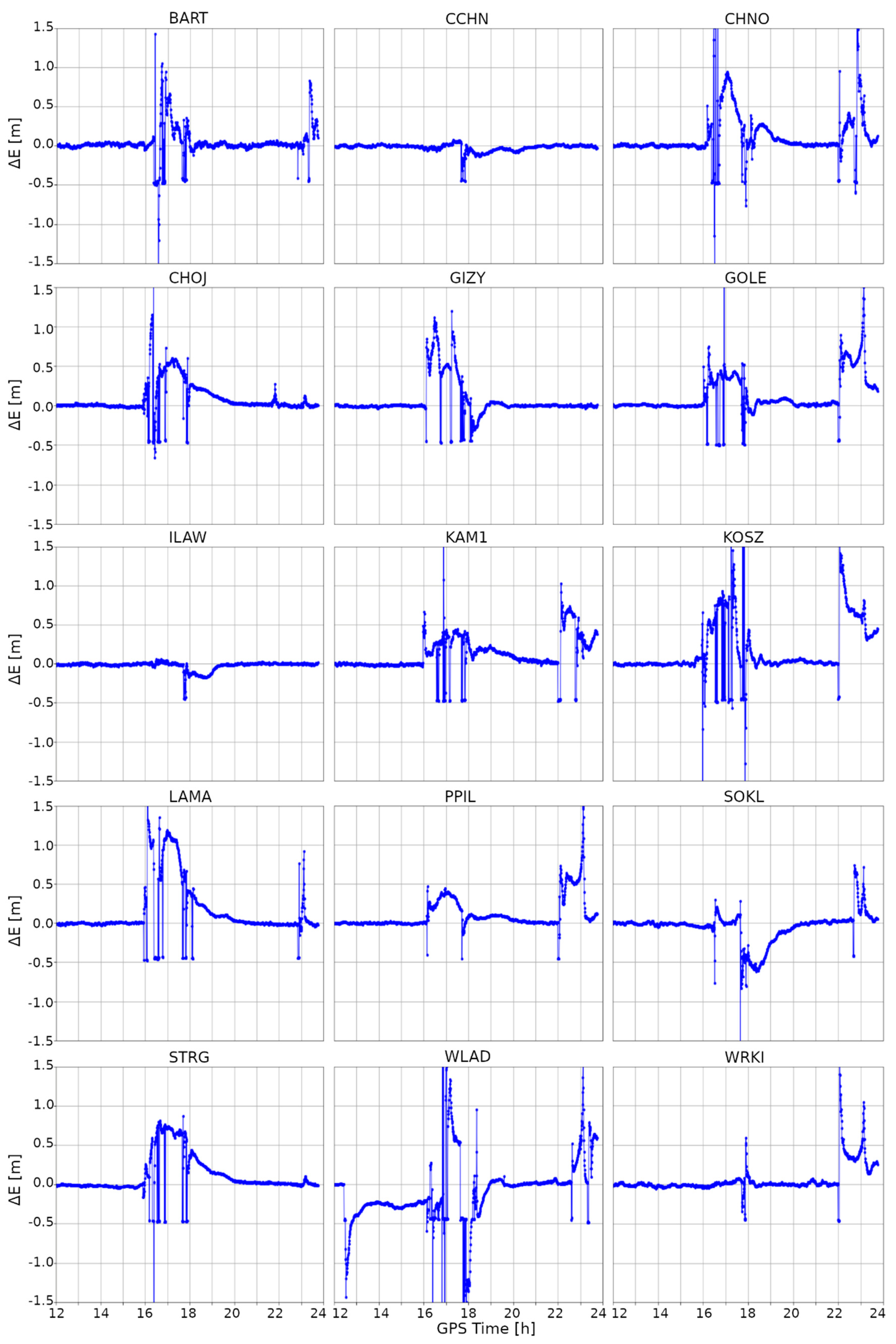
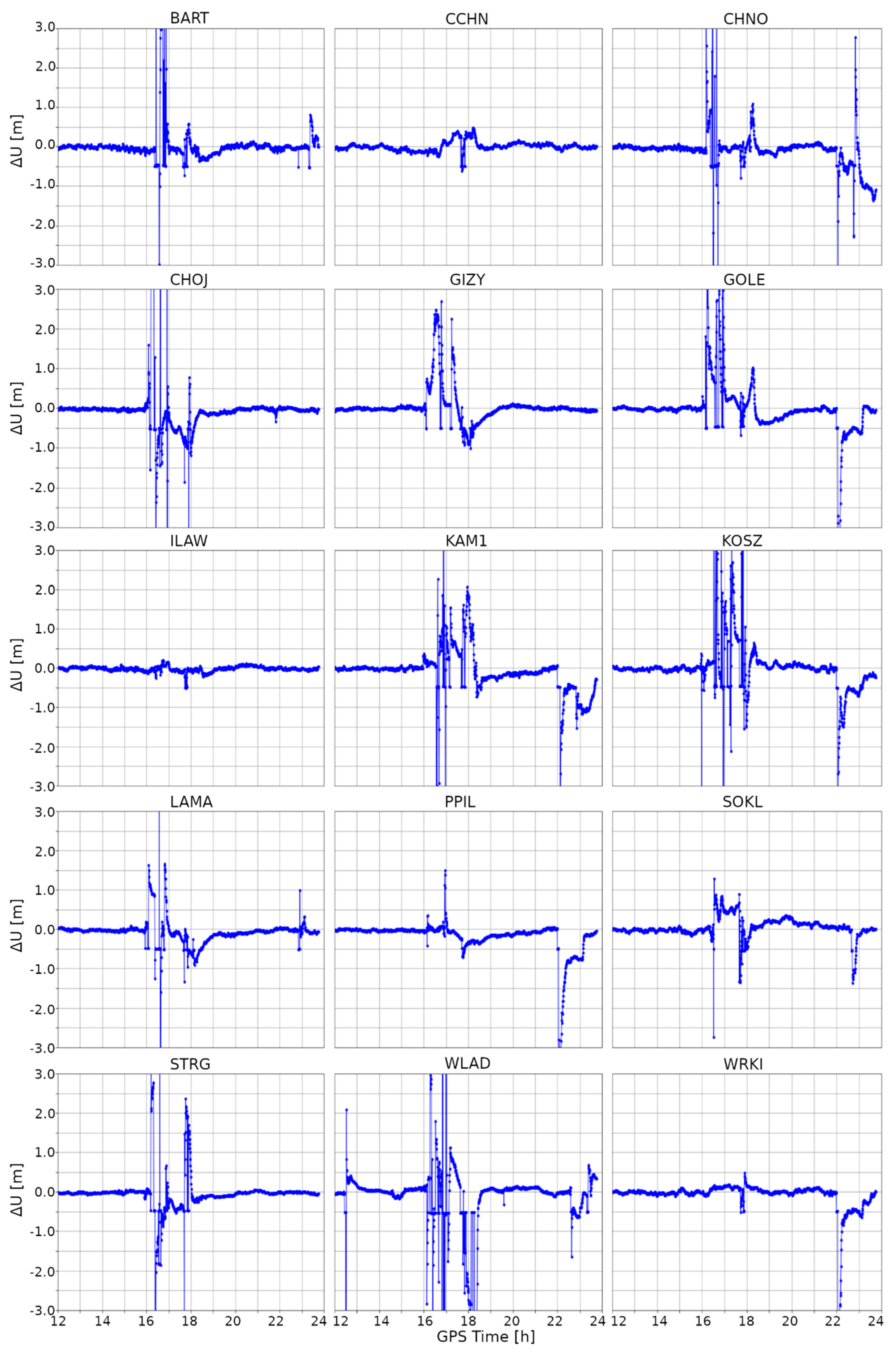
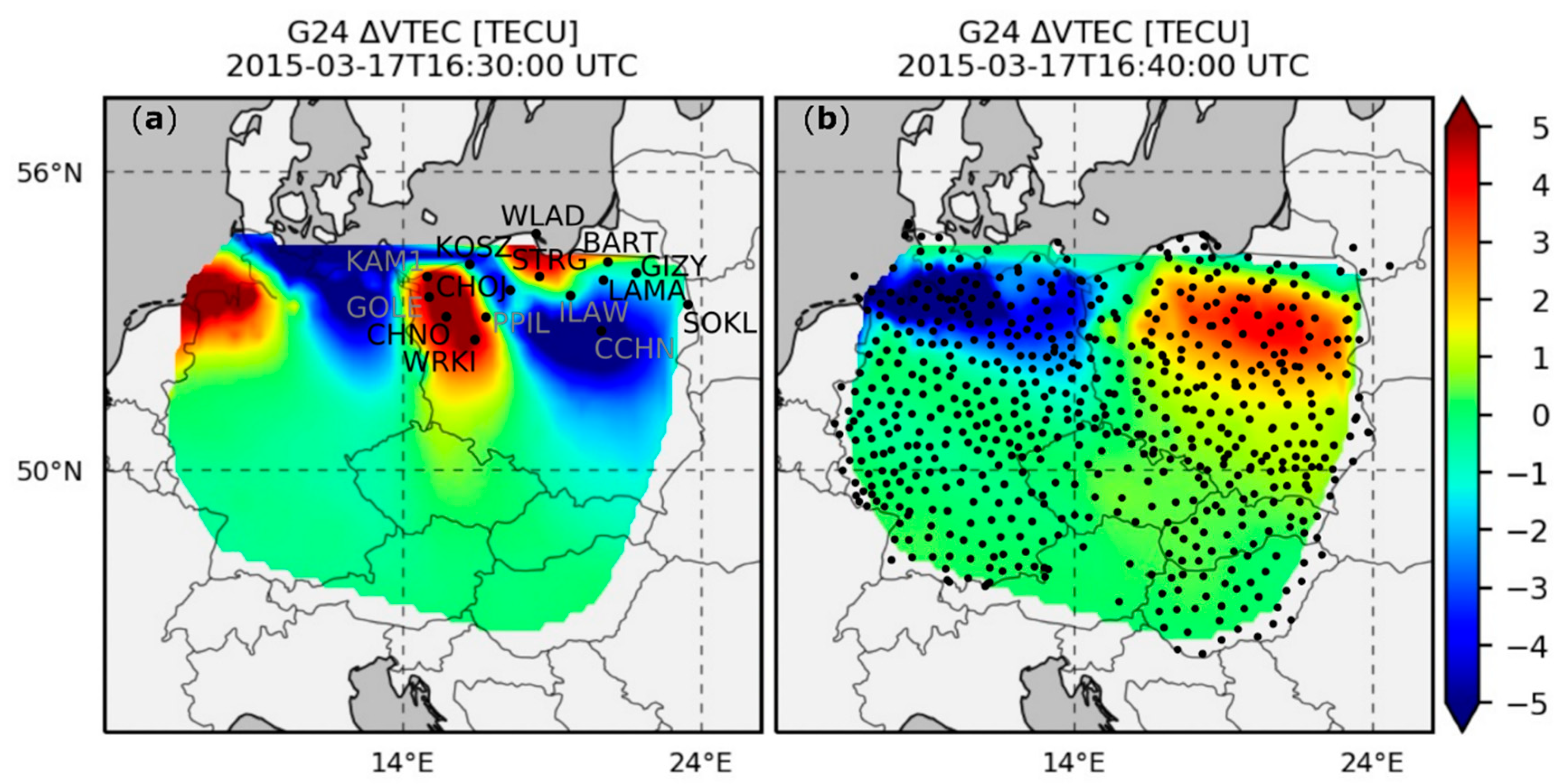
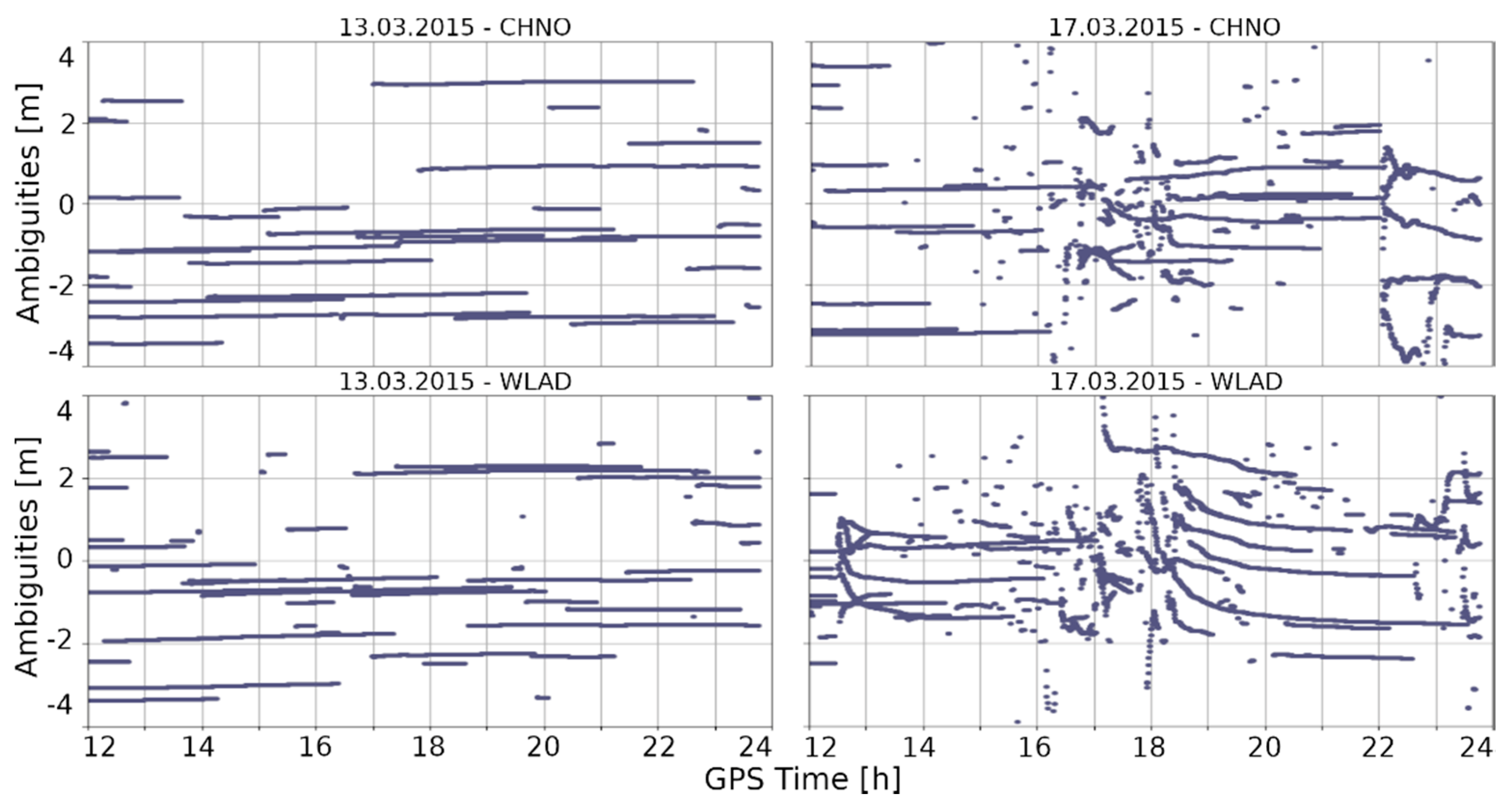
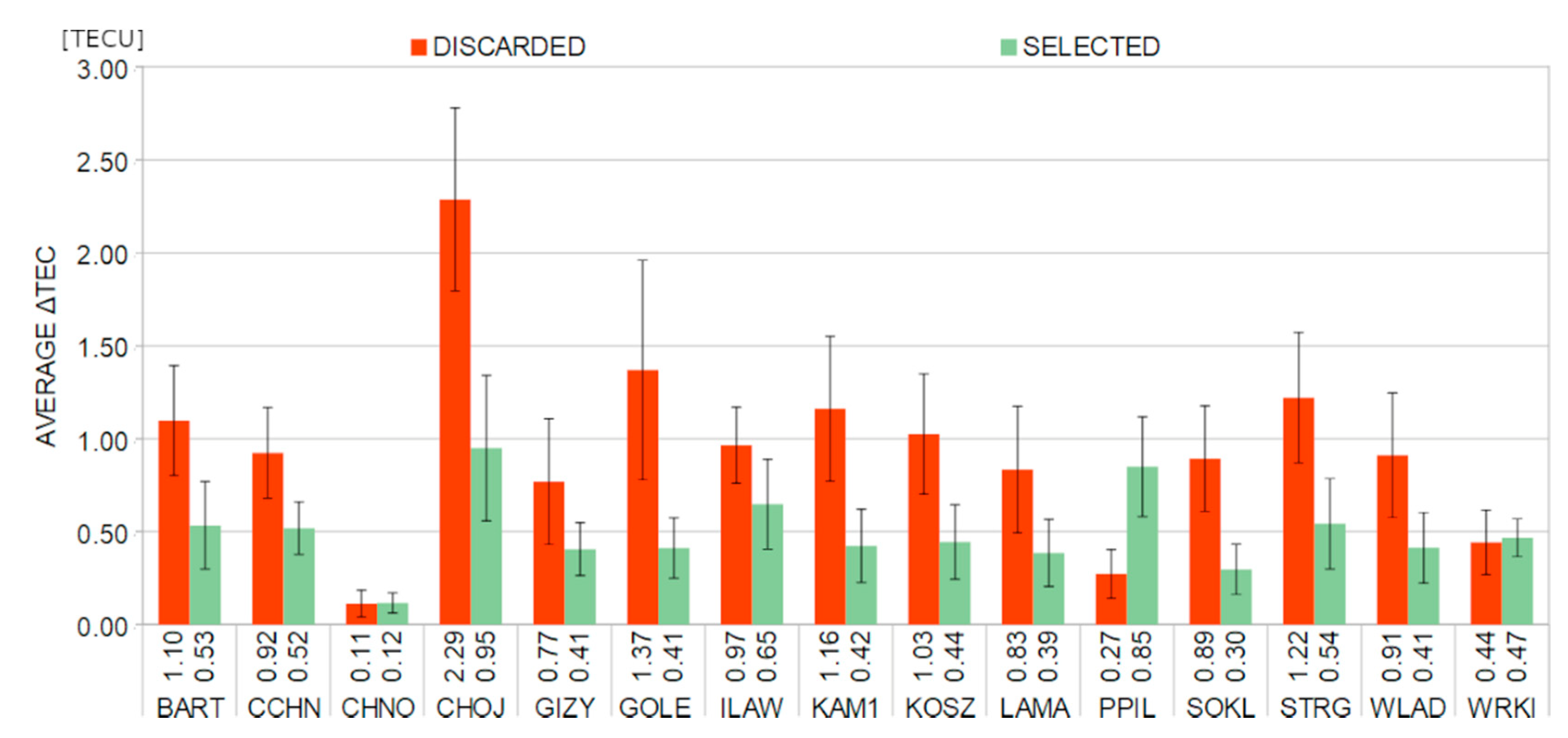
4. Results
5. Discussion
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A
| Station Name | Latitude | Longitude | Altitude (m) |
|---|---|---|---|
| BART | 54°15″02.52′ | 20°48″55.57′ | 93.2 |
| CCHN | 52°52″58.03′ | 20°35″52.18′ | 167.7 |
| CHNO | 53°09″50.58′ | 15°24″50.54′ | 105.4 |
| CHOJ | 53°41″42.65′ | 17°33″08.47′ | 204.1 |
| GIZY | 54°02″08.81′ | 20°10″02.53′ | 166.8 |
| GOLE | 53°33″31.36′ | 14°50″16.81′ | 68.8 |
| ILAW | 53°35″13.64′ | 19°34″04.66′ | 162.8 |
| KAM1 | 53°57″47.01′ | 14°46″38.61′ | 57.8 |
| KOSZ | 54°12″12.19′ | 16°11″51.79′ | 123.2 |
| LAMA | 53°53″32.63′ | 20°40″11.77′ | 187.0 |
| PPIL | 53°09″25.11′ | 16°44″17.73′ | 121.7 |
| SOKL | 53°24″30.75′ | 23°29″44.66′ | 222.2 |
| STRG | 53°57″58.70′ | 18°31″47.96′ | 136.3 |
| WLAD | 54°47″48.32′ | 18°25″07.49′ | 34.7 |
| WRKI | 52°42″19.98′ | 16°22″15.87′ | 95.3 |

References
- Klobuchar, J.A. Ionospheric Time-Delay Algorithm for Single-Frequency GPS Users. IEEE Trans. Aerosp. Electron. Syst. 1987, 3, 325–331. [Google Scholar] [CrossRef]
- European Commission. European GNSS (Galileo) Open Service—Ionospheric Correction Algorithm for Galileo Single Frequency Users; European Commission: Brussels, Belgium, 2016; Issue 1.2. [Google Scholar]
- Wang, N.; Zishen, L.; Li, M.; Yuan, Y.; Huo, X. GPS, BDS and Galileo ionospheric correction models: An evaluation in range delay and position domain. J. Atmospheric Solar-Terrestrial Phys. 2018, 170, 83–91. [Google Scholar] [CrossRef]
- Kaplan, E.D.; Hegarty, C.J. Characterisation of GNSS Space Service Volume. In Understanding GPS: Principles and Applications, 2nd ed.; Artech House Inc.: London, UK, 2006. [Google Scholar]
- Wielgosz, P.; Kashani, I.; Grejner-Brzezinska, D. Analysis of long-range network RTK during a severe ionospheric storm. J. Geod. 2005, 79, 524–531. [Google Scholar] [CrossRef]
- Hernández-Pajares, M.; Juan, J.M.; Sanz, J.; Orús, R. Second-order ionospheric term in GPS: Implementation and impact on geodetic estimates. J. Geophys. Res. 2007, 112, B08417. [Google Scholar] [CrossRef]
- Kouba, J.; Héroux, P. Precise point positioning using IGS orbit and clock products. GPS Solutions 2001, 5, 12–28. [Google Scholar] [CrossRef]
- Ge, M.; Gendt, G.; Rothacher, M.; Shi, C.; Liu, J. Resolution of GPS carrier-phase ambiguities in Precise Point Positioning (PPP) with daily observations. J. Geod. 2008. [Google Scholar] [CrossRef]
- Veettil, S.V.; Aquino, M.; Marques, H.A.; Moraes, A. Mitigation of ionospheric scintillation effects on GNSS precise point positioning (PPP) at low latitudes. J. Geod. 2020, 94, 15. [Google Scholar] [CrossRef]
- Marques, H.A.; Marques, H.A.S.; Aquino, M.; Veettil, S.V.; Monico, J.F.G. Accuracy assessment of Precise Point Positioning with multi-constellation GNSS data under ionospheric scintillation effects. J. Space Weather. Space Clim. 2018, 8, A15. [Google Scholar] [CrossRef]
- Crowley, G.; Azeem, I. Extreme Ionospheric Storms and Their Effects on GPS Systems. Extreme Events Geospace 2018, 555–586. [Google Scholar] [CrossRef]
- Weng, D.; Shengyue, J.; Wu, C.; Zhizhao, L. Assessment and Mitigation of Ionospheric Disturbance Effects on GPS Accuracy and Integrity. J. Navig. 2014, 67, 371–384. [Google Scholar] [CrossRef][Green Version]
- Crowley, G.; Azeem, I.; Reynolds, A.; Duly, T.M.; McBride, P.; Winkler, C.; Hunton, D. Analysis of traveling ionospheric disturbances (TIDs) in GPS TEC launched by the 2011 Tohoku earthquake. Radio Sci. 2016, 51, 507–514. [Google Scholar] [CrossRef]
- Kong, J.; Yao, Y.; Xu, Y.; Kuo, C.; Zhang, L.; Liu, L.; Zhai, C. A clear link connecting the troposphere and ionosphere: Ionospheric reponses to the 2015 Typhoon Dujuan. J. Geod. 2017, 91, 1087–1097. [Google Scholar] [CrossRef]
- Luo, X.; Gu, S.; Lou, Y.; Xiong, C.; Chen, B.; Jin, X. Assessing the Performance of GPS Precise Point Positioning Under Different Geomagnetic Storm Conditions during Solar Cycle 24. Sensors 2018, 18, 1784. [Google Scholar] [CrossRef] [PubMed]
- Rao, P.V.S.R.; Krishna, S.G.; Prasad, J.V.; Prasad, S.N.V.S.; Prasad, D.S.V.V.D.; Niranjan, K. Geomagnetic storm effects on GPS based navigation. Ann. Geophys. 2009, 27, 2101–2110. [Google Scholar] [CrossRef]
- Moreno, B.; Radicella, S.; de Lacy, M.C.; Herraiz, M.; Rodriguez-Caderot, G. On the effects of the ionospheric disturbances on precise point positioning at equatorial latitudes. GPS Solutions 2011, 15, 381–390. [Google Scholar] [CrossRef]
- Jacobsen, K.S.; Andalsvik, Y.L. Overview of the 2015 St. Patrick’s day storm and its consequences for RTK and PPP positioning in Norway. J. Space Weather. Space Clim. 2016, 6, A9. [Google Scholar] [CrossRef]
- Lu, Y.; Wang, Z.; Ji, S.; Chen, W. Assessing the positioning performance under the effects of strong ionospheric anomalies with multi-GNSS in Hong Kong. Radio Sci. 2020, 55, e2019RS007004. [Google Scholar] [CrossRef]
- Yasyukevich, Y.; Vasilyev, R.; Ratovsky, K.; Setov, A.; Globa, M.; Syrovatskii, S.; Yasyukevich, A.; Kiselev, A.; Vesnin, A. Small-Scale Ionospheric Irregularities of Auroral Origin at Mid-latitudes during the 22 June 2015 Magnetic Storm and Their Effect on GPS Positioning. Remote Sens. 2020, 12, 1579. [Google Scholar] [CrossRef]
- Hernandez-Pajares, M.; Juan, J.; Sanz, J.; Orus, R.; Garcia-Rigo, A.; Feltens, J.; Komjathy, A.; Schaer, S.; Krankowski, A. The IGS VTEC maps: A reliable source of ionospheric information since 1998. J. Geodesy 2009, 83, 263–275. [Google Scholar] [CrossRef]
- Krypiak-Gregorczyk, A.; Wielgosz, P.; Gosciewski, D.; Paziewski, J. Validation of approximation techniques for local total electron content mapping. Acta Geodyn. Geomater. 2013, 10, 275–283. [Google Scholar] [CrossRef]
- Figueiredo, C.; Wrasse, C.; Takahashi, H.; Otsuka, Y.; Shiokawa, K.; Barros, D. Large-scale traveling ionospheric disturbances observed by GPS dTEC maps over North and South America on Saint Patrick’s day storm in 2015. J. Geophys. Res. Space Phys. 2017, 122, 4755–4763. [Google Scholar] [CrossRef]
- Nykiel, G.; Zanimonskiy, Y.M.; Yampolski, Y.M.; Figurski, M. Efficient Usage of Dense GNSS Networks in Central Europe for the Visualization and Investigation of Ionospheric TEC Variations. Sensors 2017, 17, 2298. [Google Scholar] [CrossRef] [PubMed]
- Yizengaw, E.; Moldwin, M. The altitude extension of the mid-latitude trough and its correlation with plasmapause position. Geophys. Res. Lett. 2005, 32, L09105. [Google Scholar] [CrossRef]
- Nykiel, G.; Zanimonskiy, Y.; Koloskov, A.; Figurski, M. The possibility of estimating the height of the ionospheric inhomogeneities based on TEC variations maps obtained from dense GPS network. Adv. Space Res. 2019, 64, 2002–2011. [Google Scholar] [CrossRef]
- Wu, C.; Liou, K.; Lepping, R.P.; Hutting, L.; Plunkett, S.; Russ, A.H.; Socker, D. The first super geomagnetic storm of solar cycle 24: “The St. Patrick’s day event (17 March 2015)”. Earth Planets Space 2016, 68, 151. [Google Scholar] [CrossRef]
- Kamide, Y.; Kusano, K. No Major Solar Flares but the Largest Geomagnetic Storm in the Present Solar Cycle. Space Weather 2015, 13, 365–367. [Google Scholar] [CrossRef]
- Watari, S. Geomagnetic storms of cycle 24 and their solar sources. Earth Planets Space 2017, 69, 70. [Google Scholar] [CrossRef]
- Ibáñez, D.; Rovira-García, A.; Sanz, J.; Juan, J.M.; Gonzalez-Casado, G.; Jimenez-Baños, D.; López-Echazarreta, C.; Lapin, I. The GNSS Laboratory Tool Suite (gLAB) updates: SBAS, DGNSS and Global Monitoring System. In Proceedings of the 9th ESA Workshop on Satellite Navigation Technologies (NAVITEC 2018), Noordwijk, The Netherlands, 5–7 December 2018. [Google Scholar] [CrossRef]
- Johnston, G.; Riddell, A.; Hausler, G. The International GNSS Service. In Springer Handbook of Global Navigation Satellite Systems, 1st ed.; Teunissen, P.J.G., Montenbruck, O., Eds.; Springer International Publishing: Cham, Switzerland, 2017; pp. 967–982. [Google Scholar] [CrossRef]
- Kiliszek, D.; Szolucha, M.; Kroszczyński, K. Accuracy of Precise Point Positioning (PPP) with the use of different International GNSS Service (IGS) products and stochastic modelling. Geod. Cartography 2018, 67, 207–238. [Google Scholar] [CrossRef]
- Blewitt, G. An Automatic Editing Algorithms for GPS Data. Geophys. Res. Lett. 1990, 17, 199–202. [Google Scholar] [CrossRef]
- Hofmann-Wellenhof, B.; Lichtenegger, H.K.; Wasle, E. GNSS-Global Navigation Satellite Systems; Springer: Wien, Austria, 2008. [Google Scholar]




| GNSS Station | RMS (m) | |||||
|---|---|---|---|---|---|---|
| Quiet Day (13 March 2015) | Stormy Day (17 March 2015) | |||||
| North | East | Up | North | East | Up | |
| BART | 0.02 | 0.02 | 0.04 | 0.24 | 0.21 | 0.52 |
| CCHN | 0.01 | 0.02 | 0.03 | 0.15 | 0.06 | 0.11 |
| CHNO | 0.01 | 0.01 | 0.03 | 0.50 | 0.33 | 0.61 |
| CHOJ | 0.01 | 0.01 | 0.03 | 0.28 | 0.23 | 0.63 |
| GIZY | 0.01 | 0.01 | 0.03 | 0.24 | 0.24 | 0.42 |
| GOLE | 0.02 | 0.03 | 0.05 | 0.56 | 0.28 | 0.67 |
| ILAW | 0.01 | 0.01 | 0.03 | 0.13 | 0.06 | 0.06 |
| KAM1 | 0.01 | 0.01 | 0.03 | 0.30 | 0.25 | 0.92 |
| KOSZ | 0.02 | 0.02 | 0.04 | 0.53 | 0.53 | 0.99 |
| LAMA | 0.01 | 0.01 | 0.03 | 0.30 | 0.38 | 0.38 |
| PPIL | 0.01 | 0.01 | 0.02 | 0.49 | 0.23 | 0.5 |
| SOKL | 0.02 | 0.02 | 0.04 | 0.39 | 0.21 | 0.27 |
| STRG | 0.01 | 0.01 | 0.02 | 0.37 | 0.28 | 0.79 |
| WLAD | 0.02 | 0.01 | 0.03 | 0.69 | 0.43 | 1.37 |
| WRKI | 0.02 | 0.02 | 0.04 | 0.34 | 0.20 | 0.45 |
| Average | 0.02 ± 0.01 | 0.02 ± 0.01 | 0.03 ± 0.01 | 0.37 ± 0.16 | 0.26 ± 0.12 | 0.58 ± 0.34 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Poniatowski, M.; Nykiel, G. Degradation of Kinematic PPP of GNSS Stations in Central Europe Caused by Medium-Scale Traveling Ionospheric Disturbances During the St. Patrick’s Day 2015 Geomagnetic Storm. Remote Sens. 2020, 12, 3582. https://doi.org/10.3390/rs12213582
Poniatowski M, Nykiel G. Degradation of Kinematic PPP of GNSS Stations in Central Europe Caused by Medium-Scale Traveling Ionospheric Disturbances During the St. Patrick’s Day 2015 Geomagnetic Storm. Remote Sensing. 2020; 12(21):3582. https://doi.org/10.3390/rs12213582
Chicago/Turabian StylePoniatowski, Mateusz, and Grzegorz Nykiel. 2020. "Degradation of Kinematic PPP of GNSS Stations in Central Europe Caused by Medium-Scale Traveling Ionospheric Disturbances During the St. Patrick’s Day 2015 Geomagnetic Storm" Remote Sensing 12, no. 21: 3582. https://doi.org/10.3390/rs12213582
APA StylePoniatowski, M., & Nykiel, G. (2020). Degradation of Kinematic PPP of GNSS Stations in Central Europe Caused by Medium-Scale Traveling Ionospheric Disturbances During the St. Patrick’s Day 2015 Geomagnetic Storm. Remote Sensing, 12(21), 3582. https://doi.org/10.3390/rs12213582






