Satellite Investigation of Semidiurnal Internal Tides in the Sulu-Sulawesi Seas
Abstract
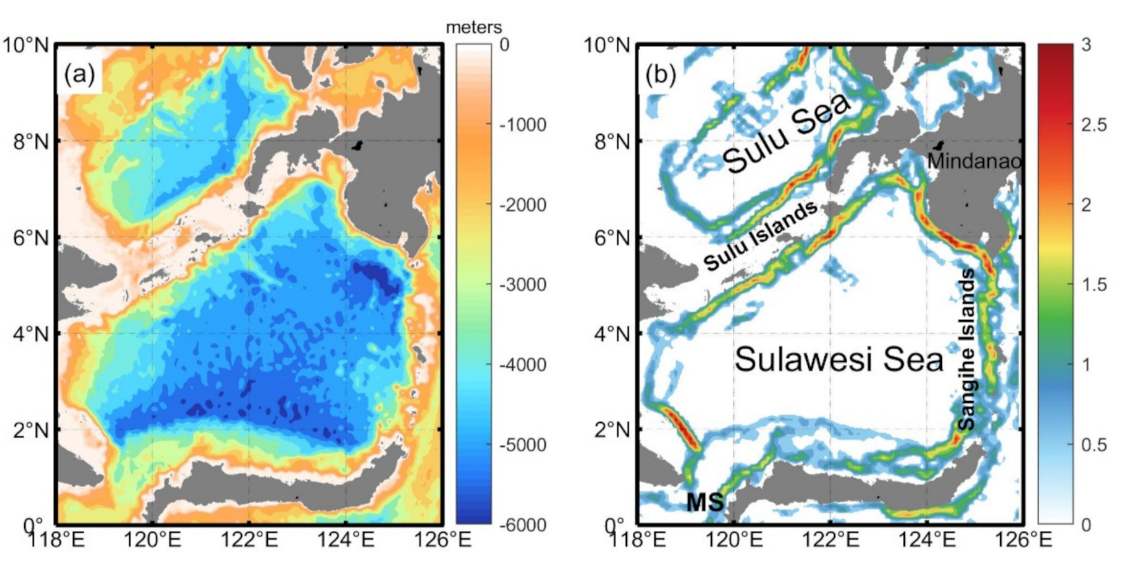
:1. Introduction
2. Data and Methods
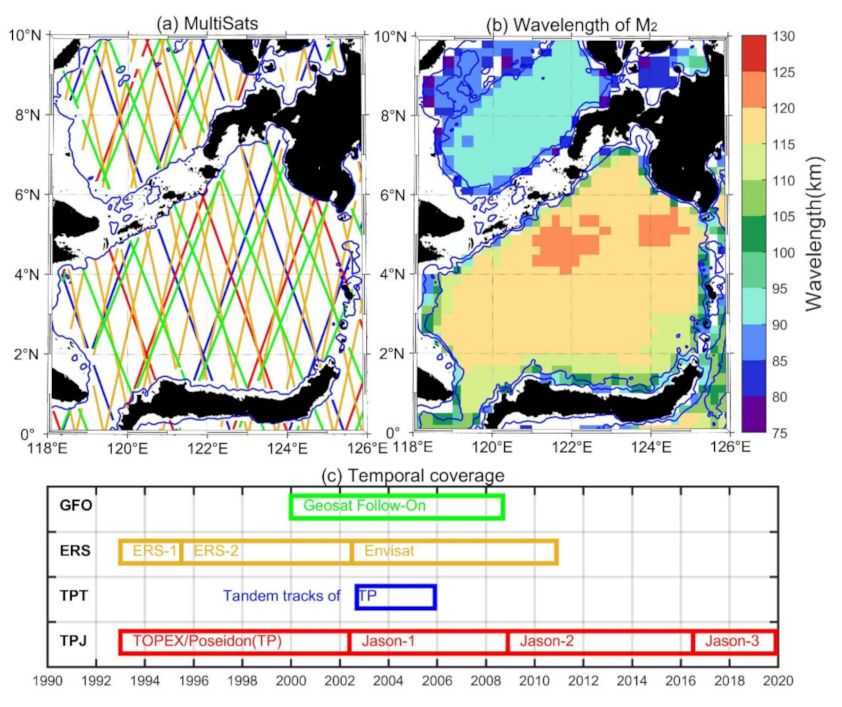
2.1. Satellite Altimeter Data
2.2. Two-Dimensional Plane-Wave Fit
2.3. Two-Dimensional Bandpass Filter
2.4. Phase Speed and Energy Flux
3. Results
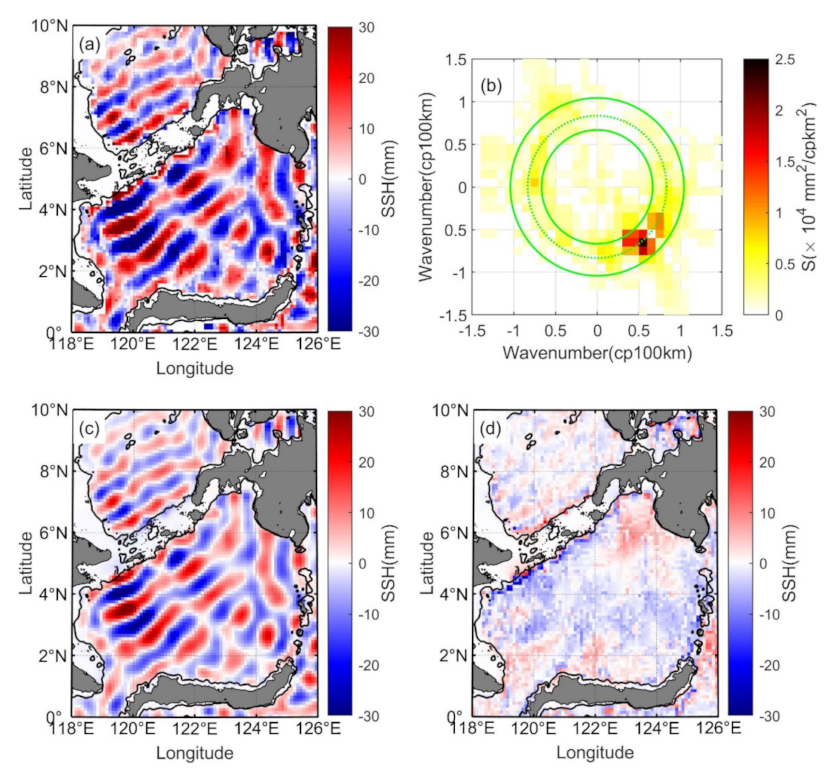
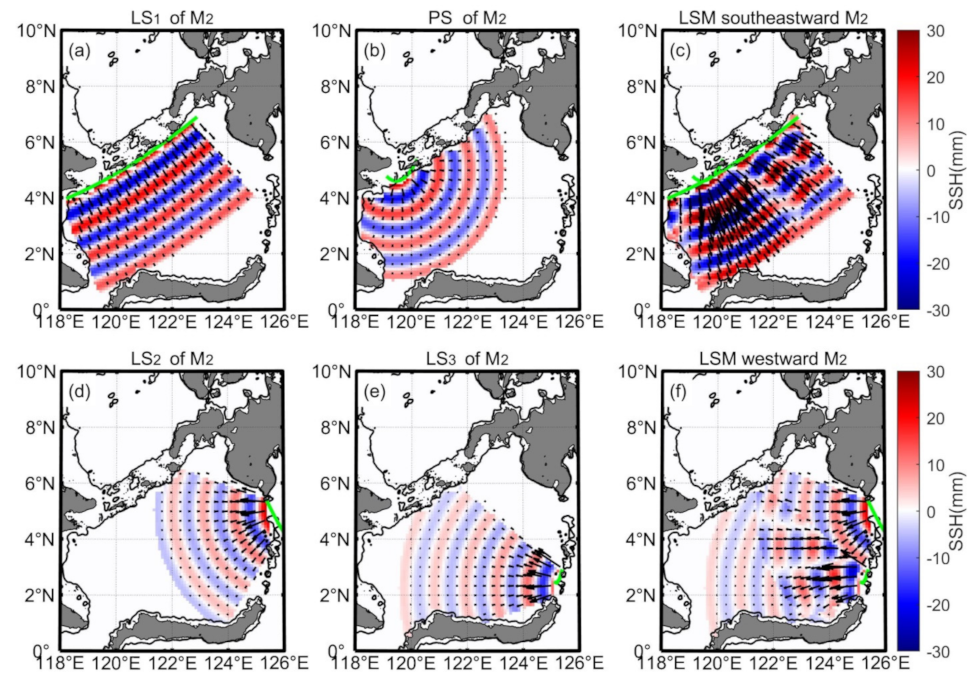
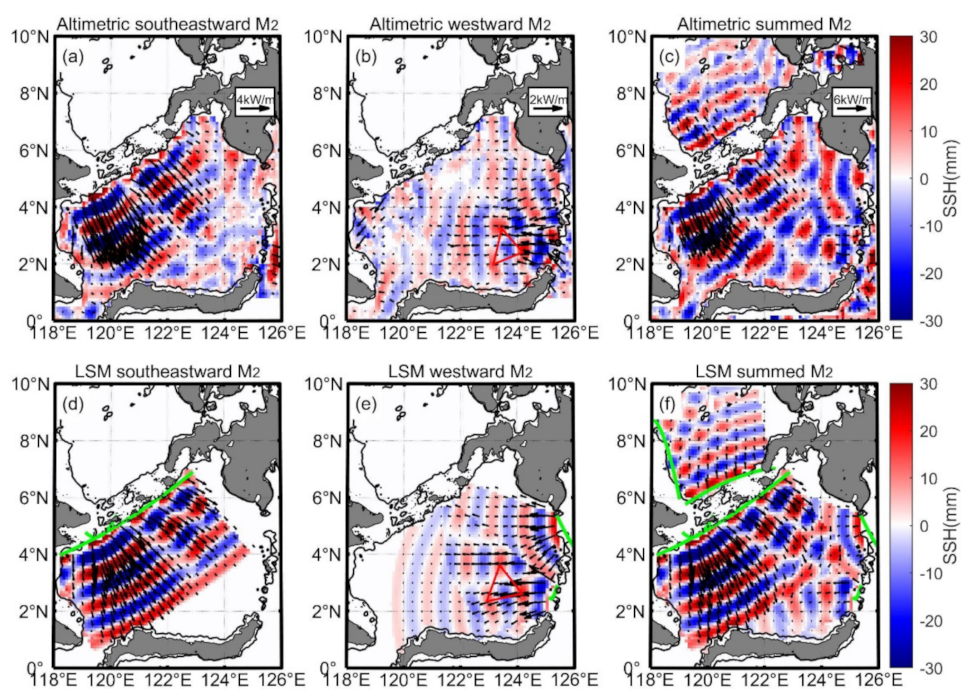
3.1. Decomposed M2 Internal Tides
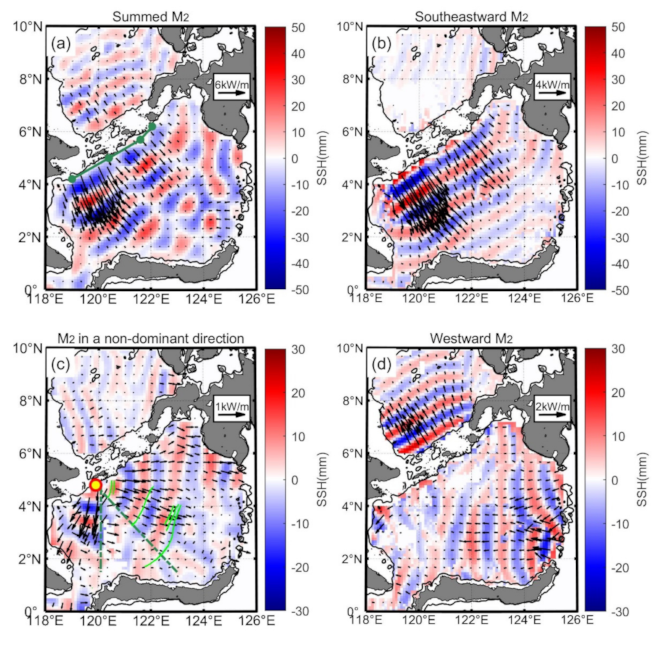
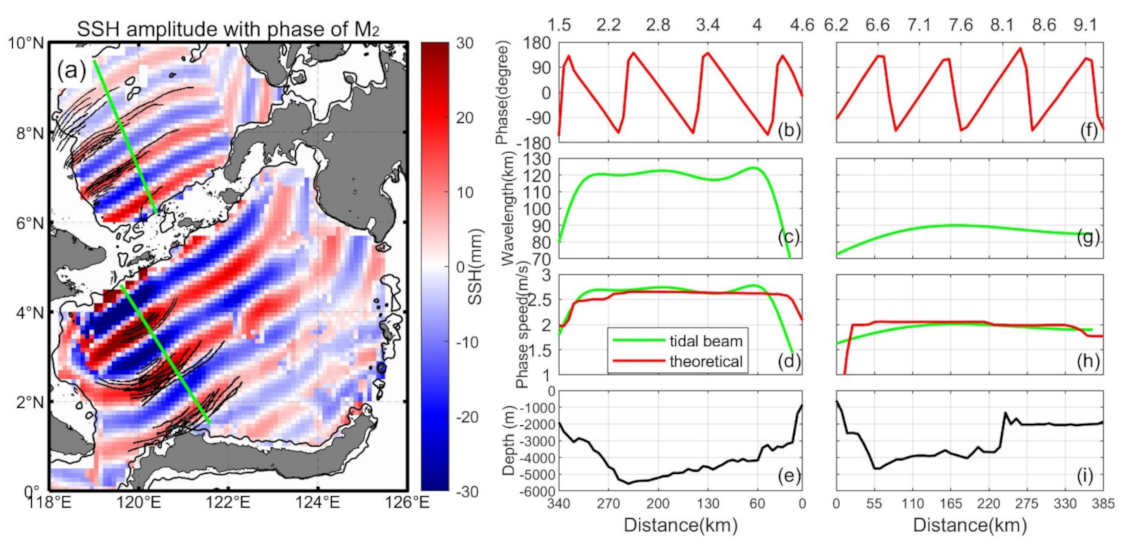
3.2. The Dominant Tidal Beam and Internal Solitary Waves
3.3. Sulu Sea vs. Sulawesi Sea
3.3.1. Comparison of Multiple Generation Sources
3.3.2. Comparison of Multiwave Interference Process
3.4. S2 Internal Tides
4. Discussion
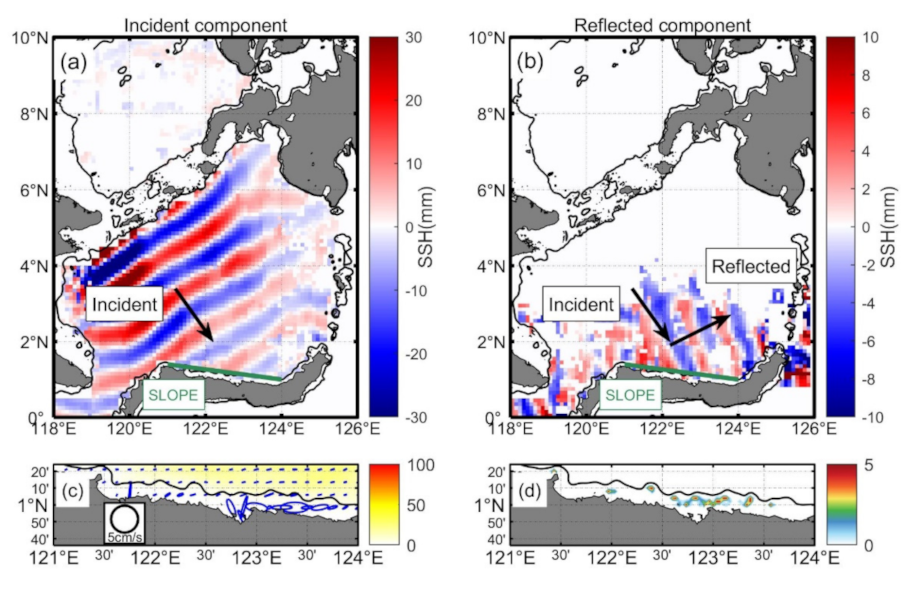
4.1. Reflection on the Sulawesi Continental Slope
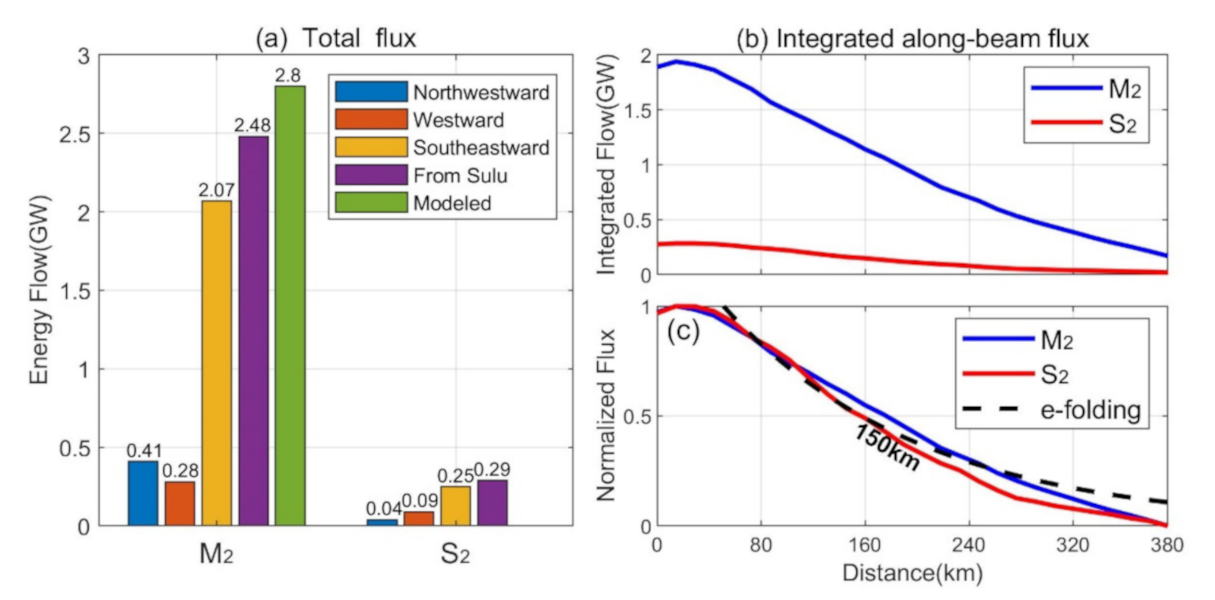
4.2. Internal Tide Energetics
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Munk, W.; Wunsch, C. Abyssal recipes II: Energetics of tidal and wind mixing. Deep Sea Res. Part I Oceanogr. Res. Pap. 1998, 45, 1977–2010. [Google Scholar] [CrossRef]
- Alford, M.H. Redistribution of energy available for ocean mixing by long-range propagation of internal waves. Nature 2003, 423, 156–162. [Google Scholar] [CrossRef] [PubMed]
- Neale, R.; Slingo, J. The Maritime Continent and Its Role in the Global Climate: A GCM Study. J. Clim. 2003, 16, 834–848. [Google Scholar] [CrossRef]
- Alford, M.H.; Zhao, Z. Global Patterns of Low-Mode Internal-Wave Propagation. Part I: Energy and Energy Flux. J. Phys. Oceanogr. 2007, 37, 1829–1848. [Google Scholar] [CrossRef]
- Egbert, G.D.; Ray, R.D. Semi-diurnal and diurnal tidal dissipation from TOPEX/Poseidon altimetry. Geophys. Res. Lett. 2003, 30, 1907. [Google Scholar] [CrossRef]
- MacKinnon, J.A.; Alford, M.H.; Ansong, J.K.; Arbic, B.K.; Barna, A.; Briegleb, B.P.; Bryan, F.O.; Buijsman, M.C.; Chassignet, E.P.; Danabasoglu, G.; et al. Climate Process Team on Internal Wave-Driven Ocean Mixing. Bull. Am. Meteorol. Soc. 2017, 98, 2429–2454. [Google Scholar] [CrossRef] [Green Version]
- Rainville, L.; Johnston, T.M.S.; Carter, G.S.; Merrifield, M.A.; Pinkel, R.; Worcester, P.F.; Dushaw, B.D. Interference Pattern and Propagation of the M2 Internal Tide South of the Hawaiian Ridge. J. Phys. Oceanogr. 2010, 40, 311–325. [Google Scholar] [CrossRef]
- Zhao, Z.; Alford, M.H.; Girton, J.B.; Rainville, L.; Simmons, H.L. Global Observations of Open-Ocean Mode-1 M2 Internal Tides. J. Phys. Oceanogr. 2016, 46, 1657–1684. [Google Scholar] [CrossRef]
- Chang, H.; Xu, Z.; Yin, B.; Hou, Y.; Liu, Y.; Li, D.; Wang, Y.; Cao, S.; Liu, A.K. Generation and Propagation of M2 Internal Tides Modulated by the Kuroshio Northeast of Taiwan. J. Geophys. Res. Ocean. 2019, 124, 2728–2749. [Google Scholar] [CrossRef]
- Wang, Y.; Xu, Z.; Yin, B.; Hou, Y.; Chang, H. Long-Range Radiation and Interference Pattern of Multisource M2 Internal Tides in the Philippine Sea. J. Geophys. Res. Ocean. 2018, 123, 5091–5112. [Google Scholar] [CrossRef] [Green Version]
- Xu, Z.; Liu, K.; Yin, B.; Zhao, Z.; Wang, Y.; Li, Q. Long-range propagation and associated variability of internal tides in the South China Sea. J. Geophys. Res. Ocean. 2016, 121, 8268–8286. [Google Scholar] [CrossRef]
- Zhao, C.; Xu, Z.; Robertson, R.; Li, Q.; Wang, Y.; Yin, B. The Three-Dimensional Internal Tide Radiation and Dissipation in the Mariana Arc-Trench System. J. Geophys. Res. Ocean. 2021, 126. [Google Scholar] [CrossRef]
- Simmons, H.L.; Hallberg, R.W.; Arbic, B.K. Internal wave generation in a global baroclinic tide model. Deep Sea Res. Part II Top. Stud. Oceanogr. 2004, 51, 3043–3068. [Google Scholar] [CrossRef]
- Robertson, R. Interactions between tides and other frequencies in the Indonesian seas. Ocean Dyn. 2011, 61, 69–88. [Google Scholar] [CrossRef]
- Robertson, R.; Ffield, A. Baroclinic tides in the Indonesian seas: Tidal fields and comparisons to observations. J. Geophys. Res. 2008, 113, C07031. [Google Scholar] [CrossRef] [Green Version]
- Koch-Larrouy, A.; Lengaigne, M.; Terray, P.; Madec, G.; Masson, S. Tidal mixing in the Indonesian Seas and its effect on the tropical climate system. Clim. Dyn. 2010, 34, 891–904. [Google Scholar] [CrossRef]
- Ffield, A.; Gordon, A.L. Tidal Mixing Signatures in the Indonesian Seas. J. Phys. Oceanogr. 1996, 26, 1924–1937. [Google Scholar] [CrossRef] [Green Version]
- Hatayama, T.; Awaji, T.; Akitomo, K. Tidal currents in the Indonesian Seas and their effect on transport and mixing. J. Geophys. Res. Ocean. 1996, 101, 12353–12373. [Google Scholar] [CrossRef]
- Ray, R.D.; Mitchum, G.T. Surface manifestation of internal tides in the deep ocean: Observations from altimetry and island gauges. Prog. Oceanogr. 1997, 40, 135–162. [Google Scholar] [CrossRef]
- Tian, J.; Zhou, L.; Zhang, X.; Liang, X.; Zheng, Q.; Zhao, W. Estimates of M2 internal tide energy fluxes along the margin of Northwestern Pacific using TOPEX/POSEIDON altimeter data. Geophys. Res. Lett. 2003, 30, 17. [Google Scholar] [CrossRef]
- Zaron, E.D. Baroclinic Tidal Sea Level from Exact-Repeat Mission Altimetry. J. Phys. Oceanogr. 2019, 49, 193–210. [Google Scholar] [CrossRef]
- Zhao, Z. Mapping Internal Tides from Satellite Altimetry without Blind Directions. J. Geophys. Res. Ocean. 2019, 124, 8605–8625. [Google Scholar] [CrossRef]
- Jithin, A.K.; Subeesh, M.P.; Francis, P.A.; Ramakrishna, S. Intensification of tidally generated internal waves in the north-central Bay of Bengal. Sci. Rep. 2020, 10, 6059. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Zhao, Z. Southward Internal Tides in the Northeastern South China Sea. J. Geophys. Res. Ocean. 2020, 125. [Google Scholar] [CrossRef]
- Koch-Larrouy, A.; Madec, G.; Bouruet-Aubertot, P.; Gerkema, T.; Bessières, L.; Molcard, R. On the transformation of Pacific Water into Indonesian Throughflow Water by internal tidal mixing. Geophys. Res. Lett. 2007, 34. [Google Scholar] [CrossRef] [Green Version]
- Egbert, G.D.; Erofeeva, S.Y. Efficient Inverse Modeling of Barotropic Ocean Tides. J. Atmos. Ocean. Technol. 2002, 19, 183–204. [Google Scholar] [CrossRef] [Green Version]
- Nagai, T.; Hibiya, T. Internal tides and associated vertical mixing in the Indonesian Archipelago. J. Geophys. Res. Ocean. 2015, 120, 3373–3390. [Google Scholar] [CrossRef]
- Buijsman, M.C.; Arbic, B.K.; Richman, J.G.; Shriver, J.F.; Wallcraft, A.J.; Zamudio, L. Semidiurnal internal tide incoherence in the equatorial Pacific. J. Geophys. Res. Ocean. 2017, 122, 5286–5305. [Google Scholar] [CrossRef]
- Ray, R.D.; Zaron, E.D. M2 Internal Tides and Their Observed Wavenumber Spectra from Satellite Altimetry. J. Phys. Oceanogr. 2016, 46, 3–22. [Google Scholar] [CrossRef]
- Ray, R.D.; Cartwright, D.E. Estimates of internal tide energy fluxes from Topex/Poseidon Altimetry: Central North Pacific. Geophys. Res. Lett. 2001, 28, 1259–1262. [Google Scholar] [CrossRef] [Green Version]
- Locarnini, R.A.; Mishonov, A.V.; Baranova, O.K.; Boyer, T.P.; Locarnini, R.A. World Ocean Atlas 2018, Volume 1: Temperature; NOAA Atlas NESDIS: Silver Spring, MD, USA, 2019. [Google Scholar]
- Hao, Z.; Xu, Z.; Feng, M.; Li, Q.; Yin, B. Spatiotemporal Variability of Mesoscale Eddies in the Indonesian Seas. Remote Sens. 2021, 13, 1017. [Google Scholar] [CrossRef]
- He, Y.; Feng, M.; Xie, J.; Liu, J.; Chen, Z.; Xu, J.; Fang, W.; Cai, S. Spatiotemporal Variations of Mesoscale Eddies in the Sulu Sea. J. Geophys. Res. Ocean. 2017, 122, 7867–7879. [Google Scholar] [CrossRef]
- Wunsch, C. Internal tides in the ocean. Rev. Geophys. 1975, 13, 167–182. [Google Scholar] [CrossRef]
- Gill, A. Atmosphere-Ocean Dynamics; Academic Press: Cambridge, MA, USA, 1982. [Google Scholar]
- Zhao, Z.; Alford, M.H. New Altimetric Estimates of Mode-1 M2 Internal Tides in the Central North Pacific Ocean. J. Phys. Oceanogr. 2009, 39, 1669–1684. [Google Scholar] [CrossRef]
- Muller, M. On the space- and time-dependence of barotropic-to-baroclinic tidal energy conversion. Ocean Model. 2013, 72, 242–252. [Google Scholar] [CrossRef]
- Hurlburt, H.; Metzger, J.; Sprintall, J.; Riedlinger, S.; Arnone, R.; Shinoda, T.; Xu, X. Circulation in the Philippine Archipelago Simulated by 1/12° and 1/25° Global HYCOM and EAS NCOM. Oceanography 2011, 24, 28–47. [Google Scholar] [CrossRef]
- Metzger, E.J.; Hurlburt, H.E.; Xu, X.; Shriver, J.F.; Gordon, A.L.; Sprintall, J.; Susanto, R.D.; van Aken, H.M. Simulated and observed circulation in the Indonesian Seas: 1/12° global HYCOM and the INSTANT observations. Dyn. Atmos. Ocean. 2010, 50, 275–300. [Google Scholar] [CrossRef]
- Nugroho, D.; Koch-Larrouy, A.; Gaspar, P.; Lyard, F.; Reffray, G.; Tranchant, B. Modelling explicit tides in the Indonesian seas: An important process for surface sea water properties. Mar. Pollut. Bull. 2018, 131, 7–18. [Google Scholar] [CrossRef]
- Chen, G.-Y.; Su, F.-C.; Wang, C.-M.; Liu, C.-T.; Tseng, R.-S. Derivation of internal solitary wave amplitude in the South China Sea deep basin from satellite images. J. Oceanogr. 2011, 67, 689–697. [Google Scholar] [CrossRef]
- Tessler, Z.D.; Gordon, A.L.; Jackson, C.R. Early Stage Soliton Observations in the Sulu Sea. J. Phys. Oceanogr. 2012, 42, 1327–1336. [Google Scholar] [CrossRef]
- Zhang, X.; Li, X.; Zhang, T. Characteristics and generations of internal wave in the Sulu Sea inferred from optical satellite images. J. Oceanol. Limnol. 2020, 38, 1435–1444. [Google Scholar] [CrossRef]
- Apel, J.R.; Holbrook, J.R.; Liu, A.K.; Tsai, J.J. The Sulu Sea Internal Soliton Experiment. J. Phys. Oceanogr. 1985, 15, 1625–1651. [Google Scholar] [CrossRef] [Green Version]
- Liu, B.; D’Sa, E.J. Oceanic Internal Waves in the Sulu–Celebes Sea Under Sunglint and Moonglint. IEEE Trans. Geosci. Remote Sens. 2019, 57, 6119–6129. [Google Scholar] [CrossRef]
- Ray, R.D.; Egbert, G.D.; Erofeeva, S. A brief overview of tides in the Indonesian Seas. Oceanography 2005, 18, 74–79. [Google Scholar] [CrossRef]
- St. Laurent, L.; Stringer, S.; Garrett, C.; Perrault-Joncas, D. The generation of internal tides at abrupt topography. Deep Sea Res. Part I Oceanogr. Res. Pap. 2003, 50, 987–1003. [Google Scholar] [CrossRef]
- Zhao, Z. The Global Mode-1 S2 Internal Tide. J. Geophys. Res. Ocean. 2017, 122, 8794–8812. [Google Scholar] [CrossRef] [Green Version]
- Zhao, Z.; Alford, M.H.; Simmons, H.L.; Brazhnikov, D.; Pinkel, R. Satellite Investigation of the M2 Internal Tide in the Tasman Sea. J. Phys. Oceanogr. 2018, 48, 687–703. [Google Scholar] [CrossRef]
- Wang, S.; Cao, A.; Chen, X.; Li, Q.; Song, J.; Meng, J. Estimation of the Reflection of Internal Tides on a Slope. J. Ocean Univ. China 2020, 19, 489–496. [Google Scholar] [CrossRef]
- Hermansyah, H.; Atmadipoera, A.S.; Prartono, T.; Jaya, I.; Syamsudin, F. Energetics of internal tides over the Sangihe-Talaud ridge—Sulawesi sea. J. Phys. Conf. Ser. 2019, 1341, 082001. [Google Scholar] [CrossRef] [Green Version]
- Xu, Z.; Wang, Y.; Liu, Z.; McWilliams, J.C.; Gan, J. Insight into the Dynamics of the Radiating Internal Tide Associated with the Kuroshio Current. J. Geophys. Res. Ocean. 2021, 126. [Google Scholar] [CrossRef]
- Song, P.; Chen, X. Investigation of the Internal Tides in the Northwest Pacific Ocean Considering the Background Circulation and Stratification. J. Phys. Oceanogr. 2020, 50, 3165–3188. [Google Scholar] [CrossRef]
- Xu, Z.; Yin, B.; Hou, Y.; Liu, A.K. Seasonal variability and north–south asymmetry of internal tides in the deep basin west of the Luzon Strait. J. Mar. Syst. 2014, 134, 101–112. [Google Scholar] [CrossRef]
- Xu, Z.; Yin, B.; Hou, Y.; Xu, Y. Variability of internal tides and near-inertial waves on the continental slope of the northwestern South China Sea. J. Geophys. Res. Ocean. 2013, 118, 197–211. [Google Scholar] [CrossRef]



| Source 1 | Elevation (mm) | Radius (km): R0 | Direction (°): θ |
|---|---|---|---|
| PS | 20 | 100 | 305 ± 85 |
| LS1 | 30 | 2000 2 | 302 ± 8 |
| LS2 | 15 | 150 | 200 ± 40 |
| LS3 | 15 | 150 | 170 ± 30 |
| LS4 | 20 | 800 | 112 ± 12 |
| LS5 | 5 | 500 | 20 ± 20 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, X.; Xu, Z.; Feng, M.; Li, Q.; Zhang, P.; You, J.; Gao, S.; Yin, B. Satellite Investigation of Semidiurnal Internal Tides in the Sulu-Sulawesi Seas. Remote Sens. 2021, 13, 2530. https://doi.org/10.3390/rs13132530
Zhao X, Xu Z, Feng M, Li Q, Zhang P, You J, Gao S, Yin B. Satellite Investigation of Semidiurnal Internal Tides in the Sulu-Sulawesi Seas. Remote Sensing. 2021; 13(13):2530. https://doi.org/10.3390/rs13132530
Chicago/Turabian StyleZhao, Xiaoyu, Zhenhua Xu, Ming Feng, Qun Li, Peiwen Zhang, Jia You, Song Gao, and Baoshu Yin. 2021. "Satellite Investigation of Semidiurnal Internal Tides in the Sulu-Sulawesi Seas" Remote Sensing 13, no. 13: 2530. https://doi.org/10.3390/rs13132530
APA StyleZhao, X., Xu, Z., Feng, M., Li, Q., Zhang, P., You, J., Gao, S., & Yin, B. (2021). Satellite Investigation of Semidiurnal Internal Tides in the Sulu-Sulawesi Seas. Remote Sensing, 13(13), 2530. https://doi.org/10.3390/rs13132530








