Improved Ionosonde Monitoring of the Sporadic E Layer Using the Frequency Domain Interferometry Technique
Abstract
:1. Introduction
2. Experiment Setup and Methods
2.1. Instruments and Experimental Setup
2.2. Es Layer Imaging Based on the FDI Technique
3. Results
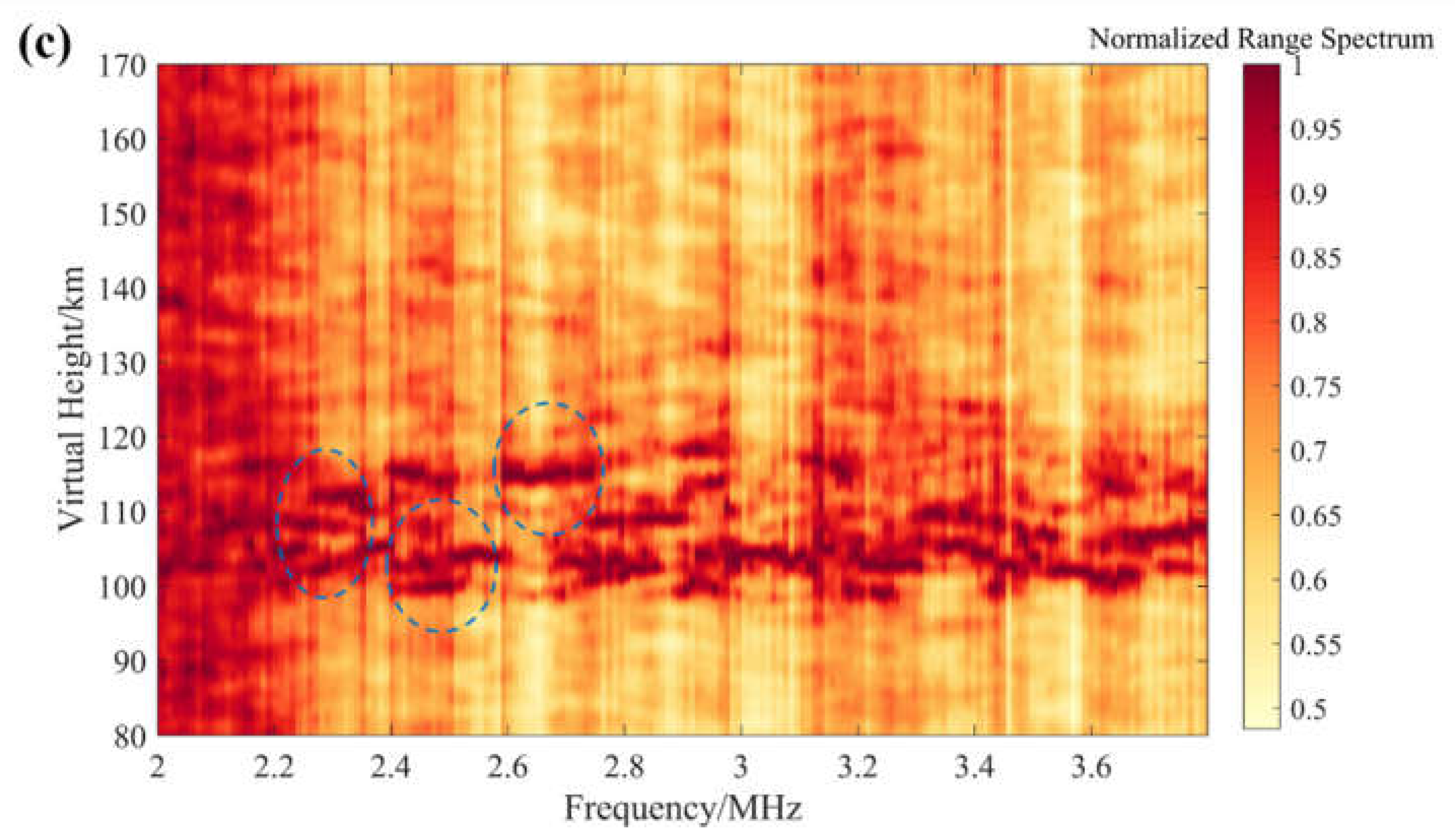
3.1. The Inhomogeneous Es Layer
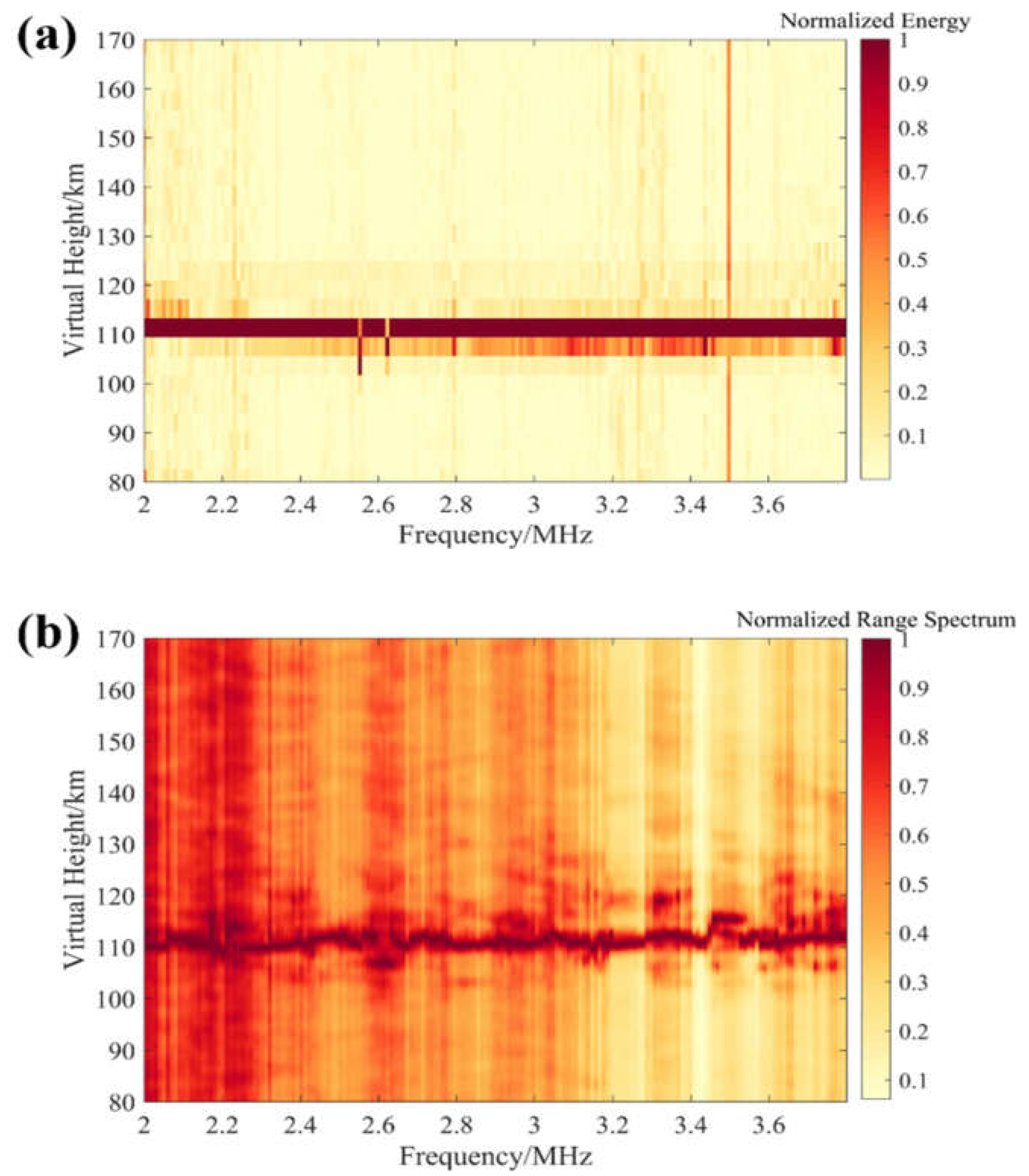
3.2. Quiet Es Layer
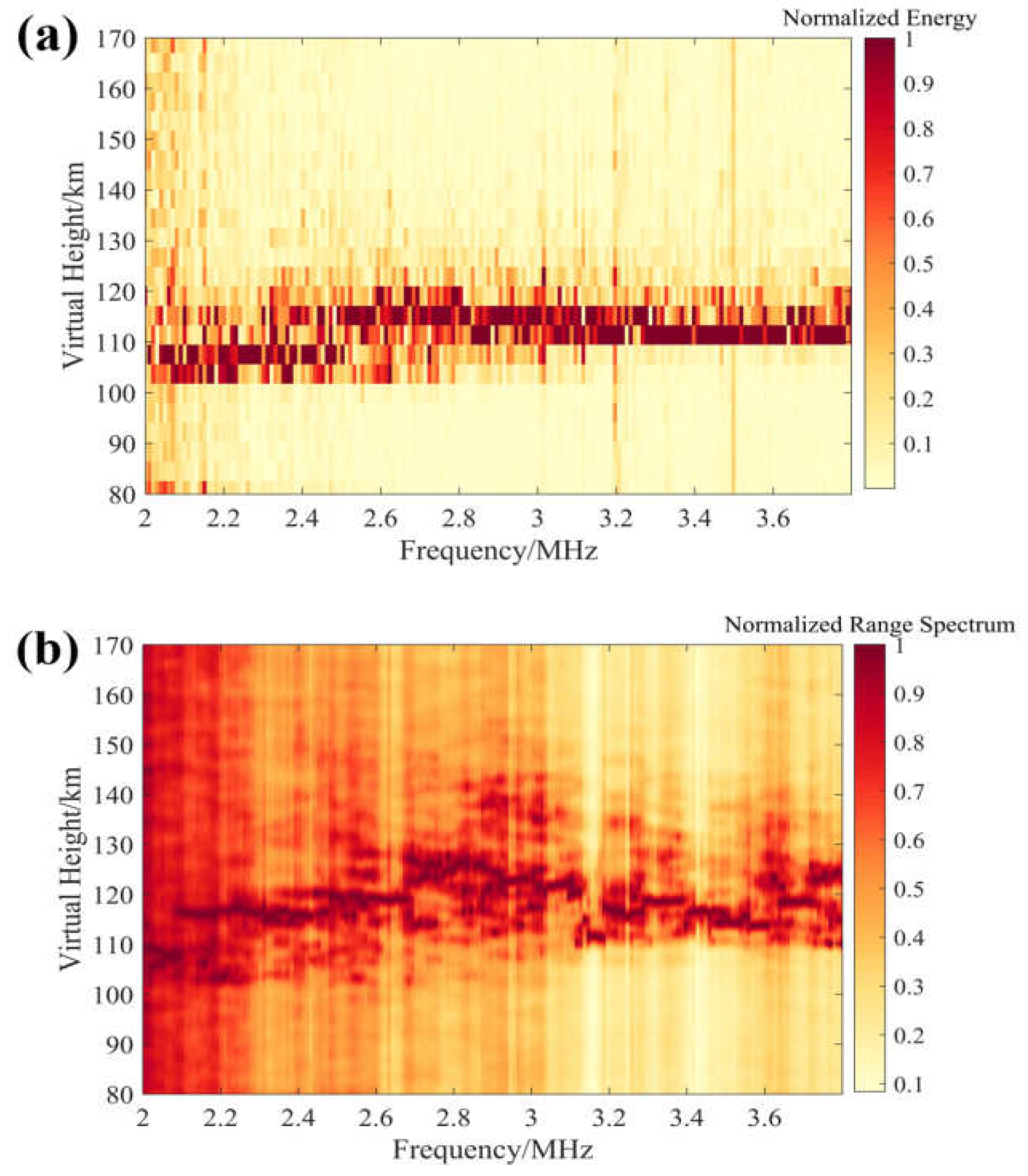
3.3. Short-Term Evolution of the Es Layer
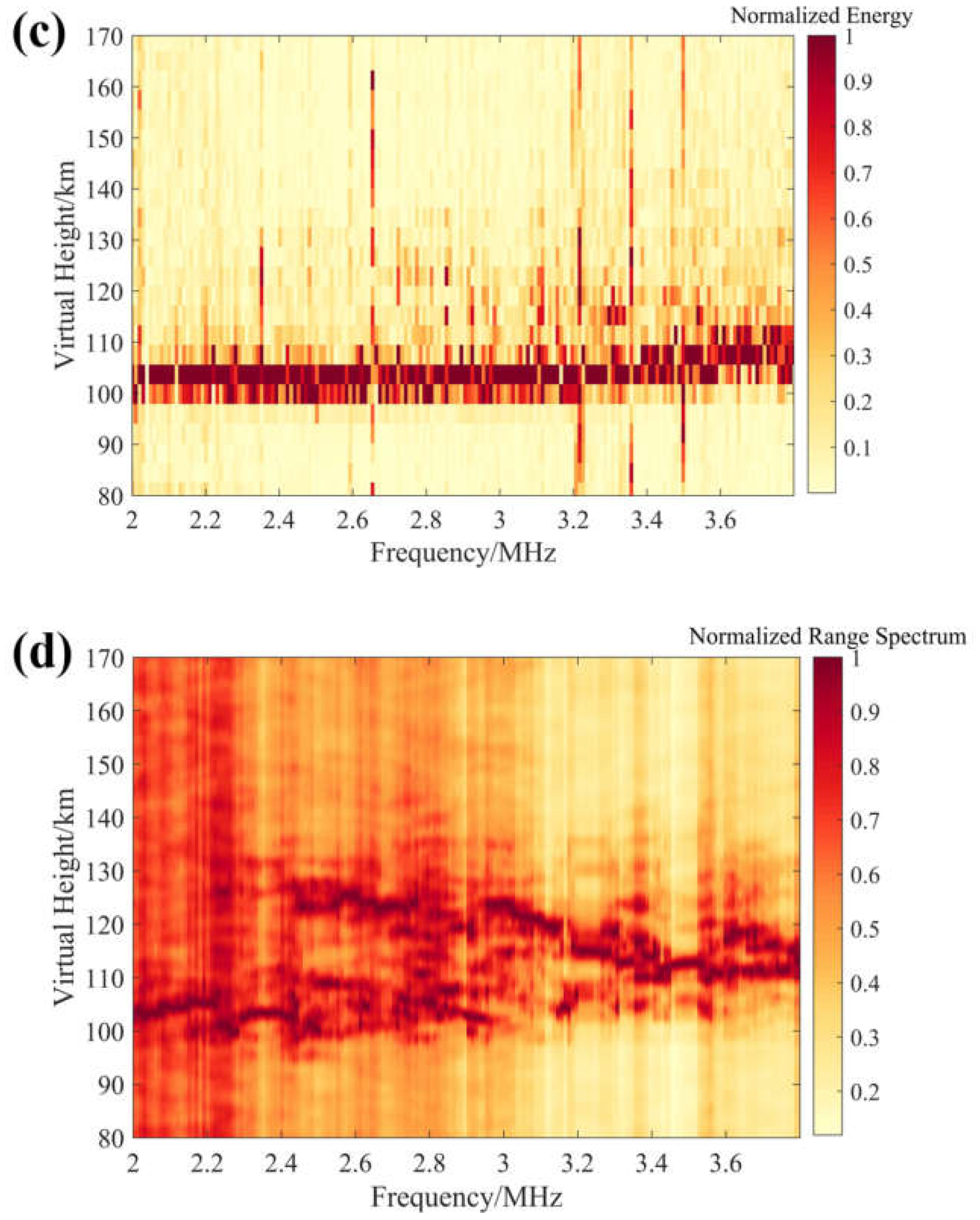
3.4. Different Types of Es Layer
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Pietrella, M.; Bianchi, C. Occurrence of sporadic-E layer over the ionospheric station of Rome: Analysis of data for thirty-two years. Adv. Space Res. 2009, 44, 72–81. [Google Scholar] [CrossRef]
- Haldoupis, C.; Pancheva, D.; Singer, W.; Meek, C.; Macdougall, J. An explanation for the seasonal dependence of midlatitude sporadic E layers. J. Geophys. Res. 2007, 112, A6. [Google Scholar] [CrossRef]
- Whitehead, J.D. Formation of the sporadic E layer in the temperate zones. J. Atmos. Terr. Phys. 1961, 20, 49–58. [Google Scholar] [CrossRef]
- Axford, W.; Cunnold, D. The wind shear theory of temperate zone sporadic E. Radio Sci. 1966, 1, 191–197. [Google Scholar] [CrossRef]
- Nygrén, T.; Lanchester, B.S.; Huuskonen, A.; Jalonen, L.; Eyken, A. Interference of tidal and gravity waves in the ionosphere and an associated sporadic E-layer. J. Atmos. Sol.-Terr. Phys. 1990, 52, 609–623. [Google Scholar] [CrossRef]
- Goldsbrough, P.F.; Ellyett, C.D. Relationship of meteors to sporadic E, 2. statistical evidence for class 1 Em. J. Geophys. Res. Atmos. 1976, 81, 6135–6140. [Google Scholar] [CrossRef]
- Barta, V.; Haldoupis, C.; Sátori, G.; Buresova, D.; Bencze, P. Searching for effects caused by thunderstorms in midlatitude sporadic E layers. J. Atmos. Sol.-Terr. Phys. 2017, 161, 150–159. [Google Scholar] [CrossRef] [Green Version]
- Bernhardt, P. The modulation of sporadic-E layers by Kelvin–Helmholtz billows in the neutral atmosphere. J. Atmos. Sol.-Terr. Phys. 2002, 64, 1487–1504. [Google Scholar] [CrossRef]
- Cosgrove, R.; Tsunoda, R. Wind-shear-driven, closed-current dynamos in midlatitude sporadic E. Geophys. Res. Lett. 2002, 29, 1020. [Google Scholar] [CrossRef]
- Haldoupis, C.; Pancheva, D.; Mitchell, N.J. A study of tidal and planetary wave periodicities present in midlatitude sporadic E layers. J. Geophys. Res. Space Phys. 2004, 109, A02302. [Google Scholar] [CrossRef]
- Haldoupis, C.; Pancheva, D. Terdiurnal tidelike variability in sporadic E layers. J. Geophys. Res. Space Phys. 2006, 111, A07303. [Google Scholar] [CrossRef]
- Tsunoda, R.T.; Fukao, S.; Yamamoto, M.; Hamasaki, T. First 24.5-MHz radar measurements of quasi-periodic backscatter from field-aligned irregularities in midlatitude sporadic E. Geophys. Res. Lett. 1998, 25, 1765–1768. [Google Scholar] [CrossRef]
- Woodman, R.F.; Yamamoto, M.; Fukao, S. Gravity wave modulation of gradient drift instabilities in mid-latitude sporadic E irregularities. Geophys. Res. Lett. 1991, 18, 1197–1200. [Google Scholar] [CrossRef]
- Maeda, J.; Suzuki, T.; Furuya, M.; Heki, K. Imaging the midlatitude sporadic E plasma patches with a coordinated observation of spaceborne insar and gps total electron content. Geophys. Res. Lett. 2016, 43, 1419–1425. [Google Scholar] [CrossRef] [Green Version]
- Yuan, T.; Wang, J.; Cai, X.; Sojka, J.; Rice, D.; Oberheide, J.; Criddle, N. Investigation of the seasonal and local time variations of the high-altitude sporadic Na layer (Nas) formation and the associated midlatitude descending E layer (Es) in lower E region. J. Geophys. Res. Space Phys. 2014, 119, 5985–5999. [Google Scholar] [CrossRef]
- Hysell, D.; Larsen, M.; Fritts, D.; Laughman, B.; Sulzer, M. Major upwelling and over-turning in the mid-latitude F region ionosphere. Nat. Commun. 2018, 9, 3326. [Google Scholar] [CrossRef]
- Mori, H.; Oyama, K.I. Sounding rocket observation of sporadic-E layer electron-density irregularities. Geophys. Res. Lett. 1998, 25, 1785–1788. [Google Scholar] [CrossRef]
- Mori, H.; Oyama, K.I. Rocket observation of sporadic-E layers and electron density irregularities over midlatitude. Adv. Space Res. 2000, 26, 1251–1255. [Google Scholar] [CrossRef]
- Bernhardt, P.A.; Selcher, C.A.; Siefring, C.; Wilkens, M.; Compton, C.; Bust, G.; Yamamoto, M.; Fukao, S.; Takayuki, O.; Wakabayashi, M. Radio tomographic imaging of sporadic-E layers during SEEK-2. Ann. Geophys. 2005, 23, 2357–2368. [Google Scholar] [CrossRef]
- Damtie, B.; Nygrén, T.; Lehtinen, M.S.; Huuskonen, A. High resolution observations of sporadic-E layers within the polar cap ionosphere using a new incoherent scatter radar experiment. Ann. Geophys. 2003, 20, 1429–1438. [Google Scholar] [CrossRef] [Green Version]
- Turunen, T.; Nygrén, T.; Huuskonen, A.; Jalonen, L. Incoherent scatter studies of sporadic-E using 300 m resolution. J. Atmos. Terr. Phys. 1988, 50, 277–287. [Google Scholar] [CrossRef]
- Zaalov, N.Y.; Moskaleva, E.V. Statistical analysis and modelling of sporadic E layer over Europe. Adv. Space Res. 2019, 64, 1243–1255. [Google Scholar] [CrossRef]
- Whitehead, J.D. The structure of sporadic e from a radio experiment. Radio Sci. 1972, 7, 355–358. [Google Scholar] [CrossRef]
- Dudeney, J.R.; Rodger, A.S. Spatial structure of high latitude sporadic E. J. Atmos. Terr. Phys. 1985, 47, 529–535. [Google Scholar] [CrossRef]
- Hysell, D.L.; Nossa, E.; Aveiro, H.C.; Larsen, M.F.; Munro, J.; Sulzer, M.P.; Gonzalez, S.A. Fine structure in midlatitude sporadic E layers. J. Atmos. Sol.-Terr. Phys. 2013, 103, 16–23. [Google Scholar] [CrossRef]
- Hysell, D.L.; Munk, J.; Mccarrick, M. Sporadic E ionization layers observed with ra-dar imaging and ionospheric modification. Geophys. Res. Lett. 2014, 41, 6987–6993. [Google Scholar] [CrossRef]
- Hocke, K.; Igarashi, K.; Nakamura, M.; Wilkinson, P.; Wu, J.; Pavelyev, A.; Wickert, J. Global sounding of sporadic E layers by the GPS/MET radio occultation experiment. J. Atmos. Sol.-Terr. Phys. 2001, 63, 1973–1980. [Google Scholar] [CrossRef]
- Maeda, J.; Heki, K. Two-dimensional observations of midlatitude sporadic E irregularities with a dense gps array in japan. Radio Sci. 2016, 49, 28–35. [Google Scholar] [CrossRef] [Green Version]
- Palmer, R.D.; Yu, T.-Y.; Chilson, P.B. Range imaging using frequency diversity. Radio Sci. 1999, 34, 1485–1496. [Google Scholar] [CrossRef]
- Liu, T.; Yang, G.; Hu, Y.; Jiang, C.; Lan, T.; Zhao, Z.; Ni, B. A novel ionospheric sounding network based on complete complementary code and its application. Sensors 2019, 19, 779. [Google Scholar] [CrossRef] [Green Version]
- Jiang, C.; Yang, G.; Zhao, Z.; Zhang, Y.; Chen, Z. A method for the automatic calculation of electron density profiles from vertical incidence ionograms. J. Atmos. Sol.-Terr. Phys. 2014, 107, 20–29. [Google Scholar] [CrossRef]
- Jiang, C.; Chen, Z.; Jing, L.; Lan, T.; Yang, G.; Zhao, Z.; Peng, Z.; Sun, H.; Xiao, C. Comparison of the kriging and neural network methods for modeling foF2 maps over north china region. Adv. Space Res. 2015, 56, 38–46. [Google Scholar] [CrossRef]
- Matzka, J.; Stolle, C.; Yamazaki, Y.; Bronkalla, O.; Morschhauser, A. The geomagnetic Kp index and derived indices of geomagnetic activity. Space Weather 2021, 19, e2020SW002641. [Google Scholar] [CrossRef]
- Chen, J.; Zecha, M. Multiple-frequency range imaging using the oswin VHF radar: Phase calibration and first results. Radio Sci. 2009, 44, 1–16. [Google Scholar] [CrossRef] [Green Version]
- Chen, J.; Chu, Y.; Su, C.; Hashiguchi, H.; Ying, L. Observations of field-aligned irregularities in the ionosphere using multi-frequency range imaging technique. In Proceedings of the Geoscience & Remote Sensing Symposium, Milan, Italy, 26–31 July 2015. [Google Scholar] [CrossRef]
- Bilitza, D.; Altadill, D.; Truhlik, V.; Shubin, V.; Galkin, I.; Reinisch, B.; Huang, X. International Reference Ionosphere 2016: From ionospheric climate to real-timeweather predictions. Space Weather 2017, 15, 418–429. [Google Scholar] [CrossRef]
- Wakai, N.; Ohyama, H.; Koizumi, T. Manual of Ionogram Scaling Third Version; Radio Research Laboratory Ministry of Posts and Telecommunications: Tokyo, Japan, 1987. [Google Scholar]
- Reddy, C.; Rao, M.; Matsushita, S.; Smith, L.G. Rocket observations of electron densities in the night-time auroral E-region at Fort Churchill, Canada. Planet. Space Sci. 1969, 17, 617–628. [Google Scholar] [CrossRef]
- Kagan, L.M.; Bakhmet'Eva, N.V.; Belikovich, V.V.; Tolmacheva, A.V.; Kelley, M.C. Structure and dynamics of sporadic layers of ionization in the ionospheric E region. Radio Sci. 2002, 37, 1–12. [Google Scholar] [CrossRef]
- Cosgrove, R.; Tsunoda, R. Polarization electric fields sustained by closed-current dynamo structures in midlatitude sporadic E. Geophys. Res. Lett. 2001, 28, 1455–1458. [Google Scholar] [CrossRef]
- Cosgrove, R.; Tsunoda, R. A direction-dependent instability of sporadic E layers in the nighttime midlatitude ionosphere. Geophys. Res. Lett. 2002, 29, 1864. [Google Scholar] [CrossRef]
- Cosgrove, R.; Tsunoda, R. Simulation of the nonlinear evolution of the sporadic-E lay-er instability in the nighttime midlatitude ionosphere. J. Geophys. Res. 2003, 108, 1283. [Google Scholar] [CrossRef]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, T.; Yang, G.; Zhou, C.; Jiang, C.; Xu, W.; Ni, B.; Zhao, Z. Improved Ionosonde Monitoring of the Sporadic E Layer Using the Frequency Domain Interferometry Technique. Remote Sens. 2022, 14, 1915. https://doi.org/10.3390/rs14081915
Liu T, Yang G, Zhou C, Jiang C, Xu W, Ni B, Zhao Z. Improved Ionosonde Monitoring of the Sporadic E Layer Using the Frequency Domain Interferometry Technique. Remote Sensing. 2022; 14(8):1915. https://doi.org/10.3390/rs14081915
Chicago/Turabian StyleLiu, Tongxin, Guobin Yang, Chen Zhou, Chunhua Jiang, Wei Xu, Binbin Ni, and Zhengyu Zhao. 2022. "Improved Ionosonde Monitoring of the Sporadic E Layer Using the Frequency Domain Interferometry Technique" Remote Sensing 14, no. 8: 1915. https://doi.org/10.3390/rs14081915
APA StyleLiu, T., Yang, G., Zhou, C., Jiang, C., Xu, W., Ni, B., & Zhao, Z. (2022). Improved Ionosonde Monitoring of the Sporadic E Layer Using the Frequency Domain Interferometry Technique. Remote Sensing, 14(8), 1915. https://doi.org/10.3390/rs14081915








