Hydraulic Fracturing Shear/Tensile/Compressive Crack Investigation Using Microseismic Data
Abstract
:1. Introduction
2. Method
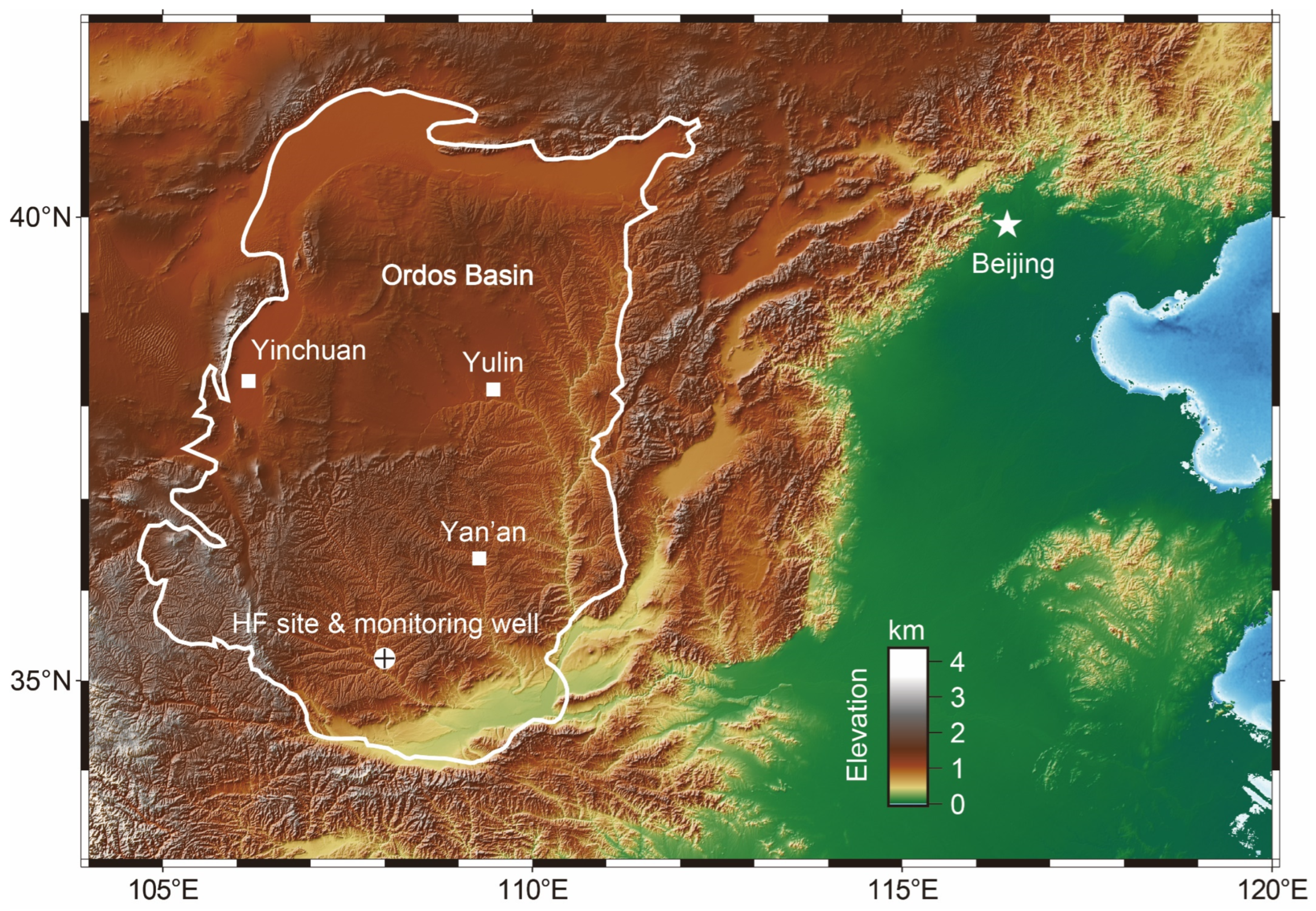
3. Data
4. Results
- (1)
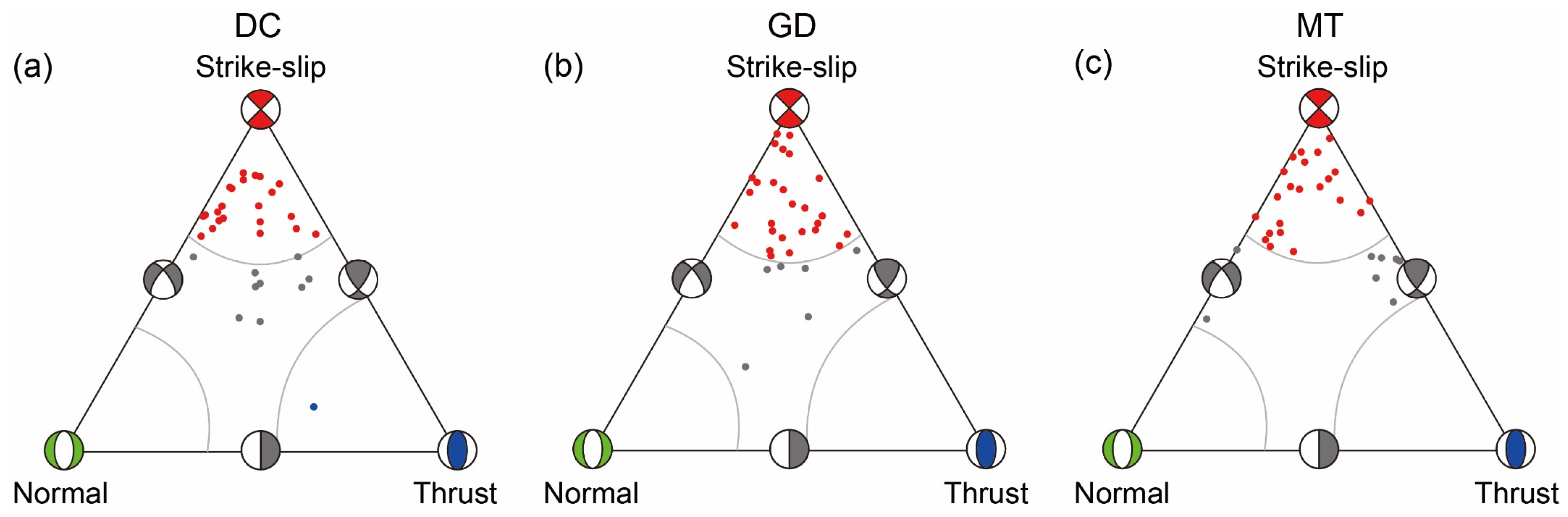
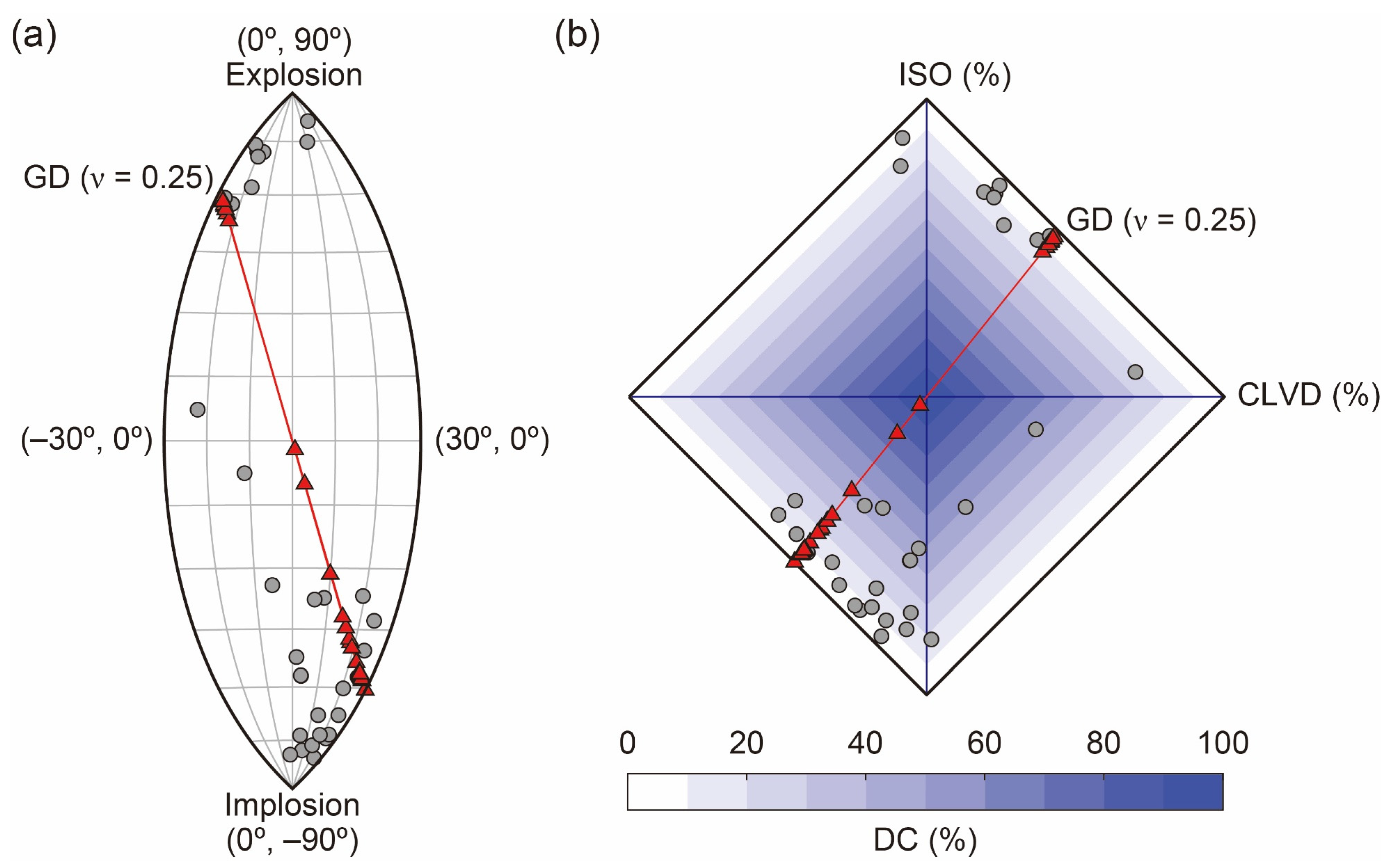
- The inverted DC or GD fault plane results of different events are similar, the nodal lines of the GD results are very close (along the northeast or southwest direction), and the consistency between MT results is worse than the DC and GD results;
- (2)
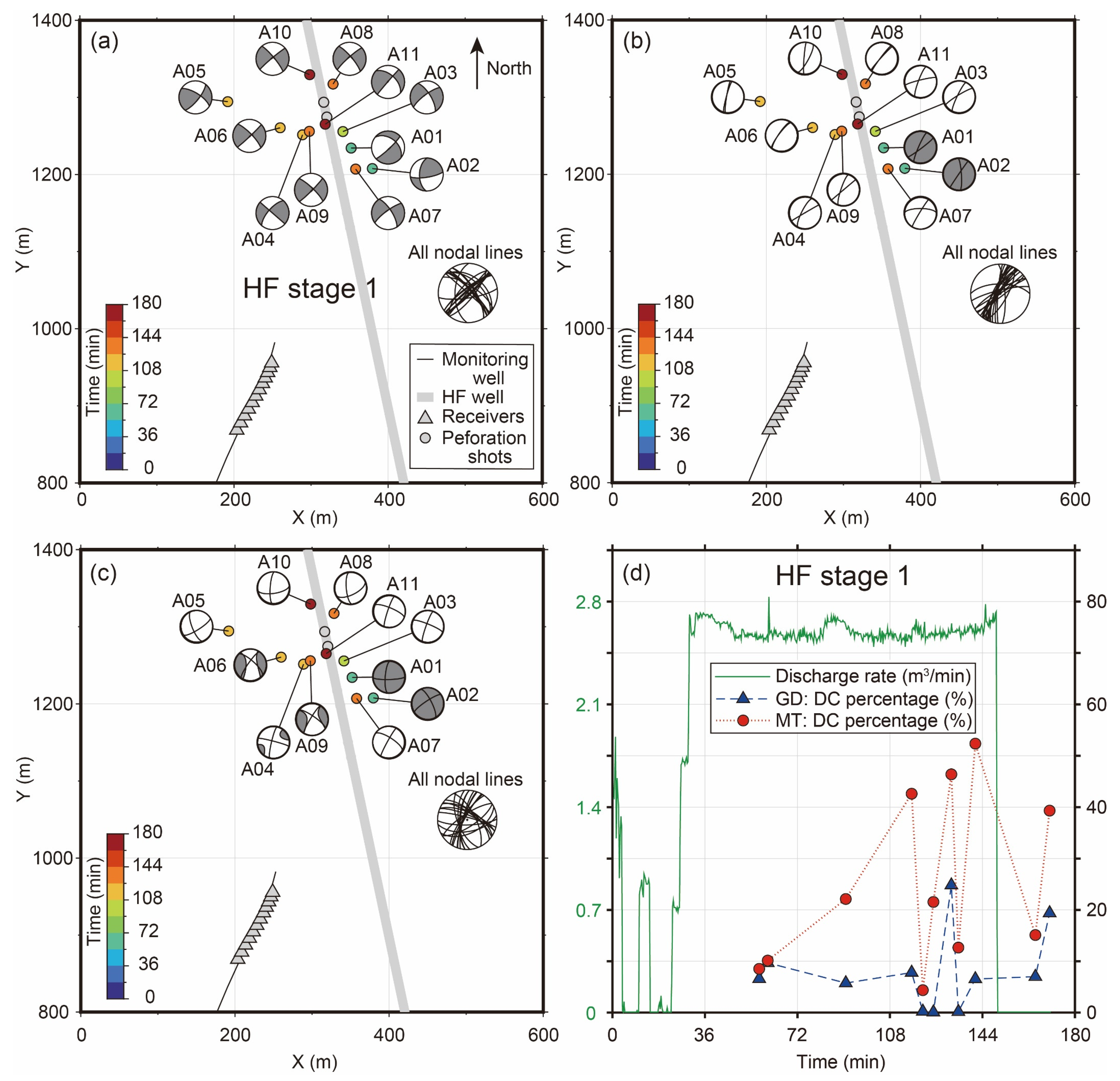
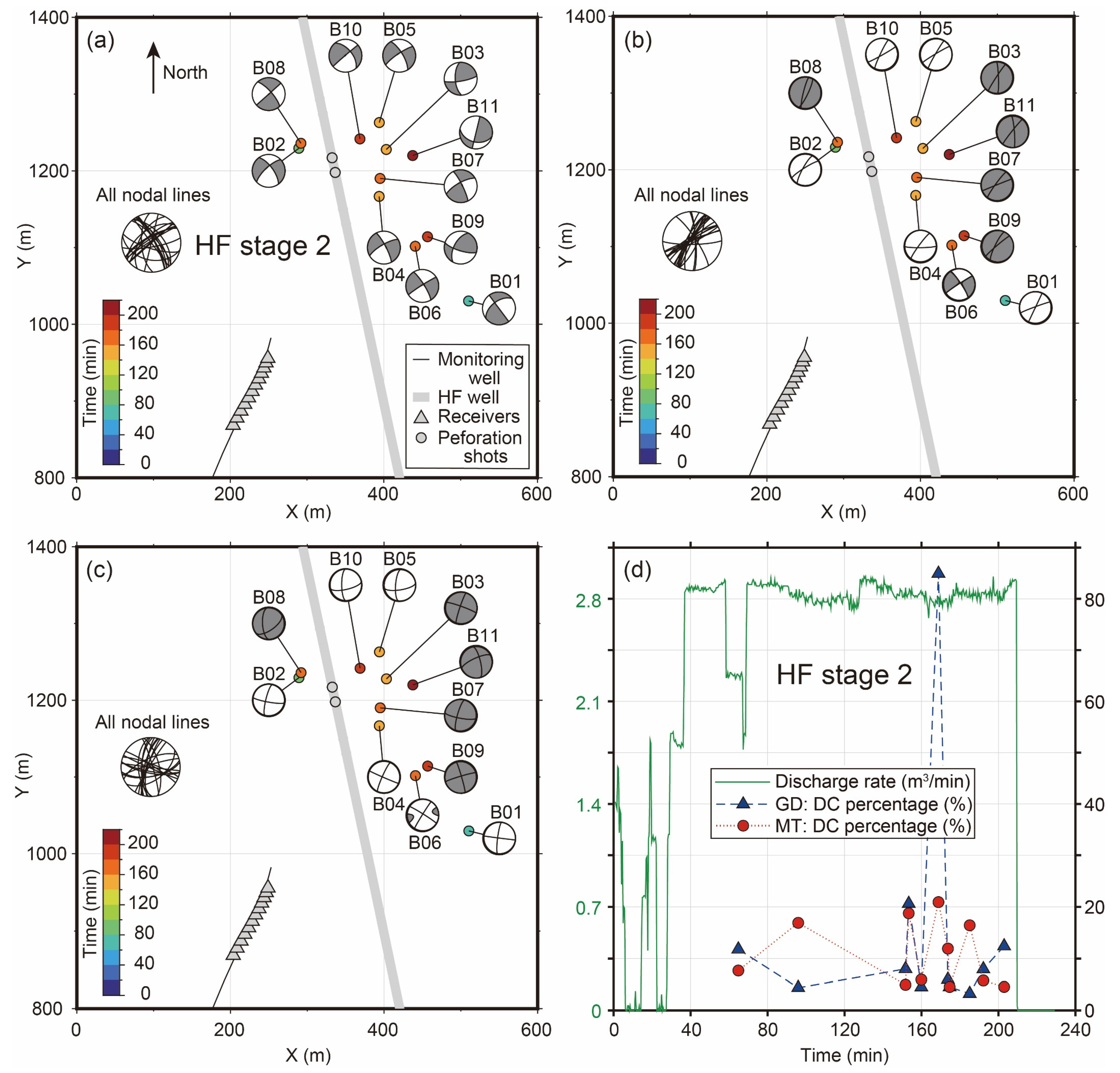
- The non-DC mechanisms (represented by the relative proportion of shadow areas in the focal mechanism plots) and DC percentages of the GD and MT results show similar tendencies over time;
- (3)
5. Discussion
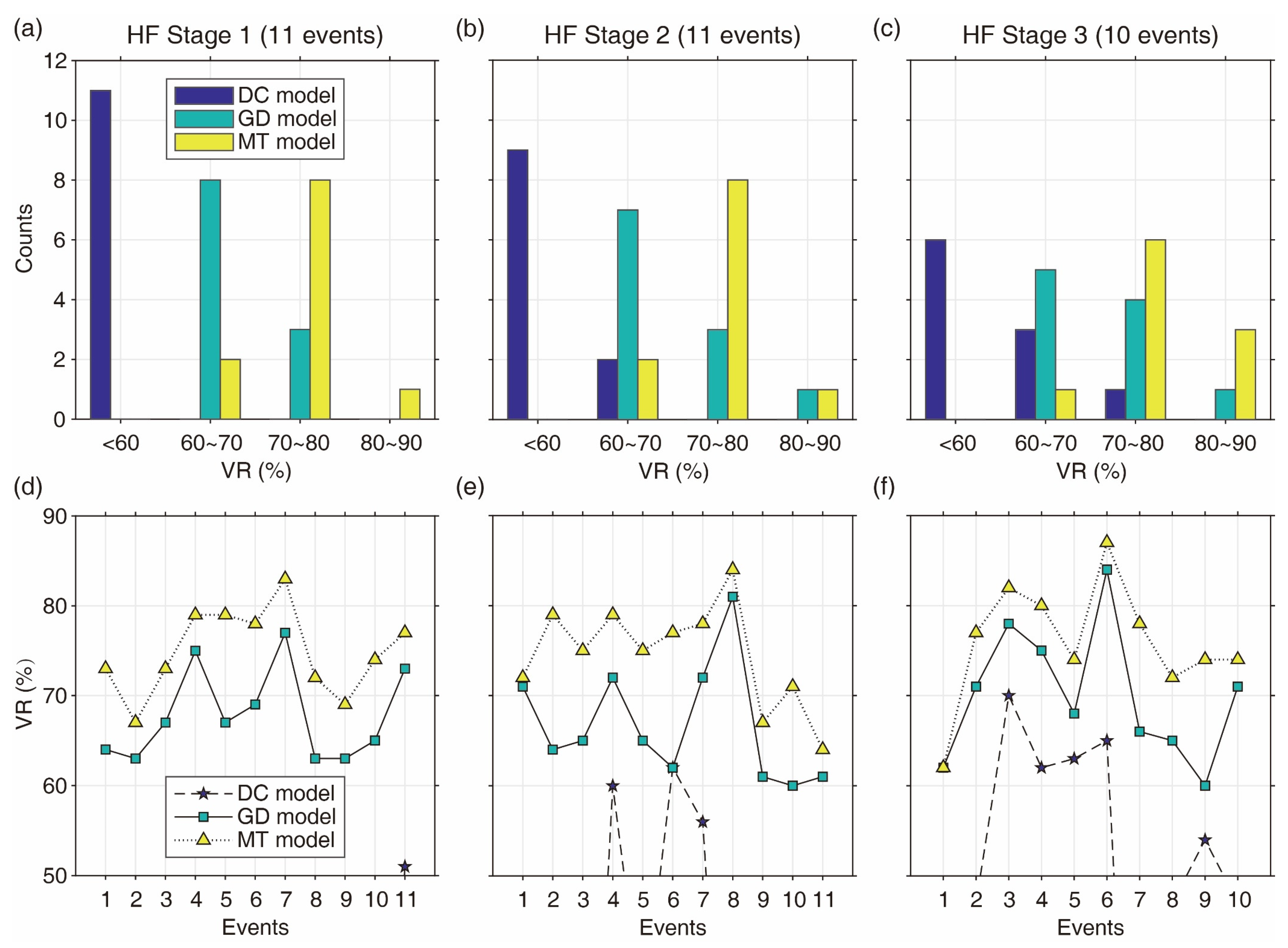
- The inversion quality (i.e., the goodness of fit between the observed data and synthetic seismograms, represented by the VR value) increases from DC → GD → MT (Figure 4), which is certain because the number of the model parameters increases from DC → GD → MT [46,47]. All the GD and MT results have VR values of ≥60%, whereas only 6 of 32 DC results have VR values in this range. Although the VR values of the MT results are better than those of the GD results, this does not mean that the MT results are certainly better. This is because: (1) the full MT inversion is not as stable as the GD inversions in our numerical tests with the same observation geometry [28] and in previous studies on acoustic emission events [26,48,49]; (2) for most of the 32 events, the difference between the VR values of the GD and MT results is not very large (see Figure 4d–f); and (3) from the GD to MT results in Figure 5 and Figure 6, the main improvement in the synthetic and observed waveform fitting (represented by the VR values) is related to the S/P amplitude ratio, which may be affected by the noise in the data.
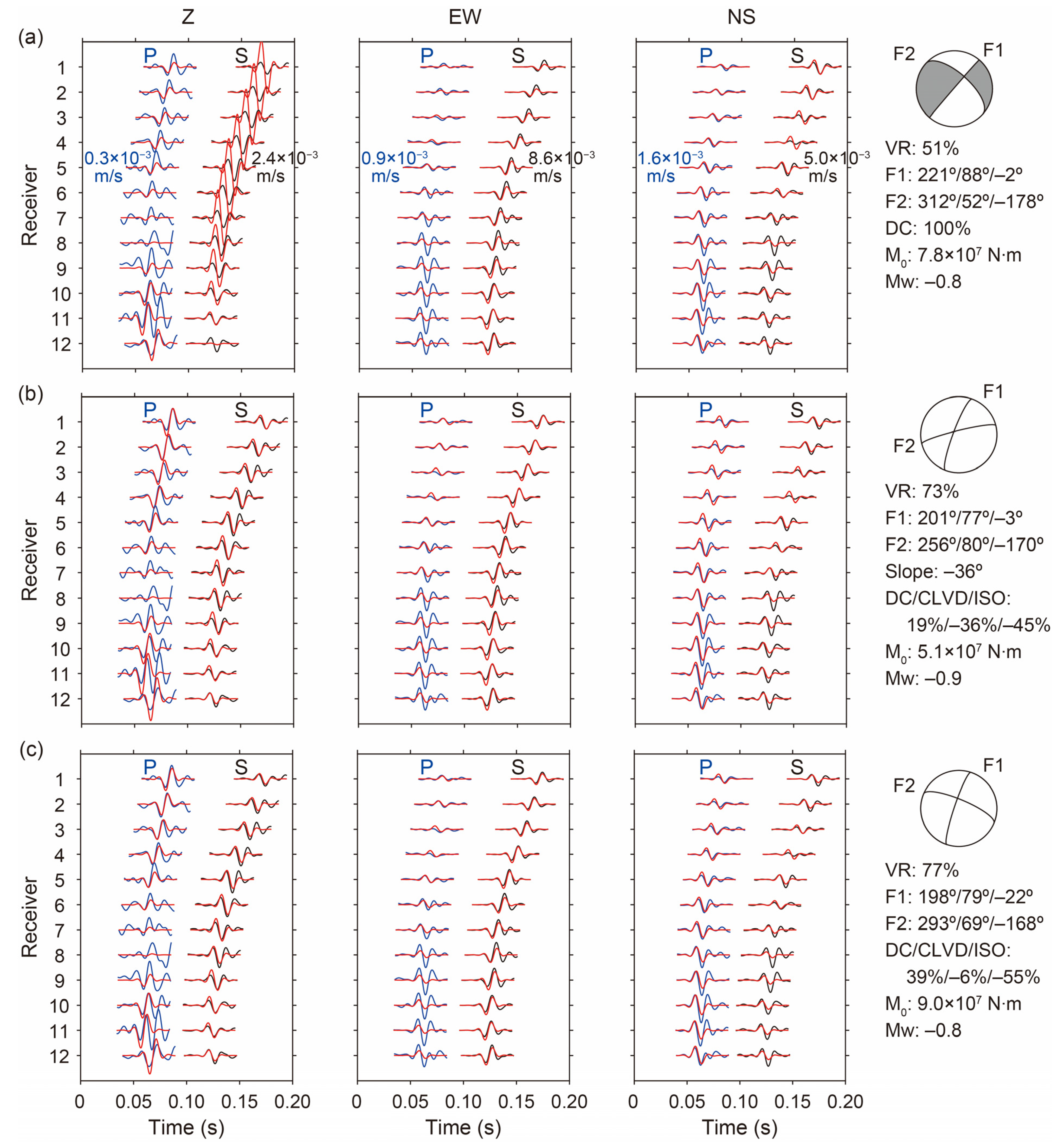
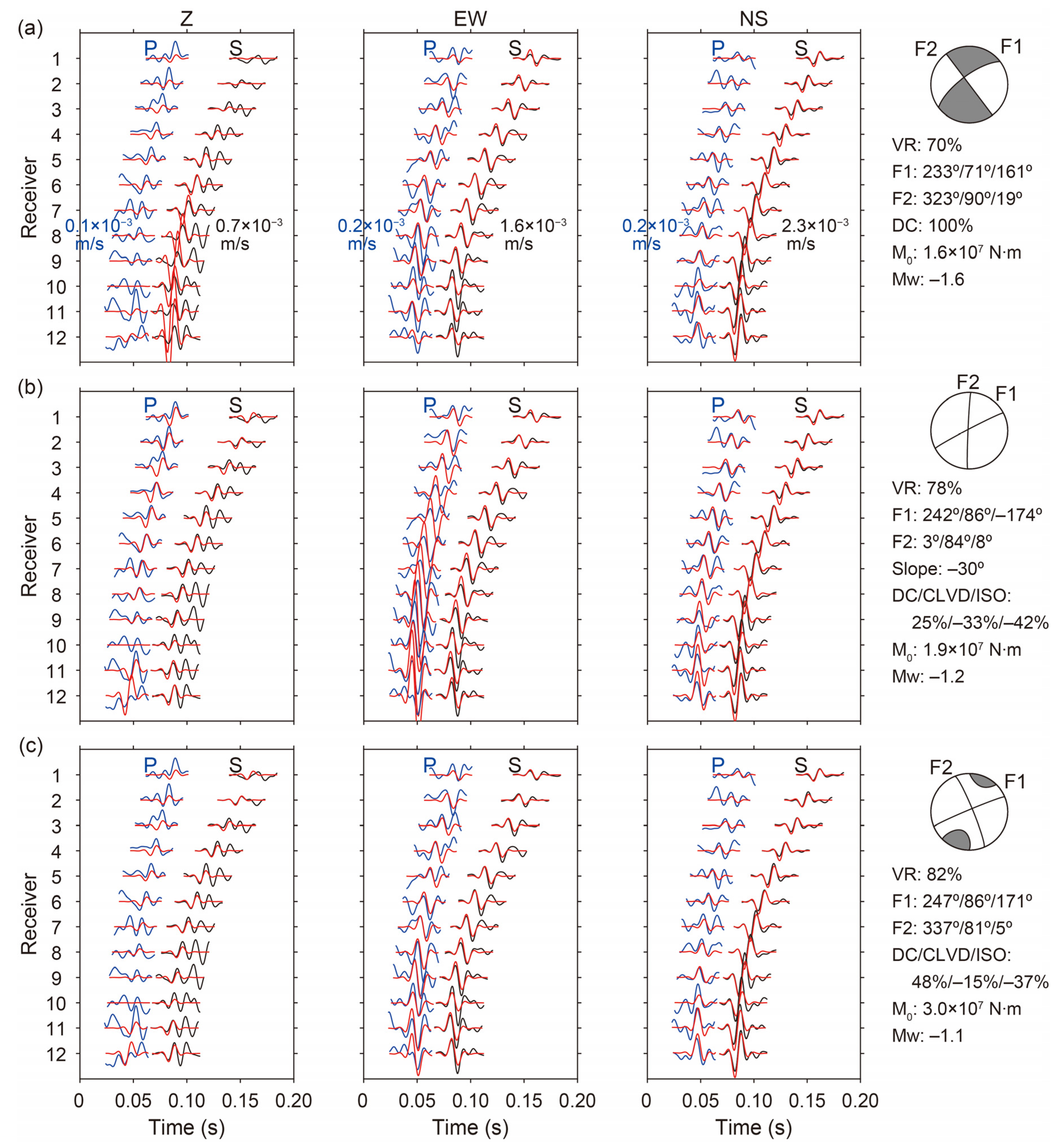
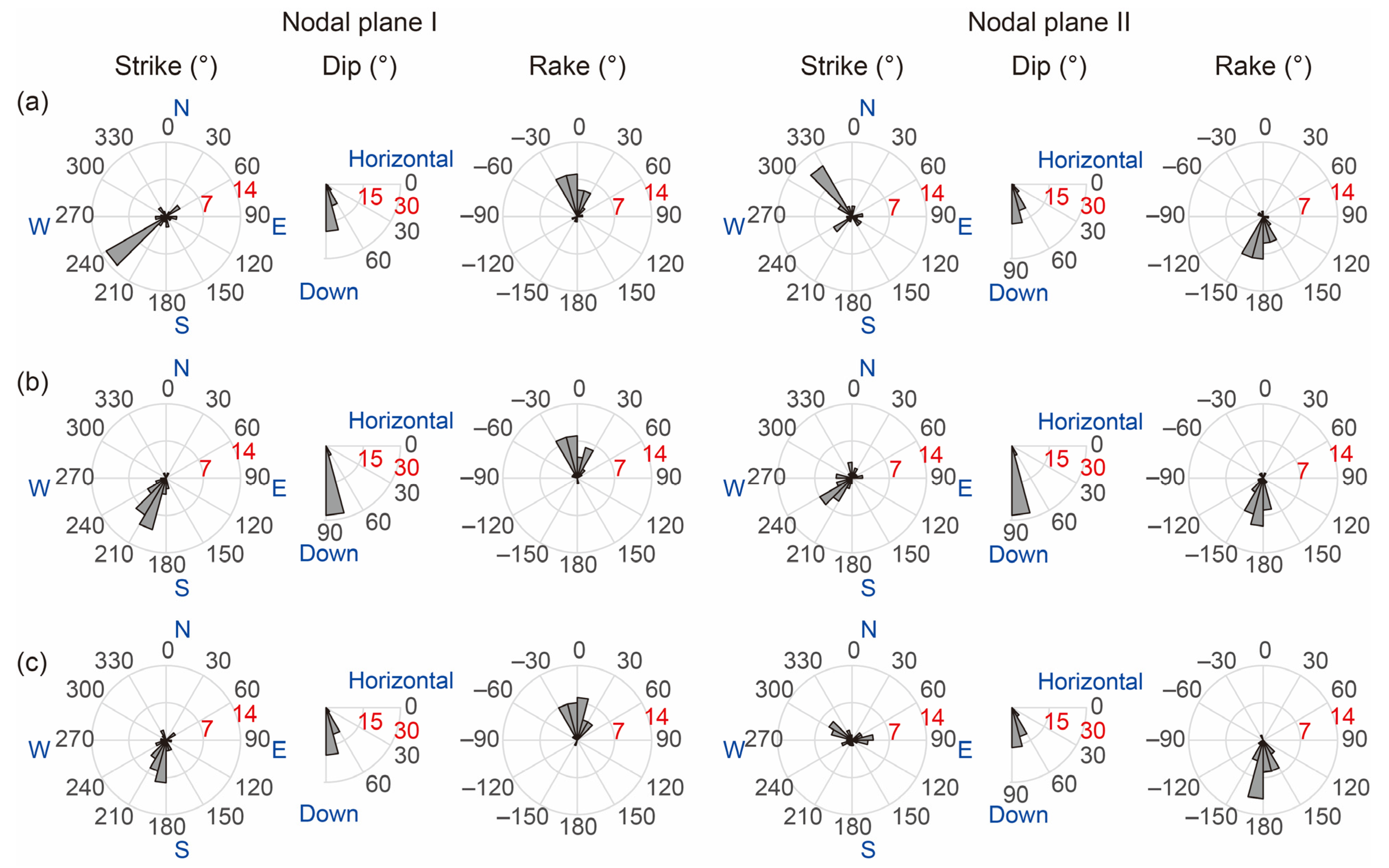
- In Figure 5, Figure 6, and Figure 10, the difference between the strike angle of nodal plane F2 (or II) from the GD results and that from the DC and MT results is quite large (>25°), whereas the other inverted parameters using these three models are similar. This may be because most of the inverted slope angles are non-zero (with –36° and –30° for events A11 and C03, respectively), and for a GD source with slope angle , the angle between its two possible nodal lines is not 90° (equal to , where is the absolute value of ; refer to Li et al. [9]). Similar observations can also be found in the nodal lines shown in Figure 7, Figure 8 and Figure 9. Taking event A06 in Figure 7b as an example, we see that its two possible nodal lines are nearly the same. From Table S1, for event A06, the inverted slope angle is –87°, then the angle between its two possible nodal lines is , and the inverted strike, dip, and rake angles of the two nodal lines are (214°, 73°, 22°) and (217°, 75°, 25°), respectively. Considering that the GD inversion is stable compared to the MT inversion in numerical tests using the same geometry [28] and the DC inversion quality for event A06 is very poor (with a VR of only –37%), we may draw the conclusion that event A06 is nearly a pure compressive crack occurring on a strike-slip fault with strike, dip, and rake angles of 214°–217°, 73°–75°, and 22°–25°, respectively. During the GD inversion, the non-zero slope angle can help to reduce the inversion uncertainty. The larger the inverted slope angle is, the smaller the angle between the two possible nodal lines (if the slope angle is nearly ±90°, the two possible nodal lines for a GD source are nearly the same [9]). According to the GD results in Figure 7, Figure 8, Figure 9, Figure 10 and Figure 11, the fracture geometry for most of the 32 events corresponds to shear/tensile/compressive strike-slip faulting, with strike angles of 195°–240° and dip angles of 75°–90°. These strike ranges are approximately perpendicular to the direction of the horizontal fracturing well. This is reasonable because during HF stimulations, the fracturing well is usually designed and set to be approximately perpendicular to the direction of the regional minimum principal stress. The inverted strike-slip fault type is also consistent with previous studies showing that strike-slip and dip-slip failures are two representative HF microseismic focal mechanisms [15,50,51].
- From the DC percentage curves in Figure 7, Figure 8 and Figure 9, we see that the maximum difference between the GD and MT results is >60% (in Figure 8d). This is consistent with previous numerical tests using the same geometry, in which the MT inversion shows a maximum deviation of ~60% in terms of DC percentages [28]. According to the GD results, most of the HF cracks have non-negligible tensile/compressive mechanisms, especially in HF stage 1; all the microseismic events occur in the order of opening cracks first and then closing cracks. In this study, we use 32 events (with signal-to-noise ratio ≥ 4) to perform focal mechanism inversion. Future work involving this study will be related to data processing of the microseismic events with signal-to-noise ratio values of 2–4, in order to study the spatial-temporal distribution of shear/tensile/compressive HF cracks and the non-DC fracture growth in detail.
6. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Maxwell, S.C.; Urbancic, T.I. The Role of Passive Microseismic Monitoring in the Instrumented Oil Field. Lead. Edge 2001, 20, 636–639. [Google Scholar] [CrossRef]
- Li, L.; Tan, J.; Wood, D.A.; Zhao, Z.; Becker, D.; Lyu, Q.; Shu, B.; Chen, H. A Review of the Current Status of Induced Seismicity Monitoring for Hydraulic Fracturing in Unconventional Tight Oil and Gas Reservoirs. Fuel 2019, 242, 195–210. [Google Scholar] [CrossRef]
- Li, H.; Chang, X. A Review of the Microseismic Focal Mechanism Research. Sci. China Earth Sci. 2021, 64, 351–363. [Google Scholar] [CrossRef]
- Wamriew, D.; Dorhjie, D.B.; Bogoedov, D.; Pevzner, R.; Maltsev, E.; Charara, M.; Pissarenko, D.; Koroteev, D. Microseismic Monitoring and Analysis Using Cutting-Edge Technology: A Key Enabler for Reservoir Characterization. Remote Sens. 2022, 14, 3417. [Google Scholar] [CrossRef]
- Ma, C.; Yan, W.; Xu, W.; Li, T.; Ran, X.; Wan, J.; Tong, K.; Lin, Y. Parallel Processing Method for Microseismic Signal Based on Deep Neural Network. Remote Sens. 2023, 15, 1215. [Google Scholar] [CrossRef]
- Baig, A.; Urbancic, T. Microseismic Moment Tensors: A Path to Understanding Frac Growth. Lead. Edge 2010, 29, 320–324. [Google Scholar] [CrossRef]
- Maxwell, S.C.; Chorney, D.; Goodfellow, S.D. Microseismic Geomechanics of Hydraulic-Fracture Networks: Insights into Mechanisms of Microseismic Sources. Lead. Edge 2015, 34, 904–910. [Google Scholar] [CrossRef]
- Vavryčuk, V. Tensile Earthquakes: Theory, Modeling, and Inversion. J. Geophys. Res. Solid Earth 2011, 116, B12320. [Google Scholar] [CrossRef]
- Li, H.; Chang, X.; Hao, J.; Wang, Y. The General Dislocation Source Model and Its Application to Microseismic Focal Mechanism Inversion. Geophysics 2021, 86, KS79–KS93. [Google Scholar] [CrossRef]
- Minson, S.E.; Dreger, D.S. Stable Inversions for Complete Moment Tensors. Geophys. J. Int. 2008, 174, 585–592. [Google Scholar] [CrossRef]
- Alvizuri, C.; Silwal, V.; Krischer, L.; Tape, C. Estimation of Full Moment Tensors, Including Uncertainties, for Nuclear Explosions, Volcanic Events, and Earthquakes. J. Geophys. Res. Solid Earth 2018, 123, 5099–5119. [Google Scholar] [CrossRef]
- Eaton, D.W.; Forouhideh, F. Solid Angles and the Impact of Receiver-Array Geometry on Microseismic Moment-Tensor Inversion. Geophysics 2011, 76, WC77–WC85. [Google Scholar] [CrossRef]
- Shang, X.; Tkalčić, H. Point-Source Inversion of Small and Moderate Earthquakes From P-wave Polarities and P/S Amplitude Ratios Within a Hierarchical Bayesian Framework: Implications for the Geysers Earthquakes. J. Geophys. Res. Solid Earth 2020, 125, e2019JB018492. [Google Scholar] [CrossRef]
- Šílený, J. Resolution of Non-Double-Couple Mechanisms: Simulation of Hypocenter Mislocation and Velocity Structure Mismodeling. Bull. Seismol. Soc. Am. 2009, 99, 2265–2272. [Google Scholar] [CrossRef]
- Staněk, F.; Eisner, L.; Jan Moser, T. Stability of Source Mechanisms Inverted from P-Wave Amplitude Microseismic Monitoring Data Acquired at the Surface. Geophys. Prospect. 2014, 62, 475–490. [Google Scholar] [CrossRef]
- Julian, B.R.; Miller, A.D.; Foulger, G.R. Non-Double-Couple Earthquakes (1) Theory. Rev. Geophys. 1998, 36, 525–549. [Google Scholar] [CrossRef]
- Eyre, T.S.; van der Baan, M. The Reliability of Microseismic Moment-Tensor Solutions: Surface versus Borehole Monitoring. Geophysics 2017, 82, KS113–KS125. [Google Scholar] [CrossRef]
- Vavryčuk, V. Inversion for Parameters of Tensile Earthquakes. J. Geophys. Res. Solid Earth 2001, 106, 16339–16355. [Google Scholar] [CrossRef]
- Šílený, J.; Jechumtálová, Z.; Dorbath, C. Small Scale Earthquake Mechanisms Induced by Fluid Injection at the Enhanced Geothermal System Reservoir Soultz (Alsace) in 2003 Using Alternative Source Models. Pure Appl. Geophys. 2014, 171, 2783–2804. [Google Scholar] [CrossRef]
- Kwiatek, G.; Ben-Zion, Y. Assessment of P and S Wave Energy Radiated from Very Small Shear-Tensile Seismic Events in a Deep South African Mine. J. Geophys. Res. Solid Earth 2013, 118, 3630–3641. [Google Scholar] [CrossRef]
- Dahm, T.; Manthei, G.; Eisenblätter, J. Automated Moment Tensor Inversion to Estimate Source Mechanisms of Hydraulically Induced Micro-Seismicity in Salt Rock. Tectonophysics 1999, 306, 1–17. [Google Scholar] [CrossRef]
- Šílený, J.; Horálek, J. Shear-Tensile Crack as a Tool for Reliable Estimates of the Non-Double-Couple Mechanism: West Bohemia-Vogtland Earthquake 1997 Swarm. Phys. Chem. Earth 2016, 95, 113–124. [Google Scholar] [CrossRef]
- Dufumier, H.; Rivera, L. On the Resolution of the Isotropic Component in Moment Tensor Inversion. Geophys. J. Int. 1997, 131, 595–606. [Google Scholar] [CrossRef]
- Tape, W.; Tape, C. The Classical Model for Moment Tensors. Geophys. J. Int. 2013, 195, 1701–1720. [Google Scholar] [CrossRef]
- Stierle, E.; Vavryčuk, V.; Šílený, J.; Bohnhoff, M. Resolution of Non-Double-Couple Components in the Seismic Moment Tensor Using Regional Networks-i: A Synthetic Case Study. Geophys. J. Int. 2014, 196, 1869–1877. [Google Scholar] [CrossRef]
- Petružálek, M.; Jechumtálová, Z.; Kolář, P.; Adamová, P.; Svitek, T.; Šílený, J.; Lokajíček, T. Acoustic Emission in a Laboratory: Mechanism of Microearthquakes Using Alternative Source Models. J. Geophys. Res. Solid Earth 2018, 123, 4965–4982. [Google Scholar] [CrossRef]
- Pesicek, J.D.; Sileny, J.; Prejean, S.G.; Thurber, C.H. Determination and Uncertainty of Moment Tensors for Microearthquakes at Okmok Volcano, Alaska. Geophys. J. Int. 2012, 190, 1689–1709. [Google Scholar] [CrossRef]
- Li, H.; Chang, X.; Hao, J.; Wang, Y. Comparison of Single-Well Microseismic Focal Mechanism Inversions with Different Source Models. Bull. Seismol. Soc. Am. 2021, 111, 3103–3117. [Google Scholar] [CrossRef]
- Aki, K.; Richards, P.G. Quantitative Seismology, 2nd ed.; University Science Books: Mill Valley, CA, USA, 2002; ISBN 0-935702-96-2. [Google Scholar]
- Ou, G. Bin Seismological Studies for Tensile Faults. Terr. Atmos. Ocean. Sci. 2008, 19, 463–471. [Google Scholar] [CrossRef]
- Langston, C.A. Source Inversion of Seismic Waveforms: The Koyna, India, Earthquakes of 13 September 1967. Bull. Seismol. Soc. Am. 1981, 71, 1–24. [Google Scholar] [CrossRef]
- Yao, Z.; Harkrider, D.G. A Generalized Reflection-Transmission Coefficient Matrix and Discrete Wavenumber Method for Synthetic Seismograms. Bull. Seismol. Soc. Am. 1983, 73, 1685–1699. [Google Scholar]
- Rothman, D.H. Automatic Estimation of Large Residual Statics Corrections. Geophysics 1986, 51, 332–346. [Google Scholar] [CrossRef]
- Yao, Z.; Ji, C. The Inverse Problem of Finite Fault Study in Time Domain. Chin. J. Geophys. 1997, 5, 691–701. [Google Scholar]
- Song, F.; Toksöz, M.N. Full-Waveform Based Complete Moment Tensor Inversion and Source Parameter Estimation from Downhole Microseismic Data for Hydrofracture Monitoring. Geophysics 2011, 76, WC103–WC116. [Google Scholar] [CrossRef]
- Jost, M.L.; Herrmann, R.B. A Student’s Guide to and Review of Moment Tensors. Seismol. Res. Lett. 1989, 60, 37–57. [Google Scholar] [CrossRef]
- Kanamori, H. The Energy Release in Great Earthquakes. J. Geophys. Res. 1977, 82, 2981–2987. [Google Scholar] [CrossRef]
- Knopoff, L.; Randall, M.J. The Compensated Linear-Vector Dipole: A Possible Mechanism for Deep Earthquakes. J. Geophys. Res. 1970, 75, 4957–4963. [Google Scholar] [CrossRef]
- Chiang, A.; Ichinose, G.A.; Dreger, D.S.; Ford, S.R.; Matzel, E.M.; Myers, S.C.; Walter, W.R. Moment Tensor Source-Type Analysis for the Democratic People’s Republic of Korea-Declared Nuclear Explosions (2006–2017) and 3 September 2017 Collapse Event. Seismol. Res. Lett. 2018, 89, 2152–2165. [Google Scholar] [CrossRef]
- Krížová, D.; Málek, J. Focal Mechanisms of West Bohemia, Central Europe, Earthquakes-End of May 2014: Evidence of Volume Changes. Seismol. Res. Lett. 2021, 92, 3398–3415. [Google Scholar] [CrossRef]
- Li, X.S.; Wu, L.P.; Yin, L.; Judd, T. An Integrated Disciplinary Approach towards Hydraulic Fracturing Optimization of Tight Oil Wells in the Ordos Basin, China. In Proceedings of the SPE Asia Pacific Unconventional Resources Conference and Exhibition, Brisbane, QLD, Australia, 11 November 2013; Volume 2, pp. 631–642. [Google Scholar]
- Hubbert, M.K.; Willis, D.G. Mechanics Of Hydraulic Fracturing. Trans. AIME 1957, 210, 153–168. [Google Scholar] [CrossRef]
- Frohlich, C. Triangle Diagrams: Ternary Graphs to Display Similarity and Diversity of Earthquake Focal Mechanisms. Phys. Earth Planet. Inter. 1992, 75, 193–198. [Google Scholar] [CrossRef]
- Tape, W.; Tape, C. A Geometric Setting for Moment Tensors. Geophys. J. Int. 2012, 190, 476–498. [Google Scholar] [CrossRef]
- Vavryčuk, V. Moment Tensor Decompositions Revisited. J. Seismol. 2015, 19, 231–252. [Google Scholar] [CrossRef]
- Menke, W. Geophysical Data Analysis: Discrete Inverse Theory; Academic press: Cambridge, MA, USA, 2018. [Google Scholar]
- Aster, R.C.; Borchers, B.; Thurber, C.H. Parameter Estimation and Inverse Problems, 3rd ed.; Elsevier: Amsterdam, The Netherlands, 2019. [Google Scholar]
- Petružálek, M.; Jechumtálová, Z.; Šílený, J.; Kolář, P.; Svitek, T.; Lokajíček, T.; Turková, I.; Kotrlý, M.; Onysko, R. Application of the Shear-Tensile Source Model to Acoustic Emissions in Westerly Granite. Int. J. Rock Mech. Min. Sci. 2020, 128, 104246. [Google Scholar] [CrossRef]
- Ren, Y.; Vavryčuk, V.; Wu, S.; Gao, Y. Accurate Moment Tensor Inversion of Acoustic Emissions and Its Application to Brazilian Splitting Test. Int. J. Rock Mech. Min. Sci. 2021, 141, 104707. [Google Scholar] [CrossRef]
- Rutledge, J.T.; Phillips, W.S. Hydraulic Stimulation of Natural Fractures as Revealed by Induced Microearthquakes, Carthage Cotton Valley Gas Field, East Texas. Geophysics 2003, 68, 441–452. [Google Scholar] [CrossRef]
- Eisner, L.; Williams-Stroud, S.; Hill, A.; Duncan, P.; Thornton, M. Beyond the Dots in the Box: Microseismicity-Constrained Fracture Models for Reservoir Simulation. Lead. Edge 2010, 29, 326–333. [Google Scholar] [CrossRef]
- Tape, W.; Tape, C. Angle between Principal Axis Triples. Geophys. J. Int. 2012, 191, 813–831. [Google Scholar] [CrossRef]
- Tape, W.; Tape, C. A Uniform Parametrization of Moment Tensors. Geophys. J. Int. 2015, 202, 2074–2081. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, H.; Chang, X.; Hao, J. Hydraulic Fracturing Shear/Tensile/Compressive Crack Investigation Using Microseismic Data. Remote Sens. 2024, 16, 1902. https://doi.org/10.3390/rs16111902
Li H, Chang X, Hao J. Hydraulic Fracturing Shear/Tensile/Compressive Crack Investigation Using Microseismic Data. Remote Sensing. 2024; 16(11):1902. https://doi.org/10.3390/rs16111902
Chicago/Turabian StyleLi, Han, Xu Chang, and Jinlai Hao. 2024. "Hydraulic Fracturing Shear/Tensile/Compressive Crack Investigation Using Microseismic Data" Remote Sensing 16, no. 11: 1902. https://doi.org/10.3390/rs16111902
APA StyleLi, H., Chang, X., & Hao, J. (2024). Hydraulic Fracturing Shear/Tensile/Compressive Crack Investigation Using Microseismic Data. Remote Sensing, 16(11), 1902. https://doi.org/10.3390/rs16111902




