The Effect of Oil Viscosity on Droplet Generation Rate and Droplet Size in a T-Junction Microfluidic Droplet Generator
Abstract
1. Introduction
2. Materials and Methods
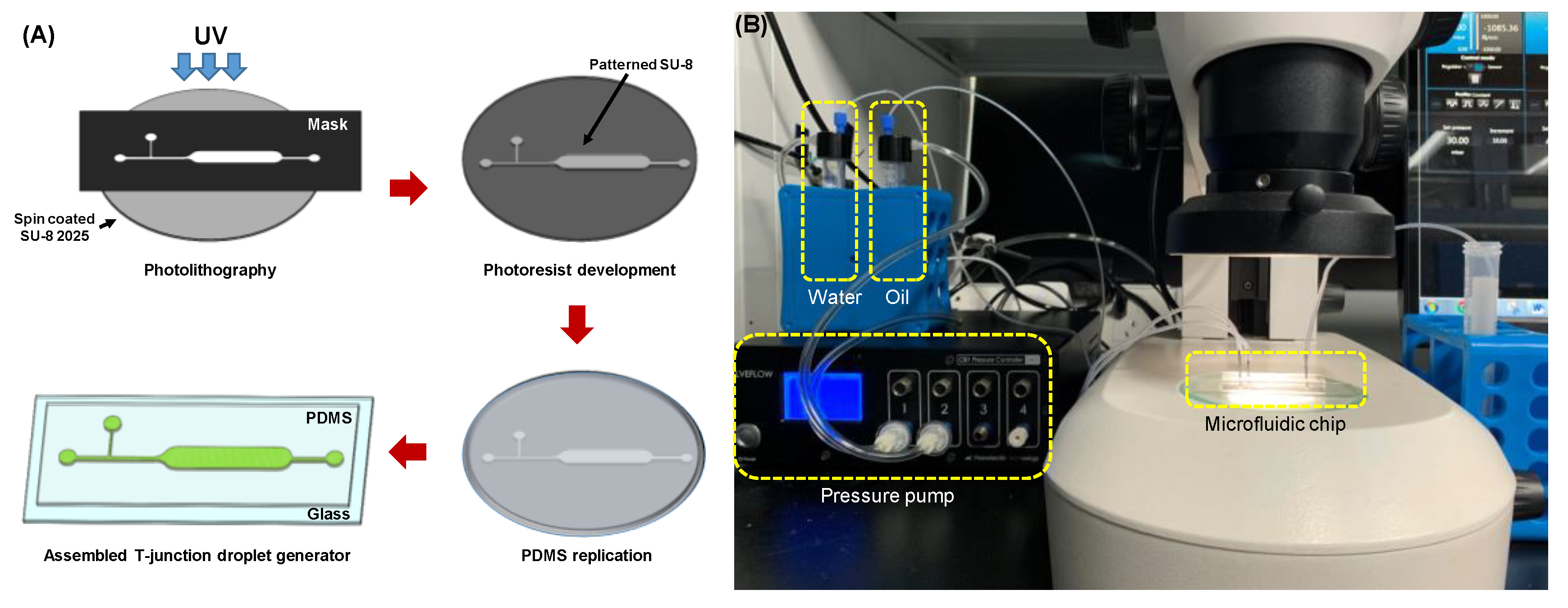
2.1. Design and Fabrication
2.2. Droplet Generation
2.3. Data Analysis
3. Results and Discussion
3.1. Effect of the Continuous Phase Viscosity on Droplet Sizes
3.2. Effect of the Continuous Phase Viscosity on Droplet Generation Rates
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Seemann, R.; Brinkmann, M.; Pfohl, T.; Herminghaus, S. Droplet based microfluidics. Rep. Prog. Phys. 2012, 75, 016601–016645. [Google Scholar] [CrossRef] [PubMed]
- Rakszewska, A.; Tel, J.; Chokkalingam, V.; Huck, W.T.S. One drop at a time: Toward droplet microfluidics as a versatile tool for single-cell analysis. NPG Asia Mater. 2014, 6, e133. [Google Scholar] [CrossRef]
- Kim, H.S.; Guzman, A.R.; Thapa, H.R.; Devarenne, T.P.; Han, A. A droplet microfluidics platform for rapid microalgal growth and oil production analysis. Biotechnol. Bioeng. 2016, 113, 1691–1701. [Google Scholar] [CrossRef] [PubMed]
- Han, S.I.; Kim, H.S.; Han, A. In-droplet cell concentration using dielectrophoresis. Biosens. Bioelectron. 2017, 97, 41–45. [Google Scholar] [CrossRef] [PubMed]
- Mazutis, L.; Gilbert, J.; Ung, W.L.; Weitz, D.A.; Griffiths, A.D.; Heyman, J.A. Single-cell analysis and sorting using droplet-based microfluidics. Nat. Protoc. 2013, 8, 870–891. [Google Scholar] [CrossRef]
- Brouzes, E.; Medkova, M.; Savenelli, N.; Marran, D.; Twardowski, M.; Hutchison, J.B.; Rothberg, J.M.; Link, D.R.; Perrimon, N.; Samuels, M.L. Droplet microfluidic technology for single-cell high-throughput screening. Proc. Natl. Acad. Sci. USA 2009, 106, 14195–14200. [Google Scholar] [CrossRef]
- Kim, H.S.; Hsu, S.-C.; Han, S.I.; Thapa, H.R.; Guzman, A.R.; Browne, D.R.; Tatli, M.; Devarenne, T.P.; Stern, D.B.; Han, A. High-throughput droplet microfluidics screening platform for selecting fast-growing and high lipid-producing microalgae from a mutant library. Plant Direct 2017, 1, e00011. [Google Scholar] [CrossRef]
- Kim, H.S.; Waqued, S.C.; Nodurft, D.T.; Devarenne, T.P.; Yakovlev, V.V.; Han, A. Raman spectroscopy compatible PDMS droplet microfluidic culture and analysis platform towards on-chip lipidomics. Analyst 2017, 142, 1054–1060. [Google Scholar] [CrossRef]
- Pinho, D.; Muñoz-Sánchez, B.N.; Anes, C.F.; Vega, E.J.; Lima, R. Flexible PDMS microparticles to mimic RBCs in blood particulate analogue fluids. Mech. Res. Commun. 2019, 100, 103399. [Google Scholar] [CrossRef]
- Zheng, B.; Tice, J.D.; Roach, L.S.; Ismagilov, R.F. A droplet-based, composite PDMS/glass capillary microfluidic system for evaluating protein crystallization conditions by microbatch and vapor-diffusion methods with on-chip X-ray diffraction. Angew. Chem. Int. Edit. 2004, 43, 2508–2511. [Google Scholar] [CrossRef]
- Markey, A.L.; Mohr, S.; Day, P.J.R. High-throughput droplet PCR. Methods 2010, 50, 277–281. [Google Scholar] [CrossRef] [PubMed]
- Shembekar, N.; Chaipan, C.; Utharala, R.; Merten, C.A. Droplet-based microfluidics in drug discovery, transcriptomics and high-throughput molecular genetics. Lab Chip 2016, 16, 1314–1331. [Google Scholar] [CrossRef] [PubMed]
- Munoz-Sanchez, B.N.; Silva, S.F.; Pinho, D.; Vega, E.J.; Lima, R. Generation of micro-sized PDMS particles by a flow focusing technique for biomicrofluidics applications. Biomicrofluidics 2016, 10, 014122. [Google Scholar] [CrossRef] [PubMed]
- Anes, C.F.; Pinho, D.; Munoz-Sanchez, B.N.; Vega, E.J.; Lima, R. Shrinkage and colour in the production of micro-sized PDMS particles for microfluidic applications. J. Micromech. Microeng. 2018, 28, 075002. [Google Scholar] [CrossRef]
- Shi, W.; Qin, J.; Ye, N.; Lin, B. Droplet-based microfluidic system for individual Caenorhabditis elegans assay. Lab Chip 2008, 8, 1432–1435. [Google Scholar] [CrossRef]
- Leung, K.; Zahn, H.; Leaver, T.; Konwar, K.M.; Hanson, N.W.; Pagé, A.P.; Lo, C.-C.; Chain, P.S.; Hallam, S.J.; Hansen, C.L. A programmable droplet-based microfluidic device applied to multiparameter analysis of single microbes and microbial communities. Proc. Natl. Acad. Sci. USA 2012, 109, 7665–7670. [Google Scholar] [CrossRef]
- Seo, M.; Paquet, C.; Nie, Z.; Xu, S.; Kumacheva, E. Microfluidic consecutive flow-focusing droplet generators. Soft Matter 2007, 3, 986–992. [Google Scholar] [CrossRef]
- Hong, Y.; Wang, F. Flow rate effect on droplet control in a co-flowing microfluidic device. Microfluid. Nanofluid. 2006, 3, 341–346. [Google Scholar] [CrossRef]
- Romero, P.A.; Abate, A.R. Flow focusing geometry generates droplets through a plug and squeeze mechanism. Lab Chip 2012, 12, 5130–5132. [Google Scholar] [CrossRef]
- Gupta, A.; Murshed, S.M.S.; Kumar, R. Droplet formation and stability of flows in a microfluidic T-junction. Appl. Phys. Lett. 2009, 94, 164107. [Google Scholar] [CrossRef]
- Gupta, A.; Kumar, R. Flow regime transition at high capillary numbers in a microfluidic T-junction: Viscosity contrast and geometry effect. Phys. Fluids 2010, 22, 122001. [Google Scholar] [CrossRef]
- He, P.; Barthès-Biesel, D.; Leclerc, E. Flow of two immiscible liquids with low viscosity in Y shaped microfluidic systems: Effect of geometry. Microfluid. Nanofluid. 2009, 9, 293–301. [Google Scholar] [CrossRef]
- Opalski, A.S.; Kaminski, T.S.; Garstecki, P. Droplet microfluidics as a tool for the generation of granular matters and functional emulsions. KONA Powder Part. J. 2019, 36, 50–71. [Google Scholar] [CrossRef]
- Teigen, K.E.; Song, P.; Lowengrub, J.; Voigt, A. A diffuse-interface method for two-phase flows with soluble surfactants. J. Comput. Phys. 2011, 230, 375–393. [Google Scholar] [CrossRef] [PubMed]
- Garstecki, P.; Fuerstman, M.J.; Stone, H.A.; Whitesides, G.M. Formation of droplets and bubbles in a microfluidic T-junction—scaling and mechanism of break-up. Lab Chip 2006, 6, 437–446. [Google Scholar] [CrossRef] [PubMed]
- Jamalabadi, M.Y.A.; DaqiqShirazi, M.; Kosar, A.; Shadloo, M.S. Effect of injection angle, density ratio, and viscosity on droplet formation in a microfluidic T-junction. Theor. Appl. Mech. Lett. 2017, 7, 243–251. [Google Scholar] [CrossRef]
- Teh, S.Y.; Lin, R.; Hung, L.-H.; Lee, A. Droplet microfluidics. Lab Chip 2008, 8, 198–220. [Google Scholar] [CrossRef] [PubMed]
- Zeng, W.; Li, S.; Wang, Z. Characterization of syringe-pump-driven versus pressure-driven microfluidic flows. In Proceedings of the 2015 International Conference on Fluid Power and Mechatronics, Harbin, China, 5–7 August 2015. [Google Scholar]
- Sutera, S.P.; Skalak, R. The history of Poiseuille’s law. Annu. Rev. Fluid Mech. 1993, 25, 1–20. [Google Scholar] [CrossRef]
- Loizou, K.; Wong, V.-L.; Hewakandamby, B. Examining the effect of flow rate ratio on droplet generation and regime transition in a microfluidic T-junction at constant capillary numbers. Inventions 2018, 3, 54. [Google Scholar] [CrossRef]


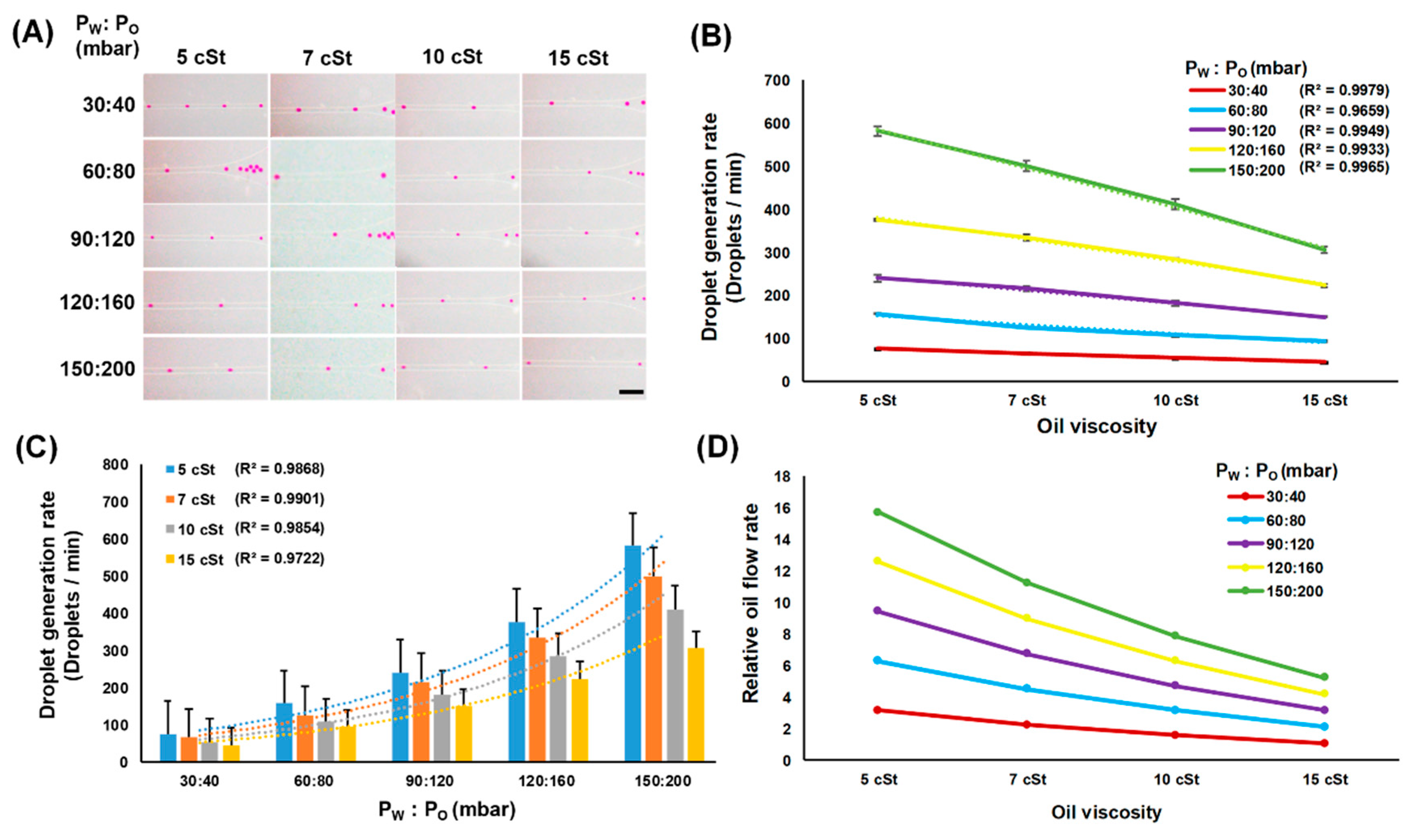
| PW:PO (mbar) | 5 cSt | 7 cSt | 10 cSt | 15 cSt |
|---|---|---|---|---|
| 30:40 | 68.3 ± 2.0 | 57.0 ± 1.7 | 51.0 ± 1.7 | 46.3 ± 1.8 |
| 60:80 | 43.6 ± 2.1 | 39.5 ± 1.8 | 35.5 ± 1.7 | 32.2 ± 0.9 |
| 90:120 | 37.1 ± 1.8 | 33.4 ± 2.0 | 31.0 ± 1.0 | 28.9 ± 0.9 |
| 120:160 | 34.0 ± 1.9 | 30.7 ± 0.9 | 28.1 ± 1.0 | 26.0 ± 1.1 |
| 150:200 | 32.1 ± 1.0 | 29.1 ± 1.1 | 26.9 ± 1.1 | 25.1 ± 1.3 |
| PW:PO (mbar) | 5 cSt | 7 cSt | 10 cSt | 15 cSt |
|---|---|---|---|---|
| 30:40 | 76 ± 1 | 66 ± 1 | 54 ± 1 | 45 ± 0 |
| 60:80 | 157 ± 0 | 126 ± 2 | 107 ± 3 | 93 ± 2 |
| 90:120 | 239 ± 8 | 215 ± 6 | 182 ± 6 | 149 ± 1 |
| 120:160 | 375 ± 3 | 334 ± 6 | 283 ± 3 | 223 ± 3 |
| 150:200 | 581 ± 12 | 499 ± 12 | 411 ± 12 | 305 ± 8 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yao, J.; Lin, F.; Kim, H.S.; Park, J. The Effect of Oil Viscosity on Droplet Generation Rate and Droplet Size in a T-Junction Microfluidic Droplet Generator. Micromachines 2019, 10, 808. https://doi.org/10.3390/mi10120808
Yao J, Lin F, Kim HS, Park J. The Effect of Oil Viscosity on Droplet Generation Rate and Droplet Size in a T-Junction Microfluidic Droplet Generator. Micromachines. 2019; 10(12):808. https://doi.org/10.3390/mi10120808
Chicago/Turabian StyleYao, Junyi, Fan Lin, Hyun Soo Kim, and Jaewon Park. 2019. "The Effect of Oil Viscosity on Droplet Generation Rate and Droplet Size in a T-Junction Microfluidic Droplet Generator" Micromachines 10, no. 12: 808. https://doi.org/10.3390/mi10120808
APA StyleYao, J., Lin, F., Kim, H. S., & Park, J. (2019). The Effect of Oil Viscosity on Droplet Generation Rate and Droplet Size in a T-Junction Microfluidic Droplet Generator. Micromachines, 10(12), 808. https://doi.org/10.3390/mi10120808






