Ultra-Precision Cutting and Characterization of Reflective Convex Spherical Blazed Grating Elements
Abstract
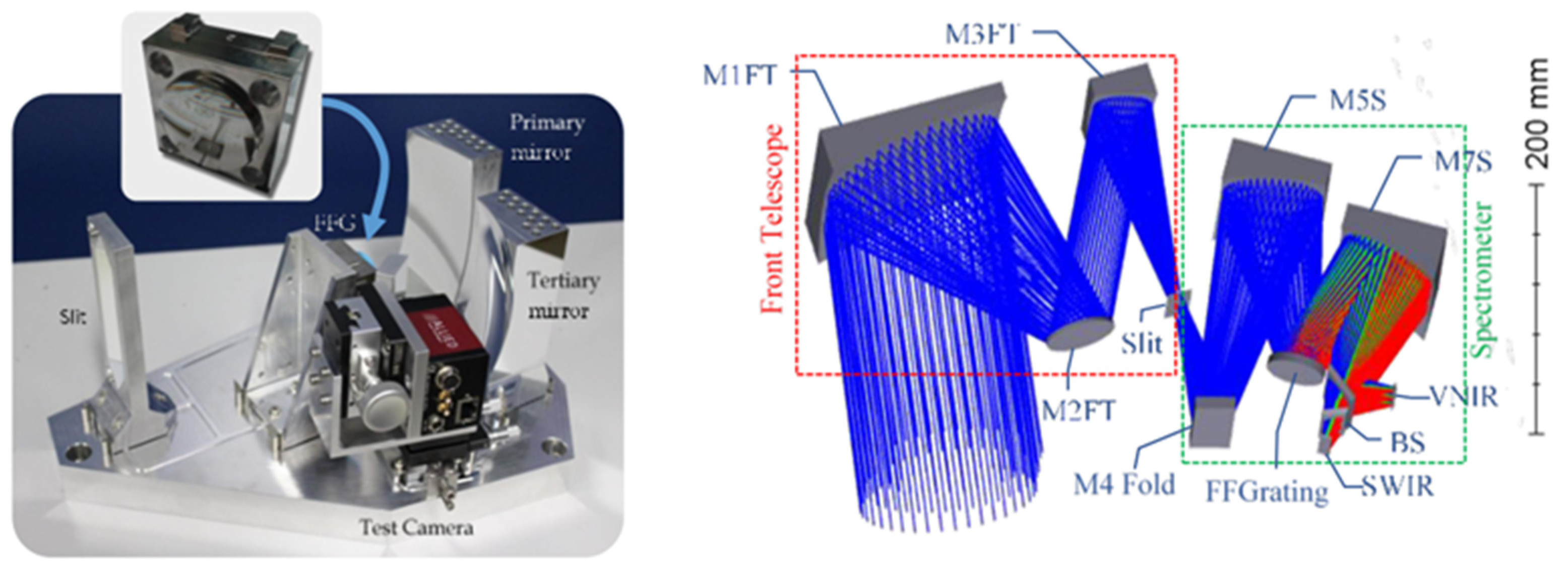
:1. Introduction
2. Reflective Blazed Grating Technical Requirements
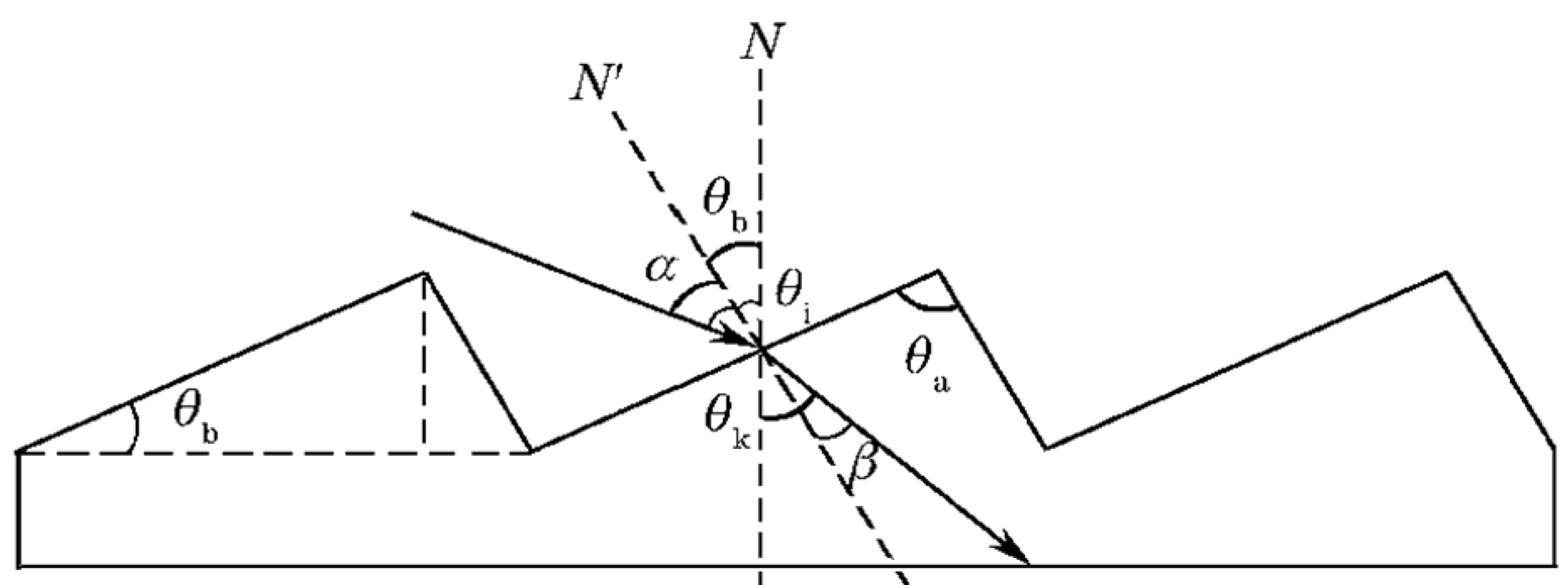
2.1. Diffraction Principle of Blazed Grating
2.2. Accuracy Requirements of Convex Spherical Blazed Grating
2.2.1. Dimensional and Surface Accuracy
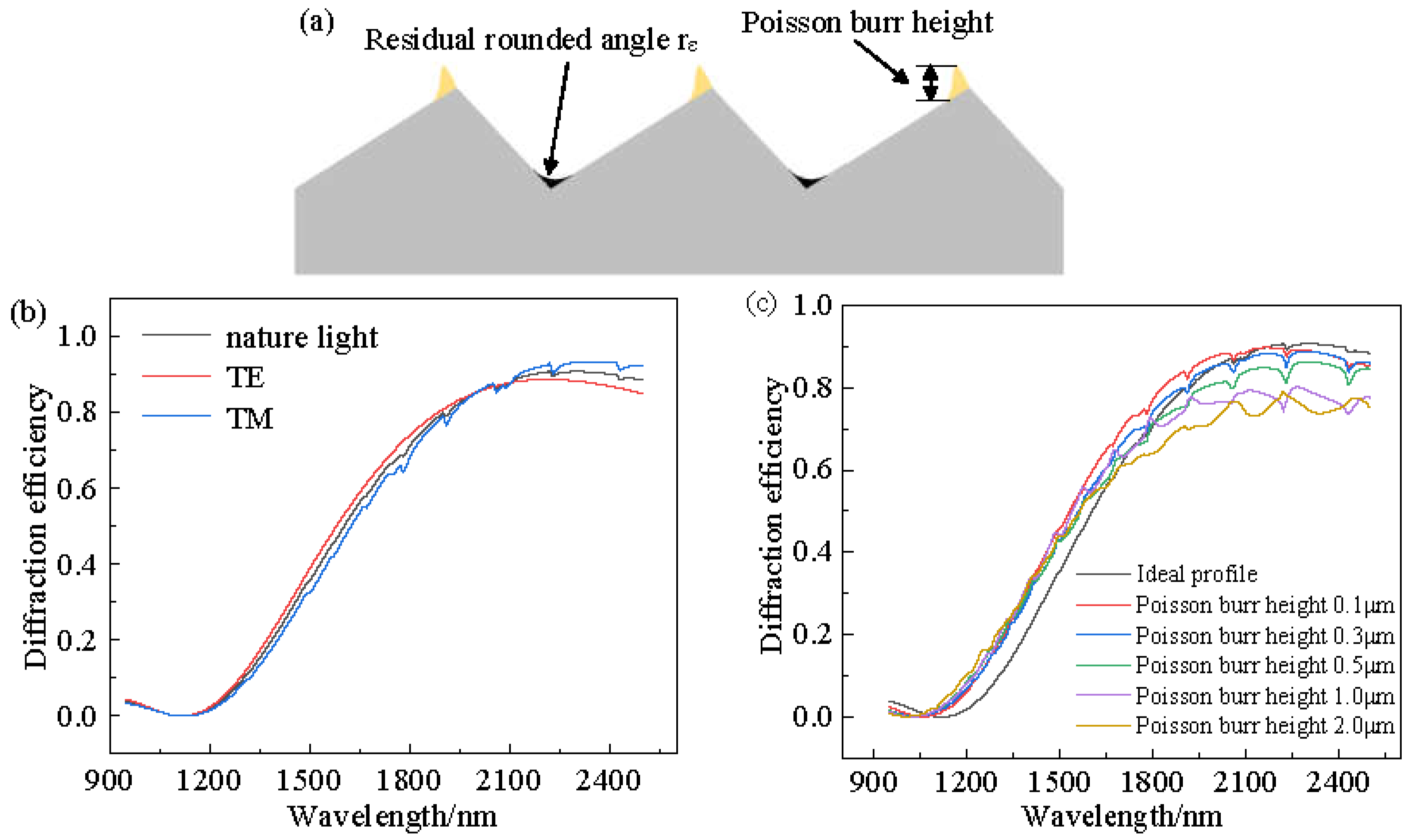
2.2.2. Effect of Shape Accuracy on Diffraction Efficiency
3. Cutting Experiments for Convex Spherical Blazed Grating
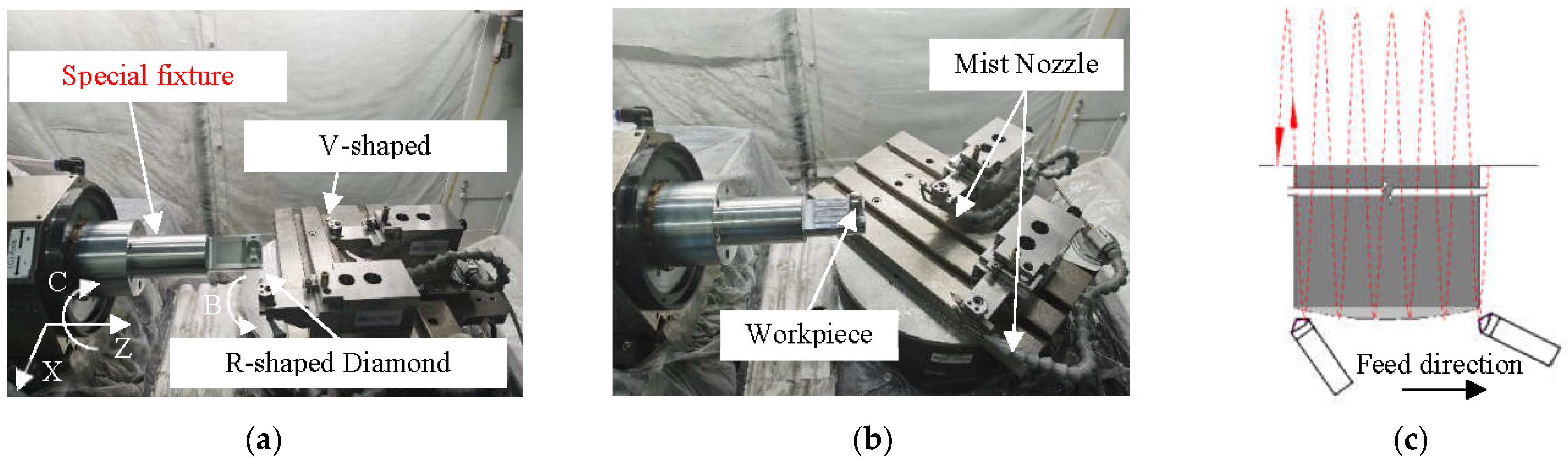
3.1. Machining Equipment and Tools
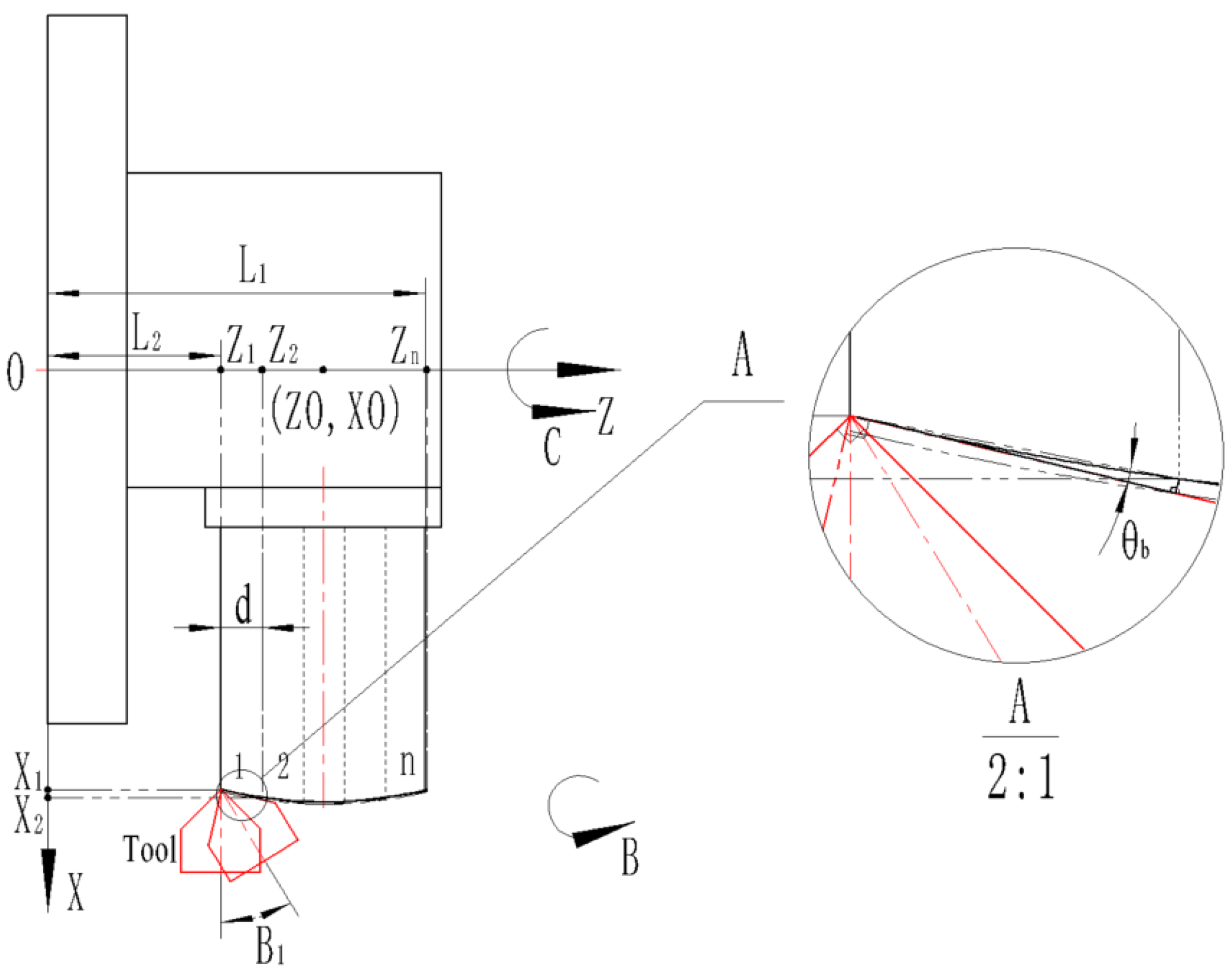
3.2. Machining Path Planning
3.3. Cutting Parameter Selection
4. Cutting Quality Characterization for Convex Spherical Blazed Grating
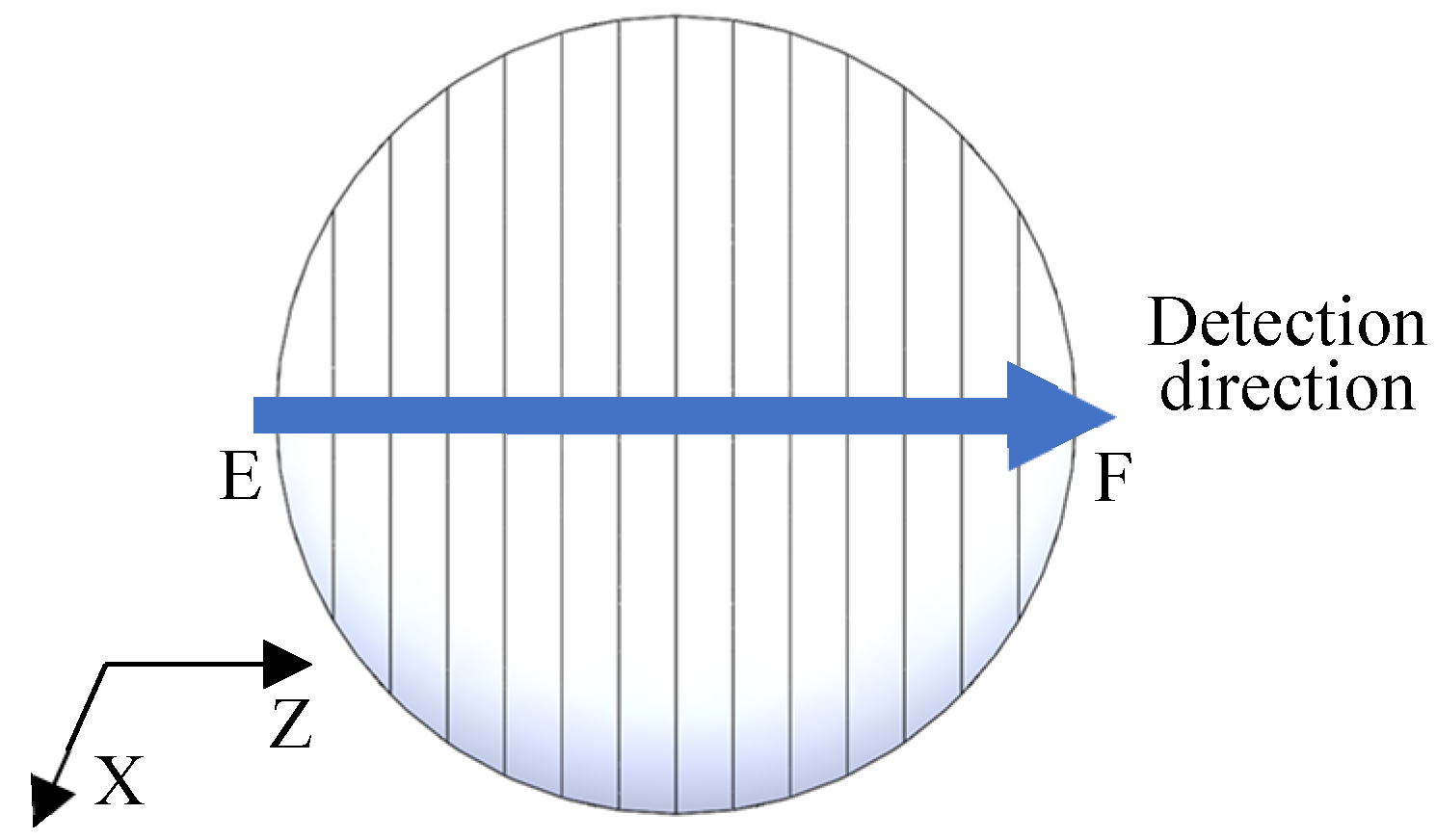
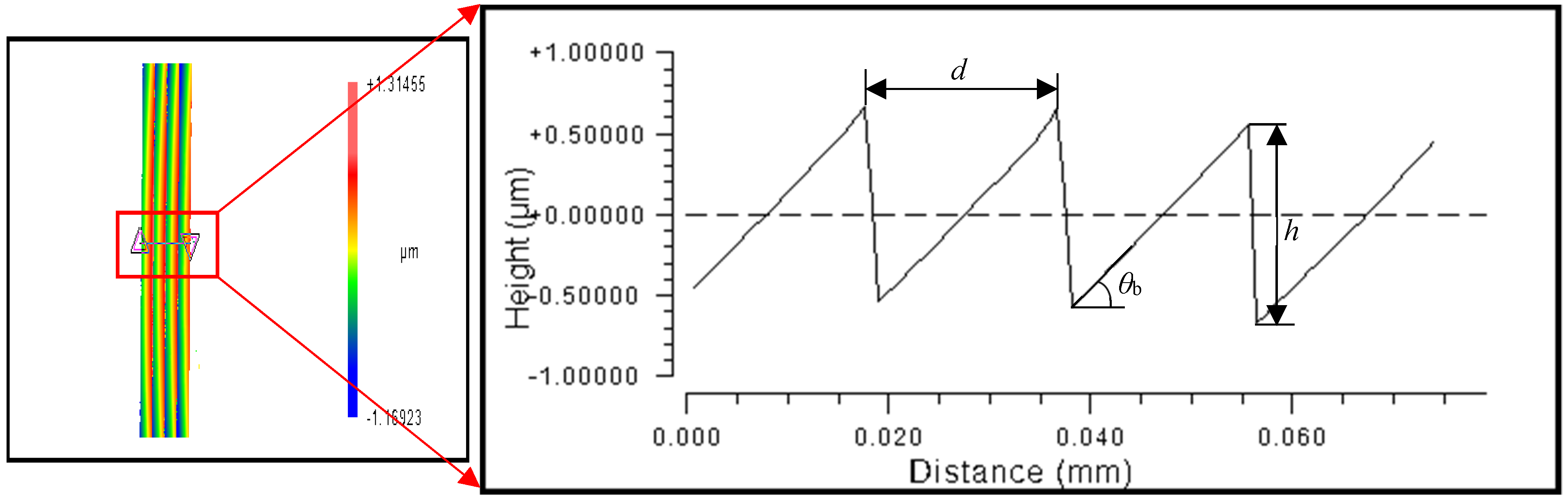
4.1. Surface Roughness Distribution in the Diameter Direction
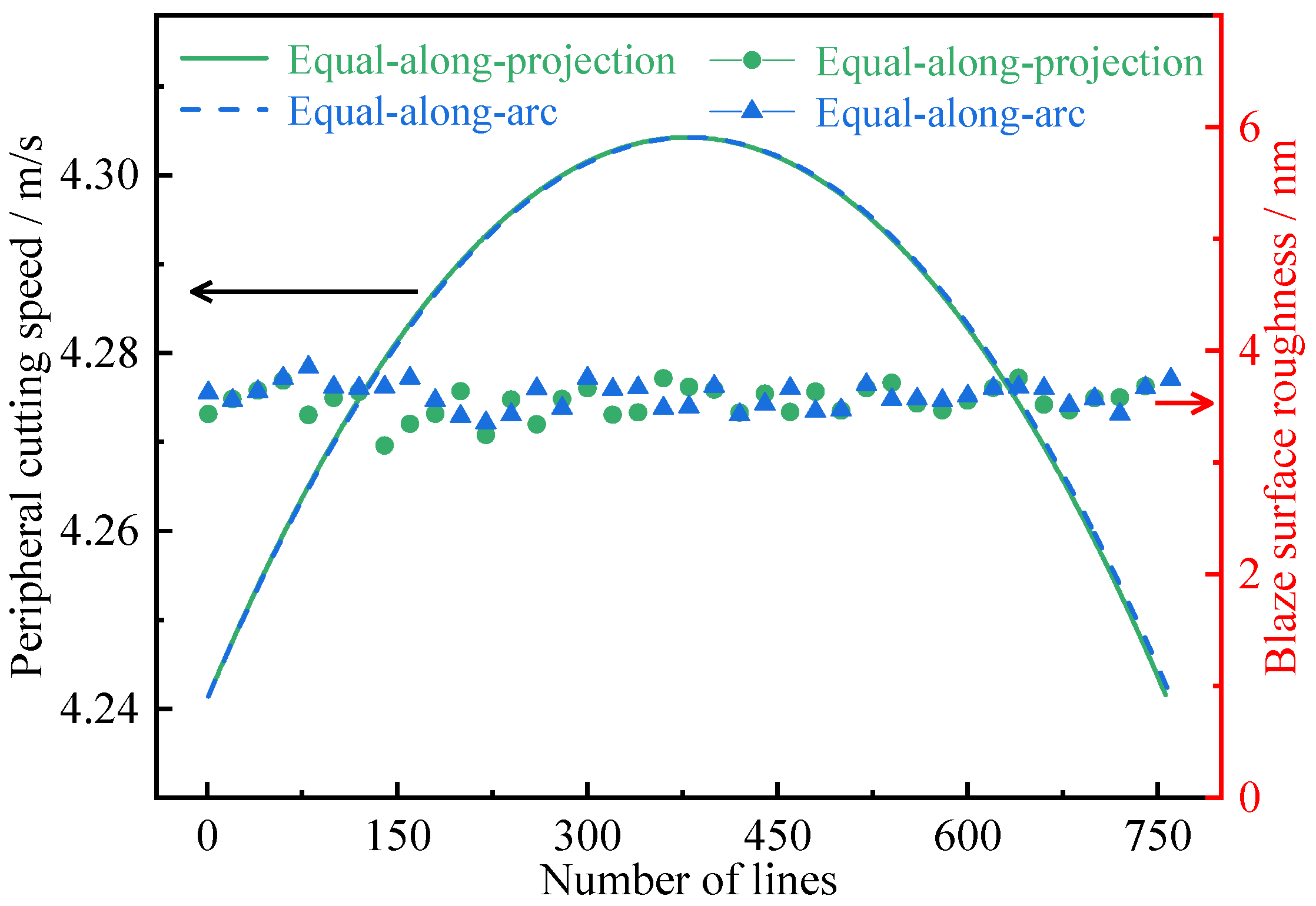
4.2. The Effect of Grating Unit Layout on Dimension Accuracy
4.3. The Effect of the Workpiece Material on the Roughness of the Blaze Surface
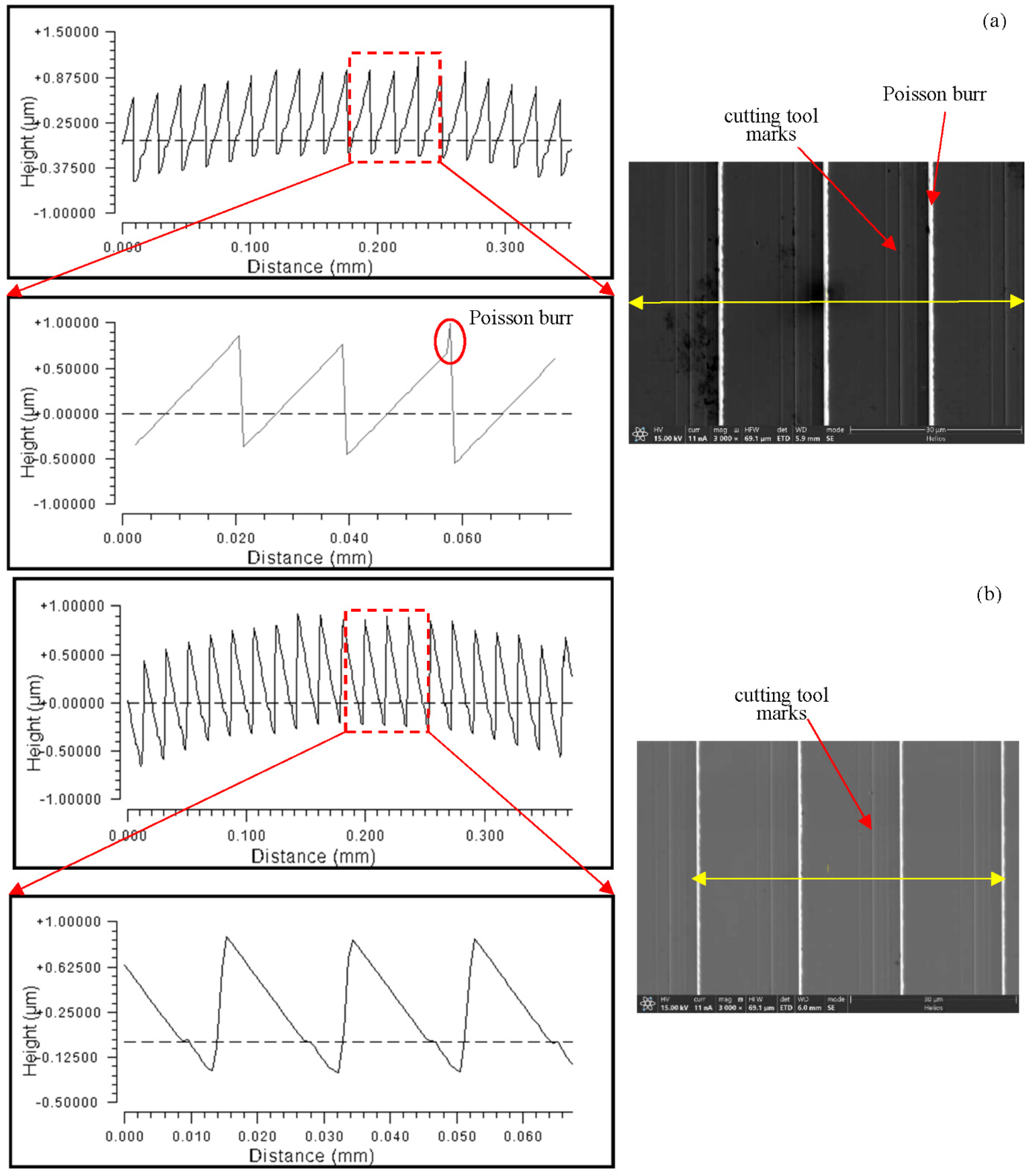
4.4. The Effect of Workpiece Material on the Height of the Poisson Burr
5. Conclusions
- (1)
- Simulation results of the effect of different residual rounded corners and Poisson burr sizes on the diffraction efficiency of the actual profile of the convex spherical blazed grating showed that the diffraction efficiency of the grating gradually decreased in the spectral range as the residual rounded corners and Poisson burr sizes increased. When the Poisson burr height is less than 0.5 μm, the effect of Poisson burrs on the grating diffraction efficiency can be neglected.
- (2)
- The use of low spindle speed in the ultra-precision machining system can effectively reduce the actual cutting speed, which is conducive to the entry of cutting fluid into the first deformation zone and chip removal. In addition, it can avoid large fluctuations in cutting force caused by changes in cutting temperature and can improve the machining accuracy of microstructures.
- (3)
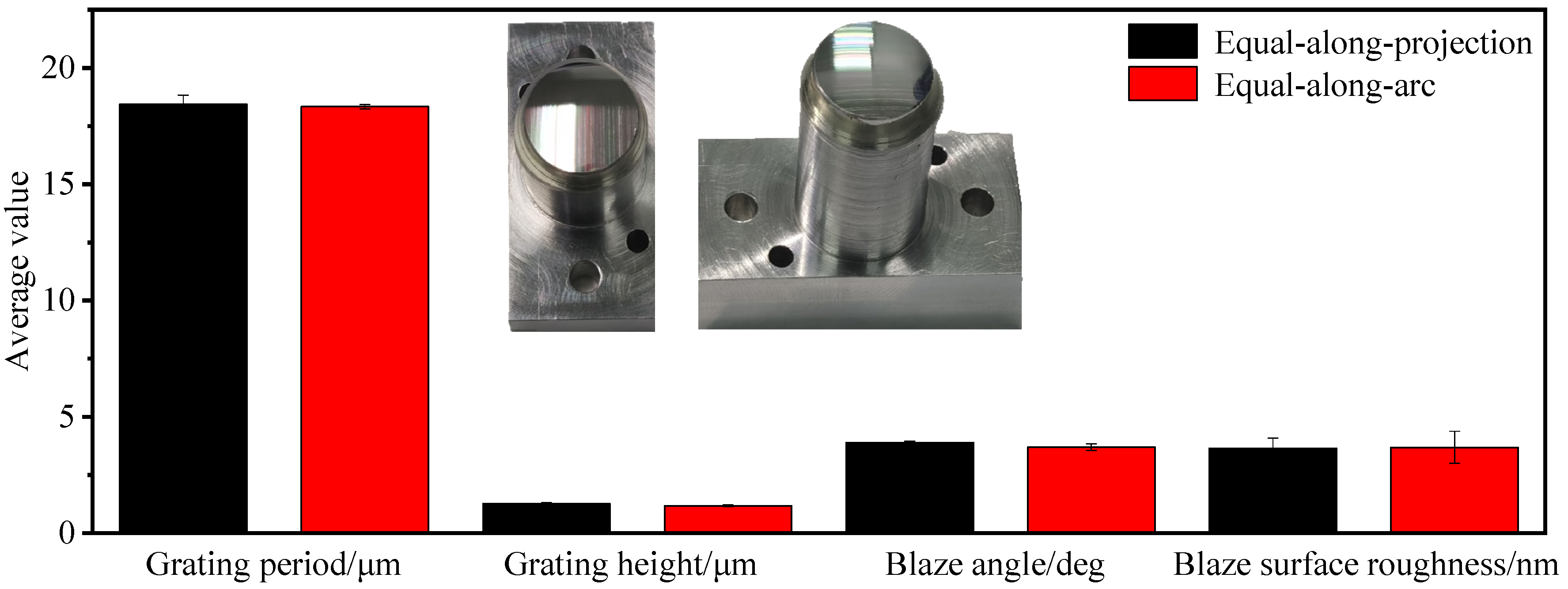
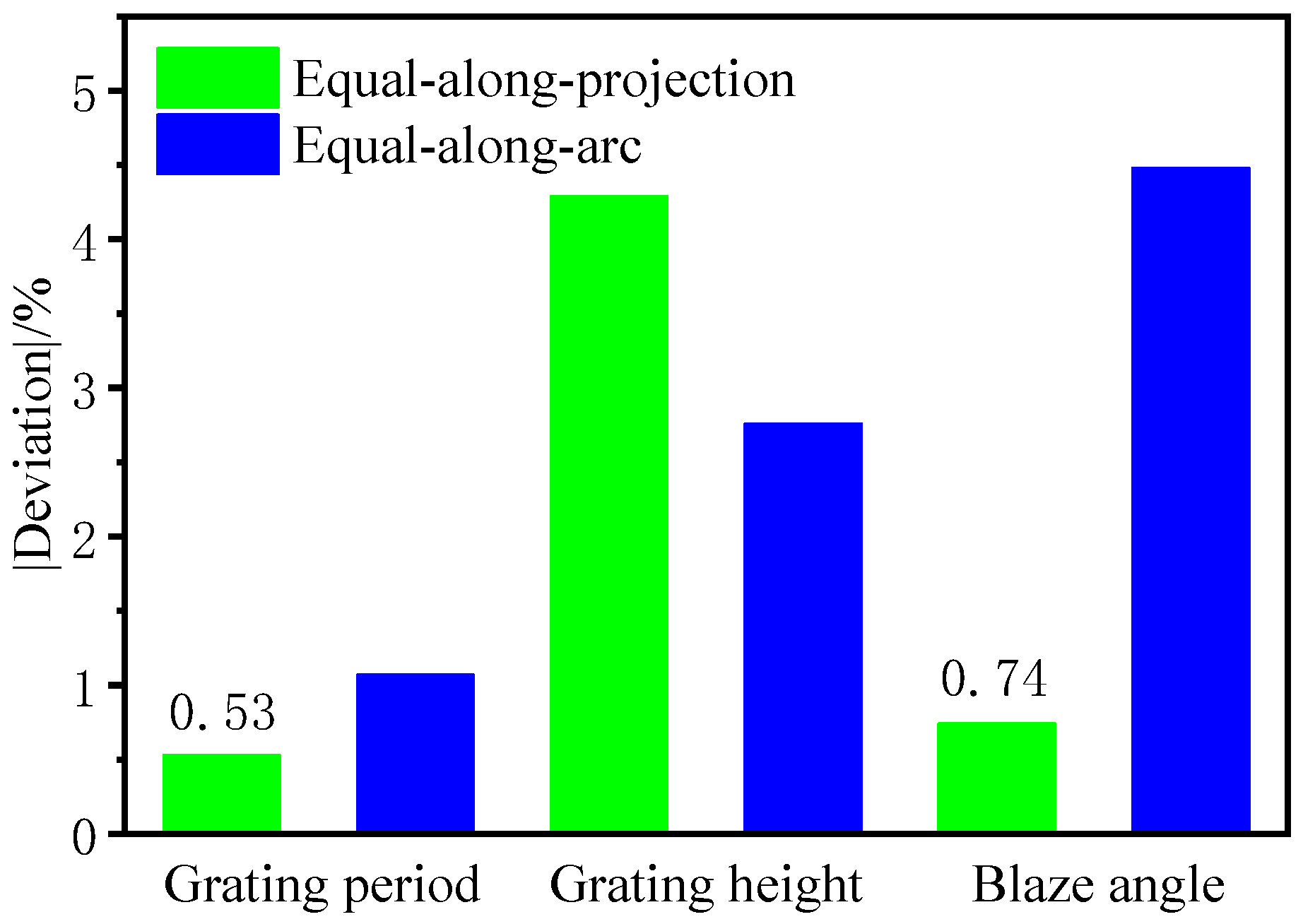
- Under the same cutting parameters, the grating with an equal-along-projection layout and equal-along-arc can maintain the dimensional accuracy of the grating period. However, in terms of grating height dimensional accuracy, the equal-along-arc layout is superior to equal-along-projection. In terms of blaze angle dimensional accuracy, equal-along-projection is superior to equal-along-arc.
- (4)
- Both grating layouts have the same roughness of blaze surface, and the dimensional accuracy of the grating period is close, but the diffraction efficiency is superior that of the equal-along-arc layout due to the higher accuracy of the blaze angle of the equal-along-projection layout.
- (5)
- The RSA6061+ chemically plated NiP material is superior for diamond turning of convex blazed gratings, because it has fewer Poisson burrs on the top of the grating and the blaze surface roughness value is lowered to Ra1.523 nm.
Author Contributions
Funding
Conflicts of Interest
References
- Zhang, S.; Zhou, Y.; Zhang, H.; Xiong, Z.; To, S. Advances in ultra-precision machining of micro-structured functional surfaces and their typical applications. Int. J. Mach. Tools Manuf. 2019, 142, 16–41. [Google Scholar] [CrossRef]
- Hutley, M.C. Diffraction Gratings; Academic Press: New York, NY, USA, 1982. [Google Scholar]
- Born, M.; Wolf, E. Principles of Optics; Macmillan: New York, NY, USA, 2005. [Google Scholar]
- Loewen, E.G.; Popov, E. Diffraction Gratings and Applications; CRC Press: Boca Raton, FL, USA, 2018. [Google Scholar]
- Offner, A. Unit Power Imaging Catoptric Anastigmat. U.S. Patent US3748015 A[P], 24 July 1973. [Google Scholar]
- De Clercq, C.; Moreau, V.; Jamoye, J.F.; Marchi, A.Z.; Gloesener, P. ELOIS: An innovative spectrometer design using a free-form grating. In Proceedings of the SPIE Optical Systems Design, Jena, Germany, 23 September 2015; Volume 9626, p. 96261O. [Google Scholar]
- Fang, F.Z.; Zhang, X.D.; Weckenmann, A.; Zhang, G.X.; Evans, C. Manufacturing and measurement of freeform optics. CIRP Ann. Manuf. Technol. 2013, 62, 823–846. [Google Scholar] [CrossRef]
- Brinksmeier, E.; Schönemann, L. Generation of discontinuous microstructures by Diamond Micro Chiseling. CIRP Ann. Manuf. Technol. 2014, 63, 49–52. [Google Scholar] [CrossRef]
- Davies, M.A.; Dutterer, B.S.; Suleski, T.J.; Silny, J.F.; Kim, E.D. Diamond machining of diffraction gratings for imaging spectrometers. Precis. Eng. 2012, 36, 334–338. [Google Scholar] [CrossRef]
- Lee, C.; Lee, S.K. Fabrication and molding testing of the blazed gratings for microoptics applications. Key. Eng. Mater. 2010, 447, 396–400. [Google Scholar] [CrossRef]
- Xu, D.; Owen, J.D.; Papa, J.C.; Reimers, J.; Suleski, T.J.; Troutman, J.R.; Davies, M.A.; Thompson, K.P.; Rolland, J.P. Design, fabrication, and testing of convex reflective diffraction gratings. Opt. Express 2017, 25, 15252–15268. [Google Scholar] [CrossRef]
- Lee, C.; Kuriyagawa, T.; Woo, D.K.; Lee, S.K. Optimizing the fabrication process of a high-efficiency blazed grating through diamond scribing and molding. J. Micromechanics Microengineering 2010, 20, 055028. [Google Scholar] [CrossRef]
- Liu, C.W.; Yan, J.; Lin, S.C. Diamond turning of high-precision roll-to-roll imprinting molds for fabricating subwavelength gratings. Opt. Eng. 2016, 55, 064105. [Google Scholar] [CrossRef]
- Tan, N.Y.; Zhou, G.; Liu, K.; Kumar, A.S. Diamond shaping of blazed gratings on freeform surfaces. Precis. Eng. 2021, 72, 899–911. [Google Scholar] [CrossRef]
- Zheng, Z.Z.; Yang, Z.; Xiu, L.C. Development and application of shortwave infrared convex blazed grating with high diffraction efficiency. Acta Opt. Sin. 2020, 40, 1205002. [Google Scholar] [CrossRef]
- Graham, C.; Girkin, J.M.; Bourgenot, C. Freeform based hYperspectral imager for MOisture Sensing (FYMOS). Opt. Express 2021, 29, 16007–16018. [Google Scholar] [CrossRef]
- Bourgenot, C.; Robertson, D.J.; Stelter, D.; Eikenberry, S. Towards freeform curved blazed gratings using diamond machining. In Proceedings of the Advances in Optical and Mechanical Technologies for Telescopes and Instrumentation II, Edinburgh, UK, 26 June–1 July 2016; Volume 9912, p. 99123M. [Google Scholar]
- Bourgenot, C.; Calcines, A.; Sharples, R. New opportunities of freeform gratings using diamond machining. In Proceedings of the Advances in Optical and Mechanical Technologies for Telescopes and Instrumentation III, Austin, TX, USA, 10 June–15 July 2018; Volume 10706, p. 1070626. [Google Scholar]
- Moreau, V.; Borguet, B.; Jamoye, J.F.; Maresi, L.; Marchi, A.Z.; Miranda, M. Freeform Grating-Based Hyperspectral Instruments: When SmallSat Solutions Benefit to Big Missions. In Proceedings of the Small Satellite Conference, Logan, UT, USA, 16 July 2019. [Google Scholar]
- Wood, R.W., LXXXV. The echelette grating for the infra-red. The London, Edinburgh, and Dublin. Philos. Mag. J. Sci. 1910, 20, 770–778. [Google Scholar] [CrossRef]
- Xie, J.; Zhou, T.; Ruan, B.; Du, Y.; Wang, X. Effects of interface thermal resistance on surface morphology evolution in precision glass molding for microlens array. Appl. Opt. 2017, 56, 6622–6630. [Google Scholar] [CrossRef]
- Li, H.; Xu, Z.; Ji, Z.; Pi, J. Quantitative characterization of Poisson burrs on the V-shaped texture based on the volume method. J. Mater. Res. Technol. 2020, 9, 16280–16288. [Google Scholar] [CrossRef]
- Jardret, V.; Zahouani, H.; Loubet, J.L.; Mathia, T.G. Understanding and quantification of elastic and plastic deformation during a scratch test. Wear 1998, 218, 8–14. [Google Scholar] [CrossRef]
- Bucaille, J.L.; Felder, E.; Hochstetter, G. Mechanical analysis of the scratch test on elastic and perfectly plastic materials with the three-dimensional finite element molding. Wear 2001, 249, 422–432. [Google Scholar] [CrossRef]
- Leo Kumar, S.P.; Jerald, J.; Kumanan, S.; Prabakaranet, R. A review on current research aspects in tool-based micromachining processes. Mater. Manuf. Processes 2014, 29, 1291–1337. [Google Scholar] [CrossRef]
- Wu, X.; Li, L.; He, N. Investigation on the burr formation mechanism in micro cutting. Precis. Eng. 2017, 47, 191–196. [Google Scholar] [CrossRef]
- Fang, F.Z.; Liu Y, C. On minimum exit-burr in micro cutting. J. Micromechanics Microengineering 2004, 14, 984. [Google Scholar] [CrossRef]
- Wu, X.; Li, L.; He, N.; Hao, X.; Yao, C.; Zhong, L. Investigation on the ploughing force in microcutting considering the cutting edge radius. Int. J. Adv. Manuf. Technol. 2016, 86, 2441–2447. [Google Scholar] [CrossRef]
- Luo, S.; Bayesteh, A.; Ko, J.; Dong, Z.; Jun, M.B. Numerical simulation of chip ploughing volume in micro ball-end mill machining. Int. J. Precis. Eng. Manuf. 2017, 18, 915–922. [Google Scholar] [CrossRef]
- Li, H.; Xu, Z.L.; Pi, J.; Zhou, F. Precision Cutting of the Molds of an Optical Functional Texture Film with a Triangular Pyramid Texture. Micromachines 2020, 11, 248. [Google Scholar] [CrossRef] [PubMed] [Green Version]
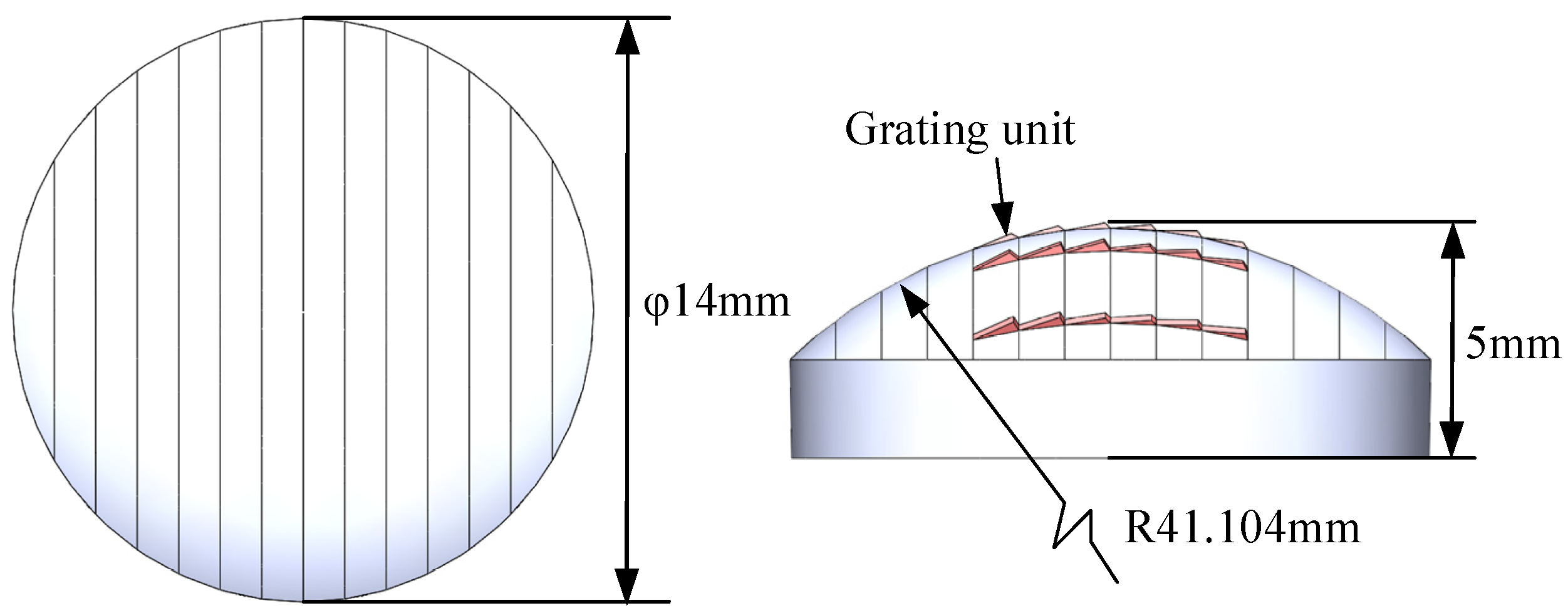



| Parameter | Content |
|---|---|
| Wavelength | 0.95–2.5 μm (SWIR) |
| Surface profile | Convex spherical |
| Surface shape | Circular |
| Substrate radius of curvature (mm) | 41.104 |
| Clear aperture/Diameter (mm) | 14 |
| Groove frequency (line/mm) | 53.97 |
| Substrate material | Optical grade aluminum |
| Coating material | Gold |
| Incidence angle (°) | −25.962 |
| Diffraction order used | −1 |
| Max diffraction efficiency | >82% |
| No. | Type | Rake Angle (°) | Clearance Angle (°) | Included Angle (°) | Tool Nose Radius (μm) | Cutting Edge Radius (μm) |
|---|---|---|---|---|---|---|
| 1 | R-shaped diamond tool | 0 | 10 | 60 | 200 | 0.1 |
| 2 | V-shaped diamond tool | 0 | 15 | 110 | 0.1 | 0.1 |
| Material | Density ρ/g·cm−3 | Young’s Modulus E/GPa | Tensile/Compressive Strength бb/MPa | Yield Strength σ0.2/MPa | Thermal Conductivity /W·m−1·K−1 | Poisson’s Ratio | Hardness |
|---|---|---|---|---|---|---|---|
| RSA6061 | 2.7 | 70 | 330 | 300 | 160 | 0.33 | 110 HB |
| RSA6061+ chemically plated NiP | 7.75 | 50~70 | 700 | \ | 4.19 | 0.3 | 485 HB |
| Single crystalline diamond | 3.5 | 960 | 2000 | N.A. | 2000 | 0.2 | 8000 HB |
| Grating Layouts | Random Sampling Area | Grating Period/d | Grating Height/h | Blaze Angle/θb | Blaze Surface Roughness (nm) | |||
|---|---|---|---|---|---|---|---|---|
| Average Value (μm) | Deviation | Average Value (μm) | Deviation | Average Value (deg) | Deviation | |||
| Equal-along-projection | 1 | 18.3 | −1.23% | 1.23750 | 2.03% | 3.89952 | 0.81% | 3.89 |
| 2 | 18.3 | −1.23% | 1.28228 | 5.72% | 3.86385 | −0.11% | 3.611 | |
| 3 | 18.7 | 0.92% | 1.27487 | 5.11% | 3.92659 | 1.51% | 3.443 | |
| Average value | 18.43 | −0.53% | 1.26488 | 4.29% | 3.89665 | 0.74% | 3.645 | |
| Equal-along-arc | 1 | 18.3 | −1.23% | 1.20314 | −0.80% | 3.62372 | −6.32% | 3.817 |
| 2 | 18.3 | −1.23% | 1.16814 | −3.69% | 3.69477 | −4.48% | 3.285 | |
| 3 | 18.4 | −0.70% | 1.16703 | −3.78% | 3.76656 | −2.63% | 3.966 | |
| Average value | 18.33 | −1.07% | 1.17944 | −2.76% | 3.69502 | −4.48% | 3.689 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, H.; Peng, X.; Guan, C.; Hu, H. Ultra-Precision Cutting and Characterization of Reflective Convex Spherical Blazed Grating Elements. Micromachines 2022, 13, 1115. https://doi.org/10.3390/mi13071115
Li H, Peng X, Guan C, Hu H. Ultra-Precision Cutting and Characterization of Reflective Convex Spherical Blazed Grating Elements. Micromachines. 2022; 13(7):1115. https://doi.org/10.3390/mi13071115
Chicago/Turabian StyleLi, Huang, Xiaoqiang Peng, Chaoliang Guan, and Hao Hu. 2022. "Ultra-Precision Cutting and Characterization of Reflective Convex Spherical Blazed Grating Elements" Micromachines 13, no. 7: 1115. https://doi.org/10.3390/mi13071115
APA StyleLi, H., Peng, X., Guan, C., & Hu, H. (2022). Ultra-Precision Cutting and Characterization of Reflective Convex Spherical Blazed Grating Elements. Micromachines, 13(7), 1115. https://doi.org/10.3390/mi13071115





