Electrical Stability Modeling Based on Surface Potential for a-InGaZnO TFTs under Positive-Bias Stress and Light Illumination
Abstract
1. Introduction
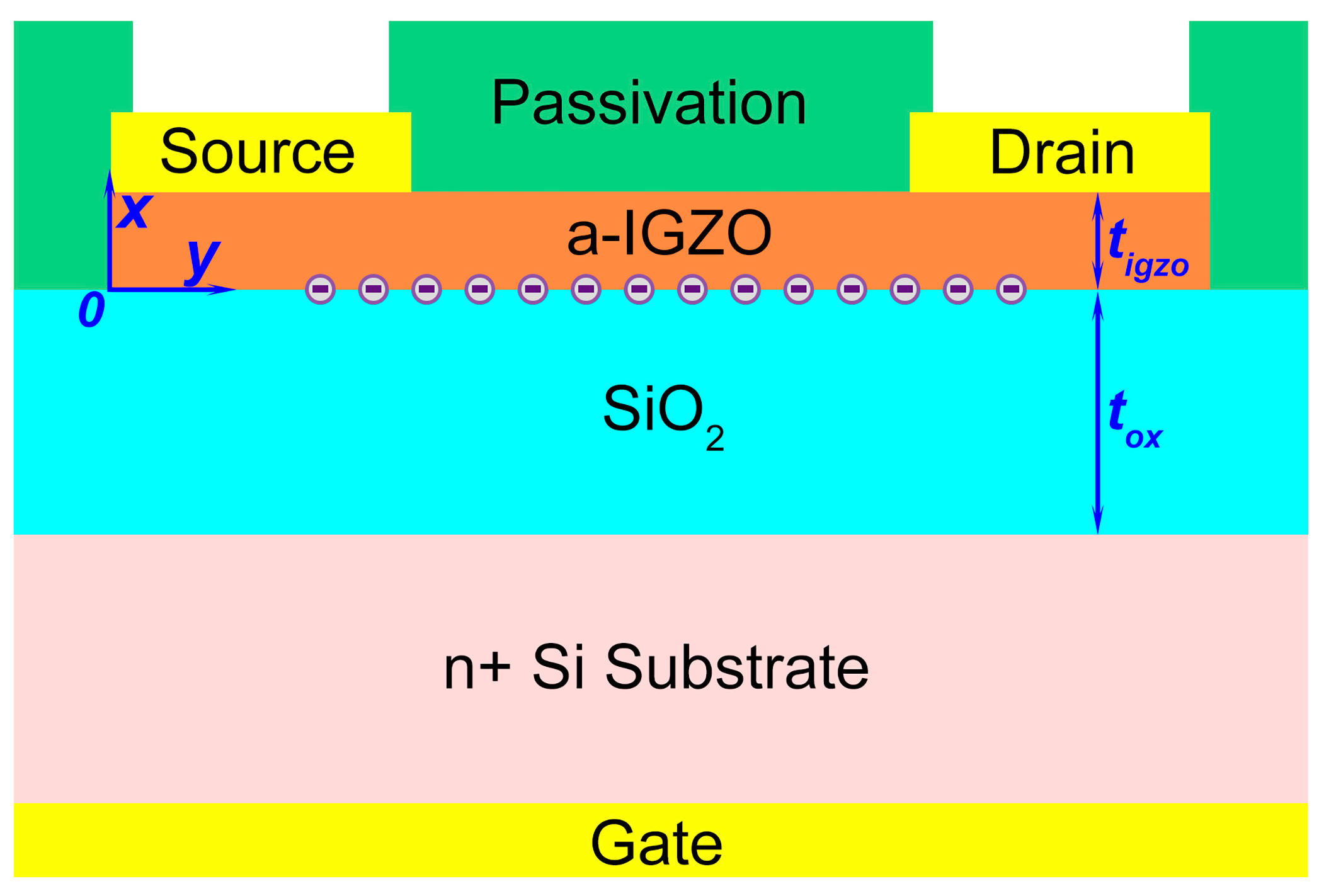
2. Model Calculation
2.1. Surface Potential Model Calculation
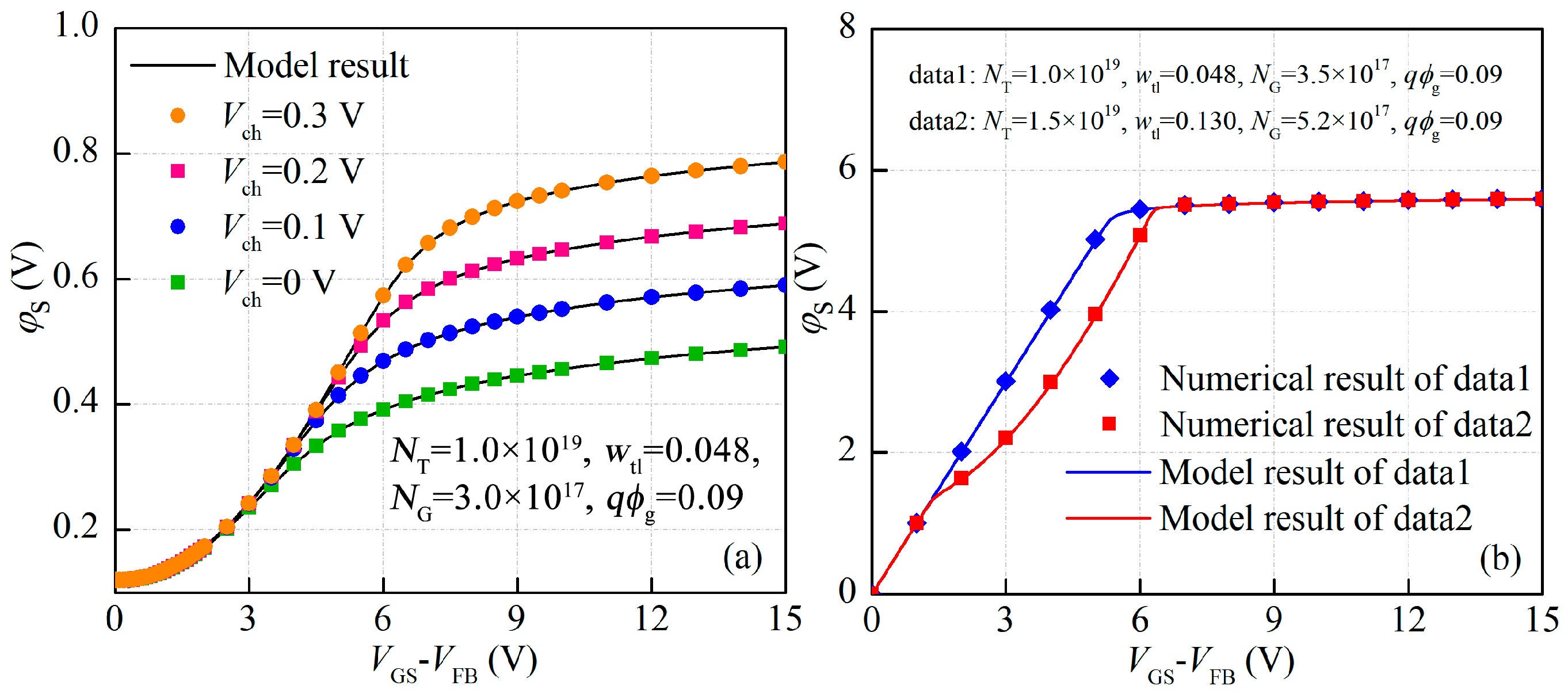
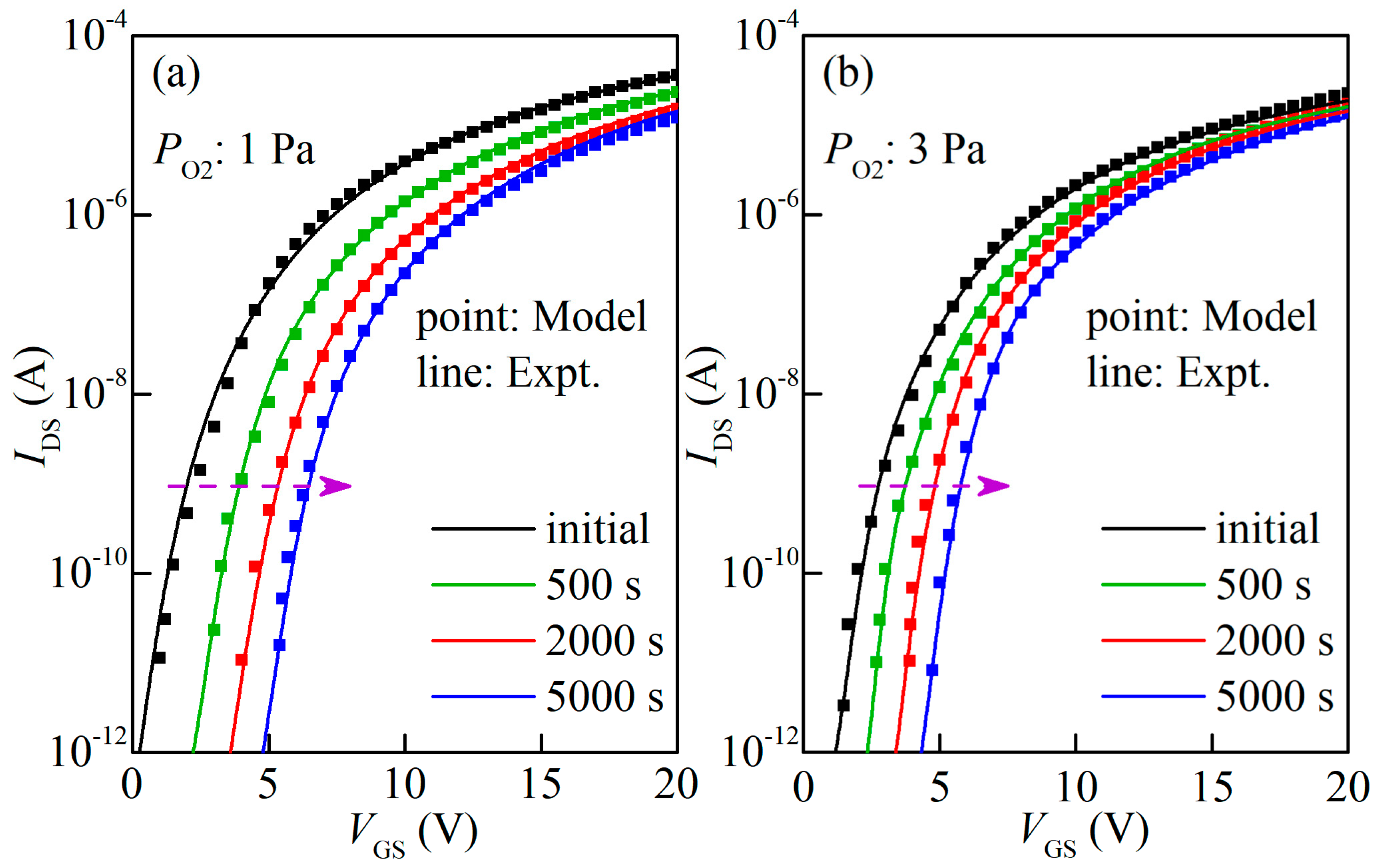
2.2. Model Verification
3. Results and Discussions
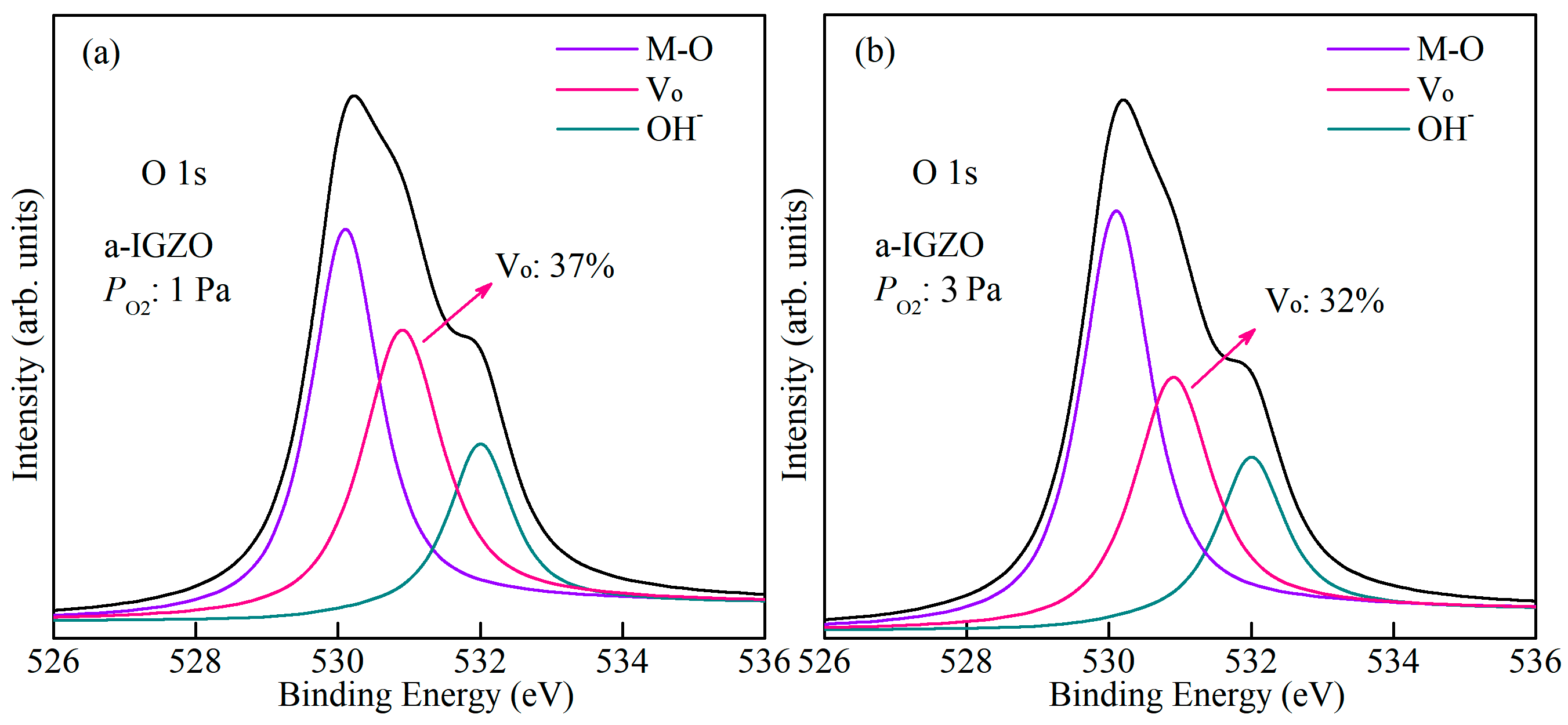
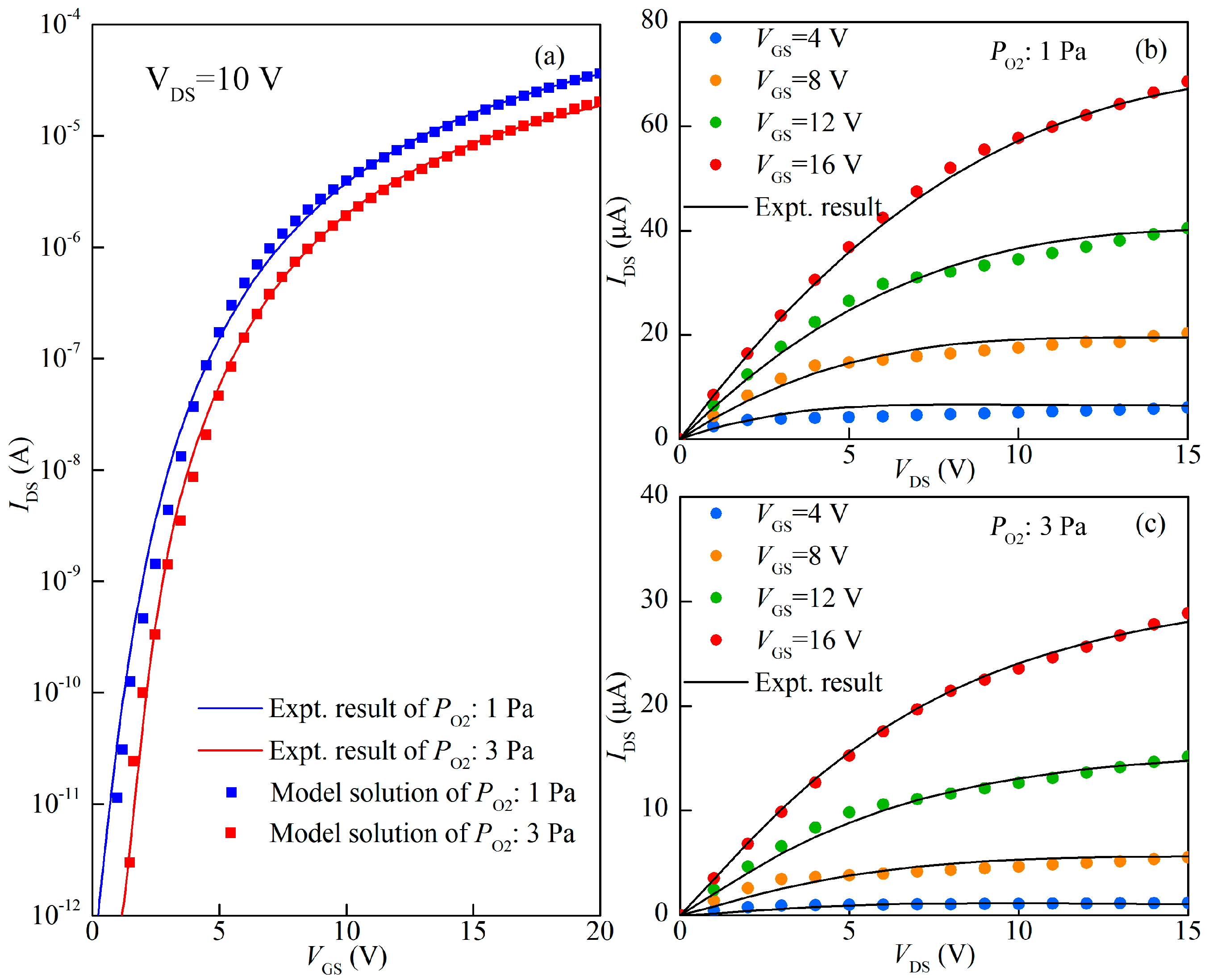
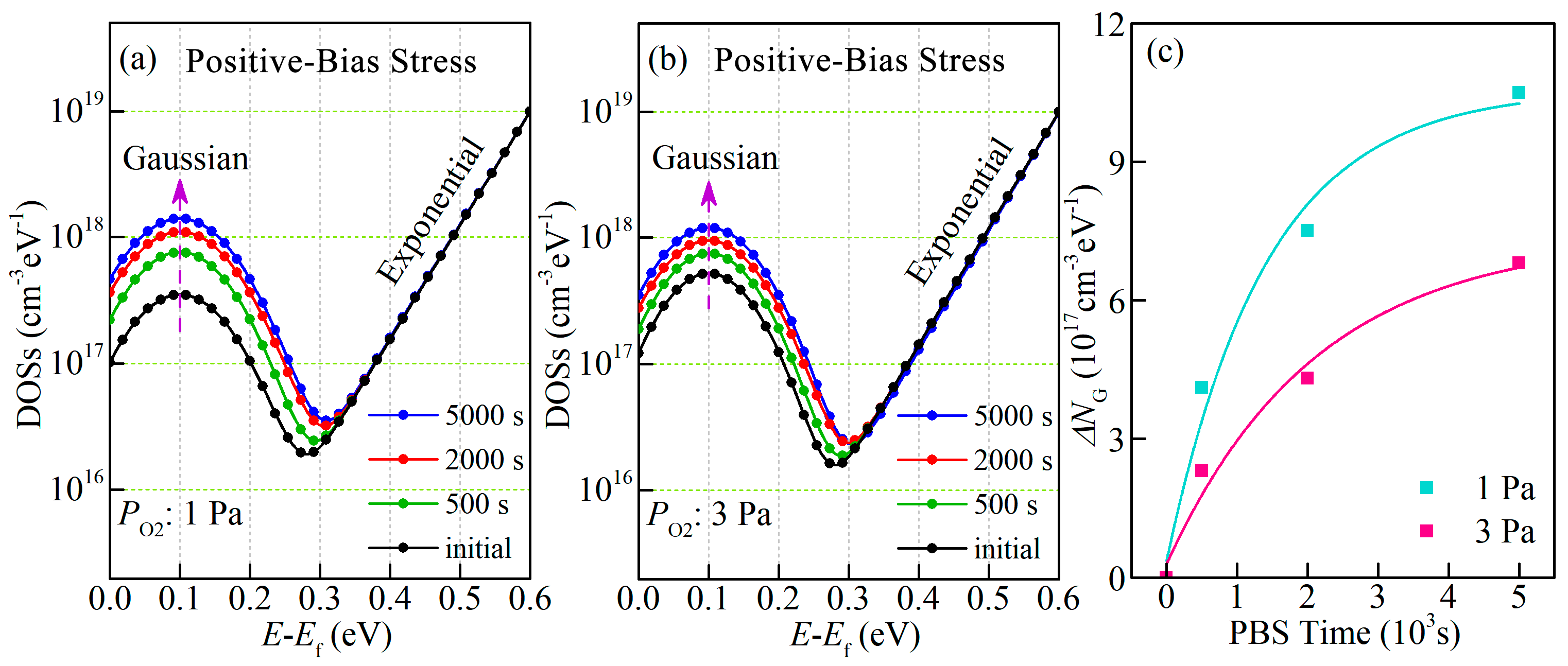
3.1. The Model of a-IGZO TFTs under PBS
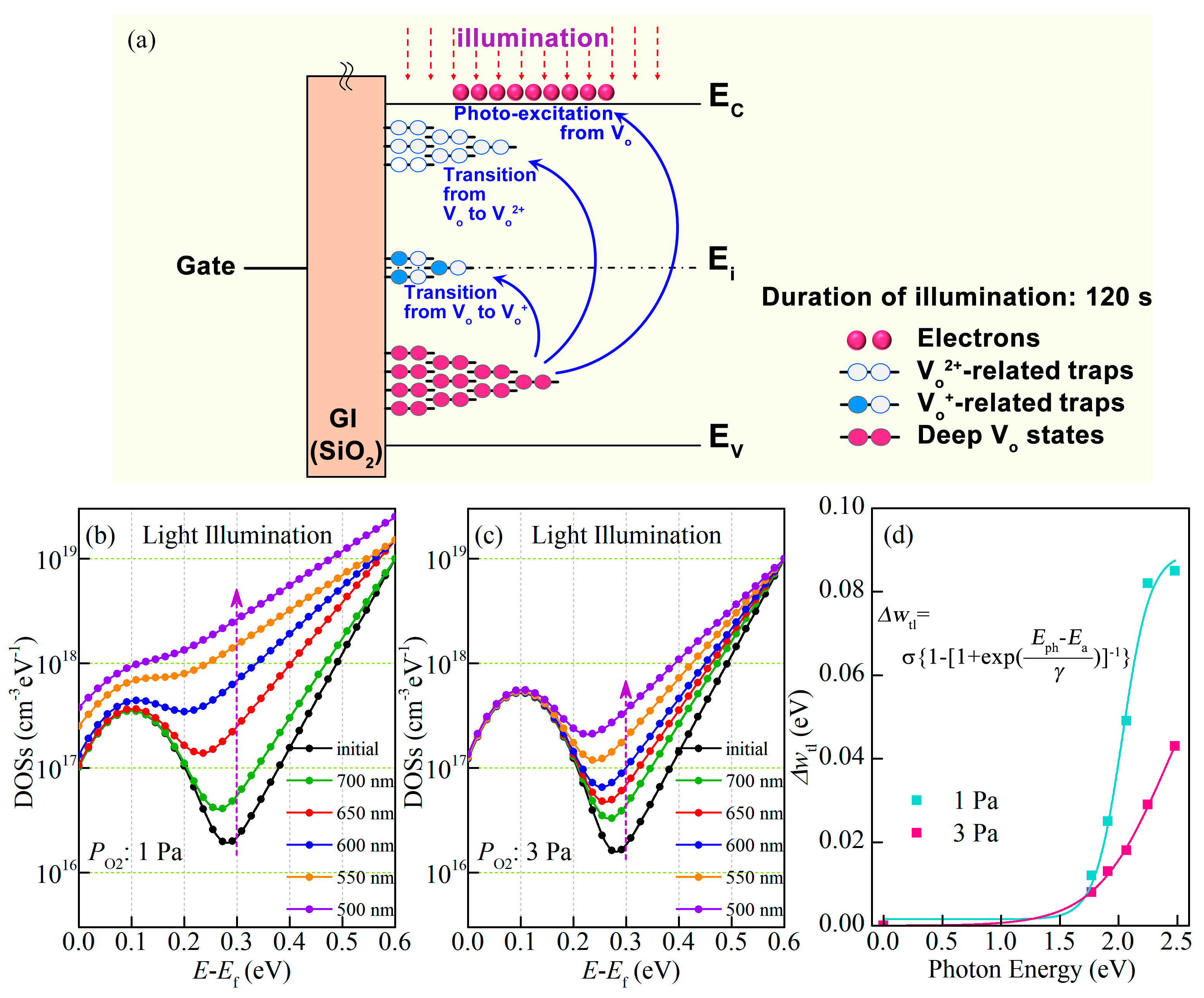
3.2. The Model of a-IGZO TFTs under Light Illumination
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Nomura, K.; Ohta, H.; Takagi, A.; Kamiya, T.; Hirano, M.; Hosono, H. Room-temperature fabrication of transparent flexible thin-film transistors using amorphous oxide semiconductors. Nature 2004, 432, 488–492. [Google Scholar] [CrossRef] [PubMed]
- Cho, M.H.; Seol, H.; Yang, H.; Yun, P.S.; Bae, J.U.; Park, K.-S.; Jeong, J.K. High-performance amorphous indium gallium zinc oxide thin-film transistors fabricated by atomic layer deposition. IEEE Electron Device Lett. 2018, 39, 688–691. [Google Scholar] [CrossRef]
- Kim, J.; Kim, H.; Oh, J.; Choi, S.-Y.; Park, H. Turn-around of threshold voltage shift in amorphous InGaZnO TFT under positive bias illumination stress. Solid-State Electron. 2023, 201, 108605. [Google Scholar] [CrossRef]
- Kim, W.; Lee, W.J.; Kwak, T.; Baek, S.; Lee, S.H.; Park, S. Influence of UV/ozone treatment on threshold voltage modulation in sol–gel IGZO thin-film transistors. Adv. Mater. Interfaces 2022, 9, 2200032. [Google Scholar] [CrossRef]
- Jeong, J. The status and perspectives of metal oxide thin-film transistors for active matrix flexible displays. Semicond. Sci. Technol. 2011, 26, 034008. [Google Scholar] [CrossRef]
- Bagheri, M.; Cheng, X.; Zhang, J.; Lee, S.; Ashtiani, S.; Nathan, A. Threshold voltage compensation error in voltage programmed AMOLED displays. J. Disp. Technol. 2016, 12, 658–664. [Google Scholar] [CrossRef]
- Zong, Z.; Li, L.; Jang, J.; Lu, N.; Liu, M. Analytical surface-potential compact model for amorphous-IGZO thin-film transistors. J. Appl. Phys. 2015, 117, 215705. [Google Scholar] [CrossRef]
- Moldovan, O.; Castro-Carranza, A.; Estrada, M.; Cerdeira, A.; Lime, F.; Iniguez, B. A complete charge-based capacitance model for IGZO TFTs. IEEE Electron Device Lett. 2019, 40, 730–733. [Google Scholar] [CrossRef]
- Deng, W.; Fang, J.; Wei, X.; Wu, W.; Huang, J. A core compact model for IGZO TFTS considering degeneration mechanism. IEEE Trans. Electron Devices 2018, 65, 1370–1376. [Google Scholar] [CrossRef]
- Chowdhury, M.; Migliorato, P.; Jang, J. Time-temperature dependence of positive gate bias stress and recovery in amorphous indium-gallium-zinc-oxide thin-film-transistors. Appl. Phys. Lett. 2011, 98, 153511. [Google Scholar] [CrossRef]
- Hung, M.; Wang, D.; Furuta, M. Investigating effect of postannealing time on positive bias stress stability of In–Ga–Zn–O TFT by conductance method. IEEE Trans. Electron Devices 2015, 62, 3697–3702. [Google Scholar] [CrossRef]
- Zhou, X.; Shao, Y.; Zhang, L.; Lu, H.; He, H.; Han, D.; Wang, Y.; Zhang, S. Oxygen interstitial creation in a-IGZO thin-film transistors under positive gate-bias stress. IEEE Electron Device Lett. 2017, 38, 1252–1255. [Google Scholar] [CrossRef]
- Lee, E.; Park, J.; Lee, S.; Na, H.; Cho, N.; Im, C.; Cho, Y.; Kim, Y. Oxygen radical control via atmospheric pressure plasma treatment for highly stable IGZO thin-film transistors. IEEE Trans. Electron Devices 2020, 67, 3135–3140. [Google Scholar] [CrossRef]
- Kuk, S.; Lee, S.; Kim, S.; Kim, B.; Park, S.; Kwon, J.; Han, M. Light-induced hysteresis of In–Ga–Zn–O thin-film transistors with various temperatures. IEEE Electron Device Lett. 2012, 33, 1279–1281. [Google Scholar] [CrossRef]
- Tsormpatzoglou, A.; Hastas, N.; Choi, N.; Mahmoudabadi, F.; Hatalis, M.; Dimitriadis, C. Analytical surface-potential-based drain current model for amorphous InGaZnO thin film transistors. J. Appl. Phys. 2013, 114, 184502. [Google Scholar] [CrossRef]
- Jang, J.; Ko, D.; Choi, S.; Kim, D.; Kim, D. Observation of hydrogen-related defect in subgap density of states and its effects under positive bias stress in amorphous InGaZnO TFT. IEEE Electron Device Lett. 2021, 42, 708–711. [Google Scholar] [CrossRef]
- Deng, W.; Huang, J.; Li, X. Surface-potential-based drain current model of polysilicon TFTs with gaussian and exponential DOS distribution. IEEE Trans. Electron Devices 2011, 59, 94–100. [Google Scholar] [CrossRef]
- Yang, G.; Park, J.; Choi, S.; Kim, C.; Kim, D.M.; Choi, S.; Bae, J.; Cho, I.; Kim, D. Total subgap range density of states-based analysis of the effect of oxygen flow rate on the bias stress instabilities in a-IGZO TFTs. IEEE Trans. Electron Devices 2022, 69, 166–173. [Google Scholar] [CrossRef]
- Oka, N.; Aoi, T.; Hayashi, R.; Kumomi, H.; Shigesato, Y. Electronic state of amorphous indium gallium zinc oxide films deposited by dc magnetron sputtering with water vapor introduction. Appl. Phys. Express 2012, 5, 075802. [Google Scholar] [CrossRef]
- Yang, S.; Ji, K.; Kim, U.; Hwang, C.; Park, S.; Hwang, C.; Jang, J.; Jeong, J. Suppression in the negative bias illumination instability of Zn-Sn-O transistor using oxygen plasma treatment. Appl. Phys. Lett. 2011, 99, 102103. [Google Scholar] [CrossRef]
- Nomura, K.; Kamiya, T.; Ikenaga, E.; Yanagi, H.; Kobayashi, K.; Hosono, H. Depth analysis of subgap electronic states in amorphous oxide semiconductor, a-In-Ga-Zn-O, studied by hard X-ray photoelectron spectroscopy. J. Appl. Phys. 2011, 109, 073726. [Google Scholar] [CrossRef]
- Ha, T.; Kim, Y.; Cho, Y.; Kang, Y.; Yu, S.; Kim, G.; Jeong, H.; Park, J.; Kim, O. Defect-creation effects on abnormal on-current under drain bias illumination stress in a-IGZO thin-film transistors. Solid-State Electron. 2020, 165, 107752. [Google Scholar] [CrossRef]
- Um, J.; Mativenga, M.; Migliorato, P.; Jang, J. Increase of interface and bulk density of states in amorphous-indium-gallium-zinc-oxide thin-film transistors with negative-bias-under-illumination-stress time. Appl. Phys. Lett. 2012, 101, 113504. [Google Scholar]
- Nomura, K.; Kamiya, T.; Hirano, M.; Hosono, H. Origins of threshold voltage shifts in room-temperature deposited and annealed a-In–Ga–Zn–O thin-film transistors. Appl. Phys. Lett. 2009, 95, 013502. [Google Scholar] [CrossRef]
- Choi, S.; Choi, S.J.; Kim, D.; Park, S.; Kim, J.; Seo, Y.; Shin, H.; Jeong, Y.; Bae, J.; Oh, C.; et al. Positive bias stress instability of InGaZnO TFTs with self-aligned top-gate structure in the threshold-voltage compensated pixel. IEEE Electron Device Lett. 2020, 41, 50–53. [Google Scholar] [CrossRef]
- Hsu, S.; He, J.; Li, Y.; Su, D.; Tsai, F.; Cheng, I. Effect of mechanical strain on electrical performance of flexible p-type SnO thin-film transistors. IEEE Trans. Electron Devices 2019, 66, 5183–5186. [Google Scholar] [CrossRef]
- Costa, J.; Kermani, A.; Cantarella, G.; Petti, L.; Vogt, C.; Daus, A.; Knobelspies, S.; Troster, G.; Munzenrieder, N. Long-term aging of Al2O3 passivated and unpassivated flexible a-IGZO TFTs. IEEE Trans. Electron Devices 2020, 67, 4934–4939. [Google Scholar] [CrossRef]
- Huang, X.; Wu, C.; Lu, H.; Ren, F.; Xu, Q.; Ou, H.; Zhang, R.; Zheng, Y. Electrical instability of amorphous indium-gallium-zinc oxide thin film transistors under monochromatic light illumination. Appl. Phys. Lett. 2012, 100, 243505. [Google Scholar] [CrossRef]
- Billah, M.; Chowdhury, M.; Mativenga, M.; Um, J.; Mruthyunjaya, R.; Heiler, G.; Tredwell, T.; Jang, J. Analysis of improved performance under negative bias illumination stress of dual gate driving a-IGZO TFT by TCAD simulation. IEEE Electron Device Lett. 2016, 37, 735–737. [Google Scholar] [CrossRef]
- Kim, Y.; Kim, S.; Kim, W.; Bae, M.; Jeong, H.; Kong, D.; Choi, S.; Kim, D.; Kim, D.H. Amorphous InGaZnO thin-film transistors—Part II: Modeling and simulation of negative bias illumination stress-induced instability. IEEE Trans. Electron Devices 2012, 59, 2699–2706. [Google Scholar] [CrossRef]
- Tsurumi, J.; Matsui, H.; Kubo, T.; Häusermann, R.; Mitsui, C.; Okamoto, T.; Watanabe, S.; Takeya, J. Coexistence of ultra-long spin relaxation time and coherent charge transport in organic single-crystal semiconductors. Nat. Phys. 2017, 13, 994–998. [Google Scholar] [CrossRef]
- Beckers, A.; Beckers, D.; Jazaeri, F.; Parvais, B.; Enz, C. Generalized boltzmann relations in semiconductors including band tails. J. Appl. Phys. 2021, 129, 045701. [Google Scholar] [CrossRef]
- Xie, H.; Wu, Q.; Xu, L.; Zhang, L.; Liu, G.; Dong, C. Nitrogen-doped amorphous oxide semiconductor thin film transistors with double-stacked channel layers. Appl. Surf. Sci. 2016, 387, 237–243. [Google Scholar] [CrossRef]
- Yang, Z.; Meng, T.; Zhang, Q.; Shieh, H. Stability of amorphous indium–tungsten oxide thin-film transistors under various wavelength light illumination. IEEE Electron Device Lett. 2016, 37, 437–440. [Google Scholar] [CrossRef]

| Parameters | Value | Unit | Parameters | Value | Unit |
|---|---|---|---|---|---|
| q | 1.6 × 10−19 | C | d | 0.05 | eV |
| εigzo | 11.5 | - | E0 | 2.7 | eV |
| COX | 1.73 × 10−4 | F/m2 | μi | 5 | cm2s−1/V1+p |
| VFB | 0 | V | p | 0.7 | - |
| VDS | 10 | V | W | 200 | µm |
| ϕf | 0.026 | V | L | 40 | µm |
| Ef | 2.6 | eV | tigzo | 50 | nm |
| Parameter | Po2 [Pa] | Initial | PBS Time [s] | ||
|---|---|---|---|---|---|
| 500 | 2000 | 5000 | |||
| NT [cm−3eV−1] | 1 | 1 × 1019 | 1 × 1019 | 1 × 1019 | 1 × 1019 |
| 3 | 1 × 1019 | 1 × 1019 | 1 × 1019 | 1 × 1019 | |
| wtl [eV] | 1 | 0.048 | 0.048 | 0.048 | 0.048 |
| 3 | 0.047 | 0.047 | 0.047 | 0.045 | |
| NG [cm−3eV−1] | 1 | 3.5 × 1017 | 7.6 × 1017 | 1.1 × 1018 | 1.4 × 1018 |
| 3 | 5.2 × 1017 | 7.5 × 1017 | 9.5 × 1017 | 1.2 × 1018 | |
| qϕg [eV] | 1 | 0.09 | 0.09 | 0.095 | 0.095 |
| 3 | 0.083 | 0.085 | 0.09 | 0.09 | |
| β | 1 | 0.85 | |||
| 3 | |||||
| τ[s] | 1 | 1544.58 | |||
| 3 | 1902.39 | ||||
| α | 1 | 6.43 × 1016 | |||
| 3 | 5.49 × 1016 | ||||
| Parameter | Po2 [Pa] | Initial | Wavelength of Light [nm] | ||||
|---|---|---|---|---|---|---|---|
| 750 | 650 | 600 | 550 | 500 | |||
| NT [cm−3eV−1] | 1 | 1 × 1019 | 1 × 1019 | 1.5 × 1019 | 1.5 × 1019 | 1.5 × 1019 | 2.5 × 1019 |
| 3 | 1 × 1019 | 1 × 1019 | 1 × 1019 | 1 × 1019 | 1 × 1019 | 1 × 1019 | |
| wtl [eV] | 1 | 0.048 | 0.060 | 0.073 | 0.097 | 0.130 | 0.133 |
| 3 | 0.047 | 0.055 | 0.060 | 0.065 | 0.076 | 0.090 | |
| NG [cm−3eV−1] | 1 | 3.5 × 1017 | 3.5 × 1017 | 3.5 × 1017 | 3.5 × 1017 | 3.5 × 1017 | 3.5 × 1017 |
| 3 | 5.2 × 1017 | 5.2 × 1017 | 5.2 × 1017 | 5.2 × 1017 | 5.2 × 1017 | 5.2 × 1017 | |
| qϕg [eV] | 1 | 0.09 | 0.09 | 0.09 | 0.09 | 0.09 | 0.09 |
| 3 | 0.083 | 0.083 | 0.083 | 0.083 | 0.083 | 0.083 | |
| Ea [eV] | 1 | 2.3 | |||||
| 3 | |||||||
| γ | 1 | 0.1 | |||||
| 3 | 0.3 | ||||||
| σ | 1 | 0.089 | |||||
| 3 | 0.076 | ||||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huang, X.; Cao, W.; Huang, C.; Chen, C.; Shi, Z.; Xu, W. Electrical Stability Modeling Based on Surface Potential for a-InGaZnO TFTs under Positive-Bias Stress and Light Illumination. Micromachines 2023, 14, 842. https://doi.org/10.3390/mi14040842
Huang X, Cao W, Huang C, Chen C, Shi Z, Xu W. Electrical Stability Modeling Based on Surface Potential for a-InGaZnO TFTs under Positive-Bias Stress and Light Illumination. Micromachines. 2023; 14(4):842. https://doi.org/10.3390/mi14040842
Chicago/Turabian StyleHuang, Xiaoming, Wei Cao, Chenyang Huang, Chen Chen, Zheng Shi, and Weizong Xu. 2023. "Electrical Stability Modeling Based on Surface Potential for a-InGaZnO TFTs under Positive-Bias Stress and Light Illumination" Micromachines 14, no. 4: 842. https://doi.org/10.3390/mi14040842
APA StyleHuang, X., Cao, W., Huang, C., Chen, C., Shi, Z., & Xu, W. (2023). Electrical Stability Modeling Based on Surface Potential for a-InGaZnO TFTs under Positive-Bias Stress and Light Illumination. Micromachines, 14(4), 842. https://doi.org/10.3390/mi14040842






