Arc Discharge System for Micromachining of Helical Fiber
Abstract
:1. Introduction
2. Principle and Simulation
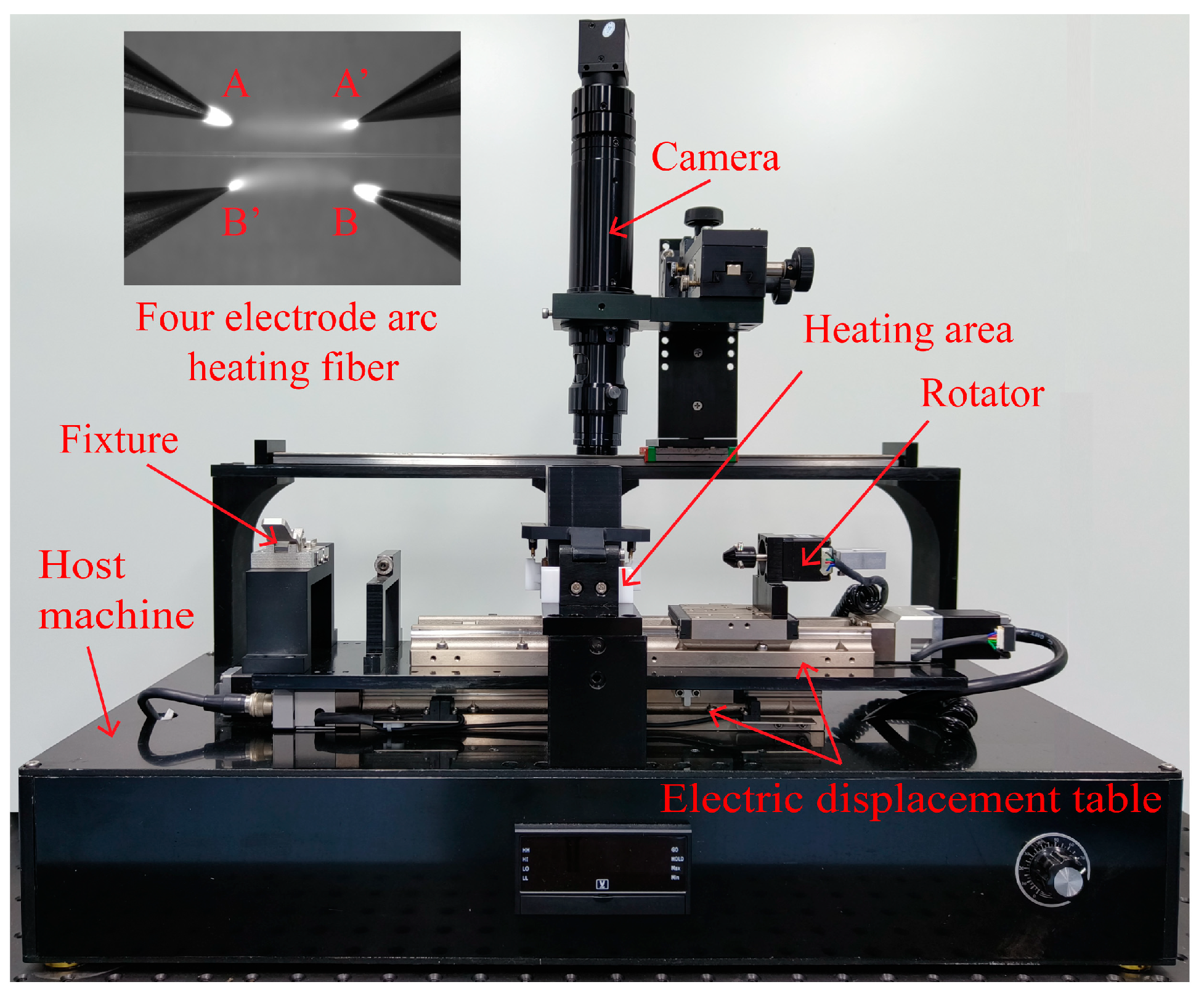
2.1. Working Principle and Process of the Four-Electrode Arc’s Helical Fiber Micromachining System
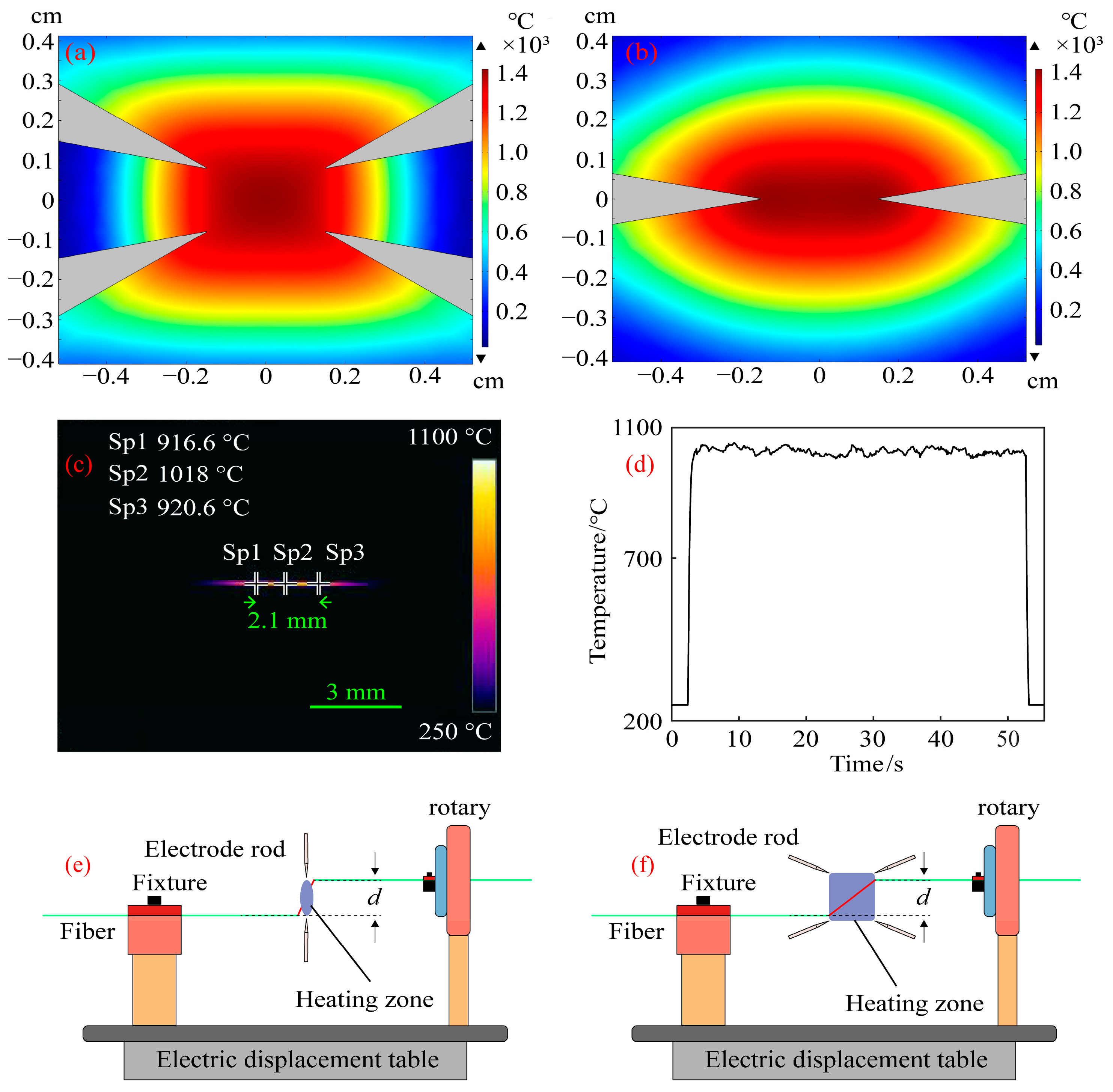
2.2. High-Temperature Heating Field of the Four-Electrode Arc
- (1)
- Laminar flow conditions:
- (2)
- Fluid heat transfer conditions:
- (3)
- Current conditions:
- (4)
- Magnetic field conditions:
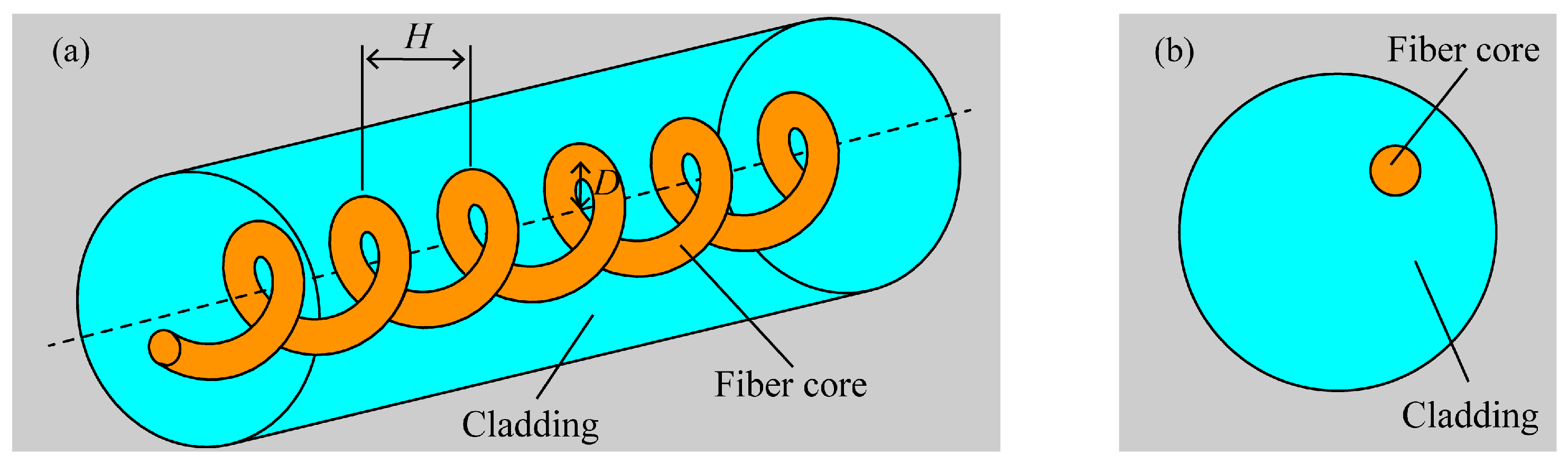
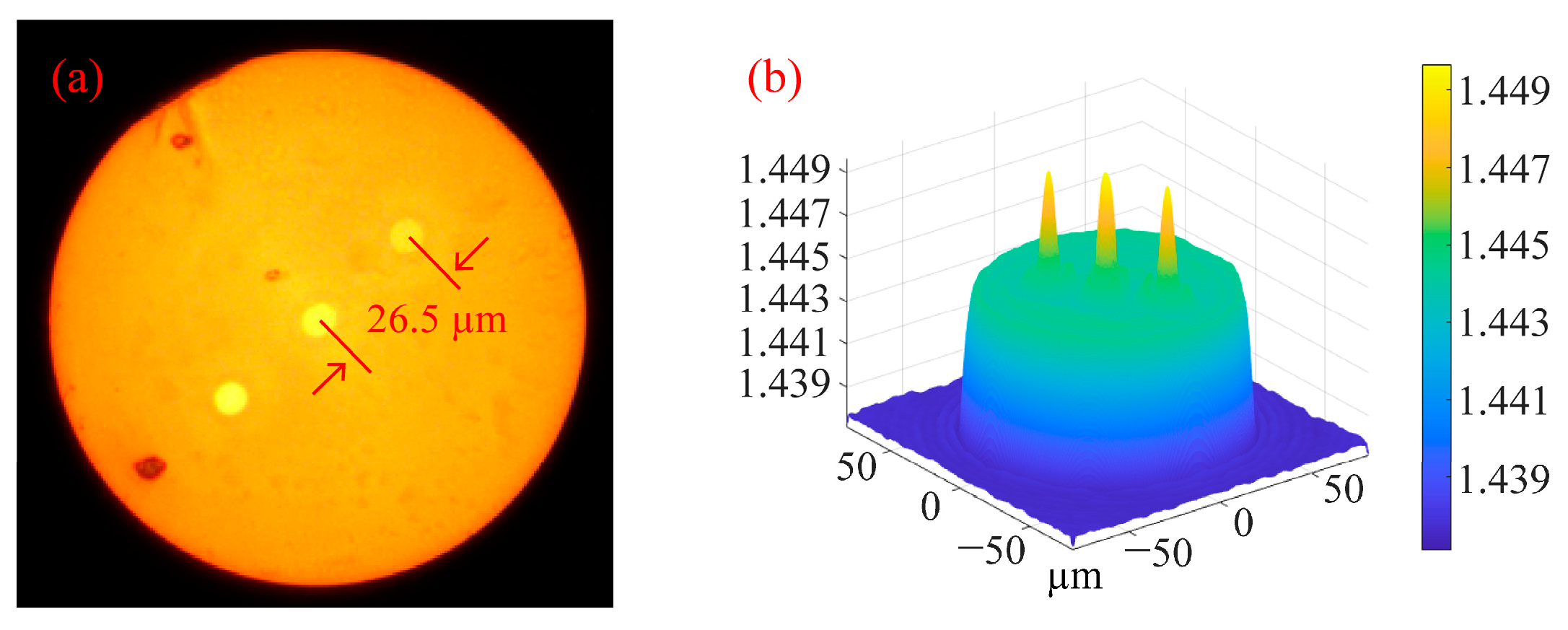
2.3. Modulation of the Refractive Index of the Multi-Core Fiber Core
3. Results and Discussion
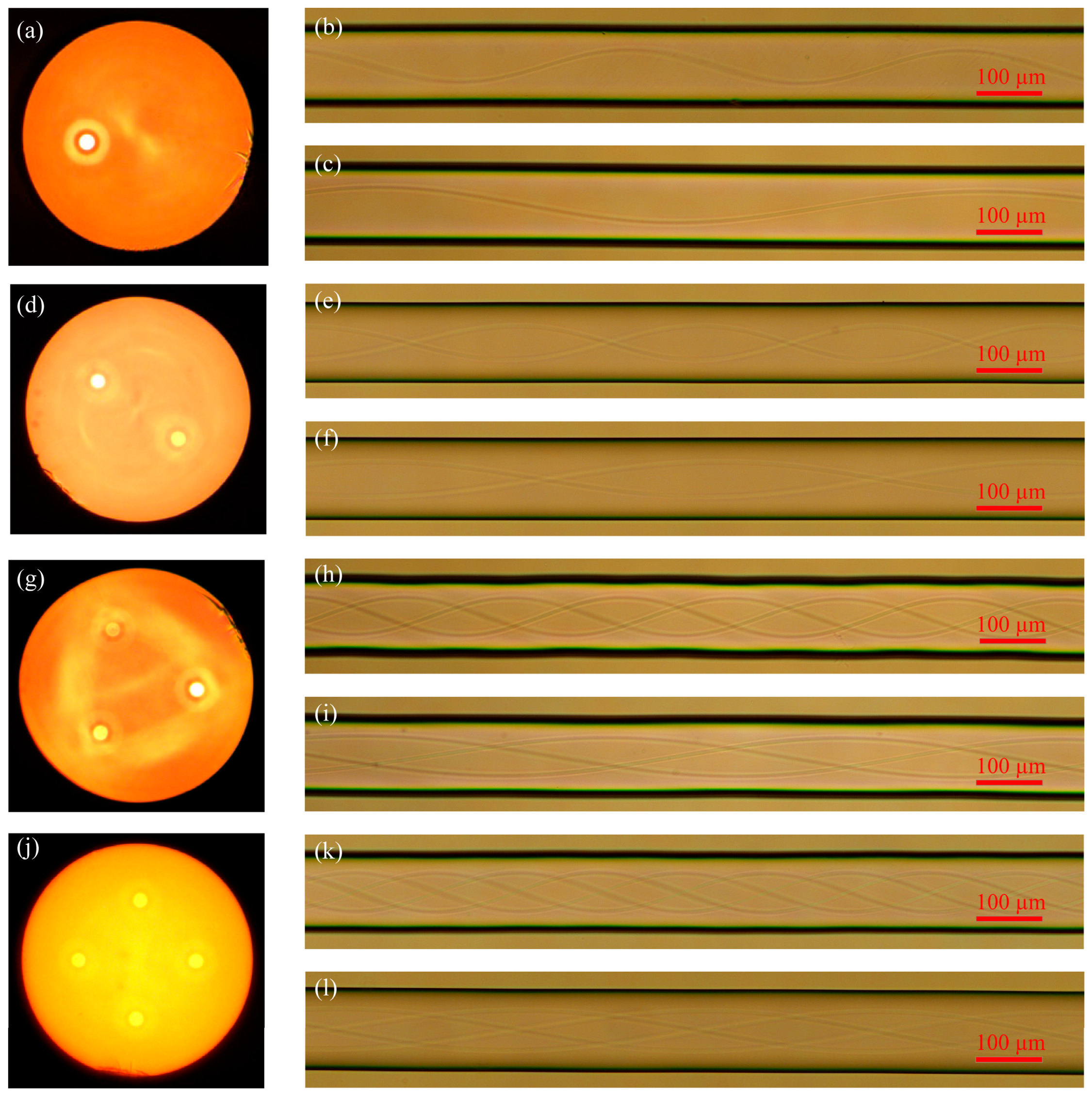
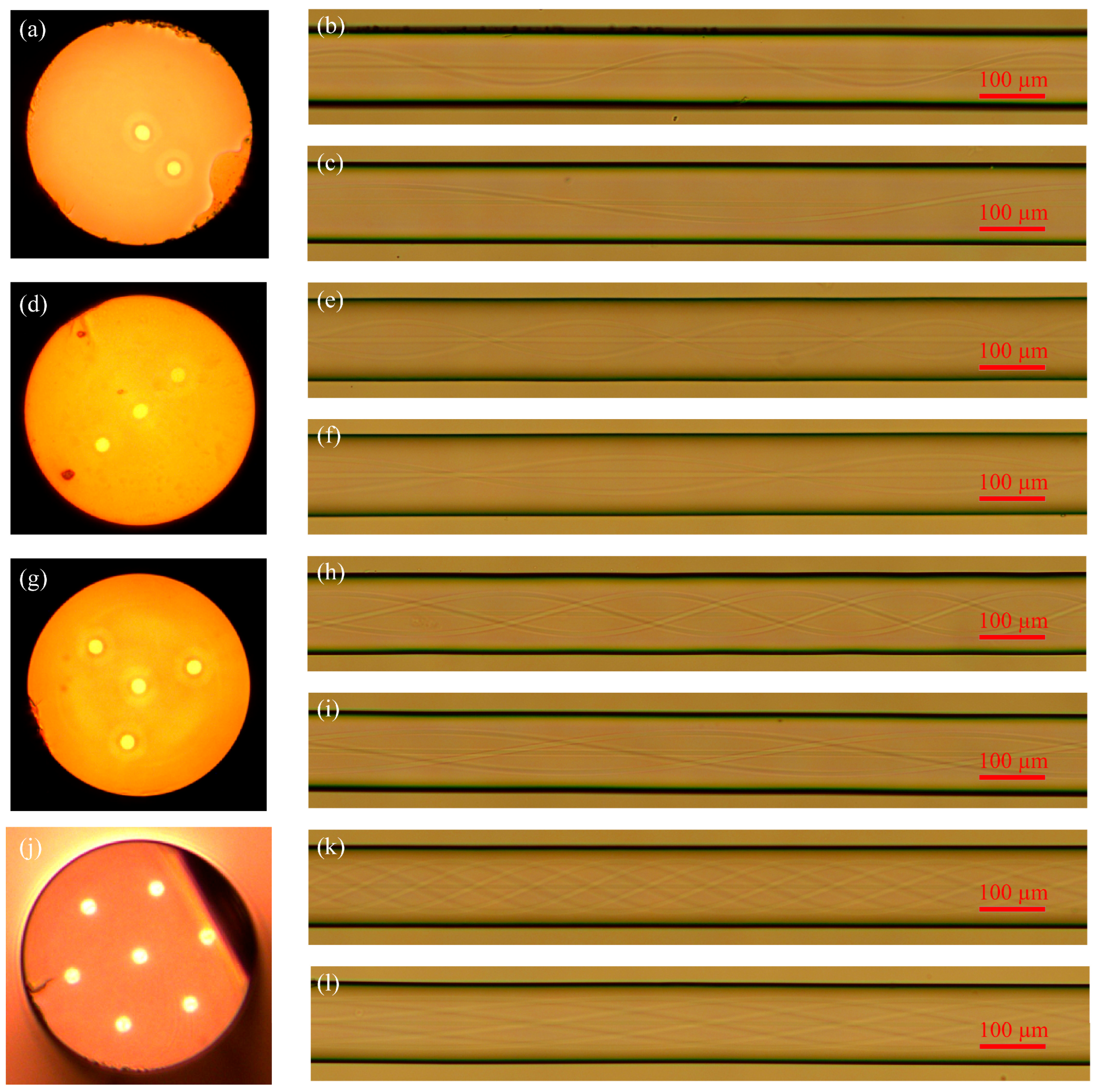
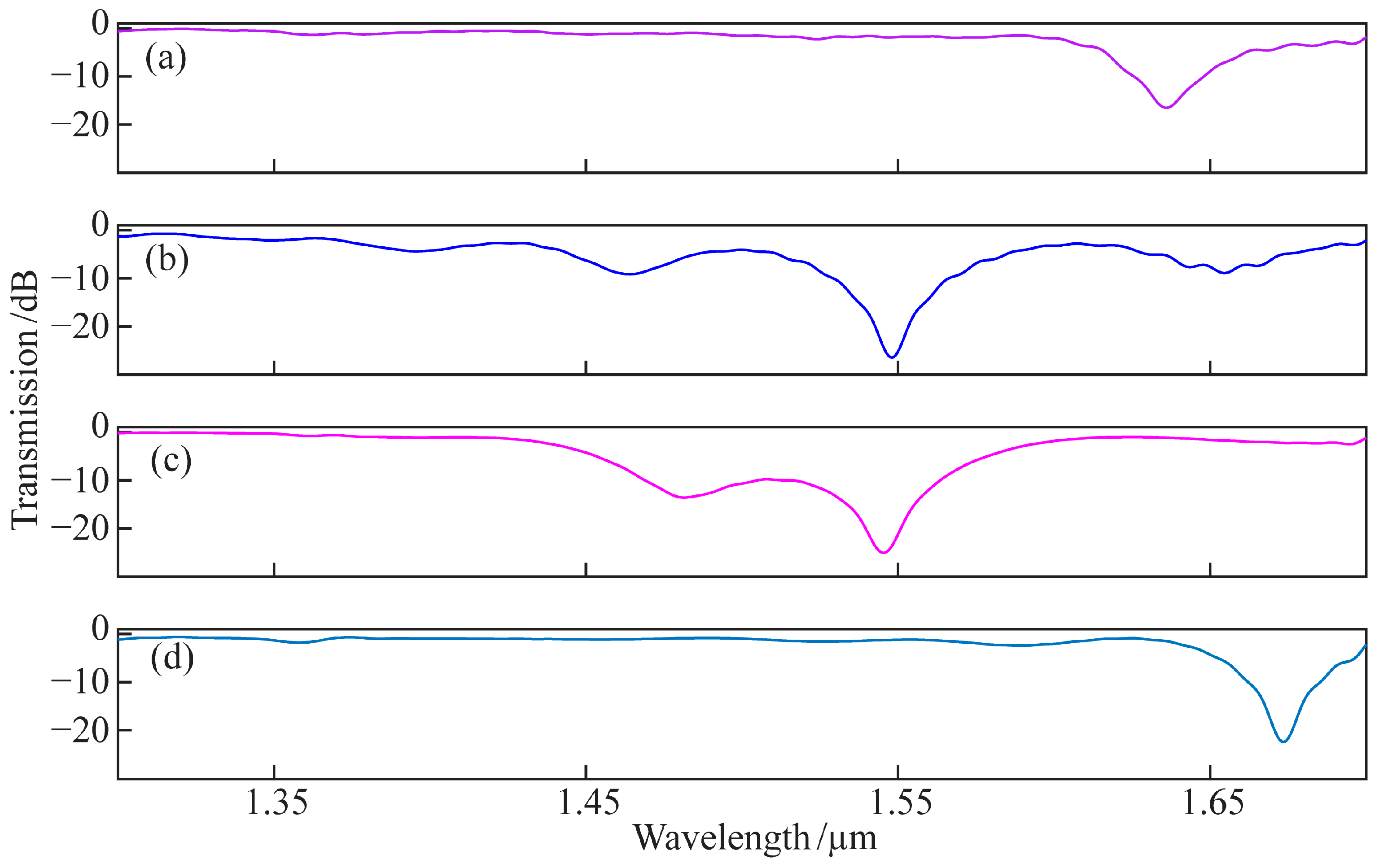
3.1. Microscopic Images of Prepared Helical Multi-Core Fibers
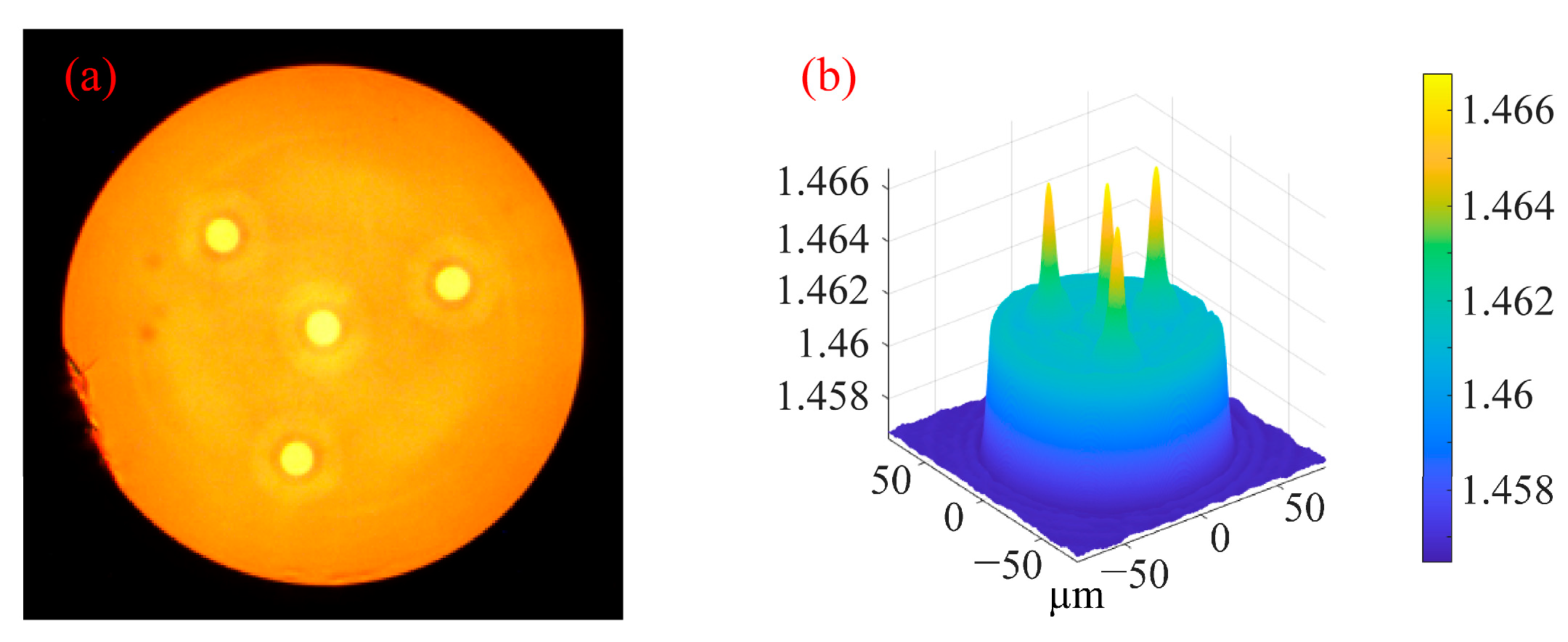
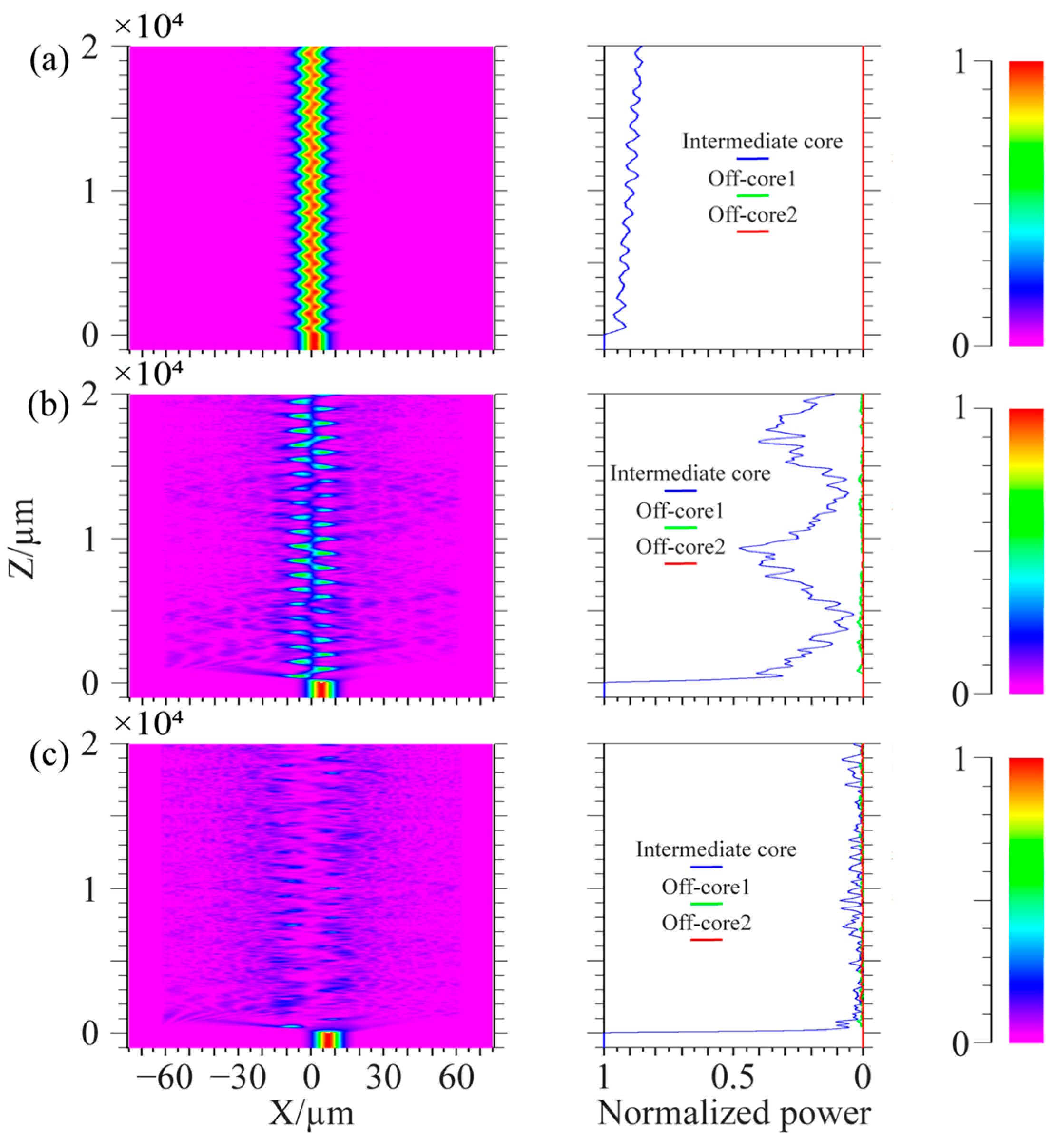
3.2. Optical Coupling between Multi-Core Fiber Cores
3.3. Multi-Core HLPFGs with an Intermediate Core
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Marcuse, D. Radiation loss of a helically deformed optical fiber. J. Opt. Soc. Am. 1976, 66, 1025–1031. [Google Scholar] [CrossRef]
- Berthold, J.W. Historical review of microbend fiber-optic sensor. J. Light. Technol. 1995, 13, 1193–1199. [Google Scholar] [CrossRef]
- Tomita, A.; Chiao, R.Y. Observation of berry’s topological phase by use of an optical fiber. Phys. Rev. Lett. 1986, 57, 937–940. [Google Scholar] [CrossRef] [PubMed]
- Kopp, V.I.; Churikov, V.M.; Singer, J.; Chao, N.; Neugroschl, D.; Genack, A.Z. Chiral fiber gratings. Science 2004, 305, 74–75. [Google Scholar] [CrossRef] [PubMed]
- Kopp, V.I.; Churikov, V.M.; Zhang, G.; Singer, J.; Draper, C.W.; Chao, N.; Neugroschl, D.; Genack, A.Z. Single- and double- helix chiral fiber sensors. J. Opt. Soc. Am. B Opt. Phys. 2007, 24, A48–A52. [Google Scholar] [CrossRef]
- Kopp, V.I.; Park, J.; Wlodawski, M.; Singer, J.; Neugroschl, D.; Genack, A.Z. Chiral fibers: Microformed optical waveguides for polarization control, sensing, coupling, amplification, and switching. J. Light. Technol. 2014, 32, 605–613. [Google Scholar] [CrossRef]
- Alexeyev, C.N.; Yavorsky, M.A. Optical vortices and the higher order modes of twisted strongly elliptical optical fibres. J. Opt. A Pure Appl. Opt. 2004, 6, 824–832. [Google Scholar] [CrossRef]
- Alexeyev, C.N.; Yavorsky, M.A. Generation and conversion of optical vortices in long-period helical-core optical fibers. Phys. Rev. A 2008, 78, 043828. [Google Scholar] [CrossRef]
- Wang, P.; Cooper, L.J.; Sahu, J.K.; Clarkson, W.A. Efficient single-mode operation of a cladding-pumped ytterbium-doped helical-core fiber laser. Opt. Lett. 2006, 31, 226–228. [Google Scholar] [CrossRef]
- Ma, X.; Liu, C.H.; Chang, G.; Galvanauskas, A. Angular-momentum coupled optical waves in chirally-coupled-core fibers. Opt. Express 2011, 19, 26515–26528. [Google Scholar] [CrossRef]
- Ma, X.; Zhu, C.; Hu, I.-N.; Kaplan, A.; Galvanauskas, A. Single-mode chirally-coupled-core fibers with larger than 50 μm diameter cores. Opt. Express 2014, 22, 9206–9219. [Google Scholar] [CrossRef] [PubMed]
- Wong, G.K.L.; Kang, M.S.; Lee, H.W.; Biancalana, F.; Conti, C.; Weiss, T.; Russell, P.S.J. Excitation of orbital angular momentum resonances in helically twisted photonic crystal fiber. Science 2012, 337, 446–449. [Google Scholar] [CrossRef] [PubMed]
- Xi, X.M.; Wong, G.L.K.; Weiss, T.; Russell, P.S.J. Measuring mechanical strain and twist using helical photonic crystal fiber. Opt. Lett. 2013, 38, 5401. [Google Scholar] [CrossRef] [PubMed]
- Xi, X.M.; Wong, G.K.L.; Frosz, M.H.; Babic, F.; Ahmed, G.; Jiang, X.; Euser, T.G.; Russell, P.S.J. Orbital-angular-momentum-preserving helical Bloch modes in twisted photonic crystal fiber. Optica 2014, 1, 165–169. [Google Scholar] [CrossRef]
- Alexeyev, C.N.; Lapin, B.P.; Yavorsky, M.A. The effect of spin–orbit coupling on the structure of the stopband in helical-core optical fibres. J. Opt. A Pure Appl. Opt. 2008, 10, 085006. [Google Scholar] [CrossRef]
- Napiorkowski, M.; Urbanczyk, W. Surface plasmon resonance effect in helical-core fibers. J. Opt. 2016, 18, 085001. [Google Scholar] [CrossRef]
- Wang, X.; Deng, H.; Yuan, L. Highly sensitive flexible SPR sensor based on side-polishing helical-core fiber: Theoretical analysis and experimental demonstration. Adv. Photonics Res. 2021, 2, 2000054. [Google Scholar] [CrossRef]
- Wang, X.; Deng, H.; Yuan, L. High sensitivity cascaded helical-core fiber SPR sensors. Chin. Opt. Lett. 2021, 19, 091201. [Google Scholar] [CrossRef]
- Ulrich, R.; Simon, A. Polarization optics of twisted single-mode fibers. Appl. Opt. 1979, 18, 2241–2251. [Google Scholar] [CrossRef]
- Zhong, J.; Liu, S.; Zou, T.; Yan, W.; Chen, P.; Liu, B.; Sun, Z.; Wang, Y. High-Sensitivity Optical Fiber-Based Glucose Sensor Using Helical Intermediate-Period Fiber Grating. Sensors 2022, 22, 6824. [Google Scholar] [CrossRef]
- Zhu, C.; Wang, P.; Zhao, H.; Mizushima, R.; Ishikami, S.; Li, H. DC-sampled helical fiber grating and its application to multi-channel OAM generator. IEEE Photonics Technol. Lett. 2019, 31, 1445–1448. [Google Scholar] [CrossRef]
- Liu, Y.; Deng, H.; Yuan, L. Arc-discharge-induced off-axis spiral long period fiber gratings and their sensing characteristics. Opt. Fiber Technol. 2019, 52, 101950. [Google Scholar] [CrossRef]
- Tachikra, M. Fusion mass-splicing for optical fibers using electric discharges between two pairs of electrodes. Appl. Opt. 1984, 23, 492–498. [Google Scholar] [CrossRef] [PubMed]
- Niu, C.; Ding, J.; Yang, F.; Dong, D.; Rong, M.; Xu, D. The Influence of Contact Space on the Arc Commutation Process in Air Circuit Breaker. Plasma Sci. Technol. 2016, 18, 460–464. [Google Scholar] [CrossRef]
- Li, Z.; Liu, S.; Bai, Z.; Fu, C.; Zhang, Y.; Sun, Z.; Liu, X.; Wang, Y. Residual-stress-induced helical long period fiber gratings for sensing applications. Opt. Express 2018, 26, 24114–24123. [Google Scholar] [CrossRef] [PubMed]
- Zhao, Y.; Peng, H.; Ma, Y.; Liu, Y.; Yang, Y.; He, Z. Helical long-period gratings in four-core fiber for multiparametric monitoring of directional bending, torsion and temperature. J. Light. Technol. 2022, 1–7. [Google Scholar] [CrossRef]
- Li, B.; Wong, L.J.; Shum, P.P.; Zheng, Y.; Zhang, H.; Wu, Z. Helical long-period grating on multicore fiber for refractive index sensing. In Proceedings of the 2020 IEEE Photonics Conference (IPC), Vancouver, BC, Canada, 28 September–1 October 2020; pp. 1–2. [Google Scholar] [CrossRef]
- Zhang, H.; Wu, Z.; Shum, P.P.; Shao, X.; Dinh, X.S.; Wang, R.; Fu, S.; Tang, M. Helical long period grating in multicore fiber for simultaneous measurement of torsion and temperature. In Proceedings of the Conference on Lasers and Electro-Optics Pacific Rim (CLEO-PR), Singapore, 31 July–4 August 2017. [Google Scholar] [CrossRef]
- Liu, S.; Zhou, M.; Shao, L.; Zhang, Z.; Bai, Z.; Wang, Y. Torsion-tunable OAM mode generator based on oxyhydrogen-flame fabricated helical long-period fiber grating. Opt. Express 2022, 30, 21085–21093. [Google Scholar] [CrossRef] [PubMed]
- Zhao, Y.; Liu, S.; Xiong, C.; Wang, Y.; Li, Z.; Sun, Z.; Li, J.; Wang, Y. Magnetic field sensor based on helical long-period fiber grating with a three-core optical fiber. Opt. Express 2021, 29, 20649–20656. [Google Scholar] [CrossRef]
- Sun, B.; Wei, W.; Liao, C.; Zhang, Z.; Chen, M.Y.; Wang, Y. Automatic arc discharge-induced helical long period fiber gratings and its sensing applications. IEEE Photonics Technol. Lett. 2017, 29, 873–876. [Google Scholar] [CrossRef]



| Device Structure | Preparation Method | Minimum Loss of Transmission Spectrum (dB) | Advantages and Disadvantages | Reference |
|---|---|---|---|---|
| Intermediate-core spiral long-period fiber grating | CO2 laser | >1 | Advantages: Flexible and high-quality. Disadvantages: Cumbersome debugging of the optical path and expensive. | [26] |
| >1 | [27] | |||
| >1 | [28] | |||
| Oxyhydrogen flame | ≈1 | Advantages: Wide heating area and uniform heating temperature. | [29] | |
| >1 | Disadvantages: Danger of using hydrogen. | [30] | ||
| Two-electrode arc discharge | >1 | Advantages: Simple and flexible. | [22] | |
| >1 | Disadvantages: Narrow constant-temperature zone. | [31] | ||
| Four-electrode arc discharge | <1 | Advantages: simple and flexible, large constant-temperature zone, and cheap price. | This work | |
| Disadvantages: The arc needs further optimization |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, J.; Ma, C.; Duan, S.; Wang, D.; Yuan, L. Arc Discharge System for Micromachining of Helical Fiber. Micromachines 2023, 14, 1120. https://doi.org/10.3390/mi14061120
Wang J, Ma C, Duan S, Wang D, Yuan L. Arc Discharge System for Micromachining of Helical Fiber. Micromachines. 2023; 14(6):1120. https://doi.org/10.3390/mi14061120
Chicago/Turabian StyleWang, Jian, Chao Ma, Shaochen Duan, Donghui Wang, and Libo Yuan. 2023. "Arc Discharge System for Micromachining of Helical Fiber" Micromachines 14, no. 6: 1120. https://doi.org/10.3390/mi14061120
APA StyleWang, J., Ma, C., Duan, S., Wang, D., & Yuan, L. (2023). Arc Discharge System for Micromachining of Helical Fiber. Micromachines, 14(6), 1120. https://doi.org/10.3390/mi14061120







