Micro-Raman for Local Strain Evaluation of GaN LEDs and Si Chips Assembled on Cu Substrates
Abstract
:1. Introduction
2. Materials and Methods
2.1. Si and GaN Samples
2.2. Set up of Raman Measurements
2.3. Determination of Strain Induced from Raman Measurements
3. Results
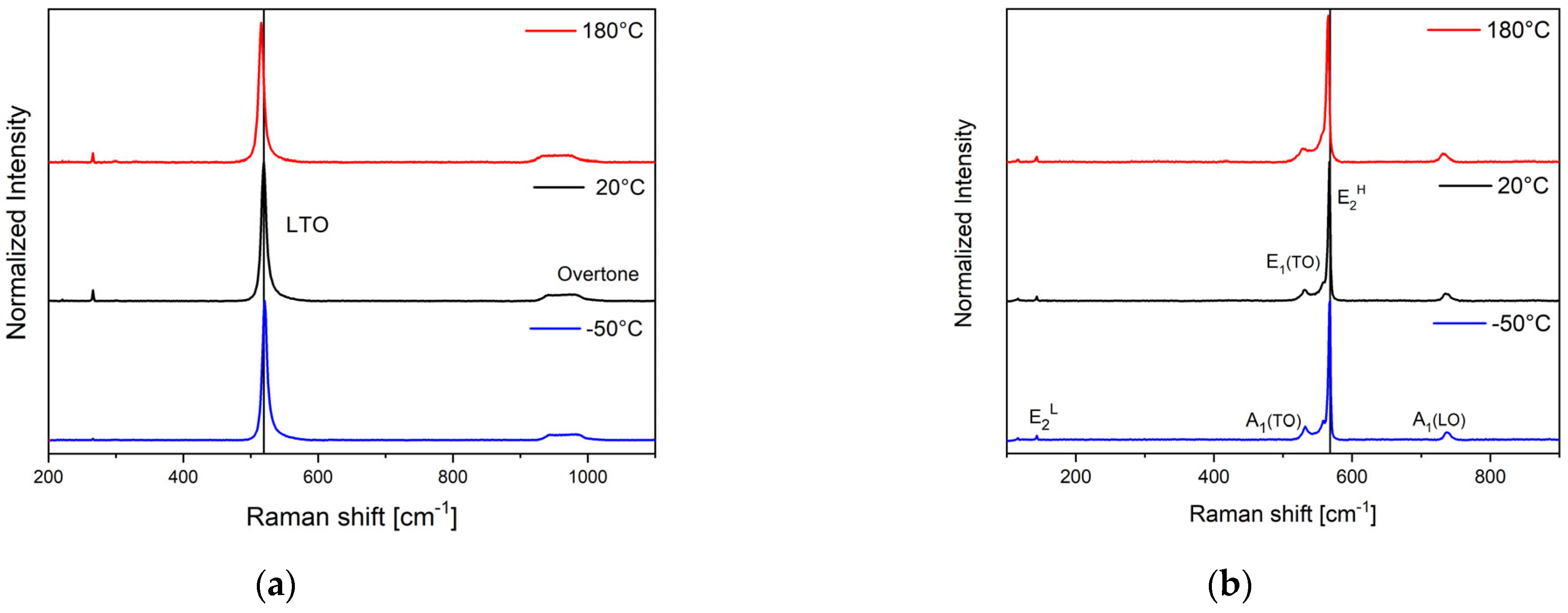
3.1. Raman Spectra of Si and GaN Samples
3.2. Samples at Room Temperature
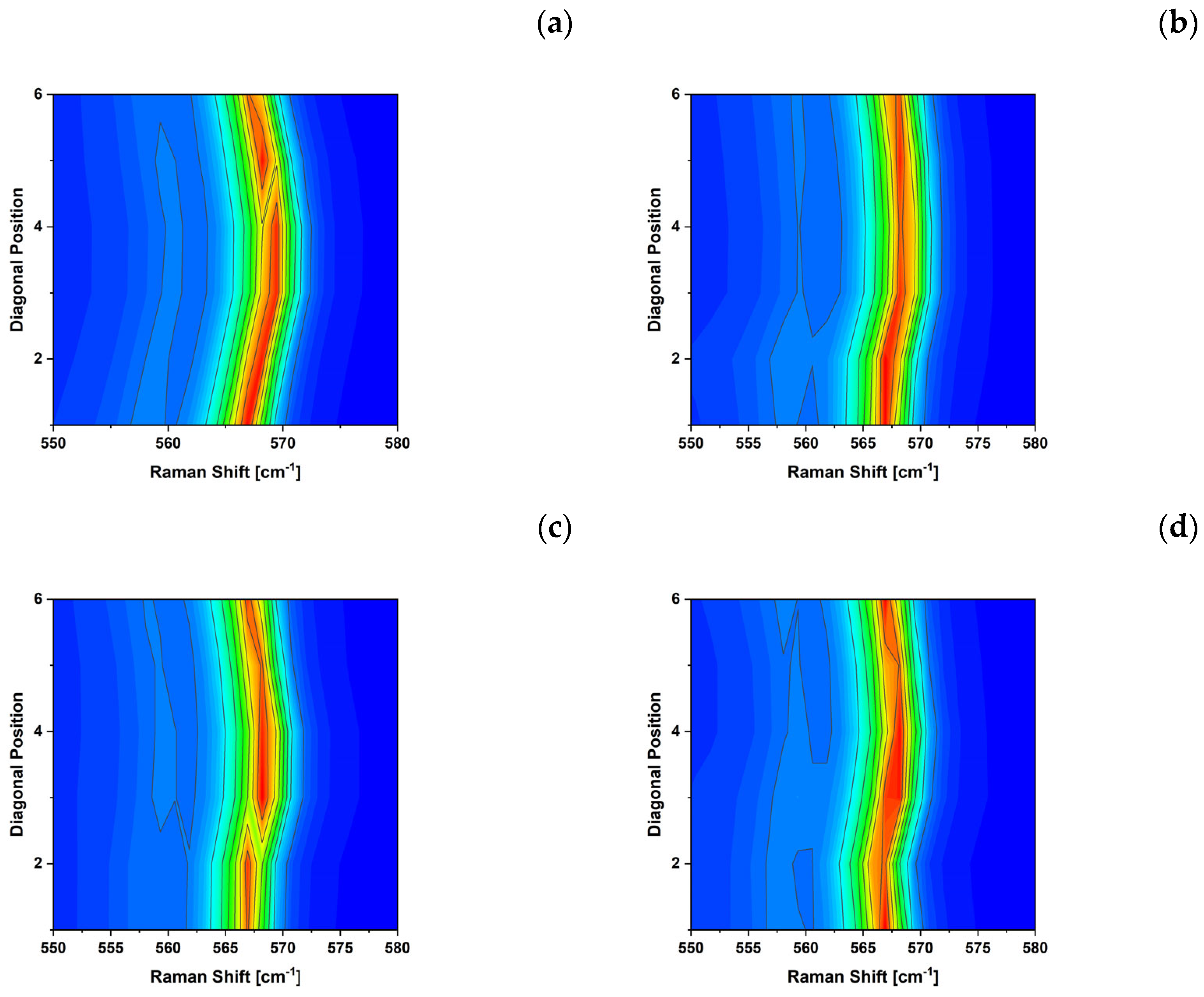
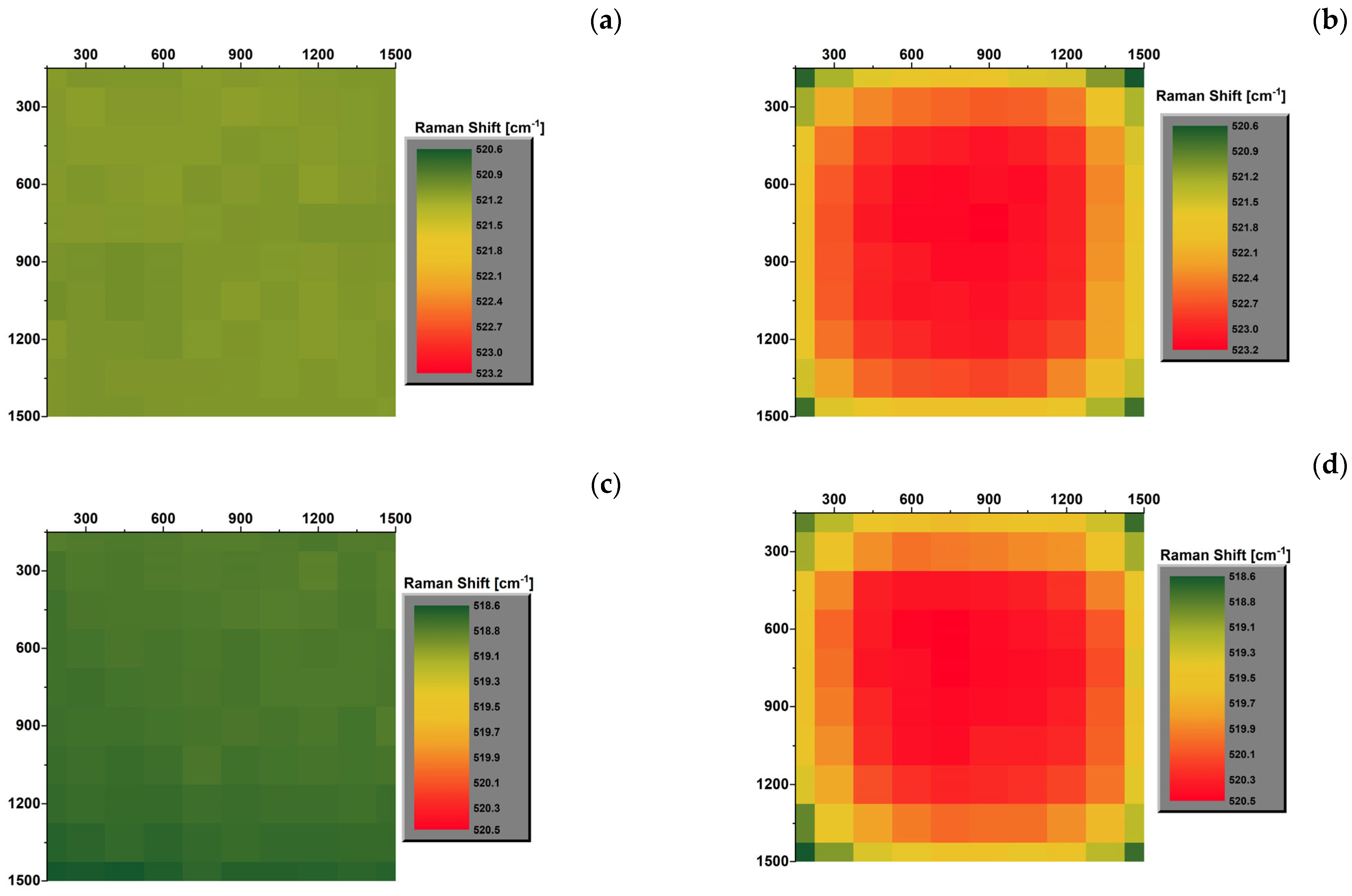
3.3. Raman Mapping of Samples at Different Temperatures
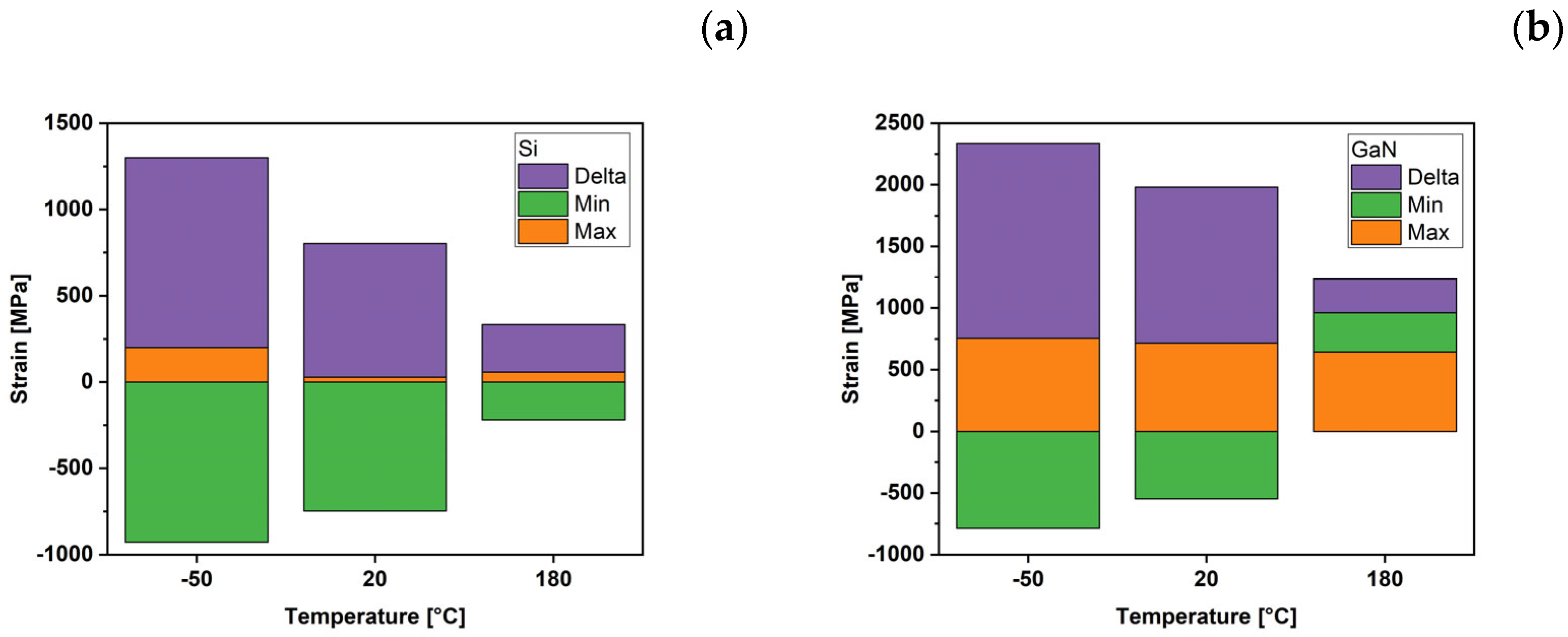
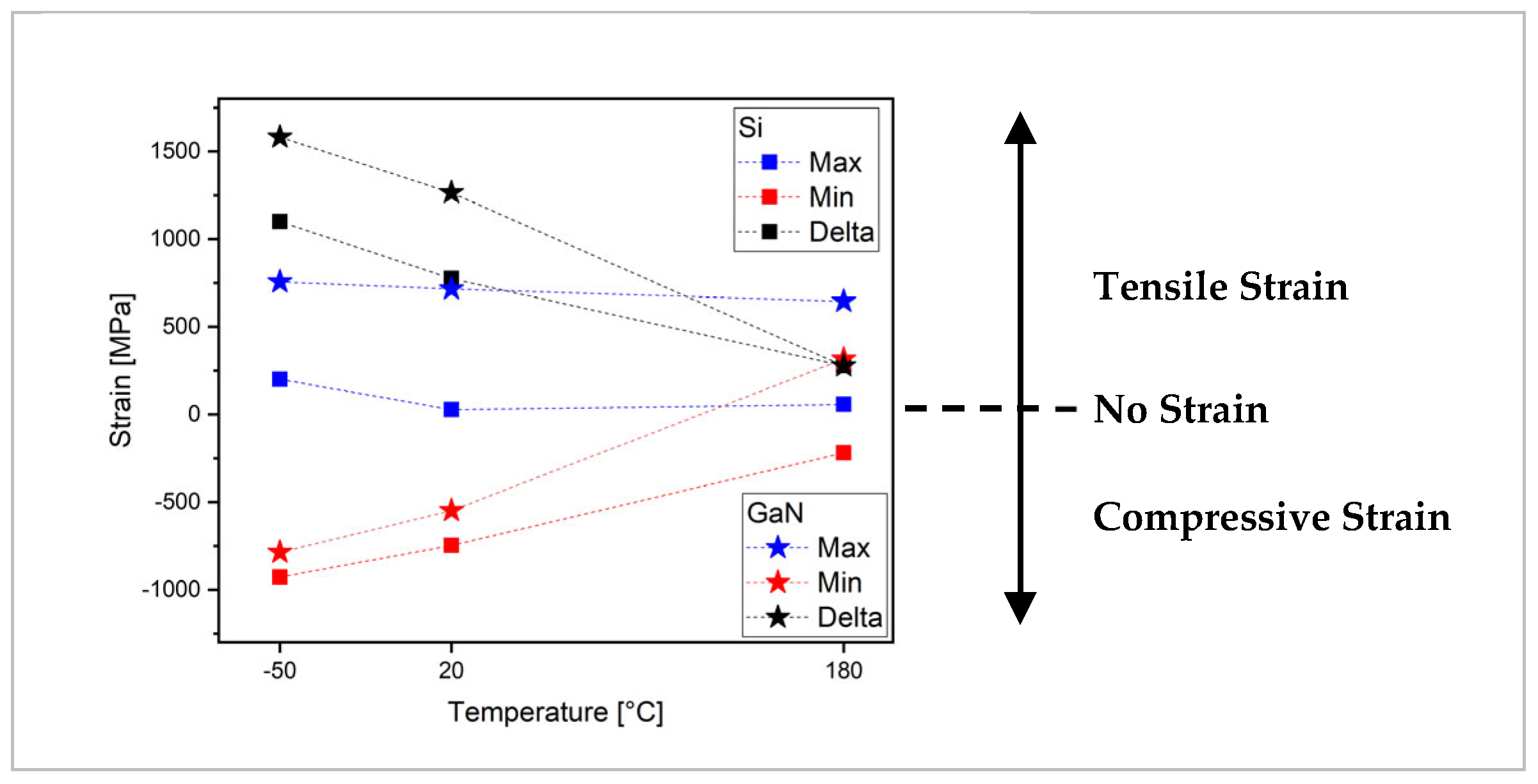
3.4. Strain Evaluation
4. Discussion
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Bullough, J.D. 20—LEDs and Automotive Lighting Applications. In Woodhead Publishing Series in Electronic and Optical Materials, Nitride Semiconductor Light-Emitting Diodes (LEDs), 2nd ed.; Huang, J.J., Kuo, H.C., Shen, S.-C., Eds.; Woodhead Publishing: Sawston, UK, 2018; pp. 647–658. [Google Scholar] [CrossRef]
- Nguyen, Q.K.; Lin, Y.J.; Sun, C.; Lee, X.-H.; Lin, S.-K.; Wu, C.-S.; Yang, T.-H.; Wu, T.-L.; Lee, T.-X.; Chien, C.-H.; et al. GaN-based mini-LED matrix applied to multi-functional forward lighting. Sci. Rep. 2022, 12, 6444. [Google Scholar] [CrossRef]
- Akasaki, I.; Amano, H. Crystal growth and conductivity control of group III nitride semiconductors and their application to short wavelength light emitters. Jpn. J. Appl. Phys. 1997, 36, 5393–5408. [Google Scholar] [CrossRef]
- Nakamura, S. The Roles of Structural Imperfections in InGaN-Based Blue Light-Emitting Diodes and Laser Diodes. Science 1998, 281, 956–961. [Google Scholar] [CrossRef]
- Jia, H.; Guo, L.; Wang, W.; Chen, H. Recent Progress in GaN-Based Light-Emitting Diodes. Adv. Mat. 2009, 21, 4641–4646. [Google Scholar] [CrossRef]
- Kim, H.; Ohta, J.; Ueno, K.; Kobayashi, A.; Morita, M.; Tokumoto, Y.; Fujiok, H. Fabrication of full-color GaN-based light-emitting diodes on nearly lattice-matched flexible metal foils. Sci. Rep. 2017, 7, 2112. [Google Scholar] [CrossRef]
- Langpoklakpam, C.; Liu, A.-C.; Hsiao, Y.-K.; Lin, C.-H.; Kuo, H.-C. Vertical GaN MOSFET Power Devices. Micromachines 2023, 14, 1937. [Google Scholar] [CrossRef]
- Rafin, S.M.S.H.; Ahmed, R.; Haque, M.A.; Hossain, M.K.; Haque, M.A.; Mohammed, O.A. Power Electronics Revolutionized: A Comprehensive Analysis of Emerging Wide and Ultrawide Bandgap Devices. Micromachines 2023, 14, 2045. [Google Scholar] [CrossRef]
- Fathy, M.; Gad, S.; Anis, B.; Kashyout, A.E.-H.B. Crystal Growth of Cubic and Hexagonal GaN Bulk Alloys and Their Thermal-Vacuum-Evaporated Nano-Thin Films. Micromachines 2021, 12, 1240. [Google Scholar] [CrossRef]
- Sheremet, V.; Gheshlaghi, N.; Sözen, M.; Elçi, M.; Sheremet, N.; Aydınlı, A.; Altuntaş, I.; Ding, K.; Avrutin, V.; Özgür, Ü.; et al. InGaN strain compensation layers in InGaN/GaN blue LEDs with step graded electron injectors. Superlattices Microstruct. 2018, 116, 253–261. [Google Scholar] [CrossRef]
- Dvořák, N.; Fazeli, N.; Ku, P.-C. Direct Shear Strain Mapping Using a Gallium Nitride LED-Based Tactile Sensor. Micromachines 2023, 14, 916. [Google Scholar] [CrossRef] [PubMed]
- De Wolf, I.; Maes, H.E.; Jones, S.K. Strain measurements in silicon devices through Raman spectroscopy: Bridging the gap between theory and experiment. J. Appl. Phys. 1996, 79, 7148–7156. [Google Scholar] [CrossRef]
- De Wolf, I. Micro-Raman spectroscopy to study local mechanical strain in silicon integrated circuits. Semicond. Sci. Technol. 1996, 11, 139. [Google Scholar] [CrossRef]
- Ross, A.J.; Mara, M.; Mihailova, B.; Alvaro, M. Stress, strain and Raman shifts. Z. Krist. Cryst. Mater. 2019, 234, 129–140. [Google Scholar] [CrossRef]
- Li, Q.; Qiu, W.; Tan, H.; Guo, J.; Kang, Y. Micro-Raman spectroscopy stress measurement method for porous silicon film. Opt. Lasers Eng. 2010, 48, 1119–1125. [Google Scholar] [CrossRef]
- Schaffer, B.; Mitterbauer, C.; Schertel, A.; Pogantsch, A.; Rentenberger, S.; Zojer, E.; Hofer, F. Cross-section analysis of organic light-emitting diodes. Ultramicroscopy 2004, 101, 123–128. [Google Scholar] [CrossRef]
- Stuer, C.; Van Landuyt, J.; Bender, H.; De Wolf, I.; Rooyackers, R.; Badenes, G. Investigation by Convergent Beam Electro Diffraction of the Stress around Shallow Trench Isolation Structures. J. Electrochem. Soc. 2001, 148, G597–G601. [Google Scholar] [CrossRef]
- Schmidt, D.; Durfee, C.; Li, J.; Loubet, N.; Cepler, A.; Neeman, L.; Meir, N.; Ofek, J.; Oren, Y.; Fishman, D. In-Line Raman Spectroscopy for Stacked Nanosheet Device Manufacturing. In Proceedings of the SPIE 11611, Metrology, Inspection, and Process Control for Semiconductor Manufacturing XXXV, 116111T, Virtual, 25 February 2021. [Google Scholar] [CrossRef]
- Hahn, B.; Galler, B.; Engl, K. Development of high-efficiency and high-power vertical light emitting diodes. Jpn. J. Appl. Phys. 2014, 53, 100208. [Google Scholar] [CrossRef]
- Plößl, A.; Baur, J.; Eißler, D.; Engl, K.; Härle, V.; Hahn, B.; Heindl, A.; Illek, S.; Klemp, C.; Rode, P.; et al. Wafer Bonding for the Manufacture of High-Brightness and High-Efficiency Light-Emitting Diodes. ECS Trans. 2010, 33, 613–624. [Google Scholar] [CrossRef]
- Bhogaraju, S.K.; Conti, F.; Kotadia, H.R.; Keim, S.; Tetzlaff, U.; Elger, G. Novel approach to copper sintering using surface enhanced brass micro flakes for microelectronics packaging. J. Alloys Compd. 2020, 844, 156043. [Google Scholar] [CrossRef]
- Bhogaraju, S.K.; Kotadia, H.R.; Conti, F.; Mauser, A.; Rubenbauer, T.; Bruetting, R.; Schneider-Ramelow, M.; Elger, G. Die-Attach Bonding with Etched Micro Brass Metal Pigment Flakes for High-Power Electronics Packaging. ACS Appl. Electron. Mater. 2021, 3, 4587–4603. [Google Scholar] [CrossRef]
- Liu, E.; Conti, F.; Bhogaraju, S.K.; Signorini, R.; Pedron, D.; Wunderle, B.; Elger, G. Thermomechanical stress in GaN-LEDs soldered onto Cu substrates studied using finite element method and Raman spectroscopy. J. Raman Spectrosc. 2020, 51, 2083–2094. [Google Scholar] [CrossRef]
- Signorini, R.; Pedron, D.; Conti, F.; Hanss, A.; Bhogaraju, S.K.; Elger, G. Thermomechanical Strain in GaN LED Soldered on Copper Substrate Evaluated by Raman Measurements and Computer Modelling. In Proceedings of the 24th International Workshop on Thermal Investigations of ICs and Systems (THERMINIC 2018), Stockholm, Sweden, 26–28 September 2018. [Google Scholar] [CrossRef]
- Liu, E.; Conti, F.; Signorini, R.; Brugnolotto, E.; Bhogaraju, S.K.; Elger, G. Modelling thermo-mechanical strain in GaN-LEDs soldered on copper substrate with simulations validated by Raman experiments. In Proceedings of the 20th International Conference on Thermal, Mechanical and Multi-Physics Simulation and Experiments in Microelectronics and Microsystems (EuroSimE 2019), Hannover, Germany, 24–27 March 2019. [Google Scholar] [CrossRef]
- Tuschel, D. Stress, Strain, and Raman Spectroscopy. Spectroscopy 2019, 34, 10–21. Available online: https://www.spectroscopyonline.com/view/stress-strain-and-raman-spectroscopy (accessed on 19 December 2023).
- Briggs, J.; Ramdas, A.K. Piexospectroscopic study of the Raman spectrum of cadmium sulfide. Phys. Rev. B 1976, 13, 5518. [Google Scholar] [CrossRef]
- Davydov, V.Y.; Averkiev, N.S.; Goncharuk, I.N.; Nelson, D.K.; Nikitina, I.P.; Polkovnikov, A.S.; Smirnov, A.N.; Jacobson, M.A.; Semchinova, O.K. Raman and photoluminescence studies of biaxial strain in GaN epitaxial layers grown on 6H–SiC. J. Appl. Phys. 1997, 82, 5097. [Google Scholar] [CrossRef]
- Demangeot, F.; Frandon, J.; Renucci, M.A.; Briot, O.; Gilb, B.; Aulombard, R.L. Raman determination of phonon deformation potentials in cx-gan. Solid State Commun. 1996, 100, 207–210. [Google Scholar] [CrossRef]
- Kim, S.T.; Lee, Y.J.; Moon, D.C.; Lee, C.; Park, H.Y. Optical Properties of Thick-Film GaN Grown by Hydride Vapor Phase Epitaxy on MgAl2O4 Substrate. J. Electron. Mater. 1998, 27, 10. [Google Scholar] [CrossRef]
- Kisielowski, C.; Kruger, J.; Ruvimov, S.; Suski, T.; Ager III, J.W.; Jones, E.; Liliental-Weber, Z.; Rubin, M.; Weber, E.R. Strain-related phenomena in GaN thin films. Phys. Rev. B 1996, 54, 24. [Google Scholar] [CrossRef]
- Chaudhuri, J.; Ng, M.H.; Koleske, D.D.; Wickenden, A.E.; Henry, R.L. High resolution X-ray diffraction and X-ray topography study of GaN on sapphire. Mater. Sci. Eng. B 1999, 64, 99–106. [Google Scholar] [CrossRef]
- Tripathy, S.; Soni, R.K.; Asahi, H.; Iwata, K.; Kuroiwa, R.; Asami, K.; Gonda, S. Optical properties of GaN layers grown on C-, A-, R-, and M-plane sapphire substrates by gas source molecular beam epitaxy. J. Appl. Phys. 1999, 85, 8386. [Google Scholar] [CrossRef]
- Kozawa, T.; Kachi, T.; Kano, H.; Nagase, H.; Koide, N.; Manabe, K. Thermal strain in GaN epitaxial layers grown on sapphire substrates. J. Appl. Phys. 1995, 77, 4389. [Google Scholar] [CrossRef]
- Rieger, W.; Metzger, T.; Angerer, H.; Dimitrov, R.; Ambacher, O.; Stutzmann, M. Influence of substrate-induced biaxial compressive strain on the optical properties of thin GaN films. Appl. Phys. Lett. 1996, 68, 970. [Google Scholar] [CrossRef]
- Choi, S.; Heller, E.; Dorsey, D.; Vetury, R.; Graham, S. The impact of mechanical stress on the degradation of AlGaN/GaN high electron mobility transistors. J. Appl. Phys. 2013, 114, 164501. [Google Scholar] [CrossRef]
- Bagnall, K.R.; Moore, E.A.; Badescu, S.C.; Zhang, L.; Wang, E.N. Simultaneous measurement of temperature, stress, and electric field in GaN HEMTs with micro-Raman spectroscopy. Rev. Sci. Instrum. 2017, 88, 113111. [Google Scholar] [CrossRef] [PubMed]
- Anastassakis, E.; Pinczuk, A.; Burstein, E.; Pollak, F.H.; Cardona, M. Effect of static uniaxial stress on the Raman spectrum of silicon. Solid State Commun. 1993, 88, 1053–1058. [Google Scholar] [CrossRef]
- Mezzalira, C.; Conti, F.; Pedron, D.; Signorini, R. Thermomechanical Stresses in Silicon Chips for Optoelectronic Devices. Appl. Sci. 2023, 13, 2737. [Google Scholar] [CrossRef]
- Hopcroft, M.A.; Nix, W.D.; Kenny, T.W. What is the Young’s Modulus of Silicon? J. Microelectromech. Syst. 2010, 19, 229–238. [Google Scholar] [CrossRef]
- Miyatake, T.; Pezzotti, G. Determination of stress components in a complex stress condition using micro-Raman spectroscopy. Phys. Status Solidi Appl. Mater. Sci. 2011, 208, 1151–1158. [Google Scholar] [CrossRef]
- Parker, J.H.; Feldman, D.W.; Ashkin, M. Raman Scattering by Silicon and Germanium. Phys. Rev. 1967, 155, 712–714. [Google Scholar] [CrossRef]
- Harima, H. Properties of GaN and related compounds studied by means of Raman scattering. J. Phys. Condens. Matter 2002, 14, R967–R993. [Google Scholar] [CrossRef]
- Pecht, M. Handbook of Electronic Package Design; CRC Press: Boca Raton, FL, USA, 1991. [Google Scholar] [CrossRef]
- Engineering Tool Box. Coefficients of Linear Thermal Expansion. 2003. Available online: https://www.engineeringtoolbox.com/linear-expansion-coefficients-d_95.html (accessed on 19 December 2023).
- Zhou, T.; Bobal, T.; Oud, M.; Songliang, J. Au/Sn Solder Alloy and Its Applications in Electronics Packaging; Coining Inc.: Montvale, NJ, USA, 1999; pp. 1–7. Available online: https://www.inseto.co.uk/wp-content/uploads/2020/05/Gold-Tin-Soldering-in-Electronic-Packaging.pdf (accessed on 19 December 2023).
- Jin, T. Investigation on Viscoplastic Properties of Au-Sn Die-Attach Solder. Master’s Thesis, Delft University of Technology, Delft, The Netherlands, 24 October 2017. [Google Scholar]
- Leszczynski, M.; Suski, T.; Teisseyre, H.; Perlin, P.; Grzegory, I.; Jun, J.; Porowski, S. Thermal expansion of gallium nitride. J. Appl. Phys. 1994, 76, 4909. [Google Scholar] [CrossRef]
- Tan, K.-Z.; Lee, S.-K.; Low, H.-C. LED lifetime prediction under thermal-electrical stress. IEEE Trans. Device Mater. Reliab. 2021, 21, 310–319. [Google Scholar] [CrossRef]
- Ke, H.; Hao, J.; Tu, J.; Miao, P.; Wang, C.; Chen, D.; Yang, S.; Cui, J. Study on the chromaticity of LED lamps given by online test during accelerated aging under thermal stress. Optik 2018, 164, 510–518. [Google Scholar] [CrossRef]
- Lee, J.; Known, K.-Y. Thermal stress analysis for high CRI LED indoor lighting module. J. Microel. Electron. Packag. 2018, 15, 126–131. [Google Scholar] [CrossRef]
- Roccato, N.; Piva, F.; De Santi, C.; Zanoni, E.; Meneghini, M. Modeling the electrical characteristic of InGaN/GaN blue-violet LED structure under electrical stress. Microelecytonics Reliab. 2022, 138, 114724. [Google Scholar] [CrossRef]
- Elger, G.; Kandaswamy, S.V.; Derix, R.; Conti, F. Detection of Solder Joint Cracking of High Power Leds on AI-IMS during Temperature Shock Test by Transient Thermal Analysis. In Proceedings of the 20th International Workshop on Thermal Investigations of ICs and Systems (THERMINIC 2014), Greenwich, UK, 24–26 September 2014; p. 6972530. [Google Scholar] [CrossRef]
- Elger, G.; Kandaswamy, S.V.; Von Kouwen, M.; Derix, R. In-Situ Measurements of the Relative Thermal Resistance: Highly Sensitive Method to Detect Crack Propagation in Solder Joints. In Proceedings of the 2014 IEEE 64th Electronic Components and Technology Conference (ECTC), Orlando, FL, USA, 27–30 May 2014; pp. 1464–1470. [Google Scholar] [CrossRef]
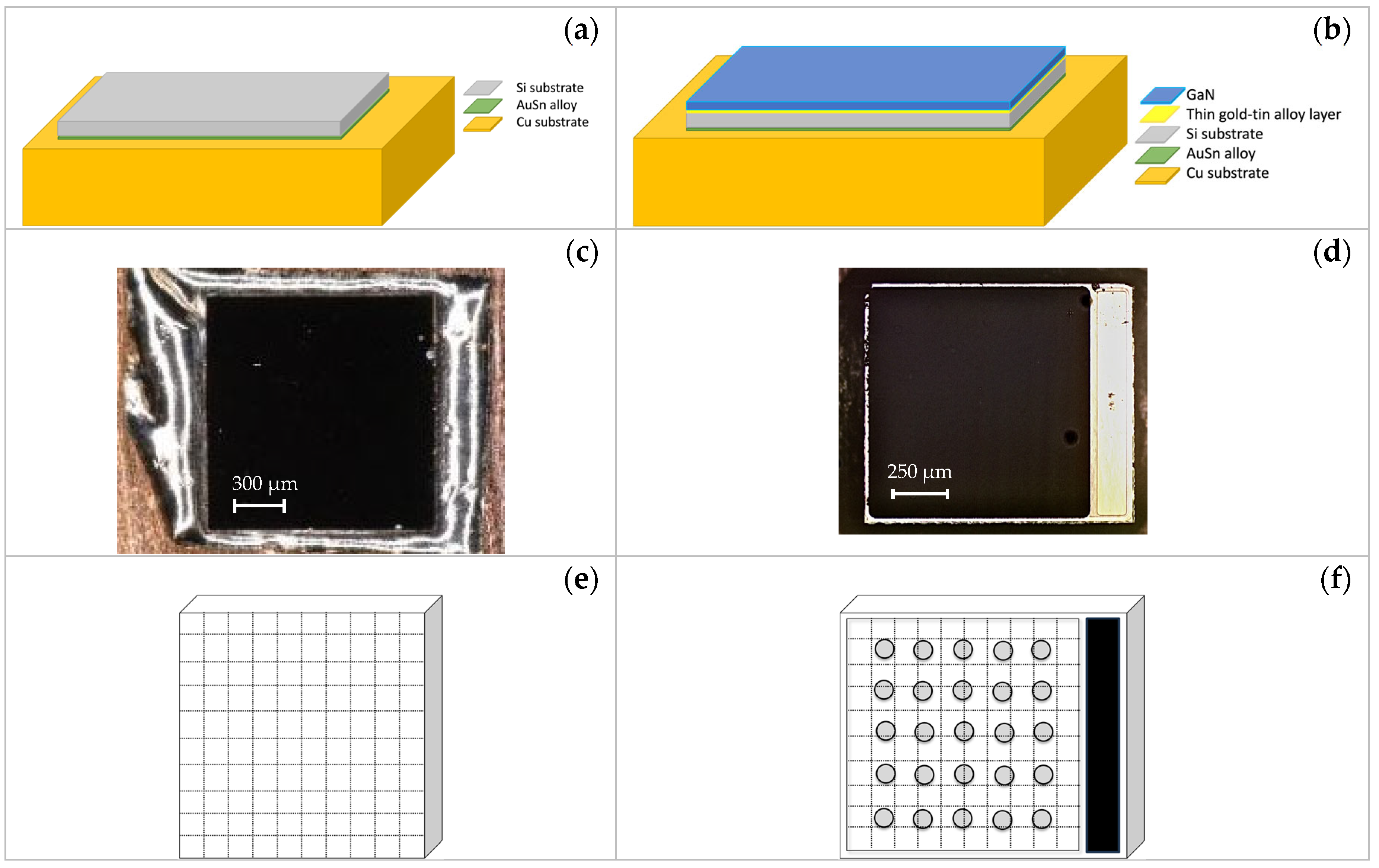

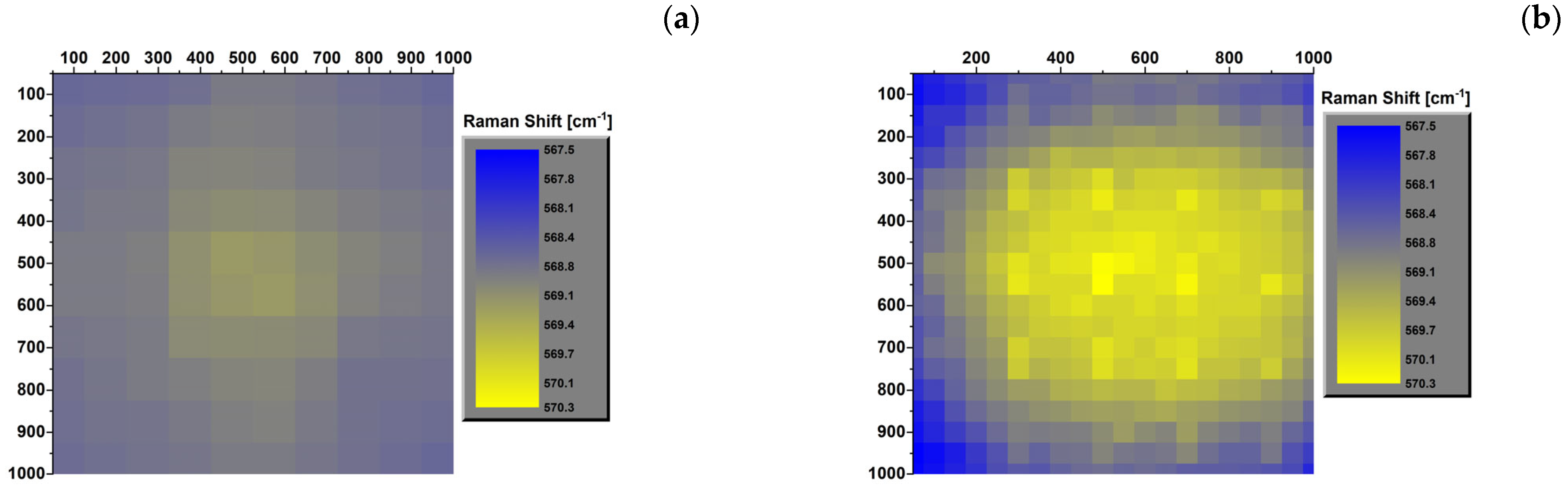
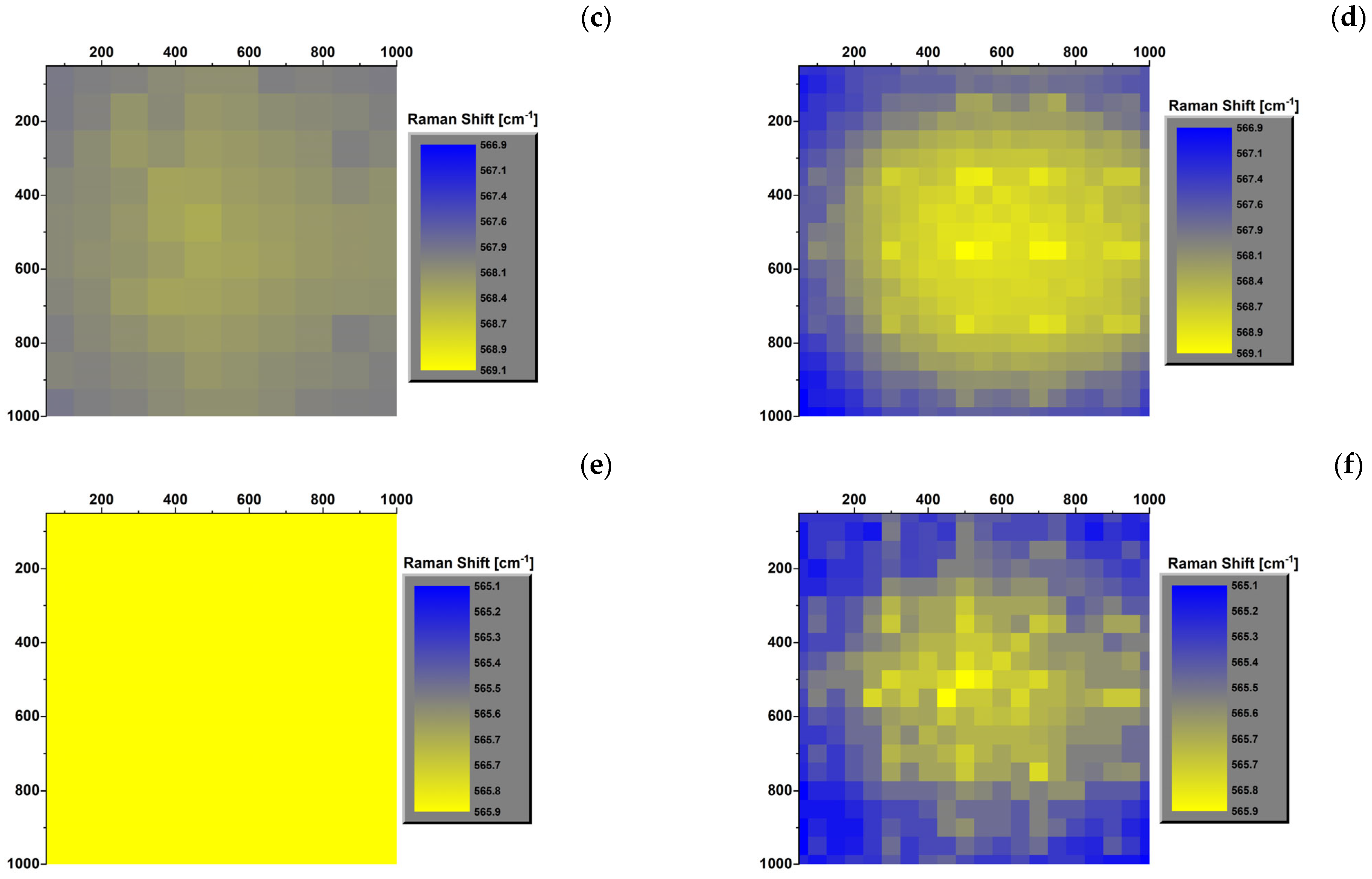

| Sample Name | Chip Dimensions [mm3] | Number of Investigated Samples | Cu Substrate Dimensions [mm3] | Pressure [N] |
|---|---|---|---|---|
| Si1–Si3 | 1.5 × 1.5 × 0.12 | 3 | / | / |
| A_Si_p | 3 | 5 × 5 × 1 | 5 | |
| A_Si | 3 | 5 × 5 × 1 | / | |
| SV1-3 LED | 1 × 1.2 × 0.12 | 3 | / | / |
| S1 | 1 | 6 × 6 × 1.5 | / | |
| S2 | 1 | 6 × 6 × 0.2 | / | |
| S3 | 1 | 6 × 6 × 1.5 | 21 | |
| S4 | 1 | 6 × 6 × 0.2 | 21 |
| K [cm−1 GPa−1] | Growth Process | Substrate Type | Thickness [µm] | References |
|---|---|---|---|---|
| 2.7 | MOCVD (samples) CHVPE (reference) | 6H Silicon carbide | 0.5–3 300 | [28] |
| 2.9 | MOPVE | Sapphire | unspecified | [29] |
| 3.93 | HVPE | Spinel | 10–100 | [30] |
| 4.2 | MOCVD, MBE | Sapphire (AlN buffer, homoepitaxial buffer) | 0.5–5 | [31] |
| 4.3 | MOCVD | Si, SiC | 1 | [32] |
| 4.86 | MBE | Sapphire (different orientations, GaN buffer) | 0.3–1 | [33] |
| 6.2 | MOCVD | Sapphire (50 nm AlN buffer) | 2.5–50 | [34] |
| 7.9 | MOCVD | Sapphire (20–250 nm AlN buffer) | 0.75 | [35] |
| Sample | [cm−1] | ||
|---|---|---|---|
| −50 °C | 20 °C | 180 °C | |
| Si | 0.11 | 0.22 | 0.23 |
| A_Si_p | - | - | - |
| A_Si | 2.59 | 1.88 | 0.55 |
| SV1-3 Pristine LED | 0.56 | 0.46 | 0.53 |
| S1 | 2.81 | 2.34 | 0.82 |
| S2 | 0.65 | 1.64 | 0.39 |
| S3 | 2.31 | 1.86 | 0.52 |
| S4 | 1.87 | 1.65 | 0.87 |
| Temperature [°C] | Maximum Value | Minimum Value | Average Value | Maximum Variation |
|---|---|---|---|---|
| −50 | 200 ± 22 | −928 ± 22 | −535 ± 30 | 1100 ± 44 |
| 20 | 28 ± 22 | −747 ± 22 | −471 ± 21 | 775 ± 44 |
| 180 | 57 ± 22 | −219 ± 22 | −110 ± 6 | 276 ± 44 |
| Temperature [°C] | Maximum Value | Minimum Value | Average Value | Maximum Variation |
|---|---|---|---|---|
| −50 | 755 ± 22 | −787 ± 22 | −200 ± 35 | 1542 ± 44 |
| 20 | 716 ± 22 | −548 ± 22 | −35 ± 27 | 1264 ± 44 |
| 180 | 645 ± 22 | 316 ± 22 | 515 ± 6 | 329 ± 44 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Brugnolotto, E.; Mezzalira, C.; Conti, F.; Pedron, D.; Signorini, R. Micro-Raman for Local Strain Evaluation of GaN LEDs and Si Chips Assembled on Cu Substrates. Micromachines 2024, 15, 25. https://doi.org/10.3390/mi15010025
Brugnolotto E, Mezzalira C, Conti F, Pedron D, Signorini R. Micro-Raman for Local Strain Evaluation of GaN LEDs and Si Chips Assembled on Cu Substrates. Micromachines. 2024; 15(1):25. https://doi.org/10.3390/mi15010025
Chicago/Turabian StyleBrugnolotto, Enrico, Claudia Mezzalira, Fosca Conti, Danilo Pedron, and Raffaella Signorini. 2024. "Micro-Raman for Local Strain Evaluation of GaN LEDs and Si Chips Assembled on Cu Substrates" Micromachines 15, no. 1: 25. https://doi.org/10.3390/mi15010025
APA StyleBrugnolotto, E., Mezzalira, C., Conti, F., Pedron, D., & Signorini, R. (2024). Micro-Raman for Local Strain Evaluation of GaN LEDs and Si Chips Assembled on Cu Substrates. Micromachines, 15(1), 25. https://doi.org/10.3390/mi15010025







