Hot-Carrier Damage in N-Channel EDMOS Used in Single Photon Avalanche Diode Cell through Quasi-Static Modeling
Abstract
1. Introduction
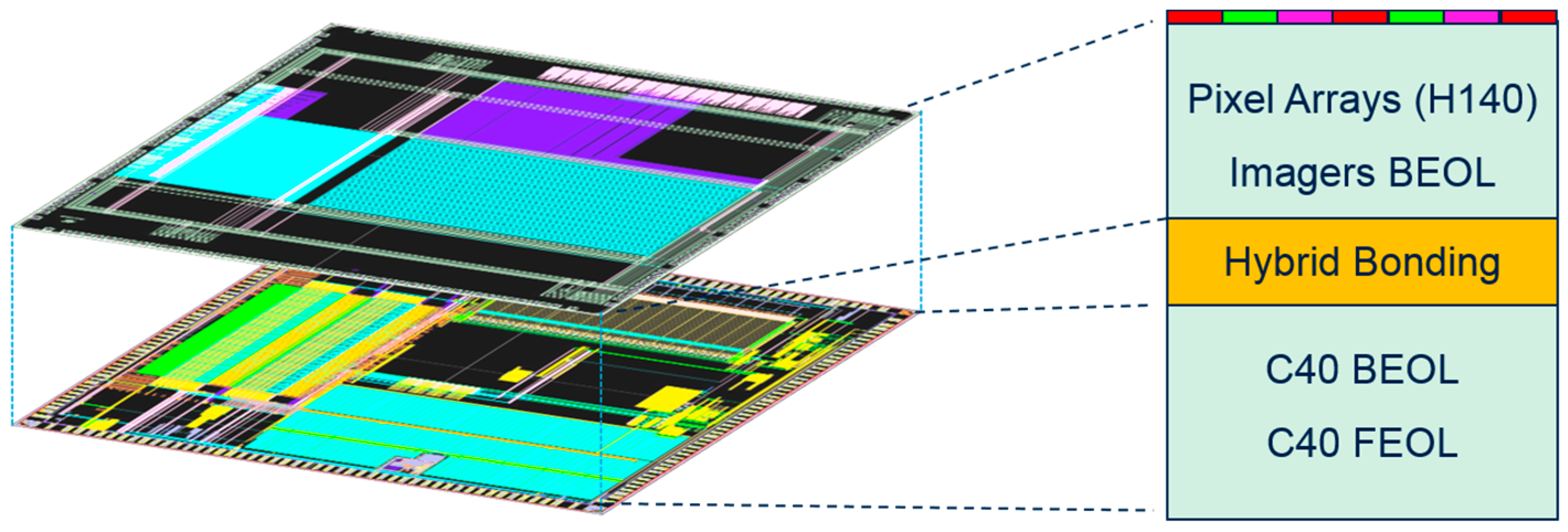
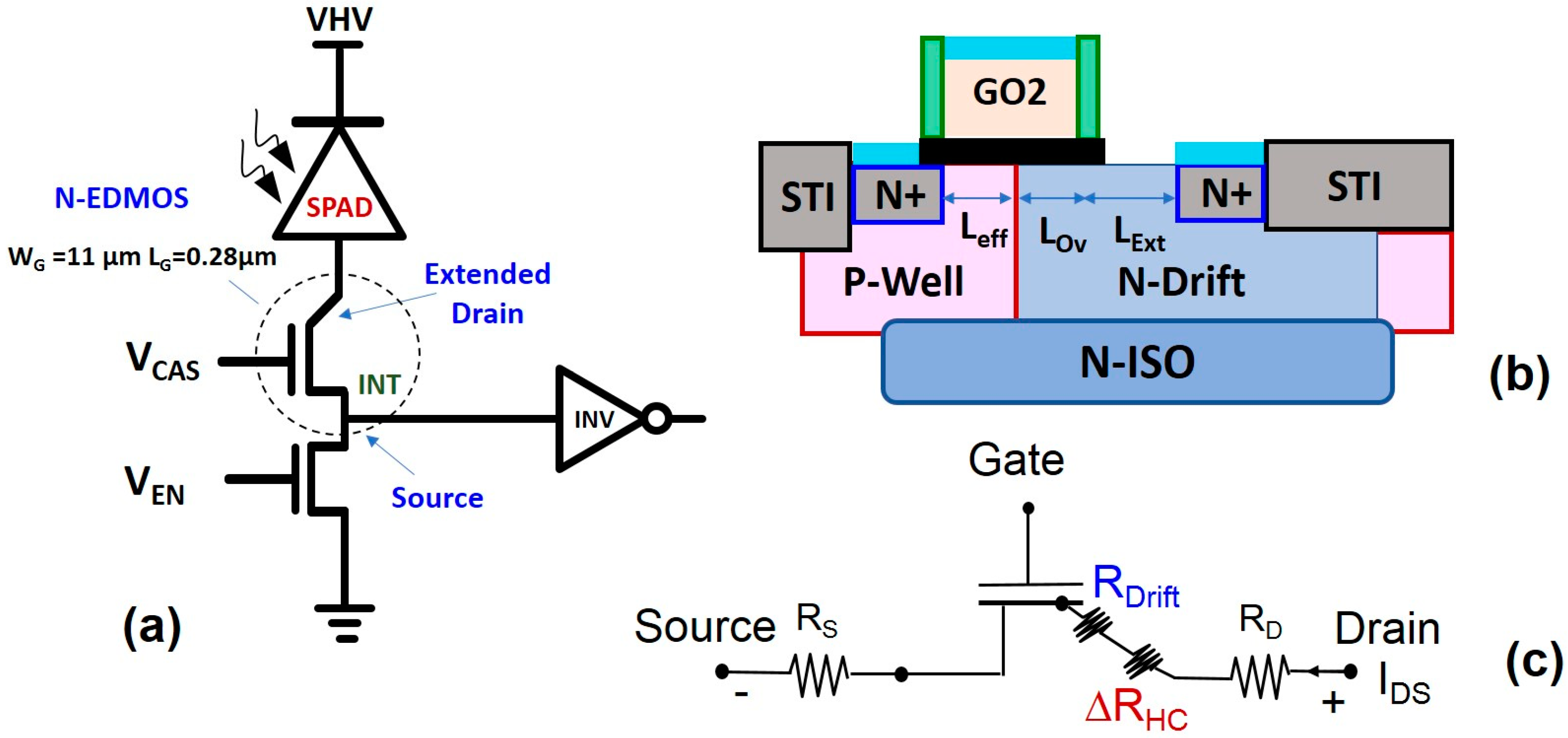
2. The SPAD Cell with EDMOS Transistor
3. Devices and Experiments
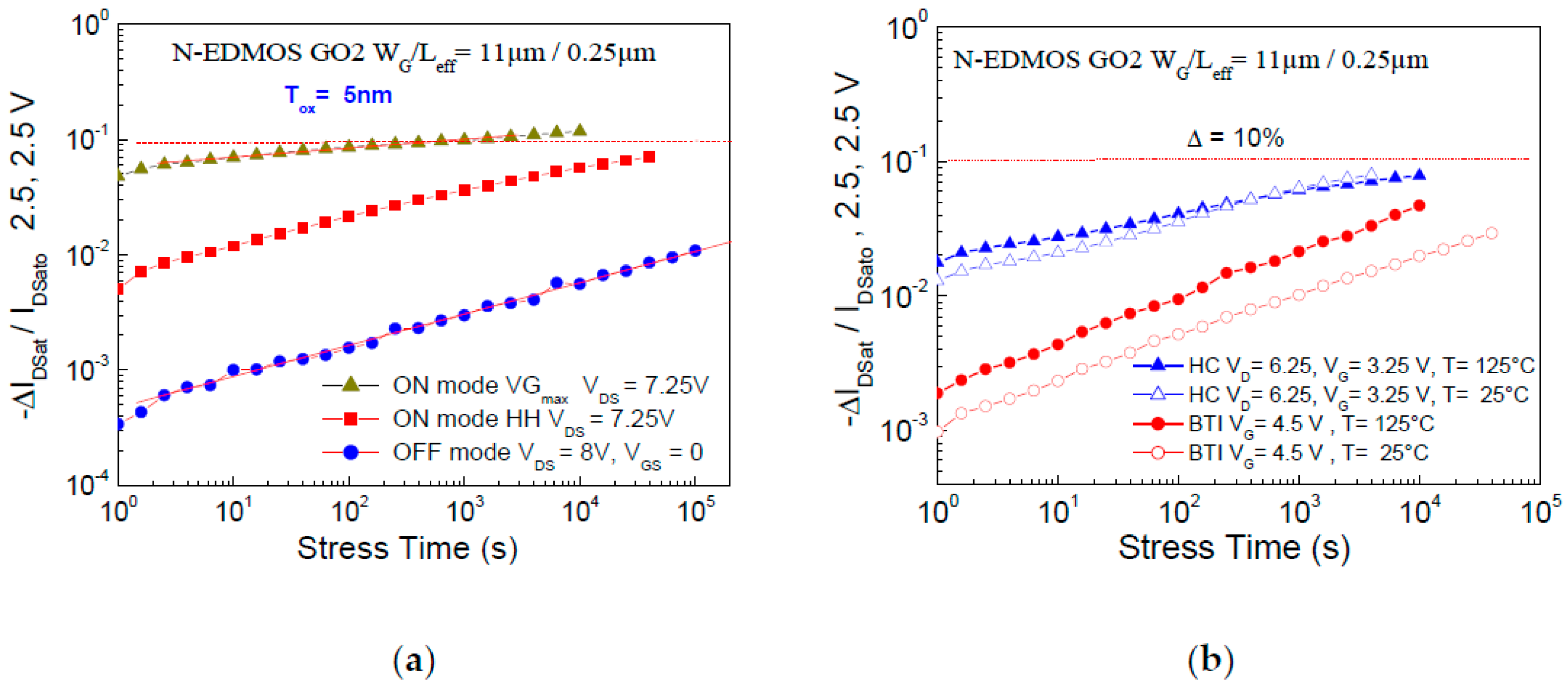
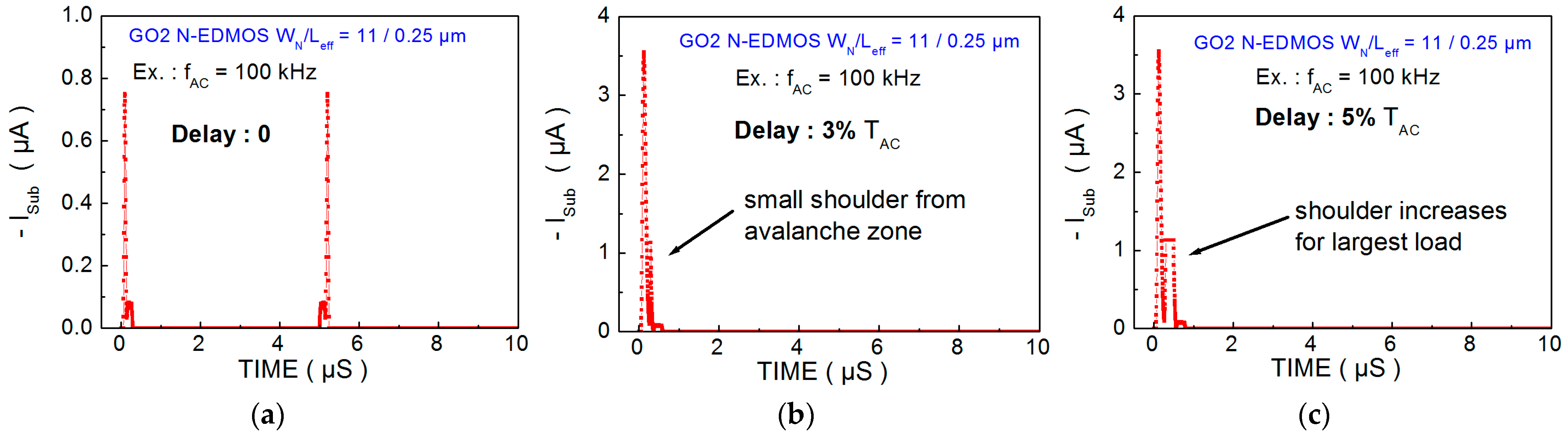
4. Worst Case DC Degradation in N-EDMOS
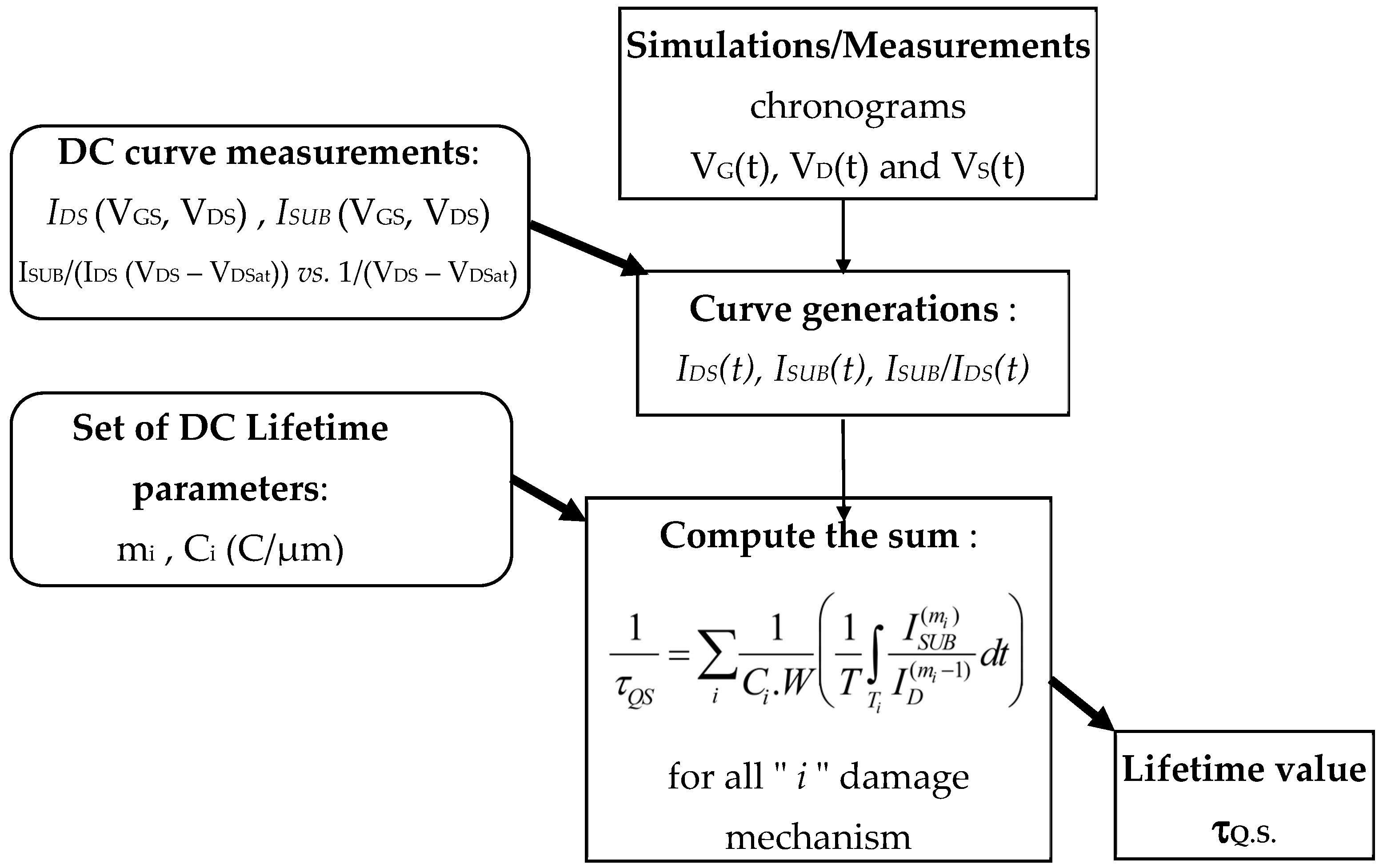
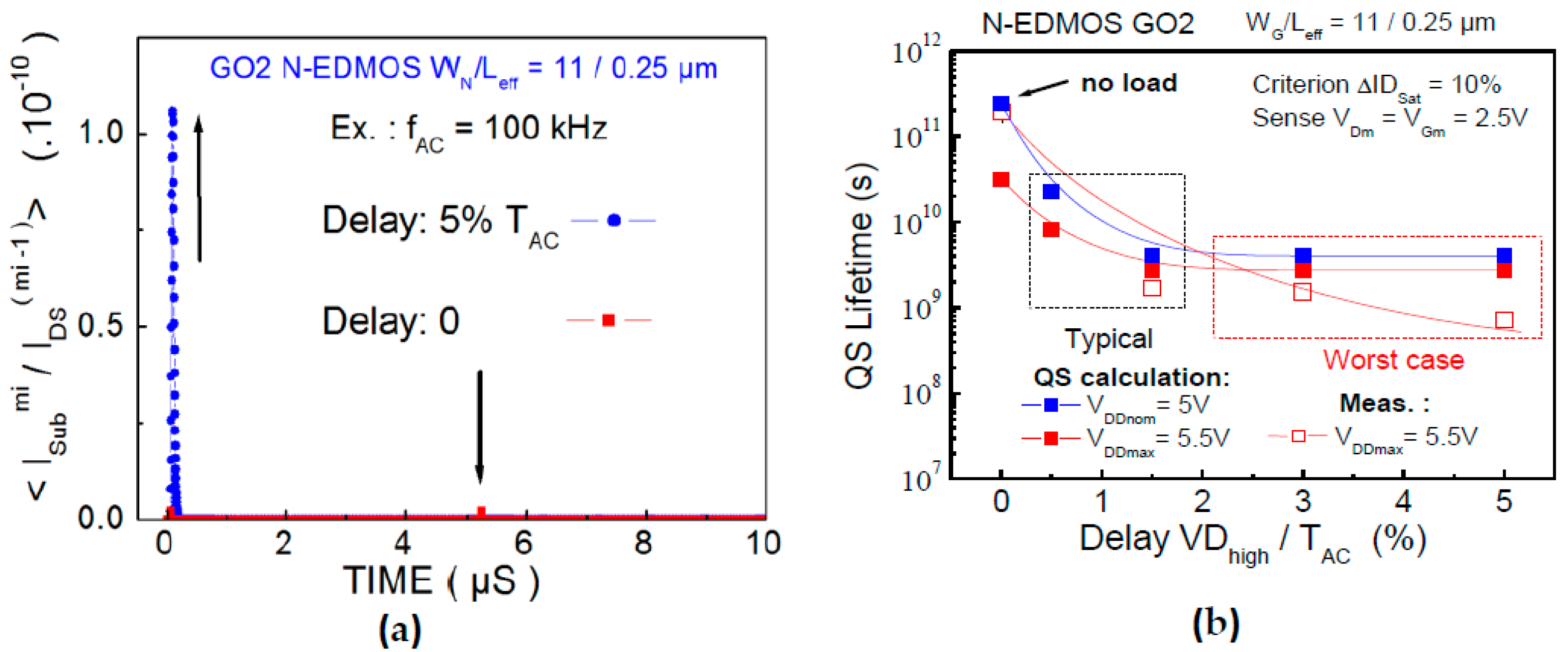
5. Quasi-Static Lifetime Extraction
- (mHH, CHH) corresponding to time duration under HH degradation where voltage satisfies VTh ≤ VGS < 0.18 VDS
- (mIB, CIB) corresponding to time duration under ISUB peak degradation where we have 0.2 VDS ≤ VGS < 0.44 VDS
- (mVgmax, CVgmax) corresponding to time duration under VGSmax degradation where we have 0.46 VDS ≤ VGS < 0.5 VDS
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| BEOL | Back-End of the Line |
| BTI | Bias Temperature Instability |
| EDMOS | Extended-Drain Metal Oxide Semiconductor |
| HBD | Hard Breakdown |
| HCD | Hot-Carrier Damage |
| HH | Hot Hole |
| HKMG | High-K Metal Gate |
| II | Impact Ionization |
| LOCOS | Locally Oxidized Silicon |
| MOSFET | Metal-Oxide-Semiconductor Field Effect Transistor |
| PBTI | Positive Bias Temperature Instability |
| QS | Quasi-Static |
| STI | Shallow Trench Isolation |
| SPAD | Single Photon Avalanche Diode |
| FDSOI | Fully Depleted Silicon on Insulator |
| TSV | Through Silicon Via |
References
- Lee, M.-J.; Charbon, E. Progress in single-photon avalanche diode image sensors in standard CMOS: From two-dimensional monolithic to three dimensional-stacked technology. Jpn. J. Appl. Phys. 2018, 57, 1002A3-1–1002A3-6. [Google Scholar] [CrossRef]
- Chaves, T.A.; Issartel, D.; Clerc, R.; Pittet, P.; Cellier, R.; Uhring, W.; Cathelin, A.; Calmon, F. Body-biasing considerations with SPAD FDSOI: Advantages and drawbacks. In Proceedings of the European Solid-State Device Research Conference Proc (ESSDERC), Krakow, Poland, 23–26 September 2019; pp. 210–213. [Google Scholar] [CrossRef]
- Ayoub, B.; Moreau, S.; Lhostis, S.; Frémont, H.; Mermoz, S.; Souchier, E.; Deloffre, E.; Escoubas, S.; Cornelius, T.; Thomas, O. In-situ characterization of thermomechanical behavior of copper nano-interconnect for 3D integration. Microelectron. Eng. 2022, 261, 111809. [Google Scholar] [CrossRef]
- Haruta, T.; Nakajima, T.; Hashizume, J.; Umebayashi, T.; Takahashi, H.; Taniguchi, K.; Kuroda, M.; Sumihiro, H.; Enoki, K.; Yamasaki, T. 4.6 a 1/2.3 inch 20mpixel 3-layer stacked CMOS image sensor with DRAM. In Proceedings of the IEEE International Solid-State Circuits Conference (ISSCC), San Francisco, CA, USA, 5–9 February 2017; pp. 76–77. [Google Scholar] [CrossRef]
- Skotnicki, T.; Fenouillet-Beranger, C.; Gallon, C.; Bœuf, F.; Monfray, S.; Payet, F.; Pouydebasque, A.; Szczap, M.; Farcy, A.; Arnaud, F.; et al. Innovative Materials, Devices, and CMOS Technologies for Low-Power Mobile Multimedia. IEEE Trans. Electron Dev. 2008, 55, 96–130. [Google Scholar] [CrossRef]
- Bravaix, A.; Guérin, C.; Huard, V.; Roy, D.; Roux, J.-M.; Vincent, E. Hot-Carrier Acceleration Factors for Low Power Management in DC-AC stressed 40 nm NMOS node at High Temperature. In Proceedings of the IEEE International Reliability Physics Symposium (IRPS), Montreal, QC, Canada, 26–30 April 2009; pp. 531–548. [Google Scholar] [CrossRef]
- Tsai, H.-C.; Yadav, Y.; Liou, R.-H.; Wu, K.-M.; Lin, Y.-C. Breakdown voltage model and electrical characteristics of CMOS compatible RESURF STI drain extended MOS transistors. IEEE Trans. Electron Devices 2015, 62, 1958–1963. [Google Scholar] [CrossRef]
- Tyaginov, S.; Bina, M.; Franco, J.; Osintsev, D.; Triebl, O.; Kaczer, B.; Grasser, T. Physical Modeling of Hot-Carrier Degradation for Short- and Long-Channel MOSFETs. In Proceedings of the International Reliability Physics Symposium (IRPS), Waikoloa, HI, USA, 1–5 June 2014; pp. XT.16.1–XT.16.8. [Google Scholar] [CrossRef]
- Grasser, T.; Stampfer, B.; Waltl, M.; Rzepa, G.; Rupp, K.; Schanovsky, F.; Pobegen, G.; Puschkarsky, K.; Reisinger, H.; O’Sullivan, B.; et al. Characterization and physical modeling of the temporal evolution of near-interfacial states resulting from NBTI/PBTI stress in nMOS/pMOS transistors. In Proceedings of the IEEE International Reliability Physics Symposium (IRPS), Burlingame, CA, USA, 11–15 March 2018; pp. 2A.2.1–2A.2.10. [Google Scholar] [CrossRef]
- Varghese, D.; Kufluoglu, H.; Reddy, V.; Shichijo, H.; Mosher, D.; Krishnan, S.; Ashraful Alam, M. Off-State Degradation in Drain-Extended NMOS Transistors: Interface Damage and Correlation to Dielectric Breakdown. IEEE Trans. Electron Devices 2007, 54, 2669–2678. [Google Scholar] [CrossRef]
- Li, S.; Bravaix, A.; Kussener, E.; Ney, D.; Federspiel, X.; Cacho, F. Hot-Carrier Degradation in P- and N-Channel EDMOS for Smart Power Application. Microelectron. Reliab. 2020, 114, 113811. [Google Scholar] [CrossRef]
- Arabi, M.; Federspiel, X.; Cacho, F.; Rafik, M.; Nguyen, A.P.; Garros, X.; Ghibaudo, G. New Insights on Device Level TDDB at GHz Speed in Advanced CMOS Nodes. IEEE Trans. Device Mater. Reliab. 2019, 19, 255–261. [Google Scholar] [CrossRef]
- Tallarico, A.N.; Reggiani, S.; Depetro, R.; Torti, A.M.; Croce, G.; Sangiorgi, E.; Fiegna, C. Hot-Carrier Degradation in Power LDMOS: Selective LOCOS- Versus STI-Based Architecture. IEEE J. Electron Devices Soc. (JEDS) 2018, 6, 219–226. [Google Scholar] [CrossRef]
- Liu, S.; Sun, W.; Qian, Q.; Wei, J.; Fang, J.; Li, T.; Zhang, C.; Shi, L. A Review on Hot-Carrier-Induced Degradation of Lateral DMOS Transistor. IEEE Trans. Device Mater. Reliab. 2018, 18, 298–312. [Google Scholar] [CrossRef]
- Okawa, T.; Eguchi, H.; Taki, M.; Hamada, K. 2000 V SOI LDMOS with new drift structure for HVICs. In Proceedings of the International Symposium on Power Semiconductor Devices and Ics (ISPSD), Prague, Czech Republic, 12–16 June 2016; pp. 435–438. [Google Scholar] [CrossRef]
- Mansoori, H.A.; Orouji, A.A.; Dideban, A. New technique to extend the vertical depletion region at SOI-LDMOSFETs. J. Comput. Electron. 2017, 16, 666–675. [Google Scholar] [CrossRef]
- Disney, D.; Lin, W.-C.; Liu, X.X.; Pandey, S.; Kim, J. 180nm HVIC technology for digital AC/DC power conversion. In Proceedings of the International Symposium on Power Semiconductor Devices and Ics (ISPSD), Sapporo, Japan, 28 May–1 June 2017; pp. 287–290. [Google Scholar]
- Tsai, J.-Y.; Hu, H.-H. Novel poly-Si SJ-LDMOS for system on-panel application. IEEE Trans. Electron Devices 2016, 63, 2482–2487. [Google Scholar] [CrossRef]
- Denais, M.; Bravaix, A.; Huard, V.; Parthasarathy, C.; Ribes, G.; Perrier, F.; Revil, N.; Rey-Tauriac, Y. “On-the-fly” Characterization of NBTI in ultra-thin gate-oxide PMOSFET’s. In Proceedings of the IEEE International Electron Devices Meeting (IEDM), San Francisco, CA, USA, 13–15 December 2004; pp. 109–112. [Google Scholar] [CrossRef]
- Kerber, A.; Cartier, E.A. Reliability Challenges for CMOS Technology Qualifications With Hafnium Oxide/Titanium Nitride Gate Stacks. IEEE Trans. Devices Mater. Reliab. 2009, 9, 147–162. [Google Scholar] [CrossRef]
- Sasse, G.T.; Claes, J.A.M.; De Vries, B. An LDMOS hot carrier model for circuit reliability simulation. In Proceedings of the IEEE International Reliability Physics Symposium (IRPS), Waikoloa, HI, USA, 1–5 June 2014; pp. 5D.5.1–5D.5.6. [Google Scholar] [CrossRef]
- Tyaginov, S.; Bury, E.; Grill, A.; Yu, Z.; Makarov, A.; De Keersgieter, A.; Vexler, M.I.; Vandemaele, M.; Wang, R.; Spessot, A.; et al. On the Contribution of Secondary Holes in Hot-Carrier Degradation—A Compact Physics Modeling Perspective. In Proceedings of the IEEE Electron Devices Technology & Manufacturing Conference (EDTM), Seoul, Republic of Korea, 7–10 March 2023; pp. 1–3. [Google Scholar] [CrossRef]
- Starkov, I.; Enichlmair, H.; Tyaginov, S.; Grasser, T. Analysis of the threshold voltage turn-around effect in high-voltage n-MOSFETs due to hot-carrier stress. In Proceedings of the IEEE International Reliability Physics Symposium (IRPS), Anaheim, CA, USA, 15–19 April 2012; pp. XT.7.1–XT.7.6. [Google Scholar] [CrossRef]
- Tyaginov, S.; Starkov, I.; Triebl, O.; Ceric, H.; Grasser, T.; Enichlmair, H.; Park, J.-M.; Jungemann, C. Secondary generated holes as a crucial component for modeling of HC degradation in high-voltage n-MOSFET. In Proceedings of the International Conference on Simulation of Semiconductor Processes and Devices (SISPAD), Osaka, Japan, 8–10 September 2011; pp. 123–126. [Google Scholar] [CrossRef]
- Sharma, P.; Tyaginov, S.; Jech, M.; Wimmer, Y.; Rudolf, F.; Enichlmair, H.; Bina, M.; Enichlmair, H.; Park, J.-M.; Ceric, H.; et al. The role of cold carriers and the multiple-carrier process of Si–H bond dissociation for hot-carrier degradation in n- and p-channel LDMOS devices. Solid-State Electron. 2016, 115, 185–191. [Google Scholar] [CrossRef]
- Jech, M.; Rott, G.; Reisinger, H.; Tyaginov, S.; Rzepa, G.; Grill, A.; Jabs, D.; Jungemann, C.; Waltl, M.; Grasser, T. Mixed Hot-Carrier/Bias Temperature Instability Degradation Regimes in Full {VG, VD} Bias Space: Implications and Peculiarities. IEEE Trans. Electron Devices 2020, 67, 3315–3322. [Google Scholar] [CrossRef]
- Federspiel, X.; Rafik, M.; Angot, D.; Cacho, F.; Roy, D. Interaction between BTI and HCI degradation in high-K devices. In Proceedings of the IEEE International Reliability Physics Symposium (IRPS), Monterey, CA, USA, 14–18 April 2013; pp. XT.9.1–XT.9.4. [Google Scholar] [CrossRef]
- Wang, J.; Li, R.; Dong, Y.; Zou, X.; Shao, L.; Shiau, W.T. Substrate current characterization and optimization of high voltage LDMOS transistors. Solid-State Electron. 2008, 52, 886–891. [Google Scholar] [CrossRef]
- Gill, G.; Singhal, A.; Pahwa, G.; Hu, C.; Agarwal, H. Compact Modeling of Impact Ionization in High-Voltage Devices. IEEE Trans. Electron Devices 2023, 70, 2389–2394. [Google Scholar] [CrossRef]
- Pitard, H.; Bravaix, A.; Kussener, E.; Federspiel, X.; Fillon, R.; Cacho, F. DC to AC Analysis of HC vs. BTI damage in N-EDMOS used in Single Photon Avalanche Diode cell. Microelectron. Reliab. 2023, 150, 115111. [Google Scholar] [CrossRef]
- Scholten, A.J.; Stephens, D.; Smit, G.D.J.; Sasse, G.T.; Bisschop, J. The Relation Between Degradation Under DC and RF Stress Conditions. IEEE Trans. Electron Devices 2011, 58, 2721–2728. [Google Scholar] [CrossRef]
- Mistry, K.R.; Doyle, B. AC versus DC Hot-Carrier Degradation in n-Channel MOSFET’s. IEEE Trans. Electron Devices 1993, 40, 96–104. [Google Scholar] [CrossRef]
- Quader, K.N.; Fang, P.; Yue, J.T.; Ko, P.K.; Hu, C. Hot-Carrier-Reliability Design Rules for Translating Device Degradation to CMOS Digital Circuit Degradation. IEEE Trans. Electron Devices 1994, 41, 681–691. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bravaix, A.; Pitard, H.; Federspiel, X.; Cacho, F. Hot-Carrier Damage in N-Channel EDMOS Used in Single Photon Avalanche Diode Cell through Quasi-Static Modeling. Micromachines 2024, 15, 205. https://doi.org/10.3390/mi15020205
Bravaix A, Pitard H, Federspiel X, Cacho F. Hot-Carrier Damage in N-Channel EDMOS Used in Single Photon Avalanche Diode Cell through Quasi-Static Modeling. Micromachines. 2024; 15(2):205. https://doi.org/10.3390/mi15020205
Chicago/Turabian StyleBravaix, Alain, Hugo Pitard, Xavier Federspiel, and Florian Cacho. 2024. "Hot-Carrier Damage in N-Channel EDMOS Used in Single Photon Avalanche Diode Cell through Quasi-Static Modeling" Micromachines 15, no. 2: 205. https://doi.org/10.3390/mi15020205
APA StyleBravaix, A., Pitard, H., Federspiel, X., & Cacho, F. (2024). Hot-Carrier Damage in N-Channel EDMOS Used in Single Photon Avalanche Diode Cell through Quasi-Static Modeling. Micromachines, 15(2), 205. https://doi.org/10.3390/mi15020205





