Analytical Investigation of Replica-Molding-Enabled Nanopatterned Tribocharging Process on Soft-Material Surfaces
Abstract
:1. Introduction
2. Materials and Methods
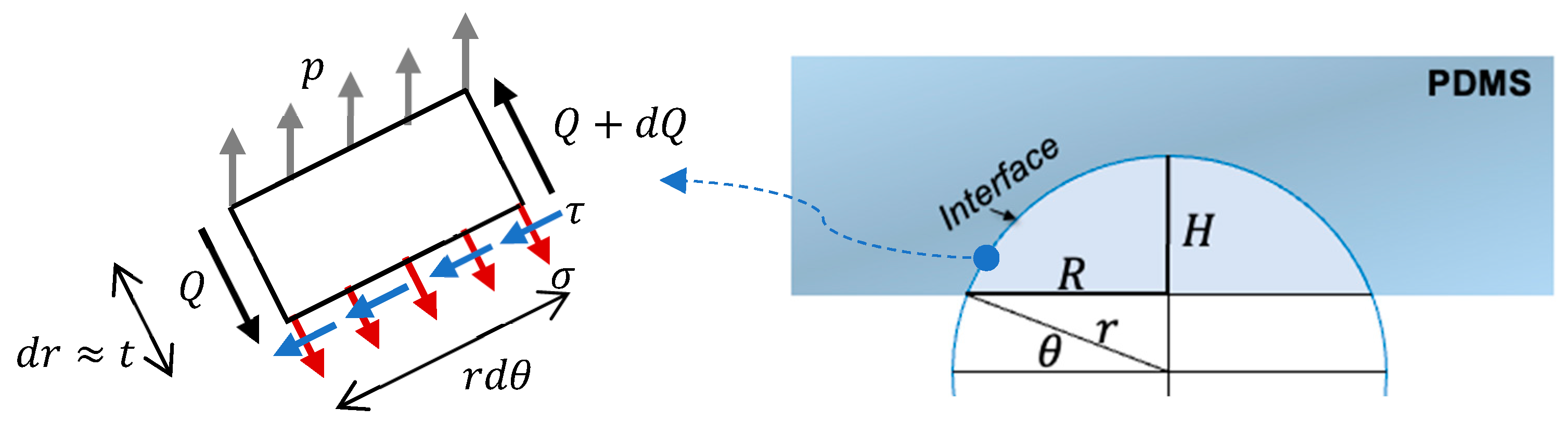
2.1. Analysis Target Structures
2.2. Ring-Shaped Tribocharge Explained by the Cohesive Zone Failure Mechanism
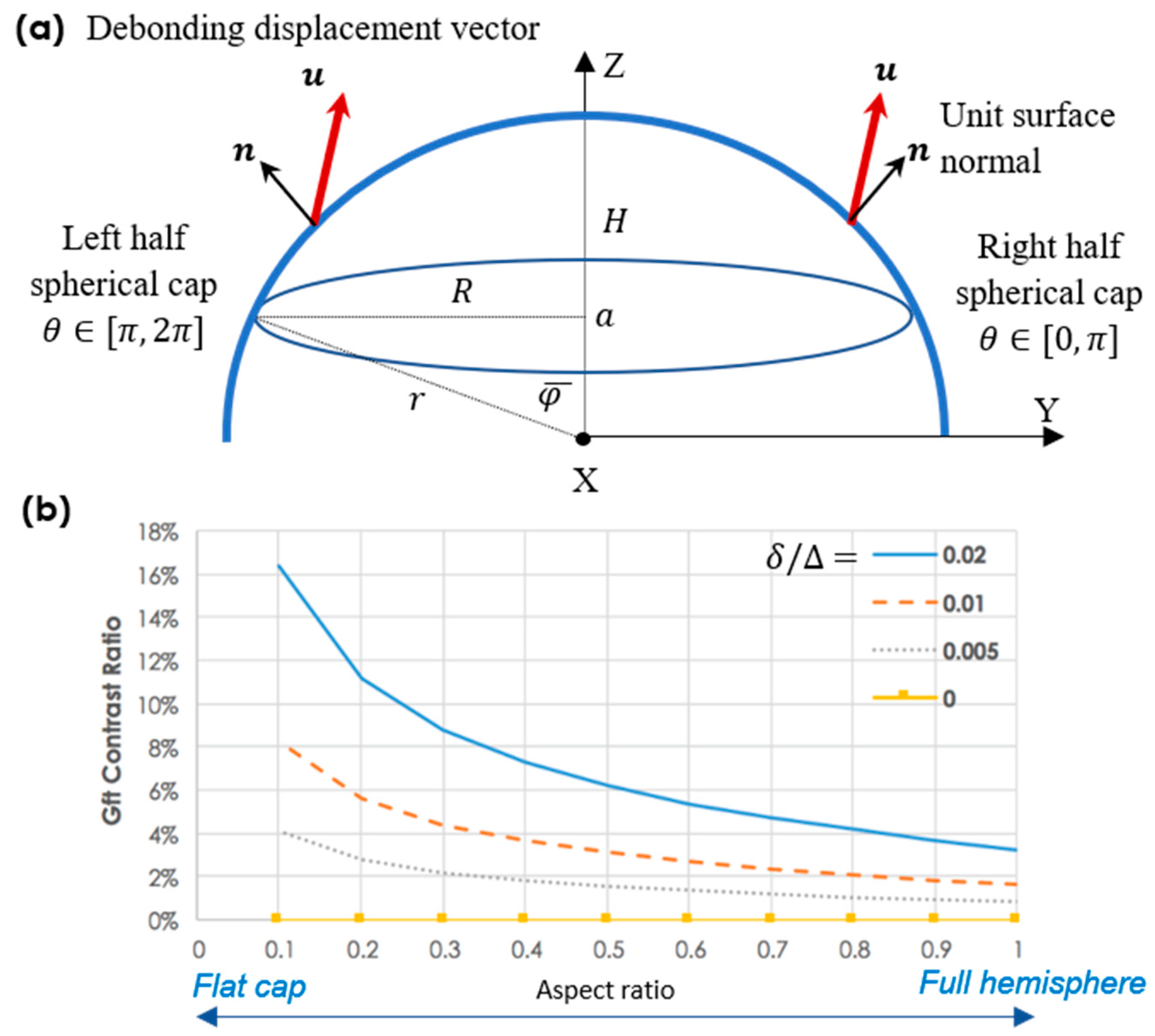
2.3. Eclipse-Shaped Tribocharge Explained by Cumulative Fracture Energy
3. Discussion
3.1. Remarks on Tribocharging Patterns
- When the half nanocup is demolded in one direction and the other half in the opposite direction, the debonding displacement vector will be and for right- and left-half caps, respectively. This leads to , meaning that only a symmetric ring charge will be generated;
- A perfectly vertical debonding without any inclination at all (i.e., ) also leads to as well as a ring charge for all aspect ratios (see the case = 0 in Figure 5b);
- When the debonding direction is controlled to have a large inclination angle, i.e., , rapidly increases, further distorting the symmetric tribocharging pattern.
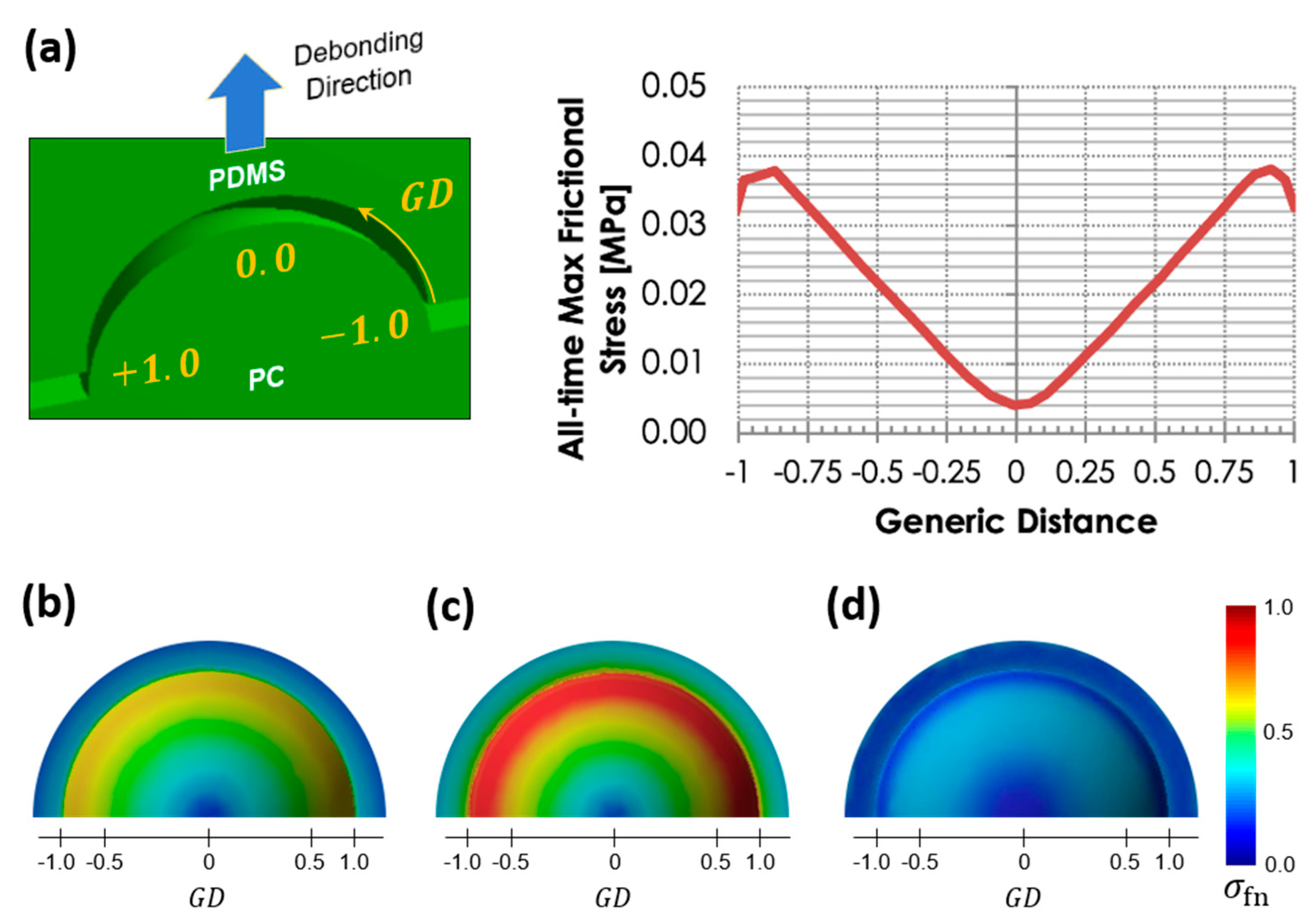
3.2. Computational Confirmation of the Ring-Shaped Tribocharging
3.3. Remarks on Limitations
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Jacobs, H.O.; Campbell, S.A.; Steward, M.G. Approaching nanoxerography: The use of electrostatic forces to position nanoparticles with 100 nm scale resolution. Adv. Mater. 2002, 14, 1553–1557. [Google Scholar] [CrossRef]
- Wang, Z.L.; Chen, J.; Lin, L. Progress in triboelectric nanogenerators as a new energy technology and self-powered sensors. Energy Environ. Sci. 2015, 8, 2250–2282. [Google Scholar] [CrossRef]
- Li, Q.; Peer, A.; Cho, I.; Biswas, R.; Kim, J. Observation of nanopatterned triboelectric charges on elastomer surfaces induced by replica molding. Nat. Commun. 2018, 9, 974. [Google Scholar] [CrossRef] [PubMed]
- Li, Q.; Cho, I.; Biswas, R.; Kim, J. Nanoscale modulation of friction and triboelectrification via surface nanotexturing. Nano Lett. 2019, 19, 850–856. [Google Scholar] [CrossRef] [PubMed]
- Ji, M.G.; Li, Q.; Biswas, R.; Kim, J. Stability and temporal decay of nanopatterned tribocharge on nanotextured elastomer surfaces. Nano Energy 2021, 79, 105441. [Google Scholar] [CrossRef]
- Lacks, D.J.; Shinbrot, T. Long-standing and unresolved issues in triboelectric charging. Nat. Rev. Chem. 2019, 3, 465–476. [Google Scholar] [CrossRef]
- Zou, H.; Zhang, Y.; Guo, L.; Wang, P.; He, X.; Dai, G.; Zheng, H.; Chen, C.; Wang, A.C.; Xu, C.; et al. Quantifying the triboelectric series. Nat. Commun. 2019, 10, 1427. [Google Scholar] [CrossRef] [PubMed]
- Fu, R.; Shen, X.; Lacks, D.J. First-principles study of the charge distributions in water confined between dissimilar surfaces and implications in regard to contact electrification. J. Phys. Chem. C 2017, 121, 12345–12349. [Google Scholar] [CrossRef]
- Pandey, R.K.; Kakehashi, H.; Nakanishi, H.; Soh, S. Correlating material transfer and charge transfer in contact electrification. J. Phys. Chem. C 2018, 122, 16154–16160. [Google Scholar] [CrossRef]
- Dudem, B.; Huynh, N.D.; Kim, W.; Kim, D.H.; Hwang, H.J.; Choi, D.; Yu, J.S. Nanopillar-Array Architectured PDMS-Based Triboelectric Nanogenerator Integrated with a Windmill Model for Effective Wind Energy Harvesting. Nano Energy 2017, 42, 269–281. [Google Scholar] [CrossRef]
- Cole, J.J.; Barry, C.R.; Wang, X.; Jacobs, H.O. Nanocontact Electrification through Forced Delamination of Dielectric Interfaces. ACS Nano 2010, 4, 7492–7498. [Google Scholar] [CrossRef] [PubMed]
- Cole, J.J.; Barry, C.R.; Knuesel, R.J.; Wang, X.; Jacobs, H.O. Nanocontact Electrification: Patterned Surface Charges Affecting Adhesion, Transfer, and Printing. Langmuir 2011, 27, 7321–7329. [Google Scholar] [CrossRef] [PubMed]
- Zhou, Y.S.; Liu, Y.; Zhu, G.; Lin, Z.; Pan, C.; Jing, Q.; Wang, Z.L. In Situ Quantitative Study of Nanoscale Triboelectrification and Patterning. Nano Lett. 2013, 13, 2771–2776. [Google Scholar] [CrossRef] [PubMed]
- Collins, A.L.; Camara, C.G.; Van Cleve, E.; Putterman, S.J. Simultaneous Measurement of Triboelectrification and Triboluminescence of Crystalline Materials. Rev. Sci. Instrum. 2018, 89, 013901. [Google Scholar] [CrossRef] [PubMed]
- Bhatia, D.; Kim, W.; Lee, S.; Kim, S.W.; Choi, D. Tandem Triboelectric Nanogenerators for Optimally Scavenging Mechanical Energy with Broadband Vibration Frequencies. Nano Energy 2017, 33, 515–521. [Google Scholar] [CrossRef]
- Xu, C.; Zi, Y.; Wang, A.C.; Zou, H.; Dai, Y.; He, X.; Wang, P.; Wang, Y.C.; Feng, P.; Li, D.; et al. On the Electron-Transfer Mechanism in the Contact-Electrification Effect. Adv. Mater. 2018, 30, 1706790. [Google Scholar] [CrossRef]
- Xu, C.; Wang, A.C.; Zou, H.; Zhang, B.; Zhang, C.; Zi, Y.; Pan, L.; Wang, P.; Feng, P.; Lin, Z.; et al. Raising the Working Temperature of a Triboelectric Nanogenerator by Quenching Down Electron Thermionic Emission in Contact-Electrification. Adv. Mater. 2018, 30, 1803968. [Google Scholar] [CrossRef]
- Ji, M.G.; Bazroun, M.; Cho, I.; Slafer, W.D.; Biswas, R.; Kim, J. Mechano-triboelectric Analysis of Surface Charge Generation on Replica-molded Elastomeric Nanocones. Micromachines 2021, 12, 1460. [Google Scholar] [CrossRef]
- Cho, I.; Li, Q.; Biswas, R.; Kim, J. A Framework for Glass-Box Physics Rule Learner and Its Application to Nanoscale Phenomena. Nat. Commun. Phys. 2020, 3, 78. [Google Scholar] [CrossRef]
- Alfano, G.; Crisfield, M.A. Finite Element Interface Models for the Delamination Anaylsis of Laminated Composites: Mechanical and Computational Issues. Int. J. Numer. Methods Eng. 2001, 50, 1701–1736. [Google Scholar] [CrossRef]
- Schneider, F.; Fellner, T.; Wilde, J.; Wallrabe, U. Mechanical properties of silicones for MEMS. J. Micromech. Microeng. 2008, 18, 065008. [Google Scholar] [CrossRef]
- Johnston, I.D.; McCluskey, D.K.; Tan, C.K.L.; Tracey, M.C. Mechanical characterization of bulk Sylgard 184 for microfluidics and microengineering. J. Micromech. Microeng. 2014, 24, 035017. [Google Scholar] [CrossRef]
- Hoefnagels, J.P.M.; Neggers, J.; Timmermans, P.H.M.; van der Sluis, O.; Geers, M.G.D. Copper-rubber interface delamination in stretchable electronics. Scr. Mater. 2010, 63, 875–878. [Google Scholar] [CrossRef]
- Nunes, L.C.S. Mechanical characterization of hyperelastic polydimethylsiloxane by simple shear test. Mater. Sci. Eng. A 2011, 528, 1799–1804. [Google Scholar] [CrossRef]
- ANSYS®. Academic Research Mechanical; Release 18.2; ANSYS Inc.: Canonsburg, PA, USA, 2017. [Google Scholar]
- Wang, Z.L.; Wang, A.C. On the origin of contact-electrification. Mater. Today 2019, 30, 34–51. [Google Scholar] [CrossRef]
- Aymard, A.; Delplanque, E.; Dalmas, D.; Scheibert, J. Designing metainterfaces with specified friction laws. Science 2024, 383, 200–204. [Google Scholar] [CrossRef]
- Mariello, M.; Fachechi, L.; Guido, F.; De Vittorio, M. Conformal, Ultra-thin Skin-Contact-Actuated Hybrid Piezo/Triboelectric Wearable Sensor Based on AIN and Parylene-Encapsulated Elastomeric Blend. Adv. Funct. Mater. 2021, 31, 2101047. [Google Scholar] [CrossRef]
- Kuang, S.Y.; Zhu, G.; Wang, Z.L. Triboelectrification-Enabled Self-Powered Data Storage. Adv. Sci. 2018, 5, 1700658. [Google Scholar] [CrossRef]
- Vallem, V.; Sargolzaeiaval, Y.; Ozturk, M.; Lai, Y.C.; Dickey, M.D. Energy Harvesting and Storage with Soft and Stretchable Materials. Adv. Mater. 2021, 33, 2004832. [Google Scholar] [CrossRef]
- Palleau, E.; Ressier, L. Combinatorial Particle Patterning by Nanoxerography. Adv. Funct. Mater. 2018, 28, 4–7. [Google Scholar] [CrossRef]



| Nanoscale Tribocharging Patterns | Figures | Reference |
|---|---|---|
| Ring shape | Figure 2 | [3] |
| Ring shape | Figure 2g | [2] |
| Eclipse shape | Figure 4c | [19] |
| Eclipse shape | Figure 2i | [2] |
| Asymmetric on parallel nanoridges (special case of eclipse shape) | Figure 5a | [19] |
| Asymmetric on parallel nanoridges (special case of eclipse shape) | Figure S2 | [2] |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cho, I.H.; Ji, M.G.; Kim, J. Analytical Investigation of Replica-Molding-Enabled Nanopatterned Tribocharging Process on Soft-Material Surfaces. Micromachines 2024, 15, 417. https://doi.org/10.3390/mi15030417
Cho IH, Ji MG, Kim J. Analytical Investigation of Replica-Molding-Enabled Nanopatterned Tribocharging Process on Soft-Material Surfaces. Micromachines. 2024; 15(3):417. https://doi.org/10.3390/mi15030417
Chicago/Turabian StyleCho, In Ho, Myung Gi Ji, and Jaeyoun Kim. 2024. "Analytical Investigation of Replica-Molding-Enabled Nanopatterned Tribocharging Process on Soft-Material Surfaces" Micromachines 15, no. 3: 417. https://doi.org/10.3390/mi15030417
APA StyleCho, I. H., Ji, M. G., & Kim, J. (2024). Analytical Investigation of Replica-Molding-Enabled Nanopatterned Tribocharging Process on Soft-Material Surfaces. Micromachines, 15(3), 417. https://doi.org/10.3390/mi15030417







