HfS2 and TiS2 Monolayers with Adsorbed C, N, P Atoms: A First Principles Study
Abstract
1. Introduction
2. Methodology
3. Results and Discussion
3.1. HfS and TiS Materials
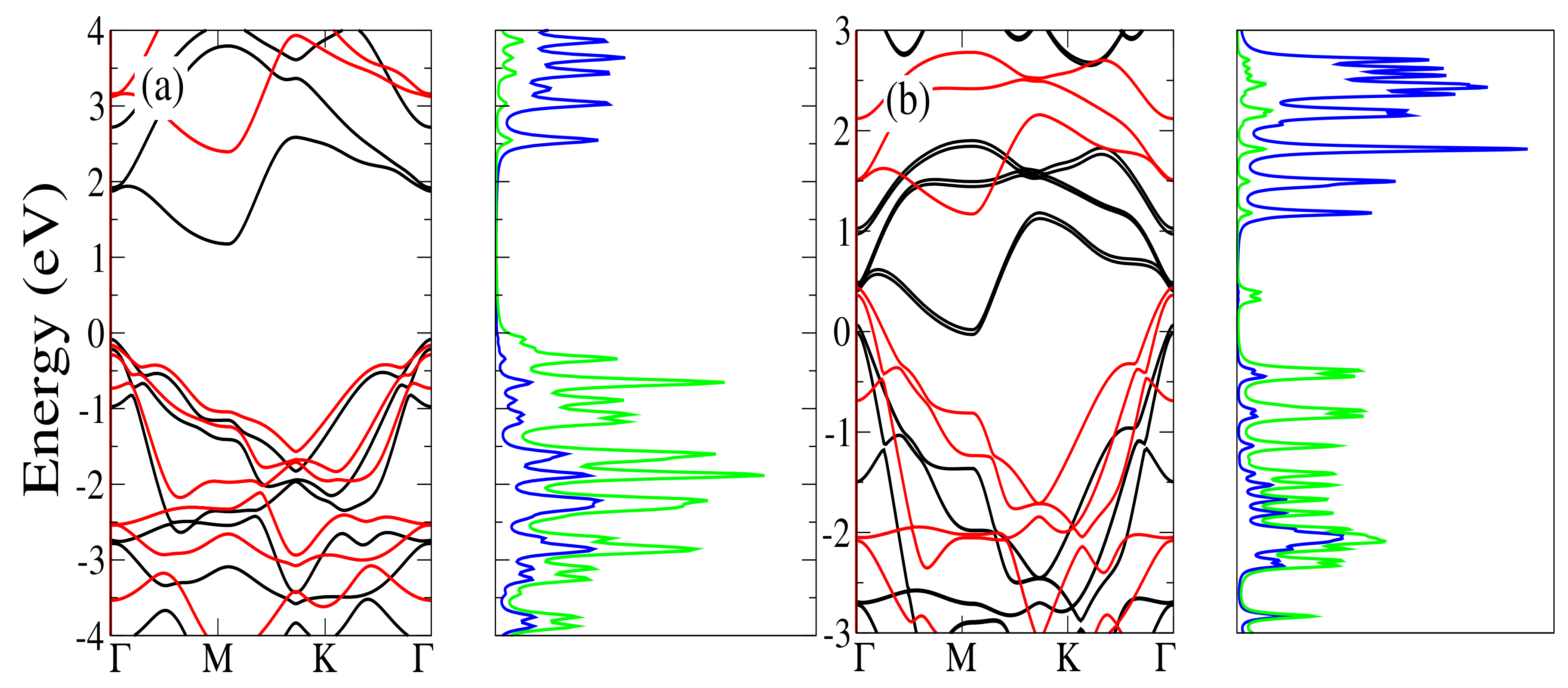
3.2. 1T HfS and TiS Monolayers
3.2.1. 1T HfS Monolayer
3.2.2. 1T TiS Monolayer
3.3. HfS and TiS Monolayers with Adsorbed Atoms
3.3.1. Energetic and Structural Properties
3.3.2. Electronic Properties
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Novoselov, K.S.; Geim, A.K.; Morosov, S.V.; Jiang, D.; Zhang, Y.; Dubonos, S.V.; Grigorieva, I.V.; Firsov, A.A. Electric Field Effect in Atomically Thin Carbon Films. Science 2004, 306, 666–669. [Google Scholar] [CrossRef] [PubMed]
- Jirawala, D.; Sangwan, V.K.; Lauhon, L.J.; Marks, T.J.; Hersam, M.C. Emerging Device Applications for Semiconducting Two-Dimensional Transition Metal Dichalcogenides. ACS Nano 2014, 8, 1102–1120. [Google Scholar] [CrossRef] [PubMed]
- Radisavljevic, B.; Radenovic, A.; Brivio, J.; Giacometti, V.; Kis, A. Single-layer MoS2 transistors. Nat. Nanotechnol. 2011, 6, 147–149. [Google Scholar] [CrossRef] [PubMed]
- Wang, Q.H.; Kalantar-Zadeh, K.; Kis, A.; Coleman, J.N.; Strano, M.S. Characteristics of n- and p-type dopants in 1T-HfS2 monolayer. Nat. Nanotechnol. 2012, 7, 699–712. [Google Scholar] [CrossRef] [PubMed]
- Mak, K.F.; Shan, J. Photonics and optoelectronics of 2D semiconductor transition metal dichalcogenides. Nat. Photonics 2016, 10, 216–226. [Google Scholar] [CrossRef]
- Mak, K.F.; Lee, C.; Hone, J.; Sham, J.; Heinz, T.F. Atomically Thin MoS2: A New Direct-Gap Semiconductor. Phys. Rev. Lett. 2010, 105, 136805. [Google Scholar] [CrossRef] [PubMed]
- Lv, R.; Robinson, J.A.; Schaak, R.E.; Sun, D.; Sun, Y.; Mallouk, T.E.; Terrones, M. Transition Metal Dichalcogenides and Beyond: Synthesis, Properties, and Applications of Single- and Few-Layer Nanosheets. Acc. Chem. Res. 2015, 48, 56–64. [Google Scholar] [CrossRef]
- Chhowalla, M.; Shin, H.S.; Eda, G.; Li, L.J.; Loh, K.P.; Zhang, H. The chemistry of two-dimensional layered transition metal dichalcogenide nano sheets. Nat. Chem. 2013, 5, 263. [Google Scholar] [CrossRef]
- Liu, H.; Neal, A.T.; Ye, P.D. Channel Length Scaling of MoS2 MOSFETs. ACS Nano 2012, 6, 8563–8569. [Google Scholar] [CrossRef]
- Briggs, N.; Subramanian, S.; Lin, Z.; Li, X.; Zhang, X.; Zhang, K.; Xiao, K.; Geohegan, D.; Wallace, R.; Chen, L.Q.; et al. A roadmap for electronic grade 2D materials. 2D Mater. 2019, 6, 022001. [Google Scholar] [CrossRef]
- Zhao, X.; Xia, C.; Wang, T.; Dai, X.; Yang, L. Electronics and optoelectronics of two-dimensional transition metal dichalcogenides. J. Alloys Compd. 2016, 689, 302–306. [Google Scholar] [CrossRef]
- Singh, D.; Gupta, S.K.; Sonvane, Y.; Kumar, A.; Ahuja, R. 2D-HfS2 as an efficient photocatalyst for water splitting. Catal. Sci. Technol. 2016, 6, 6605. [Google Scholar] [CrossRef]
- Xu, K.; Wang, Z.; Wang, F.; Huang, Y.; Wang, F.; Yin, L.; Jiang, C.; He, J. Ultrasensitive Phototransistors Based on Few-Layered HfS2. Adv. Mater. 2015, 27, 7881–7887. [Google Scholar] [CrossRef] [PubMed]
- Li, G.; Yao, K.; Gao, G. Strain-induced enhancement of thermoelectric performance of TiS2 monolayer based on first-principles phonon and electron band structures. Nanotechnology 2018, 29, 015204. [Google Scholar] [CrossRef]
- Das, T.; Chakraborty, S.; Ahuja, R.; Das, G.P. TiS2 Monolayer as an Emerging Ultrathin Bifunctional Catalyst: Influence of Defects and Functionalization. Chem. Phys. Chem. 2019, 20, 608–617. [Google Scholar] [CrossRef]
- Samad, A.; Shafique, A.; Shin, Y.H. Stability, spontaneous and induced polarization in monolayer MoC, WC, WS, and WSes. Nanotechnology 2017, 28, 175401. [Google Scholar] [CrossRef]
- Gao, J.; Li, B.; Tai, J.; Chow, P.; Lu, T.M.; Koratkar, N. Aging of Transition Metal Dichalcogenide Monolayers. ACS Nano 2016, 10, 2628–2635. [Google Scholar] [CrossRef]
- Perdew, J.P.; Burke, K.; Ernzerhof, M. Generalized Gradient Approximation Made Simple. Phys. Rev. Lett. 1997, 77, 3865. [Google Scholar] [CrossRef]
- Blöchl, P.E. Projector Augmented-Wave Method. Phys. Rev. B 1994, 50, 17953. [Google Scholar] [CrossRef]
- Monkhorst, H.J.; Pack, J.D. Special points for Brillouin zone integration. Phys. Rev. B 1976, 13, 5188–5192. [Google Scholar] [CrossRef]
- Grimme, S. Accurate description of van der Waals complexes by density functional theory including empirical corrections. J. Comput. Chem. 2004, 25, 1463. [Google Scholar] [CrossRef] [PubMed]
- Ferreira, L.G.; Marques, M.; Teles, L.K. Approximation to density functional theory for the calculation of band gap of semiconductors. Phys. Rev. B 2008, 78, 125116. [Google Scholar] [CrossRef]
- Kresse, G.; Furthmüller, J. Efficiency of ab-initio total energy calculations for metals and semiconductors using plane-wave basis set. Comput. Mater. Sci. 1996, 6, 15. [Google Scholar] [CrossRef]
- Kresse, G.; Furthmüller, J. Efficient iterative schemes for ab initio total-energy calculations using a plane-wave basis set. Phys. Rev. B 1996, 54, 11169. [Google Scholar] [CrossRef]
- Zhao, Q.; Guo, Y.; Si, K.; Ref, Z.; Bai, J.; Xu, X. Elastic, electronic, and dielectric properties of bulk and monolayer ZrS2, ZrSe2, HfS2, HfSe2 from van der Waals density-functional theory. Phys. Status Solidi B 2017, 254, 1700033. [Google Scholar] [CrossRef]
- Hodul, D.T.; Stacy, A.M. Anomalies in the Properties Hf(S2-xTex)1-y and Hf(Se2-xTex)1-y near the Metal-Insulator Transition. J. Solid State Chem. 1984, 54, 438. [Google Scholar] [CrossRef]
- Fang, C.M.; de Groot, R.A.; Haas, C. Bulk and surface electronic structure of 1T-TiS2 and 1T-TiSe2. Phys. Rev. B 1997, 56, 4455. [Google Scholar] [CrossRef]
- Greenaway, D.L.; Nitsche, R. Preparation and optical properties of group IV-VI2 chalcogenides having the CdI2 structure. J. Phys. Chem. Solids 1965, 26, 1445–1458. [Google Scholar] [CrossRef]
- Ugeda, M.G.; Bradley, A.J.; Shi, S.F.; da Jornada, F.H.; Zhang, Y.; Qiu, D.Y.; Ruan, W.; Mo, S.K.; Hussian, Z.; Shen, Z.X.; et al. Giant bandgap renormalization and excitonic effects in a monolayer transition metal dichalcogenide semiconductor. Nat. Mater. 2014, 13, 1091–1095. [Google Scholar] [CrossRef]
- Allan, D.R.; Kelsey, A.A.; Clark, S.J.; Angel, R.J.; Ackland, G.J. High-pressure semiconductor-semimetal transition in TiS2. Phys. Rev. B 1998, 57, 5106. [Google Scholar] [CrossRef]
- Sharma, S.; Nautiyal, T.; Singh, G.S.; Auluck, S.; Blaha, P.; Ambrosch-Draxl, C. Electronic structure of 1T-TiS2. Phys. Rev. B 1999, 59, 14833. [Google Scholar] [CrossRef]
- Zhuang, H.L.; Hennig, R.G. Computational Search for Single-Layer Transition-Metal Dichalcogenide Photocatalysts. J. Phys. Chem. C 2013, 117, 20440–20445. [Google Scholar] [CrossRef]
- Rasmussen, F.A.; Thygesen, K.S. Computational 2D Materials Database: Electronic Structure of Transition-Metal Dichalcogenides and Oxides. J. Phys. Chem. C 2015, 119, 13169–13183. [Google Scholar] [CrossRef]
- Wu, Z.Y.; Lemoigno, F.; Gressier, P.; Ouvrard, G.; Moreau, P.; Rouxel, J.; Natoli, C.R. Experimental and theoretical studies of the electronic structure of TiS2. Phys. Rev. B 1996, 54, R11009. [Google Scholar] [CrossRef] [PubMed]
- Bullett, D.W. Electronic band structure and bonding in transition metal layered dichalcogenides by atomic orbital methods. J. Phys. C Solid State 1978, 11, 4501–4514. [Google Scholar] [CrossRef]
- Suga, S.; Tusche, C.; Matsushita, Y.; Ellguth, M.; Irizawa, A.; Kirschner, J. Momentum microscopy of the layered semiconductor TiS2 and Ni intercalated Ni1/3TiS2. New J. Phys. 2015, 17, 083010. [Google Scholar] [CrossRef]
- Humphrey, W.; Dalke, A.; Schulten, K. VMD—Visual Molecular Dynamics. J. Mol. Graph. 1996, 14, 33–38. [Google Scholar] [CrossRef]



| Adsorbed Species | E | |||
|---|---|---|---|---|
| HfS | TiS | HfS | TiS | |
| 8.57 | 8.26 | |||
| C | 2.82 | 3.09 | 6.89 | 7.53 |
| N | 2.08 | 1.84 | 7.35 | 7.61 |
| P | 1.25 | 1.49 | 6.80 | 7.22 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Berwanger, M.; Ahuja, R.; Piquini, P.C. HfS2 and TiS2 Monolayers with Adsorbed C, N, P Atoms: A First Principles Study. Catalysts 2020, 10, 94. https://doi.org/10.3390/catal10010094
Berwanger M, Ahuja R, Piquini PC. HfS2 and TiS2 Monolayers with Adsorbed C, N, P Atoms: A First Principles Study. Catalysts. 2020; 10(1):94. https://doi.org/10.3390/catal10010094
Chicago/Turabian StyleBerwanger, Mailing, Rajeev Ahuja, and Paulo Cesar Piquini. 2020. "HfS2 and TiS2 Monolayers with Adsorbed C, N, P Atoms: A First Principles Study" Catalysts 10, no. 1: 94. https://doi.org/10.3390/catal10010094
APA StyleBerwanger, M., Ahuja, R., & Piquini, P. C. (2020). HfS2 and TiS2 Monolayers with Adsorbed C, N, P Atoms: A First Principles Study. Catalysts, 10(1), 94. https://doi.org/10.3390/catal10010094






