Numerical Assessment of Flow Pulsation Effects on Reactant Conversion in Automotive Monolithic Reactors
Abstract
:1. Introduction
1.1. Background
1.2. Theory
2. Materials and Methods
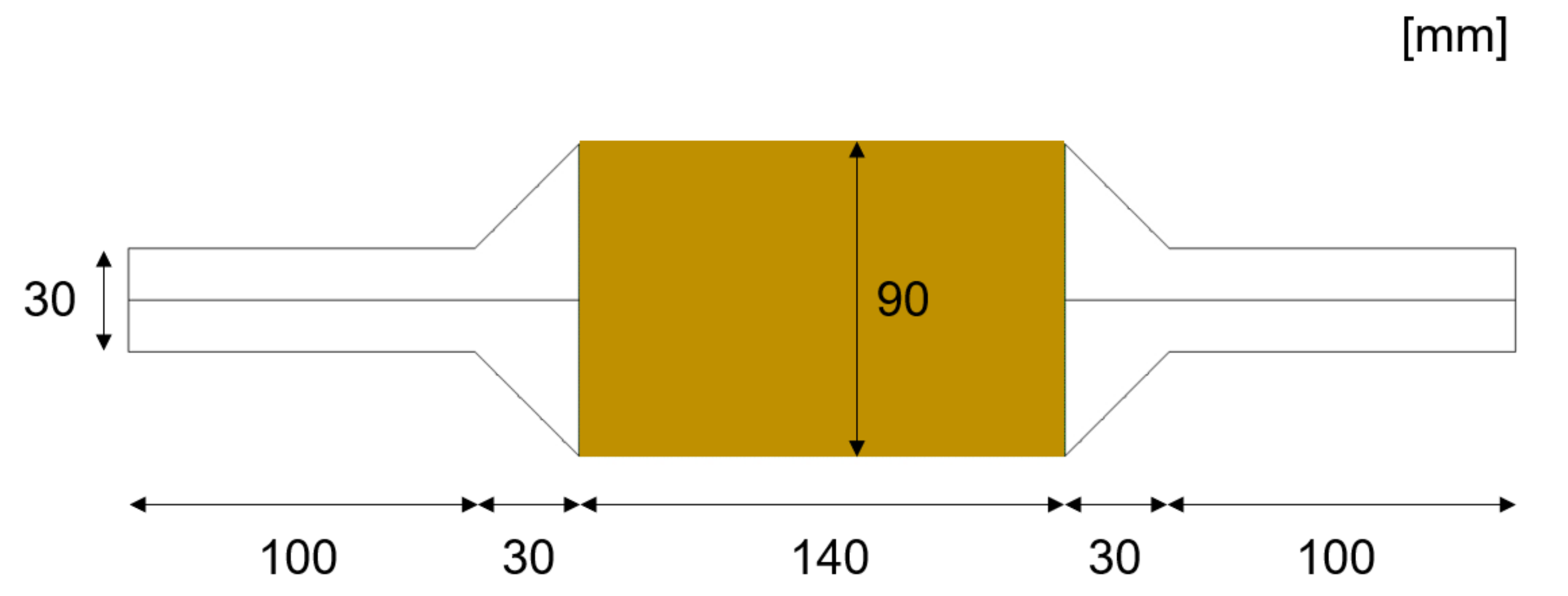
2.1. Geometry and Mesh
2.2. Mathematical Model
2.2.1. Governing Equations
2.2.2. Governing Equations
2.2.3. Monolith
2.2.4. Reaction
2.2.5. Material Properties
2.3. Boundary Conditions
2.4. Numerical Details
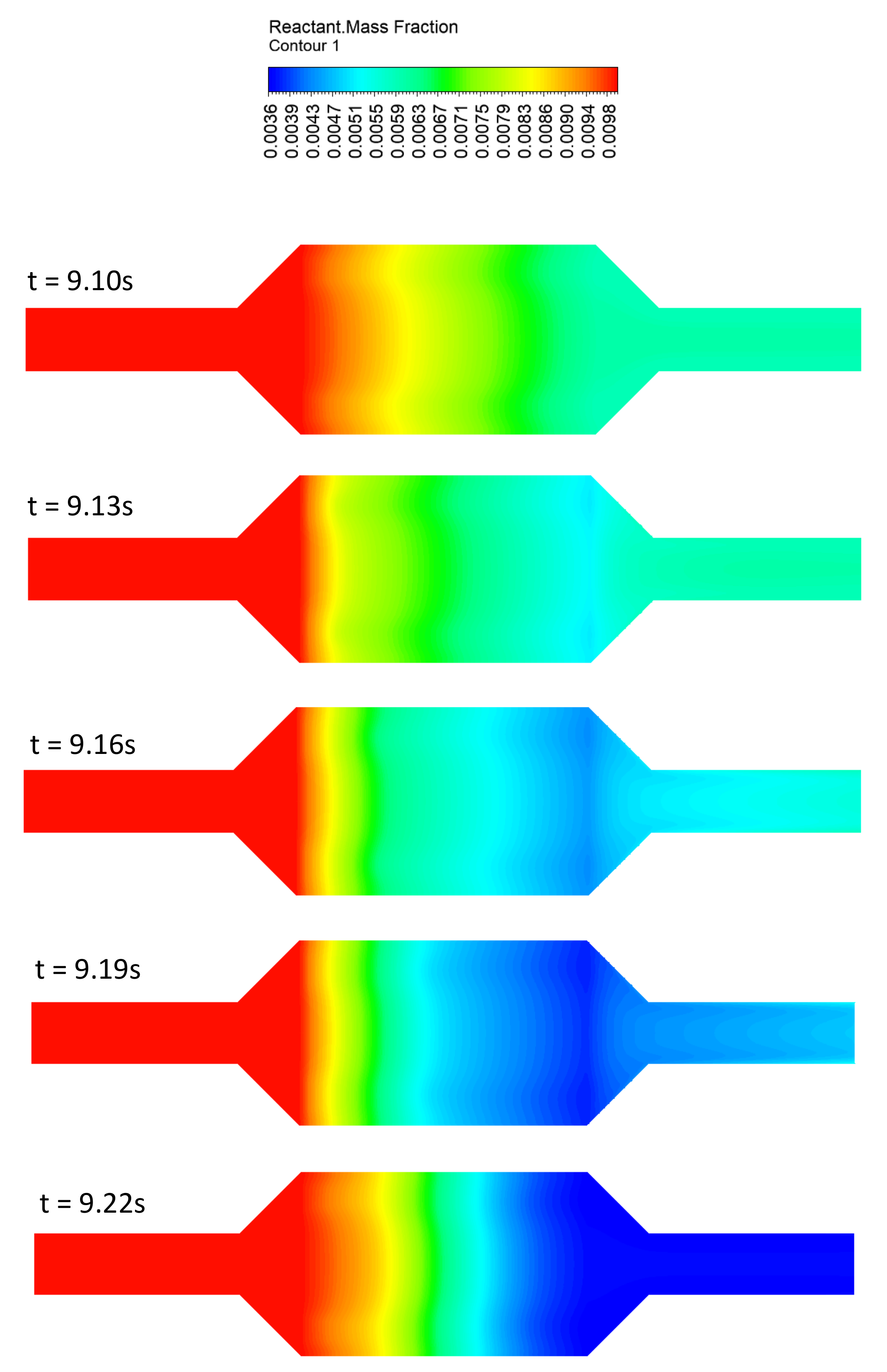
3. Results
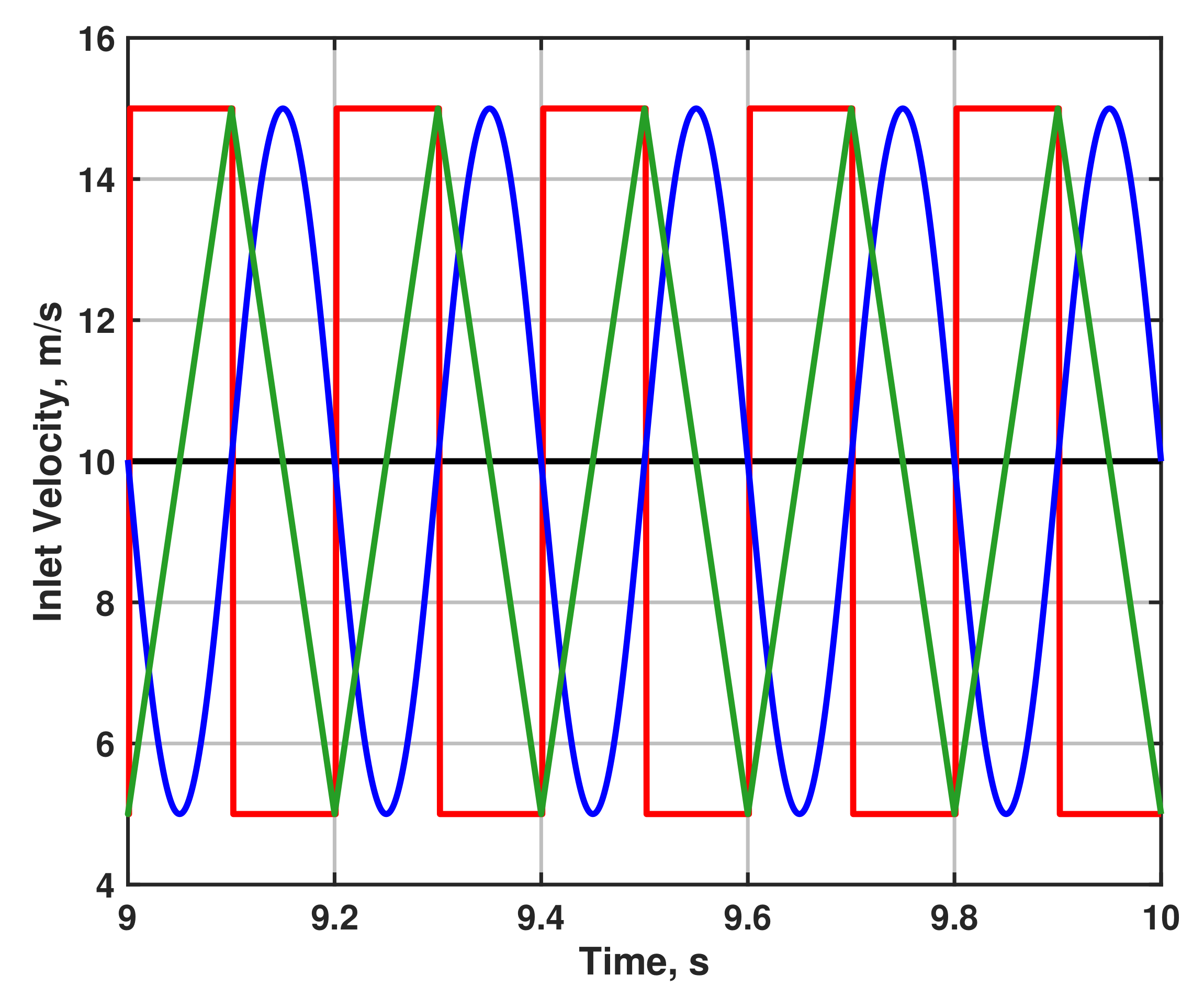
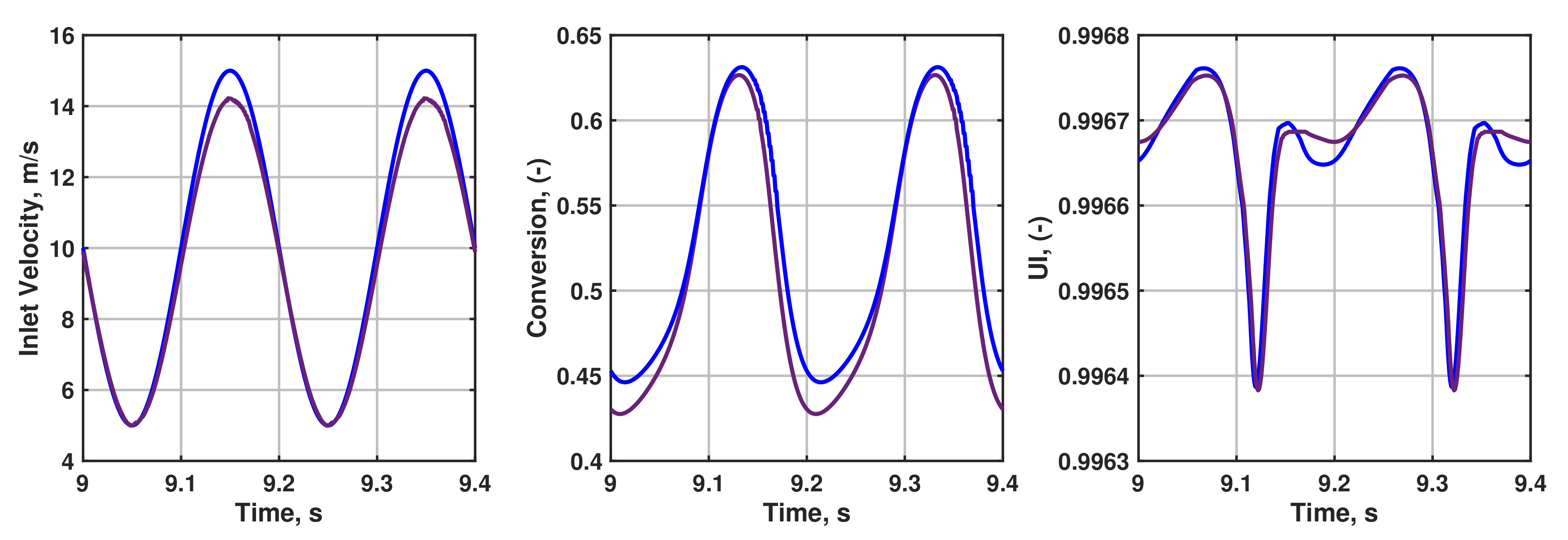
3.1. Comparison of Resulting Inlet Velocity Profiles
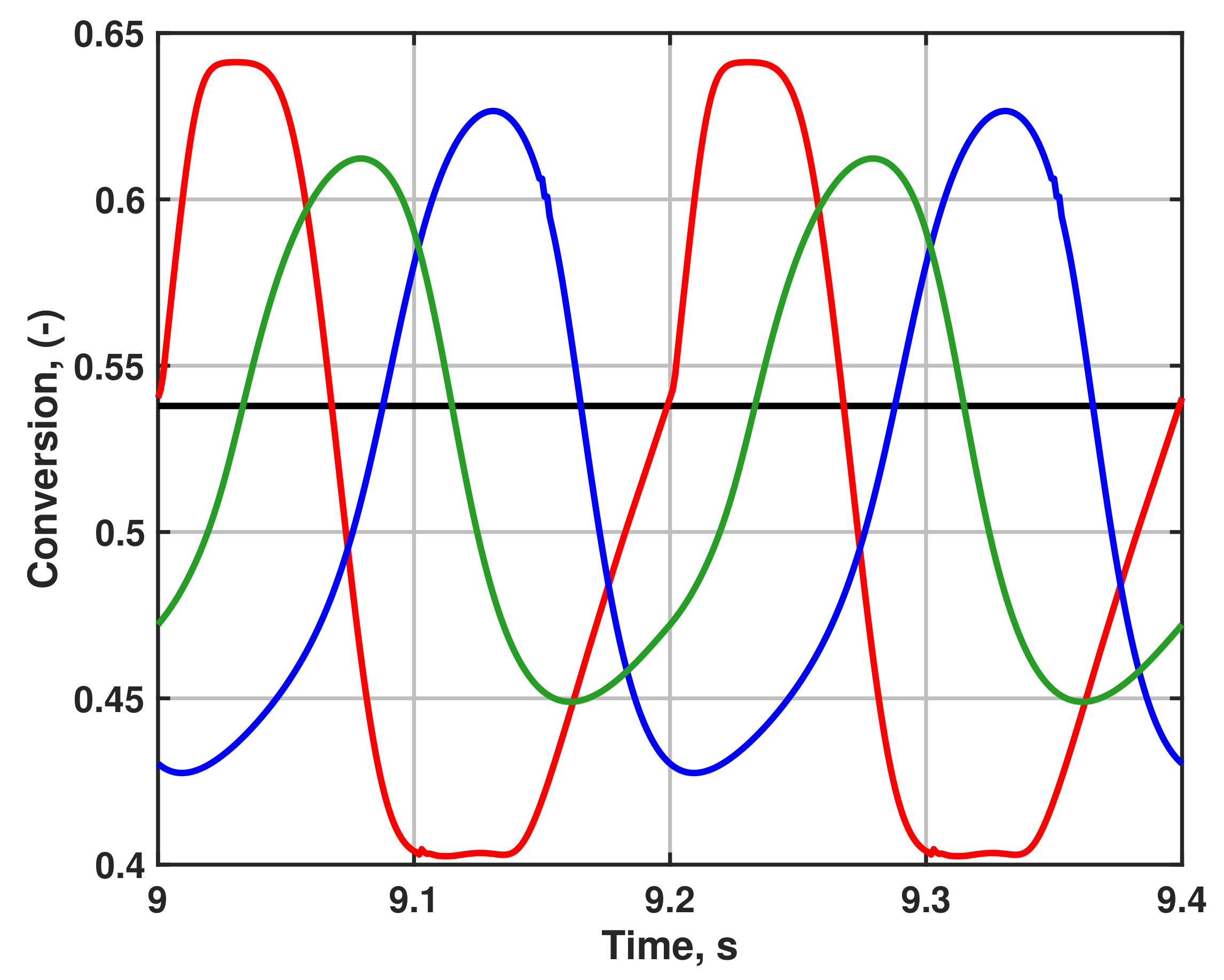
3.2. Time-Averaged Quantities
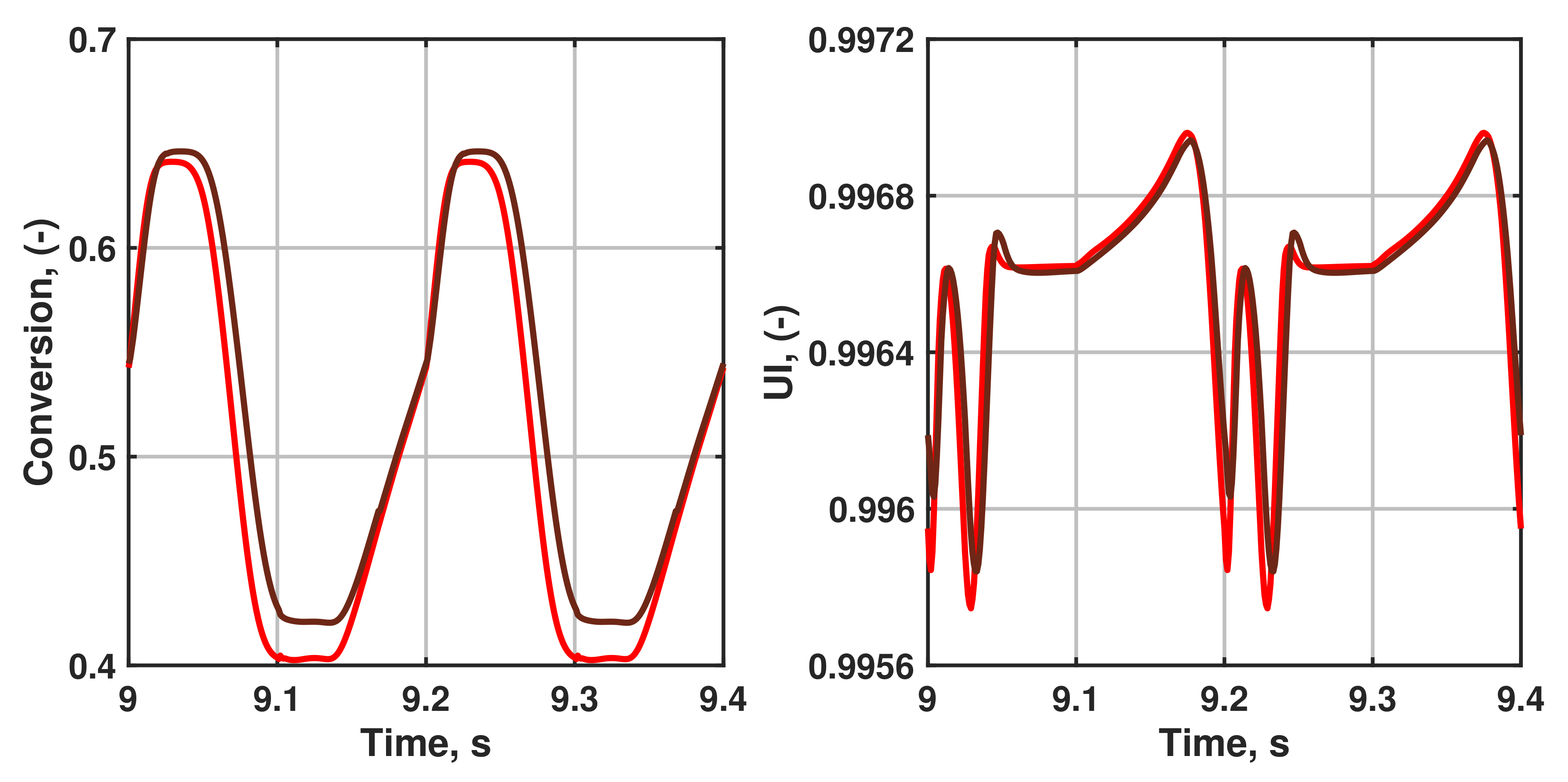
3.3. Time-Resolved Quantities
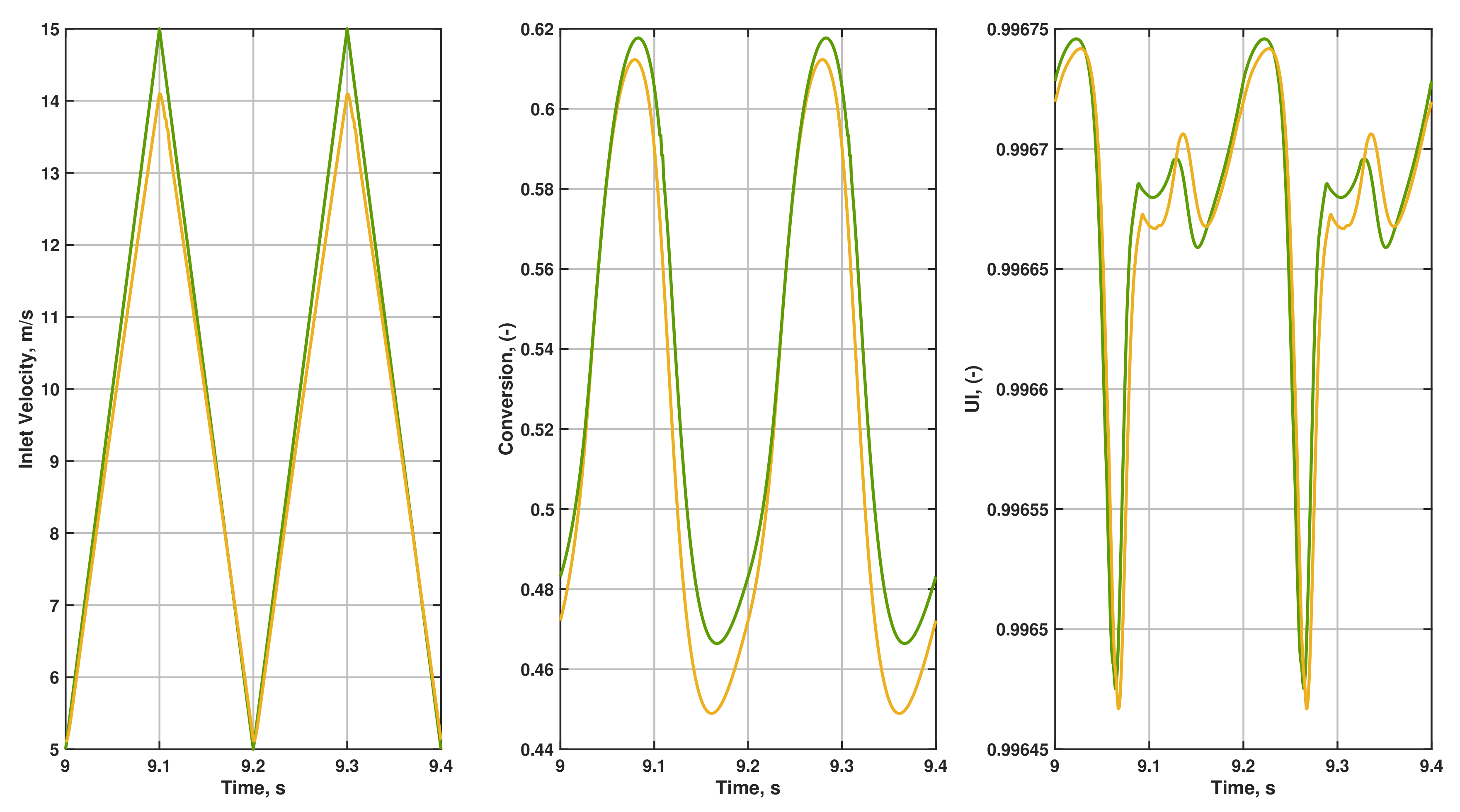
3.4. Influence of Prescribing Pressure Instead of Velocity at the Inlet Boundary
3.5. Influence of Inlet Temperature Variations
4. Discussion
5. Conclusions
- Fluctuations and pulsations in the incoming flow to a monolithic reactor in an aftertreatment system both affects the transient response of the reactor as well as its time-averaged performance;
- Pulsations act via two main mechanisms: modulation of the average retention time in the catalyst and modulation of the retention time distribution via the flow maldistribution over the reactor cross-section;
- The range spanned by the mixed-cup conversion at the outlet of an aftertreatment system is not identical for geometrically identical systems spanning the same velocity range, as dispersion mechanisms inside the monolith and the outlet cone and pipe sections create mixing environments that depend on the fluctuations and their interactions with the geometry itself:
- The dispersion mechanisms depend in a non-trivial way on the temporal specification of the flow inlet boundary condition;
- Simulations of phenomena that depend on time-resolved boundary conditions from experiments are likely to require interpolation in sparsely sampled information—the method of recreating the signal at the boundary may then influence the results;
- Prescribing an inlet pressure rather than an inlet velocity in simulations of monolithic reactors in aftertreatment systems results in smoother transient boundary condition profiles and avoidance of intermittent flow discontinuity propagation through the system, at the expense of a loss of direct proportionality between fluctuation and resulting velocity (due to non-linear losses at high velocities);
- Temperature fluctuations have a potential to affect reaction rates profoundly due to the strong non-linear dependence, but the effect is partially out-weighted by anti-correlated retention-time fluctuations.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| CDF | Cumulative Distribution Function |
| CFD | Computational Fluid Dynamics |
| MUSCL | Monotonic Upstream-centered Scheme for Conservation Laws |
| RHS | Right Hand Side |
| SCM | Single Channel Model |
| SST | Shear Stress Transport |
| URANS | Unsteady Reynolds-Averaged Navier-Stokes |
References
- Heck, R.M.; Farrauto, R.J.; Gulati, S.T. Catalytic Air Pollution Control: Commercial Technology; John Wiley & Sons: Hoboken, NJ, USA, 2016. [Google Scholar]
- Deutschmann, O. Modeling of the interactions between catalytic surfaces and gas-phase. Catal. Lett. 2015, 145, 272–289. [Google Scholar] [CrossRef]
- Martin, A.P.; Will, N.S.; Bordet, A.; Cornet, P.; Gondoin, C.; Mouton, X. Effect of flow distribution on emissions performance of catalytic converters. SAE Trans. 1998, 107, 384–390. [Google Scholar]
- Chen, J.; Yang, H.; Wang, N.; Ring, Z.; Dabros, T. Mathematical modeling of monolith catalysts and reactors for gas phase reactions. Appl. Catal. Gen. 2008, 345, 1–11. [Google Scholar] [CrossRef]
- Walander, M.; Sjöblom, J.; Creaser, D.; Agri, B.; Löfgren, N.; Tamm, S.; Edvardsson, J. Modelling of mass transfer resistances in non-uniformly washcoated monolith reactors. Emiss. Control. Sci. Technol. 2021, 7, 153–162. [Google Scholar] [CrossRef]
- Chakravarthy, V.K.; Conklin, J.C.; Daw, C.S.; D’Azevedo, E.F. Multi-dimensional simulations of cold-start transients in a catalytic converter under steady inflow conditions. Appl. Catal. A Gen. 2003, 241, 289–306. [Google Scholar] [CrossRef]
- Bella, G.; Rocco, V.; Maggiore, M. A Study of Inlet Flow Distortion Effects on Automotive Catalytic Converters. J. Eng. Gas Turbines Power 1991, 113, 419–426. [Google Scholar] [CrossRef]
- Zygourakis, K. Transient operation of monolith catalytic converters: A two-dimensional reactor model and the effects of radially nonuniform flow distributions. Chem. Eng. Sci. 1989, 44, 2075–2086. [Google Scholar] [CrossRef]
- Bressler, H.; Rammoser, D.; Neumaier, H.; Terres, F. Experimental and predictive investigation of a close coupled catalyst converter with pulsating flow. SAE Trans. 1996, 105, 255–267. [Google Scholar]
- Liu, Z.; Benjamin, S.F.; Roberts, C.A. Pulsating flow maldistribution within an axisymmetric catalytic converter-flow rig experiment and transient cfd simulation. SAE Tech. Pap. 2003. [Google Scholar] [CrossRef]
- Benjamin, S.; Roberts, C.; Wollin, J. A study of pulsating flow in automotive catalyst systems. Exp. Fluids 2002, 33, 629–639. [Google Scholar] [CrossRef]
- Jeong, S.-J. A full transient three-dimensional study on the effect of pulsating exhaust flow under real running condition on the thermal and chemical behavior of closed-coupled catalyst. Chem. Eng. Sci. 2014, 117, 18–30. [Google Scholar] [CrossRef]
- Tsinoglou, D.; Koltsakis, G. Influence of pulsating flow on close-coupled catalyst performance. J. Eng. Gas Turbines Power 2005, 127, 676–682. [Google Scholar] [CrossRef]
- Ström, H.; Sasic, S.; Andersson, B. Turbulent operation of diesel oxidation catalysts for improved removal of particulate matter. Chem. Eng. Sci. 2012, 69, 231–239. [Google Scholar] [CrossRef]
- Fogler, H.S. Elements of Chemical Reaction Engineering; Pearson Education: London, UK, 1999. [Google Scholar]
- Menter, F.R. Two-equation eddy-viscosity turbulence models for engineering applications. AIAA J. 1994, 32, 1598–1605. [Google Scholar] [CrossRef] [Green Version]
- Bodin, O.; Wang, Y.; Mihaescu, M.; Fuchs, L. LES of the Exhaust Flow in a Heavy-Duty Engine. Oil Gas Sci. Technol. Rev. IFP Energies Nouv. 2014, 69, 177–188. [Google Scholar] [CrossRef] [Green Version]
- Semlitsch, B.; Wang, Y.; Mihaescu, M. Flow effects due to pulsation in an internal combustion engine exhaust port. Energy Convers. Manag. 2001, 86, 520–536. [Google Scholar] [CrossRef] [Green Version]
- Torregrosa, A.J.; Serrano, J.R.; Arnau, F.J.; Piqueras, P. A fluid dynamic model for unsteady compressible flow in wall-flow diesel particulate filters. Energy 2011, 36, 671–684. [Google Scholar] [CrossRef] [Green Version]
- Kim, M.-H. Three-Dimensional Numerical Study on the Pulsating Flow Inside Automotive Muffler with Complicated Flow Path. SAE Trans. 2001, 110, 805–811. [Google Scholar]
- Ström, H.; Sasic, S. Heat and mass transfer in automotive catalysts—The influence of turbulent velocity fluctuations. Chem. Eng. Sci. 2012, 83, 128–137. [Google Scholar] [CrossRef]
- Tanno, K.; Kurose, R.; Michioka, T.; Makino, H.; Komori, S. Direct numerical simulation of flow and surface reaction in de-NOx catalyst. Adv. Powder Technol. 2013, 24, 879–885. [Google Scholar] [CrossRef]
- Cornejo, I.; Nikrityuk, P.; Hayes, R.E. Turbulence Decay Inside the Channels of an Automotive Catalytic Converter Monolith. Emiss. Control Sci. Technol. 2017, 3, 302–309. [Google Scholar] [CrossRef]
- Hewakandamby, B.N. A numerical study of heat transfer performance of oscillatory impinging jets. Int. J. Heat Mass Transf. 2009, 52, 396–406. [Google Scholar] [CrossRef]
- Suga, K.; Matsumura, Y.; Ashitaka, Y.; Tominaga, S.; Kaneda, M. Effects of wall permeability on turbulence. Int. J. Heat Fluid Flow 2010, 31, 974–984. [Google Scholar] [CrossRef]
- Suga, K. Understanding and Modelling Turbulence Over and Inside Porous Media. Flow Turbul. Combust. 2016, 96, 717–756. [Google Scholar] [CrossRef]
- Andersson, R.; Andersson, B. Enhanced mass and heat transfer in catalytic reactors. Catal. Today 2013, 216, 117–120. [Google Scholar] [CrossRef]




| Phenomenon | Timescale |
|---|---|
| Turbo pulsations | |
| Lambda control | |
| Driver interaction | |
| Catalyst heatup |
| Property | Value |
|---|---|
| ideal gas law 1 | |
| Sutherland’s law 2 | |
| J/(kg·K) | |
| k | W/(m·K) |
| m2/s |
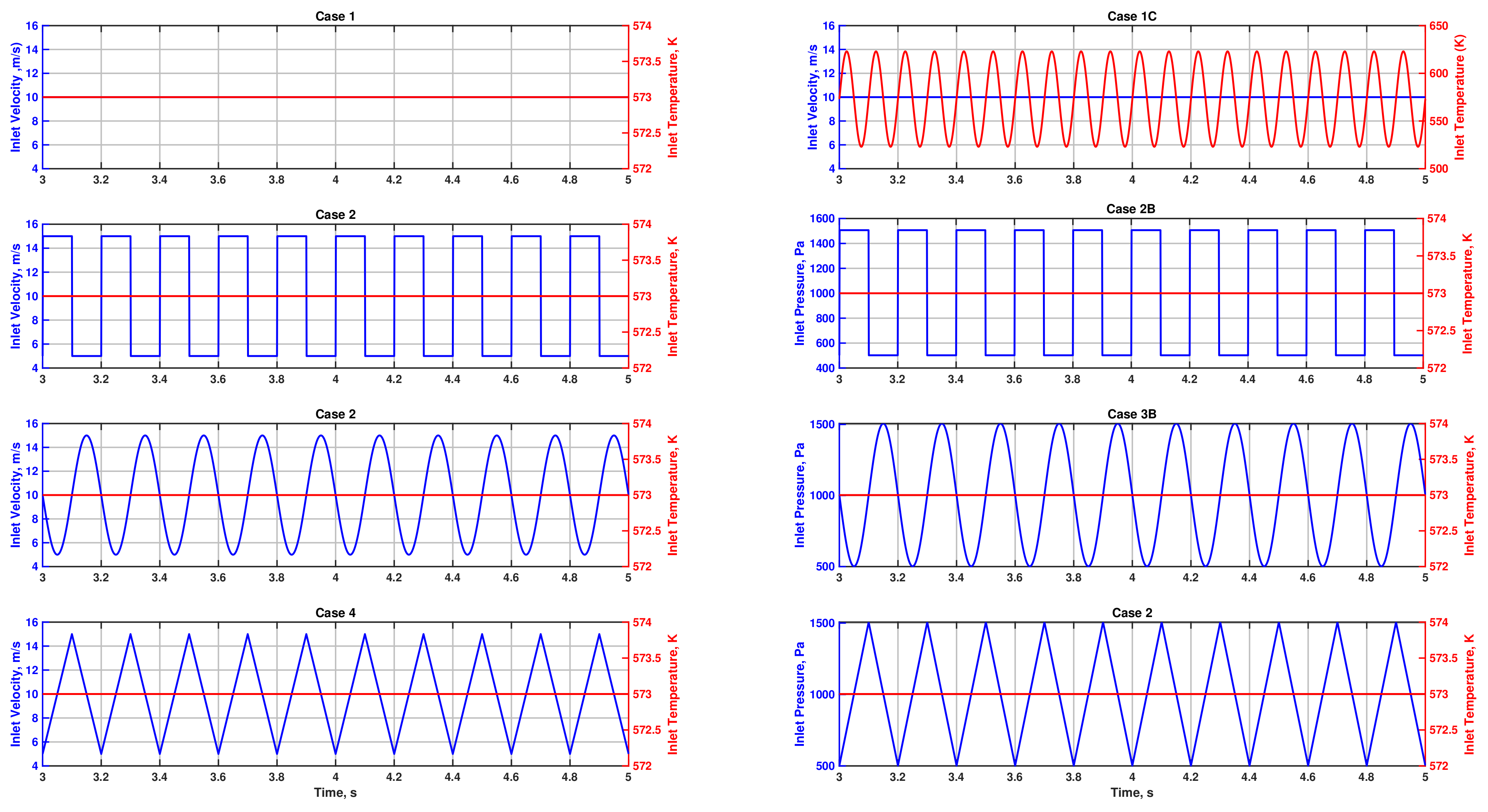
| Case | Flow Inlet Boundary Condition 1 | Temperature Boundary Condition 2 |
|---|---|---|
| Case 1 | ||
| Case 1C | ||
| Case 2 | ||
| Case 2B | ||
| Case 3 | ||
| Case 3B | ||
| Case 4 | ||
| Case 4B |
| Case | Velocity [m/s] | Conversion | Uniformity Index | Temperature [K] |
|---|---|---|---|---|
| Case 1 | ||||
| Case 1C | ||||
| Case 2 | ||||
| Case 2B | ||||
| Case 3 | ||||
| Case 3B | ||||
| Case 4 | ||||
| Case 4B |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chanda Nagarajan, P.; Ström, H.; Sjöblom, J. Numerical Assessment of Flow Pulsation Effects on Reactant Conversion in Automotive Monolithic Reactors. Catalysts 2022, 12, 613. https://doi.org/10.3390/catal12060613
Chanda Nagarajan P, Ström H, Sjöblom J. Numerical Assessment of Flow Pulsation Effects on Reactant Conversion in Automotive Monolithic Reactors. Catalysts. 2022; 12(6):613. https://doi.org/10.3390/catal12060613
Chicago/Turabian StyleChanda Nagarajan, Pratheeba, Henrik Ström, and Jonas Sjöblom. 2022. "Numerical Assessment of Flow Pulsation Effects on Reactant Conversion in Automotive Monolithic Reactors" Catalysts 12, no. 6: 613. https://doi.org/10.3390/catal12060613
APA StyleChanda Nagarajan, P., Ström, H., & Sjöblom, J. (2022). Numerical Assessment of Flow Pulsation Effects on Reactant Conversion in Automotive Monolithic Reactors. Catalysts, 12(6), 613. https://doi.org/10.3390/catal12060613






