Evolution Mechanism of Transient Strain and Residual Stress Distribution in Al 6061 Laser Welding
Abstract
:1. Introduction
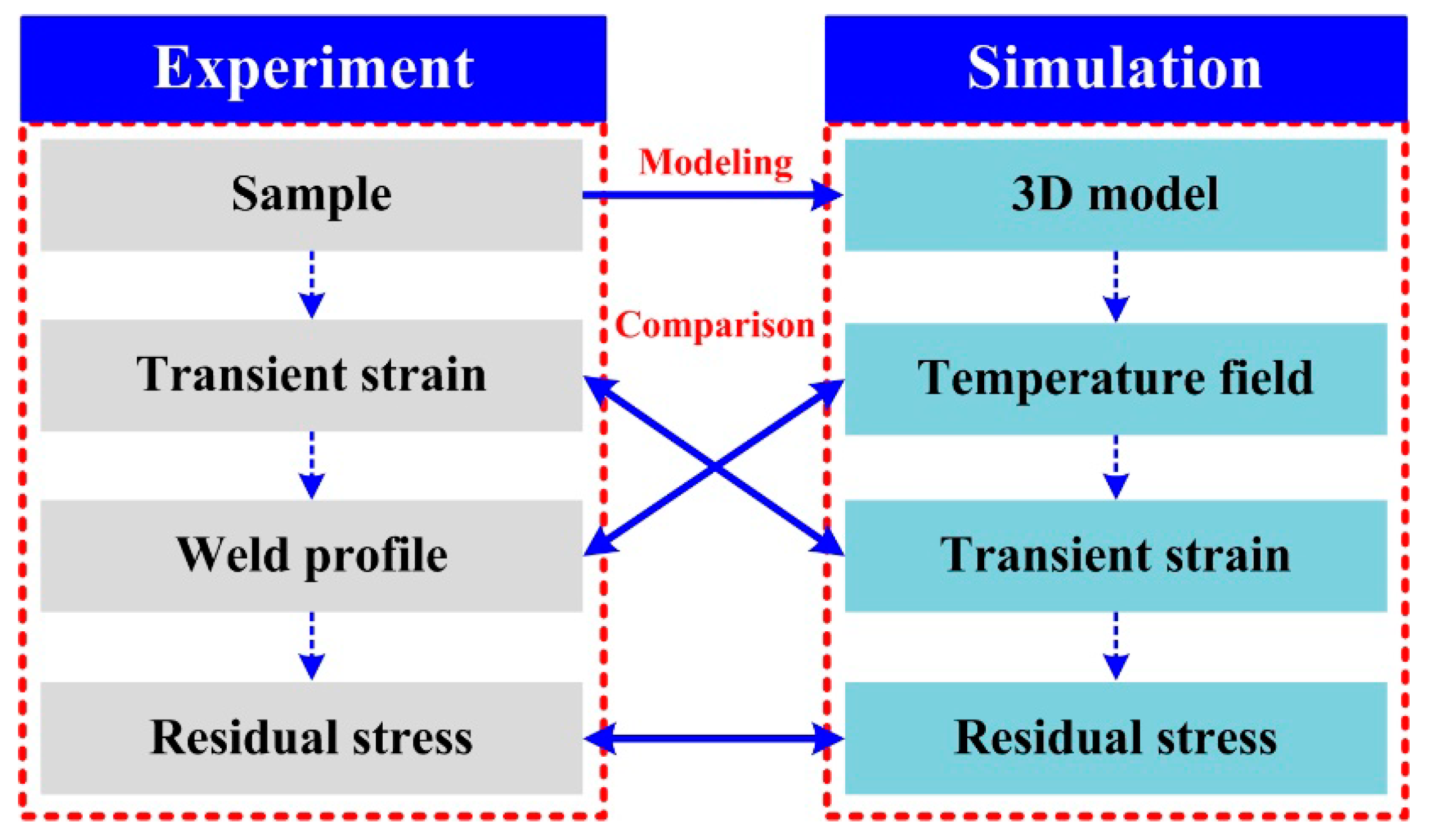
2. Experiment and Measurement
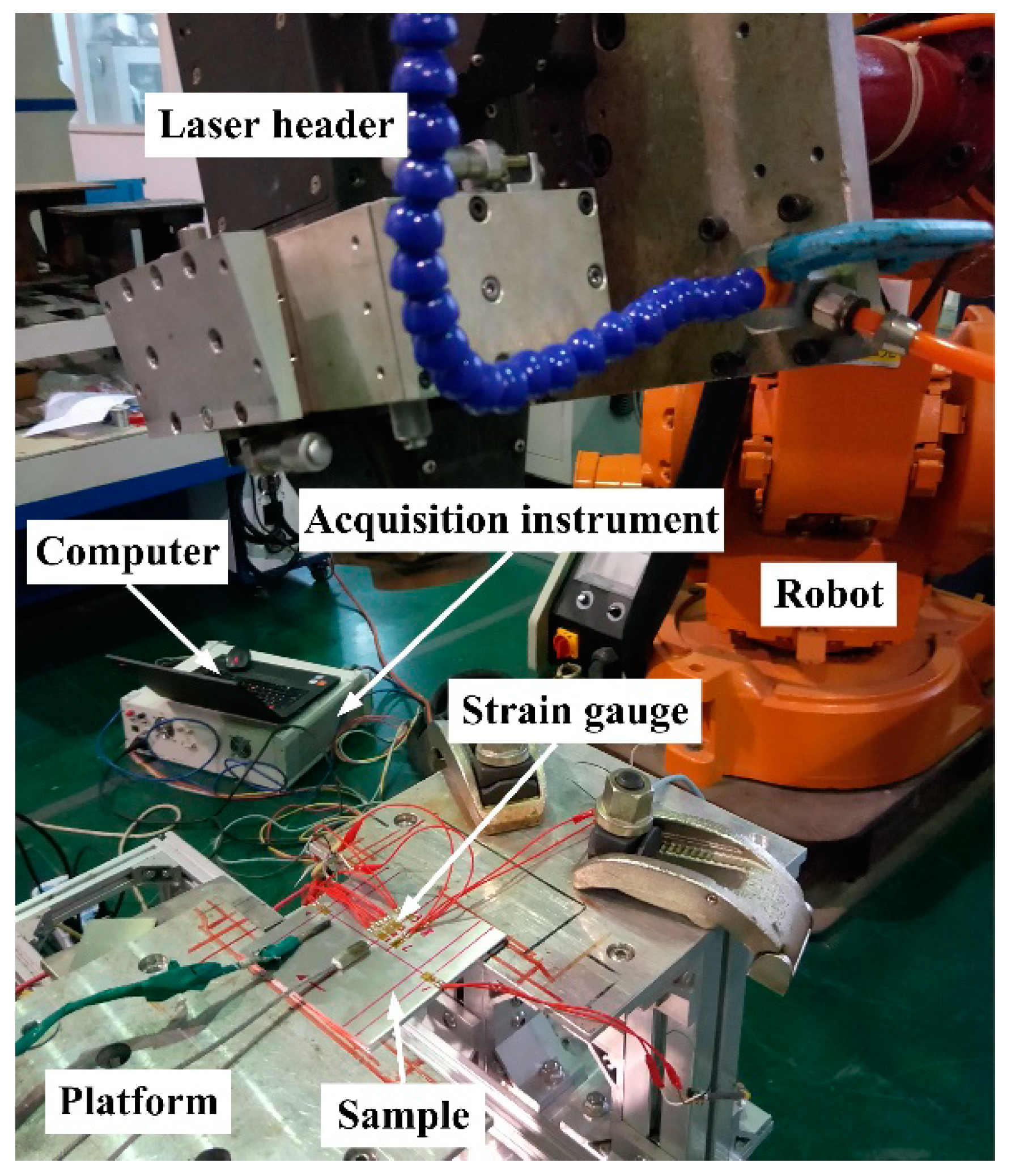
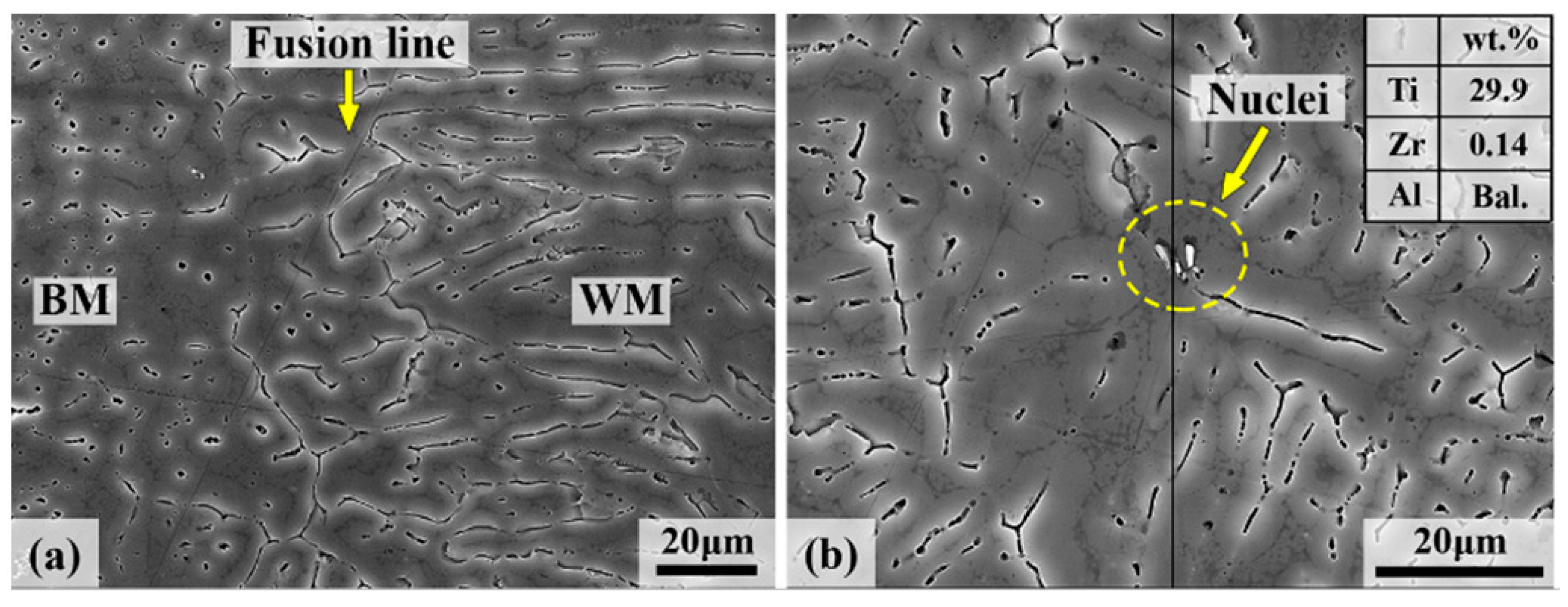
2.1. Materials and Experimental Procedures
2.2. Measurement Methods
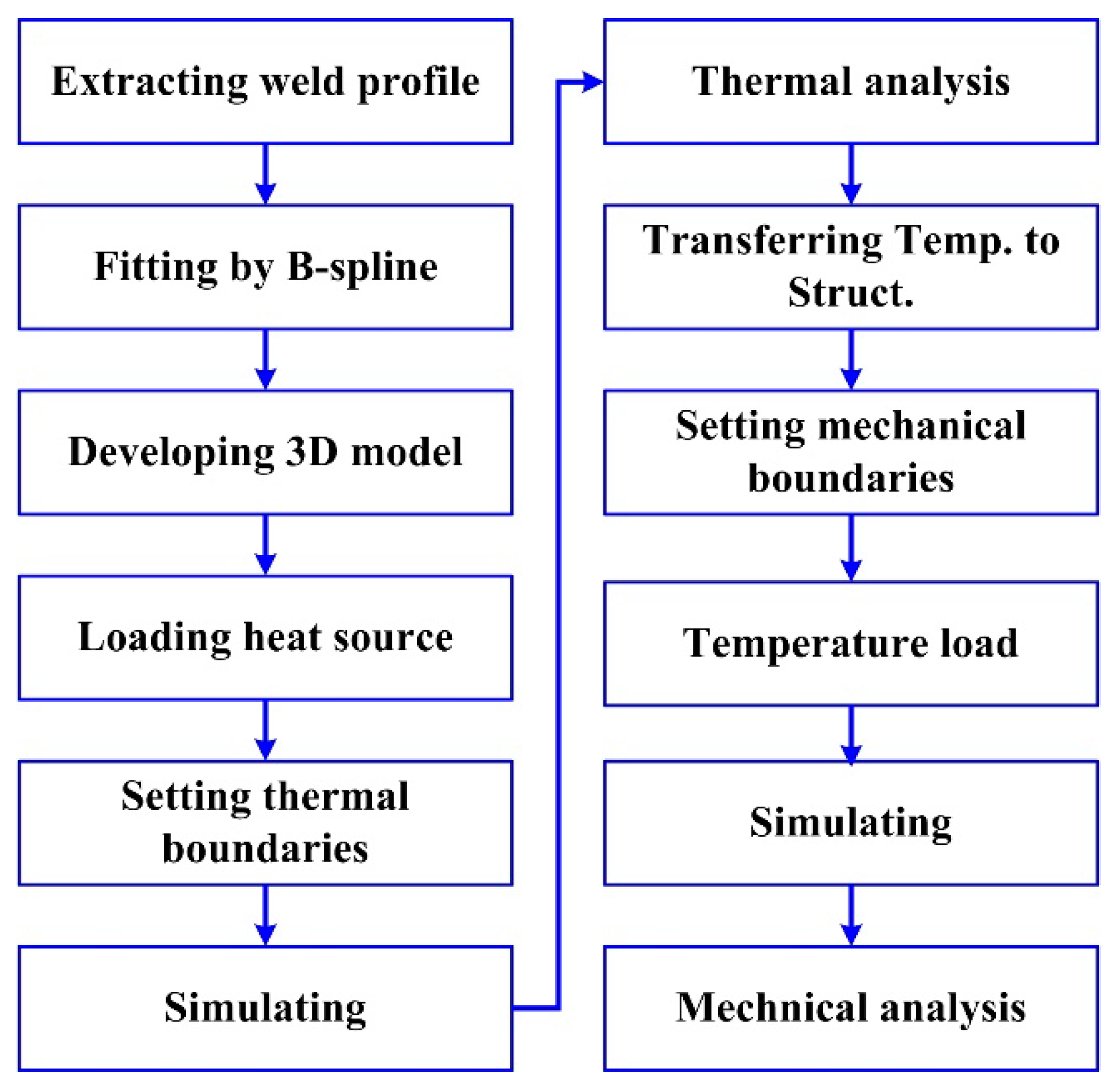
3. Modeling and Simulation
3.1. TEP-FEA Framework
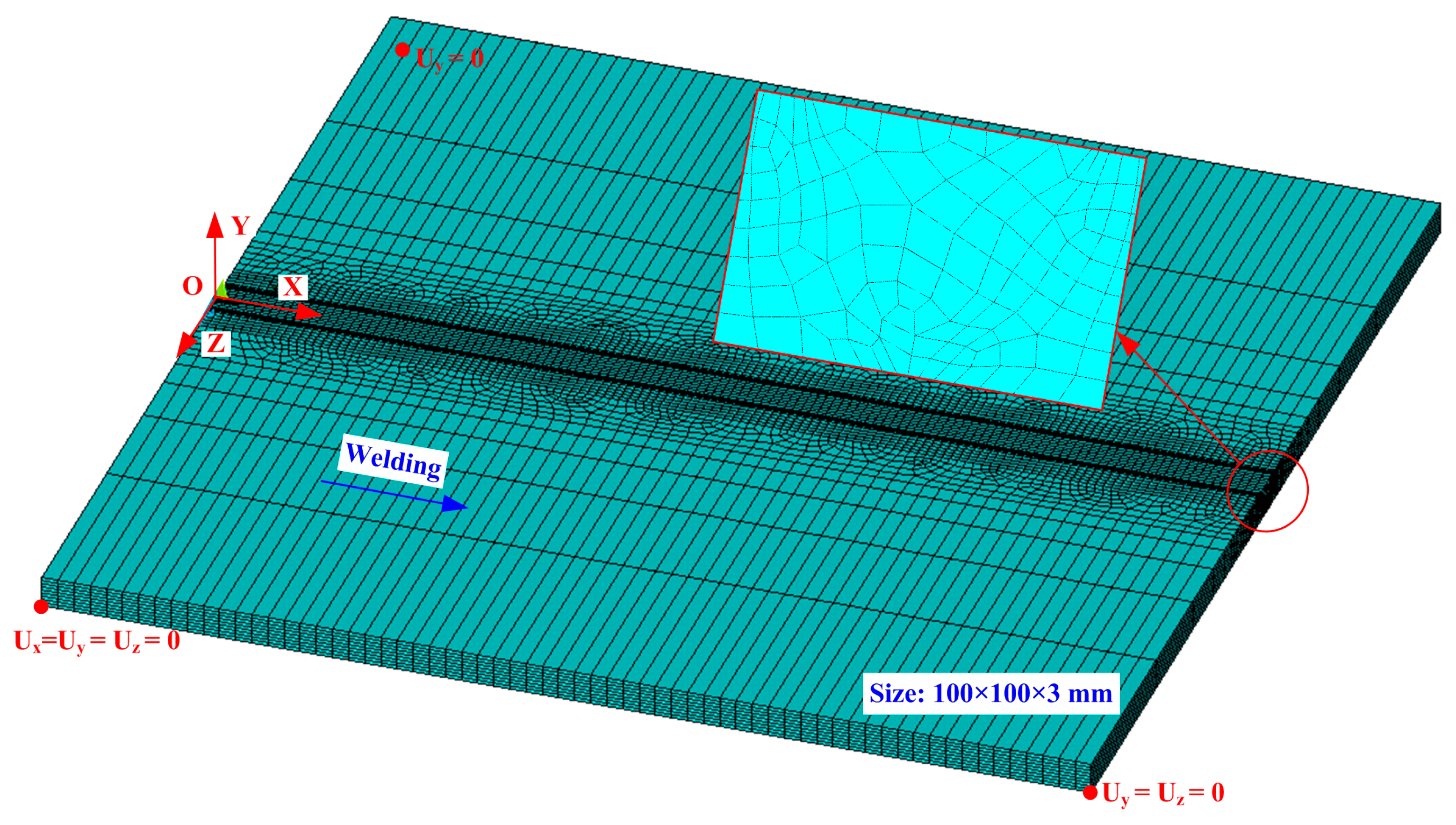
3.2. Thermal Analysis Method
3.3. Mechanical Analysis Method
4. Results and Discussion
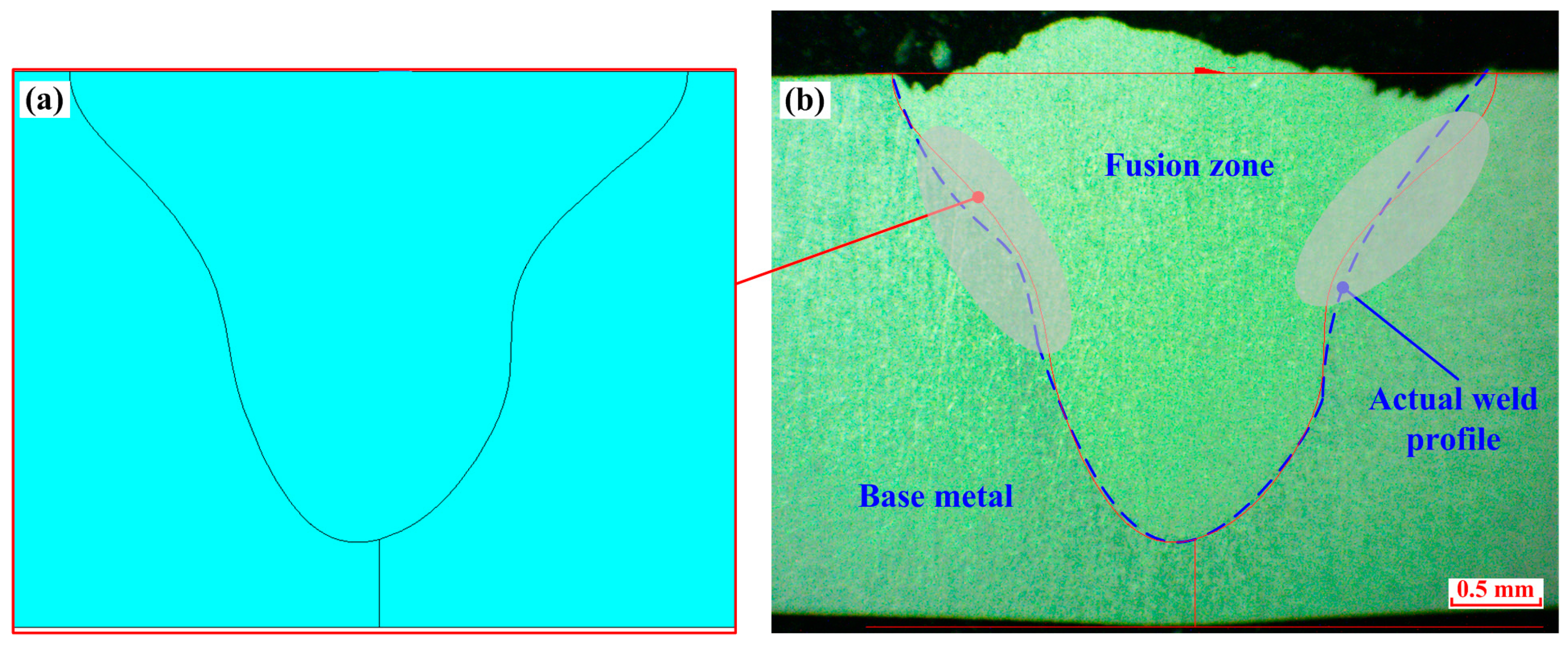
4.1. Reconstruction of Weld Profile by B-Spline Curve
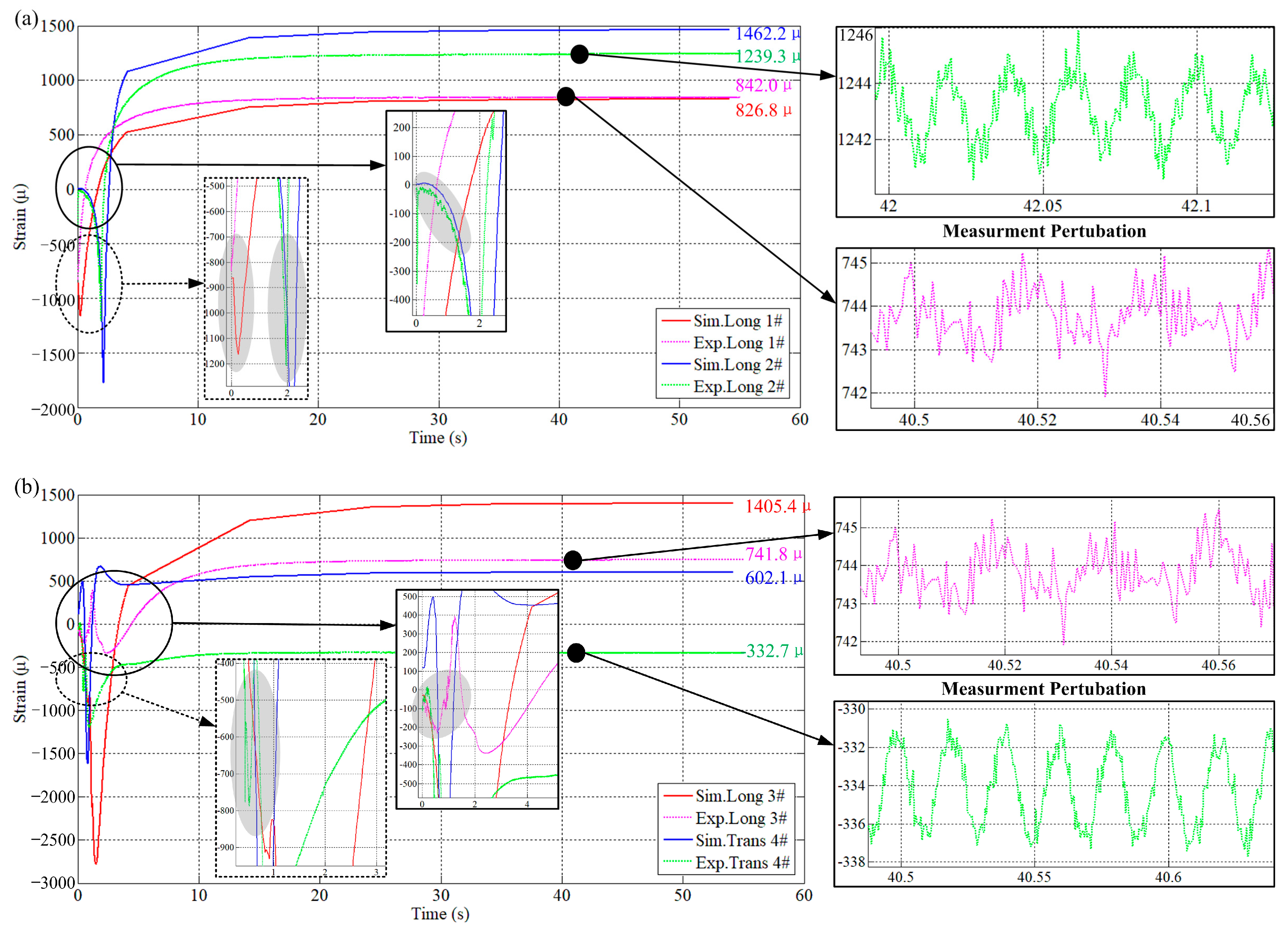
4.2. Fluctuation Analysis of the Transient Strain
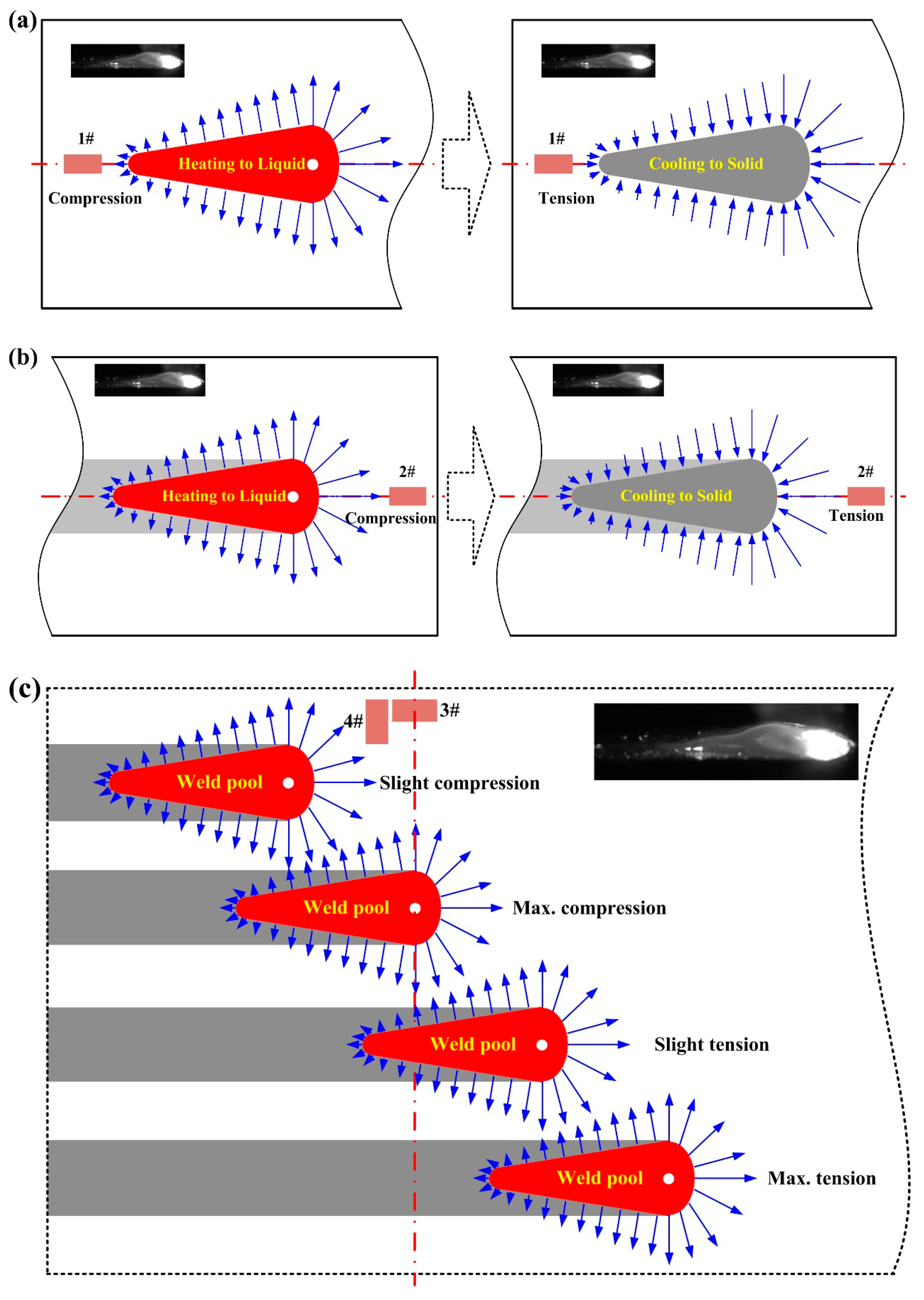
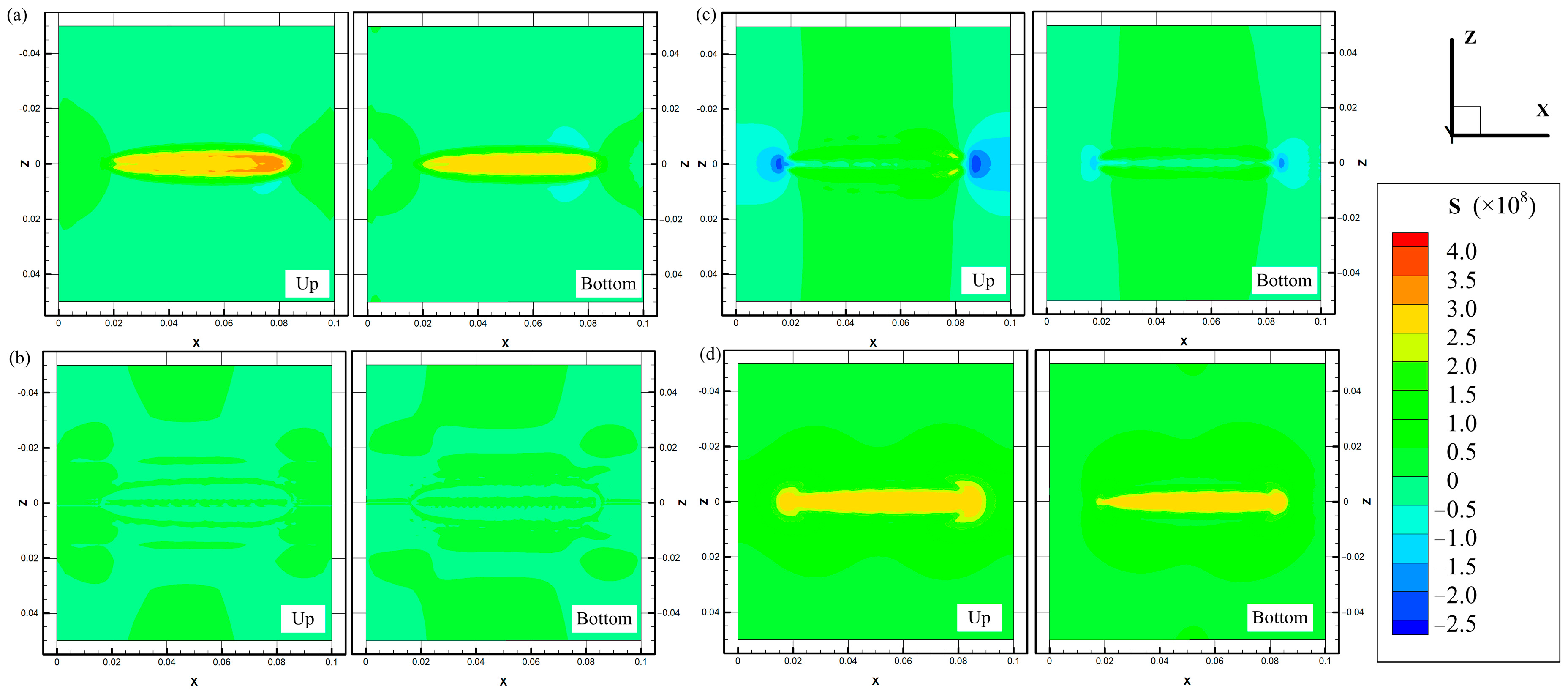
4.3. Discussion of Evolution Mechanism of Transient Strain
4.4. Residual Distortion and Stress Analysis
5. Conclusions
- (1)
- The actual weld profile was first fitted by a B-spline curve with 6 control points to load the efficient heat source model in TEP-FEA. The fitting profile kept a good consistency with the actual weld shape, especially at the lower part of the whole weld. A maximum fitting deviation of 0.13 mm was measured at the upper part, which was mainly due to the number and position limitations of control points.
- (2)
- Fluctuation analysis of the transient strain at four points was investigated by coupling experimental analysis with TEP-FEA. A common characteristic of 1# and 2# was that longitudinal strain started from a fluctuating compressive state and progressed to an ultimate residual tension state. Experimental and simulation results of residual strain are 842.0 μ and 826.8 μ, with a relative error of 1.805% at the starting position and −17.986% at the ending position. At 3# and 4#, the mechanical state was complexly influenced by thermal expansion and contraction in the weld zone and the reaction binding force of the solid metal, and simulated strains were nearly twice as high as experiment results because liquid flow in the weld pool was ignored in TEP-FEA.
- (3)
- The evolution mechanism of transient strain in laser welding of the aluminum alloy was also discussed in terms of the unbalanced procedure of melting and solidification, considering that it originated from the external laser beam and the fundamental driving force of inhomogeneous mechanical behaviors.
- (4)
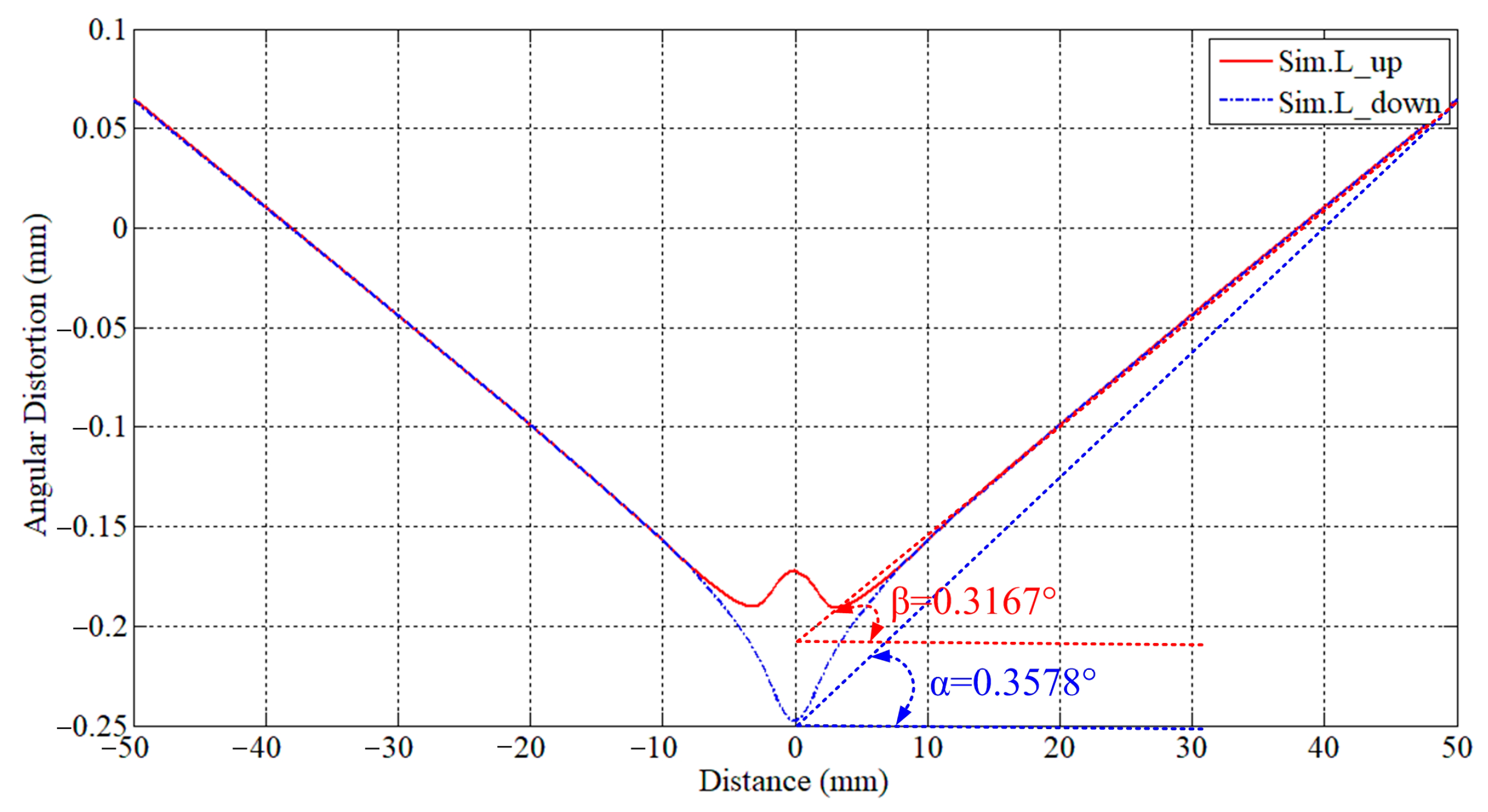
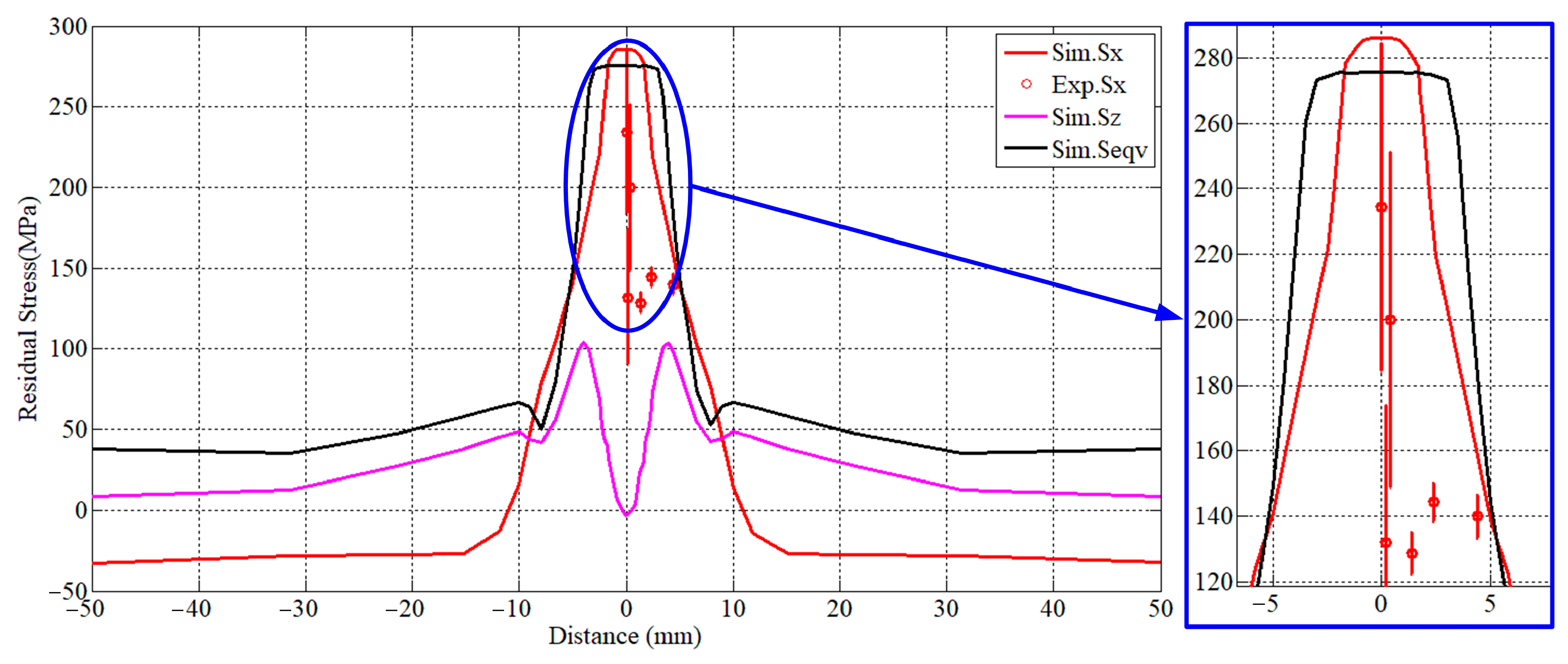
- Angular distortion along the transverse center line of the weld sample was 0.33725°. Longitudinal stress was in a tension state within a distance between −10 mm and 10 mm. In the range of −10 mm to 10 mm, transverse stress fluctuated greatly with a high gradient (~100 MPa). The tendency of equivalent stress was similar to that of transverse stress far from the weld center and longitudinal stress near the weld center.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Wang, L.; Wei, Y.; Chen, J.; Zhao, W. Macro-micro modeling and simulation on columnar grains growth in the laser welding pool of aluminum alloy. Int. J. Heat Mass Transf. 2018, 123, 826–838. [Google Scholar] [CrossRef]
- Ye, Z.; Huang, J.; Cheng, Z.; Gao, W.; Zhang, Y.; Chen, S.; Yang, J. Combined effects of MIG and TIG arcs on weld appearance and interface properties in Al/steel double-sided butt welding-brazing. J. Mater. Process. Technol. 2017, 250, 25–34. [Google Scholar] [CrossRef]
- Olshanskaya, T.V.; Salomatova, E.S.; Belenkiy, V.Y.; Trushnikov, D.N.; Permyakov, G.L. Electron beam welding of aluminum alloy AlMg6 with a dynamically positioned electron beam. Int. J. Adv. Manuf. Technol. 2017, 89, 3439–3450. [Google Scholar] [CrossRef]
- Wang, Z.B.; He, Z.B.; Fan, X.B.; Zhou, L.; Lin, Y.L.; Yuan, S.J. High temperature deformation behavior of friction stir welded 2024-T4 aluminum alloy sheets. J. Mater. Process. Technol. 2017, 247, 184–191. [Google Scholar] [CrossRef]
- Huang, L.; Wu, D.; Hua, X.; Liu, S.; Jiang, Z.; Li, F.; Wang, H.; Shi, S. Effect of the welding direction on the microstructural characterization in fiber laser-GMAW hybrid welding of 5083 aluminum alloy. J. Manuf. Process. 2018, 31, 514–522. [Google Scholar] [CrossRef]
- Tan, Y.B.; Wang, X.M.; Ma, M.; Zhang, J.X.; Liu, W.C.; Fu, R.D.; Xiang, S. A study on microstructure and mechanical properties of AA 3003 aluminum alloy joints by underwater friction stir welding. Mater. Charact. 2017, 127, 41–52. [Google Scholar] [CrossRef]
- Tabasi, M.; Farahani, M.; Givi, M.K.B.; Farzami, M.; Moharami, A. Dissimilar friction stir welding of 7075 aluminum alloy to AZ31 magnesium alloy using SiC nanoparticles. Int. J. Adv. Manuf. Technol. 2016, 86, 705–715. [Google Scholar] [CrossRef]
- Martin, J.H.; Yahata, B.D.; Hundley, J.M.; Mayer, J.A.; Schaedler, T.A.; Pollock, T.M. 3D printing of high-strength aluminium alloys. Nature 2017, 549, 365–369. [Google Scholar] [CrossRef]
- Wu, D.; Hua, X.; Huang, L.; Zhao, J. Numerical simulation of spatter formation during fiber laser welding of 5083 aluminum alloy at full penetration condition. Opt. Laser Technol. 2018, 100, 157–164. [Google Scholar] [CrossRef] [Green Version]
- Zhang, D.; Li, C.; Liu, X.; Cao, Y.; Wu, D. Numerical study of spatter formation during fiber laser welding of aluminum alloy. J. Manuf. Process. 2018, 31, 72–79. [Google Scholar] [CrossRef]
- Huang, L.; Hua, X.; Wu, D.; Li, F. Numerical study of keyhole instability and porosity formation mechanism in laser welding of aluminum alloy and steel. J. Mater. Process. Technol. 2018, 252, 421–431. [Google Scholar] [CrossRef]
- Moraitis, G.A.; Labeas, G.N. Residual stress and distortion calculation of laser beam welding for aluminum lap joints. J. Mater. Process. Technol. 2008, 198, 260–269. [Google Scholar] [CrossRef]
- Ji, P.; Zhang, J.; Zheng, L.; Xiao, Y.; Dou, S.; Cui, X.; Lian, Y. Comparison of residual stress determination using different crystal planes by short-wavelength X-ray diffraction in a friction-stir-welded aluminum alloy plate. J. Mater. Sci. 2017, 52, 12834–12847. [Google Scholar] [CrossRef]
- Eisazadeh, H.; Aidun, D.K. Investigation of transient/residual strain and stress in dissimilar weld. J. Manuf. Process. 2017, 26, 372–381. [Google Scholar] [CrossRef]
- Balokhonov, R.; Romanova, V.; Batukhtina, E.; Martynov, S.; Zinoviev, A.; Zinovieva, O. A mesomechanical analysis of the stress–strain localization in friction stir welds of polycrystalline aluminium alloys. Meccanica 2016, 51, 319–328. [Google Scholar] [CrossRef]
- Wang, X.; Li, B.; Li, M.; Huang, C.; Chen, H. Study of local-zone microstructure, strength and fracture toughness of hybrid laser-metal-inert-gas-welded A7N01 aluminum alloy joint. Mater. Sci. Eng. A Struct. Mater. Prop. Microstruct. Process. 2017, 688, 114–122. [Google Scholar] [CrossRef]
- Sheikhi, M.; Ghaini, F.M.; Assadi, H. Prediction of solidification cracking in pulsed laser welding of 2024 aluminum alloy. Acta Mater. 2015, 82, 491–502. [Google Scholar] [CrossRef]
- Wang, L.; Gao, M.; Zhang, C.; Zeng, X. Effect of beam oscillating pattern on weld characterization of laser welding of AA6061-T6 aluminum alloy. Mater. Des. 2016, 108, 707–717. [Google Scholar] [CrossRef]
- Zhu, Z.Y.; Deng, C.Y.; Wang, Y.; Yang, Z.W.; Ding, J.K.; Wang, D.P. Effect of post weld heat treatment on the microstructure and corrosion behavior of AA2219 aluminum alloy joints welded by variable polarity tungsten inert gas welding. Mater. Des. 2015, 65, 1075–1082. [Google Scholar] [CrossRef]
- Zhang, D.K.; Zhao, Y.; Dong, M.Y.; Wang, G.Q.; Wu, A.P.; Shan, J.G.; Meng, D.Y.; Liu, X.L.; Song, J.L.; Zhang, Z.P. Effects of weld penetration on tensile properties of 2219 aluminum alloy TIG-welded joints. Trans. Nonferrous Met. Soc. China 2019, 29, 1161–1168. [Google Scholar] [CrossRef]
- Duan, C.F.; Yang, S.L.; Gu, J.X.; Xiong, Q.; Wang, Y. Study on microstructure and fatigue damage mechanism of 6082 Aluminum Alloy T-Type Metal Inert Gas (MIG)Welded Joint. Appl. Sci. 2018, 8, 1741. [Google Scholar] [CrossRef] [Green Version]
- Lu, N.C.; Wang, M.H.; Hao, G.D.; Wang, Y.T. Finite element analysis of residual welding stresses and deformation for a 5A06 aluminum alloy plate. Strength Mater. 2020, 52, 532–538. [Google Scholar] [CrossRef]
- Nie, L.; Wu, Y.X.; Gong, H. Numerical Investigation on the Stress Evolution of Welding Process in Aluminium Alloy 2219. Mechanika 2019, 25, 57–63. [Google Scholar] [CrossRef] [Green Version]
- Khosiroyan, A.; Darvazi, A.R. Effects of welding parameters and welding sequence on residual stress and distortion in Al6061-T6 aluminum alloy for T-shaped welded joint. Trans. Nonferrous Met. Soc. China 2020, 30, 76–89. [Google Scholar] [CrossRef]
- Choi, M.; Wu, C.B.; Kim, J.W. Numerical Optimization of the Welding Sequence for Mitigating Welding Deformation in Aluminum Pipe Structures by Using a Genetic Algorithm. Int. J. Precis. Eng. Manuf. 2020, 21, 2323–2333. [Google Scholar] [CrossRef]
- Zhao, Y.Q.; Zhan, X.H.; Chen, S.; Bai, M.Y.; Gong, X.R. Study on the shear performance and fracture mechanism of T-joints for 2219 aluminum alloy by dual laser-beam bilateral synchronous welding. J. Alloy. Compd. 2020, 847, 156511. [Google Scholar] [CrossRef]
- Fu, D.F.; Zhou, C.Q.; Li, C.; Wang, G.; Li, L.X. Effect of welding sequence on residual stress in thin-walled octagonal pipe−plate structure. Trans. Nonferrous Met. Soc. China 2014, 24, 657–664. [Google Scholar] [CrossRef]
- Rong, Y.; Xu, J.; Lei, T.; Huang, Y.; Shao, X.; Wang, C. Magnetism aided mitigation of deformation and residual stress in dissimilar joint 316L with EH36. J. Mater. Process. Technol. 2018, 259, 23–32. [Google Scholar] [CrossRef]
- Rong, Y.; Lei, T.; Xu, J.; Huang, Y.; Wang, C. Residual stress modelling in laser welding marine steel EH36 considering a thermodynamics-based solid phase transformation. Int. J. Mech. Sci. 2018, 146–147, 180–190. [Google Scholar] [CrossRef]
- Speleers, H. Algorithm 999: Computation of Multi-Degree B-Splines. ACM Trans. Math. Softw. 2019, 45, 43. [Google Scholar] [CrossRef] [Green Version]
- Chu, Q.L.; Bai, R.X.; Jian, H.G.; Lei, Z.K.; Hu, N.; Yan, C. Microstructure in laser welding of Al 6061 beam welded joints. Mater. Charact. 2018, 137, 269–276. [Google Scholar] [CrossRef]
- Kou, S.; Le, Y. Welding parameters and the grain structure of weld metal-A thermodynamic consideration. Metall. Trans. A 1988, 19A, 1075–1082. [Google Scholar] [CrossRef]
- Zhang, C.; Gao, M.; Jiang, M.; Zeng, X.Y. Effect of weld characteristic on mechanical strength of laser-arc hybrid-welded Al-Mg-Si-Mn aluminum alloy. Metall. Mater. Trans. A 2016, 47A, 5438–5449. [Google Scholar] [CrossRef]


| Al | Si | Mg | Cu | Fe | Mn | Zn | Ti |
|---|---|---|---|---|---|---|---|
| 97.63 | 0.561 | 0.986 | 0.31 | 0.289 | 0.052 | 0.024 | 0.018 |
| Temperature (°C) | Density (kg·m−3) | Heat Conductivity (W·m−1·°C−1) | Specific Heat Capacity (J·kg−1·°C−1) | Expansion Coefficient (10−6·°C−1) | Elastic Modulus (GPa) | Yield Stress (MPa) |
|---|---|---|---|---|---|---|
| 20.0 | 2700 | 162 | 945 | 23.45 | 68.54 | 274.4 |
| 93.3 | 2685 | 177 | 978 | 24.61 | 66.19 | 264.6 |
| 204.4 | 2657 | 192 | 1028 | 26.60 | 59.16 | 218.6 |
| 260.0 | 2657 | 201 | 1052 | 27.56 | 53.99 | 159.7 |
| 371.0 | 2630 | 217 | 1104 | 29.57 | 40.34 | 36.84 |
| 482.2 | 2602 | 226 | 1136 | 31.71 | 20.2 | 10.49 |
| NO. | Stress Direction | Symbol | Residual Stress (MPa) | |
|---|---|---|---|---|
| Min. | Max. | |||
| 1 | Longitudinal direction | Sx | −77.65 | 367.45 |
| 2 | Thickness direction | Sy | −62.45 | 86.11 |
| 3 | Transverse direction | Sz | −242.89 | 244.95 |
| 4 | Equivalent stress | Seqv | 3.96 | 278.24 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rong, Y.; Huang, Y.; Wang, L. Evolution Mechanism of Transient Strain and Residual Stress Distribution in Al 6061 Laser Welding. Crystals 2021, 11, 205. https://doi.org/10.3390/cryst11020205
Rong Y, Huang Y, Wang L. Evolution Mechanism of Transient Strain and Residual Stress Distribution in Al 6061 Laser Welding. Crystals. 2021; 11(2):205. https://doi.org/10.3390/cryst11020205
Chicago/Turabian StyleRong, Youmin, Yu Huang, and Lu Wang. 2021. "Evolution Mechanism of Transient Strain and Residual Stress Distribution in Al 6061 Laser Welding" Crystals 11, no. 2: 205. https://doi.org/10.3390/cryst11020205
APA StyleRong, Y., Huang, Y., & Wang, L. (2021). Evolution Mechanism of Transient Strain and Residual Stress Distribution in Al 6061 Laser Welding. Crystals, 11(2), 205. https://doi.org/10.3390/cryst11020205








