Enhanced THz Transmission by Bull’s Eye Structure Integrated with a Concentric Gold Hemisphere
Abstract
:1. Introduction
2. Materials and Methods
3. Results and Discussion
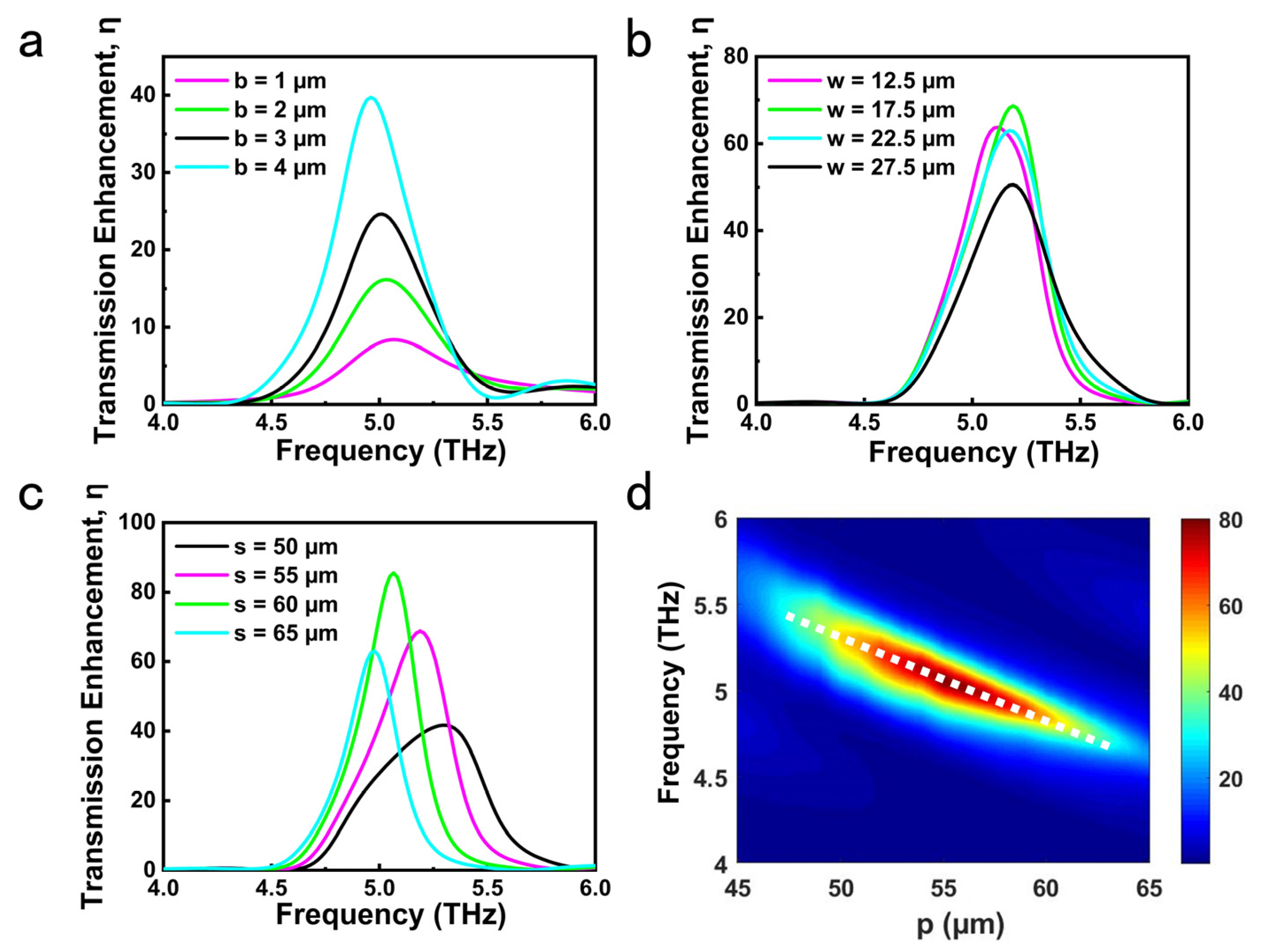
3.1. Groove Geometric Parameters
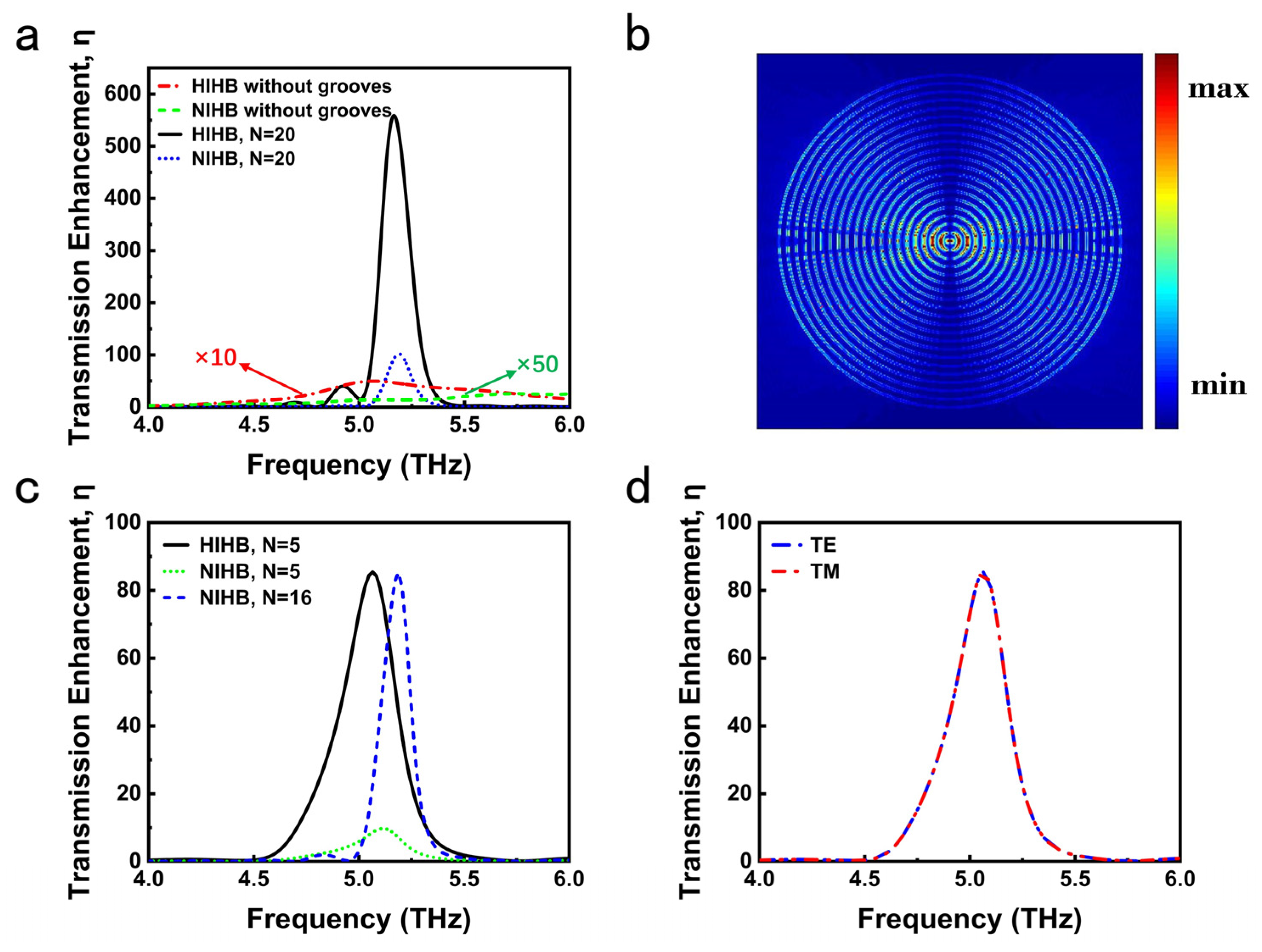
3.2. Enhanced THz Transmission and Its Polarization Independence
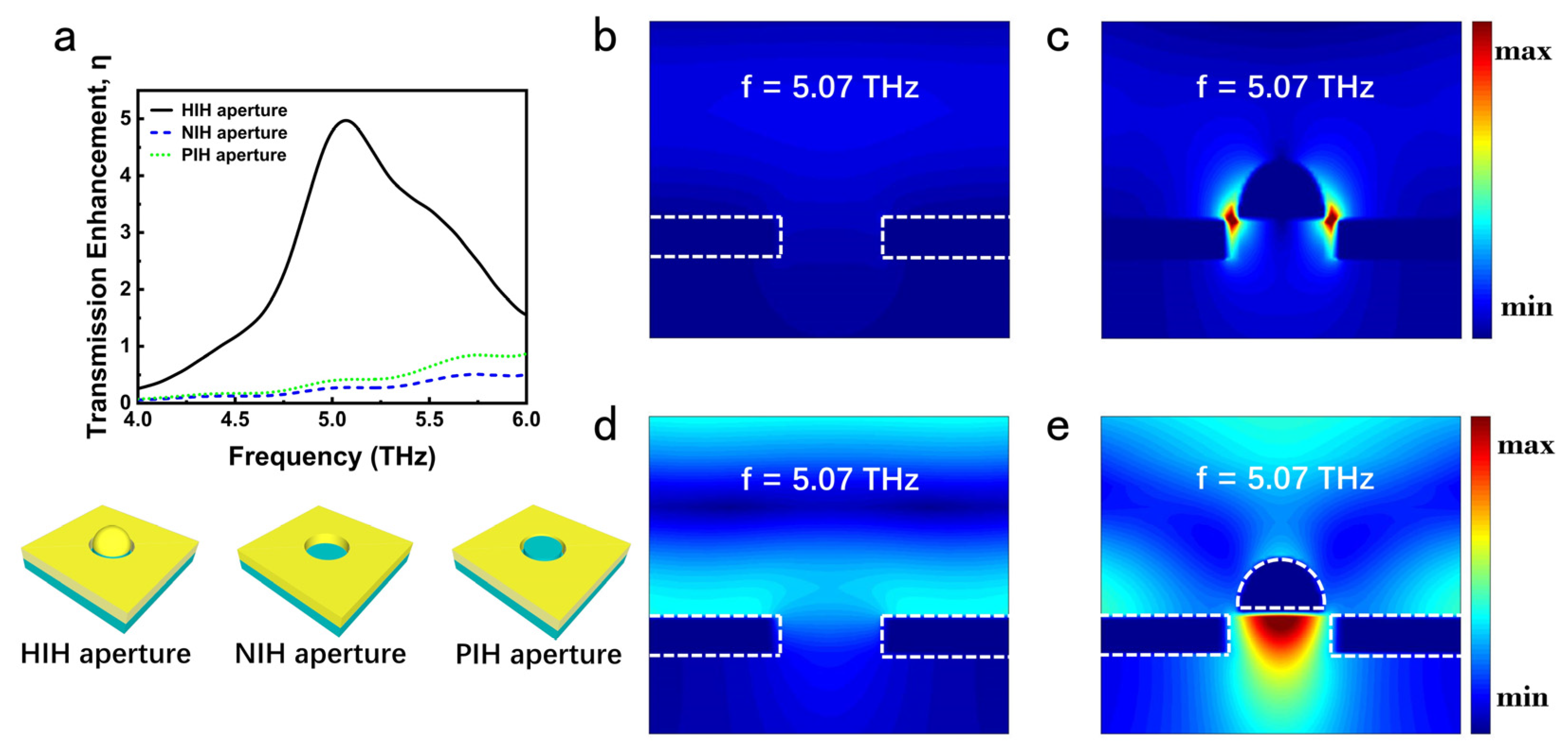
3.3. The Plasmon Resonances
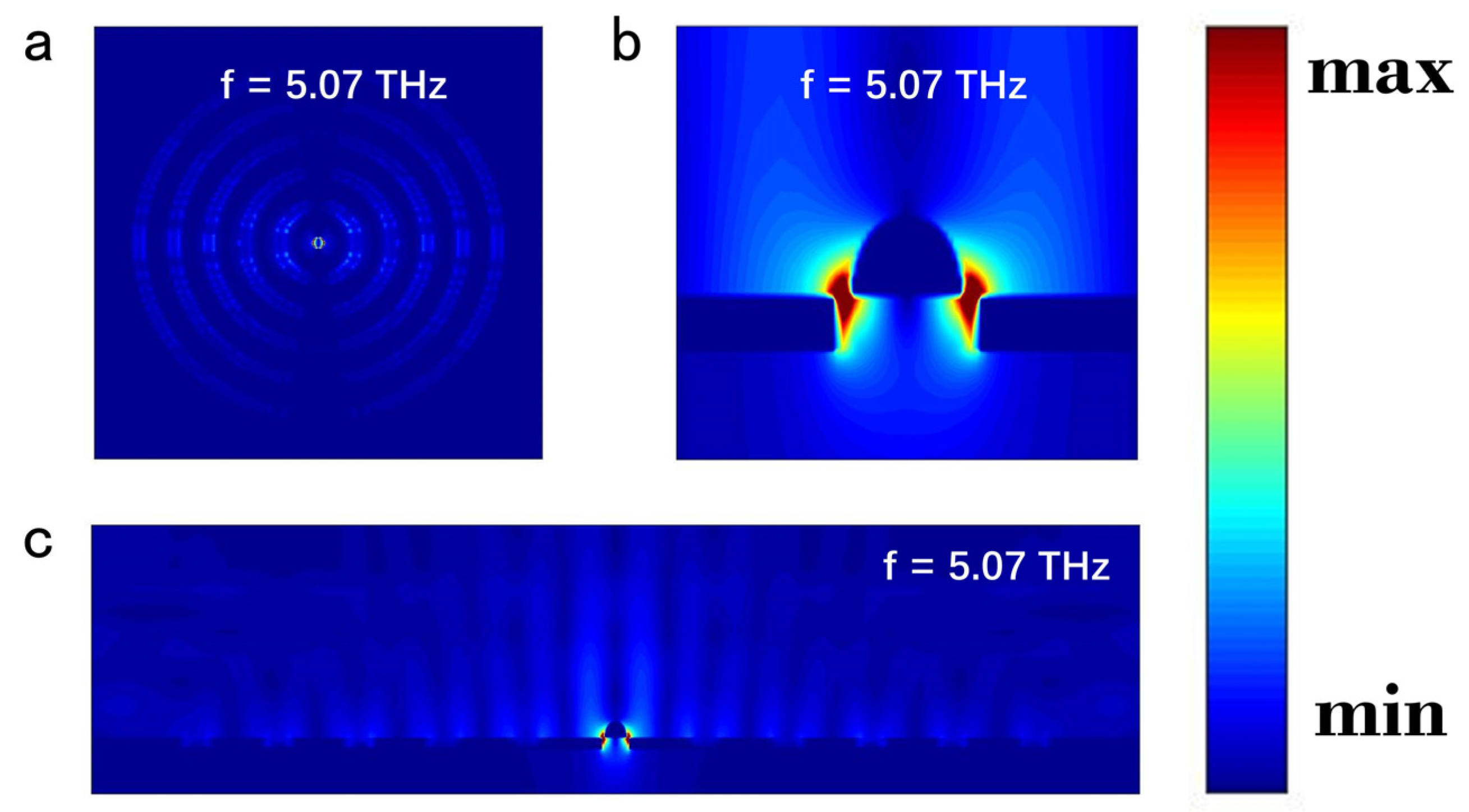
3.3.1. Localized Surface Plasmon Resonances
3.3.2. Coupling of SPPs and LSPRs
3.4. Comparison of HIHB and NIHB Structure with Varying N
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Arabi, H.E.; Joe, H.E.; Nazari, T.; Min, B.K.; Oh, K. A high throughput supra-wavelength plasmonic bull’s eye photon sorter spatially and spectrally multiplexed on silica optical fiber facet. Opt. Express 2013, 21, 28083–28094. [Google Scholar] [CrossRef] [PubMed]
- Agrawal, A.; Cao, H.; Nahata, A. Time-domain analysis of enhanced transmission through a single subwavelength aperture. Opt. Express 2005, 13, 3535–3542. [Google Scholar] [CrossRef] [PubMed]
- Wang, J.W.; Cheng, Y.; Liu, X.J. Manipulation of extraordinary acoustic transmission by a tunable bull’s eye structure. Chin. Phys. B 2014, 23, 054301. [Google Scholar] [CrossRef]
- Ko, H.; Kim, H.C.; Cheng, M. Light focusing at metallic annular slit structure coated with dielectric layers. Appl. Opt. 2010, 49, 950–954. [Google Scholar] [CrossRef]
- Carretero-Palacios, S.; Mahboub, O.; Garcia-Vidal, F.J.; Martin-Moreno, L.; Rodrigo, S.G.; Gene, C.; Ebbesen, T.W. Mechanisms for extraordinary optical transmission through bull’s eye structures. Opt. Express 2011, 19, 10429–10442. [Google Scholar] [CrossRef]
- Garcia-Vidal, F.J.; Lezec, H.J.; Ebbesen, T.W.; Martin-Moreno, L. Multiple Paths to Enhance Optical Transmission through a Single Subwavelength Slit. Phys. Rev. Lett. 2003, 90, 213901. [Google Scholar] [CrossRef]
- Ishi, T.; Fujikata, J.; Makita, K.; Baba, T.; Ohashi, K. Si Nano-Photodiode with a Surface Plasmon Antenna. Jpn. J. Appl. Phys. 2005, 44, L364–L366. [Google Scholar] [CrossRef]
- Ren, F.F.; Ang, K.W.; Ye, J.; Yu, M.; Lo, G.Q.; Kwong, D.L. Split Bull’s Eye Shaped Aluminum Antenna for Plasmon-Enhanced Nanometer Scale Germanium Photodetector. Nano Lett. 2011, 11, 1289–1293. [Google Scholar] [CrossRef]
- Okamoto, D.; Fujikata, J.; Ohashi, K. InGaAs Nano-Photodiode Enhanced Using Polarization-Insensitive Surface-Plasmon Antennas. Jpn. J. Appl. Phys. 2011, 50, 120201. [Google Scholar] [CrossRef]
- Chen, W.; Abeysinghe, D.C.; Nelson, R.L.; Zhan, Q. Plasmonic Lens Made of Multiple Concentric Metallic Rings under Radially Polarized Illumination. Nano Lett. 2009, 9, 4320–4325. [Google Scholar] [CrossRef] [Green Version]
- Aouani, H.; Mahboub, O.; Devaux, E.; Rigneault, H.; Ebbesen, T.W.; Wenger, J. Plasmonic Antennas for Directional Sorting of Fluorescence Emission. Nano Lett. 2011, 11, 2400–2406. [Google Scholar] [CrossRef] [PubMed]
- Aouani, H.; Mahboub, O.; Bonod, N.; Devaux, E.; Popov, E.; Rigneault, H.; Ebbesen, T.W.; Wenger, J. Bright Unidirectional Fluorescence Emission of Molecules in a Nanoaperture with Plasmonic Corrugations. Nano Lett. 2011, 11, 637–644. [Google Scholar] [CrossRef]
- Pournoury, M.; Arabi, H.E.; Oh, K. Strong polarization dependence in the optical transmission through a bull’s eye with an elliptical sub-wavelength aperture. Opt. Express 2012, 20, 26798–26805. [Google Scholar] [CrossRef] [PubMed]
- Ishihara, K.; Hatakoshi, G.; Ikari, T.; Minamide, H.; Ito, H.; Ohashi, K. Terahertz Wave Enhanced Transmission through a Single Subwavelength Aperture with Periodic Surface Structures. Jpn. J. Appl. Phys. 2005, 44, L1005–L1007. [Google Scholar] [CrossRef]
- Degiron, A.; Ebbesen, T.W. Analysis of the transmission process through single apertures surrounded by periodic corrugations. Opt. Express 2004, 12, 3694–3700. [Google Scholar] [CrossRef]
- Mahboub, O.; Palacios, C.S.; Genet, C.; Garcia-Vidal, F.J.; Rodrigo, S.G.; Martin-Moreno, L.; Ebbesen, T.W. Optimization of bull’s eye structures for transmission enhancement. Opt. Express 2010, 18, 11292–11299. [Google Scholar] [CrossRef]
- Fukai, R.; Nakagawa, T.; Kiyama, H.; Oiwa, A. Design of bull’s eye structures on gate-defined lateral quantum dots. Jpn. J. Appl. Phys. 2017, 56, 04CK04. [Google Scholar] [CrossRef]
- Yamada, A.; Terakawa, M. Reverse design of a bull’s eye structure based on the plasmonic far-field pattern. Opt. Express 2013, 21, 21273–21284. [Google Scholar] [CrossRef]
- Villate-Guio, F.; Lopez-Tejeira, F.; Garcia-Vidal, F.J.; Martin-Moreno, L.; Leon-Perez, F. Optimal light harvesting structures at optical and infrared frequencies. Opt. Express 2012, 20, 25441–25453. [Google Scholar] [CrossRef]
- Pournoury, M.; Joe, H.E.; Park, J.H.; Nazari, T.; Sung, Y.M.; Min, B.K.; Im, S.; Kim, D.; Oh, K. Polarization-dependent transmission through a bull’s eye with an elliptical aperture. Opt. Commun. 2014, 316, 1–4. [Google Scholar] [CrossRef]
- Ishihara, K.; Ohashi, K.; Ikari, T.; Minamide, H.; Yokoyama, H.; Shikata, J.; Ito, H. Polarization-dependent transmission through a bull’s eye with an elliptical aperture Terahertz-wave near-field imaging with subwavelength resolution using surfacewave-assisted bow-tie aperture. Appl. Phys. Lett. 2006, 89, 201120. [Google Scholar] [CrossRef]
- Xu, W.Z.; Ren, F.F.; Jevtics, D.; Hurtado, A.; Li, L.; Gao, Q.; Ye, J.; Wang, F.; Guilhabert, B.; Fu, L.; et al. Vertically emitting indium phosphide nanowire lasers. Nano Lett. 2018, 18, 3414–3420. [Google Scholar] [CrossRef] [PubMed]
- Wang, D.; Yang, T.; Crozier, K.B. Optical antennas integrated with concentric ring gratings: Electric field enhancement and directional radiation. Opt. Express 2011, 19, 2148–2157. [Google Scholar] [CrossRef]
- Hang, N.T.N.; Yang, Y.; Nam, N.Q.T.; Nogami, M.; Phuc, L.H.; Tri, N.H.; Cuu, H.V.; Long, N.V. Controlled Synthesis of Au Nanoparticles by Modified Polyol Methods: Determination of Their Size, Shape, and Crystal Structure. Crystals 2021, 11, 1297. [Google Scholar] [CrossRef]
- Sun, J.; Ding, Z.; Yu, Y.; Xie, C. A Theoretical Investigation about Photoswitching of Azobenzene Adsorbed on Ag Nanoparticles. Crystals 2022, 12, 248. [Google Scholar] [CrossRef]
- Zhong, T.; Zhang, H. Spoof surface plasmon polaritons excited leaky-wave antenna with continuous scanning range from endfire to forward. Chin. Phys. B 2020, 29, 094101. [Google Scholar] [CrossRef]
- Hu, X.; Liang, X.; Tang, L.; Liu, W. Enhanced Light Extraction Efficiency and Modulation Bandwidth of Deep-Ultraviolet Light-Emitting Diodes with Al Nanospheres. Crystals 2022, 12, 289. [Google Scholar] [CrossRef]
- Nazari, T.; Khazaeinezhad, R.; Kassani, S.H.; Jung, W.; Shin, I.; Kang, D.; Oh, K. Polarization dependent enhanced optical transmission through a sub-wavelength polygonal aperture surrounded by polygonal grooves. Opt. Express 2014, 22, 27476–27488. [Google Scholar] [CrossRef]
- Nazari, T.; Khazaeinezhad, R.; Jung, W.; Joo, B.; Kong, B.J.; Oh, K. Enhanced optical transmission through a star-shaped bull’s eye at dual resonant-bands in UV and the visible spectral range. Opt. Express 2015, 23, 18589–18601. [Google Scholar] [CrossRef]
- Yang, M.; Ren, F.F.; Pu, L.; Xiao, L.; Sheng, Y.; Wang, J.; Zheng, Y.; Shi, Y. Split Bull’s Eye Antenna for High-Speed Photodetector in the Range of Visible to Mid-Infrared. IEEE Photonics Technol. Lett. 2016, 28, 1177–1180. [Google Scholar] [CrossRef]
- Ghosh, R.R.; Dhawan, A. Extremely large near-field enhancements in the vicinity of plasmonic nanoantennas on top of bull’s eye structures exhibiting the extraordinary transmission of light. OSA Continuum 2021, 4, 193–211. [Google Scholar] [CrossRef]
- Kampouridou, D.; Feresidis, A. Broadband THz Corrugated Bull’s Eye Antennas. IEEE Access 2021, 9, 104460–104468. [Google Scholar] [CrossRef]
- Song, J.; Shi, Y.; Liu, X.; Li, M.; Wang, X.; Yang, F. Enhanced broadband extraordinary terahertz transmission through plasmon coupling between metal hemisphere and hole arrays. Opt. Mater. Express 2021, 11, 2700–2710. [Google Scholar] [CrossRef]
- Zhang, Q.; Hu, P.; Liu, C. Realization of enhanced light directional beaming via a Bull’s eye structure composited with circular disk and conical tip. Opt. Commun. 2015, 339, 216–221. [Google Scholar] [CrossRef]
- Ordal, M.A.; Bell, R.J.; Alexander, R.W.; Newquist, L.A.; Querry, M.R. Optical properties of Al, Fe, Ti, Ta, W, and Mo at submillimeter wavelengths. Appl. Opt. 1988, 26, 1203–1208. [Google Scholar] [CrossRef] [PubMed]
- Cui, Y.; He, Y.; Jin, Y.; Ding, F.; Yang, L.; Ye, Y.; Zhong, S.; Lin, Y.; He, S. Plasmonic and metamaterial structures as electromagnetic absorbers. Laser Photonics Rev. 2014, 8, 495–520. [Google Scholar] [CrossRef]
- Laux, E.; Genet, C.; Skauli, T.; Ebbesen, T.W. Plasmonic photon sorters for spectral and polarimetric imaging. Nat. Photonics 2008, 2, 161–164. [Google Scholar] [CrossRef]
- Lezec, H.J.; Degiron, A.; Devaux, E.; Linke, R.A.; Martin-Moreno, L.; Garcia-Vidal, F.J.; Ebbesen, T.W. Beaming Light from a Subwavelength Aperture. Science 2002, 297, 820–822. [Google Scholar] [CrossRef]
- Bhat, R.D.R.; Panoiu, N.C.; Brueck, S.R.J.; Osgood, R.M. Enhancing the signal-to-noise ratio of an infrared photodetector with a circular metal grating. Opt. Express 2008, 16, 4588–4596. [Google Scholar] [CrossRef]
- Xia, M.; Zhang, P.; Qiao, K.; Bai, Y.; Xie, Y.H. Coupling SPP with LSPR for Enhanced Field Confinement: A Simulation Study. J. Phys. Chem. C 2016, 120, 527–533. [Google Scholar] [CrossRef] [Green Version]
- Liu, Z.; Steele, J.M.; Lee, H.; Zhang, X. Tuning the focus of a plasmonic lens by the incident angle. Appl. Phys. Lett. 2006, 88, 171108. [Google Scholar] [CrossRef]
- Liu, Z.; Steele, J.M.; Srituravanich, W.; Pikus, Y.; Sun, C.; Zhang, X. Focusing Surface Plasmons with a Plasmonic Lens. Nano Lett. 2005, 5, 1726–1729. [Google Scholar] [CrossRef]
- Li, J.; Chen, S.; Yu, P.; Cheng, H.; Zhou, W.; Tian, J. Large enhancement and uniform distribution of optical near field through combining periodic bowtie nanoantenna with rectangular nanoaperture array. Opt. Lett. 2011, 36, 4014–4016. [Google Scholar] [CrossRef] [PubMed]
- Chen, Y.; Liu, X.; Chen, W.; Xie, Z.; Huang, Y.; Li, L. Effects of thickness & shape on localized surface plasmon resonance of sexfoil nanoparticles. Chin. Phys. B 2017, 26, 017807. [Google Scholar]
- Barnes, W.L.; Dereux, A.; Ebbesen, T.W. Surface plasmon subwavelength optics. Nature 2003, 424, 824–830. [Google Scholar] [CrossRef]
- Ren, F.F.; Xu, W.Z.; Ye, J.; Ang, K.W.; Lu, H.; Zhang, R.; Yu, M.; Lo, G.Q.; Tan, H.H.; Jagadish, C. Second-order surface-plasmon assisted responsivity enhancement in germanium nanophotodetectors with bull’s eye antennas. Opt. Express 2014, 22, 15949–15956. [Google Scholar] [CrossRef]
- Brunazzo, D.; Descrovi, E.; Martin, Q.J.F. Narrowband optical interactions in a plasmonic nanoparticle chain coupled to a metallic film. Opt. Lett. 2009, 34, 1405–1407. [Google Scholar] [CrossRef] [PubMed]
- Yu, Z.; Veronis, G.; Fan, S.; Brongersma, M.L. Design of midinfrared photodetectors enhanced by surface plasmons on grating structures. Appl. Phys. Lett. 2006, 89, 151116. [Google Scholar] [CrossRef]
- Janssen, O.T.A.; Urbach, H.P.; ’t Hooft, G.W. Giant Optical Transmission of a Subwavelength Slit Optimized Using the Magnetic Field Phase. Phys. Rev. Lett. 2007, 99, 043902. [Google Scholar] [CrossRef] [Green Version]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sun, M.; Liu, Y.; Shi, Y.; Zhang, Y.; Song, J.; Li, M.; Shi, S.; Zhang, S.; Wang, X.; Yang, F. Enhanced THz Transmission by Bull’s Eye Structure Integrated with a Concentric Gold Hemisphere. Crystals 2022, 12, 1210. https://doi.org/10.3390/cryst12091210
Sun M, Liu Y, Shi Y, Zhang Y, Song J, Li M, Shi S, Zhang S, Wang X, Yang F. Enhanced THz Transmission by Bull’s Eye Structure Integrated with a Concentric Gold Hemisphere. Crystals. 2022; 12(9):1210. https://doi.org/10.3390/cryst12091210
Chicago/Turabian StyleSun, Mingqi, Yueyang Liu, Yanpeng Shi, Yifei Zhang, Jinmei Song, Meiping Li, Shengnan Shi, Shan Zhang, Xiaodong Wang, and Fuhua Yang. 2022. "Enhanced THz Transmission by Bull’s Eye Structure Integrated with a Concentric Gold Hemisphere" Crystals 12, no. 9: 1210. https://doi.org/10.3390/cryst12091210
APA StyleSun, M., Liu, Y., Shi, Y., Zhang, Y., Song, J., Li, M., Shi, S., Zhang, S., Wang, X., & Yang, F. (2022). Enhanced THz Transmission by Bull’s Eye Structure Integrated with a Concentric Gold Hemisphere. Crystals, 12(9), 1210. https://doi.org/10.3390/cryst12091210







