Extending Ab Initio Phasing up to 2.2 Å Resolution: New Superposition Techniques
Abstract
:1. Introduction
2. Fourier Syntheses for Vector Superposition Techniques
2.1. The Ideal - and -Syntheses
2.2. The Observed Synthesis
2.3. The P(u) Patterson Synthesis
- (i)
- with intensity , or, roughly speaking, proportional to ;
- (ii)
- , for i ≠ j, with intensity
2.4. The FoFo-Synthesis
- (i)
- is the convolution of with itself, which causes the individual peaks to be broader than in a typically observed electron density map.
- (ii)
- If the quality of the model is sufficiently high (that is, if for a non-negligible percentage of observed reflections ), then peaks are expected to be located at for i, j = 1, 2, …, N, i.e., at the sum of positional vectors rather than at the interatomic vectors (as in the Patterson synthesis). Thus, the number of peaks in the unit cell is equal to N2, as in the Patterson map.
- (iii)
- The peak intensity is expected to be proportional to as in the Patterson synthesis, but the presence of modulates the contribution of each reflection to the right-hand side of Equation (7). In analogy with the appendix in Paper I the observed electron density at the target atomic positions is expected to be The larger the phase error, the weaker will the peaks be.
- (iv)
- and show the same rotational symmetry, but different translational symmetries. In particular, the space group symmetry elements of the target electron density transform according to the following rules: 21 → 2, (41, 43) → 42, 31 → 32, (61, 65) → 62, (62, 64) → 64. Glide planes a, b, c, n transform into the mirror plane m and glide planes d into n.
- (v)
- for reflections with symmetry restricted phase. Since , this subset of reflections is not subject to the disturbing effect of .
- (vi)
- If the quality of the model is sufficiently high, may be replaced by its mean value, and the -map can be computed.
2.5. The -Synthesis
- (i)
- is the convolution between and , and therefore peaks are broader than in a typical electron density map.
- (ii)
- The intensity of the peaks is modulated by the factor ; for sufficiently good models the maxima are expected at , for i = 1,…, N and j = 1, … Np, with intensity proportional to (approximately proportional to ). Their number is expected to be equal to NNp, usually much smaller than the peak number in the Patterson map; peaks’ superposition is therefore lower.
- (iii)
- A strong peak is expected at , corresponding to the cases for which ; its amplitude becomes larger and larger when the model improves. Thus, non-zero peaks correspond only to interatomic distances with ; they involve atoms which are not in the model.
- (iv)
- Equation (10) is not computable in practical cases unless is replaced by its expected value , but it becomes centric, so sharing the Patterson symmetry.
2.6. The -Synthesis
- (i)
- The map is the convolution between and the Patterson synthesis Therefore, its peaks will be broader compared to those in or .
- (ii)
- The maxima are at , for j, l = 1, …, N and p = 1, …, Np, with intensity proportional to . Therefore, peaks involving heavy atom positions will be dominant, in the order of peaks involving three, two and one heavy atom. The map is expected to contain NpN2 peaks, with large mutual overlap.
- (iii)
- The strongest peaks are expected to lie at , i = 1, …, Np, and each of them has multiplicity equal to N because j = l for N times. Less dominant peaks lie at , for i = 1, …, N, and each of them will have multiplicity equal to Np only if, for Np times, . Unfortunately, this condition is not frequent because the model atoms may be close to or far away from the target atoms. This last characteristic makes less appealing; indeed, the usefulness of maps dedicated to the target structure recovery may be estimated from the prominence of the peaks corresponding to target atoms. We will see that more useful maps may correspond to the -syntheses.
2.7. The -Synthesis
- (i)
- The map is the convolution between and the Patterson synthesis , thus single peaks will be broader than in or
- (ii)
- If the model is of sufficiently high quality the maxima are expected at , for i = 1, …, N and j, l = 1, …, Np, with intensity proportional to the product . However, if the model is poor, the factor may reduce the intensities of all peaks and shift them from the expected positions.
- (iii)
- Peaks involving heavy atom positions will be dominant (in the order of peaks involving three, two and one heavy atom). is expected to contain NNp2 peaks, with large mutual overlap, usually NNp2 << NpN2, so that overlap in the -synthesis is less severe than in .
- (iv)
- If the model is sufficiently good, large peaks are expected at for i = 1, …, N, with multiplicity of order Np (since l = j for Np times). Peaks are also expected at , j = 1, …, Np, with each having multiplicity equal to Np only if for Np times. Fortunately, this condition is not common because the model atoms may be close to or far away from the target atoms; consequently, an image of the target structure may be obtained in favorable cases.
- (v)
- Synthesis (12) is not practically computable, so in our calculations is replaced by its expected value .
2.8. The Difference Patterson Synthesis
3. Patterson Superposition Techniques
- (i)
- The symmetry minimum function (SMF) is calculated by combining symmetry-independent Harker domains according to
- (ii)
- A pivot peak in the SMF map is selected and used to calculate the minimum superposition function between the SMF and the translated Patterson map, according to
4. Vector Superposition Techniques
4.1. The Shifted FoFo-Synthesis
4.2. The Shifted -Synthesis
- (i)
- The noise peaks are located at ; their number is of order NNp, much smaller than the number of noise peaks (N2) in .
- (ii)
- The unknown phase error parameter on the right-hand side of Equation (18) is replaced by the most convenient parameter in Equation (19). Both parameters affect the image of the target structure resulting from the two syntheses, but the second one is more manageable.
- (iii)
- cannot lead to a high-quality image of the target structure, but the minimum function may help to reduce the number of noise peaks and consequently the mean phase error.
4.3. The Shifted -Synthesis
4.4. The Shifted -Synthesis
4.5. The Shifted Difference Patterson Synthesis
5. Discussion
- (a)
- Calculation of the G synthesis;
- (b)
- Calculation of the minimum function ;
- (c)
- Calculation of the map and its Fourier inversion, followed by a few EDM cycles.
- (i)
- The quality of the DSR phases is higher than that of the VST phases.
- (ii)
- For most of the test structures good sets of phases are obtained and FFOM (or RAT) is able to recognize them. That is particularly true for the three structures with RES > 2.05.
- (iii)
- The final average phase errors for 1crm and 1z1y remain high for all the syntheses. We do not know why these test structures resist the phasing procedure.
- (iv)
- None of the four columns in Table 2 provides a set of phases systematically better than the others; nevertheless, a minor effectiveness may be associated with the synthesis and a major effectiveness with the synthesis .
6. The CAB Application
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| is the observed structure factor of the target structure; is its phase value. | |
| structure factor of the current structure model. | |
| ideal electron density map, calculated via observed amplitudes and true phases | |
| electron density map of the current structure model, calculated by using amplitudes and estimated phases . Usually, it contains heavy atoms. | |
| symmetry operators of the space group. | |
| N | number of atoms in the target unit cell. |
| Np | number of atoms in the model unit cell. |
| NL | number of atoms in the substructure. |
| Zi | atomic number of the ith atom of the target structure. |
| Zpi | atomic number of the ith atom of the model structure. |
| , , | positional vector of the ith atom of the target structure, of the ith atom of the model structure and of the ith heavy atom, respectively. Frequently, a heavy atom is part of the model structure. |
| complex conjugates of F and Fp. | |
| normalized structure factors of F, Fp, respectively. | |
| the average is found per resolution shell. | |
| modified Bessel function of order i. | |
| , where . | |
| asu | asymmetric unit. |
| EDM | electron density modification. |
| PST | Patterson superposition technique. |
| VST | vector superposition technique. |
| * | convolution symbol. |
| Paper I [17] | https://doi.org/10.1107/S0021889806017894. |
| Paper II [16] | https://doi.org/10.3390/cryst10060538. |
References
- Wrinch, D.M. X. The Geometry of Discrete Vector Maps. Lond. Edinb. Dublin Philos. Mag. J. Sci. 1939, 27, 98–122. [Google Scholar] [CrossRef]
- Beevers, C.A.; Robertson, J.H. Interpretation of the Patterson Synthesis. Acta Cryst. 1950, 3, 164. [Google Scholar] [CrossRef]
- Buerger, M.J. Phase Determination with the Aid of Implication Theory. Phys. Rev. 1948, 73, 927–928. [Google Scholar] [CrossRef]
- Hauptman, H. A New Method in the Probabilistic Theory of the Structure Invariants. Acta Crystallogr. A Cryst. Phys. Diffr. Theor. Gen. Crystallogr. 1975, 31, 680–687. [Google Scholar] [CrossRef]
- Giacovazzo, C. Direct Methods in Crystallography; Academic Press: London, UK; New York, NY, USA, 1980; ISBN 978-0-12-282450-0. [Google Scholar]
- Giacovazzo, C. The Method of Representations of Structure Seminvariants. II. New Theoretical and Practical Aspects. Acta Crystalogr. A 1980, 36, 362–372. [Google Scholar] [CrossRef]
- Weeks, C.M.; DeTitta, G.T.; Hauptman, H.A.; Thuman, P.; Miller, R. Structure Solution by Minimal-Function Phase Refinement and Fourier Filtering. II. Implementation and Applications. Acta Crystallogr. A 1994, 50, 210–220. [Google Scholar] [CrossRef]
- Sheldrick, G.M. SHELX Applications to Macromolecules. In Direct Methods for Solving Macromolecular Structures; Fortier, S., Ed.; Springer: Dordrecht, The Netherlands, 1998; pp. 401–411. ISBN 978-94-015-9093-8. [Google Scholar]
- Foadi, J.; Woolfson, M.M.; Dodson, E.J.; Wilson, K.S.; Jia-xing, Y.; Chao-de, Z. A Flexible and Efficient Procedure for the Solution and Phase Refinement of Protein Structures. Acta Crystallogr. D 2000, 56, 1137–1147. [Google Scholar] [CrossRef] [Green Version]
- Burla, M.C.; Carrozzini, B.; Cascarano, G.L.; Giacovazzo, C.; Polidori, G. More Power for Direct Methods: SIR2002. Z. Krist. Cryst. Mater. 2002, 217, 629–635. [Google Scholar] [CrossRef]
- Nordman, C.E. Vector Space Search and Refinement Procedures. Trans. Am. Crystallogr. Assoc. 1966, 2, 29–38. [Google Scholar]
- Richardson, J.W.; Jacobson, R.A. Patterson and Pattersons: Fifty Years of the Patterson Function: Proceedings of a Symposium Held at the Institute for Cancer Research, the Fox Chase Cancer Center, Philadelphia, PA, USA, 13–15 November 1984; Glusker, J.P., Patterson, B.K., Rossi, M., Eds.; International Union of Crystallography Crystallographic Symposia; International Union of Crystallography; Oxford University Press: New York, NY, USA, 1987; ISBN 978-0-19-855230-7. [Google Scholar]
- Sheldrick, G.M. Tutorial on Automated Patterson Interpretation to Find Heavy Atoms. In Crystallographic Computing 5: From Chemistry to Biology; Moras, D., Podjarny, A.D., Thierry, C., Eds.; IUCr Crystallographic Symposia; International Union of Crystallography; Oxford University Press: New York, NY, USA, 1991; pp. 145–157. ISBN 978-0-19-855384-7. [Google Scholar]
- Pavelčík, F.; Kuchta, L.; Sivý, J. Patterson-Oriented Automatic Structure Determination. Utilizing Patterson Peaks. Acta Crystallogr. A 1992, 48, 791–796. [Google Scholar] [CrossRef]
- Burla, M.C.; Caliandro, R.; Carrozzini, B.; Cascarano, G.L.; De Caro, L.; Giacovazzo, C.; Polidori, G. Ab Initio Protein Phasing: The Patterson Deconvolution Method in SIR2002. J. Appl. Crystallogr. 2004, 37, 258–264. [Google Scholar] [CrossRef] [Green Version]
- Burla, M.C.; Carrozzini, B.; Cascarano, G.L.; Giacovazzo, C.; Polidori, G. Properties of Fourier Syntheses and New Syntheses. Crystals 2020, 10, 538. [Google Scholar] [CrossRef]
- Burla, M.C.; Caliandro, R.; Carrozzini, B.; Cascarano, G.L.; De Caro, L.; Giacovazzo, C.; Polidori, G.; Siliqi, D. The Revenge of the Patterson Methods. I. Protein Ab Initio Phasing. J. Appl. Crystallogr. 2006, 39, 527–535. [Google Scholar] [CrossRef]
- Carrozzini, B.; Cascarano, G.L.; Giacovazzo, C. The Cross-Correlation Function: Main Properties and First Applications. J. Appl. Crystallogr. 2010, 43, 221–226. [Google Scholar] [CrossRef]
- Caliandro, R.; Carrozzini, B.; Cascarano, G.L.; Comunale, G.; Giacovazzo, C. On the Use of the C Map in Patterson Deconvolution Procedures. Acta Crystallogr. A Found. Crystallogr. 2013, 69, 98–107. [Google Scholar] [CrossRef]
- Caliandro, R.; Carrozzini, B.; Cascarano, G.L.; Comunale, G.; Giacovazzo, C.; Mazzone, A. Protein Phasing at Non-Atomic Resolution by Combining Patterson and VLD Techniques. Acta Crystallogr. D Biol. Crystallogr. 2014, 70, 1994–2006. [Google Scholar] [CrossRef]
- Cowtan, K. Error Estimation and Bias Correction in Phase-Improvement Calculations. Acta Crystallogr. D Biol. Crystallogr. 1999, 55, 1555–1567. [Google Scholar] [CrossRef] [Green Version]
- Burla, M.C.; Giacovazzo, C.; Polidori, G. From a Random to the Correct Structure: The VLD Algorithm. J. Appl. Crystallogr. 2010, 43, 825–836. [Google Scholar] [CrossRef]
- Burla, M.C.; Caliandro, R.; Giacovazzo, C.; Polidori, G. The Difference Electron Density: A Probabilistic Reformulation. Acta Crystallogr. A Found. Crystallogr. 2010, 66, 347–361. [Google Scholar] [CrossRef]
- Burla, M.C.; Carrozzini, B.; Cascarano, G.L.; Giacovazzo, C.; Polidori, G. Cyclic Automated Model Building (CAB) Applied to Nucleic Acids. Crystals 2020, 10, 280. [Google Scholar] [CrossRef] [Green Version]
- Cascarano, G.L.; Giacovazzo, C. Towards the Automatic Crystal Structure Solution of Nucleic Acids: Automated Model Building Using the New CAB Program. Acta Crystallogr. D Struct. Biol. 2021, 77, 1602–1613. [Google Scholar] [CrossRef] [PubMed]
- Cowtan, K. The Buccaneer Software for Automated Model Building. 1. Tracing Protein Chains. Acta Crystallogr. D 2006, 62, 1002–1011. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Cowtan, K. Automated Nucleic Acid Chain Tracing in Real Time. IUCrJ 2014, 1, 387–392. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Carrozzini, B.; Cascarano, G.L.; Giacovazzo, C. The Automatic Solution of Macromolecular Crystal Structures via Molecular Replacement Techniques: REMO22 and Its Pipeline. Int. J. Mol. Sci. 2023, 24, 6070. [Google Scholar] [CrossRef]
- Cascarano, G.L.; Cuocci, C.; Mallamo, M.; Carrozzini, B.; Moliterni, A. JAV (Just Another Viewer)—Unpublished Software. 2021. [Google Scholar]
- Burling, F.T.; Weis, W.I.; Flaherty, K.M.; Brünger, A.T. Direct Observation of Protein Solvation and Discrete Disorder with Experimental Crystallographic Phases. Science 1996, 271, 72–77. [Google Scholar] [CrossRef] [Green Version]
- Voegtli, W.C.; Sommerhalter, M.; Saleh, L.; Baldwin, J.; Bollinger, J.M.; Rosenzweig, A.C. Variable Coordination Geometries at the Diiron(II) Active Site of Ribonucleotide Reductase R2. J. Am. Chem. Soc. 2003, 125, 15822–15830. [Google Scholar] [CrossRef]
- Högbom, M.; Andersson, M.E.; Nordlund, P. Crystal Structures of Oxidized Dinuclear Manganese Centres in Mn-Substituted Class I Ribonucleotide Reductase from Escherichia Coli: Carboxylate Shifts with Implications for O2 Activation and Radical Generation. J. Biol. Inorg. Chem. 2001, 6, 315–323. [Google Scholar] [CrossRef]
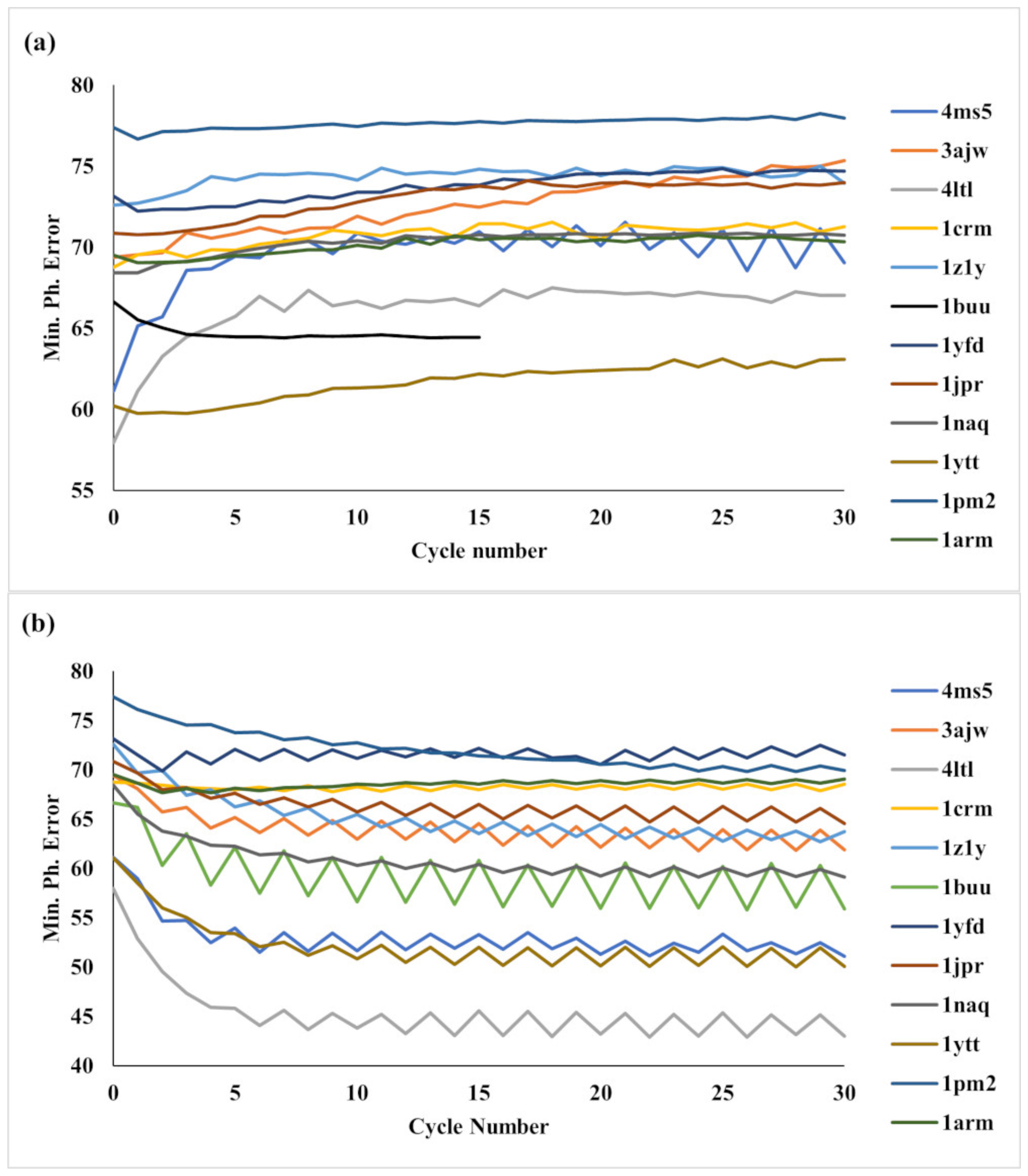

| Synthesis | VH | Exp. Signal | Exp. Sig. Ampl. | Noise Peaks |
|---|---|---|---|---|
| P | ri, for i = 1, …, N | |||
| ri, for i = 1, …, N | ||||
| ri, for i = 1, …, N | ||||
| ri, for i = 1, …, N | ||||
| ri, for i = 1, …, N | ||||
| ri, for i = 1, …, N rpi, for i = 1, …, Np |
| PDB | RES | ||||||
|---|---|---|---|---|---|---|---|
| 4ms5 | 2.23 | 59.92 | 69.05 | 51.77 | 51.08 | 56.21 | 55.48 |
| 3ajw | 2.10 | 69.40 | 75.35 | 60.71 | 61.91 | 60.92 | 61.18 |
| 4ltl | 2.07 | 57.35 | 67.05 | 43.28 | 42.99 | 52.35 | 49.88 |
| 1crm | 2.02 | 68.77 | 71.28 | 68.15 | 68.53 | 73.44 | 73.57 |
| 1z1y | 2.00 | 72.59 | 73.95 | 63.11 | 63.73 | 74.88 | 73.62 |
| 1buu | 1.93 | 66.64 | 64.45 | 56.73 | 55.93 | 48.46 | 47.70 |
| 1yfd | 1.90 | 73.17 | 74.70 | 71.99 | 71.54 | 70.01 | 69.73 |
| 1jpr | 1.89 | 70.87 | 74.00 | 64.67 | 64.58 | 65.73 | 65.59 |
| 1naq | 1.86 | 68.44 | 70.75 | 59.33 | 59.12 | 68.85 | 69.02 |
| 1ytt | 1.80 | 61.06 | 63.08 | 50.21 | 50.07 | 52.37 | 53.01 |
| 1pm2 | 1.80 | 77.40 | 77.96 | 69.93 | 69.94 | 78.81 | 78.53 |
| 1arm | 1.80 | 69.42 | 70.34 | 68.34 | 69.08 | 74.33 | 70.99 |
| PDB | ||||
|---|---|---|---|---|
| 4ms5 | 50 (2.93) | 49 (2.92) | 48 (2.98) | 49 (2.99) |
| 3ajw | 49 (3.08) | 49 (3.11) | 49 (3.23) | 49 (3.20) |
| 4ltl | 42 (3.01) | 42 (3.16) | 42 (3.21) | 41 (3.17) |
| 1crm | 71 (2.29) | 69 (2.31) | 89 (2.15) | 70 (2.23) |
| 1z1y | 69 (2.28) | 68 (2.43) | 76 (2.29) | 89 (2.24) |
| 1buu | 31 (4.85) | 32 (3.95) | 32 (3.78) | 30 (4.87) |
| 1yfd | 52 (3.12) | 52 (3.01) | 89 (2.93) | 50 (3.03) |
| 1jpr | 47 (2.99) | 47 (3.13) | 51 (2.92) | 46 (3.22) |
| 1naq | 51 (2.26) | 51 (2.10) | 51 (2.14) | 51 (2.13) |
| 1ytt | 43 (3.08) | 43 (2.91) | 43 (3.08) | 43 (3.09) |
| 1pm2 | 48 (2.93) | 48 (3.01) | 47 (2.91) | 48 (2.96) |
| 1arm | 88 (2.26) | 58 (2.49) | 89 (2.42) | 88 (2.32) |
| PDB | PIV | MA | |
|---|---|---|---|
| 3ajw | 1 | 36 | 0.63 |
| 1buu | 1 | 30 | 0.87 |
| 1jpr | 1 | 35 | 0.89 |
| 1ytt | 1 | 55 | 0.60 |
| 1pm2 | 1 | 33 | 0.89 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Burla, M.C.; Carrozzini, B.; Cascarano, G.L.; Giacovazzo, C.; Polidori, G. Extending Ab Initio Phasing up to 2.2 Å Resolution: New Superposition Techniques. Crystals 2023, 13, 874. https://doi.org/10.3390/cryst13060874
Burla MC, Carrozzini B, Cascarano GL, Giacovazzo C, Polidori G. Extending Ab Initio Phasing up to 2.2 Å Resolution: New Superposition Techniques. Crystals. 2023; 13(6):874. https://doi.org/10.3390/cryst13060874
Chicago/Turabian StyleBurla, Maria Cristina, Benedetta Carrozzini, Giovanni Luca Cascarano, Carmelo Giacovazzo, and Giampiero Polidori. 2023. "Extending Ab Initio Phasing up to 2.2 Å Resolution: New Superposition Techniques" Crystals 13, no. 6: 874. https://doi.org/10.3390/cryst13060874
APA StyleBurla, M. C., Carrozzini, B., Cascarano, G. L., Giacovazzo, C., & Polidori, G. (2023). Extending Ab Initio Phasing up to 2.2 Å Resolution: New Superposition Techniques. Crystals, 13(6), 874. https://doi.org/10.3390/cryst13060874








