1. Introduction
Many macromolecules with linear chemical architecture are neither perfectly flexible nor entirely rigid-rod-like chain molecules, but exhibit instead only local stiffness and are called semiflexible. Within a coarse-grained description, such a polymer chain is modeled as a curve in continuous space
, with
s a coordinate along the backbone of the macromolecule. The stiffness is due to a nonzero bending modulus
, which is proportional to the persistence length
, describing the length along the contour over which the orientations of subsequent bonds (or tangent vectors along
, respectively) are correlated [
1,
2,
3]. This Kratky–Porod model [
3] (also-called Wormlike Chain (WLC) model) is widely accepted as a proper phenomenological description of semiflexible polymers, in particular, of biopolymers such as the double-stranded (ds) DNA [
4], filamentous (F)-actin [
5], etc.
When one deals with the liquid–crystalline order of semiflexible polymers in lyotropic solution [
6,
7], it is clear that a description in terms of two lengths only, the contour length
L of the WLC and its persistence length,
, does not suffice: the effective chain diameter
D controls the interchain repulsion and hence the possible onset of nematic order (see, e.g., [
8,
9,
10,
11]). However, for single semiflexible chains in
dimensions (as they matter in dilute solutions), the excluded volume interactions due to nonzero
D do not matter for large
, provided
L is much smaller than
[
12,
13]. For this reason, the WLC model is broadly accepted as kind of a “gold standard” as far as the statistical mechanics of semiflexible chains is concerned.
Care is needed, however, when one deals with the adsorption of semiflexible polymers on planar surfaces [
14,
15,
16,
17,
18,
19,
20]. When a polymer gets adsorbed, its conformation changes from three-dimensional to (quasi) two-dimensional [
21,
22,
23]. Now, the WLC model implies that a polymer, which in
exhibits a decay of the tangent–tangent correlation function with persistence length
, would exhibit in
a corresponding decay with
. In fact, adsorbed polymers on planar substrates are not at all strictly confined into a two-dimensional plane, one rather expects loops and tails. For flexible polymers, the structure of the adsorbed “pancake“ is rather a two-dimensional self-avoiding walk (SAW) of more or less spherical ”blobs“ of radius
r attached to the surface whereby
. Here,
is a variable denoting the relative distance from the adsorption transition point, using the temperature or the strength of the adsorption potential as control variable. The exponent
is the Flory exponent [
23] characterizing the size of a flexible polymer in bulk three-dimensional solution under good solvent conditions, and
[
24] is the so-called
crossover exponent [
22,
25]. For Gaussian chains (e.g., appropriate for
-solvents [
8,
9]), the corresponding exponents are
[
8,
9], and
[
21,
22,
23,
24,
25]. Thus,
r exceeds the diameter
D of the effective monomers for weak adsorption (
) while
r is of the same order as
D for the case of strong adsorption (
). Only for the latter case can an orientation of the bond vectors between subsequent effective monomers predominantly parallel to the adsorbing substrate be anticipated.
For semiflexible polymers with
, the regime of weak adsorption is predicted to be much narrower [
15],
, where
is the range of the adsorption potential (we assume for simplicity an adsorption potential of short range
of the same order as
D). Note that, for adsorbed semiflexible polymers, long loops for
are expected to be very rare, but if they occur they must bend away from the substrate over distances larger than
[
15]. However, short wormlike loops, which are nearly parallel to the surface, are still expected in the regime of strong adsorption, their length being gradually suppressed with increasing
. The fraction of monomers
f within the potential range
is, however, predicted [
15] to be close to unity already when
exceeds
.
These predictions imply that an adsorbed semiflexible polymer is not identical to a strictly two-dimensional chain confined in a plane parallel to the adsorbing surface. While for a two-dimensional semiflexible polymer the doubling of the decay length (
) of orientational correlations can be understood simply from the fact that in
there is a single direction orthogonal to the tangent vector of a chain, in
, there are two orthogonal directions. Since, as emphasized above, adsorbed chains are not strictly two-dimensional, it is questionable what their decay length
of orientational correlations actually is. Therefore, even for
L of the same order as
, their lateral chain dimensions cannot be straightforwardly predicted from the WLC model [
26,
27]. For
, an additional problem arises that excluded volume matters since in
chain intersection is strictly forbidden, and the crossover to
SAW behavior (mean square end-to-end distance
) [
28,
29,
30] sets in when
L exceeds
. These problems are potentially relevant for experiments such as atomic force microscopy (AFM) studies of DNA fragments adsorbed on various substrates; see, e.g., [
31,
32,
33,
34,
35,
36,
37].
The existing theory [
15,
16] refers mostly to the limit
, and, being based on the WLC model, ignores excluded volume completely. In the present work, we wish to elucidate further both the effects of excluded volume and of finite chain length on the properties of adsorbed semiflexible chains, carrying out Molecular Dynamics (MD) simulations of a coarse-grained model. We have used that model in previous work [
26,
27] to estimate how the adsorption transition of semiflexible polymers depends on their stiffness. While our previous work focused on estimating the critical strength
of the potential of the adsorbing wall where adsorption occurs, and the crossover of the decay length
from
to
upon chain adsorption, we now focus on the properties of the adsorbed polymers for wall potentials chosen such that
(note that
). We expect that understanding the properties of such more or less strongly adsorbed semiflexible polymers should be useful for the interpretation of pertinent experiments.
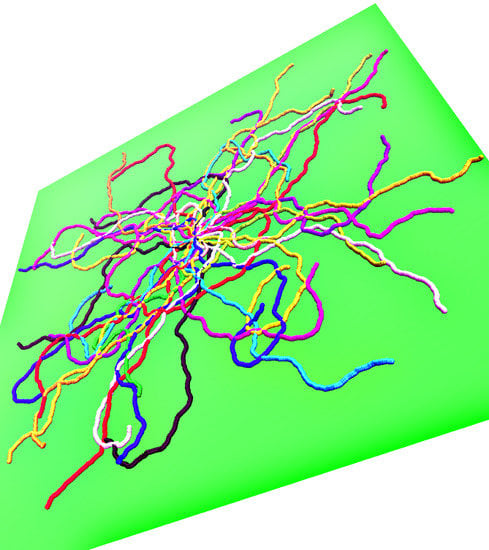
In
Figure 1, we illustrate the above introductory discussion by snapshots for weakly adsorbed (left) or more strongly adsorbed (right) semiflexible polymers, resulting from our simulations. Note that in each figure 50 independent conformations are superimposed such that the grafting site for all chains is identical (since a single chain configuration needs not be typical, a whole ensemble of independent chains is shown in both cases). The adsorbing planar surface is shown in green, and monomers with
z coordinate within the range of the adsorption potential are colored in blue while monomers in loops and tails (i.e., monomers outside the range) are displayed in yellow. Note that for the case of weak adsorption most of the monomers are in flat loops, i.e., the perpendicular extension of the loops from the wall amounts to a few monomer diameters only. In the case of strong adsorption, even if the majority of the monomers reside within the potential range, the polymer conformation still contains many small loops in a rather random succession along the chain.
In
Section 2, we briefly summarize the model, simulation method, and describe the properties that will be analyzed.
Section 3 describes our numerical results for the properties of adsorbed chains and discusses them, comparing to pertinent theoretical predictions and related simulation work when appropriate.
Section 4 summarizes our findings for the distribution functions of the lengths of loops, trains and tails.
Section 5 contains our conclusions.
2. Simulated Model
Given the fact that systems of interest such as ds-DNA involve mesoscopic length scales (where the persistence length
is accepted to be 50 nm, meaning that about 150 base pairs correspond to one persistence length), a simulation of a chemically realistic model (with water and added salt as a solvent) is extremely difficult. As in our previous related work [
26,
27], we use a coarse-grained model of bead-spring type. The diameter
of the beads is chosen to represent the effective diameter of the real wormlike polymer, e.g.,
nm in the case of (ds)-DNA. Excluded volume between the beads is described by a truncated and shifted Lennard–Jones potential,
while for larger distances between the beads the effective potential is zero,
. This choice represents good solvent conditions (solvent molecules are not considered explicitly). The strength
of the potential is used as unit of energy,
(and temperature
as well), while henceforth
is chosen as unit of length,
. Chain connectivity is obtained via the finitely extensible nonlinear elastic (FENE) potential between subsequent beads along the chain,
with
Choosing the parameters as [
38]
,
has the effect that the bond length
, i.e., this does not introduce a new length scale in the problem. Other models [
39] yield
much smaller than
and such strongly overlapping spheres may be a bit closer to reality for some semiflexible polymers but simulating stiff polymers with
using such a model would be very difficult.
Stiffness is introduced by means of a bending potential,
where
is the angle that the bond vector
forms with the preceding bond vector
, (
being the positions of the subsequent beads). This model is a discrete counterpart of the KP model of WLCs where the coordinate
s along the contour is a continuous variable while here only discrete positions
are possible. Note that for large
one has
so locally only small
occur. The present model differs substantially from the KP model by inclusion of excluded volume (EV), but a great advantage of MD is that EV interactions (apart from nearest neighbors along the chain) can be kept or switched off to obtain a direct test for their effect. In this model, the contour length of a chain containing
N beads is simply
while the effective persistence length,
, characterizing the initial decay of the bond orientational correlation function, is conveniently estimated from
Equations (
3) and (
4) yield directly [
26,
27] for large
when
Equation (
5) is compatible with the KP model, irrespective of whether EV is included or not.
The adsorbing substrate is approximated by a structureless rigid wall at the plane
at which the potential acts
where the constant
so that the minimum at
has the depth
, cf.
Figure 2a. The potential in Equation (
6), the so-called “Mie potential”, can be thought of resulting from integrating a 12–6 Lennard-Jones potential over all the atoms of an (infinite) two-dimensional plane. The resulting attraction, decaying proportional to
, hence, is realistic when polymers are adsorbed on quasi-twodimensional membranes, graphene sheets, etc., or when a surface of a three-dimensional substrate is coated with a suitable surfactant monolayer to control the wettability conditions [
40].
We show in
Figure 2a the corresponding distributions of the monomer density for a typical case, for which the adsorption transition is predicted to occur at [
26,
27]
. Choosing
as our control parameter, this means that
Figure 2a includes both ‘mushrooms’, just before the onset of adsorption (
), chains in the transition regime (
), and strongly adsorbed chains (
). As far as in all simulations the first monomer of a chain is “anchored” at the surface, we choose
. In addition, for the mushrooms, there occurs an enhanced monomer density near the surface while a tail of
extends to large
z. For strongly adsorbed chains, however, almost all monomers reside in the range
. Monomers are seldom found in the region
where the wall potential is repulsive.
Apparently, the position of the minimum of
does not coincide with the position of the maximum of the monomer density distribution. Actually, most of the monomers are localized further away from the wall, even if the whole chain is strongly bound to the wall. This fact becomes evident when we examine the distribution of the center of mass position of the chain,
Figure 2b. It is, therefore, of interest how the center of mass position,
, and its distribution function,
, depend on the parameters
, and
,
Figure 2b. Evidently,
decreases with increasing
only rather slowly and shorter chains such as
are rather loosely bound to the attractive surface, compared to the longer ones. While
Figure 2b refers to
as far as in the framework of our coarse-grained model this case might mimic ds-DNA, it is clear that for the rigid rod limit and large
N would only be slightly enhanced beyond
(see
Appendix A). Such long rods would undergo almost harmonic fluctuations of their position around
as well as accompanying very small fluctuations of their orientation. Apparently, semiflexible polymers behave very differently from hard rods in this respect: the latter have a
, which resembles a Gaussian, centered at
with a width scaling as
.
It is convenient to define a range
of the adsorption potential from the condition that
, which yields
. This parameter allows for making comparisons with analytical work [
15,
16], where as adsorption potential a square well potential of depth
u and range
is used. Since our choice of
is somewhat arbitrary, we define an adsorbed monomer fraction as
rather than defining
f from the monomers within the range of
[
26,
27]. A similar model as Equations (
1)–(
6) has been used in early work [
41] but only rather short chains were accessible.
While
f can be taken as order parameter of the adsorption transition, another interesting characteristic is the orientation of the bond vectors
relative to the surface normal,
with
being the the angle between a bond vector and the
z-axis (the average
includes average over all
bond vectors of the chain). In addition to
, the mean square gyration radius
of the polymers is also analyzed, distinguishing perpendicular,
, and parallel
components. We note that such long wave length properties of the chains relax much more slowly than the local properties
, and hence it is much more difficult to both equilibrate them and obtain meaningful statistical accuracy via MD simulations [
26]. We have performed multiple runs for every parameter combination,
, using the HOOMD-Blue software [
42,
43] on graphics processing units (GPUs). For each run,
chains are studied in parallel, choosing a MD time step
with
(whereby the monomer mass
). The length of each run was at least
and typically
runs were carried out for
. As discussed in more detail in [
26], the rapid increase of relaxation times with chain length limits the accessible range to
.
3. Numerical Results for the Properties of Adsorbed Chains
The methods used to identify the critical value
as a function of
have already been discussed in [
26,
27]. For the sake of completeness, in
Figure 3 we add here an example not shown previously. When the polymer chain is in the non-adsorbed mushroom state, all components
with
are of the same order whereas in the adsorbed state
should converge to a finite value as
while
, recalling that in
excluded volume also matters for semiflexible chains [
28,
29,
30] and the Flory exponents is
[
1].
Thus, the ratio
for large
N converges to a constant (which would be
, if all
were equal) for
being less than the critical value
, whereas this ratio converges to zero for
. Scaling theory [
22,
25] predicts that for large enough
N right at
the ratios
cross at a universal crossing point which has been estimated (for SAWs [
24]) to be around
. For our model and the available chain lengths, the crossing occurs,
Figure 3a, near
for
, while for smaller
N the crossings are shifted to smaller values. In addition, the ordinate of the crossing occurs near
rather than near the theoretical value. We interpret these problems as being due to strong corrections to scaling. In fact, near
, the chain conformations are still of mostly three-dimensional character, and, therefore, given this choice of stiffness, excluded volume matters for very long chains only [
12,
13] as discussed in the Introduction.
An alternative method to search for
is the study of the variation of
f with
N [
25]. In the mushroom regime, only few monomers are close to the surface, and hence
while in the regime of strong adsorption
f should converge to nonzero values close to unity. Right at
, a power law decay,
, is predicted [
22,
23,
24,
25], with
for Gaussian chains while
in the presence of excluded volume [
24]. In the example shown in
Figure 3b, one sees that for chains that are clearly non-adsorbed (such as for
),
is verified, but only for large
N. For chains that are clearly adsorbed (such as for
), one observes undoubtedly a convergence to a nonzero value for
. For intermediate values of
, there is a curvature in the log-log plot, and with decreasing
the sign of the curvature changes, suggesting that for
a nonzero extrapolation is reached, while for
ultimately
should result (although
would be needed to see this).
Much larger values of
N would be required to estimate
more precisely, too difficult to access with MD, while Monte Carlo studies where Equation (
3) is used for SAWs on the simple cubic lattice allow the choice of
N up to 25,000 [
44]. However, the fact that only a discrete bond angle
is possible on this lattice eliminates all bending fluctuations under small angles that are clearly important for polymers such as DNA, and are captured by the present model. For the lattice model, trains would simply be straight lines (typically of length
) in the lattice plane adjacent to the surface and hence have no entropic contributions due to bending. In contrast, the present model exhibits bending fluctuations on small scales also in
z-direction perpendicular to surface,
Figure 4a–c. We see that
varies monotonically from some minimum position (typically in the range
) to a maximum
. If this maximum is still within the train, we take the distance
as an entry for the distribution of the so-called
deflection length
[
45]. This concept was introduced for a semiflexible polymer confined in a cylindrical pore of diameter
. On average, the bond vectors are oriented parallel to the pore axis. However, an individual bond vector from
to
can make an angle
with this axis, even though subsequent angles are strongly correlated due to stiffness. By estimating the mean square displacement
from the pore axis, resulting from adding up such misalignment (using the KP model), one finds the length scale
, where
, as
[
45].
Qualitatively, one can take over this concept to confinement of semiflexible polymers in the potential well due to adsorption potential. There is a simple scaling argument to predict the variation of
with
[
15]: A string of
monomers, when adsorbed, wins an energy of order
, whereas the entropy change due to adsorption of such a string is of order
. Hence, the adsorption transition occurs when
, i.e.,
[
15]. This variation has been confirmed in our previous work [
26,
27]. It is important to note that the standard picture of an adsorbed chain as a sequence of ”trains“, ”loops“, and (one or two) ”tails’ [
21] must not be misunderstood as implying that “trains” are strictly two-dimensional chains restricted to the plane
. Such a picture does occur in the simplistic lattice model [
25,
44], however. There a train is a self-avoiding walk formed from straight lines (of lengths typically of order
) in the lattice plane next to the adsorbing wall, as stated above, and hence one finds
for such a model [
44,
46,
47].
It must be realized that there is only a vague analogy between the confinement of a polymer between two equivalent hard walls a distance apart and the confinement caused by the attractive potential associated with a single wall within the soft “potential well” of width . While in the slit pore case the average position of the center of mass of the polymer is trivially equidistant to both walls, and the monomer density distribution is symmetric around this position, the situation here is much more complicated. As we shall see, the center of mass position depends in a nontrivial way on all parameters of the problem (), and the monomer distribution has no symmetry properties. In addition, the confinement is by no means perfect: the formation of loops means the chain can “leak out” from the region where it is localized on average. Therefore, it is not apparent to what extent the deflection length concept can be applied here.
Indeed, when we analyze configurations as shown in
Figure 4a–c along the lines described above to sample the distribution function
of deflection lengths, we find a (perfectly fitted by a logarithmic function) monotonous decay of
with
, cf.
Figure 4d, whereby with increasing
large values of
are only slightly favored. The parameters,
in the empirical relation
,
Figure 4d, depend on
only weakly. This distribution implies that all lengths
, up to
, are very frequent while large values of
are systematically suppressed. The large weight of small
, etc. is clearly due to immediate reversals of the contours shown in
Figure 4a–c and other small-scale structures. One could filter out such features, but such a procedure is somewhat arbitrary. Therefore, we have decided to estimate an effective deflection length from the moments of
. The result is shown in
Figure 5 for
where the inset displays the variation of
for three values of
as function of stiffness
. However, as expected from the logarithmic distribution,
Figure 4d, the numbers extracted from the different moments somewhat disagree with each other, and the theoretical variation proportional to
is not confirmed. This problem clearly shows the limitations of the confinement analogy.
One consequence of the fact that adsorbed chains have a nontrivial structure also in the
z-direction,
Figure 4, was already studied in [
26,
27]. The orientational correlation function
exhibits a very nontrivial variation with
s: for
, the initial decay is still controlled by the three-dimensional persistence length
before a crossover to a larger value
sets in, i.e.,
. For
and
, one has
and
, as expected from Equation (
5). If these inequalities are not fulfilled, the amplitude
A is somewhat smaller than unity, and
is also reduced in comparison to its theoretical value of Equation (
5). Moreover, for
, a slow crossover to the power law decay
[
28,
29,
30] takes place (for
). The latter regime is due to excluded volume effects, and hence out of scope of the KP model treatment [
15,
16].
An interesting issue is also the perpendicular linear dimension
of strongly adsorbed chains. While a rather short strongly adsorbed chain (which is like a flexible rod) cannot have any loops in contrast to much longer chains, one might expect that
increases with growing
N albeit
Figure 6 demonstrates the opposite trend. In addition, when
(i.e.,
), the variation seems a bit steeper than for the case where
L and
are comparable. The explanation for this unexpected behavior is that for the same choice of
and
a longer chain is more strongly bound to the wall than a shorter one. This emerges from a study of both
f,
Figure 7, and
,
Figure 8. While near
the presence (or absence) of excluded volume does make a difference with respect to both
f and
, and in some cases a plot vs.
then shows a non-monotonic variation, this is not the case here. For stiff chains and
,
f is extremely close to its saturation value
, which means that the effect of loops is completely negligible.
We note that the order parameter
can be written in terms of the complementary angle
that a bond makes with the surface plane, as
, and hence
, when
is small. It is also interesting to study both
and
vs.
at fixed
N for the case of strong adsorption,
Figure 9. It is seen that both quantities vary proportionally to
within reasonable error limits. The residual dependence on
N almost disappears when
is plotted against
, see
Figure 9c.
We now turn to the interpretation of the apparent power laws visible in
Figure 9. Naively, one might think that the problem is fully analogous to the problem of a semiflexible polymer confined between two hard walls a distance
H apart, for which one finds [
48]
, with
. However, using
would yield
, while the data on
Figure 9a rather support
.
Thus, we must assume that the actual region where the monomers are localized should not be identified with a constant region
, but must be clearly of the same order as
. Thus, we would conclude
Figure 9 shows that indeed
is compatible with a power law of
, and while both
and
reveal individually a pronounced dependence on
N, see
Figure 9a,b, the plot of
vs.
leads to an almost perfect collapse of the different chain lengths on a master curve. This master curve, however, is not the equation predicted above, but rather
. We have no explanation of this (effective?) exponent yet. While from
Figure 6b it is clear that short chains (for a given choice of
and
) are less strongly adsorbed than longer ones, the almost perfect collapse of
versus
where the chain length dependence is eliminated (
Figure 9c,d) seems nontrivial to us.
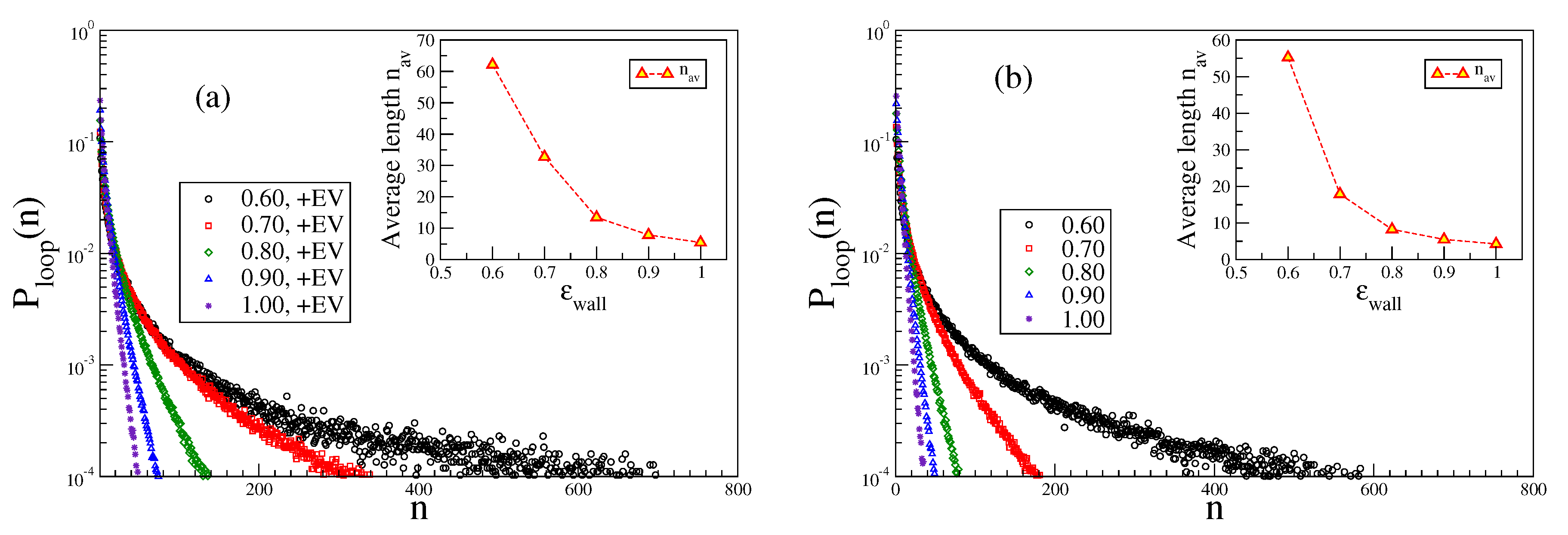
4. Distributions of Trains and Loops
The very interesting behavior of loops and tails in the vicinity of the adsorption threshold, where the lateral correlation length (i.e., the typical maximal lateral size of loops) is larger than
, has already been discussed in the literature [
15,
20]. Here, we focus rather on the behavior of trains and loops in the strongly adsorbed phase. However, since for large
the probability to observe loops and tails is extremely small, we focus here on chains with moderate stiffness,
and 8, respectively. In these cases, the adsorption transition for
occurs near
[
26,
27].
Figure 10 demonstrates (note the logarithmic ordinate scale) that for large
n the data for
are very well described by a simple exponential decay. The average train length increases strongly with
(but for the chosen parameters
, so there should not be too strong effects due to the finite length of the chain present).
In contrast, the distribution of loop lengths
exhibits a very different behavior, cf.
Figure 11. There is a rapid initial decay and then a gradual crossover to a much slower decay. We tentatively interpret this as qualitative evidence for Semenov’s [
15] prediction that not too far away from the adsorption transition there should be a coexistence of small loops, whose extension away from the plane
is of the order of
or less, with much fewer large loops which make much larger extensions away from the surface. Note that with excluded volume the loops are somewhat larger than without. Of course, the average length of the loops increases when
decreases towards
, reflecting the critical divergence of the correlation length of the adsorption transition in the limit
. Due to the finiteness of
, this divergence is rounded off, however.
In this context, it is of interest to study simply also the decay of the density distribution
as a function of the distance from the surface,
Figure 12. For large values of
and large
, there is a sharp peak near
, and the mean square width of this peak,
, is extremely small and strongly decreases as
,
Figure 12. Then, the polymers are rigid rods (of finite length
) very tightly bound to the wall. Fluctuations of their center-of-mass position and orientation will also vanish as
(see
Appendix A). However, when
is small enough so that the chosen value of
is close to
,
becomes larger than unity due to a shoulder of
for
z much larger than
. Ultimately, in a medium regime,
, for
, a power law decay
is predicted to occur [
15], and our data are qualitatively compatible with this prediction,
Figure 12a. However, a chain length
is still by far too small to yield a wide enough region
, which is needed to clearly observe this regime. For large
z,
decays exponentially with
z for
, as predicted [
15]. The phenomenological power laws indicated for the variation of
with
remain to be explained, however.
5. Conclusions
In this paper, we have studied adsorption of semiflexible polymers on a flat unstructured surface, using a coarse-grained bead-spring model, applying Molecular Dynamics methods, and varying the persistence length over a wide range. A distinguishing feature is the choice of 10–4 adsorption potential, Equation (
6), qualitatively reasonable for adsorption on a thin rigid membrane. Chain lengths are used in the range from
to
effective monomers. Unlike flexible chains, where in the regime of weak adsorption (i.e., adsorption energy
slightly exceeding the threshold
) “pancakes” formed from blobs of mesoscopic thickness occur, we find here a thin adsorbed layer with thickness comparable to the chain thickness (or, monomer diameter, respectively). The position of the center of mass of this layer does not coincide with the position
of the minimum in the adsorption potential but is clearly farther away from the adsorbing substrate surface. With increasing chain length
N (for otherwise identical parameters
and stiffness
), the binding of the chain to the substrate becomes tighter, i.e., the perpendicular linear dimension
and center-of-mass position
decrease, other parameters (adsorbed fraction
f and orientational order parameter
) come close to their saturation values, and the chain gradually adopts a quasi two-dimensional conformation. However, in no case do we come close to a situation where the polymer is strictly two-dimensional and confined to the plane
. The
Kratky–Porod model never yields a perfect representation of our results for the range of stiffness
and chain length
L accessible in our work.
While many of our findings are in qualitative agreement with the theoretical predictions of Semenov [
15], a quantitative comparison was not sought since the latter work addresses the region
, taking also
very much larger than the effective chain thickness. This regime is not easily accessed by Molecular Dynamics simulations, however. Many predictions of the theory [
15] refer to the vicinity of
, which can only be tested, if much longer chains are accessible. The theory [
15] did not discuss many of the phenomena found here such as the distribution of the center of mass of the chains and its behavior with
and
N, however, cf.
Figure 2b.
Thus, the findings here suggest that additional theoretical studies addressing such issues would be desirable. With respect to the experiment, our simulations provide overwhelming evidence that properties of an adsorbed semiflexible chain are by no means identical to properties of a strictly two-dimensional chain that “lives” in the plane , an assumption, which is implicit in many discussions in the literature. An adsorbed chain still has degrees of freedom due to its intrinsically three-dimensional character, and it is still an open problem to understand all consequences of this fact.