Influence of Silica Specific Surface Area on the Viscoelastic and Fatigue Behaviors of Silica-Filled SBR Composites
Abstract
1. Introduction
2. Experimental Section
2.1. Materials, Mixing and Curing
2.2. Tensile Testing
2.3. Critical Tearing Energy
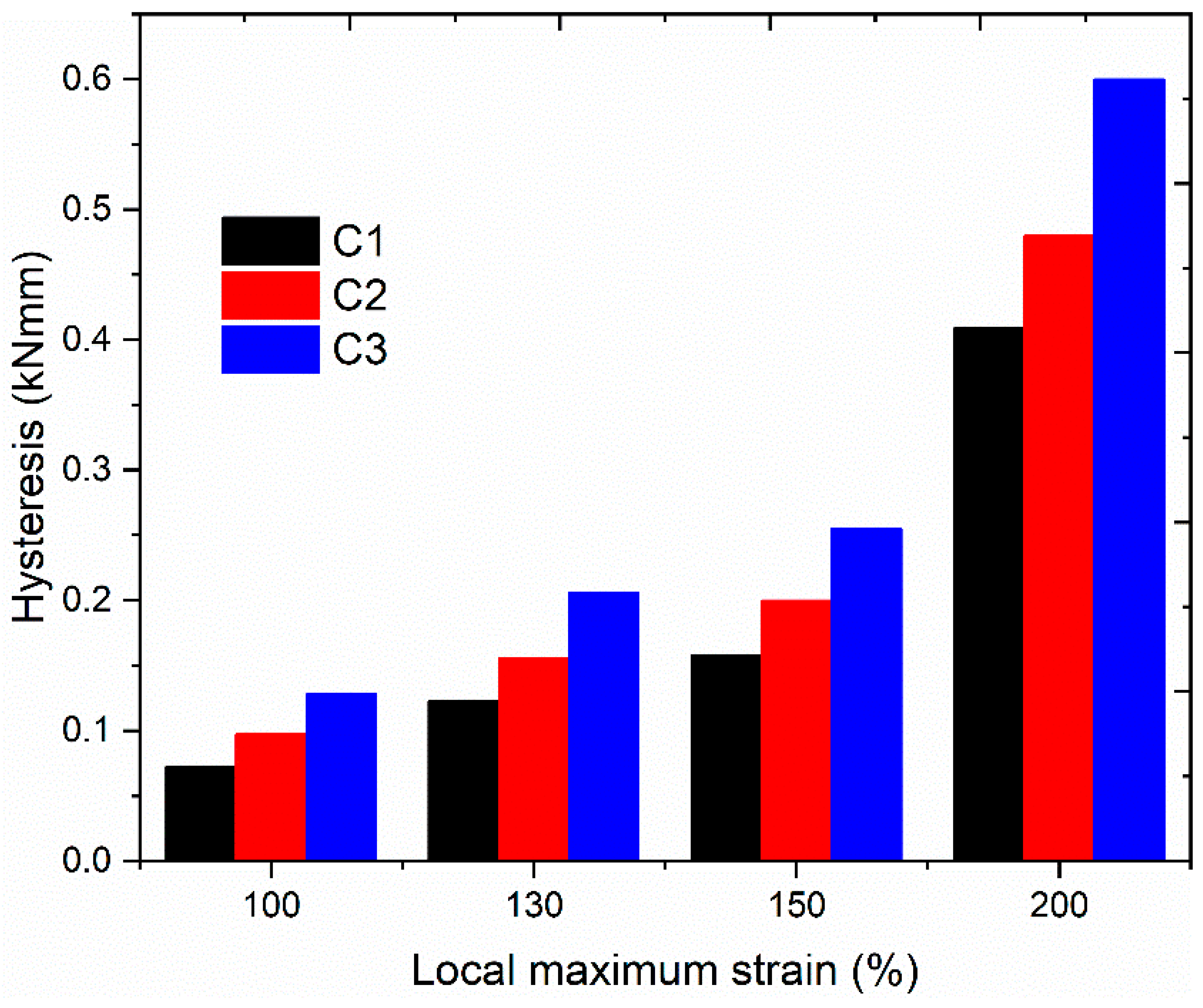
2.4. Hysteresis and Volume Strain
2.5. Dynamic Mechanical Analysis
2.6. Fatigue Testing and End of Life Criteria
2.7. Scanning Electron Microscopy
2.8. Micro-Computed X-ray Tomography
3. Results and Discussions
3.1. Initial Morphology Characterization
3.2. Crack Precursor Measurement Based on Tearing Energy
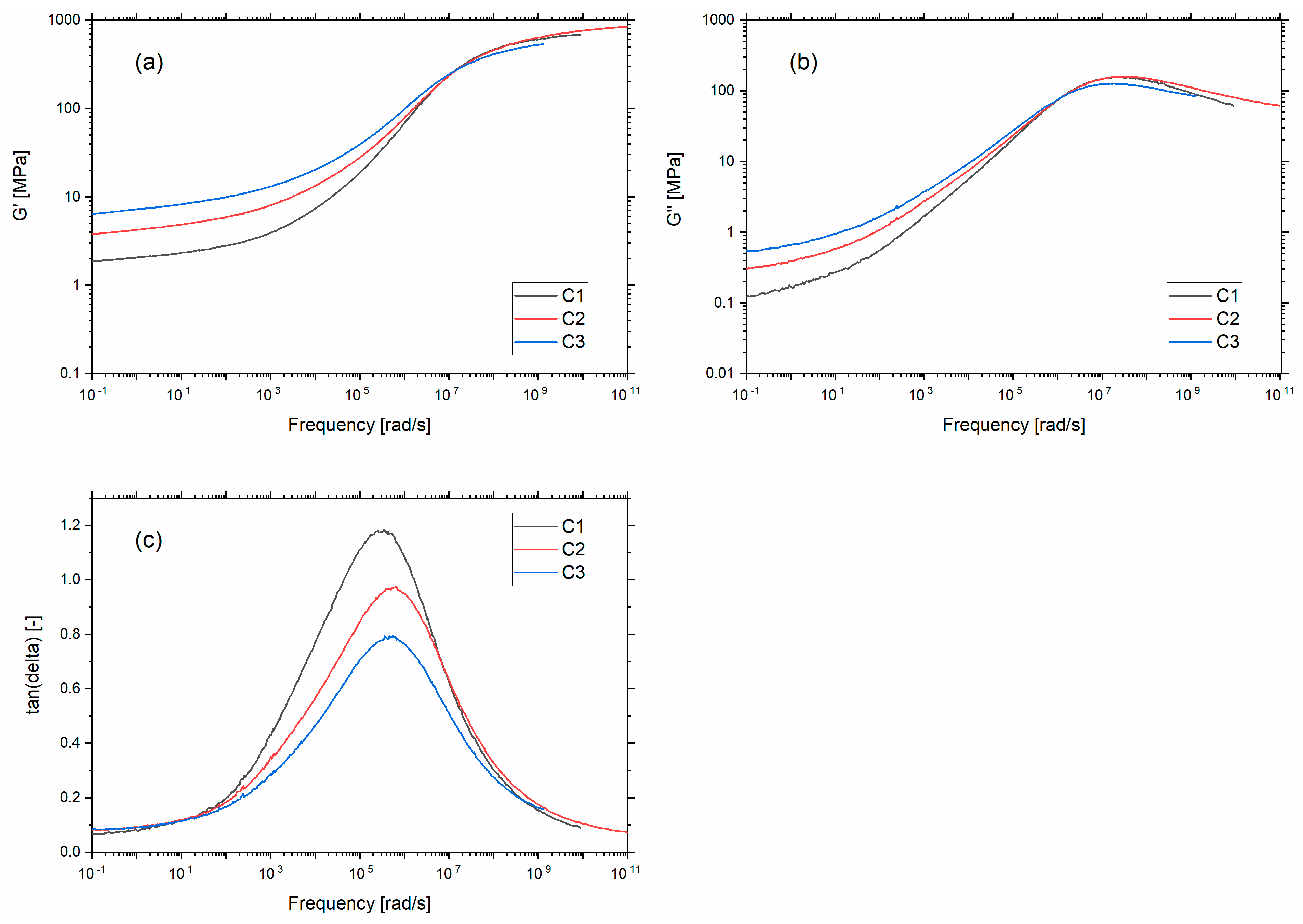
3.3. Viscoelastic Properties and Hysteresis
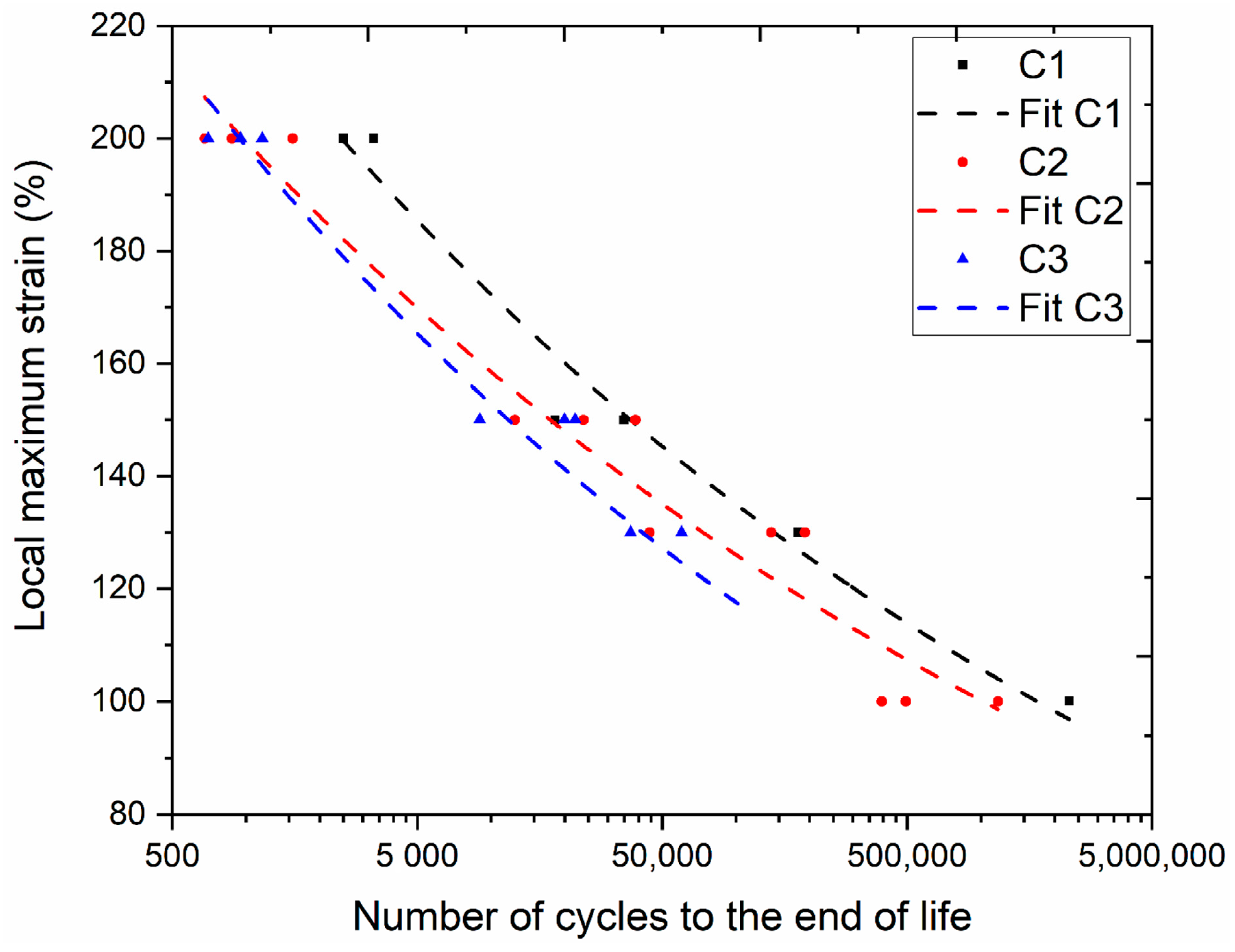
3.4. Fatigue Life
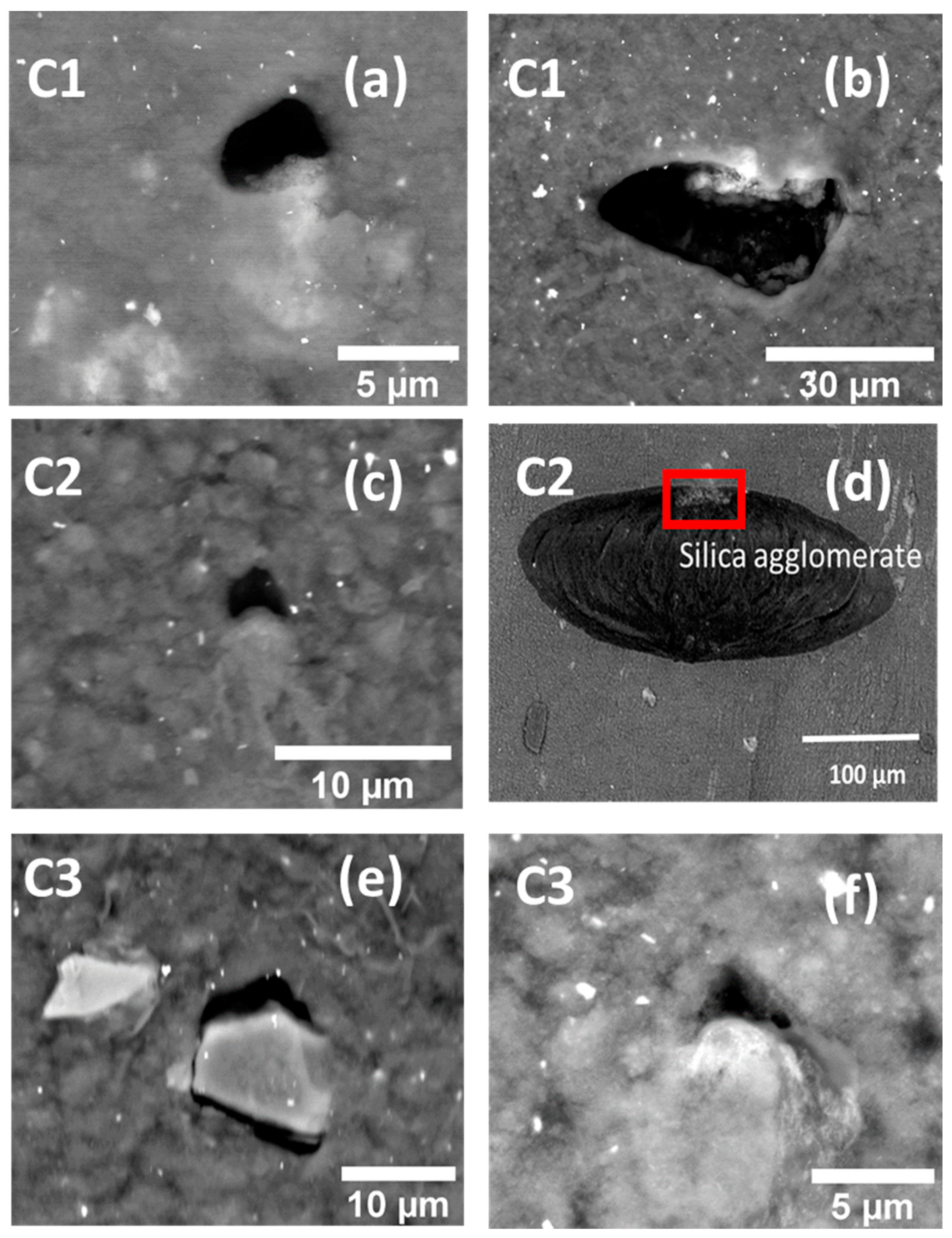
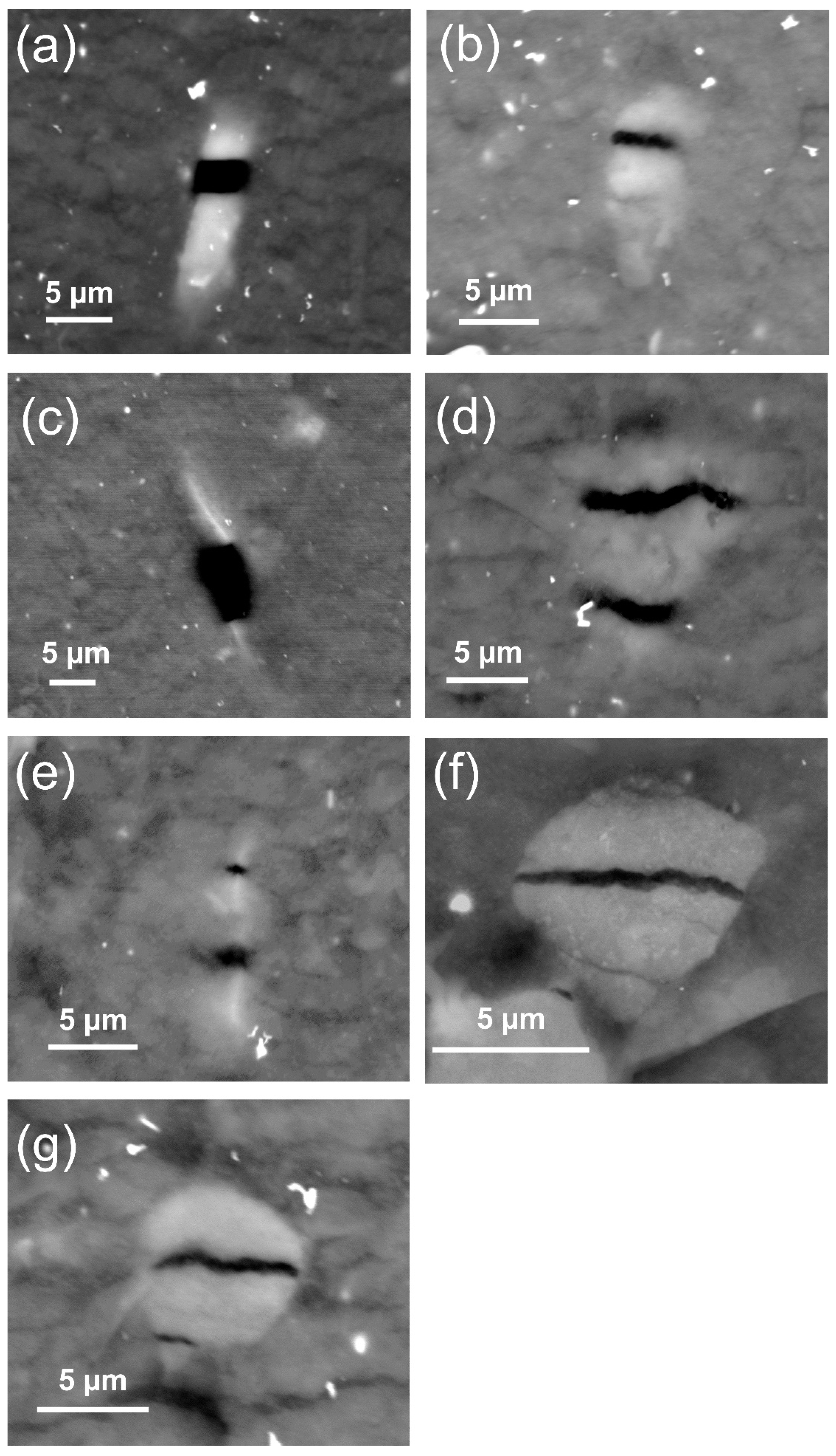
3.5. Mechanisms of Crack Initiation
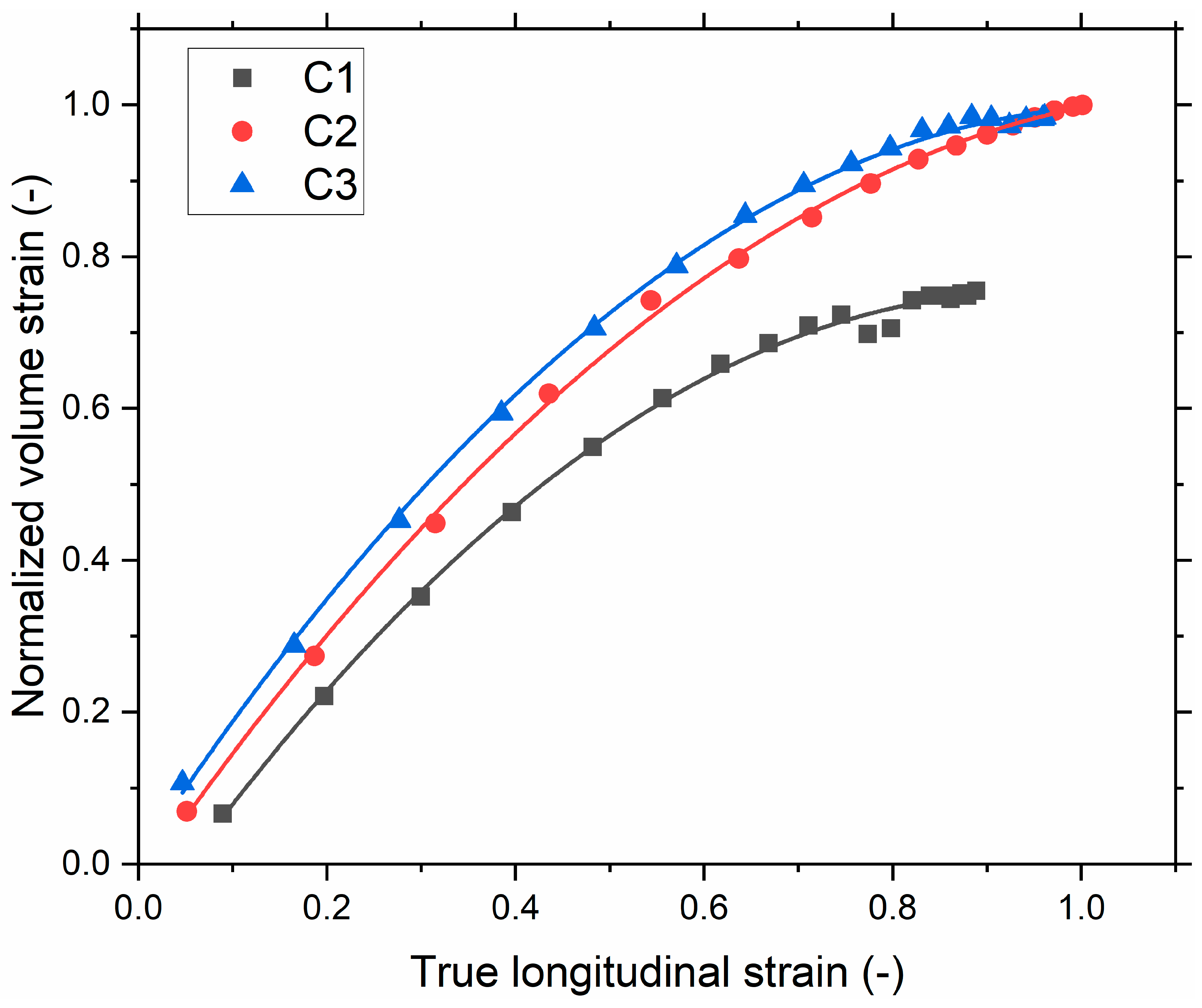
3.6. Volume of Cracks Based on µCT and DIC
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Mars, W.V.; Fatemi, A. Fatigue crack nucleation and growth in filled natural rubber. Fatigue Fract. Eng. Mater. Struct. 2003, 26, 779–789. [Google Scholar] [CrossRef]
- Huneau, B.; Masquelier, I.; Marco, Y.; Le Saux, V.; Noizet, S.; Schiel, C.; Charrier, P. Fatigue crack initiation in a carbon black–filled natural rubber. Rubber Chem. Technol. 2016, 89, 126–141. [Google Scholar] [CrossRef]
- Studebaker, M.L. The Chemistry of Carbon Black and Reinforcement. Rubber Chem. Technol. 1957, 30, 1400–1483. [Google Scholar] [CrossRef]
- Stockelhuber, K.; Svistkov, A.; Pelevin, A.; Heinrich, G. Impact of filler surface modification on large scale mechanics of styrene butadiene/silica rubber composites. Macromolecules 2011, 44, 4366–4381. [Google Scholar] [CrossRef]
- Robertson, C.G.; Hardman, N.J. Nature of Carbon Black Reinforcement of Rubber: Perspective on the Original Polymer Nanocomposite. Polymers 2021, 13, 538. [Google Scholar] [CrossRef]
- Yang, R.; Song, Y.; Zheng, Q. Payne effect of silica-filled styrene-butadiene rubber. Polymer 2017, 116, 304–313. [Google Scholar] [CrossRef]
- Payne, A.; Whittaker, R. Low strain dynamic properties of filled rubbers. Rubber Chem. Technol. 1971, 44, 440–478. [Google Scholar] [CrossRef]
- Gauthier, C.; Reynaud, E.; Vassoille, R.; Ladouce-Stelandre, L. Analysis of the non-linear viscoelastic behaviour of silica filled styrene butadiene rubber. Polymer 2004, 45, 2761–2771. [Google Scholar] [CrossRef]
- Mars, W.V.; Fatemi, A. A literature survey on fatigue analysis approaches for rubber. Int. J. Fatigue 2002, 24, 949–961. [Google Scholar] [CrossRef]
- Zhao, J.; Ghebremeskel, G.N. A Review of Some of the Factors Affecting Fracture and Fatigue in SBR and BR Vulcanizates. Rubber Chem. Technol. 2001, 74, 409–427. [Google Scholar] [CrossRef]
- Zine, A.; Benseddiq, N.; Naït Abdelaziz, M. Rubber fatigue life under multiaxial loading: Numerical and experimental investigations. Int. J. Fatigue 2011, 33, 1360–1368. [Google Scholar] [CrossRef]
- Ruellan, B.; Le Cam, J.B.; Jeanneau, I.; Canévet, F.; Mortier, F.; Robin, E. Fatigue of natural rubber under different temperatures. Int. J. Fatigue 2019, 124, 544–557. [Google Scholar] [CrossRef]
- Ostoja-Kuczynski, E.; Charrier, P.; Verron, E.; Marckmann, G.; Gornet, L.; Chagnon, G. Crack initiation in filled natural rubber: Experimental database and macroscopic observations. In Proceedings of the Third European Conference on Constitutive Models for Rubber, Londres, UK, 13–15 September 2003; pp. 41–47. [Google Scholar]
- Tee, Y.L.; Loo, M.S.; Andriyana, A. Recent advances on fatigue of rubber after the literature survey by Mars and Fatemi in 2002 and 2004. Int. J. Fatigue 2018, 110, 115–129. [Google Scholar] [CrossRef]
- Mullins, L. Effect of Stretching on the Properties of Rubber. Rubber Chem. Technol. 1948, 21, 281–300. [Google Scholar] [CrossRef]
- Diani, J.; Fayolle, B.; Gilormini, P. A review on the Mullins effect. Eur. Polym. J. 2009, 45, 601–612. [Google Scholar] [CrossRef]
- Weng, G.; Yao, H.; Chang, A.; Fu, K.; Liu, Y.; Chen, Z. Crack growth mechanism of natural rubber under fatigue loading studied by a real-time crack tip morphology monitoring method. RSC Adv. 2014, 4, 43942–43950. [Google Scholar] [CrossRef]
- Kar, K.K.; Bhowmick, A.K. Hysteresis loss in filled rubber vulcanizates and its relationship with heat generation. J. Appl. Polym. Sci. 1997, 64, 1541–1555. [Google Scholar] [CrossRef]
- Kim, J.H.; Jeong, H.Y. A study on the material properties and fatigue life of natural rubber with different carbon blacks. Int. J. Fatigue 2005, 27, 263–272. [Google Scholar] [CrossRef]
- Thaptong, P.; Sae-oui, P.; Sirisinha, C. Influences of styrene butadiene rubber and silica types on performance of passenger car radial tire tread. Rubber Chem. Technol. 2017, 90, 699–713. [Google Scholar] [CrossRef]
- Robertson, C.G.; Lin, C.J.; Bogoslovov, R.B.; Rackaitis, M.; Sadhukhan, P.; Quinn, J.D.; Roland, C.M. Flocculation, reinforcement, and glass transition effects in silica-filled styrene-butadiene rubber. Rubber Chem. Technol. 2011, 84, 507–519. [Google Scholar] [CrossRef]
- Choi, S.-S. Improvement of properties of silica-filled styrene–butadiene rubber compounds using acrylonitrile–butadiene rubber. J. Appl. Polym. Sci. 2001, 79, 1127–1133. [Google Scholar] [CrossRef]
- Ramier, J.; Gauthier, C.; Chazeau, L.; Stelandre, L.; Guy, L. Payne effect in silica-filled styrene–butadiene rubber: Influence of surface treatment. J. Polym. Sci. Part B Polym. Phys. 2007, 45, 286–298. [Google Scholar] [CrossRef]
- Federico, C.E.; Padmanathan, H.R.; Kotecky, O.; Rommel, R.; Rauchs, G.; Fleming, Y.; Addiego, F.; Westermann, S. Cavitation Micro-mechanisms in Silica-Filled Styrene-Butadiene Rubber upon Fatigue and Cyclic Tensile Testing. In Fatigue Crack Growth in Rubber Materials; Heinrich, G., Kipscholl, R., Stoček, R., Eds.; Springer: Cham, Switzerland, 2020; pp. 109–129. [Google Scholar] [CrossRef]
- Federico, C.E.; Rauchs, G.; Kotecky, O.; Westermann, S.; Addiego, F. Cavitation in thermoplastic-reinforced rubber composites upon cyclic testing: Multiscale characterization and modelling. Polymer 2020, 211, 123084. [Google Scholar] [CrossRef]
- Andersen, M.; Hopperstad, O.S.; Clausen, A.H. Volumetric strain measurement of polymeric materials subjected to uniaxial tension. Strain 2019, 55, e12314. [Google Scholar] [CrossRef]
- Starkova, O.; Aniskevich, A. Poisson’s ratio and the incompressibility relation for various strain measures with the example of a silica-filled SBR rubber in uniaxial tension tests. Polym. Test. 2010, 29, 310–318. [Google Scholar] [CrossRef]
- Robertson, C.G.; Tunnicliffe, L.B.; Maciag, L.; Bauman, M.A.; Miller, K.; Herd, C.R.; Mars, W.V. Characterizing Distributions of Tensile Strength and Crack Precursor Size to Evaluate Filler Dispersion Effects and Reliability of Rubber. Polymer 2020, 12, 203. [Google Scholar] [CrossRef]
- Li, F.; Liu, J.; Mars, W.V.; Chan, T.W.; Lu, Y.; Yang, H.; Zhang, L. Crack precursor size for natural rubber inferred from relaxing and non-relaxing fatigue experiments. Int. J. Fatigue 2015, 80, 50–57. [Google Scholar] [CrossRef]
- Ludwig, M.; Alshuth, T.; El Yaagoubi, M.; Juhre, D. Lifetime prediction of elastomers based on statistical occurrence of material defects. In Constitutive Models for Rubbers IX; Marvalova, B., Petrikova, I., Eds.; CRC Press: London, UK, 2015; pp. 445–448. [Google Scholar] [CrossRef]
- Guo, H.; Li, F.; Wen, S.; Yang, H.; Zhang, L. Characterization and Quantitative Analysis of Crack Precursor Size for Rubber Composites. Materials 2019, 12, 3442. [Google Scholar] [CrossRef]
- Chakraborty, S.K.; De, S.K. Silica- and Clay-Reinforced Carboxylated Nitrile Rubber Vulcanized by a Mixed Crosslinking System. Rubber Chem. Technol. 1982, 55, 990–1003. [Google Scholar] [CrossRef]
- Glanowski, T.; Huneau, B.; Marco, Y.; Le Saux, V.; Champy, C.; Charrier, P. Fatigue initiation mechanisms in elastomers: A microtomography-based analysis. MATEC Web Conf. 2018, 165, 08005. [Google Scholar] [CrossRef][Green Version]
- Marco, Y.; Huneau, B.; Masquelier, I.; Le Saux, V.; Charrier, P. Prediction of fatigue properties of natural rubber based on the descriptions of the cracks population and of the dissipated energy. Polym. Test. 2017, 59, 67–74. [Google Scholar] [CrossRef]
- Marco, Y.; Masquelier, I.; Le Saux, V.; Calloch, S.; Huneau, B.; Charrier, P. Contributions of IR thermography and X-ray tomography to the fatigue characterization of elastomeric materials. In Proceedings of the ECCMR VIII, San Sebastian, Spain, 25–28 June 2013; pp. 387–392. [Google Scholar]
- Weng, G.; Chang, A.; Fu, K.; Kang, J.; Ding, Y.; Chen, Z. Crack growth mechanism of styrene-butadiene rubber filled with silica nanoparticles studied by small angle X-ray scattering. RSC Adv. 2016, 6, 8406–8415. [Google Scholar] [CrossRef]
- Balutch, T.; Huneau, B.; Marco, Y.; Charrier, P.; Champy, C. Fatigue behaviour of an industrial synthetic rubber. MATEC Web Conf. 2018, 165, 22004. [Google Scholar] [CrossRef][Green Version]
- Marco, Y.; Le Saux, V.; Calloch, S.; Charrier, P. X-ray computed μ-tomography: A tool for the characterization of fatigue defect population in a polychloroprene rubber. Procedia Eng. 2010, 2, 2131–2140. [Google Scholar] [CrossRef][Green Version]
- Guy, L.; Daudey, S.; Cochet, P.; Bomal, Y. New insights in the dynamic properties of precipitated silica filled rubber using a new high surface silica. Kautsch. Gummi Kunstst. 2009, 62, 383–391. [Google Scholar]
- Kaewsakul, W.; Sahakaro, K.; Dierkes, W.K.; Noordermeer, J.W. Optimization of mixing conditions for silica-reinforced natural rubber tire tread compounds. Rubber Chem. Technol. 2012, 85, 277–294. [Google Scholar] [CrossRef]
- Leblanc, J.L.; Cartault, M. Advanced torsional dynamic methods to study the morphology of uncured filled rubber compounds. J. Appl. Polym. Sci. 2001, 80, 2093–2104. [Google Scholar] [CrossRef]
- Belkhiria, S.; Seddik, R.; Atig, A.; Ben Sghaier, R.; Hamdi, A.; Fathallah, R. Fatigue reliability prediction of rubber parts based on Wöhler diagrams. Int. J. Adv. Manuf. Technol. 2018, 96, 439–450. [Google Scholar] [CrossRef]
- Donnet, J.-B.; Custodero, E. 8-Reinforcement of Elastomers by Particulate Fillers. In Science and Technology of Rubber, 3rd ed.; Mark, J.E., Erman, B., Eirich, F.R., Eds.; Academic Press: Burlington, NJ, USA, 2005; pp. 367–400. [Google Scholar] [CrossRef]
- Luginsland, H.-D.; Frohlich, J.; Wehmeier, A. Influence of Different Silanes on the Reinforcement of Silica-Filled Rubber Compounds. Rubber Chem. Technol. 2002, 75, 563–579. [Google Scholar] [CrossRef]
- Fröhlich, J.; Niedermeier, W.; Luginsland, H.D. The effect of filler–filler and filler–elastomer interaction on rubber reinforcement. Compos. Part A Appl. Sci. Manuf. 2005, 36, 449–460. [Google Scholar] [CrossRef]
- Koh, S.-W.; Kim, J.-K.; Mai, Y.-W. Fracture toughness and failure mechanisms in silica-filled epoxy resin composites: Effects of temperature and loading rate. Polymer 1993, 34, 3446–3455. [Google Scholar] [CrossRef]
- Hosseinpour, K.; Ghasemi, A.R. Agglomeration and aspect ratio effects on the long-term creep of carbon nanotubes/fiber/polymer composite cylindrical shells. J. Sandw. Struct. Mater. 2021, 23, 1272–1291. [Google Scholar] [CrossRef]



| Compound 1 | Compound 2 | Compound 3 | ||
|---|---|---|---|---|
| N2 Silica specific surface area, BET (m2/g) | 125 | 165 | 200 | |
| SBR SE SLR 4602 * | 100 | 100 | 100 | |
| Antioxidant | 2.5 | 2.5 | 2.5 | |
| Oil Vivatec 500 | 25 | 25 | 25 | |
| Activator Stearic Acid | 3 | 3 | 3 | |
| Silica Hi-Sil 315 ** | 90 | - | - | |
| Ingredients (phr) | Silica Zeosil 1165 MP *** | - | 90 | - |
| Silica Zeosil Premium 200 MP *** | - | - | 90 | |
| Silane Si266 | 6 | 7.2 | 9 | |
| ZnO | 2.5 | 2.5 | 2.5 | |
| Sulfur | 1.4 | 1.4 | 1.4 | |
| Accelerator | 1.8 | 2.3 | 2.88 | |
| Accelerator | 2.5 | 3.2 | 4 | |
| Total phr | 234.7 | 237.1 | 240.28 |
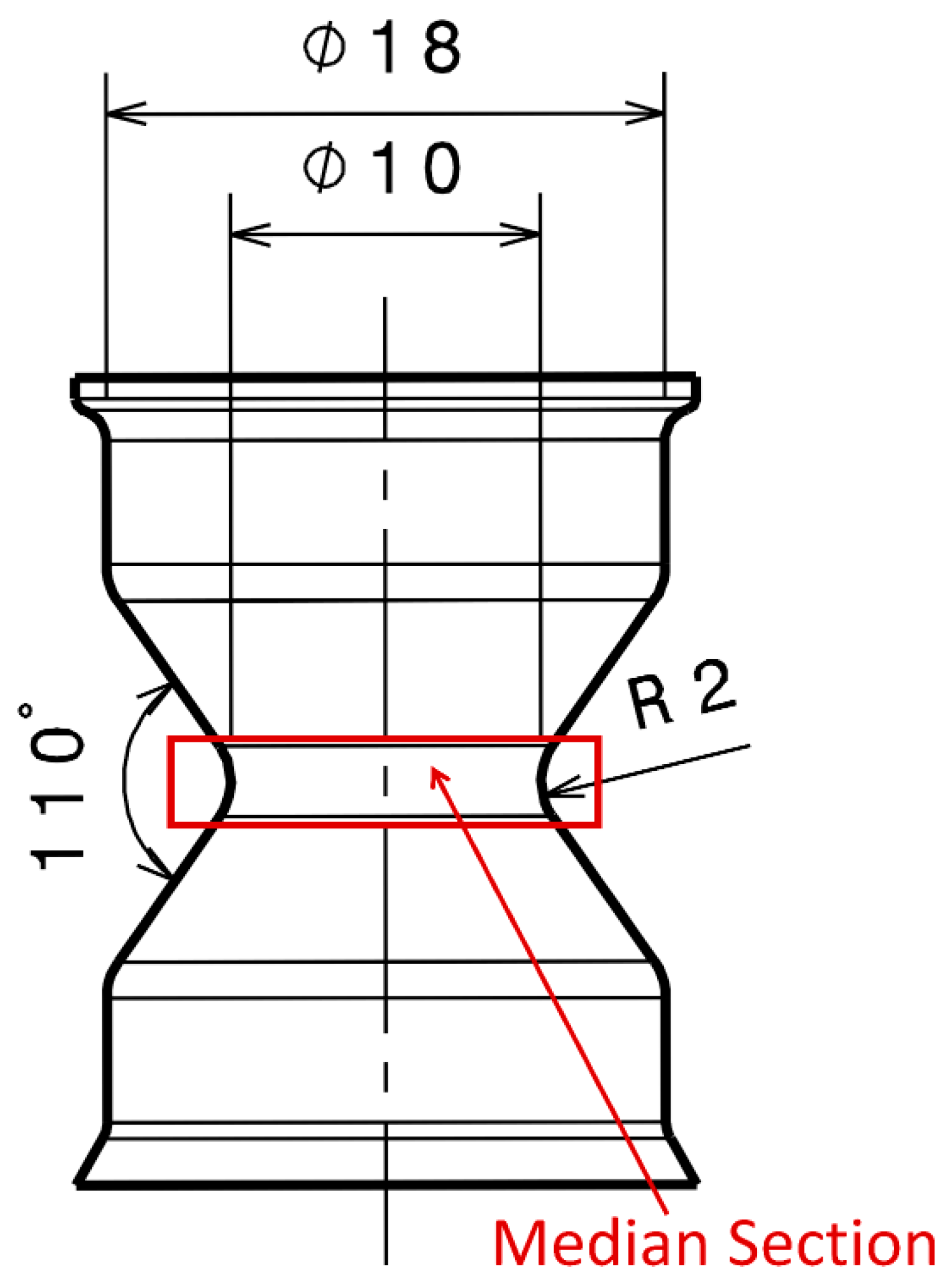
| Acquisition Parameter | Hourglass Sample | Cuboid Sample |
|---|---|---|
| SOD (mm) | 35 | 7.75 |
| SDD (mm) | 497 | 329 |
| Voxel size (µm) | 9 | 3 |
| X-ray source voltage (kV) | 45 | 40 |
| X-ray source current (µA) | 165 | 160 |
| Frame rate (frame/s) | 1 | 0.75 |
| Number of averaged frames | 5 | 3 |
| Angular range (°) | 360 | 360 |
| Angular step (°) | 0.25 | 0.25 |
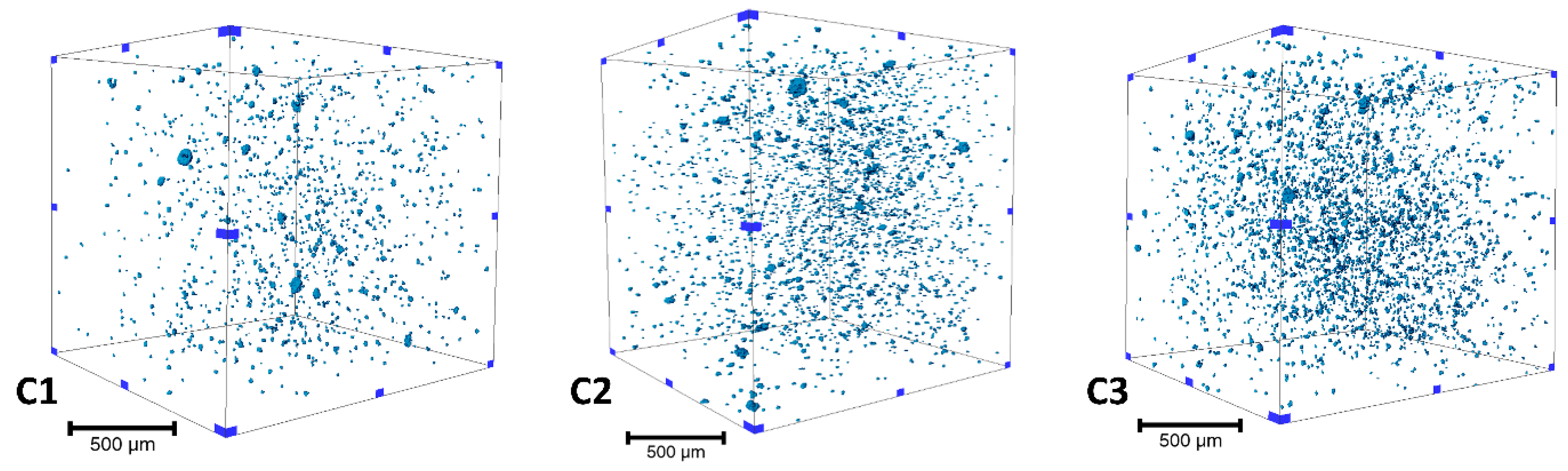
| C1 | C2 | C3 | |
|---|---|---|---|
| Median equivalent diameter (µm) | 12.42 | 12.05 | 12.10 |
| Volume fraction (%) | 0.12 | 0.24 | 0.27 |
| Density of agglomerates per mm3 | 307 | 746 | 896 |
| Stress at Break (MPa) | Strain at Break (%) | Tear Strength (N/mm) | Crack Precursor Size (µm) | |
|---|---|---|---|---|
| C1 | 18.2 ± 0.1 | 277.0 ± 5.7 | 30.0 ± 0.5 | 365.0 ± 0.7 |
| C2 | 15.6 ± 0.4 | 273.0 ± 2.1 | 35.3 ± 0.2 | 501.0 ± 16.9 |
| C3 | 19.0 ± 0.2 | 254.0 ± 0.7 | 29.9 ± 0.7 | 370.0 ± 4.9 |
| E’(0.1) | E’(10) | ∆E’ [E’(0.1)–E’(10)] | |
|---|---|---|---|
| C1 | 9.2 | 5.5 | 3.7 |
| C2 | 10.9 | 5.7 | 5.2 |
| C3 | 15.3 | 8.0 | 7.3 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Padmanathan, H.R.; Federico, C.E.; Addiego, F.; Rommel, R.; Kotecký, O.; Westermann, S.; Fleming, Y. Influence of Silica Specific Surface Area on the Viscoelastic and Fatigue Behaviors of Silica-Filled SBR Composites. Polymers 2021, 13, 3094. https://doi.org/10.3390/polym13183094
Padmanathan HR, Federico CE, Addiego F, Rommel R, Kotecký O, Westermann S, Fleming Y. Influence of Silica Specific Surface Area on the Viscoelastic and Fatigue Behaviors of Silica-Filled SBR Composites. Polymers. 2021; 13(18):3094. https://doi.org/10.3390/polym13183094
Chicago/Turabian StylePadmanathan, Hiron Raja, Carlos Eloy Federico, Frédéric Addiego, Robert Rommel, Ondřej Kotecký, Stephan Westermann, and Yves Fleming. 2021. "Influence of Silica Specific Surface Area on the Viscoelastic and Fatigue Behaviors of Silica-Filled SBR Composites" Polymers 13, no. 18: 3094. https://doi.org/10.3390/polym13183094
APA StylePadmanathan, H. R., Federico, C. E., Addiego, F., Rommel, R., Kotecký, O., Westermann, S., & Fleming, Y. (2021). Influence of Silica Specific Surface Area on the Viscoelastic and Fatigue Behaviors of Silica-Filled SBR Composites. Polymers, 13(18), 3094. https://doi.org/10.3390/polym13183094








