Geometrical Scaling Effects in the Mechanical Properties of 3D-Printed Body-Centered Cubic (BCC) Lattice Structures
Abstract
:1. Introduction
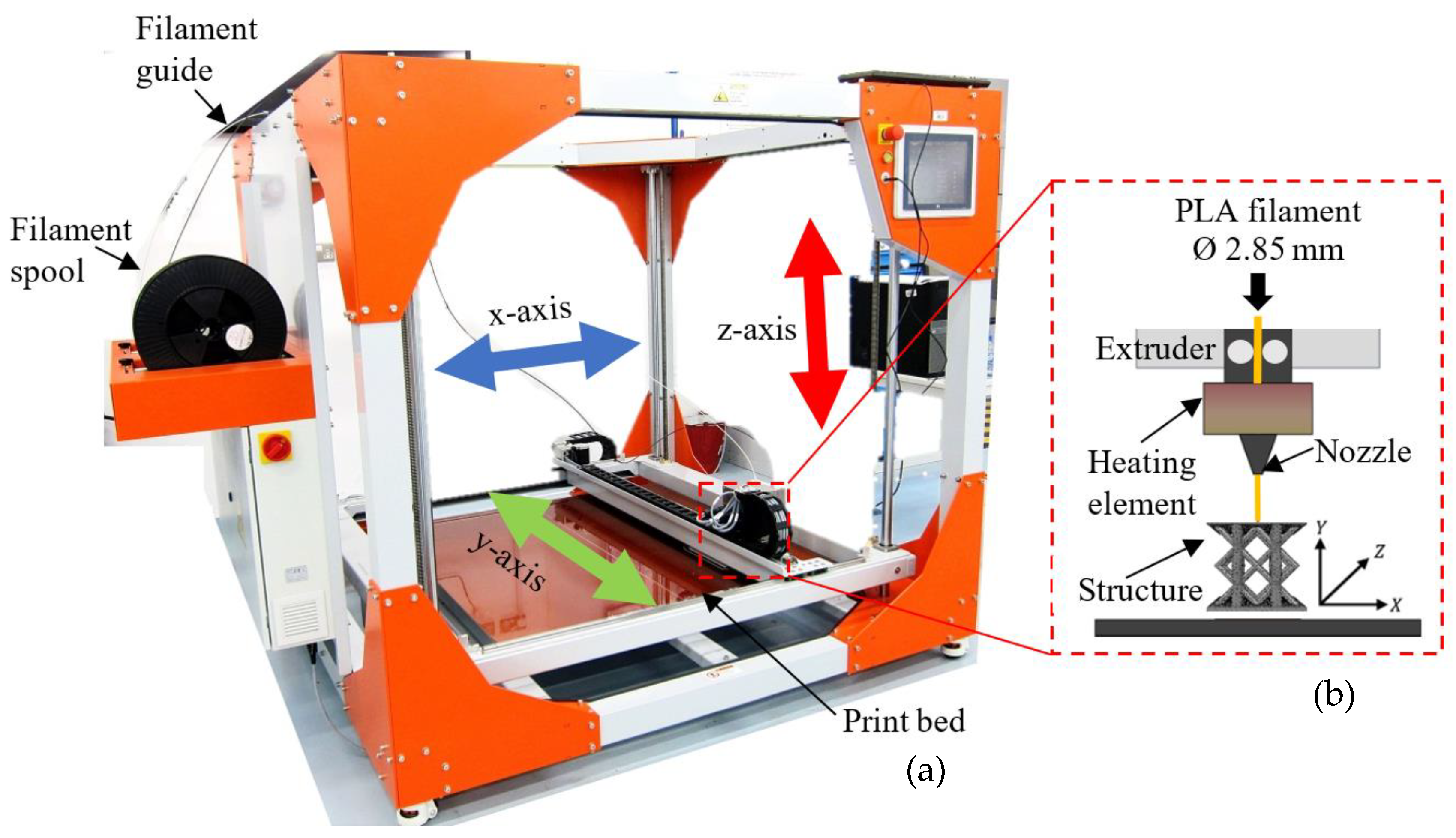
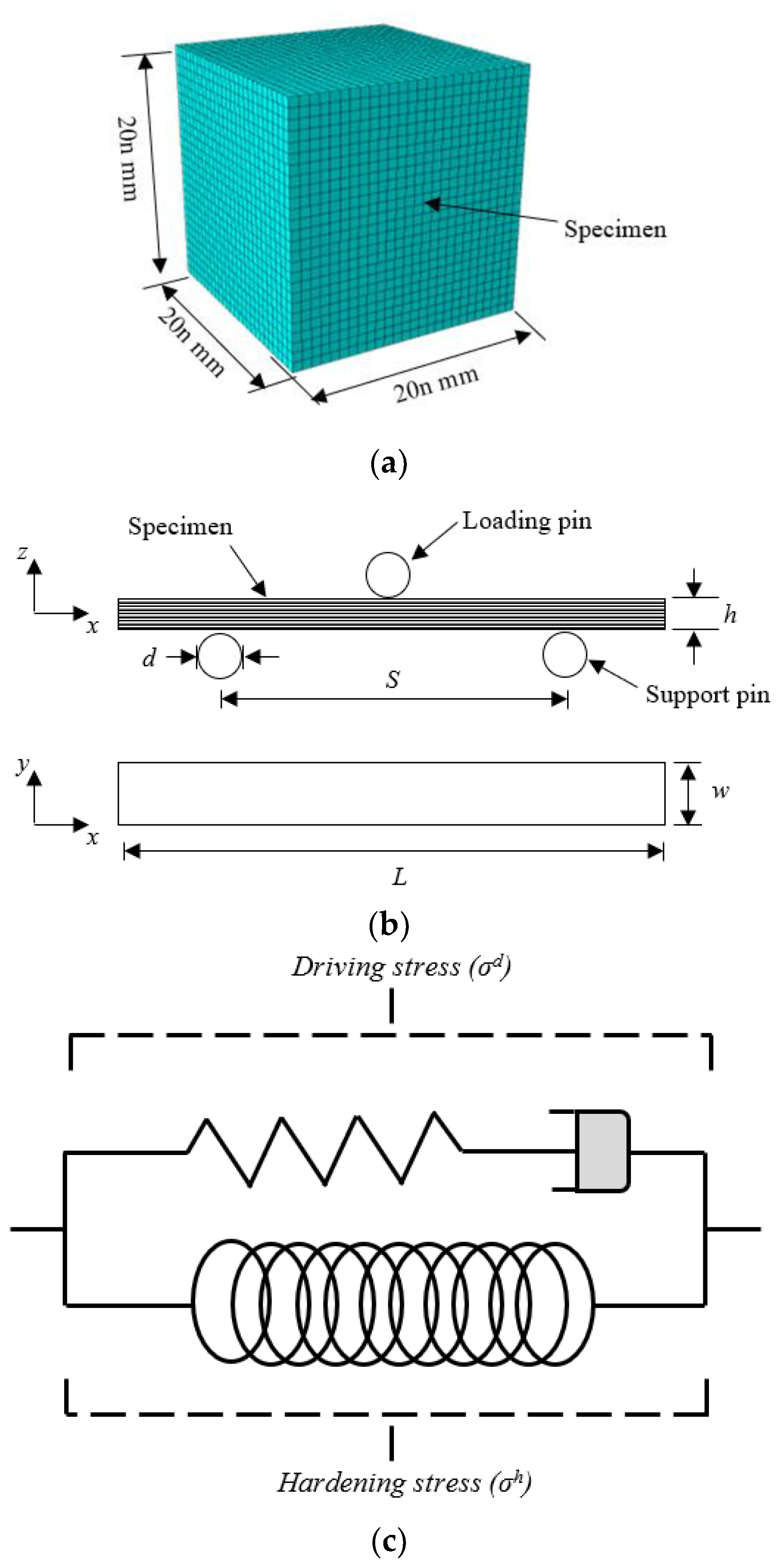
2. Materials and Method
3. Numerical Analysis
Modelling of the PLA Polymer
4. Results and Discussion
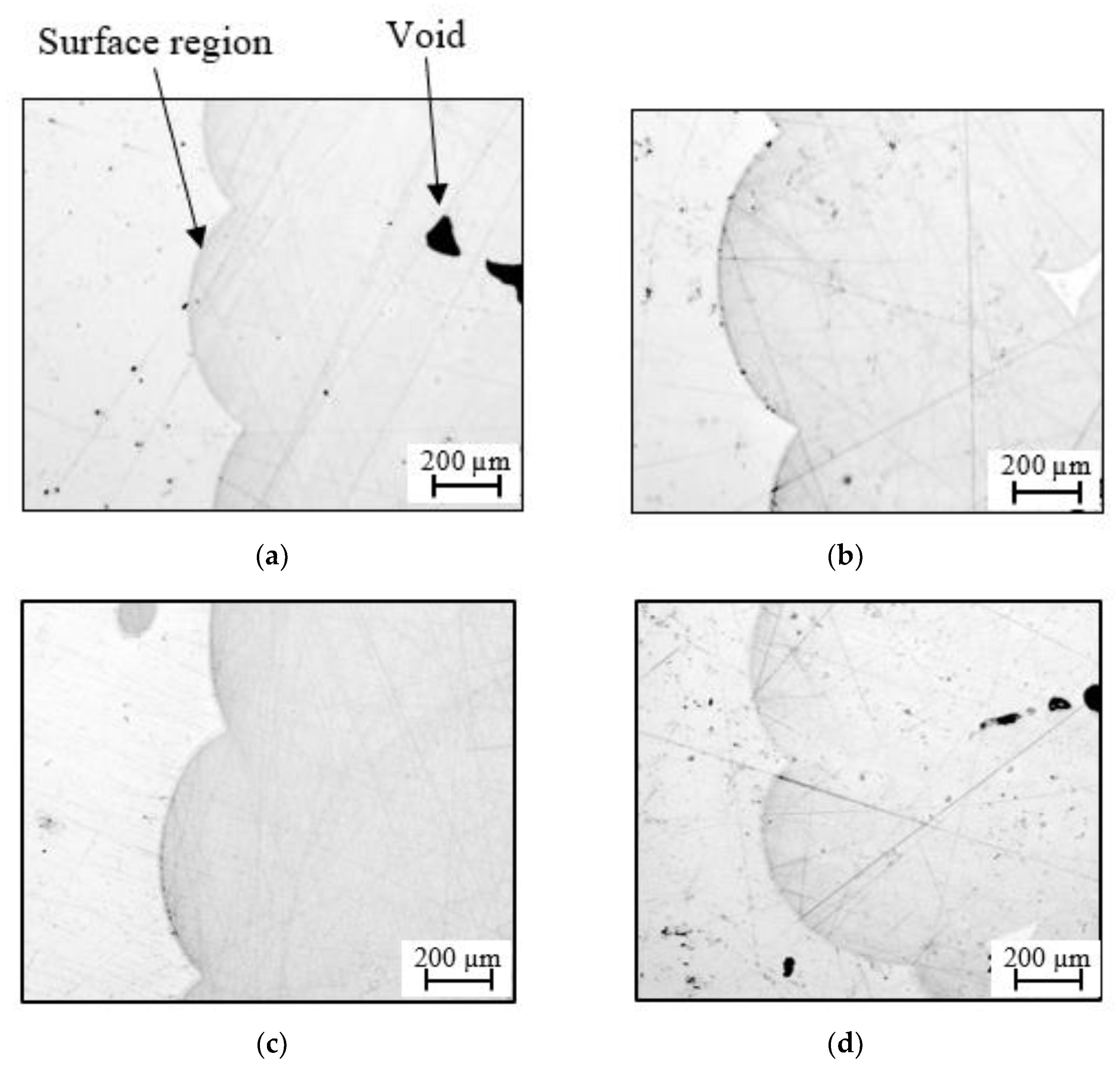
4.1. Surface Characteristics
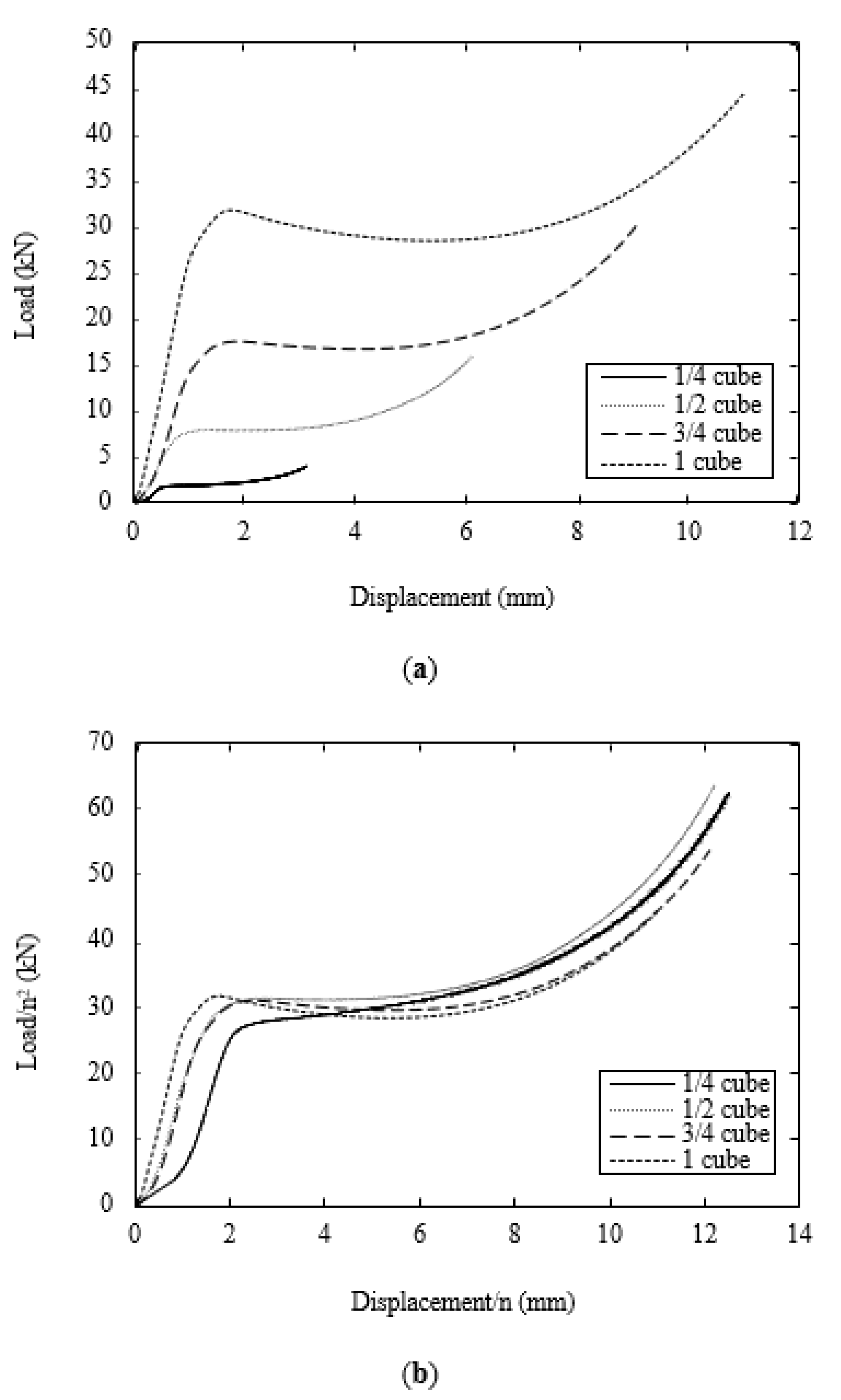
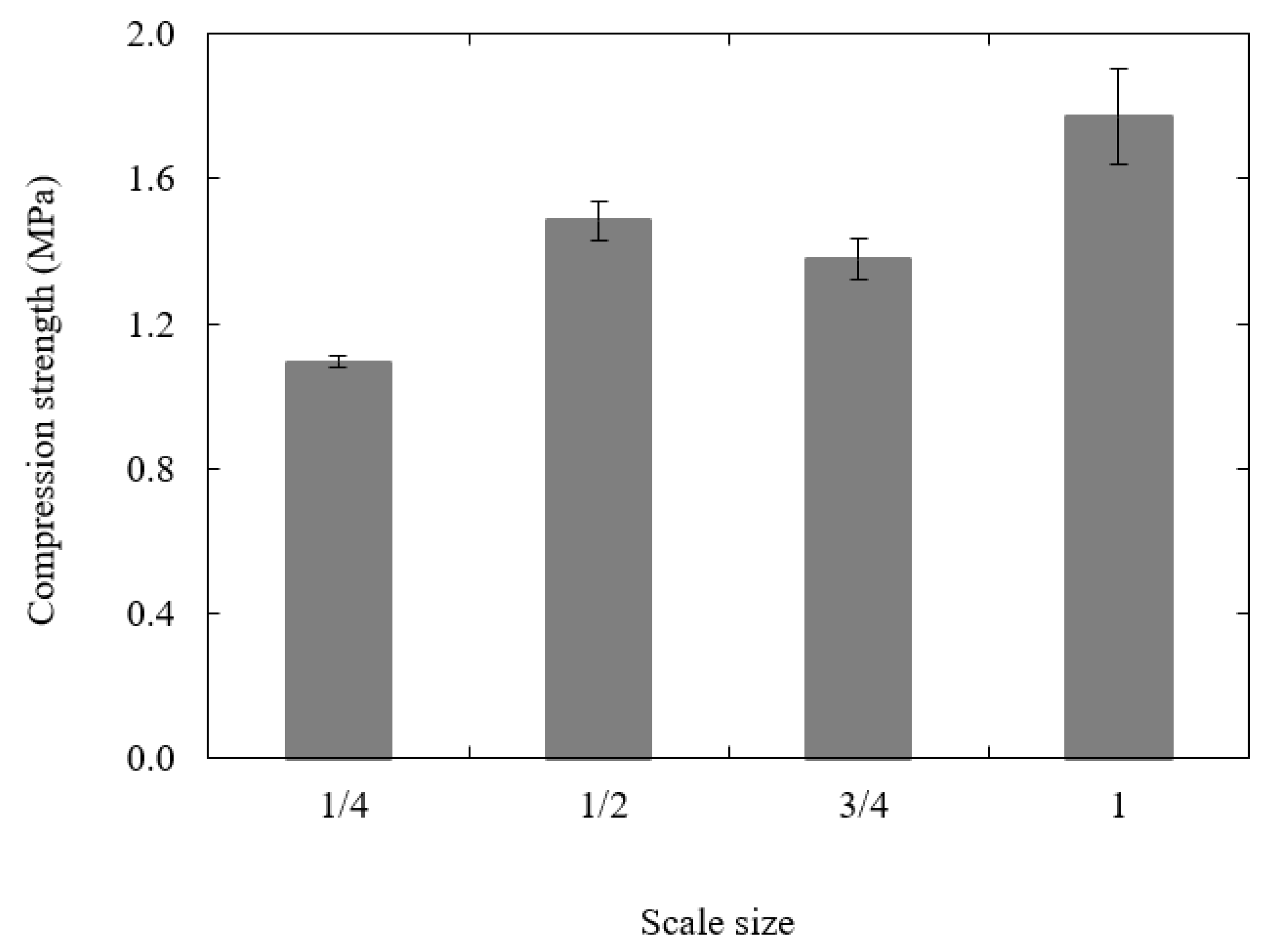
4.2. Compression Tests on the Scaled Solid Cubes
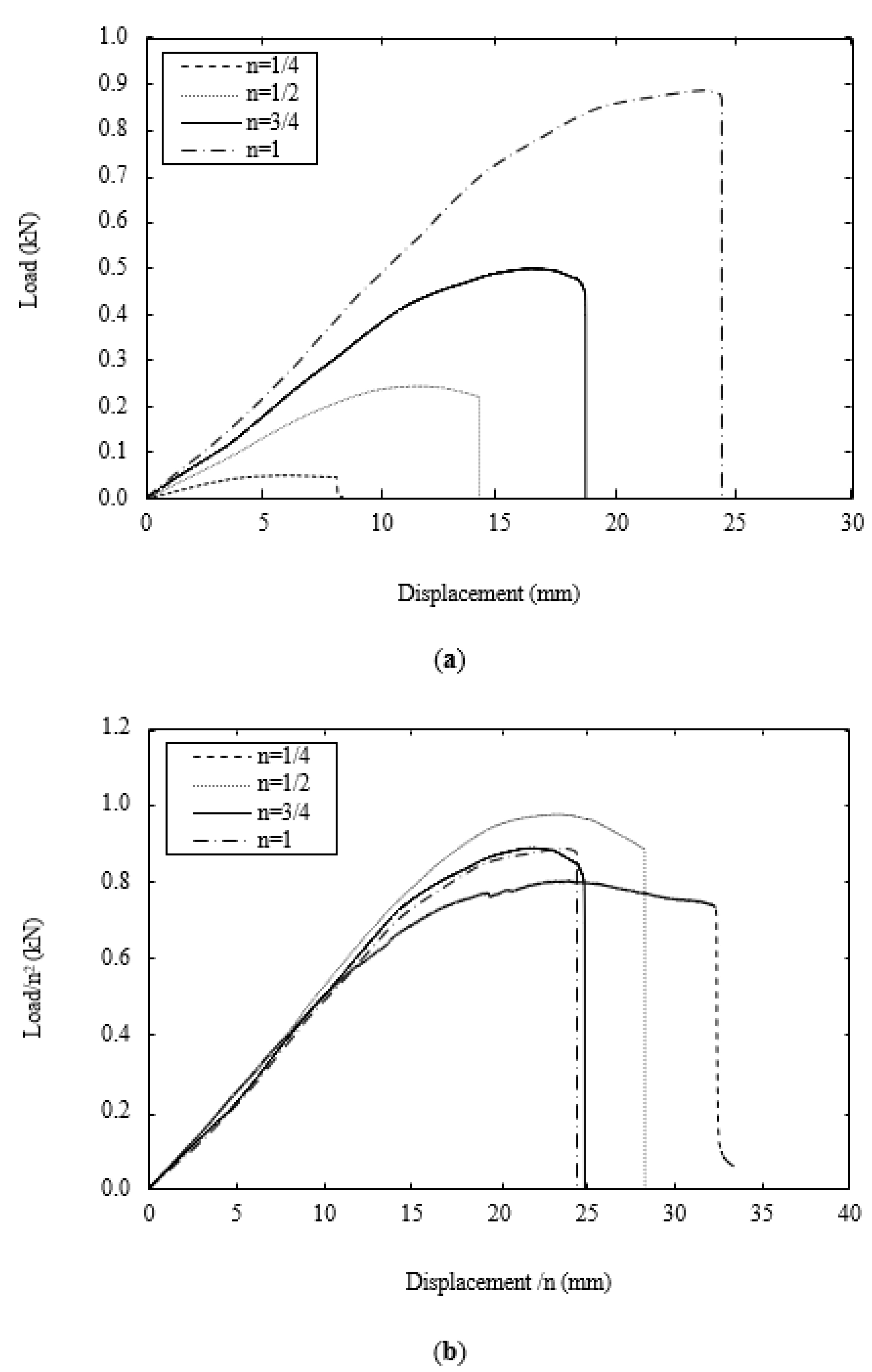
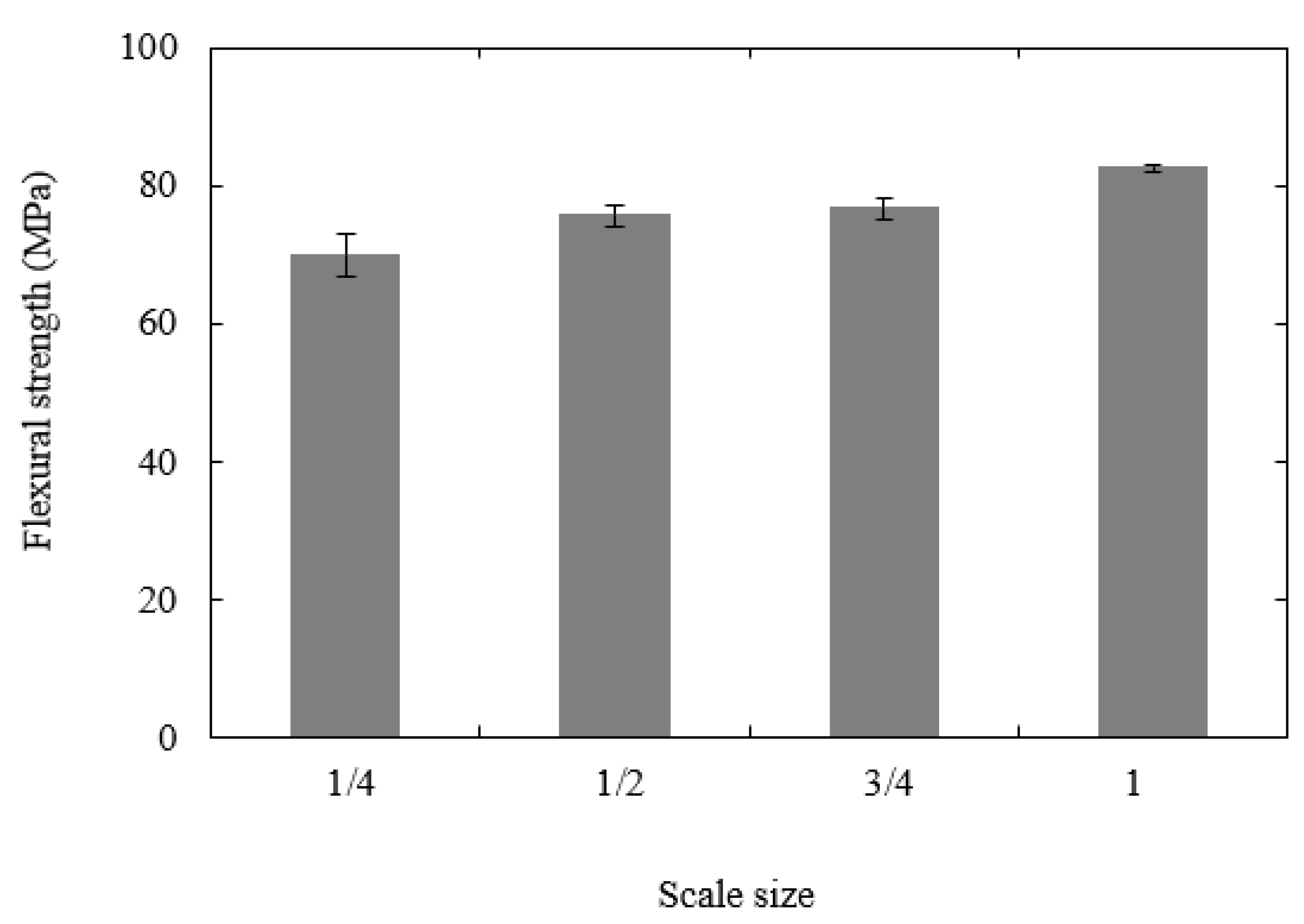
4.3. Flexural Tests on the Scaled Sizes of Rectangular Samples
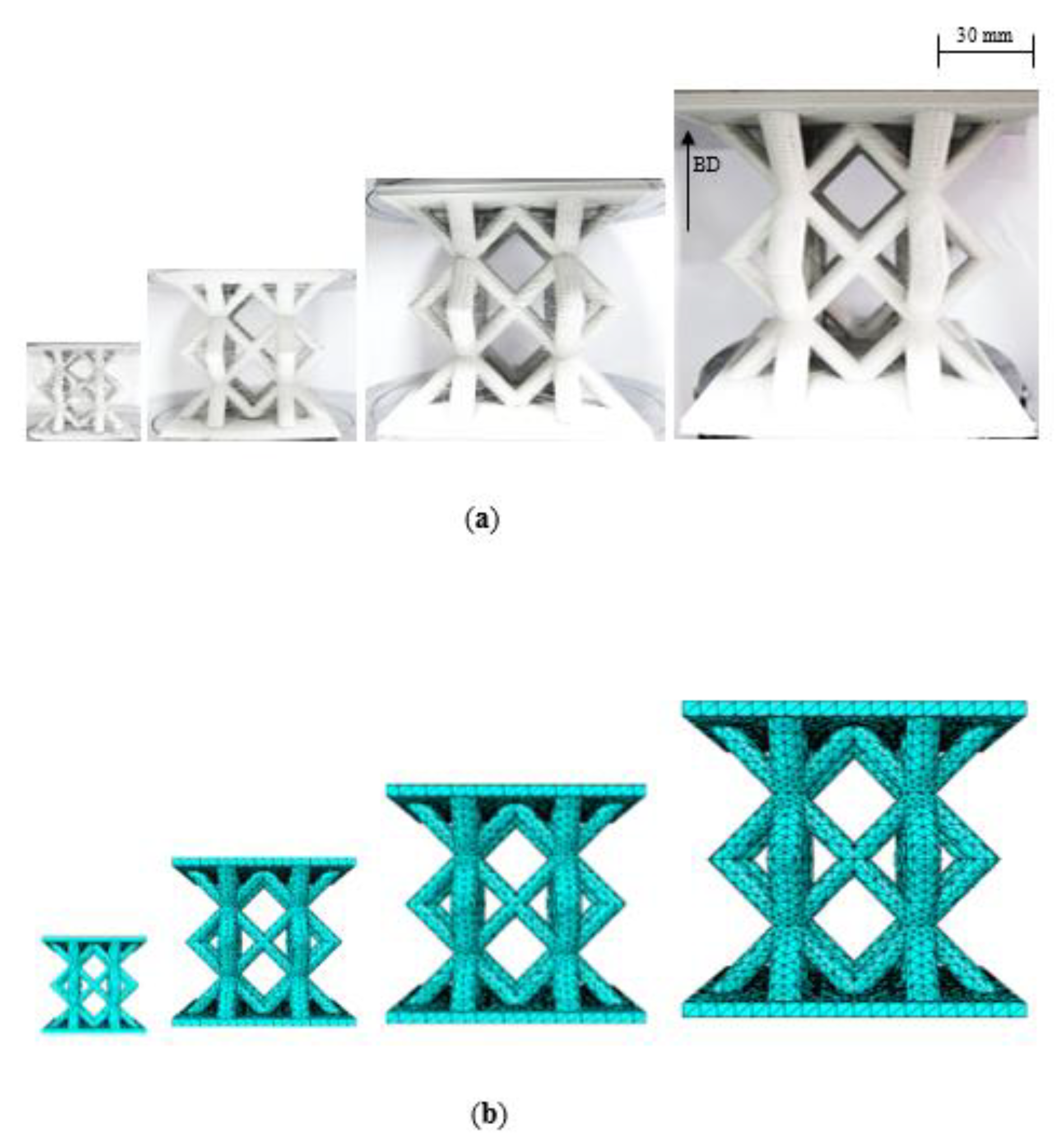
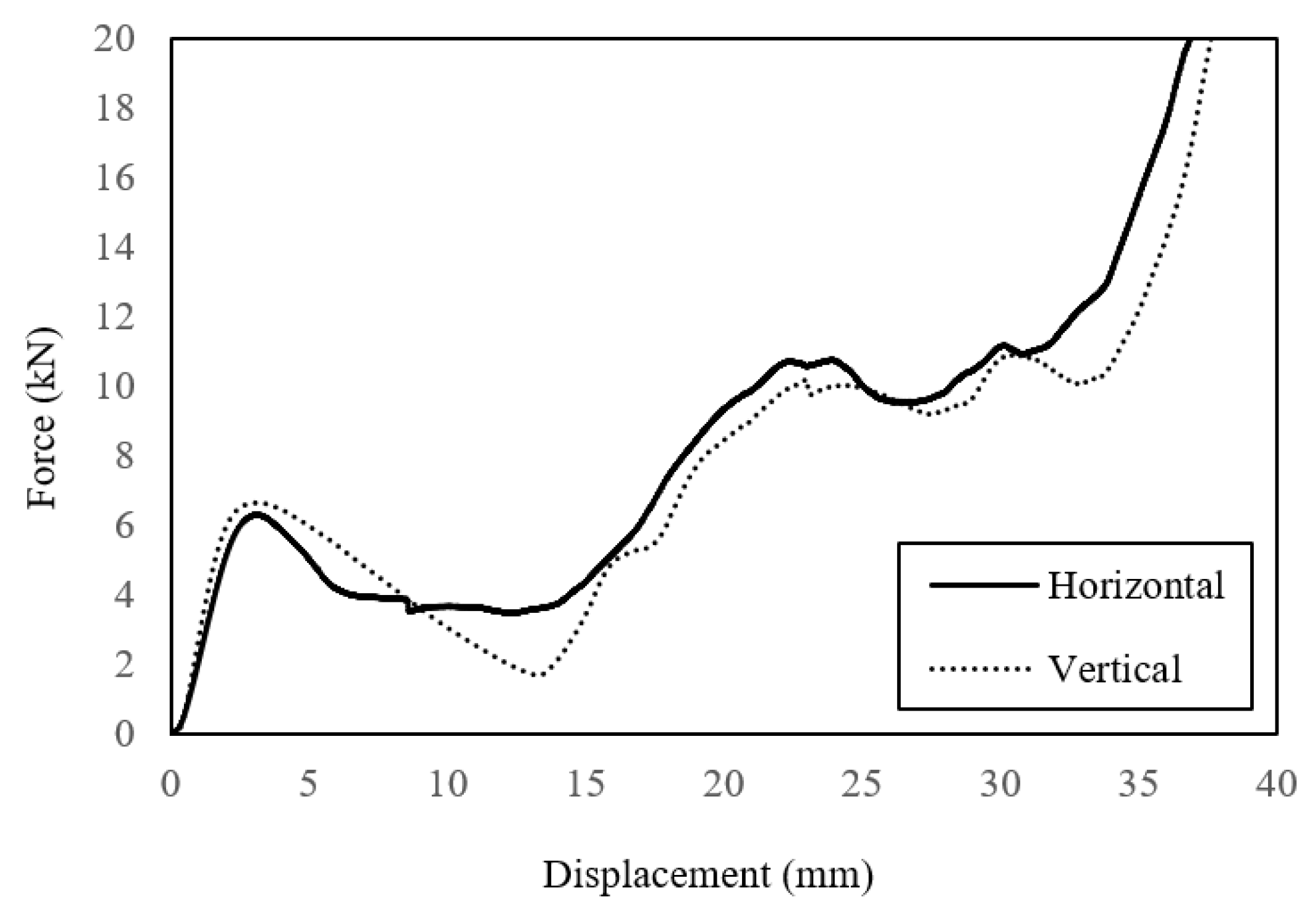
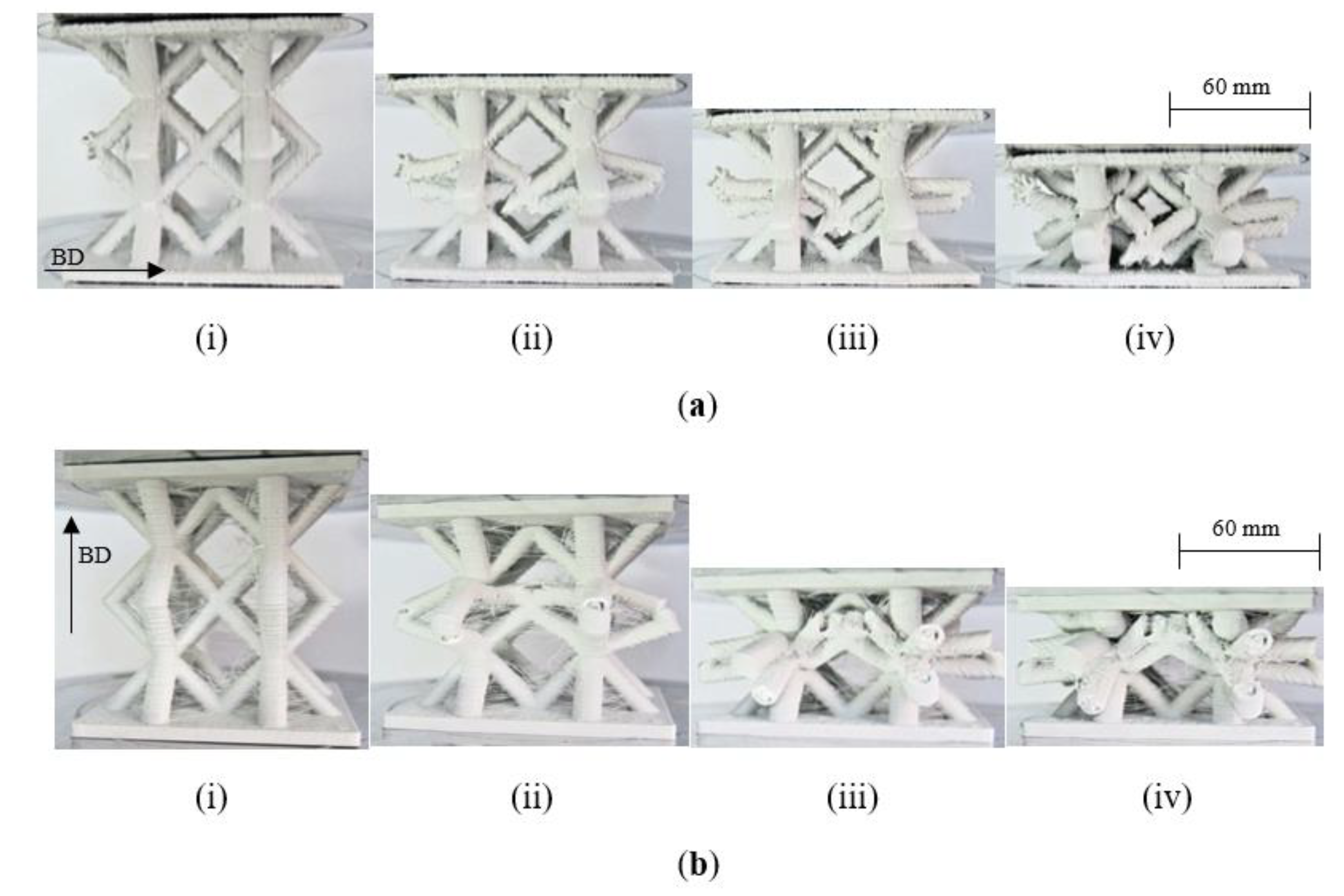
4.4. The Influence of Build Direction on the Properties of the Lattice Structures
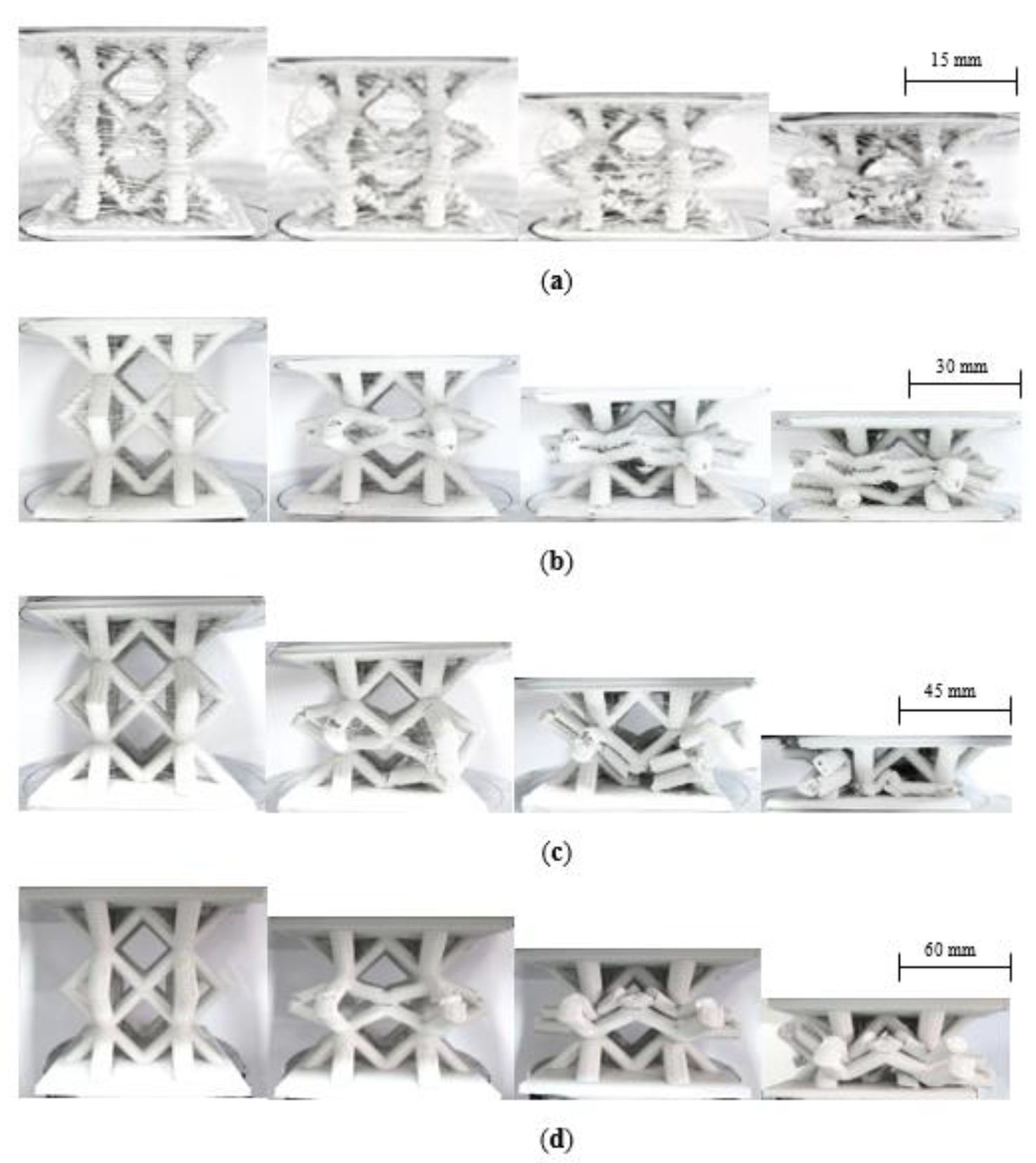
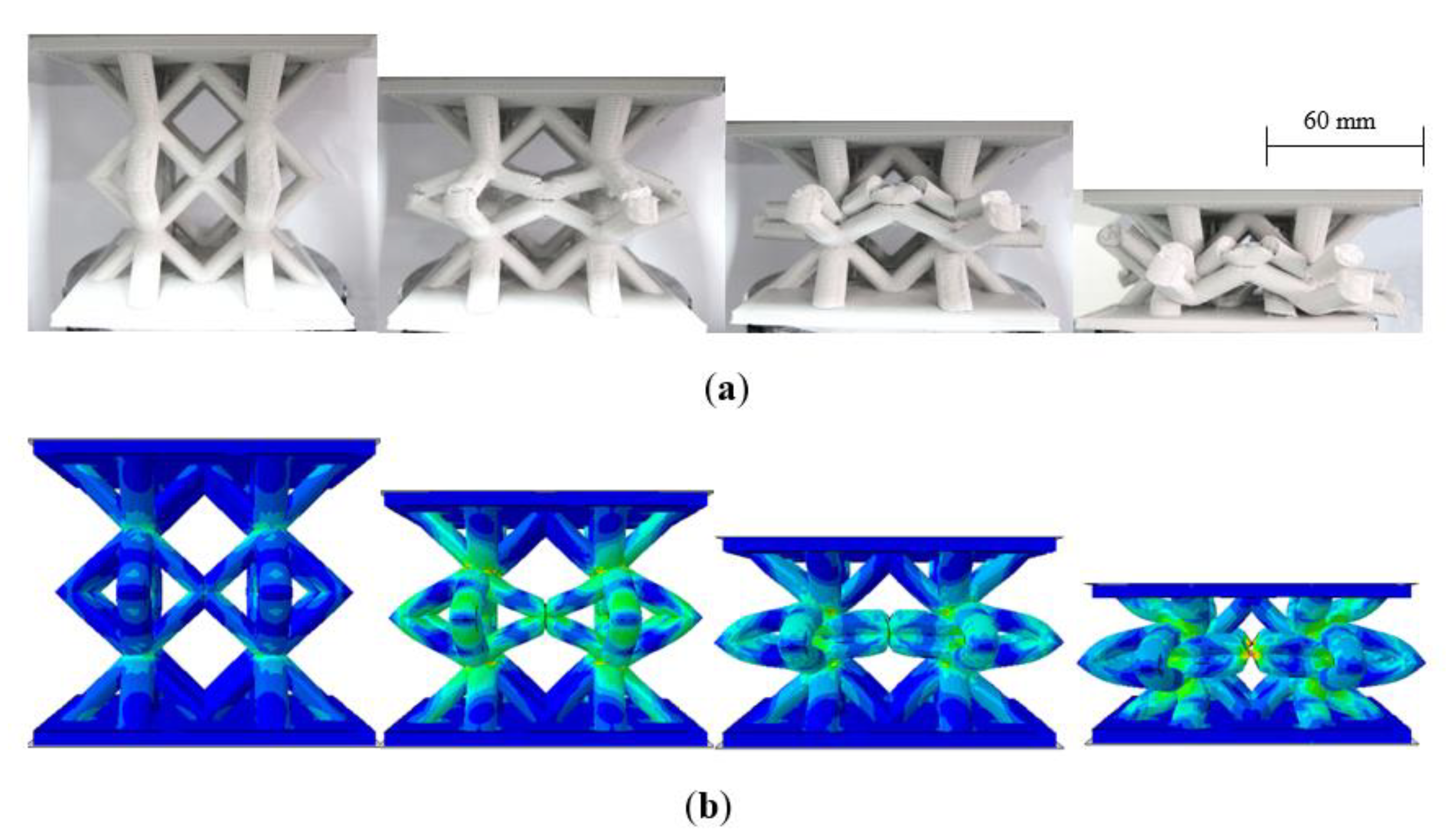
4.5. Compression Tests on the Four Scaled Sizes of Lattice Structure
4.6. Numerical Results
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Van Der Klift, F.; Koga, Y.; Todoroki, A.; Ueda, M.; Hirano, Y.; Matsuzaki, R. 3D Printing of Continuous Carbon Fibre Reinforced Thermo-Plastic (CFRTP) Tensile Test Specimens. Open J. Compos. Mater. 2016, 6, 18–27. [Google Scholar] [CrossRef] [Green Version]
- Campbell, T.A.; Ivanova, O. Additive Manufacturing as a Disruptive Technology: Implications of Three-Dimensional Printing. Technol. Innov. 2013, 15, 67–79. [Google Scholar] [CrossRef]
- Joshi, S.C.; Sheikh, A.A. 3D printing in aerospace and its long-term sustainability. Virtual Phys. Prototyp. 2015, 10, 175–185. [Google Scholar] [CrossRef]
- Fasel, U.; Keidel, D.; Baumann, L.; Cavolina, G.; Eichenhofer, M.; Ermanni, P. Composite additive manufacturing of morphing aerospace structures. Manuf. Lett. 2020, 23, 85–88. [Google Scholar] [CrossRef]
- Garzon-Hernandez, S.; Garcia-Gonzalez, D.; Jérusalem, A.; Arias, A. Design of FDM 3D printed polymers: An experimental-modelling methodology for the prediction of mechanical properties. Mater. Des. 2020, 188, 108414. [Google Scholar] [CrossRef]
- Ngo, T.D.; Kashani, A.; Imbalzano, G.; Nguyen, K.T.Q.; Hui, D. Additive manufacturing (3D printing): A review of materials, methods, applications and challenges. Compos. Part B Eng. 2018, 143, 172–196. [Google Scholar] [CrossRef]
- Attaran, M. The rise of 3-D printing: The advantages of additive manufacturing over traditional manufacturing. Bus. Horiz. 2017, 60, 677–688. [Google Scholar] [CrossRef]
- Sarvestani, H.Y.; Akbarzadeh, A.; Niknam, H.; Hermenean, K. 3D printed architected polymeric sandwich panels: Energy absorption and structural performance. Compos. Struct. 2018, 200, 886–909. [Google Scholar] [CrossRef]
- Compton, B.G.; Lewis, J.A. 3D-Printing of Lightweight Cellular Composites. Adv. Mater. 2014, 26, 5930–5935. [Google Scholar] [CrossRef]
- Kafle, A.; Luis, E.; Silwal, R.; Pan, H.M.; Shrestha, P.L.; Bastola, A.K. 3D/4D Printing of Polymers: Fused Deposition Modelling (FDM), Selective Laser Sintering (SLS), and Stereolithography (SLA). Polymers 2021, 13, 3101. [Google Scholar] [CrossRef]
- Bell, D.; Siegmund, T. 3D-printed polymers exhibit a strength size effect. Addit. Manuf. 2018, 21, 658–665. [Google Scholar] [CrossRef]
- Guessasma, S.; Belhabib, S.; Nouri, H.; Ben Hassana, O. Anisotropic damage inferred to 3D printed polymers using fused deposition modelling and subject to severe compression. Eur. Polym. J. 2016, 85, 324–340. [Google Scholar] [CrossRef]
- Pagano, C.; Rebaioli, L.; Baldi, F.; Fassi, I. Relationships between size and mechanical properties of scaffold-like structures. Mech. Adv. Mater. Struct. 2020, 1–6. [Google Scholar] [CrossRef]
- Wen, H.-M.; Jones, N. Experimental investigation of the scaling laws for metal plates struck by large masses. Int. J. Impact Eng. 1993, 13, 485–505. [Google Scholar] [CrossRef]
- Hamada, H.; Ramakrishna, S. Scaling effects in the energy absorption of carbon-fiber/PEEK composite tubes. Compos. Sci. Technol. 1995, 55, 211–221. [Google Scholar] [CrossRef]
- Jackson, K.E. Scaling effects in the flexural response and failure of composite beams. AIAA J. 1992, 30, 2099–2105. [Google Scholar] [CrossRef]
- Jackson, K.E.; Kellas, S.; Morton, J. Scale Effects in the Response and Failure of Fiber Reinforced Composite Laminates Loaded in Tension and in Flexure. J. Compos. Mater. 1992, 26, 2674–2705. [Google Scholar] [CrossRef]
- Chouchaoui, C.; Parks, P.; Ochoa, O. Similitude study for a laminated cylindrical tube under tension, torsion, bending, internal and external pressure Part II: Scale models. Compos. Struct. 1999, 44, 231–236. [Google Scholar] [CrossRef]
- Tarfaoui, M.; Gning, P.B.; Davies, P.; Collombet, F. Scale and Size Effects on Dynamic Response and Damage of Glass/Epoxy Tubular Structures. J. Compos. Mater. 2007, 41, 547–558. [Google Scholar] [CrossRef] [Green Version]
- Swanson, S.R. Scaling of impact damage in fiber composites from laboratory specimens to structures. Compos. Struct. 1993, 25, 249–255. [Google Scholar] [CrossRef]
- Rezaeepazhand, J.; Simitses, G. Use of scaled-down models for predicting vibration response of laminated plates. Compos. Struct. 1995, 30, 419–426. [Google Scholar] [CrossRef]
- Green, B.; Wisnom, M.; Hallett, S. An experimental investigation into the tensile strength scaling of notched composites. Compos. Part A Appl. Sci. Manuf. 2007, 38, 867–878. [Google Scholar] [CrossRef]
- Carrillo, J.; Cantwell, W. Scaling Effects in the Low Velocity Impact Response of Fiber-Metal Laminates. J. Reinf. Plast. Compos. 2008, 27, 893–907. [Google Scholar] [CrossRef]
- Kashani, M.H.; Sadighi, M.; Mohammadkhah, M.; Alavijeh, H.S. Investigation of scaling effects on fiber metal laminates under tensile and flexural loading. Proc. Inst. Mech. Eng. Part L J. Mater. Des. Appl. 2015, 229, 189–201. [Google Scholar] [CrossRef]
- Bates, S.R.; Farrow, I.R.; Trask, R.S. 3D printed polyurethane honeycombs for repeated tailored energy absorption. Mater. Des. 2016, 112, 172–183. [Google Scholar] [CrossRef] [Green Version]
- Li, T.; Wang, L. Bending behavior of sandwich composite structures with tunable 3D-printed core materials. Compos. Struct. 2017, 175, 46–57. [Google Scholar] [CrossRef]
- Mirkhalaf, S.; Pires, F.A.; Simoes, R. An elasto-viscoplastic constitutive model for polymers at finite strains: Formulation and computational aspects. Comput. Struct. 2016, 166, 60–74. [Google Scholar] [CrossRef] [Green Version]
- Govaert, L.L.; Timmermans, P.P.; Brekelmans, W.M. The Influence of Intrinsic Strain Softening on Strain Localization in Polycarbonate: Modeling and Experimental Validation. J. Eng. Mater. Technol. 1999, 122, 177–185. [Google Scholar] [CrossRef] [Green Version]
- Mirkhalaf, S.M.; Fagerström, M. The mechanical behavior of polylactic acid (PLA) films: Fabrication, experiments and modelling. Mech. Time-Depend. Mater. 2019, 1–13. [Google Scholar] [CrossRef] [Green Version]
- Rezgui, F.; Swistek, M.; Hiver, J.; G’Sell, C.; Sadoun, T. Deformation and damage upon stretching of degradable polymers (PLA and PCL). Polymers 2005, 46, 7370–7385. [Google Scholar] [CrossRef]
- Abeykoon, C.; Sri-Amphorn, P.; Fernando, A. Optimization of fused deposition modeling parameters for improved PLA and ABS 3D printed structures. Int. J. Lightweight Mater. Manuf. 2020, 3, 284–297. [Google Scholar] [CrossRef]
- Gautam, R.; Idapalapati, S.; Feih, S. Printing and characterisation of Kagome lattice structures by fused deposition modelling. Mater. Des. 2018, 137, 266–275. [Google Scholar] [CrossRef]



| Parameter | Details |
|---|---|
| Filament Diameter | 2.85 mm |
| Printing Nozzle Temperature | 205 °C |
| Printing Bed Temperature | 60 °C |
| Nozzle Diameter | 0.6 mm |
| Layer Thickness | 0.5 mm |
| Default Printing Speed | 3000 mm/min. |
| Infill Percentage | 100% |
| Parameter | n | Sample | ||
|---|---|---|---|---|
| Compression | Flexural Test | Lattice | ||
| Length (mm) | ¼ | 5.3 | 50 | 31.8 |
| ½ | 10.5 | 100 | 61.5 | |
| ¾ | 15.6 | 150 | 91.7 | |
| 1 | 20.5 | 200 | 120.2 | |
| Width (mm) | ¼ | 5.3 | 9.6 | 31.9 |
| ½ | 10.5 | 19.8 | 61.5 | |
| ¾ | 15.6 | 30.0 | 91.7 | |
| 1 | 20.5 | 39.6 | 120.2 | |
| Height (mm) | ¼ | 5.3 | 2.3 | 27.4 |
| ½ | 10.5 | 4.7 | 55.3 | |
| ¾ | 15.6 | 6.6 | 82.1 | |
| 1 | 20.5 | 8.6 | 107.1 | |
| Crosshead displacement rate (mm/min) | ¼ | 1 | 2 | 2 |
| ½ | 2 | 4 | 4 | |
| ¾ | 3 | 6 | 6 | |
| 1 | 4 | 8 | 8 | |
| Material Properties of PLA | |
|---|---|
| Young’s modulus in the elastic regime, E (MPa) | 1136 |
| Poisson’s ratio, ν | 0.3 |
| Density, (g/m3) | 1250 |
| The absolute temperature, T (k) | 296.15 (room temperature) |
| The universal gas constant, R (J/mol·K) | 8.3143 |
| The Eyring (yielding) properties/parameters | |
| The scalar /preexponential factor, A0 (s) | 3.65 E-32 |
| The activation energy, ΔH (J/mol) | 2.062 E+5 |
| A characteristic stress, σ0 (MPa) | 5.7 |
| Pressure coefficient, μ | 0.3 |
| The softening parameters | |
| The softening properties, h | 450 |
| The saturation value of softening, D∞ | 2.16 |
| Hardening parameter, H (MPa) | 10 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Aziz, A.R.; Zhou, J.; Thorne, D.; Cantwell, W.J. Geometrical Scaling Effects in the Mechanical Properties of 3D-Printed Body-Centered Cubic (BCC) Lattice Structures. Polymers 2021, 13, 3967. https://doi.org/10.3390/polym13223967
Aziz AR, Zhou J, Thorne D, Cantwell WJ. Geometrical Scaling Effects in the Mechanical Properties of 3D-Printed Body-Centered Cubic (BCC) Lattice Structures. Polymers. 2021; 13(22):3967. https://doi.org/10.3390/polym13223967
Chicago/Turabian StyleAziz, Alia Ruzanna, Jin Zhou, David Thorne, and Wesley James Cantwell. 2021. "Geometrical Scaling Effects in the Mechanical Properties of 3D-Printed Body-Centered Cubic (BCC) Lattice Structures" Polymers 13, no. 22: 3967. https://doi.org/10.3390/polym13223967
APA StyleAziz, A. R., Zhou, J., Thorne, D., & Cantwell, W. J. (2021). Geometrical Scaling Effects in the Mechanical Properties of 3D-Printed Body-Centered Cubic (BCC) Lattice Structures. Polymers, 13(22), 3967. https://doi.org/10.3390/polym13223967






