Preparation and Characterization of CCTO/PDMS Dielectric Elastomers with High Dielectric Constant and Low Dielectric Loss
Abstract
1. Introduction
2. Experimental
2.1. Materials
2.2. Measurements
2.3. Preparation of CCTO/PDMS Dielectric Elastomer
2.4. Preparation of CCTO/PDMS Dielectric Elastomers Modified by Different Silane Coupling Agents
2.5. Manufacturing of Dielectric Elastomer Actuators
3. Results and Discussion
3.1. Characterization of CCTO Particle Microstructure
3.2. Analysis of Microscopic Appearance of Three Modified Particles
3.3. Comparison of the Dispersion of CCTO in PDMS before and after Modification
3.4. Mechanical Properties of Dielectric Elastomer Composites
3.5. Dielectric Properties of Dielectric Elastomer Composites
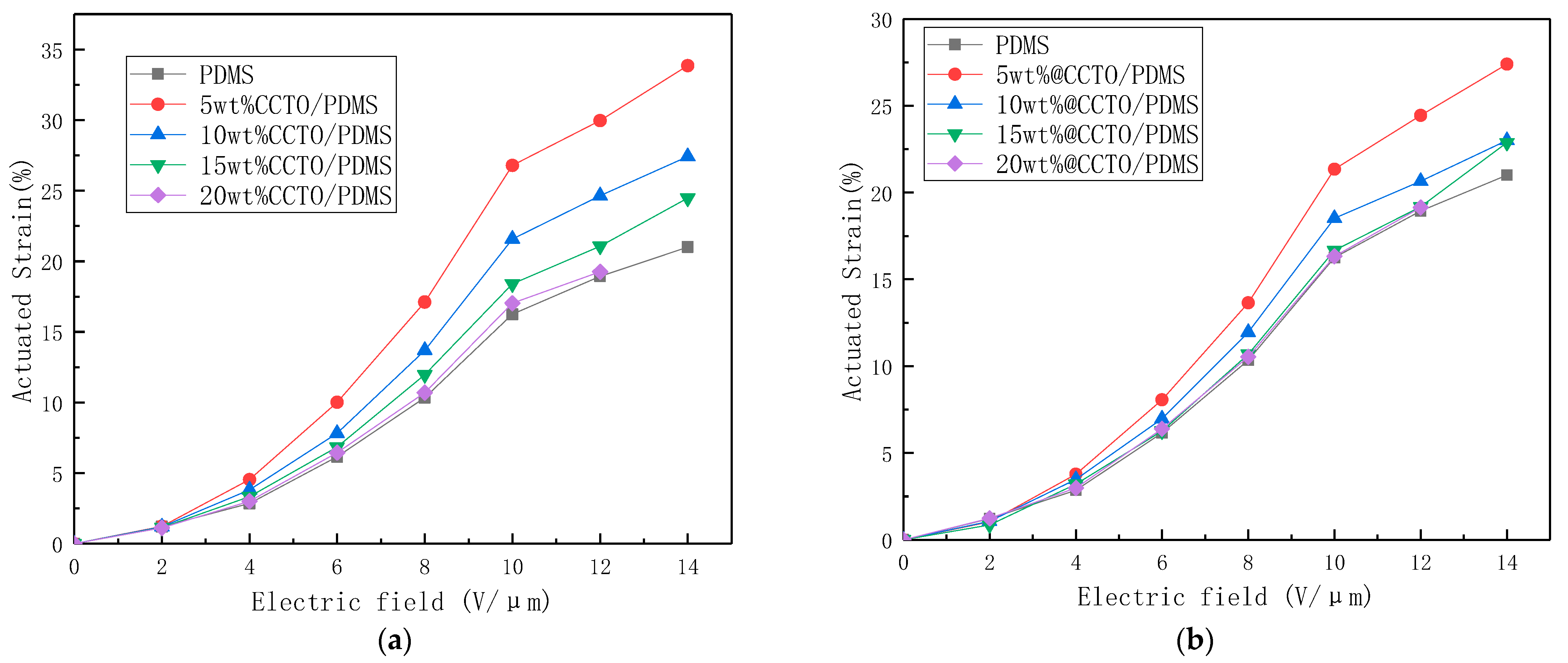
3.6. Electro-Deformation Properties of Dielectric Elastomer Composites
4. Conclusions
- 1.
- The elastic modulus and dielectric constant of the CCTO/PDMS dielectric elastomer are higher than those of the unmodified PDMS, and the conductivity (<10−7) and dielectric loss (0.0038) of the dielectric elastomer did not occur significantly. The strain parameter of 5 wt% CCTO/PDMS is increased by 2.5 times compared with PDMS, reaching 33.8%. However, the growth rate of the dielectric constant of the dielectric elastomer is lower than the growth rate of the elastic modulus. As a result, the strain parameter of the dielectric elastomer decreases as the filler content increases. The electromechanical deformation and electromechanical conversion efficiency of the modified material are higher than those of the unmodified PDMS. However, when the amount of filler is too large, the maximum deformation will decrease due to the decrease of the breakdown field strength (14 V/µm).
- 2.
- Comparing the dispersion effect of CCTO modified by three silane coupling agents (KH550, KH560, KH570) in PMDS, the dispersion effect of CCTO modified by KH560 is the best. While KH560 improves the dispersion of CCTO, it also improves the dielectric constant and elastic modulus of the dielectric elastomer. When the filler content is 20 wt%, the dielectric constant reaches 6.5 (100 Hz), which is an increase of 150% compared with the unmodified PDMS. However, the effect of KH560 on the modulus of elasticity is greater than the effect on the dielectric constant, resulting in its electromechanical conversion efficiency being lower than CCTO/PDMS. In addition, 5 wt% @CCTO/PDMS has the largest strain parameter, which is 1.93 times higher than that of the unmodified PDMS. The maximum amount of deformation reaches 27.4% at a field strength of 14 V/µm.
- 3.
- Through the preparation of CCTO/PDMS composite materials, preparations are made for exploring new research directions and further improving the performance of materials. In the next work, higher performance dielectric elastomers will be pursued.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Chidsey, C.E.D.; Murray, R.W. Electroactive polymers and macromolecular electronics. Science 1986, 231, 25–31. [Google Scholar] [CrossRef]
- Thiele Cathleen, D.A.S. Raghu. Electroactive Polymers and Devices 2013–2018: Forecasts, Technologies, Players; IDTechEx: New York, NY, USA, 2013. [Google Scholar]
- Sheng, J.; Chen, H.; Li, B.; Chang, L. Temperature dependence of the dielectric constant of acrylic dielectric elastomer. Appl. Phys. A 2013, 110, 511–515. [Google Scholar] [CrossRef]
- Jomaa, H.; Bchir, O.J. Dual Epoxy Dielectric and Photosensitive Solder Mask Coatings, and Processes of Making Same. U.S. Patent 8,276,269, 2 October 2012. [Google Scholar]
- Li, X.Q.; Li, W.B.; Zhang, W.M.; Zou, H.X.; Peng, Z.K.; Meng, G. Magnetic force induced tristability for dielectric elastomer actuators. Smart Mater. Struct. 2017, 26, 105007. [Google Scholar] [CrossRef]
- Zhao, P.; Cai, Y.; Liu, C.; Ge, D.; Li, B.; Chen, H. Study on the bio-inspired electrochromic device enabled via dielectric elastomer actuator. Opt. Mater. 2020, 111, 110569. [Google Scholar] [CrossRef]
- Chiba, S. Dielectric Elastomers; Springer: Tokyo, Japan, 2014; pp. 183–195. [Google Scholar]
- Pelrine, R.; Kornbluh, R.; Pei, Q.; Joseph, J. High-speed electrically actuated elastomers with strain greater than 100%. Science 2000, 287, 836–839. [Google Scholar] [CrossRef]
- Lai, W. Characteristics of Dielectric Elastomers and Fabrication of Dielectric Elastomer Actuators for Artificial Muscle Applications. Master’s Thesis, Iowa State University, Ames, IA, USA, 2011. [Google Scholar]
- Kofod, G. Dielectric Elastomer Actuators. Chemistry 2001. Available online: https://core.ac.uk/download/pdf/196530167.pdf (accessed on 30 December 2020).
- Carpi, F.; Migliore, A.; Serra, G.; De Rossi, D. Helical dielectric elastomer actuators. Smart Mater. Struct. 2005, 14, 1210. [Google Scholar] [CrossRef]
- Yang, D.; Huang, S.; Ruan, M.; Li, S.; Wu, Y.; Guo, W.; Zhang, L. Improved electromechanical properties of silicone dielectric elastomer composites by tuning molecular flexibility. Compos. Sci. Technol. 2018, 155, 160–168. [Google Scholar] [CrossRef]
- Goulbourne, N.C.; Mockensturm, E.M.; Frecker, M.I. Electro-elastomers: Large deformation analysis of silicone membranes. Int. J. Solids Struct. 2007, 44, 2609–2626. [Google Scholar] [CrossRef]
- Zhu, J.; Stoyanov, H.; Kofod, G.; Suo, Z. Large deformation and electromechanical instability of a dielectric elastomer tube actuator. J. Appl. Phys. 2010, 108, 074113. [Google Scholar] [CrossRef]
- Gatti, D.; Haus, H.; Matysek, M.; Frohnapfel, B.; Tropea, C.; Schlaak, H.F. The dielectric breakdown limit of silicone dielectric elastomer actuators. Appl. Phys. Lett. 2014, 104, 052905. [Google Scholar] [CrossRef]
- Kumar, A.; Patra, K.; Hossain, M. Silicone composites cured under a high electric field: An electromechanical experimental study. Polym. Compos. 2021, 42, 914–930. [Google Scholar] [CrossRef]
- Romasanta, L.J.; Leret, P.; Casaban, L.; Hernández, M.; Miguel, A.; Fernández, J.F.; Kenny, J.M.; Lopez-Manchado, M.A.; Verdejo, R. Towards materials with enhanced electro-mechanical response: CaCu3Ti4O12-polydimethylsiloxane composites. J. Mater. Chem. 2012, 22, 24705–24712. [Google Scholar] [CrossRef]
- Gallone, G.; Carpi, F.; De Rossi, D.; Levita, G.; Marchetti, A. Dielectric constant enhancement in a silicone elastomer filled with lead magnesium niobate–lead titanate. Mater. Sci. Eng. C Biomim. Supramol. Syst. 2007, 27, 110–116. [Google Scholar] [CrossRef]
- Galantini, F.; Bianchi, S.; Castelvetro, V.; Gallone, G. Functionalized carbon nanotubes as a filler for dielectric elastomer composites with improved actuation performance. Smart Mater. Struct. 2013, 22, 055025. [Google Scholar] [CrossRef]
- Mashingboon, C.; Thongbai, P.; Maensiri, S.; Yamwong, T.; Seraphin, S. Synthesis and giant dielectric behavior of CaCu3Ti4O12 ceramics prepared by polymerized complex method. Mater. Chem. Phys. 2008, 109, 262–270. [Google Scholar] [CrossRef]
- Byeong, K.K.; Hyung, S.L.; Lee, J.W.; Seung, E.L.; Yong, S.C. Dielectric and grain-boundary characteristics of hot pressed CaCu3Ti4O12. J. Am. Ceram. Soc. 2010, 93, 2419–2422. [Google Scholar]
- Wang, C.; Xu, F.; He, M.; Ding, L.; Li, S.; Wei, J. Castor oil-based polyurethane/silica nanocomposites: Morphology, thermal and mechanical properties. Polym. Compos. 2018, 39, E1800–E1806. [Google Scholar] [CrossRef]
- Li, H.; Wang, C.; Guo, Z.; Wang, H.; Zhang, Y.; Hong, R.; Peng, Z. Effects of silane coupling agents on the electrical properties of silica/epoxy nanocomposites[C]//2016 IEEE international conference on dielectrics (ICD). IEEE 2016, 2, 1036–1039. [Google Scholar]
- Kang, J.S.; Yu, C.L.; Zhang, F.A. Effect of Silane Modified SiO2 Particles on Poly (MMA-HEMA) Soap-Free Emulsion Polymerization. 2009. Available online: https://www.researchgate.net/publication/279553513_Effect_of_silane_modified_SiO2_Particles_on_PolyMMA-HEMA_Soap-free_Emulsion_Polymerization (accessed on 30 December 2020).
- Sun, Y.; Fang, X.; Ma, Z.; Xu, L.; Lu, Y.; Yu, Q.; Yuan, N.; Ding, J. Enhanced UV-light stability of organometal halide perovskite solar cells with interface modification and a UV absorption layer. J. Mater. Chem. C 2017, 5, 8682–8687. [Google Scholar] [CrossRef]
- Liu, G.; Chen, Y.; Gong, M.; Liu, X.; Cui, Z.K.; Pei, Q.; Gu, J.; Huang, C.; Zhuang, Q. Enhanced dielectric performance of PDMS-based three-phase percolative nanocomposite films incorporating a high dielectric constant ceramic and conductive multi-walled carbon nanotubes. J. Mater. Chem. C 2018, 6, 10829–10837. [Google Scholar] [CrossRef]
- Wang, G.L.; Zhang, Y.Y.; Duan, L.; Ding, K.H.; Wang, Z.F.; Zhang, M. Property reinforcement of silicone dielectric elastomers filled with self-prepared calcium copper titanate particles. J. Appl. Polym. Sci. 2015, 132. [Google Scholar] [CrossRef]
- Zhang, Y.Y.; Wang, G.L.; Zhang, J.; Ding, K.H.; Wang, Z.F.; Zhang, M. Preparation and properties of core-shell structured calcium copper titanate@ polyaniline/silicone dielectric elastomer actuators. Polym. Compos. 2019, 40, E62–E68. [Google Scholar] [CrossRef]
- Wissler, M.; Mazza, E. Electromechanical coupling in dielectric elastomer actuators. Sens. Actuators A Phys. 2007, 138, 384–393. [Google Scholar] [CrossRef]
- Dorfmann, A.; Ogden, R.W. Nonlinear electroelasticity. Acta Mech. 2005, 174, 167–183. [Google Scholar] [CrossRef]
- Suo, Z. Theory of dielectric elastomers. Acta Mech. Solida Sin. 2010, 23, 549–578. [Google Scholar] [CrossRef]
- Bustamante. Transversely isotropic non-linear electro-active elastomers. Acta Mech. 2009, 206, 237–259. [Google Scholar] [CrossRef]
- Mehnert, M.; Hossain, M.; Steinmann, P. Experimental and numerical investigations of the electro-viscoelastic behavior of VHB 4905TM. Eur. J. Mech. A/Solids 2019, 77, 103797. [Google Scholar] [CrossRef]
- Hossain, M. Modelling the curing process in particle-filled electro-active polymers with a dispersion anisotropy. Contin. Mech. Thermodyn. 2020, 32, 351–367. [Google Scholar] [CrossRef]
- Pelrine, R.E.; Kornbluh, R.D.; Joseph, J.P. Electrostriction of polymer dielectrics with compliant electrodes as a means of actuation. Sens. Actuators A Phys. 1998, 64, 77–85. [Google Scholar] [CrossRef]
- Sahu, R.K.; Saini, A.; Ahmad, D.; Patra, K.; Szpunar, J. Estimation and validation of maxwell stress of planar dielectric elastomer actuators. J. Mech. Sci. Technol. 2016, 30, 429–436. [Google Scholar] [CrossRef]



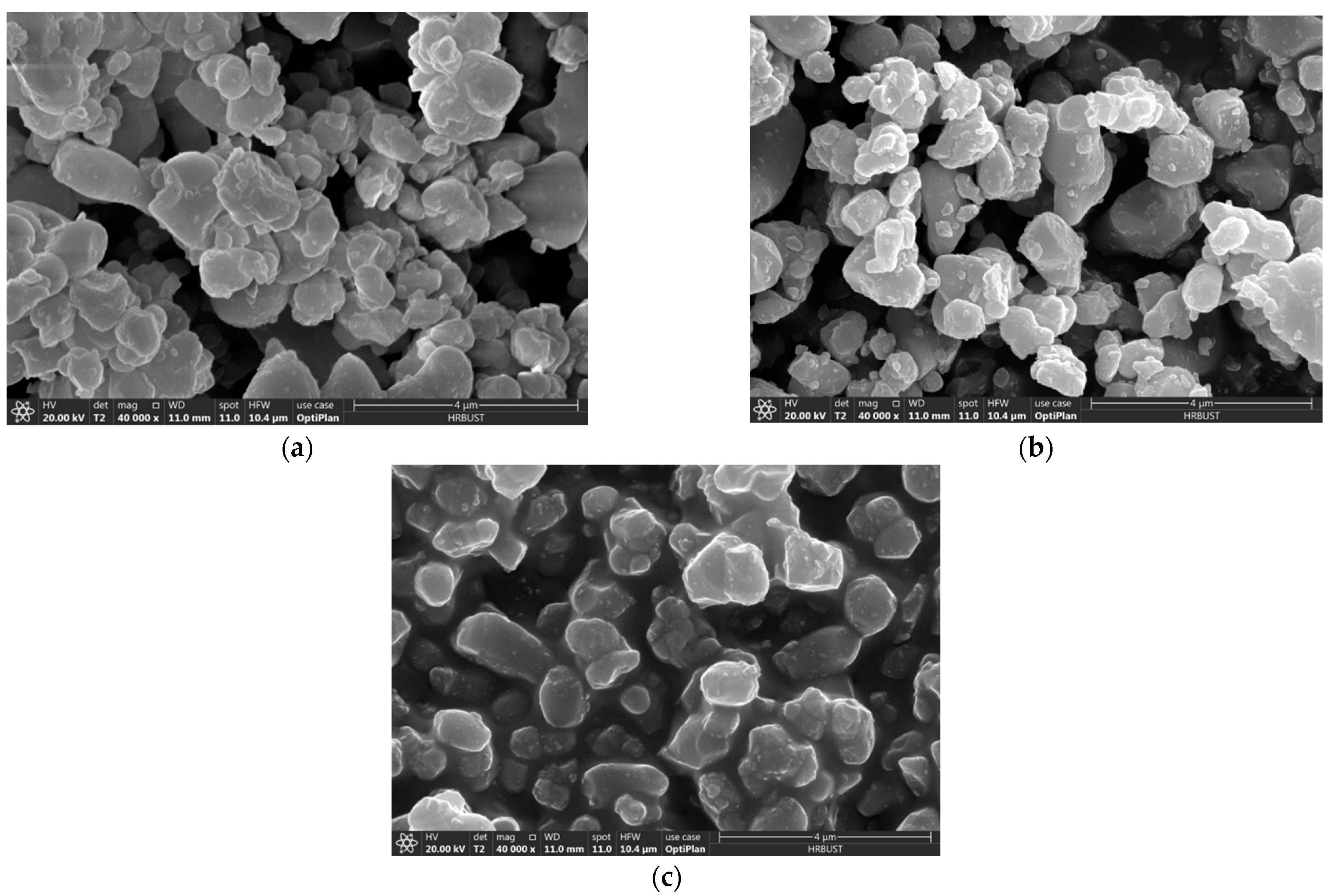
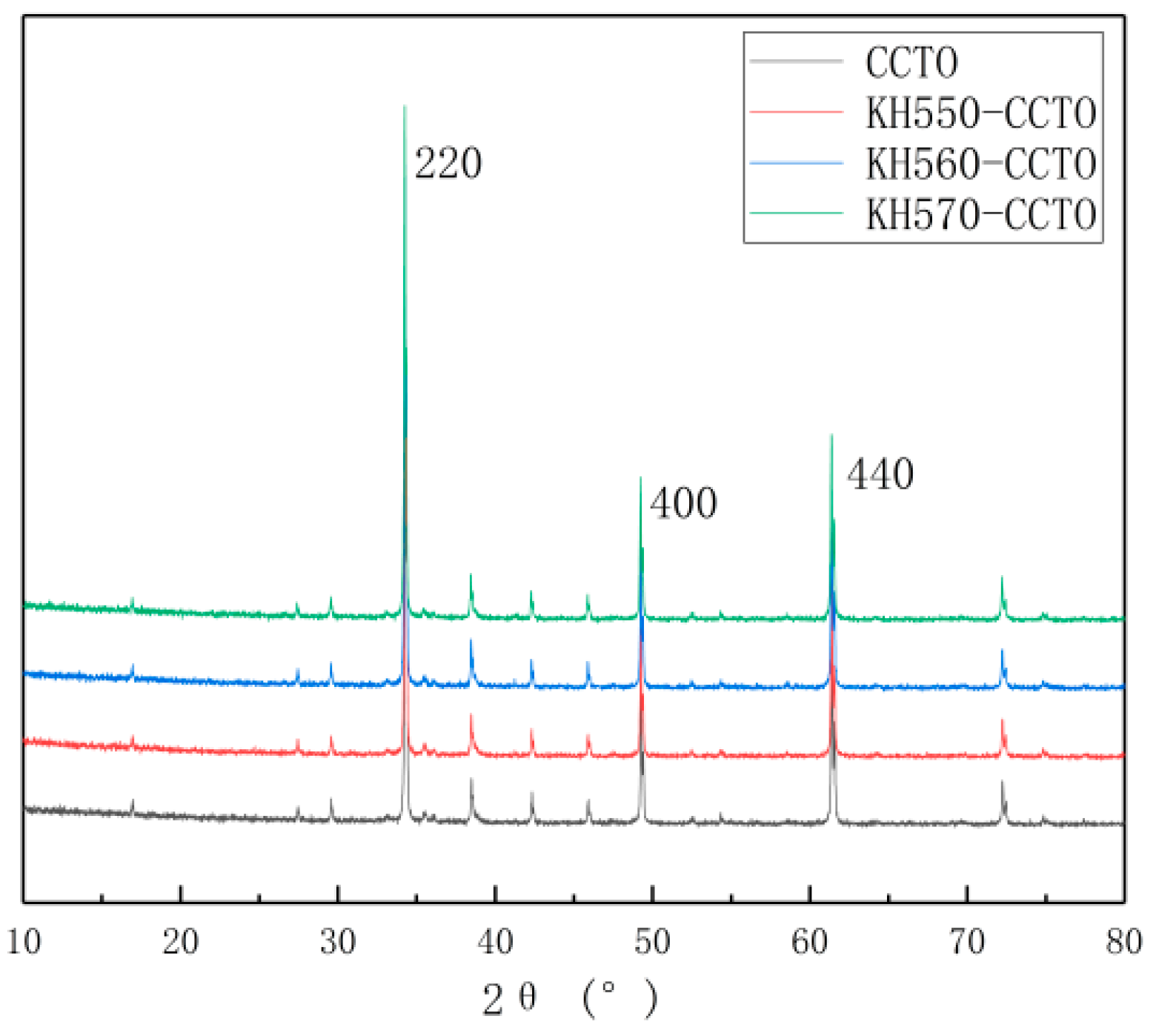
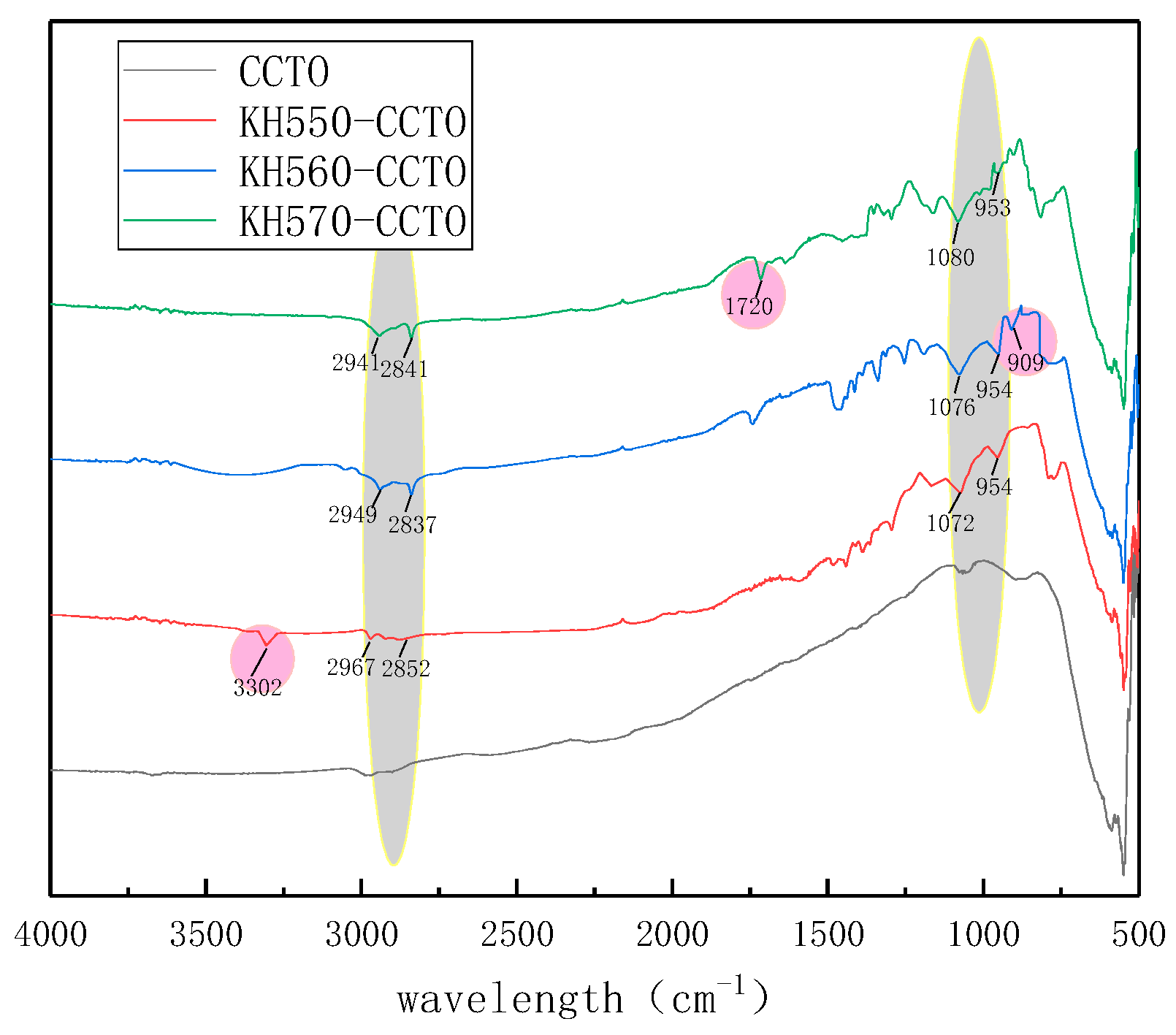
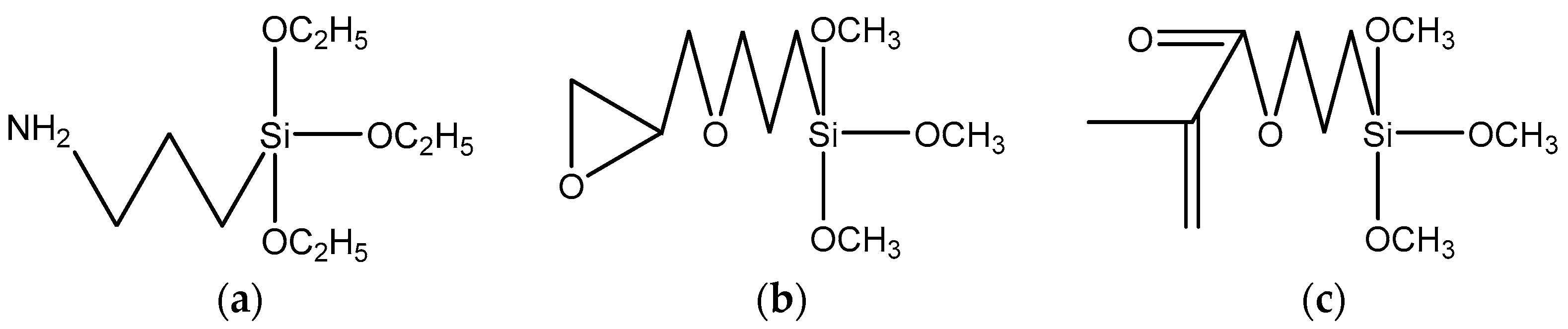
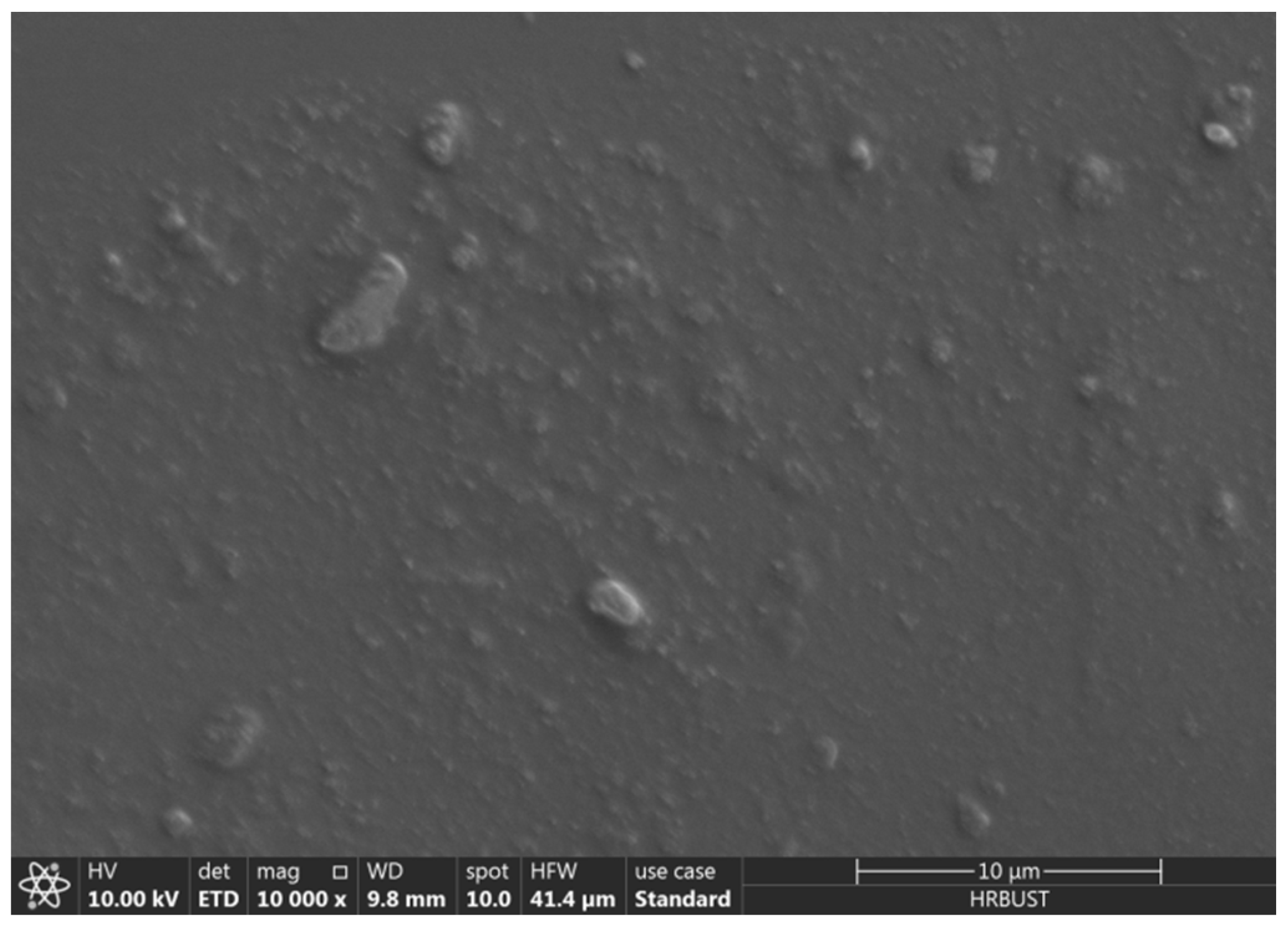
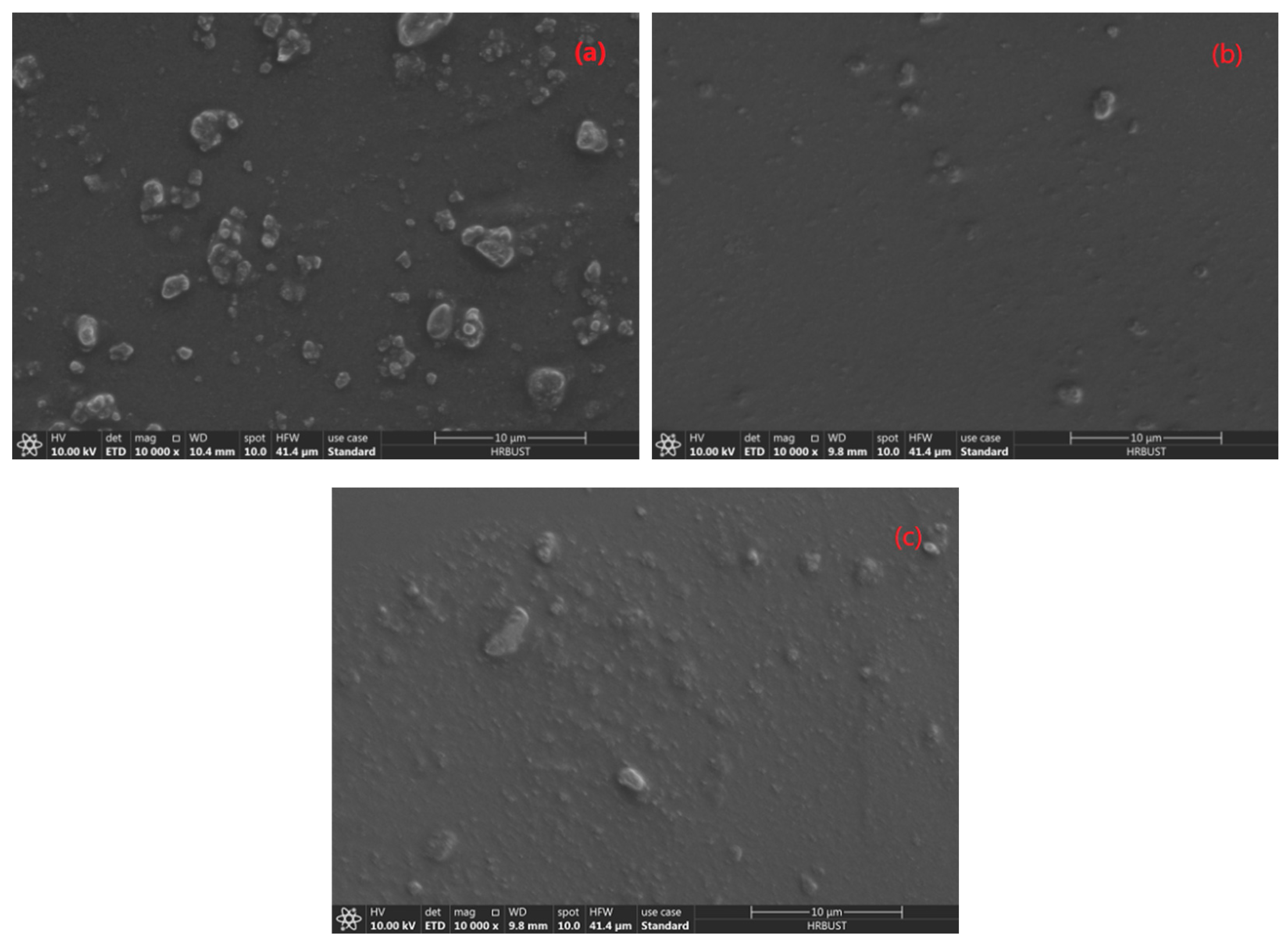




| Element | CCTO | KH550-CCTO | KH560-CCTO | KH570-CCTO |
|---|---|---|---|---|
| C | × | √ | √ | √ |
| O | × | √ | √ | √ |
| Si | × | √ | √ | √ |
| Ca | √ | √ | √ | √ |
| Ti | √ | √ | √ | √ |
| Cu | √ | √ | √ | √ |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, W.; Ren, G.; Zhou, M.; Deng, W. Preparation and Characterization of CCTO/PDMS Dielectric Elastomers with High Dielectric Constant and Low Dielectric Loss. Polymers 2021, 13, 1075. https://doi.org/10.3390/polym13071075
Wang W, Ren G, Zhou M, Deng W. Preparation and Characterization of CCTO/PDMS Dielectric Elastomers with High Dielectric Constant and Low Dielectric Loss. Polymers. 2021; 13(7):1075. https://doi.org/10.3390/polym13071075
Chicago/Turabian StyleWang, Wenqi, Guanguan Ren, Ming Zhou, and Wei Deng. 2021. "Preparation and Characterization of CCTO/PDMS Dielectric Elastomers with High Dielectric Constant and Low Dielectric Loss" Polymers 13, no. 7: 1075. https://doi.org/10.3390/polym13071075
APA StyleWang, W., Ren, G., Zhou, M., & Deng, W. (2021). Preparation and Characterization of CCTO/PDMS Dielectric Elastomers with High Dielectric Constant and Low Dielectric Loss. Polymers, 13(7), 1075. https://doi.org/10.3390/polym13071075






