Crystallinity and β Phase Fraction of PVDF in Biaxially Stretched PVDF/PMMA Films
Abstract
1. Introduction
2. Experimental Section
2.1. Materials
2.2. Preparation of PVDF/PMMA Pellets
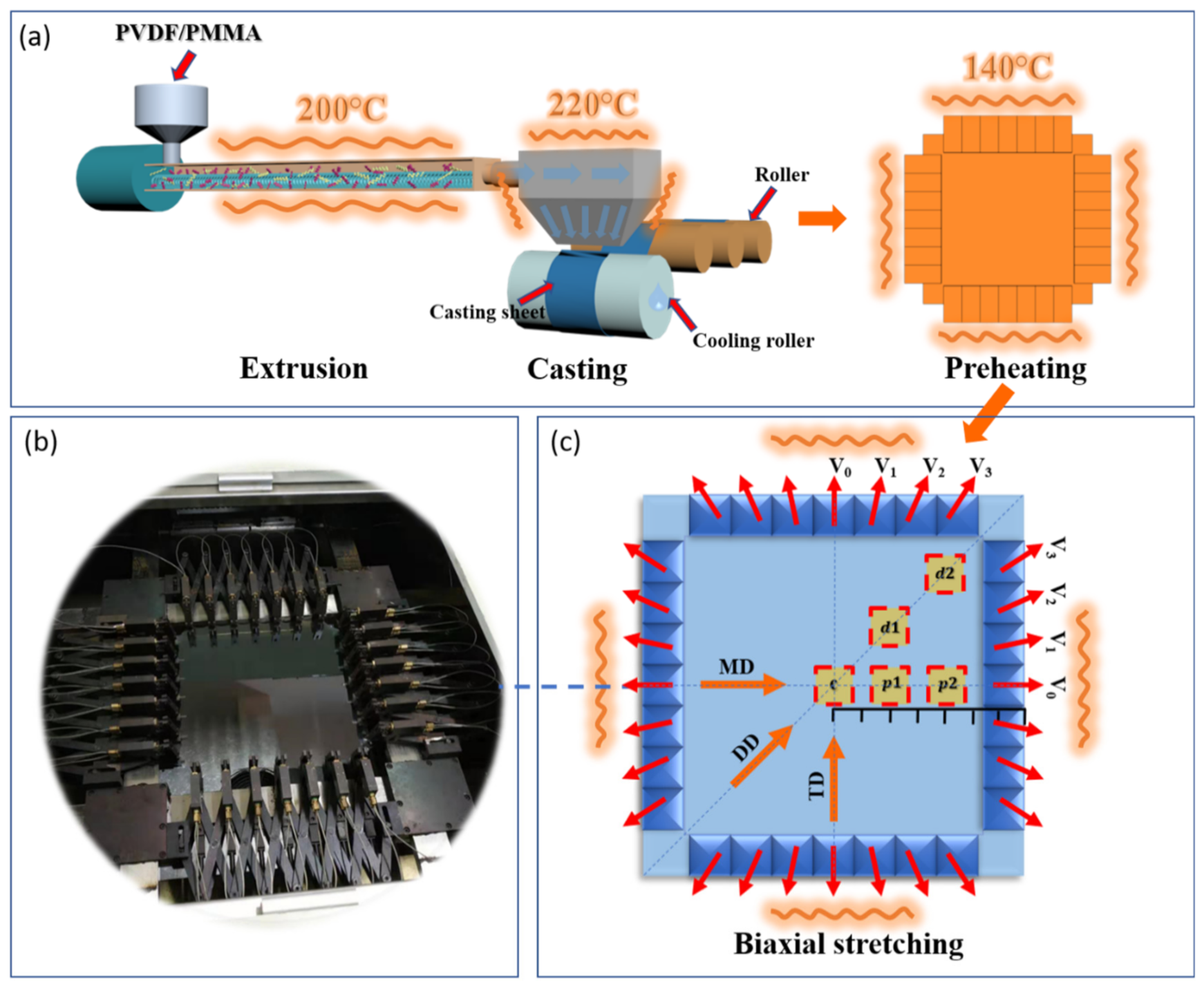
2.3. Preparation of PVDF/PMMA Casting Sheets
2.4. Biaxial Stretching of PVDF/PMMA Films
2.5. Characterizations
3. Result and Discussions
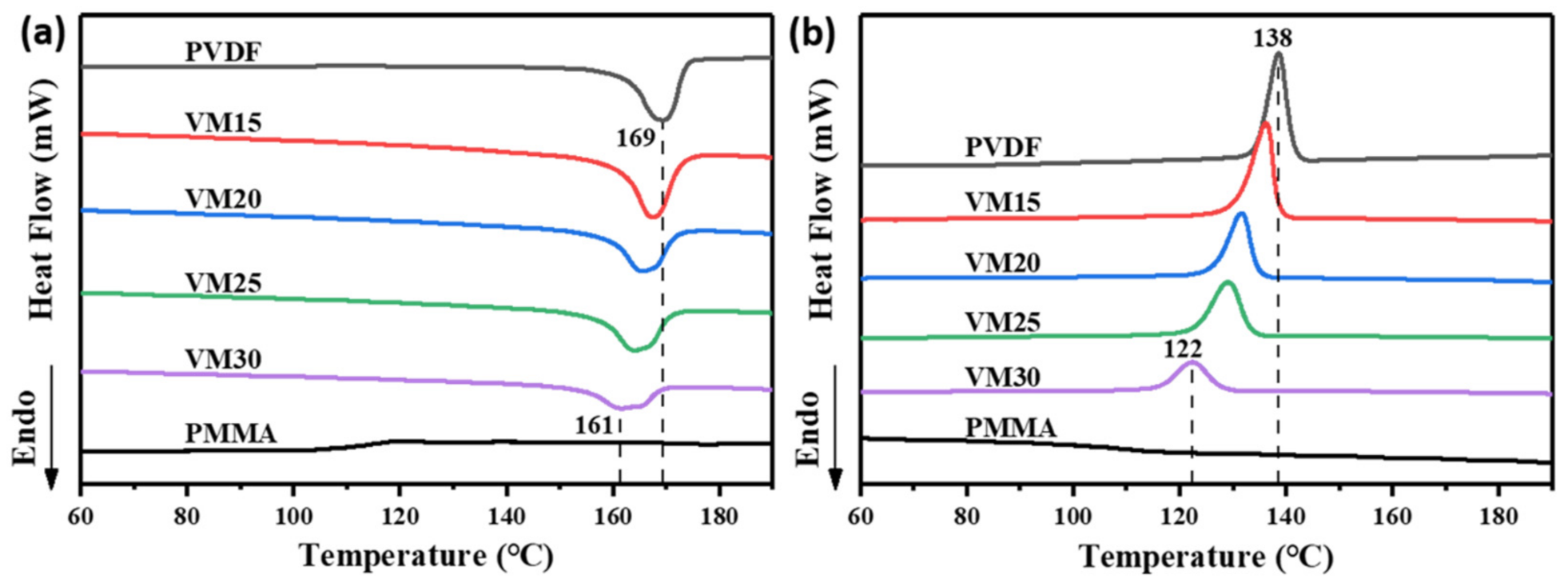
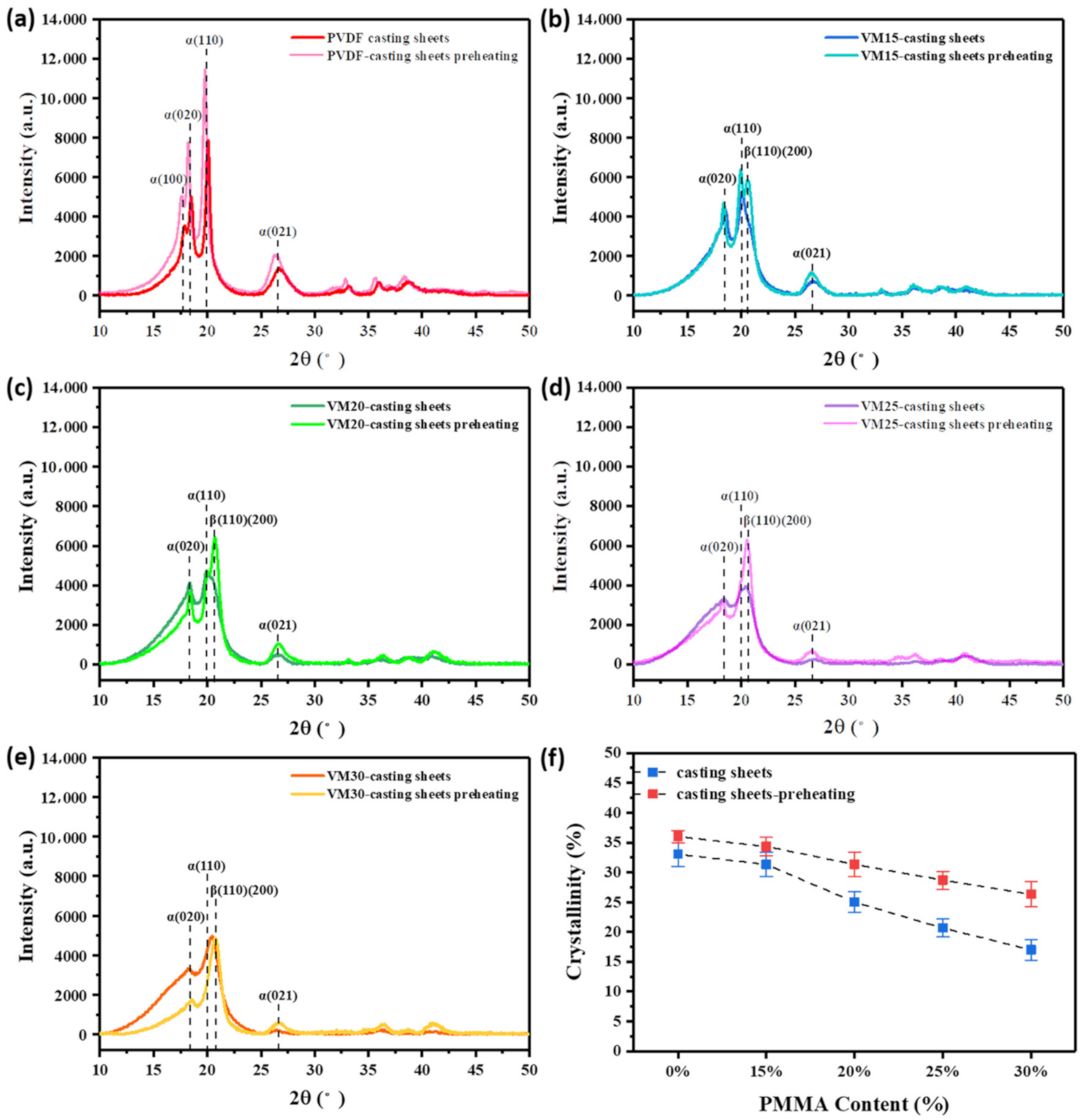
3.1. β Phase Fractions and Crystallinities of PVDF during Extrusion and Casting
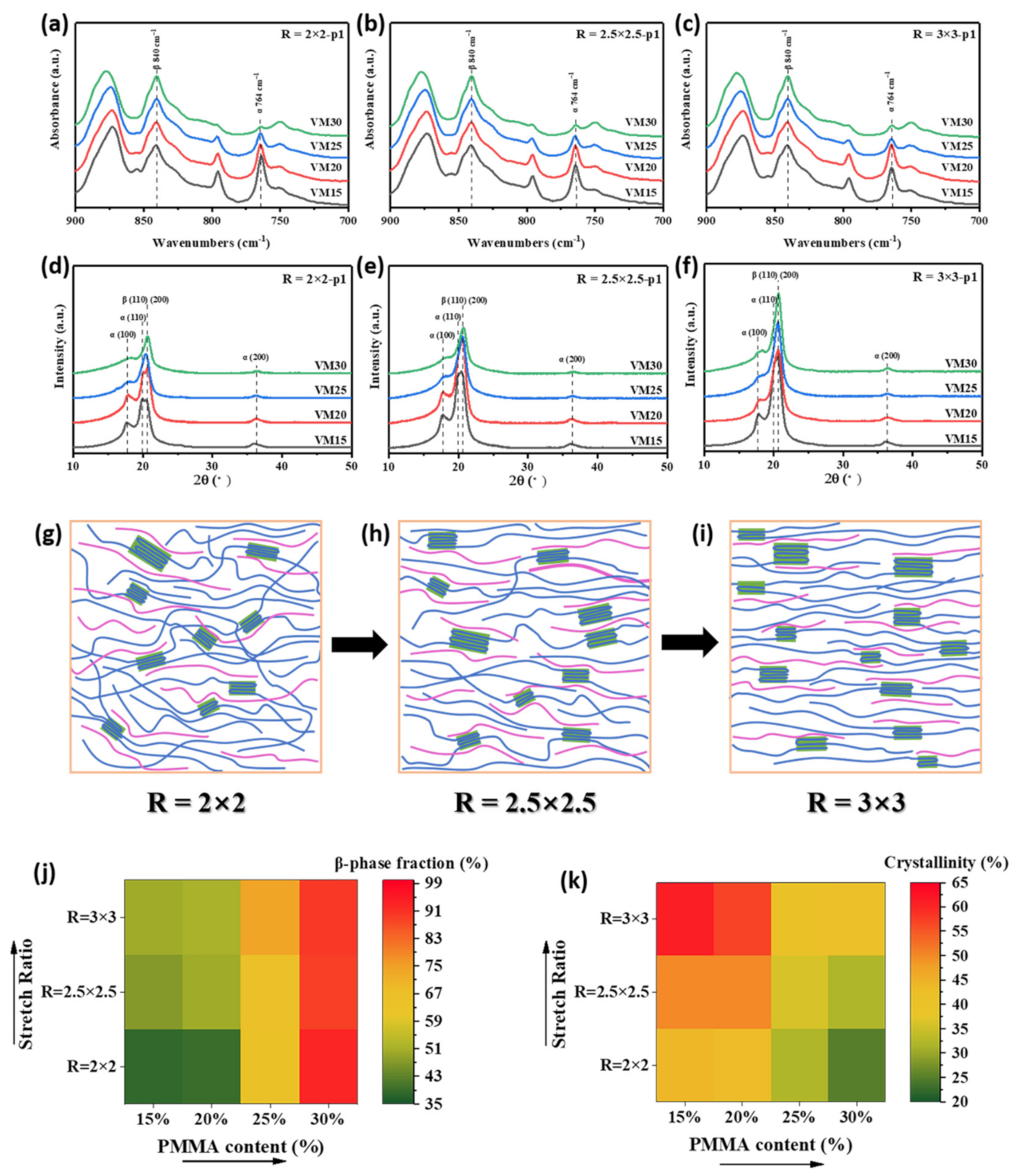
3.2. β Phase Fractions and Crystallinities of PVDF during Biaxial Stretching
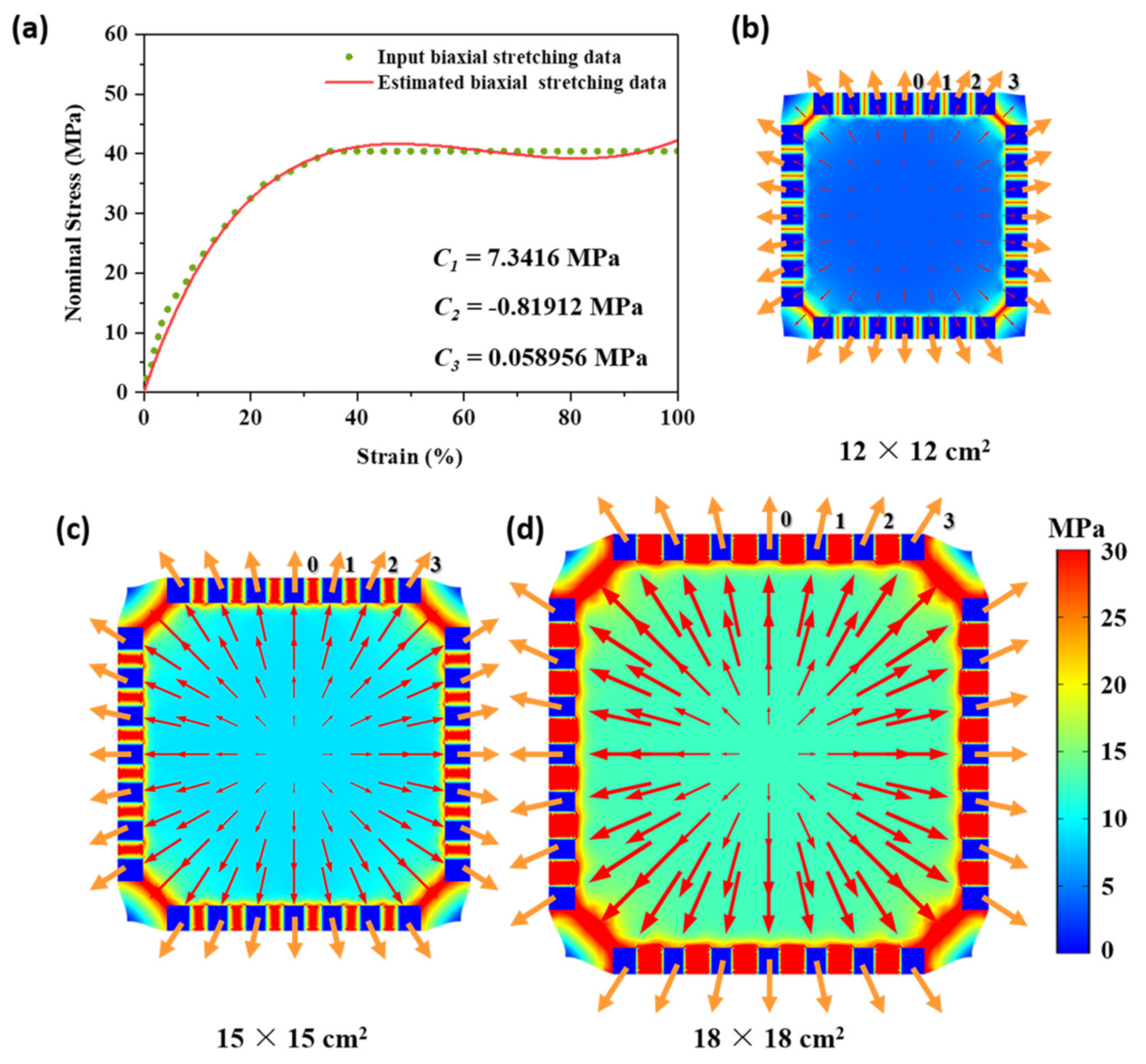
3.3. Distribution of β Phase Fractions and Crystallinities of PVDF on Biaxially Stretched Films
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Lee, M.; Chen, C.Y.; Wang, S.; Cha, S.N.; Park, Y.J.; Kim, J.M.; Chou, L.J.; Wang, Z.L. A Hybrid Piezoelectric Structure for Wearable Nanogenerators. Adv. Mater. 2012, 24, 1759–1764. [Google Scholar] [CrossRef]
- Wu, H.; Huang, Y.; Xu, F.; Duan, Y.; Yin, Z. Energy Harvesters for Wearable and Stretchable Electronics: From Flexibility to Stretchability. Adv. Mater. 2016, 28, 9881–9919. [Google Scholar] [CrossRef]
- Cha, S.; Kim, S.M.; Kim, H.; Ku, J.; Sohn, J.I.; Park, Y.J.; Song, B.G.; Jung, M.H.; Lee, E.K.; Choi, B.L.; et al. Porous PVDF As Effective Sonic Wave Driven Nanogenerators. Nano Lett. 2011, 11, 5142–5147. [Google Scholar] [CrossRef] [PubMed]
- Chang, C.; Tran, V.H.; Wang, J.; Fuh, Y.K.; Lin, L. Direct-Write Piezoelectric Polymeric Nanogenerator with High Energy Conversion Efficiency. Nano Lett. 2010, 10, 726–731. [Google Scholar] [CrossRef]
- Salimi, A.; Yousefi, A.A. FTIR studies of β-phase crystal formation in stretched PVDF films. Polym. Test. 2003, 22, 699–704. [Google Scholar] [CrossRef]
- Giannetti, E. Semi-crystalline fluorinated polymers. Polym. Int. 2001, 50, 10–26. [Google Scholar] [CrossRef]
- Correia, H.M.G.; Ramos, M.M.D. Quantum modelling of poly(vinylidene fluoride). Comput. Mater. Sci. 2005, 33, 224–229. [Google Scholar] [CrossRef]
- Martins, P.; Costa, C.M.; Benelmekki, M.; Botelho, G.; Lanceros-Mendez, S. On the origin of the electroactive poly(vinylidene fluoride) β-phase nucleation by ferrite nanoparticles via surface electrostatic interactions. CrystEngComm 2012, 14, 2807–2811. [Google Scholar] [CrossRef]
- Pan, H.; Na, B.; Lv, R.; Li, C.; Zhu, J.; Yu, Z. Polar phase formation in poly(vinylidene fluoride) induced by melt annealing. J. Polym. Sci. Part B Polym. Phys. 2012, 50, 1433–1437. [Google Scholar] [CrossRef]
- Li, Y.; Zhang, G.; Song, S.; Xu, H.; Pan, M.; Zhong, G.J. How Chain Intermixing Dictates the Polymorphism of PVDF in Poly(vinylidene fluoride)/Polymethylmethacrylate Binary System during Recrystallization: A Comparative Study on Core(-)Shell Particles and Latex Blend. Polymers 2017, 9, 448. [Google Scholar] [CrossRef]
- Zhou, X.; Cakmak, M. Phase Behavior of Rapidly Quenched PVDF/PMMA Blends as Characterized by Raman Spectroscopy, X-ray Diffraction and Thermal Techniques. J. Macromol. Sci. Part B 2007, 46, 667–682. [Google Scholar] [CrossRef]
- Zhou, X.; Cakmak, M. Stress–strain behavior as related to surface topography and thickness uniformity in uni- and biaxially stretched PVDF/PMMA blends. Polym. Eng. Sci. 2007, 47, 2110–2117. [Google Scholar] [CrossRef]
- Koseki, Y.; Aimi, K.; Ando, S. Crystalline structure and molecular mobility of PVDF chains in PVDF/PMMA blend films analyzed by solid-state 19F MAS NMR spectroscopy. Polym. J. 2012, 44, 757–763. [Google Scholar] [CrossRef]
- Sun, J.; Yao, L.; Zhao, Q.-L.; Huang, J.; Song, R.; Ma, Z.; He, L.-H.; Huang, W.; Hao, Y.-M. Modification on crystallization of poly(vinylidene fluoride) (PVDF) by solvent extraction of poly(methyl methacrylate) (PMMA) in PVDF/PMMA blends. Front. Mater. Sci. 2011, 5, 388–400. [Google Scholar] [CrossRef]
- Li, M.; Stingelin, N.; Michels, J.J.; Spijkman, M.-J.; Asadi, K.; Feldman, K.; Blom, P.W.M.; De Leeuw, D.M. Ferroelectric Phase Diagram of PVDF:PMMA. Macromolecules 2012, 45, 7477–7485. [Google Scholar] [CrossRef]
- Cheng, J.; Zhang, J.; Wang, X. Investigation on crystallization behavior and hydrophilicity of poly(vinylidene fluoride)/poly(methyl methacrylate)/poly(vinyl pyrrolidone) ternary blends by solution casting. J. Appl. Polym. Sci. 2013, 127, 3997–4005. [Google Scholar] [CrossRef]
- Mi, C.; Ren, Z.; Li, H.; Yan, S.; Sun, X. Synergistic Effect of Hydrogen Bonds and Diffusion on the β-Crystallization of Poly(vinylidene fluoride) On Poly(methyl methacrylate) Interface. Ind. Eng. Chem. Res. 2019, 58, 7389–7396. [Google Scholar] [CrossRef]
- Yan, J.; Liu, M.; Jeong, Y.G.; Kang, W.; Li, L.; Zhao, Y.; Deng, N.; Cheng, B.; Yang, G. Performance enhancements in poly(vinylidene fluoride)-based piezoelectric nanogenerators for efficient energy harvesting. Nano Energy 2019, 56, 662–692. [Google Scholar] [CrossRef]
- Martins, P.; Caparros, C.; Gonçalves, R.; Benelmekki, M.; Botelho, G.; Lanceros-Mendez, S. Role of Nanoparticle Surface Charge on the Nucleation of the Electroactive β-Poly(vinylidene fluoride) Nanocomposites for Sensor and Actuator Applications. J. Phys. Chem. C 2012, 116, 15790–15794. [Google Scholar] [CrossRef]
- Ouyang, Z.-W.; Chen, E.-C.; Wu, T.-M. Enhanced piezoelectric and mechanical properties of electroactive polyvinylidene fluoride/iron oxide composites. Mater. Chem. Phys. 2015, 149-150, 172–178. [Google Scholar] [CrossRef]
- Wu, Y.; Hsu, S.L.; Honeker, C.; Bravet, D.J.; Williams, D.S. The Role of Surface Charge of Nucleation Agents on the Crystallization Behavior of Poly(vinylidene fluoride). J. Phys. Chem. B 2012, 116, 7379–7388. [Google Scholar] [CrossRef]
- Mendes, S.F.; Costa, C.M.; Caparros, C.; Sencadas, V.; Lanceros-Méndez, S. Effect of filler size and concentration on the structure and properties of poly(vinylidene fluoride)/BaTiO3 nanocomposites. J. Mater. Sci. 2011, 47, 1378–1388. [Google Scholar] [CrossRef]
- Patro, T.U.; Mhalgi, M.V.; Khakhar, D.V.; Misra, A. Studies on poly(vinylidene fluoride)–clay nanocomposites: Effect of different clay modifiers. Polymer 2008, 49, 3486–3499. [Google Scholar] [CrossRef]
- Mahale, B.; Bodas, D.; Gangal, S.A. Study of β-phase development in spin-coated PVDF thick films. Bull. Mater. Sci. 2017, 40, 569–575. [Google Scholar] [CrossRef]
- Kim, J.; Lee, J.H.; Ryu, H.; Lee, J.-H.; Khan, U.; Kim, H.; Kwak, S.S.; Kim, S.-W. High-Performance Piezoelectric, Pyroelectric, and Triboelectric Nanogenerators Based on P(VDF-TrFE) with Controlled Crystallinity and Dipole Alignment. Adv. Funct. Mater. 2017, 1700702. [Google Scholar] [CrossRef]
- Chen, S.; Li, X.; Yao, K.; Tay, F.E.H.; Kumar, A.; Zeng, K. Self-polarized ferroelectric PVDF homopolymer ultra-thin films derived from Langmuir–Blodgett deposition. Polymer 2012, 53, 1404–1408. [Google Scholar] [CrossRef]
- Lei, T.; Yu, L.; Zheng, G.; Wang, L.; Wu, D.; Sun, D. Electrospinning-induced preferred dipole orientation in PVDF fibers. J. Mater. Sci. 2015, 50, 4342–4347. [Google Scholar] [CrossRef]
- Kudyukov, E.V.; Terziyan, T.V.; Antonov, I.D.; Balymov, K.G.; Safronov, A.P.; Vas’Kovskiy, V.O. Phase composition, crystalline structure and piezoelectric properties of thin films of polyvinylidene fluoride obtained by the spin-coating method. Prog. Org. Coat. 2020, 147, 105857. [Google Scholar] [CrossRef]
- Cardoso, V.F.; Costa, C.M.; Minas, G.; Lanceros-Mendez, S. Improving the optical and electroactive response of poly(vinylidene fluoride–trifluoroethylene) spin-coated films for sensor and actuator applications. Smart Mater. Struct. 2012, 21, 85020. [Google Scholar] [CrossRef]
- Cardoso, V.F.; Minas, G.; Costa, C.M.; Tavares, C.J.; Lanceros-Mendez, S. Micro and nanofilms of poly(vinylidene fluoride) with controlled thickness, morphology and electroactive crystalline phase for sensor and actuator applications. Smart Mater. Struct. 2011, 20, 87002. [Google Scholar] [CrossRef]
- Scheinbeim, J.; Nakafuku, C.; Newman, B.A.; Pae, K.D. High-pressure crystallization of poly(vinylidene fluoride). J. Appl. Phys. 1979, 50, 4399–4405. [Google Scholar] [CrossRef]
- Meng, N.; Ren, X.; Santagiuliana, G.; Ventura, L.; Zhang, H.; Wu, J.; Yan, H.; Reece, M.J.; Bilotti, E. Ultrahigh β-phase content poly(vinylidene fluoride) with relaxor-like ferroelectricity for high energy density capacitors. Nat. Commun. 2019, 10, 4535. [Google Scholar] [CrossRef]
- Gradys, A.; Sajkiewicz, P.; Adamovsky, S.; Minakov, A.; Schick, C. Crystallization of poly(vinylidene fluoride) during ultra-fast cooling. Thermochim. Acta 2007, 461, 153–157. [Google Scholar] [CrossRef]
- Ting, Y.S.; Prapto, S.; Chiu, C.-W.; Gunawan, H. Characteristic analysis of biaxially stretched PVDF thin films. J. Appl. Polym. Sci. 2018, 135, 46677. [Google Scholar] [CrossRef]
- Silva, M.P.; Martins, R.S.; Carvalho, H.; Nobrega, J.M.; Lanceros-Mendez, S. Extrusion of poly(vinylidene fluoride) recycled filaments: Effect of the processing cycles on the degree of crystallinity and electroactive phase content. Polym. Test. 2013, 32, 1041–1044. [Google Scholar] [CrossRef]
- Satyanarayana, K.C.; Bolton, K. Molecular dynamics simulations of α- to β-poly(vinylidene fluoride) phase change by stretching and poling. Polymer 2012, 53, 2927–2934. [Google Scholar] [CrossRef]
- Li, L.; Zhang, M.; Rong, M.; Ruan, W. Studies on the transformation process of PVDF from α to β phase by stretching. RSC Adv. 2014, 4, 3938–3943. [Google Scholar] [CrossRef]
- Wang, H.; Chen, Q.; Xia, W.; Qiu, X.; Cheng, Q.; Zhu, G. Electroactive PVDF thin films fabricated via cooperative stretching process. J. Appl. Polym. Sci. 2018, 135, 46324. [Google Scholar] [CrossRef]
- Yin, K.; Zhou, Z.; Schuele, D.E.; Wolak, M.; Zhu, L.; Baer, E. Effects of Interphase Modification and Biaxial Orientation on Dielectric Properties of Poly(ethylene terephthalate)/Poly(vinylidene fluoride-co-hexafluoropropylene) Multilayer Films. ACS Appl. Mater. Interfaces 2016, 8, 13555–13566. [Google Scholar] [CrossRef]
- Mohammadi, B.; Yousefi, A.A.; Bellah, S.M. Effect of tensile strain rate and elongation on crystalline structure and piezoelectric properties of PVDF thin films. Polym. Test. 2007, 26, 42–50. [Google Scholar] [CrossRef]
- Lu, H.; Li, L. Crystalline structure, dielectric, and mechanical properties of simultaneously biaxially stretched polyvinylidene fluoride film. Polym. Adv. Technol. 2018, 29, 3056–3064. [Google Scholar] [CrossRef]
- Cai, X.; Lei, T.; Sun, D.; Lin, L. A critical analysis of the α, β and γ phases in poly(vinylidene fluoride) using FTIR. RSC Adv. 2017, 7, 15382–15389. [Google Scholar] [CrossRef]
- Martins, P.; Lopes, A.C.; Lanceros-Mendez, S. Electroactive phases of poly(vinylidene fluoride): Determination, processing and applications. Prog. Polym. Sci. 2014, 39, 683–706. [Google Scholar] [CrossRef]
- Ma, W.; Zhang, J.; Wang, X.; Wang, S. Effect of PMMA on crystallization behavior and hydrophilicity of poly(vinylidene fluoride)/poly(methyl methacrylate) blend prepared in semi-dilute solutions. Appl. Surf. Sci. 2007, 253, 8377–8388. [Google Scholar] [CrossRef]
- Yeoh, O.H. Some Forms of the Strain Energy Function for Rubber. Rubber Chem. Technol. 1993, 66, 754–771. [Google Scholar] [CrossRef]
- Ali, A.; Hosseini, M.; Sahari, B. A Review of Constitutive Models for Rubber-Like Materials. Am. J. Eng. Appl. Sci. 2010, 3, 232–239. [Google Scholar] [CrossRef]
- Treloar, L.R.G. The Physics of Rubber Elasticity; Oxford University Press: Oxford, UK, 1975. [Google Scholar]
- Ariza Gomez, A.J.; Contreras, M.M.; Vaz, M.A.; Costa, C.A.; Costa, M.F. Temperature-time large strain mechanical model for Poly(vinylidene fluoride). Polym. Test. 2020, 82, 106312. [Google Scholar] [CrossRef]
- Song, H.; Yang, S.; Sun, S.; Zhang, H. Effect of Miscibility and Crystallization on the Mechanical Properties and Transparency of PVDF/PMMA Blends. Polym. Plast. Technol. Eng. 2013, 52, 221–227. [Google Scholar] [CrossRef]
- Sencadas, V.; Gregorio, R.; Lanceros-Méndez, S. α to β Phase Transformation and Microestructural Changes of PVDF Films Induced by Uniaxial Stretch. J. Macromol. Sci. Part B 2009, 48, 514–525. [Google Scholar] [CrossRef]
- Du, C.-H.; Zhu, B.-K.; Xu, Y.-Y. Effects of stretching on crystalline phase structure and morphology of hard elastic PVDF fibers. J. Appl. Polym. Sci. 2007, 104, 2254–2259. [Google Scholar] [CrossRef]



| Code | Material Contents wt% | Stretching Ratio (R) | |
|---|---|---|---|
| PVDF | PMMA | ||
| VM15 | 85 | 15 | 2 × 2, 2.5 × 2.5, or 3 × 3 |
| VM20 | 80 | 20 | |
| VM25 | 75 | 25 | |
| VM30 | 70 | 30 | |
| Stretch Films | Stretching Ratio R | Thickness (μm) |
|---|---|---|
| VM15 | 2 × 2 | 47 |
| 2.5 × 2.5 | 26 | |
| 3 × 3 | 22 | |
| VM20 | 2 × 2 | 54 |
| 2.5 × 2.5 | 41 | |
| 3 × 3 | 30 | |
| VM25 | 2 × 2 | 49 |
| 2.5 × 2.5 | 32 | |
| 3 × 3 | 23 | |
| VM30 | 2 × 2 | 43 |
| 2.5 × 2.5 | 34 | |
| 3 × 3 | 31 |
| Code | Elongation at Break (%) | Tensile Strength (MPa) | Young’s Modulus (MPa) |
|---|---|---|---|
| VM15-R2 | 132 | 86 | 4200 |
| VM15-R2.5 | 79 | 172 | 6492 |
| VM15-R3 | 58 | 176 | 7013 |
| VM20-R2 | 147 | 131 | 4530 |
| VM20-R2.5 | 105 | 138 | 4945 |
| VM20-R3 | 44 | 159 | 4990 |
| VM25-R2 | 162 | 94 | 4139 |
| VM25-R2.5 | 73 | 131 | 6269 |
| VM25-R3 | 72 | 175 | 6740 |
| VM30-R2 | 127 | 100 | 3113 |
| VM30-R2.5 | 83 | 138 | 3837 |
| VM30-R3 | 66 | 154 | 4636 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, Y.; Liu, W.; Tan, B.; Zhu, C.; Ni, Y.; Fang, L.; Lu, C.; Xu, Z. Crystallinity and β Phase Fraction of PVDF in Biaxially Stretched PVDF/PMMA Films. Polymers 2021, 13, 998. https://doi.org/10.3390/polym13070998
Zhou Y, Liu W, Tan B, Zhu C, Ni Y, Fang L, Lu C, Xu Z. Crystallinity and β Phase Fraction of PVDF in Biaxially Stretched PVDF/PMMA Films. Polymers. 2021; 13(7):998. https://doi.org/10.3390/polym13070998
Chicago/Turabian StyleZhou, Ye, Wenting Liu, Bin Tan, Cheng Zhu, Yaru Ni, Liang Fang, Chunhua Lu, and Zhongzi Xu. 2021. "Crystallinity and β Phase Fraction of PVDF in Biaxially Stretched PVDF/PMMA Films" Polymers 13, no. 7: 998. https://doi.org/10.3390/polym13070998
APA StyleZhou, Y., Liu, W., Tan, B., Zhu, C., Ni, Y., Fang, L., Lu, C., & Xu, Z. (2021). Crystallinity and β Phase Fraction of PVDF in Biaxially Stretched PVDF/PMMA Films. Polymers, 13(7), 998. https://doi.org/10.3390/polym13070998







