Anisotropic Tensile Characterisation of Eucalyptus nitens Timber above Its Fibre Saturation Point, and Its Application
Abstract
:1. Introduction
- Obtain the stress–strain curves of E. nitens samples in tension at grain angles of 0°, 10°, 45°, and 90°;
- Obtain the design characteristic values for E. nitens timber in tension parallel to the grain;
- Determine the anisotropic tensile characterisation (failure strengths) of E. nitens wood samples at different load-to-grain angles;
- Investigate the impact of high MC and grain angle on the tensile behaviours of E. nitens wood.
2. Materials and Methods
2.1. Sample Preparation
2.2. Testing Method and Method of Data Analysis
3. Results and Discussions
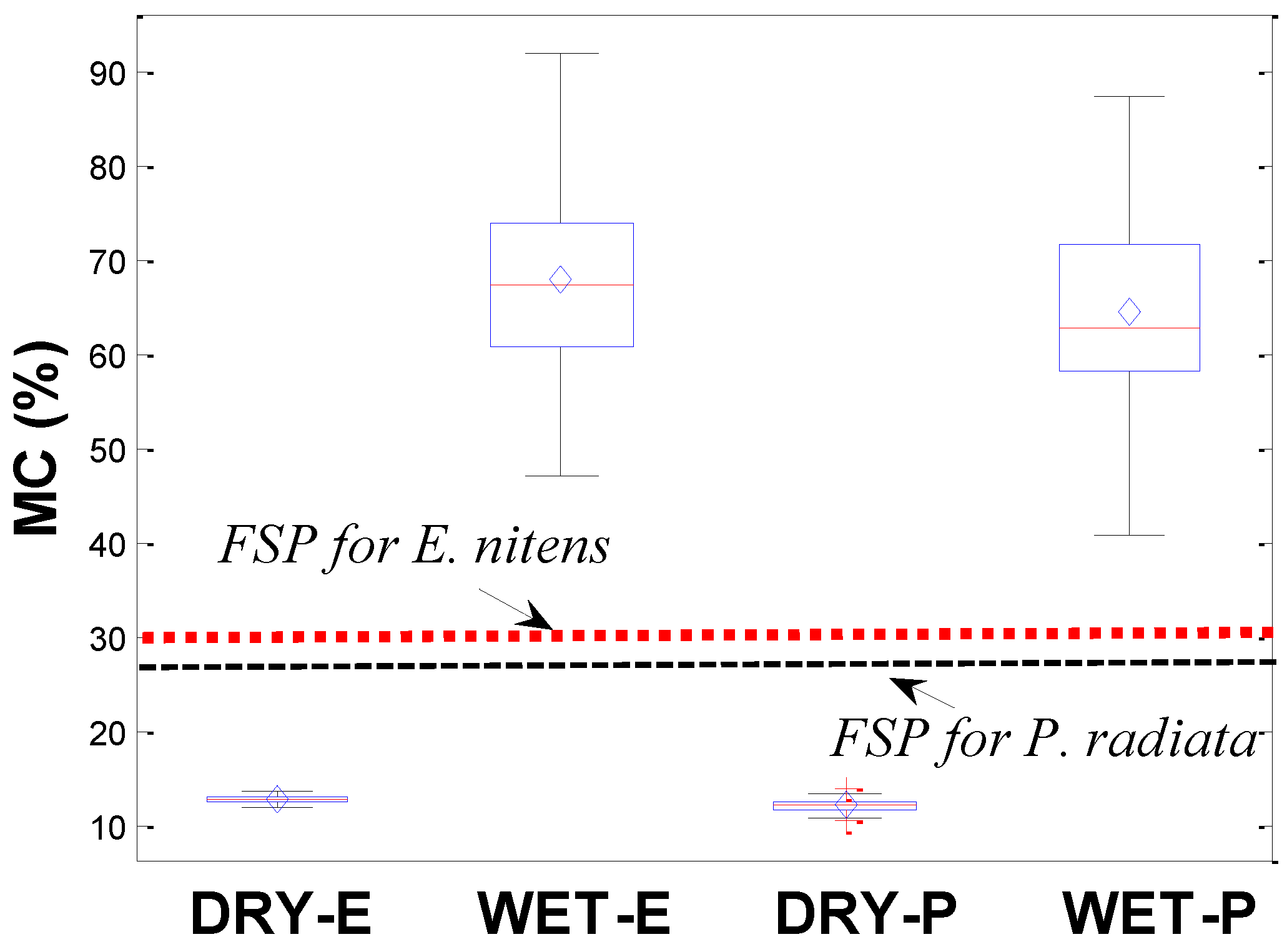
3.1. Moisture Content and Basic Densites
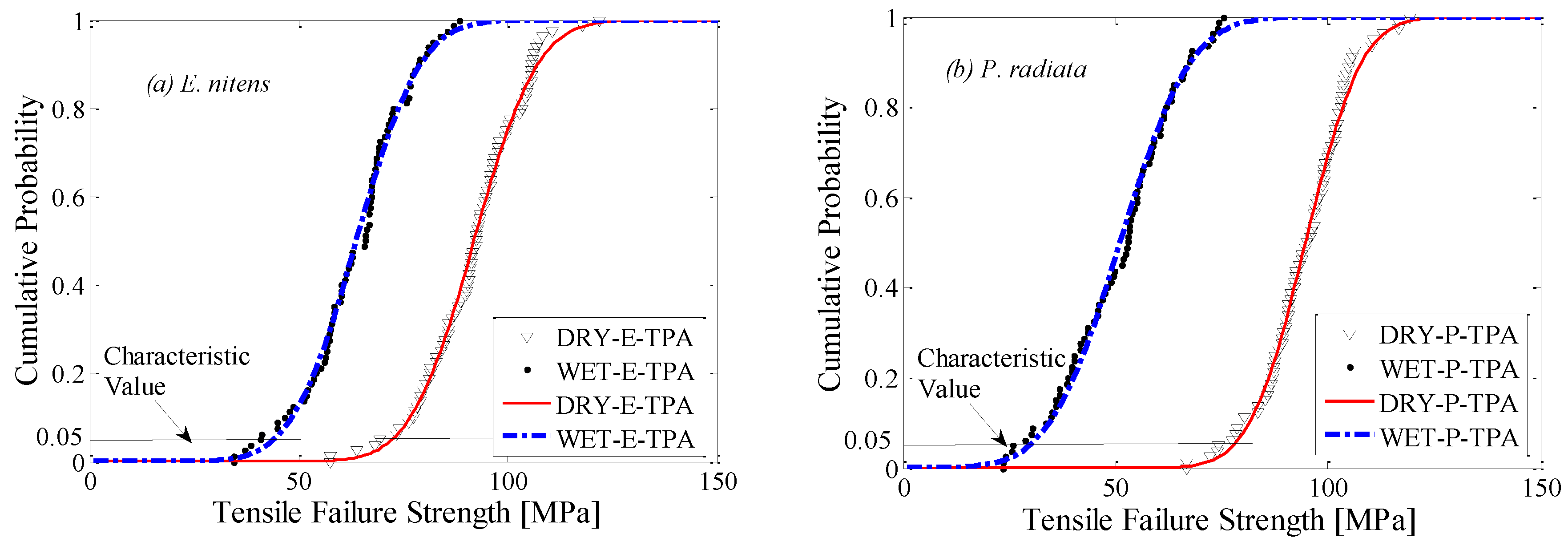
3.2. Statistical Distributions of Tensile Failure Strength Parallel to the Grain
3.3. Stress–Strain Curves
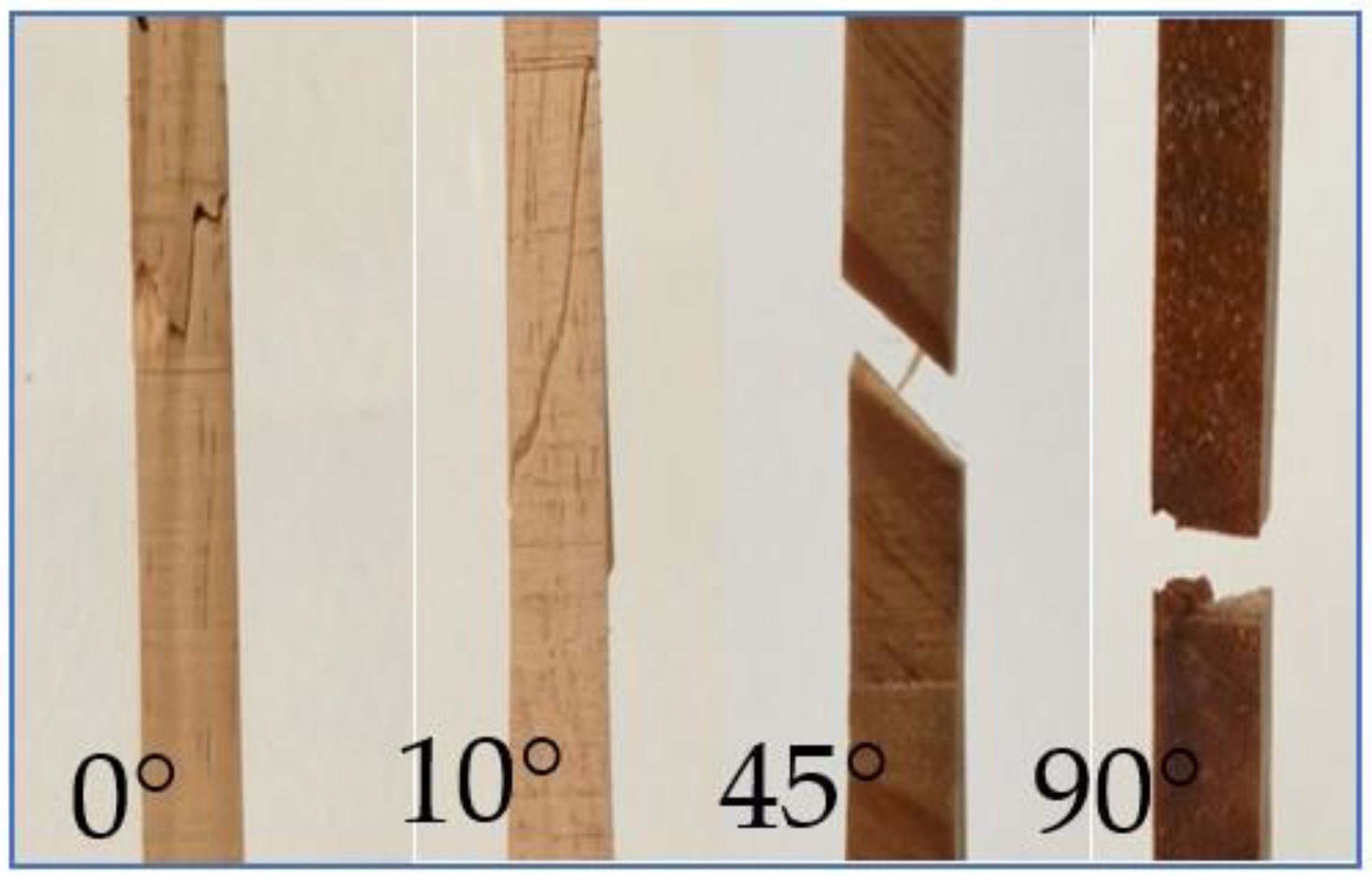
3.4. Failure Modes
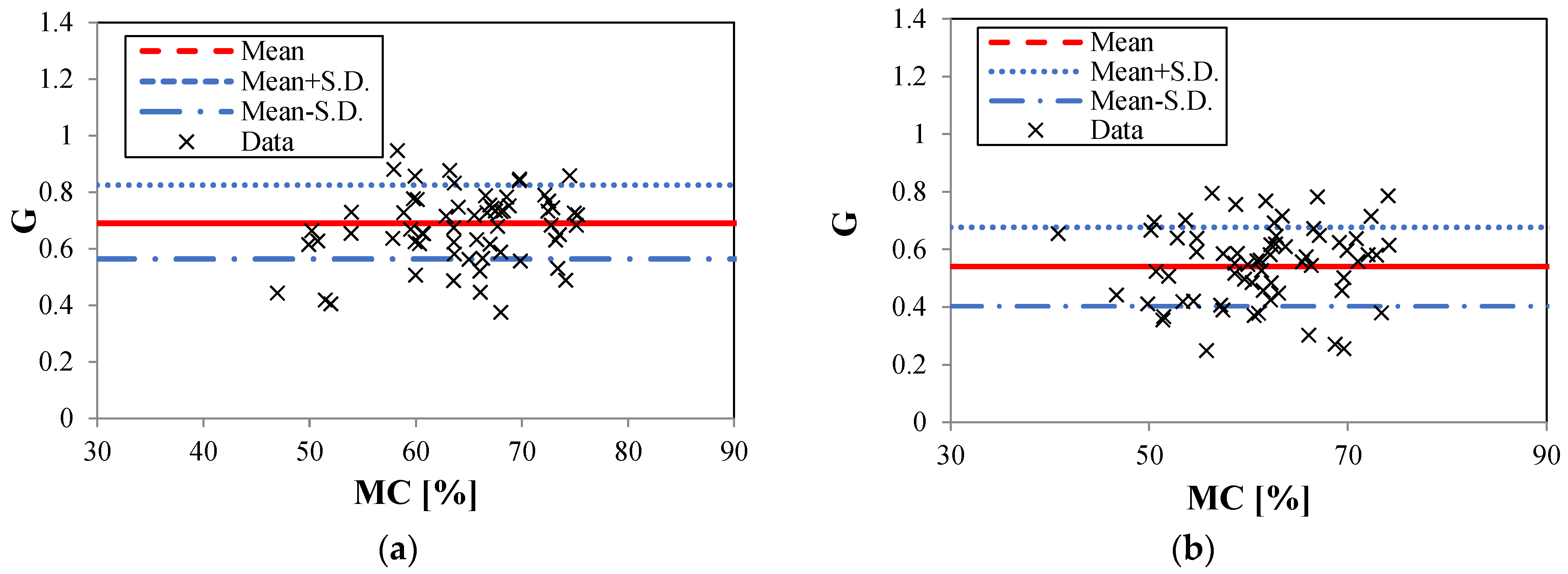
3.5. The Effect of Internal Stresses versus the Grain Angle
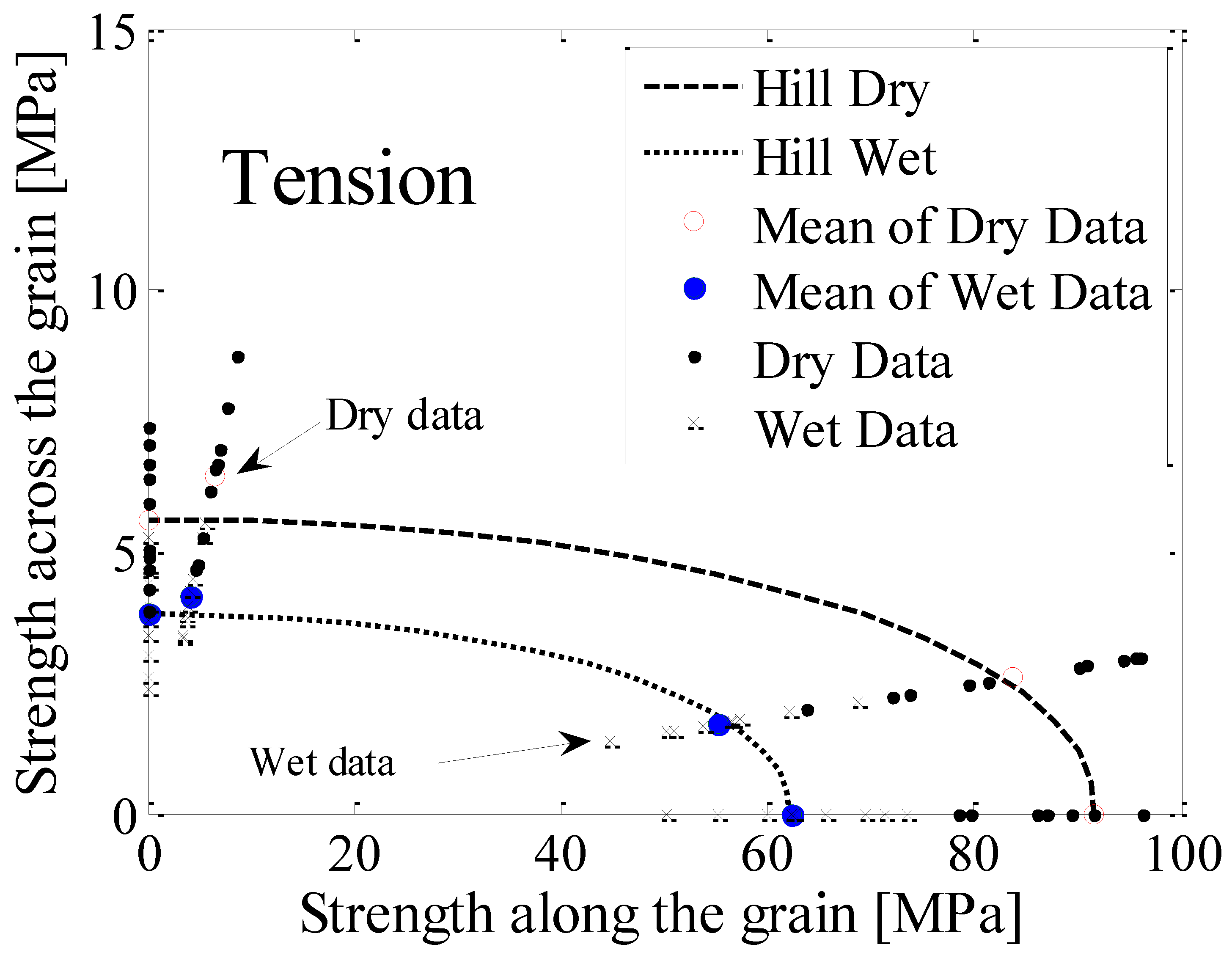
3.6. Failure Envelopes and Failure Strengths
4. Conclusions
- When MC > FSP, the traditional structural timber, P. radiata, showed a greater decrease in longitudinal tensile failure strength than was found for E. nitens. The mean strength reduction of E. nitens was less than that of traditional construction timber, for example P. radiata, indicating the E. nitens shows promise for use in the building industry, especially when exposed to water. The design characteristic values of E. nitens timber were 72.4 MPa and 44.1 Mpa, respectively.
- Samples with both low and high MCs failed by brittle fracture, while the high MC samples achieved lower values of tensile strength. The tensile failure strengths were demonstrated to be moisture content sensitive. The different failure strengths of E. nitens wood in tension and compression were also found both below and above the FSP. A similar reduction in the strength was found for high moisture content samples compared with thermally-modified E. nitens timber, indicating that moisture content affects E. nitens wood cellulose polymers.
- Plantation E. nitens timber in tension was highly anisotropic with respect to grain angle, and its ductility increased as MC increased. Compared with moisture content, the grain angle had a stronger effect on the tensile failure of E. nitens timber due to the micro-structure of E. nitens timber and differences in the internal stress state. At grain angles of 0°, the normal stress along the grain was dominant compared with the corresponding shear stress and normal stress across the grain, leading to simple tensile failure. At a loading direction of 45°, shear stresses played an important role, resulting in cross-grain tensile failure. For a grain angle of 90°, the effect of normal stress across the grain was significant, and therefore bonds between fibres in the micro-structure broke and clean tensile failure along the grain direction was observed.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ghanbari Ghazijahani, T.; Jiao, H.; Holloway, D. Composite Timber Beams Strengthened by Steel and CFRP. J. Compos. Constr. 2017, 21, 04016059. [Google Scholar] [CrossRef]
- Šubic, B.; Fajdiga, G.; Lopatič, J. Bending Stiffness, Load-Bearing Capacity and Flexural Rigidity of Slender Hybrid Wood-Based Beams. Forests 2018, 9, 703. [Google Scholar] [CrossRef] [Green Version]
- Way, D.; Sinha, A.; Kamke, F.; Fujii, J. Evaluation of a Wood-Strand Molded Core Sandwich Panel. J. Mater. Civ. Eng. 2016, 28, 04016074. [Google Scholar] [CrossRef]
- Derikvand, M.; Nolan, G.; Jiao, H.; Kotlarewski, N. What to Do with Structurally Low-Grade Wood from Australia’s Plantation Eucalyptus; Building Application? BioResources 2016, 12, 4–7. [Google Scholar] [CrossRef] [Green Version]
- Cheng, Y.; Nolan, G.; Holloway, D.; Kaur, J.; Lee, M.; Chan, A. Flexural characteristics of Eucalyptus nitens timber with high moisture content. BioResources 2021, 16, 2921–2936. [Google Scholar] [CrossRef]
- Derikvand, M.; Kotlarewski, N.; Lee, M.; Jiao, H.; Chan, A.; Nolan, G. Short-term and long-term bending properties of nail-laminated timber constructed of fast-grown plantation eucalypt. Constr. Build. Mater. 2019, 211, 952–964. [Google Scholar] [CrossRef]
- Derikvand, M.; Kotlarewski, N.; Lee, M.; Jiao, H.; Nolan, G. Characterisation of Physical and Mechanical Properties of Unthinned and Unpruned Plantation-Grown Eucalyptus nitens H.Deane & Maiden Lumber. Forests 2019, 10, 194. [Google Scholar] [CrossRef] [Green Version]
- Pagel, C.L.; Lenner, R.; Wessels, C.B. Investigation into material resistance factors and properties of young, engineered Eucalyptus grandis timber. Constr. Build. Mater. 2020, 230, 117059. [Google Scholar] [CrossRef]
- Aicher, S.; Stapf, G. Compressive strength parallel to the fiber of spruce with high moisture content. Eur. J. Wood Wood Prod. 2016, 74, 527–542. [Google Scholar] [CrossRef] [Green Version]
- Cheng, Y.; Chan, A.H.C.; Holloway, D.; Nolan, G. Anisotropic material behaviour under compression of Eucalyptus nitens with high moisture content. Constr. Build. Mater. 2022, 314, 124788. [Google Scholar] [CrossRef]
- Ross, R.J. Wood handbook: Wood as an Engineering Material; United States Department of Agriculture Forest Service, Forest Products Laboratory: Madison, WI, USA, 2010; Volume 190. [Google Scholar]
- Barrett, J.D.; Hong, J.-P. Moisture content adjustments for dynamic modulus of elasticity of wood members. Wood Sci. Technol. 2009, 44, 485–495. [Google Scholar] [CrossRef]
- Green, D.W.; Evans, J.W. Evolution of Standardized Procedures for Adjusting Lumber Properties for Change in Moisture Content; US Department of Agriculture, Forest Service, Forest Products Laboratory: Madison, WI, USA, 2001. [Google Scholar]
- Nocetti, M.; Brunetti, M.; Bacher, M. Effect of moisture content on the flexural properties and dynamic modulus of elasticity of dimension chestnut timber. Eur. J. Wood Wood Prod. 2014, 73, 51–60. [Google Scholar] [CrossRef]
- Wang, J. Drying performance of experimental wood roof assemblies. In Report to the Canadian Forest Service, Natural Resources Canada; FPInnovations: Vancouver, BC, Canada, 2014. [Google Scholar]
- Brites, R.D.; Neves, L.C.; Saporiti Machado, J.; Lourenço, P.B.; Sousa, H.S. Reliability analysis of a timber truss system subjected to decay. Eng. Struct. 2013, 46, 184–192. [Google Scholar] [CrossRef] [Green Version]
- Niklewski, J.; van Niekerk, P.B.; Brischke, C.; Frühwald Hansson, E. Evaluation of Moisture and Decay Models for a New Design Framework for Decay Prediction of Wood. Forests 2021, 12, 721. [Google Scholar] [CrossRef]
- Arnold, M. Effect of moisture on the bending properties of thermally modified beech and spruce. J. Mater. Sci. 2009, 45, 669. [Google Scholar] [CrossRef] [Green Version]
- Bergander, A.; Salmén, L. Cell wall properties and their effects on the mechanical properties of fibers. J. Mater. Sci. 2002, 37, 151–156. [Google Scholar] [CrossRef]
- ASTMD2899; Standard Practice for Establishing Allowable Stresses for Round Timber Piles. ASTM International: West Conshohocken, PA, USA, 2012.
- Polyanin, A.D.; Manzhirov, A.D. Timber: Its Nature and Behaviour; CRC Press: Boca Raton, FL, USA, 2000. [Google Scholar]
- Kramer, A.; Barbosa, A.R.; Sinha, A. Performance of Steel Energy Dissipators Connected to Cross-Laminated Timber Wall Panels Subjected to Tension and Cyclic Loading. J. Struct. Eng. 2016, 142. [Google Scholar] [CrossRef]
- Dawson, B.S.W.; Pearson, H.; Kimberley, M.O.; Davy, B.; Dickson, A.R. Effect of supercritical CO2 treatment and kiln drying on collapse in Eucalyptus nitens wood. Eur. J. Wood Wood Prod. 2020, 78, 209–217. [Google Scholar] [CrossRef] [Green Version]
- ASTMD143–09; Standard Test Methods for Small Clear Specimens of Timber. ASTM International: West Conshohocken, PA, USA, 2009.
- AS/NZS1080.1; Timber—Methods of Test—Moisture Content. Standards Australia: Sydney, Australia, 2012.
- Bryc, W. The Normal Distribution: Characterizations with Applications; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2012; Volume 100. [Google Scholar]
- Anderson, T.W.; Darling, D.A. A Test of Goodness of Fit. J. Am. Stat. Assoc. 1954, 49, 765–769. [Google Scholar] [CrossRef]
- Báder, M.; Németh, R. Moisture-dependent mechanical properties of longitudinally compressed wood. Eur. J. Wood Wood Prod. 2019, 77, 1009–1019. [Google Scholar] [CrossRef] [Green Version]
- Crespo, J.; Majano-Majano, A.; Lara-Bocanegra, A.J.; Guaita, M. Mechanical Properties of Small Clear Specimens of Eucalyptus globulus Labill. Materials 2020, 13, 906. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Mascia, N.T.; Simoni, R.A. Analysis of failure criteria applied to wood. Eng. Fail. Anal. 2013, 35, 703–712. [Google Scholar] [CrossRef]
- Milch, J.; Tippner, J.; Sebera, V.; Brabec, M. Determination of the elasto-plastic material characteristics of Norway spruce and European beech wood by experimental and numerical analyses. Holzforschung 2016, 70, 1081–1092. [Google Scholar] [CrossRef]
- Clouston, P.L.; Lam, F. A stochastic plasticity approach to strength modeling of strand-based wood composites. Compos. Sci. Technol. 2002, 62, 1381–1395. [Google Scholar] [CrossRef]
- Yuan, T.; Wang, Z.; Han, X.; Yuan, Z.; Wang, X.; Li, Y. Multi-scale evaluation of the effect of saturated steam on the micromechanical properties of Moso bamboo. Holzforschung 2021, 75, 1052–1060. [Google Scholar] [CrossRef]
- Wang, Q.; Wu, X.; Yuan, C.; Lou, Z.; Li, Y. Effect of Saturated Steam Heat Treatment on Physical and Chemical Properties of Bamboo. Molecules 2020, 25, 1999. [Google Scholar] [CrossRef] [PubMed]
- Hill, C.A.S. Wood Modification: Chemical, Thermal and Other Processes; John Wiley & Sons, Ltd.: Bangor, UK, 2006. [Google Scholar]





| Species | Moisture Content (MC) | Code | Number of Replicated Samples | Grain Angle | |
|---|---|---|---|---|---|
| GROUP I | E. nitens | 12% | DRY-E-TPA | 80 | 0° |
| >30% | WET-E-TPA | 80 | 0° | ||
| P. radiata | 12% | DRY-P-TPA | 80 | 0° | |
| >30% | WET-P-TPA | 80 | 0° | ||
| GROUP II | E. nitens | 12% | DRY-E-T0 | 10 | 0° |
| DRY-E-T10 | 10 | 10° | |||
| DRY-E-T45 | 10 | 45° | |||
| DRY-E-T90 | 10 | 90° | |||
| >30% | WET-E-T0 | 10 | 0° | ||
| WET-E-T10 | 10 | 10° | |||
| WET-E-T45 | 10 | 45° | |||
| WET-E-T90 | 10 | 90° | |||
| GROUP III | Untreated E. nitens (kiln dried) | 12% | UT-E-T0 | 10 | 0° |
| Heat-treated E. nitens | HT-E-T0 | 10 | 0° |
| Kolmogorov–Smirnov (K–S) Test | Anderson–Darling (A–D) Test | ||||||
|---|---|---|---|---|---|---|---|
| Maximum Absolute Difference | Critical Value | Acceptance | Squared Distance | Critical Value | Acceptance | ||
| E. nitens | DRY-E-TPA | 0.0716 | 0.1496 | Yes | 0.31 | 0.54 | Yes |
| WET-E-TPA | 0.0897 | 0.1496 | Yes | 0.34 | 0.47 | Yes | |
| P. radiata | DRY-P-TPA | 0.0605 | 0.1496 | Yes | 0.34 | 0.49 | Yes |
| WET-P-TPA | 0.0792 | 0.1496 | Yes | 0.35 | 0.44 | Yes | |
| Tensile Failure Strength | E. nitens | P. radiata | ||
|---|---|---|---|---|
| MC = 12% | MC > FSP | MC = 12% | MC > FSP | |
| Mean (Mpa) | 91.9 | 63.8 | 94.7 | 51.1 |
| Coefficients of variations | 13.0% | 18.8% | 11.0% | 25.4% |
| Characteristic value, fn (Mpa) | 72.4 | 44.1 | 77.6 | 29.8 |
| Code | Formulae | Notes |
|---|---|---|
| Hill Dry | = 1 | Hill criterion for dry samples in tension |
| Hill Wet | = 1 | Hill criterion for wet samples in tension |
| Grain Angle (°) | Compression [10] | Tension | ||||||
|---|---|---|---|---|---|---|---|---|
| 0 | 45 | 90 | 0 | 45 | 90 | |||
| Failure strength (MPa) | DRY (MC = 12%) | Mean | 43.2 | 18.0 | 8.3 | 91.6 | 12.9 | 5.6 |
| S.D.* | 5.5 | 2.4 | 2.0 | 10.9 | 2.4 | 1.2 | ||
| N ** | 10 | 10 | 10 | 10 | 10 | 10 | ||
| WET (MC > FSP) | Mean | 23.9 | 9.6 | 4.6 | 62.1 | 8.3 | 3.8 | |
| S.D. * | 3.1 | 1.8 | 1.0 | 7.9 | 1.4 | 0.9 | ||
| N ** | 10 | 10 | 10 | 10 | 10 | 10 | ||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, X.; Cheng, Y.; Chan, A.; Holloway, D.; Nolan, G. Anisotropic Tensile Characterisation of Eucalyptus nitens Timber above Its Fibre Saturation Point, and Its Application. Polymers 2022, 14, 2390. https://doi.org/10.3390/polym14122390
Chen X, Cheng Y, Chan A, Holloway D, Nolan G. Anisotropic Tensile Characterisation of Eucalyptus nitens Timber above Its Fibre Saturation Point, and Its Application. Polymers. 2022; 14(12):2390. https://doi.org/10.3390/polym14122390
Chicago/Turabian StyleChen, Xudong, Yingyao Cheng, Andrew Chan, Damien Holloway, and Gregory Nolan. 2022. "Anisotropic Tensile Characterisation of Eucalyptus nitens Timber above Its Fibre Saturation Point, and Its Application" Polymers 14, no. 12: 2390. https://doi.org/10.3390/polym14122390
APA StyleChen, X., Cheng, Y., Chan, A., Holloway, D., & Nolan, G. (2022). Anisotropic Tensile Characterisation of Eucalyptus nitens Timber above Its Fibre Saturation Point, and Its Application. Polymers, 14(12), 2390. https://doi.org/10.3390/polym14122390







