Analysis of Creep Properties and Factors Affecting Wood Plastic Composites
Abstract
:1. Introduction
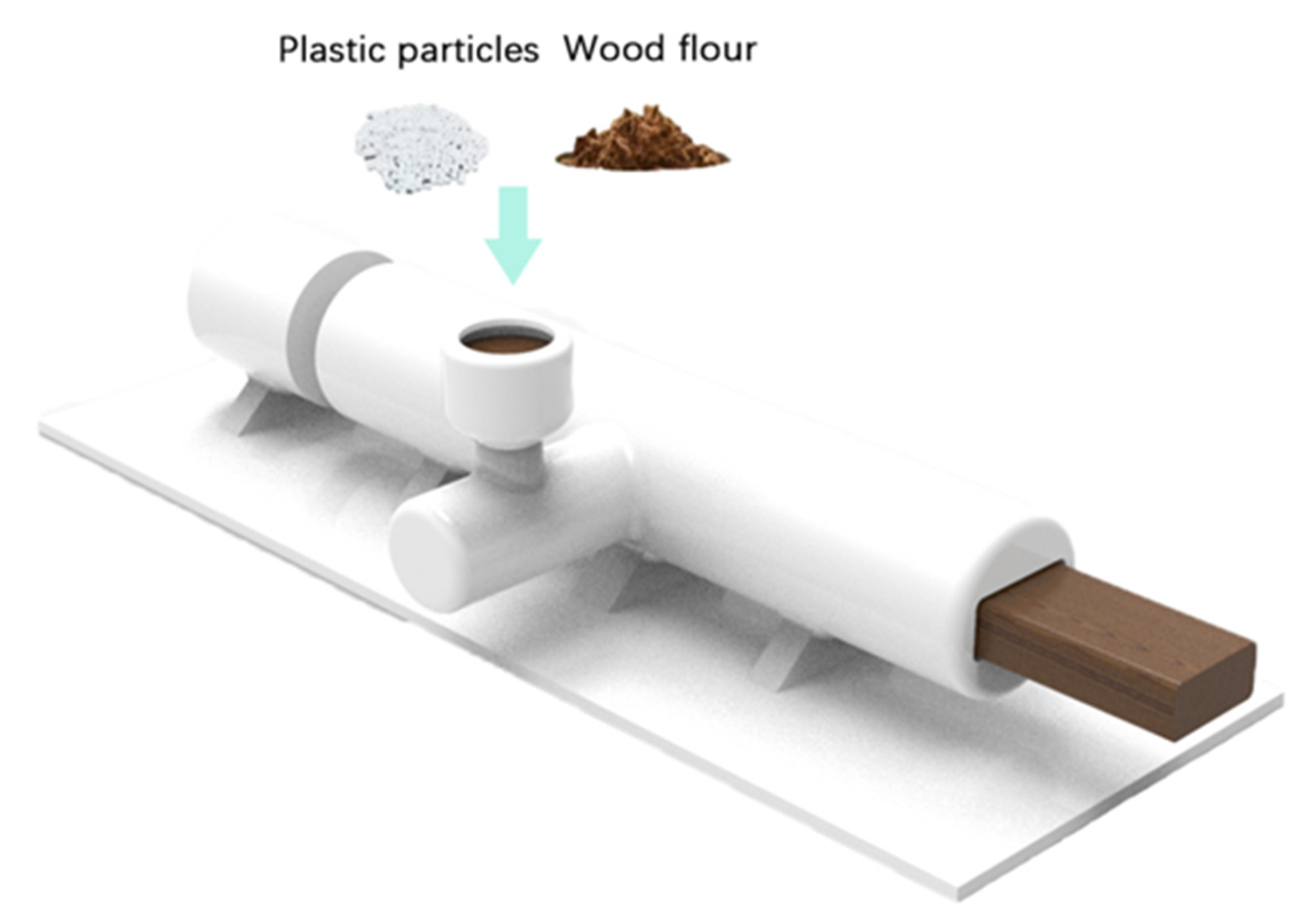
2. Materials and Methods
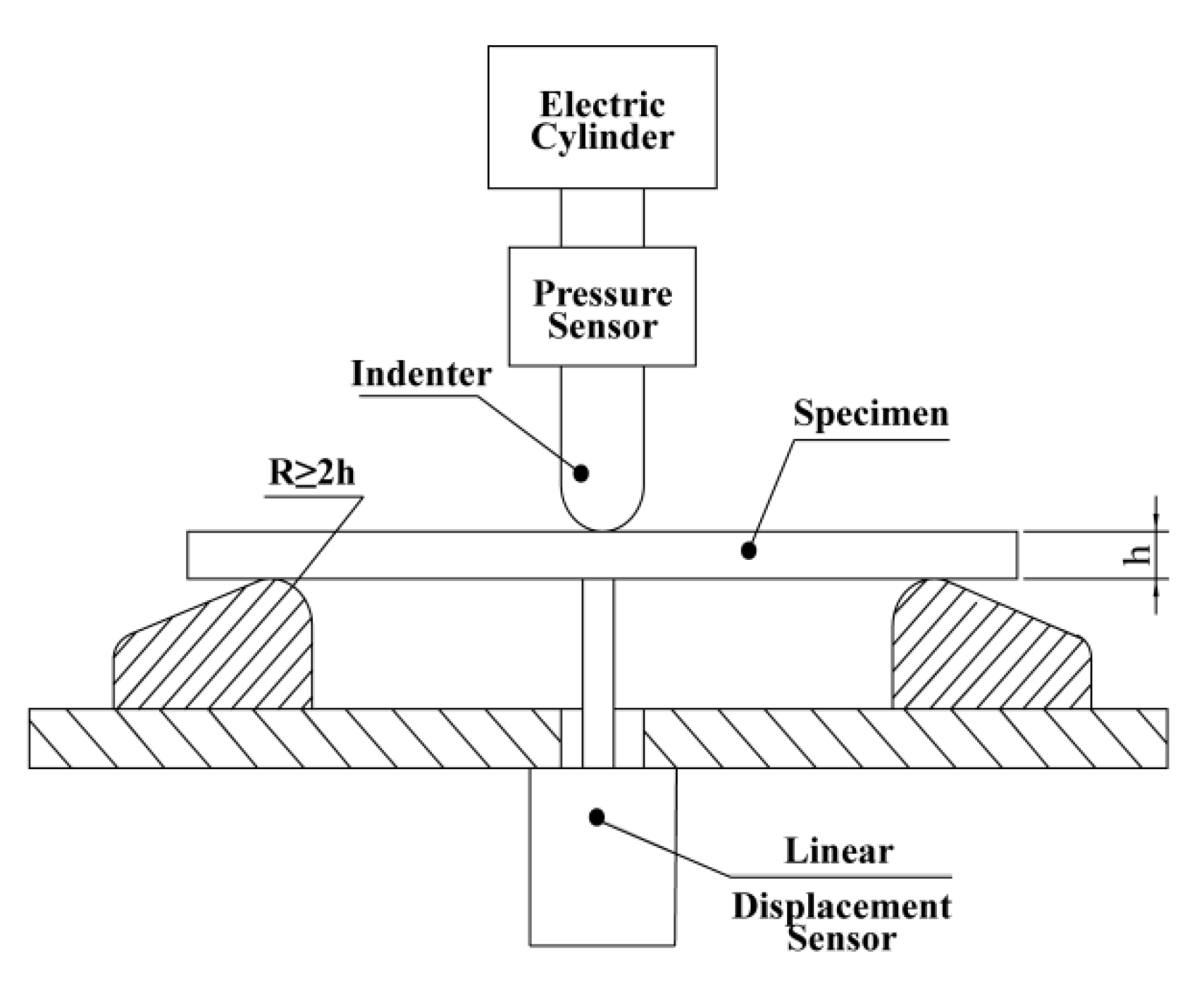
3. Creep Test
4. Results and Discussion
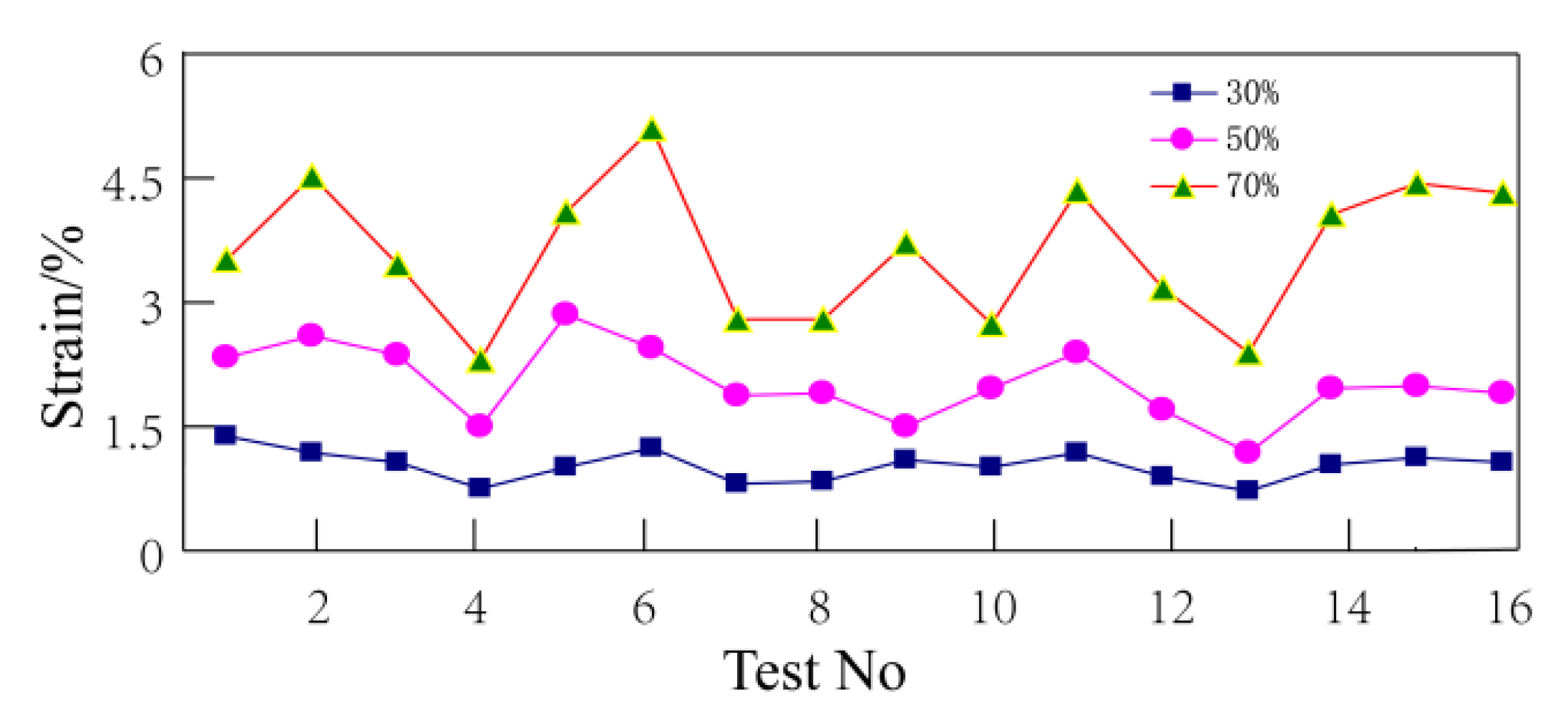
4.1. Influencing Factors of 24 h Creep of Wood–Plastic Board
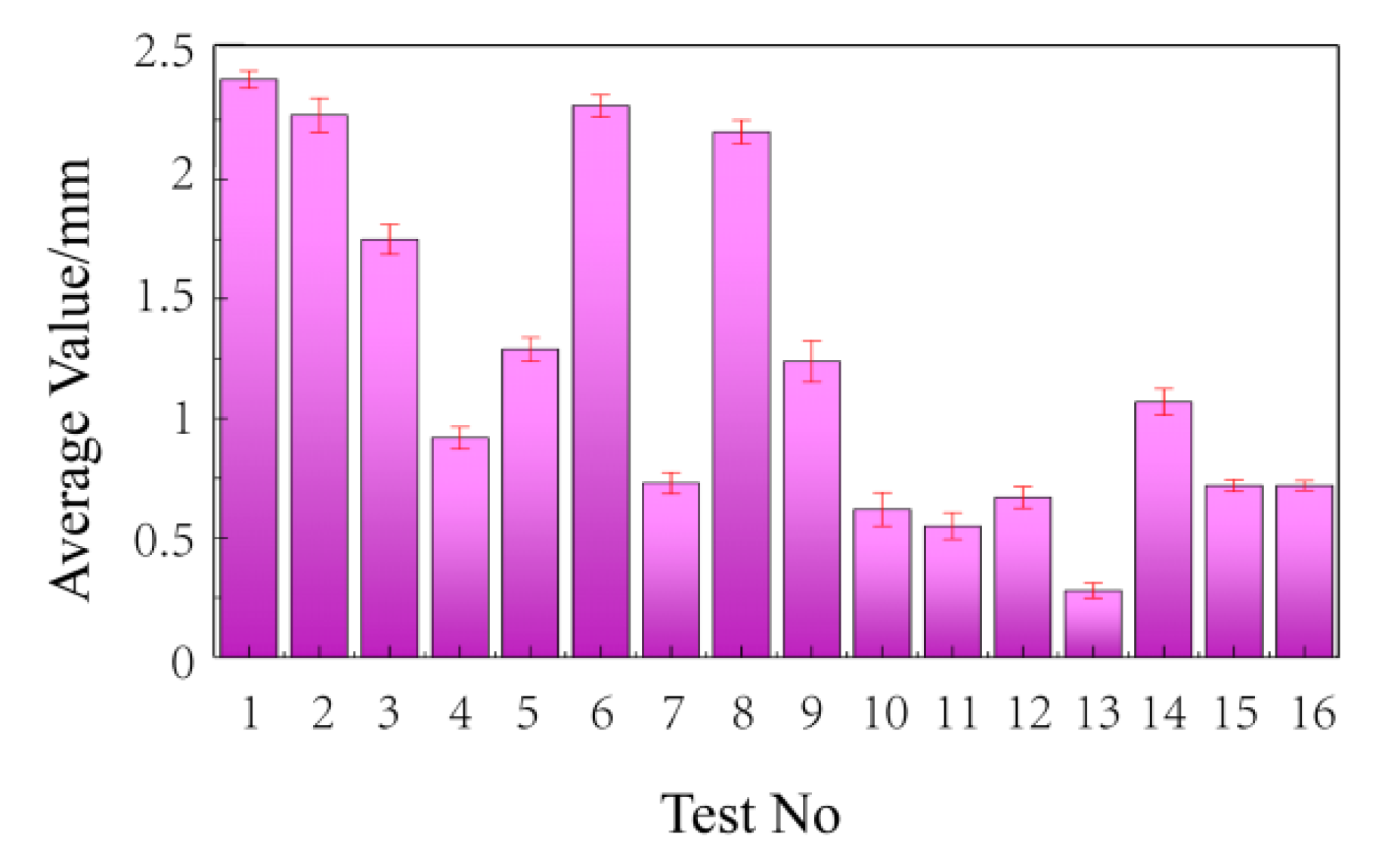
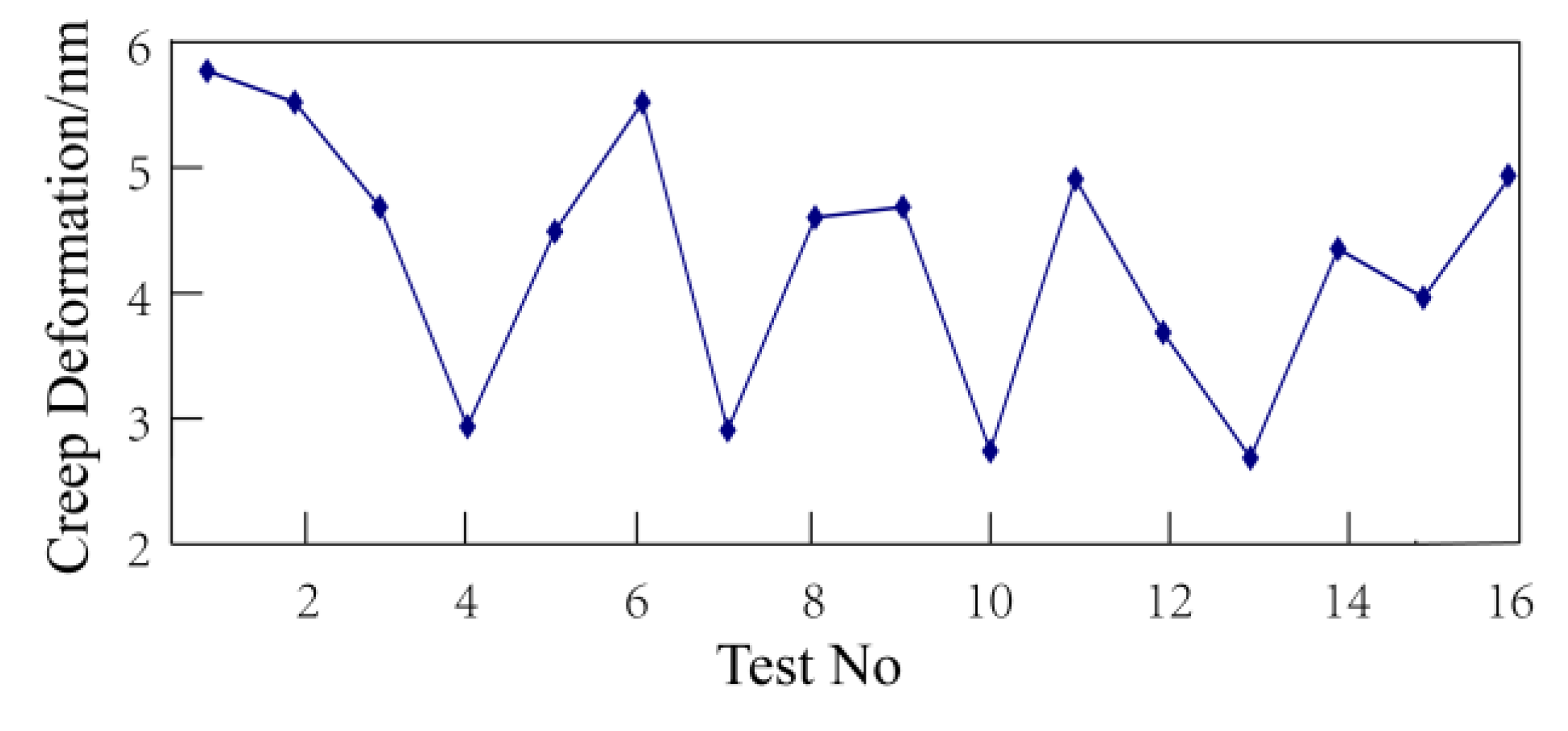
4.2. Analysis of Influencing Factors of 240 h Creep Variable of Wood–Plastic Board
4.3. Analysis of Creep Law
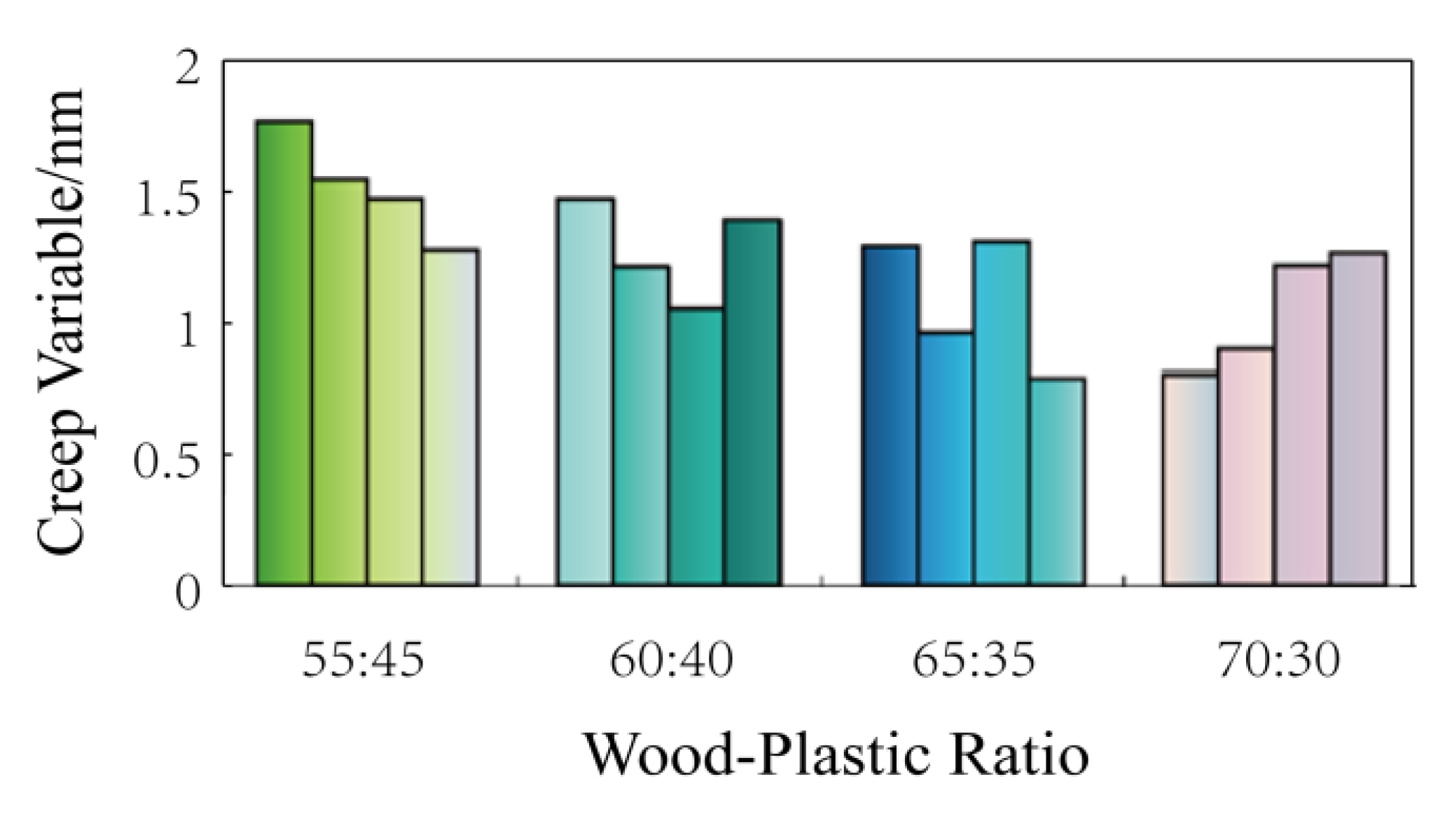
4.3.1. Effect of Wood–Plastic Ratio on 24 h Creep Variable
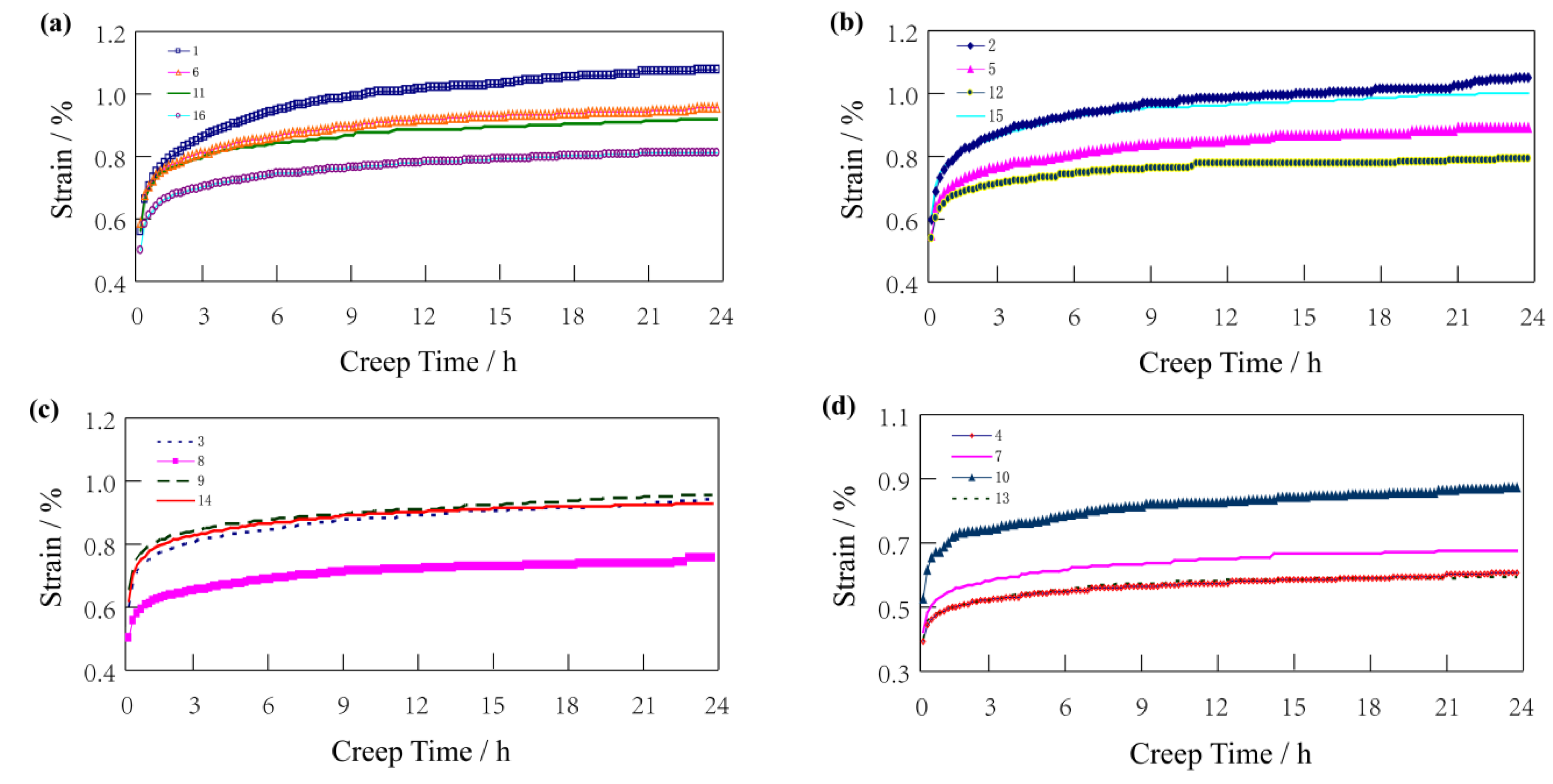
4.3.2. Effect of Wood–Plastic Ratio on 24 h Creep Trend
4.4. Analysis of 24 h Creep Recovery Trend
4.5. Influence of Creep of Wood–Plastic Composites on Structural Reliability
5. Conclusions
- (1)
- The effects of various technological factors on the creep properties of WPC are different under different loading conditions, but the wood–plastic ratio is the most significant factor. In the range of (55:45–70:30), the higher the wood powder content, the lower the creep of the material. When the wood–plastic ratio is greater than (60:40), the influence of wood powder on the 24 h creep slows down or even becomes insignificant.
- (2)
- In the 24 h creep deformation of WPC, the elastic deformation, viscoelastic deformation, and viscous deformation of WPC increase with the increase of the load, and the most important deformation is the elastic deformation, and the proportion of the elastic strain in the total strain decreases gradually with the increase of load.
- (3)
- When the load is small (30% bending strength), the wood–plastic sheet creep reliability is the highest. When the load increases, the reliability begins to reduce. Some WPC boards began to fracture during the creep test at the load of 50% bending strength. All wood–plastic panels show creep fracture within a short time when the load is at 70% bending strength, and the higher the wood–plastic ratio is, the lower the creep reliability is and the easier it is to fracture.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Tang, J.; Lu, Q.; Yuan, J. Study on the properties of PP bamboo plastic composites toughened by white carbon black and mPE. J. Mater. Sci. Eng. 2019, 37, 86–90. [Google Scholar]
- Chen, X.X. Study on the Application of Plastic Wood Composite Materials in Furniture; Zhejiang University: Hangzhou, China, 2013. [Google Scholar]
- Sun, H.; Lv, X.; Yuan, N.; Wang, Q.; Hao, X.; Sun, L. Strength analysis and extrusion process optimization of wood-plastic composite by response surface method. J. Compos. Mater. 2021, 6, 1838–1846. [Google Scholar]
- Song, L.X.; Zhang, P.; Yao, N.N.; Song, Y.Z.; Kang, M.; Song, K.P. Study on the influence of particle size and filling amount of wood powder on the mechanical properties of wood-plastic composites. Funct. Mater. 2013, 44, 2451–2454. [Google Scholar]
- Xu, H.L.; Cao, Y.; Wang, W.H.; Wang, Q.W.; Wang, H.G. Effect of poplar fiber size on mechanical and creep properties of hot pressed poplar fiber/HDPE composites. J. Compos. Mater. 2016, 33, 1168–1173. [Google Scholar]
- Hao, X.; Yi, X.; Sun, L.; Wang, Q.; Ou, R. Mechanical properties, creep resistance, and dimensional stability of core/shell structured wood flour/polyethylene composites with highly filled core layer. Constr. Build. Mater. 2019, 226, 879–887. [Google Scholar] [CrossRef]
- Homkhiew, C.; Ratanawilai, T.; Thongruang, W. Effect of Wood Flour Content and Cooling Rate on Properties of Rubberwood Flour/Recycled Polypropylene Composites. Adv. Mater. Res. 2012, 488–489, 495–500. [Google Scholar] [CrossRef]
- Avu, V.; Mengeloglu, F. Effect of Wood Particle Size on Selected Properties of Neat and Recycled Wood Polypropylene Composites. Bioresources 2020, 15, 3427–3442. [Google Scholar]
- Xue, J.; Xue, P. Study on creep resistance of HDPE/wood flour composites. Appl. Eng. Plast. 2010, 38, 9–13. [Google Scholar]
- Lee, S.Y.; Yang, H.S.; Kim, H.J.; Jeong, C.S.; Lim, B.S.; Lee, J.N. Creep behavior and manufacturing parameters of wood flour filled polypropylene composites. Compos. Struct. 2004, 65, 459–469. [Google Scholar] [CrossRef]
- Bledzki, A.K.; Faruk, O. Creep and impact properties of wood fibre–polypropylene composites: Influence of temperature and moisture conten. Compos. Sci. Technol. 2004, 64, 693–700. [Google Scholar] [CrossRef]
- Mosiewicki, M.A.; Marcovich, N.E.; Aranguren, M.I. Creep behavior of wood flour composites made from linseed oil-based polyester thermosets. J. Appl. Polym. Sci. 2011, 121, 2626–2633. [Google Scholar] [CrossRef]
- Jia, M.; Xue, P.; Zhao, Y.; Zhang, K. Creep Behaviour of Wood Flour/Poly(vinyl chloride) Composites. J. Wuhan Univ. Technol. Mater. Sci. Ed. 2009, 24, 440–447. [Google Scholar] [CrossRef]
- Febrianto, F.; Hidayat, W.; Wistara, I.; Park, S.; Kim, N. Influence of Impact Modifier–Coupling agent Combination on Mechanical Properties of Wood Flour–Reinforced Polypropylene Composit. J. Fac. Agric. Kyushu Univ. 2017, 62, 445–450. [Google Scholar] [CrossRef]
- Wang, K.J.; Zhao, Y.S.; Zhu, F.H. Flexural and creep properties of wood plastic composites filled with montmorillonite. Polym. Mater. Sci. Eng. 2007, 6, 115–118+122. [Google Scholar]
- Huang, C.W.; Yang, T.C.; Wu, T.L.; Hung, K.C.; Wu, J.H. Effects of maleated polypropylene content on the extended creep behavior of wood-polypropylene composites using the stepped isothermal method and the stepped isostress method. Wood Sci. Technol. 2018, 52, 1313–1330. [Google Scholar] [CrossRef]
- Saman, G.; Saeed, K. A Study on Creep Behavior of Wood Flour- Recycled Polypropylene Composite. Iran. J. Wood Pap. Ind. 2013, 3, 1–12. [Google Scholar]
- Park, B.D.; Balatinecz, J.J. Short term flexural creep behavior of wood-fiber/polypropylene composites. Polym. Compos. 2010, 19, 377–382. [Google Scholar] [CrossRef]
- Saman, G.; Behbood, M.; Saeed, K.N. Effect of Ethylene Vinyl Acetate as an Impact Modifier on Creep Behavior of Wood Flour- Recycled Polypropylene Composites. J. For. Wood Prod. 2016, 3, 785–798. [Google Scholar]
- Homkhiew, C.; Ratanawilai, T.; Thongruang, W. Time–temperature and stress dependent behaviors of composites made from recycled polypropylene and rubberwood flour. Constr. Build. Mater. 2014, 66, 98–104. [Google Scholar] [CrossRef]
- Jiang, Y.T.; Li, K.; Wu, Z.Y.; Ding, J.S. Study on creep properties of rice husk/HDPE wood plastic composites. Packag. Eng. 2008, 8, 4–6. [Google Scholar]
- Dong, Z.X. Simulation and prediction of creep behavior of polypropylene wood plastic composite. Polym. Mater. Sci. Eng. 2010, 26, 89–92. [Google Scholar]
- Xu, H.L.; Cao, Y.; Li, L.F. Creep properties of Masson Pine and Chinese fir reinforced polymer composites. J. Fujian For. Univ. 2019, 39, 654–659. [Google Scholar]
- Tian, X.L.; Li, K.; Jiang, Y.T.; He, Q.; Wu, C.Y. Creep behavior of wood plastic composites under different loading modes. Plast. Ind. 2008, 10, 46–49. [Google Scholar]
- Xu, Y.; Wu, Q.; Yong, L.; Fei, Y. Creep behavior of bagasse fiber reinforced polymer composites. Bioresour. Technol. 2010, 101, 3280–3286. [Google Scholar] [CrossRef] [PubMed]
- ASTMD790; Standard Test Methods for Flexural Properties of Unreinforced and Reinforced Plastics and Electrical Insulating Materials. American Society for Testing and Materials: West Conshohocken, PA, USA, 2010.
- Li, F.; Zhao, C.; Deng, Z. A Creep Testing Device for Wood Plastic Composites. China ZL201720824845.1.2018-01-05.
- ASTMD7031; Standard Guide for Evaluating Mechanical and Physical Properties of Wood-Plastic Composite Products. American Society for Testing and Materials: West Conshohocken, PA, USA, 2004.
- Du, H.; Wang, W.; Wang, H.; Wang, Q. Influence of wood fiber content on the creep behavior of wood fiber-plastic composite. Jianzhu Cailiao Xuebao/J. Build. Mater. 2015, 18, 333–339. [Google Scholar]
- Li, F.; Jun, Y. The Reliability Influence of Wood Powder Contenton on Wood-plastic Composite Material. For. Sci. Technol. 2012, 37, 44–45. [Google Scholar]


| Test No | Molding Temp/°C | Screw Speed r/min | Wood–Plastic Ratio | Coupling Agent/% | Granulation Temp/°C | Bending Strength/MPa |
|---|---|---|---|---|---|---|
| 1 | 150 | 30 | 55:45 | 2 | 150 | 55.19 |
| 2 | 150 | 50 | 60:40 | 3 | 160 | 58.25 |
| 3 | 150 | 70 | 65:35 | 4 | 170 | 58.95 |
| 4 | 150 | 90 | 70:30 | 5 | 180 | 63.14 |
| 5 | 160 | 30 | 60:40 | 4 | 180 | 58.05 |
| 6 | 160 | 50 | 55:45 | 5 | 170 | 58.45 |
| 7 | 160 | 70 | 70:30 | 2 | 160 | 59.55 |
| 8 | 160 | 90 | 65:35 | 3 | 150 | 62.06 |
| 9 | 170 | 30 | 65:35 | 5 | 160 | 63.97 |
| 10 | 170 | 50 | 70:30 | 4 | 150 | 62.45 |
| 11 | 170 | 70 | 55:45 | 3 | 180 | 58.43 |
| 12 | 170 | 90 | 60:40 | 2 | 170 | 53.47 |
| 13 | 180 | 30 | 70:30 | 3 | 170 | 51.35 |
| 14 | 180 | 50 | 65:35 | 2 | 180 | 60.14 |
| 15 | 180 | 70 | 60:40 | 5 | 150 | 60.16 |
| 16 | 180 | 90 | 55:45 | 4 | 160 | 57.33 |
| Time | Strain 1 | Strain 2 | Strain 3 | Strain 4 | Strain 5 | Strain 6 | Strain 7 | Strain 8 |
|---|---|---|---|---|---|---|---|---|
| 1 min | 0.59 | 0.53 | 0.50 | 0.35 | 0.47 | 0.62 | 0.39 | 0.38 |
| 10 min | 0.72 | 0.63 | 0.60 | 0.41 | 0.57 | 0.74 | 0.45 | 0.45 |
| 20 min | 0.78 | 0.69 | 0.64 | 0.43 | 0.60 | 0.78 | 0.47 | 0.47 |
| ⋮ | ⋮ | ⋮ | ⋮ | ⋮ | ⋮ | ⋮ | ⋮ | ⋮ |
| 1420 min | 1.28 | 1.07 | 0.94 | 0.59 | 0.88 | 1.12 | 0.66 | 0.70 |
| 1430 min | 1.28 | 1.07 | 0.94 | 0.59 | 0.88 | 1.12 | 0.66 | 0.70 |
| 1440 min | 1.28 | 1.07 | 0.94 | 0.59 | 0.88 | 1.12 | 0.66 | 0.70 |
| Model | Unstandardized Coefficients | Standard Coefficient | t | Sig. | |
|---|---|---|---|---|---|
| B | Standard Error | ||||
| (Constant) | 4.623 | 1.017 | 4.547 | 0.001 | |
| Molding Temp | −0.005 | 0.004 | −0.154 | −1.078 | 0.306 |
| Screw Speed | −0.005 | 0.002 | −0.357 | −2.493 | 0.032 |
| Wood–plastic Ratio | −0.607 | 0.113 | −0.771 | −5.381 | 0.000 |
| Coupling Agent | 0.015 | 0.042 | 0.050 | 0.347 | 0.736 |
| Granulation Temp | −0.006 | 0.004 | −0.215 | −1.502 | 0.164 |
| Model | Unstandardized Coefficients | Standard Coefficient | t | Sig. | |
|---|---|---|---|---|---|
| B | Standard Error | ||||
| (Constant) | 9.925 | 3.481 | 2.851 | 0.017 | |
| Molding Temp | −0.031 | 0.014 | −0.436 | −2.172 | 0.055 |
| Screw Speed | −0.007 | 0.007 | −0.191 | −0.949 | 0.365 |
| Wood–plastic Ratio | −1.158 | 0.386 | −0.602 | −2.996 | 0.013 |
| Coupling Agent | −0.016 | 0.143 | −0.023 | −0.113 | 0.912 |
| Granulation Temp | 0.007 | 0.014 | 0.092 | 0.455 | 0.658 |
| Model | Unstandardized Coefficients | Standard Coefficient | t | Sig. | |
|---|---|---|---|---|---|
| B | Standard Error | ||||
| (Constant) | 3.784 | 2.971 | 2.741 | 0.232 | |
| Molding Temp | 0.009 | 0.12 | 0.115 | 0.751 | 0.470 |
| Screw Speed | −0.006 | 0.006 | −0.158 | −1.029 | 0.328 |
| Wood–plastic Ratio | −1.762 | 0.330 | −0.818 | −5.334 | 0.000 |
| Coupling Agent | 0.177 | 0.122 | 0.222 | 1.445 | 0.179 |
| Granulation Temp | 0.007 | 0.012 | 0.092 | 0.603 | 0.560 |
| Model | Unstandardized Coefficients | Standard Coefficient | t | Sig. | |
|---|---|---|---|---|---|
| B | Standard Error | ||||
| (Constant) | 12.423 | 2.522 | 4.925 | 0.001 | |
| Molding Temp | −0.043 | 0.01 | −0.686 | −4.127 | 0.002 |
| Screw Speed | −0.006 | 0.005 | −0.187 | −1.126 | 0.287 |
| Wood–Plastic Ratio | −0.661 | 0.28 | −0.392 | −2.359 | 0.04 |
| Coupling Agent | 0 | 0.104 | −0.001 | −0.005 | 0.996 |
| Granulation Temp | −0.016 | 0.01 | −0.253 | −1.525 | 0.158 |
| Test No | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 | 15 | 16 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 50% | 17.9 | 19.3 | 17.7 | 10.3 | 15.7 | 19.8 | 15.7 | 18.1 | 19.2 | 19.7 | 19.9 | 17.2 | 17.6 | 17.6 | 17.3 | 19.3 |
| 50% | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| 70% | 6.25 | 8.41 | 8.01 | 5.52 | 7.45 | 8.52 | 7.45 | 8.11 | 8.39 | 8.5 | 8.55 | 7.89 | 7.98 | 7.99 | 7.9 | 8.4 |
| 70% | 1 | 1 | 1 | 0.99 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 13 | 14 | 15 | 16 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| - | - | fracture | fracture | fracture | - | fracture | - | - | fracture | - | fracture | - | - | - |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Feng, L.; Zhao, C. Analysis of Creep Properties and Factors Affecting Wood Plastic Composites. Polymers 2022, 14, 2814. https://doi.org/10.3390/polym14142814
Feng L, Zhao C. Analysis of Creep Properties and Factors Affecting Wood Plastic Composites. Polymers. 2022; 14(14):2814. https://doi.org/10.3390/polym14142814
Chicago/Turabian StyleFeng, Li, and Chunyan Zhao. 2022. "Analysis of Creep Properties and Factors Affecting Wood Plastic Composites" Polymers 14, no. 14: 2814. https://doi.org/10.3390/polym14142814
APA StyleFeng, L., & Zhao, C. (2022). Analysis of Creep Properties and Factors Affecting Wood Plastic Composites. Polymers, 14(14), 2814. https://doi.org/10.3390/polym14142814





