Fully Coupled Three-Dimensional Simulation of Downward Flame Spread over Combustible Material
Abstract
:1. Introduction
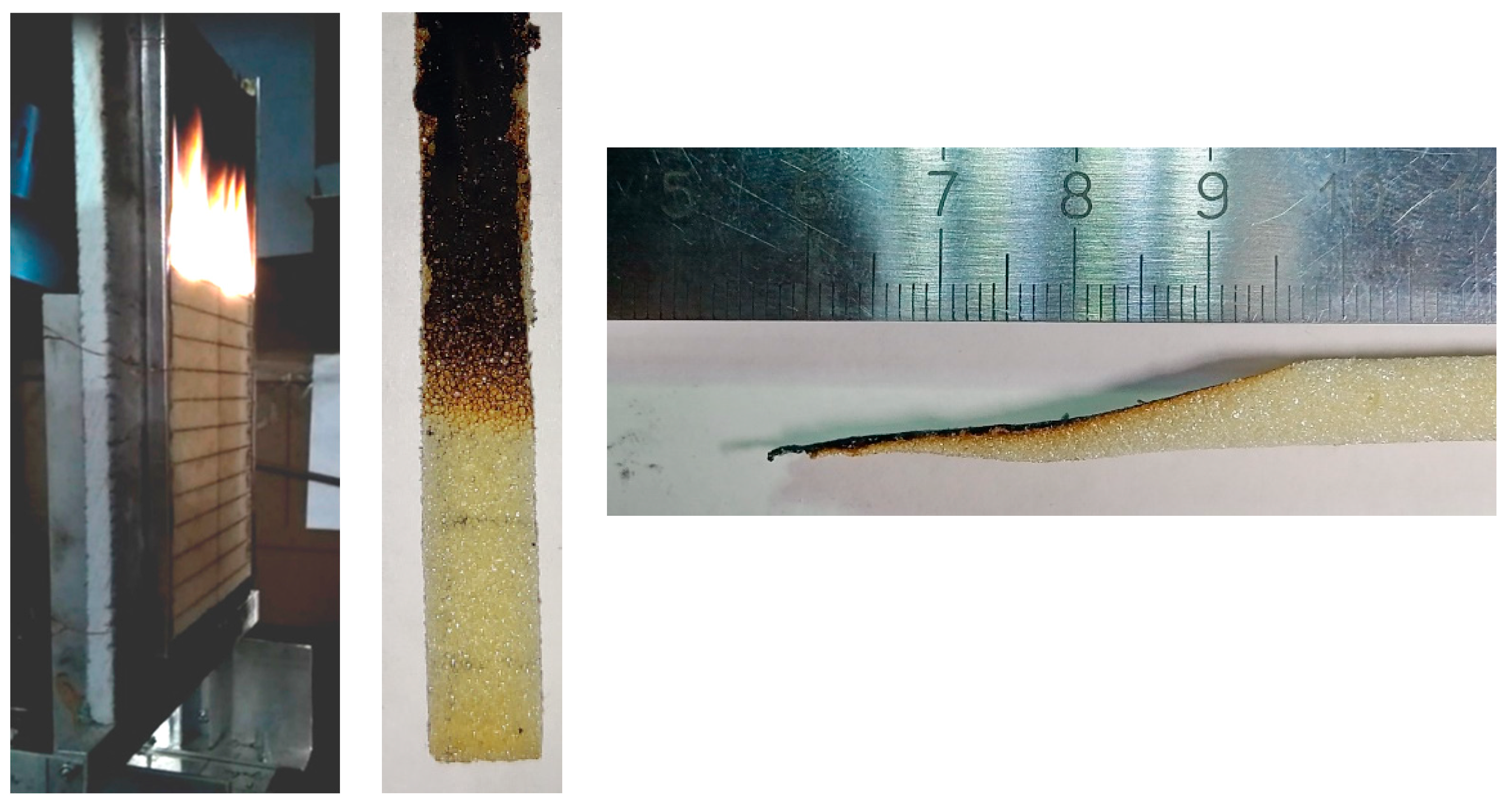
2. Experiment
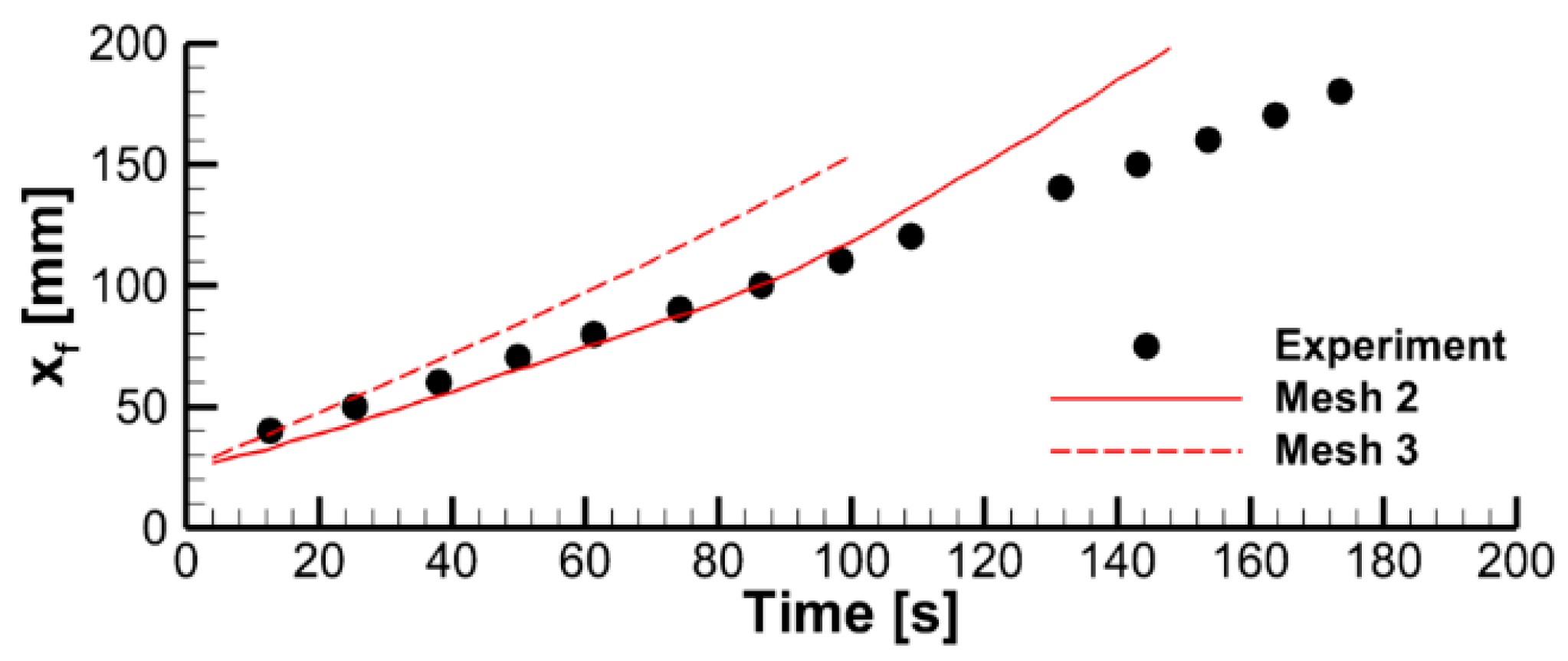
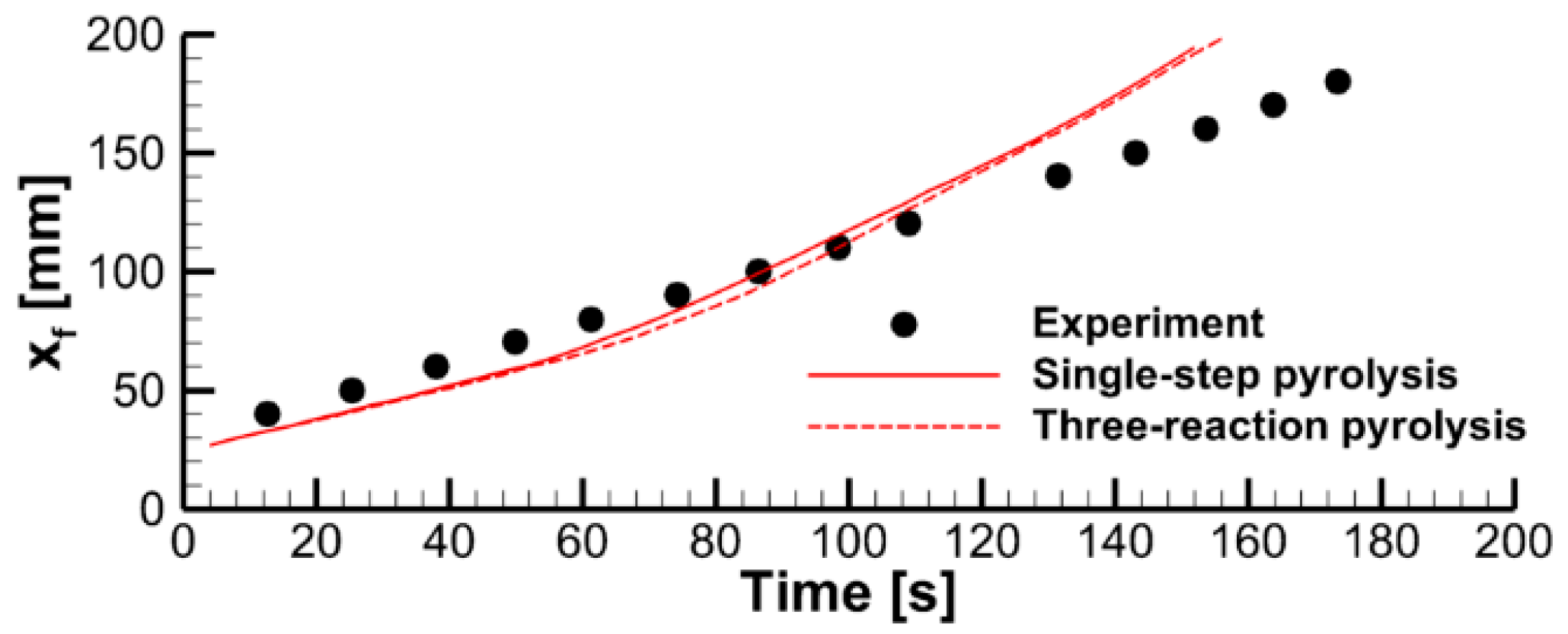
2.1. Flame Spread
2.2. Surface Incident Radiation and Surface Emissivity
3. Modeling and Simulations
3.1. Problem Setup
3.2. Solid Phase Model
3.2.1. Heat and Mass Transfer
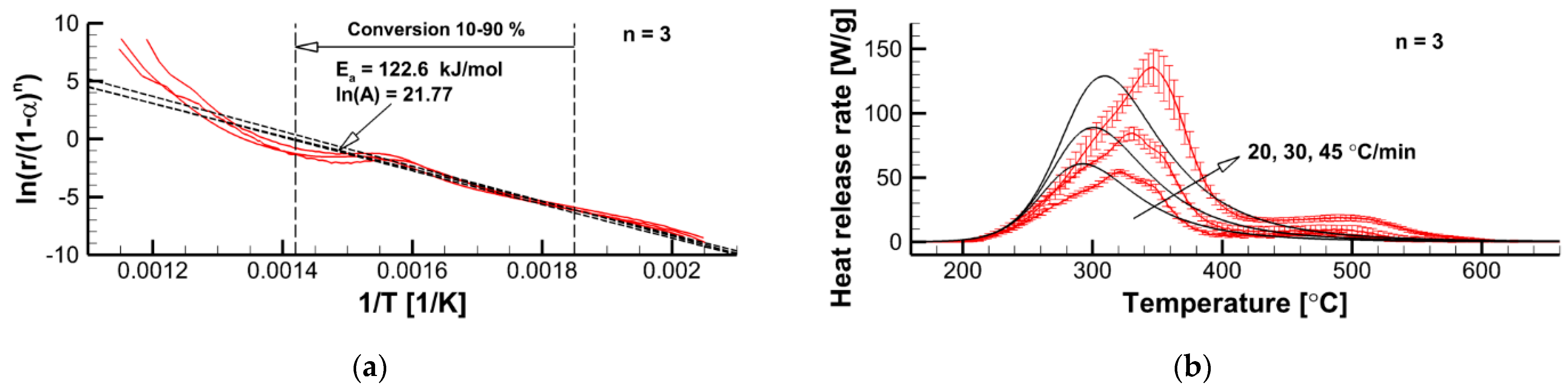
3.2.2. Formal Kinetics of Material Decomposition
- The first-order reaction model should be discarded for this experimental dataset.
- For ≈ 2 ( = 76.8 kJ/mol), the simulated curves attain its maximum at approximately the same temperatures as the experimental counterparts.
- Maximum reaction rates are best reproduced at = 2.5 ( = 98.9 kJ/mol).
- The decomposition onset is best replicated at = 3 ( = 122.6 kJ/mol).
- A further increase of the reaction order ( > 3) does not facilitate better agreement between the simulated and measured cures.
3.3. Gas Phase Model
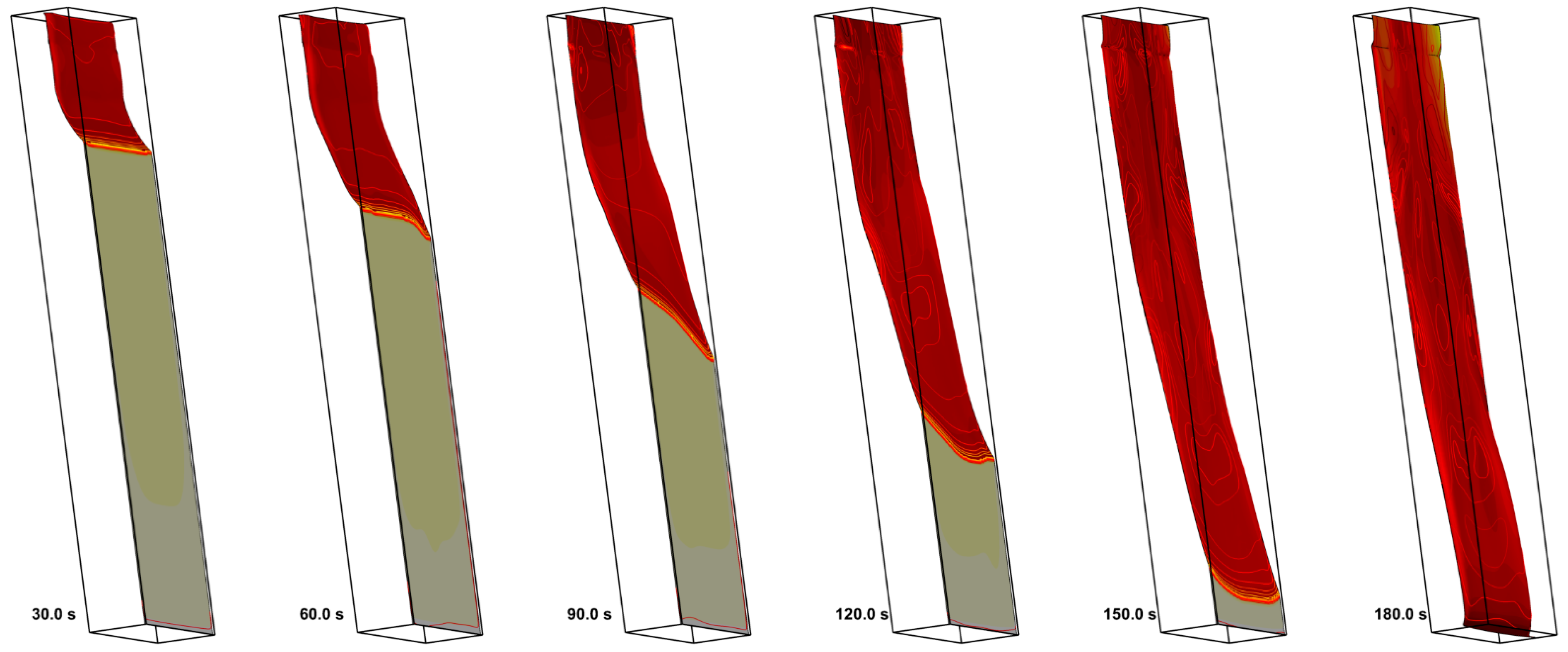
4. Result
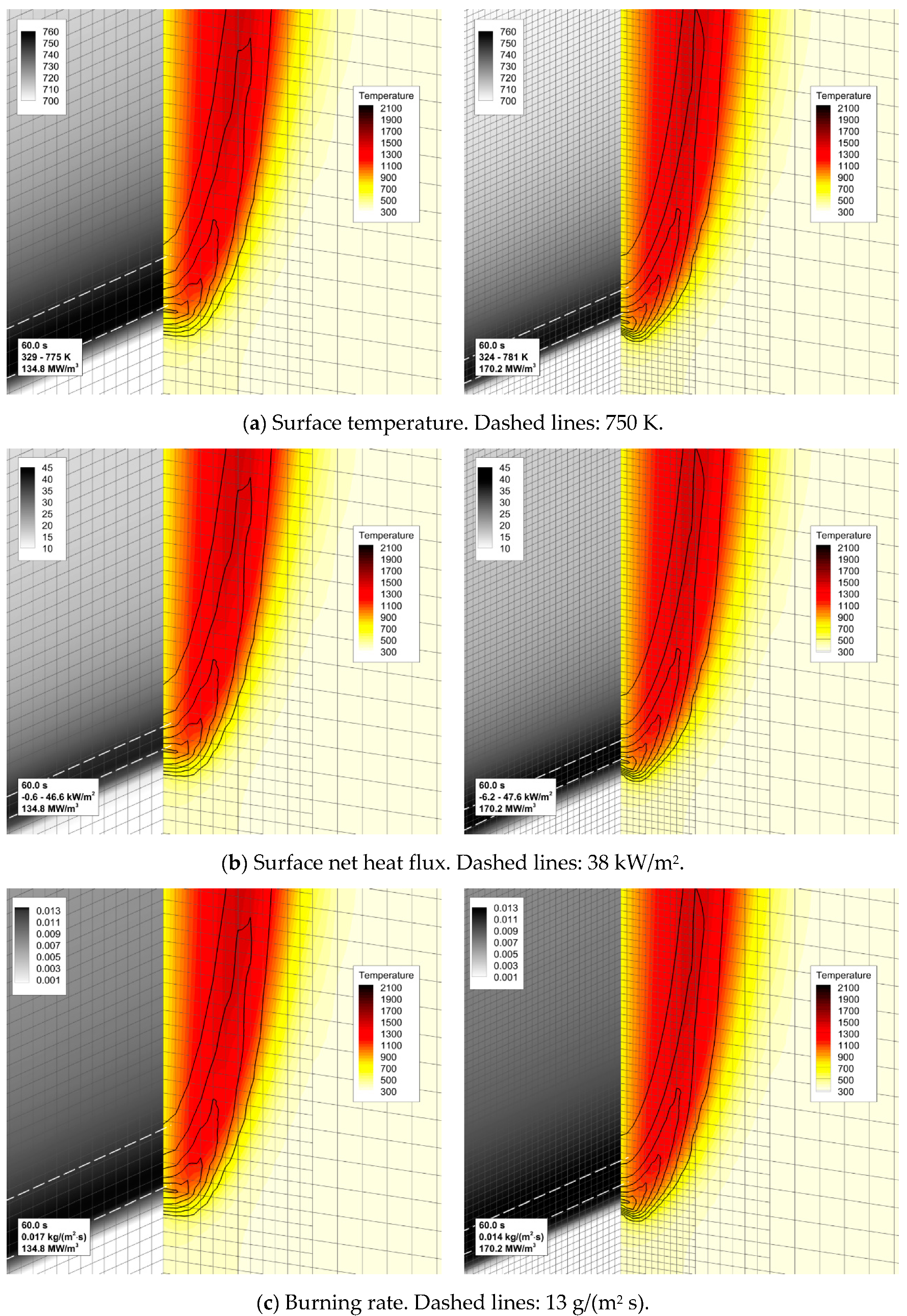
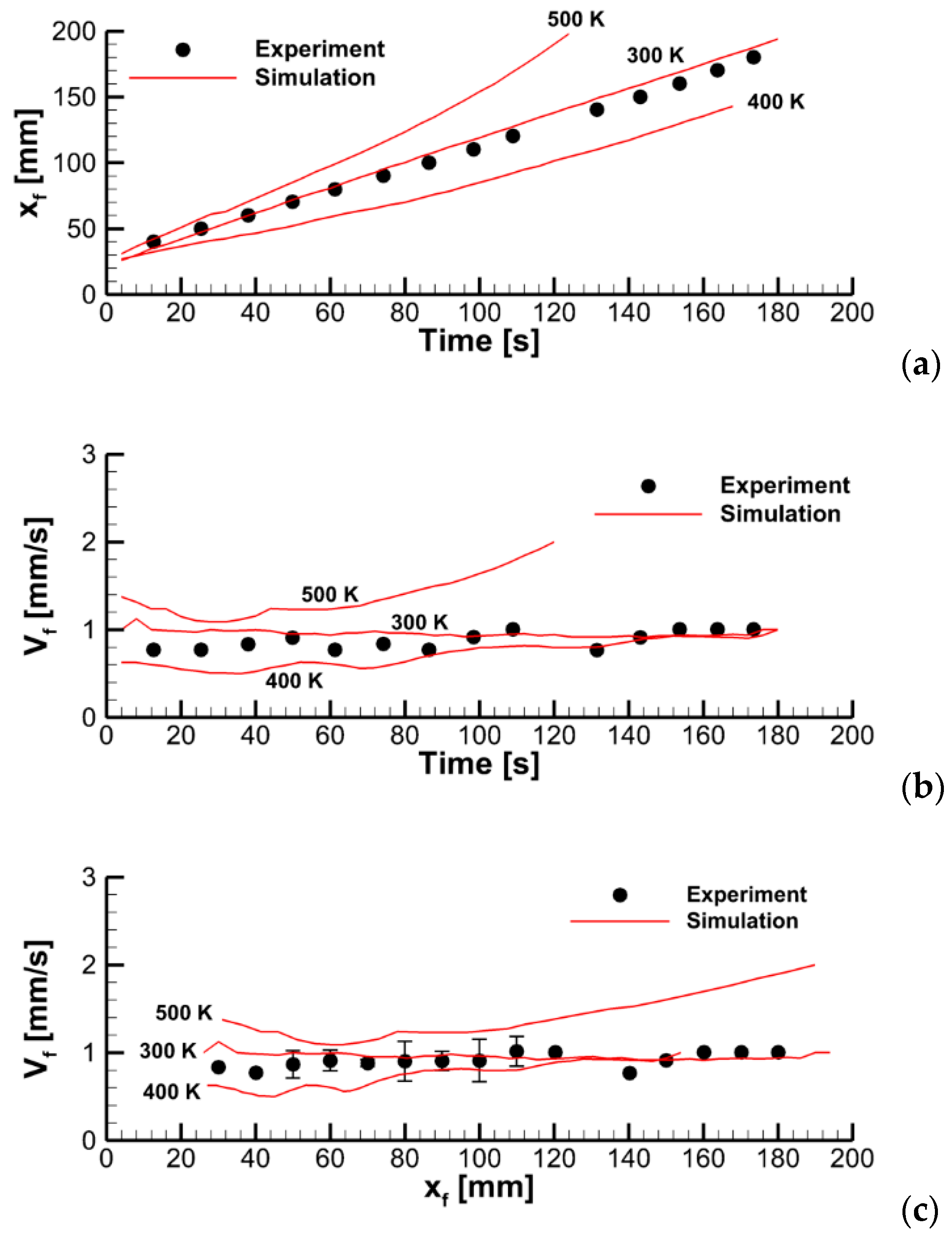
4.1. The Effect of Volatile Oxidation Kinetics
4.2. The Effect of Decomposition Kinetics of Combustible Material
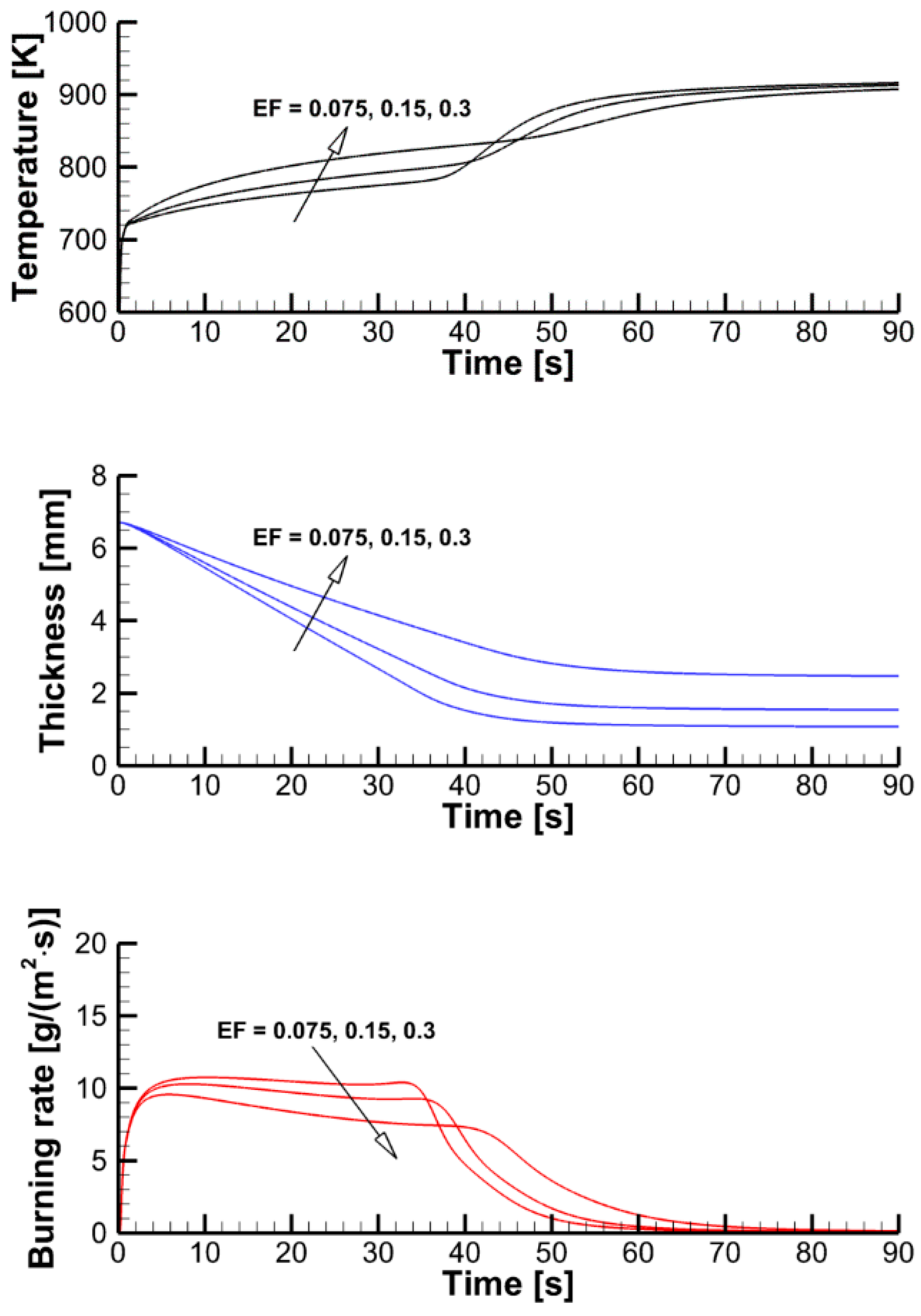
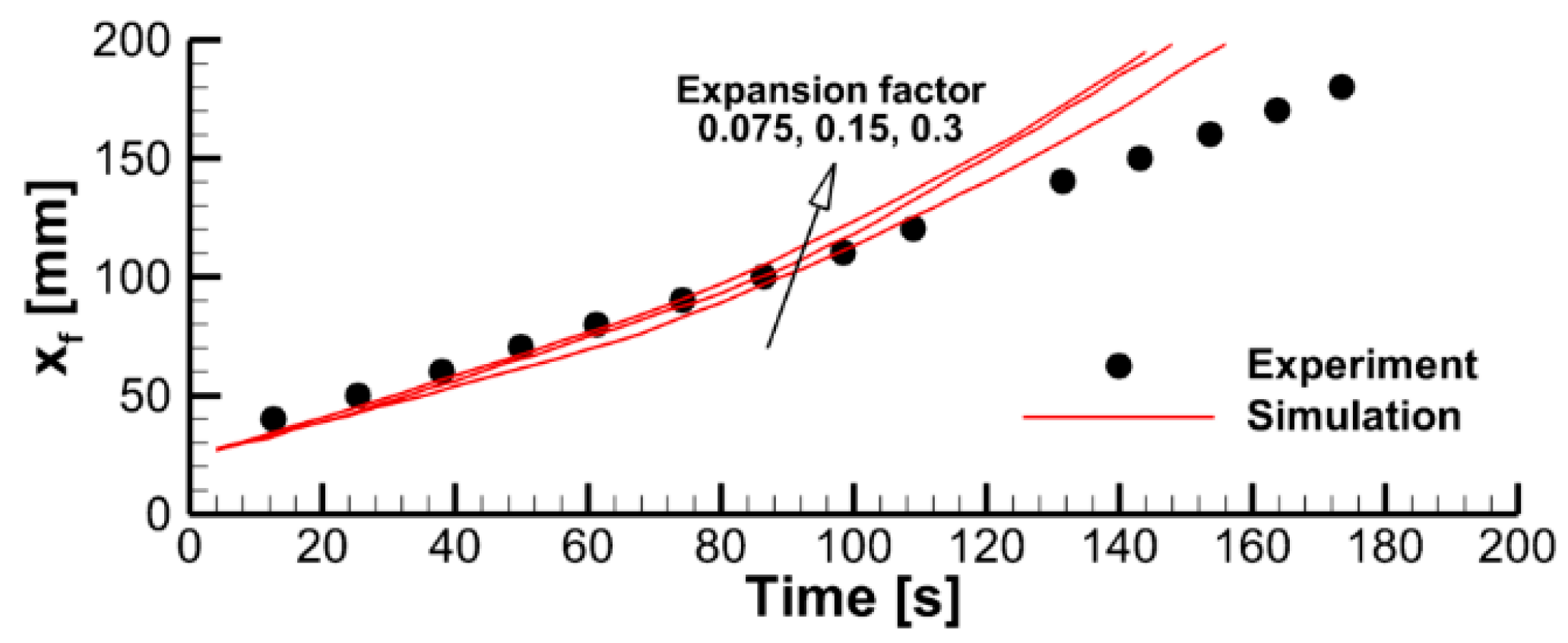
4.3. The Effect of External Heating
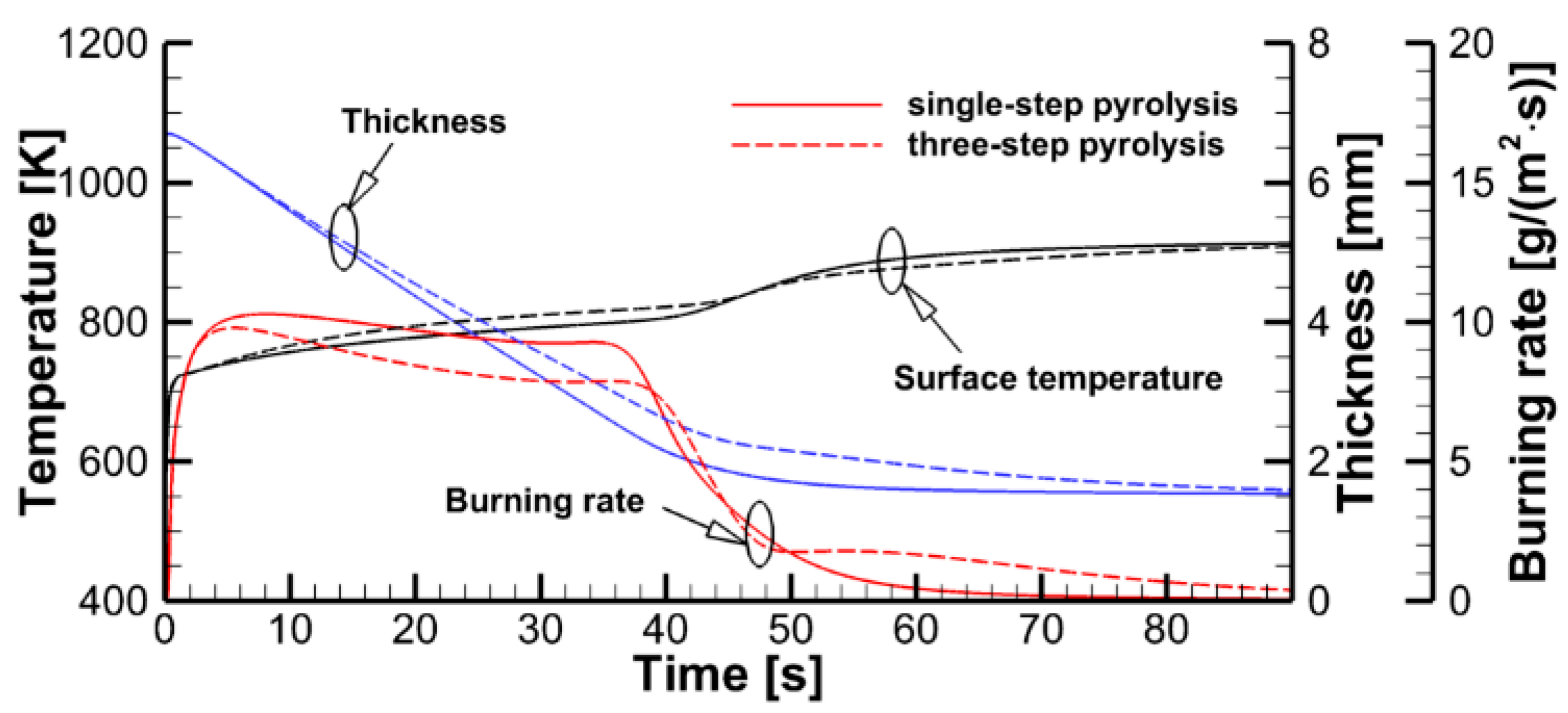
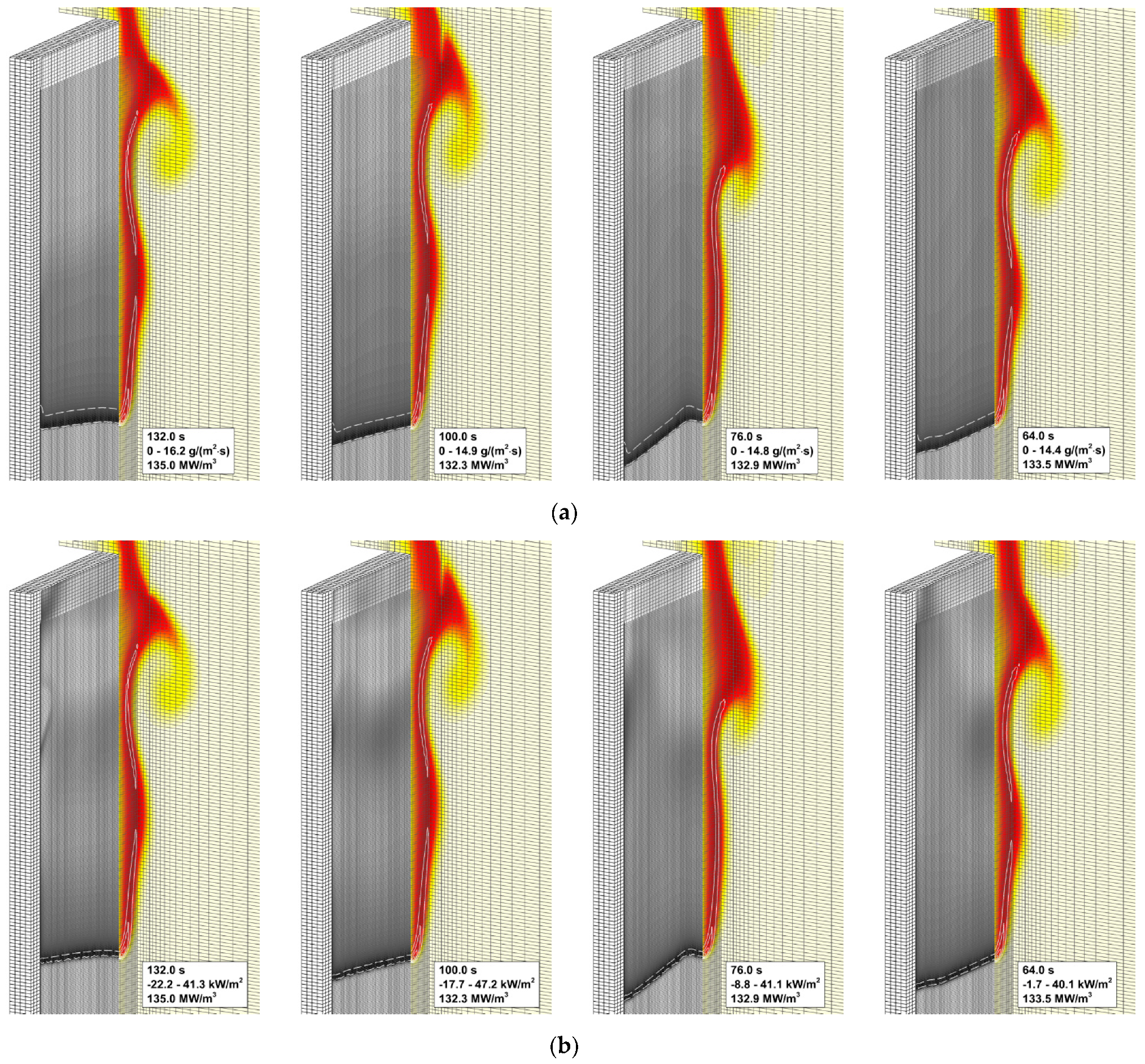
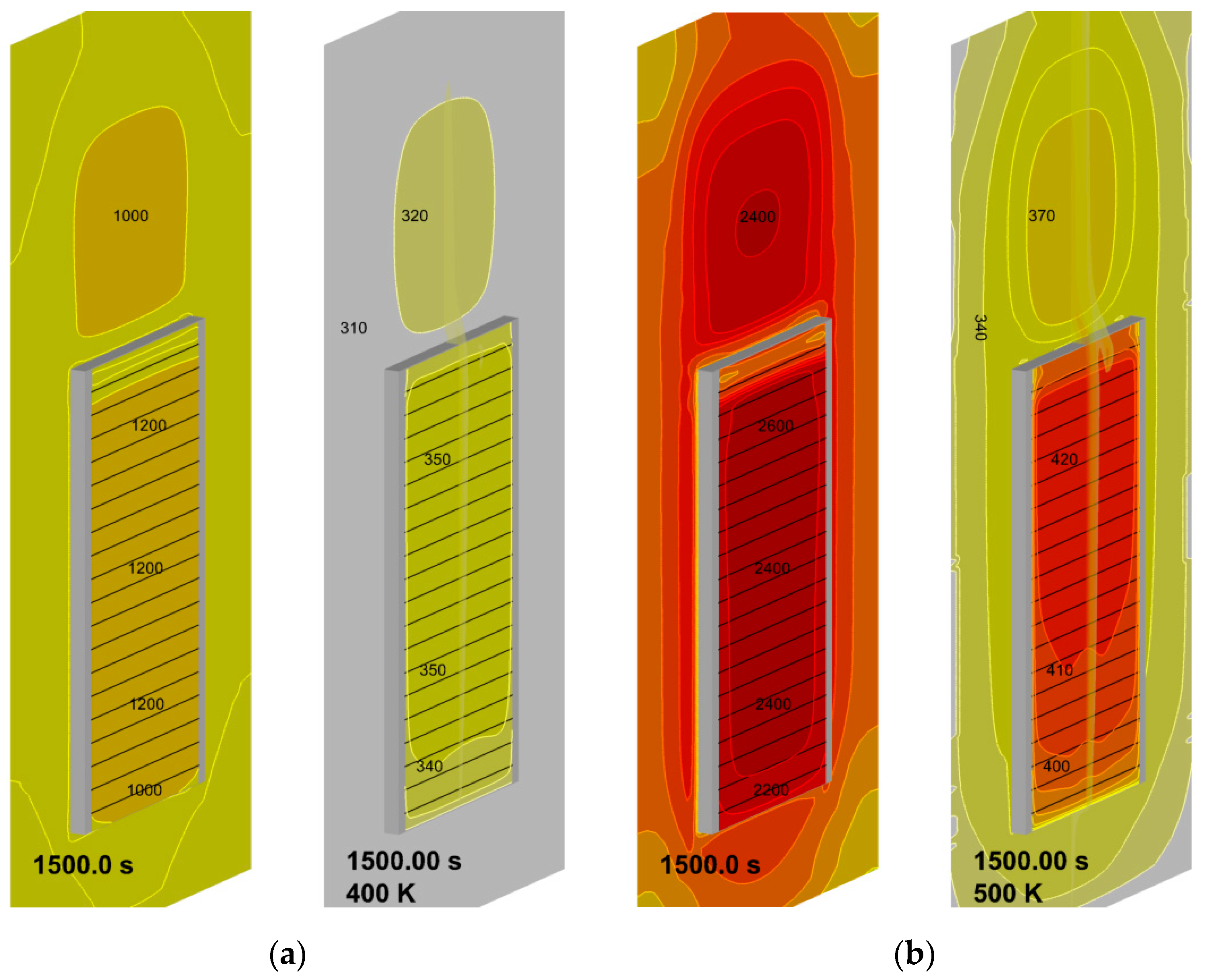
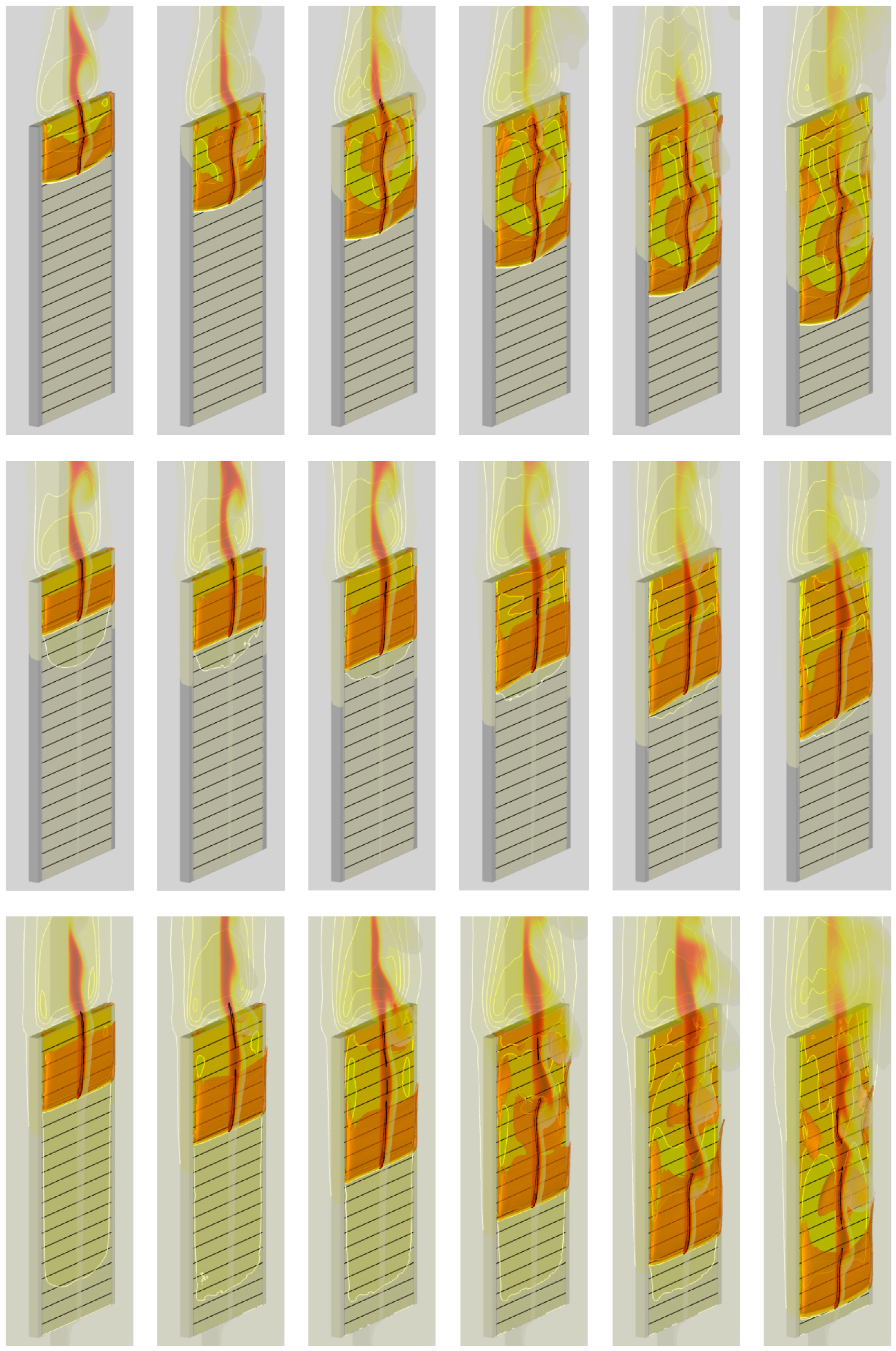
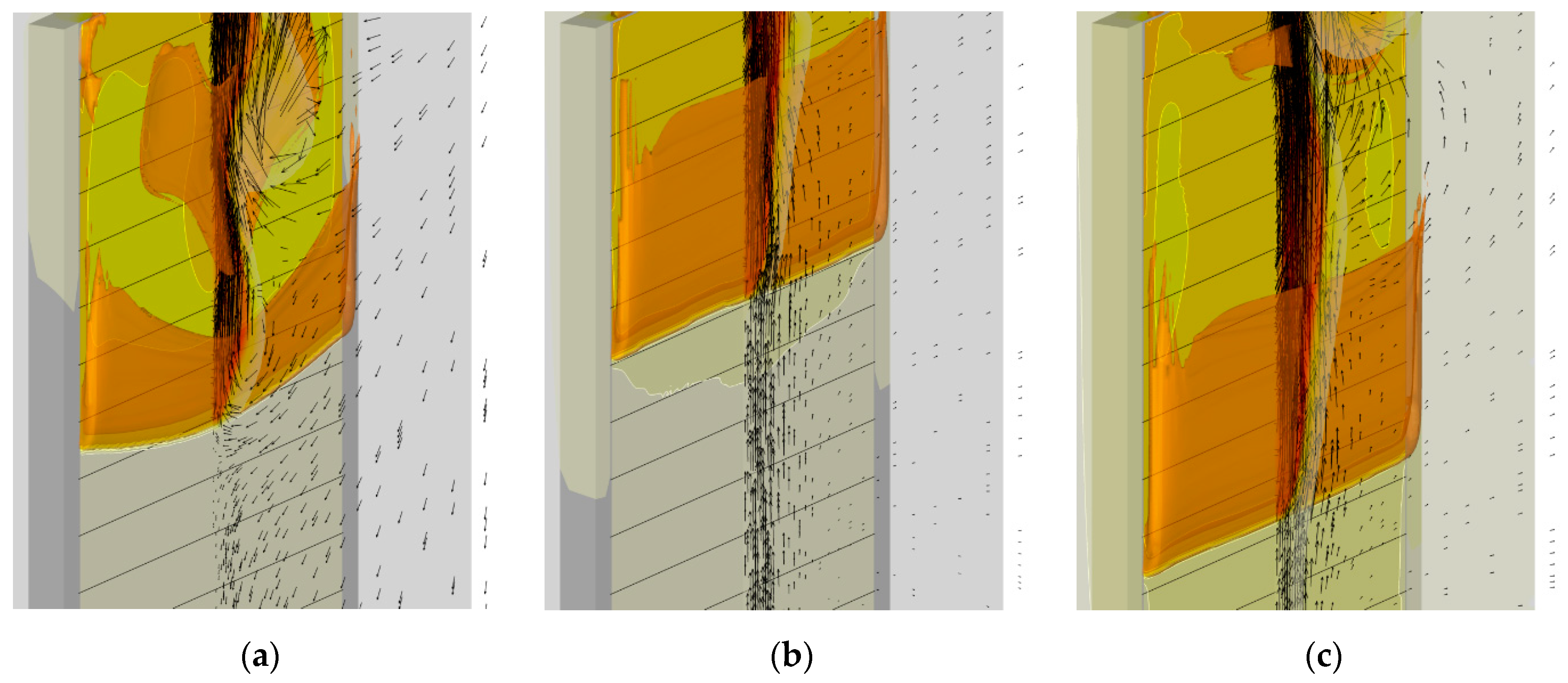
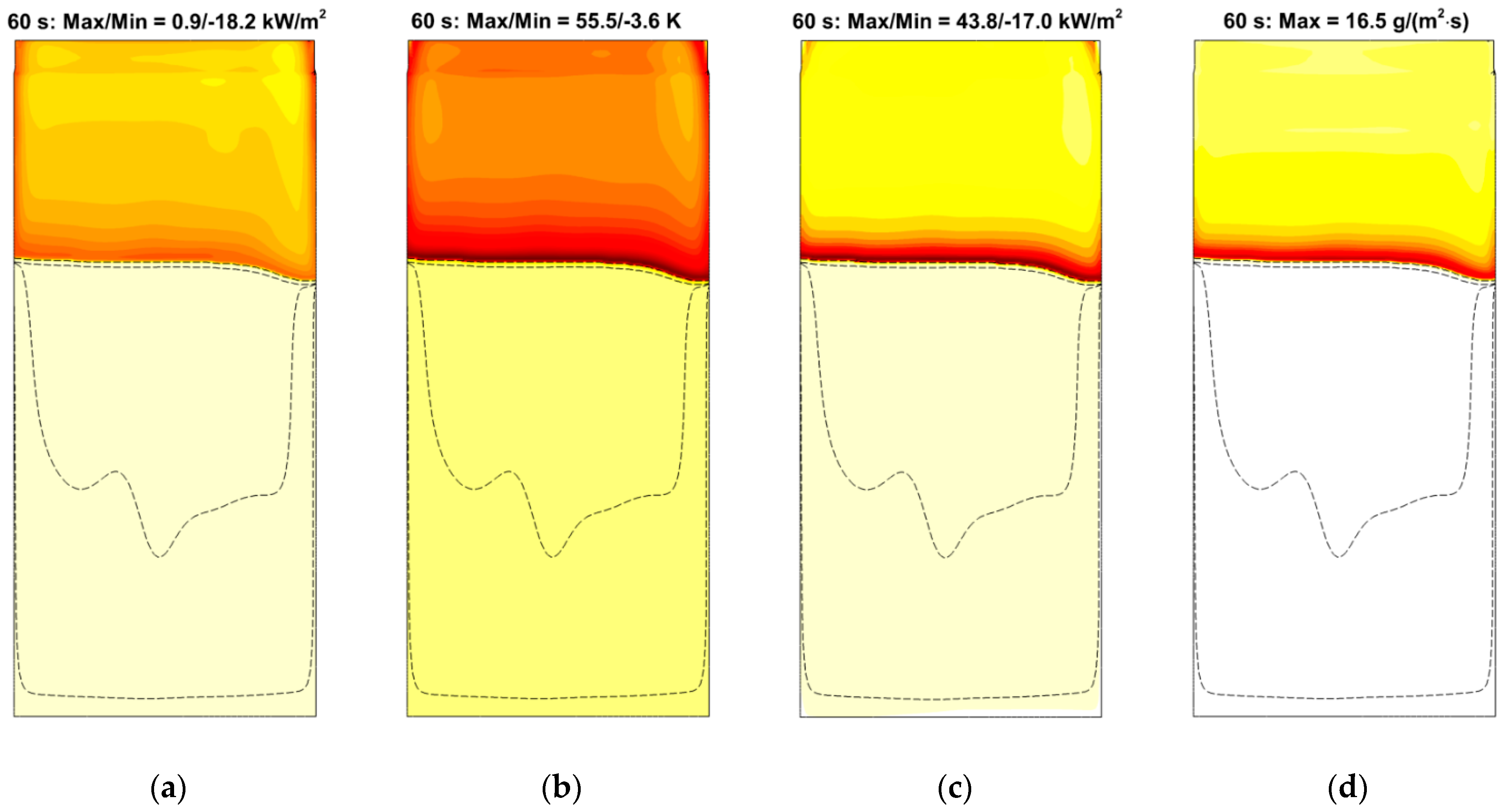
4.4. Flame Impact on the Burning Material
5. Concluding Remarks
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| pre-exponential factor (s−1) | |
| specific heat (J/(kg·K)) | |
| apparent activation energy (J/mol) | |
| expansion factor (-) | |
| conversion function (-) | |
| incident radiation (kW/m2) | |
| heat transfer coefficient (W/(m2·K)) | |
| thermal conductivity (W/(m·K)) | |
| mass flux of volatiles at the specimen surface (kg/(m2·s)) | |
| molar mass (kg/mol) | |
| heat flux (W/m2) | |
| specific heat release rate per unit mass of virgin material (W/kg) | |
| heat of combustion of volatile oxidation per unit mass of virgin material (J/kg) | |
| pyrolysis reaction rate (s−1) | |
| matrix polymer decomposition rate ((kg/s)/m2) | |
| universal gas constant, 8.314 J/(mol∙K) | |
| laminar burning velocity (m/s) | |
| time (s) | |
| volume of the mesh element (m3/m2) | |
| flame propagation velocity (m/s) | |
| gas flow velocity (m/s) | |
| weighting factor (-) | |
| flame front coordinate (m) | |
| mass fraction (-) | |
| Greek | |
| conversion (-) | |
| heating rate (K/s) | |
| emissivity (-) | |
| porosity (-) | |
| absorption coefficient (m−1) | |
| mass fraction of matrix polymer converted to volatiles (-) | |
| density (kg/m3) | |
| Stefan–Boltzmann constant, 5.67 × 10−8 W/(m2·K4) | |
| time scale (s) | |
| Subscripts | |
| convective | |
| char | |
| conductive | |
| critical | |
| flame | |
| fiber | |
| heat flux sensor | |
| gas; gasification | |
| incident | |
| solid component (mp, fib, ch) | |
| reaction number | |
| matrix polymer | |
| net value (radiative plus convective) | |
| pyrolysis | |
| radiative | |
| solid residue | |
| solid | |
| surface | |
| thermocouple | |
| initial; ambient | |
| final | |
| Abbreviations | |
| CFD | computational fluid dynamics |
| MCC | microscale combustion calorimetry |
| TGA | thermogravimetric analysis |
| TC | thermocouple |
References
- Quintiere, J.G. Surface Flame Spread. In SFPE Handbook of Fire Protection Engineering, 3rd ed.; NFPA: Quincy, MA, USA, 2002. [Google Scholar]
- Snegirev, A.Y.; Kuznetsov, E.A.; Korobeinichev, O.P.; Shmakov, A.G.; Trubachev, S.A. Ignition and burning of the composite sample impacted by the Bunsen burner flame: A fully coupled simulation. Fire Saf. J. 2021, 127, 103507. [Google Scholar] [CrossRef]
- Quintiere, J.G.; Harkleroad, M.T. New Concepts for Measuring Flame Spread Properties. In Fire Safety: Science and Engineering; Harmathy, T., Ed.; ASTM International: West Conshohocken, PA, USA, 1985; pp. 239–267. [Google Scholar]
- Bhattacharjee, S.; King, M.D.; Paolini, C. Structure of downward spreading flames: A comparison of numerical simulation, experimental results and a simplified parabolic theory. Combust. Theory Model. 2004, 8, 23–39. [Google Scholar] [CrossRef]
- Long, Y.; Wichman, I.S. Theoretical and numerical analysis of a spreading opposed-flow diffusion flame. Proc. R. Soc. A 2009, 465, 3209–3238. [Google Scholar] [CrossRef] [Green Version]
- Korobeinichev, O.P.; Karpov, A.I.; Bolkisev, A.A.; Shaklein, A.A.; Gonchikzhapov, M.B.; Paletsky, A.A.; Tereshchenko, A.G.; Shmakov, A.G.; Gerasimov, I.E.; Kumar, A. An experimental and numerical study of thermal and chemical structure of downward flame spread over PMMA surface in still air. Proc. Combust. Inst. 2019, 37, 4017–4024. [Google Scholar] [CrossRef]
- Korobeinichev, O.; Glaznev, R.; Karpov, A.; Shaklein, A.; Shmakov, A.; Paletsky, A.; Trubachev, S.; Hu, Y.; Wang, X.; Hu, W. An experimental study and numerical simulation of horizontal flame spread over polyoxymethylene in still air. Fire Saf. J. 2020, 111, 102924. [Google Scholar] [CrossRef]
- Joshi, A.K.; Kumar, A.; Raghavan, V.; Trubachev, S.A.; Shmakov, A.G.; Korobeinichev, O.P.; Kumar, P.B. Numerical and experimental study of downward flame spread along multiple parallel fuel sheets. Fire Saf. J. 2021, 125, 103414. [Google Scholar] [CrossRef]
- Korobeinichev, O.P.; Trubachev, S.A.; Joshi, A.K.; Kumar, A.; Paletsky, A.A.; Tereshchenko, A.G.; Shmakov, A.G.; Glaznev, R.K.; Raghavan, V.; Mebel, A.M. Experimental and numerical studies of downward flame spread over PMMA with and without addition of tri phenyl phosphate. Proc. Combust. Inst. 2021, 38, 4867–4875. [Google Scholar] [CrossRef]
- Kumar, C.; Kumar, A. On the role of radiation and dimensionality in predicting flow opposed flame spread over thin fuels, Combust. Theory Model. 2012, 16, 537–569. [Google Scholar] [CrossRef]
- Snegirev, A.; Talalov, V.; Stepanov, V.; Harris, J. A new model to predict pyrolysis, ignition and burning of flammable materials in fire tests. Fire Saf. J. 2013, 59, 132–158. [Google Scholar] [CrossRef]
- Ito, A.; Kashiwagi, T. Characterization of flame spread over PMMA using holographic interferometry sample orientation effects. Combust. Flame 1988, 71, 189–204. [Google Scholar] [CrossRef]
- Wichman, I.S. Theory of opposed-flow flame spread. Progr. Energy Combust. Sci. 1992, 18, 553–593. [Google Scholar] [CrossRef]
- Snegirev, A.Y.; Talalov, V.A.; Stepanov, V.V.; Korobeinichev, O.P.; Gerasimov, I.E.; Shmakov, A.G. Autocatalysis in thermal decomposition of polymers. Polym. Degrad. Stability 2017, 137, 151–161. [Google Scholar] [CrossRef]
- Snegirev, A.; Talalov, V.; Stepanov, V.; Harris, J. Formal kinetics of polymer pyrolysis for modeling of ignition and burning in fire tests. In Proceedings of the 13th International Conference Interflam 2013, Royal Holloway College University ofLondon, London, UK, 24–26 June 2013; Interscience Communications: London, UK, 2013; pp. 755–768. [Google Scholar]
- Kuznetsov, E.A.; Snegirev, A.Y.; Markus, E.S. Radiative extinction of laminar diffusion flame above the flat porous burner in microgravity: A computational study. Combust. Explos. Shock Waves 2020, 56, 394–411. [Google Scholar] [CrossRef]
- Brookes, S.J.; Moss, J.B. Prediction of Soot and Thermal Radiation in Confined Turbulent Jet Diffusion Flames. Combust. Flame 1999, 116, 486–503. [Google Scholar] [CrossRef]
- Snegirev, A.; Markus, E.; Kuznetsov, E.; Harris, J.; Wu, T. On soot and radiation modeling in buoyant turbulent diffusion flames. Heat and Mass Transfer 2018, 54, 2275–2293. [Google Scholar] [CrossRef]
- Lee, K.B.; Thring, M.W.; Beer, J.M. On the Rate of Combustion of Soot in a Laminar Soot Flame, Combust. Flame 1962, 6, 137–145. [Google Scholar] [CrossRef]





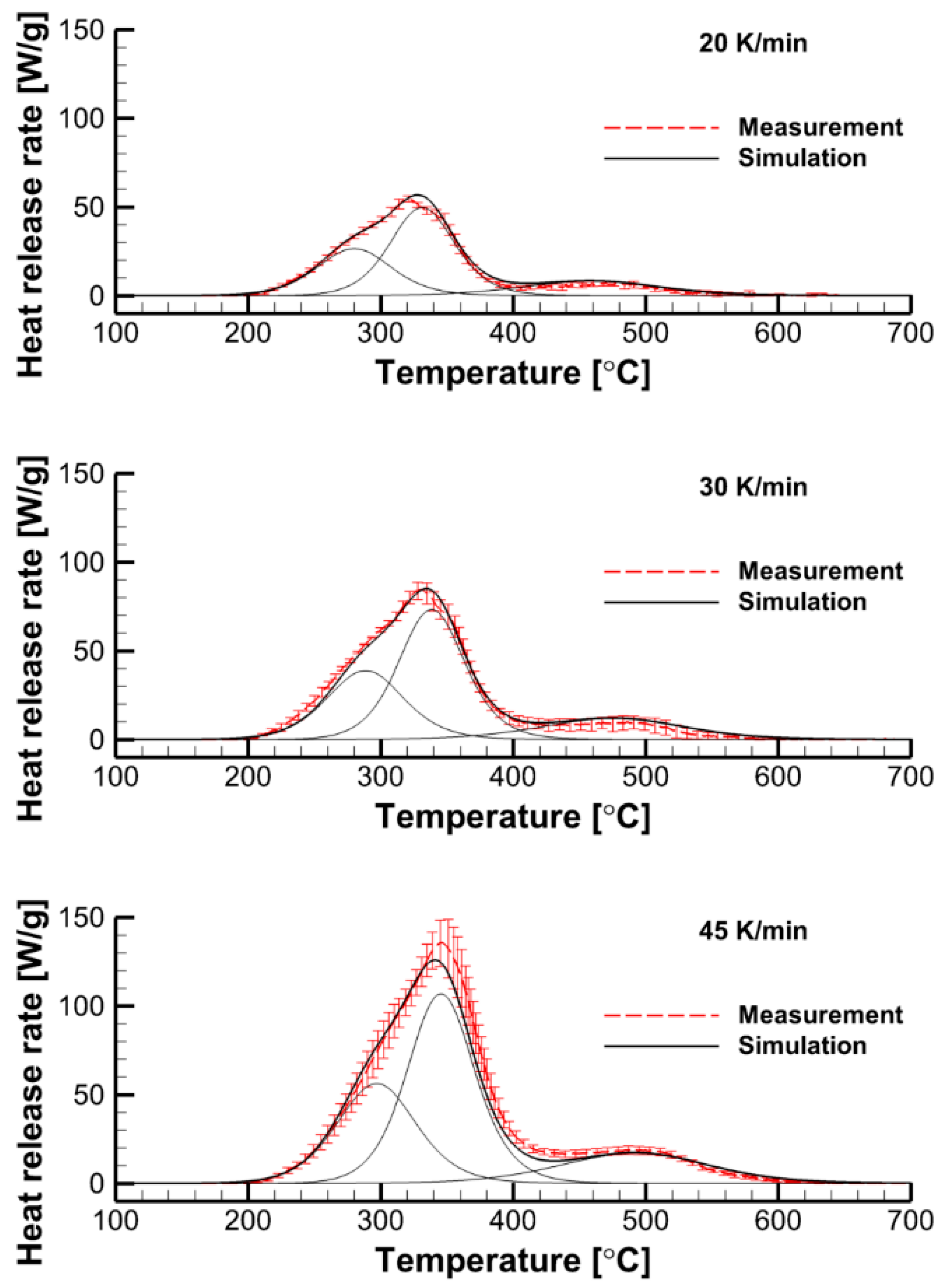






| Reaction | , kJ/mol | , kJ/kg | |||
|---|---|---|---|---|---|
| 1 | 1.7 | 20.98 | 116.0 | 0.314 | 5.95 |
| 2 | 1.8 | 29.43 | 168.2 | 0.509 | 9.64 |
| 3 | 1.5 | 11.78 | 101.8 | 0.177 | 3.36 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Snegirev, A.; Kuznetsov, E.; Korobeinichev, O.; Shmakov, A.; Paletsky, A.; Shvartsberg, V.; Trubachev, S. Fully Coupled Three-Dimensional Simulation of Downward Flame Spread over Combustible Material. Polymers 2022, 14, 4136. https://doi.org/10.3390/polym14194136
Snegirev A, Kuznetsov E, Korobeinichev O, Shmakov A, Paletsky A, Shvartsberg V, Trubachev S. Fully Coupled Three-Dimensional Simulation of Downward Flame Spread over Combustible Material. Polymers. 2022; 14(19):4136. https://doi.org/10.3390/polym14194136
Chicago/Turabian StyleSnegirev, A., E. Kuznetsov, O. Korobeinichev, A. Shmakov, A. Paletsky, V. Shvartsberg, and S. Trubachev. 2022. "Fully Coupled Three-Dimensional Simulation of Downward Flame Spread over Combustible Material" Polymers 14, no. 19: 4136. https://doi.org/10.3390/polym14194136
APA StyleSnegirev, A., Kuznetsov, E., Korobeinichev, O., Shmakov, A., Paletsky, A., Shvartsberg, V., & Trubachev, S. (2022). Fully Coupled Three-Dimensional Simulation of Downward Flame Spread over Combustible Material. Polymers, 14(19), 4136. https://doi.org/10.3390/polym14194136







