Effect of Stacking Sequence on Fatigue Performance of CFRP–Al Single-Lap Adhesive Joints: Experimental Study
Abstract
1. Introduction
2. Materials and Methods
2.1. Materials
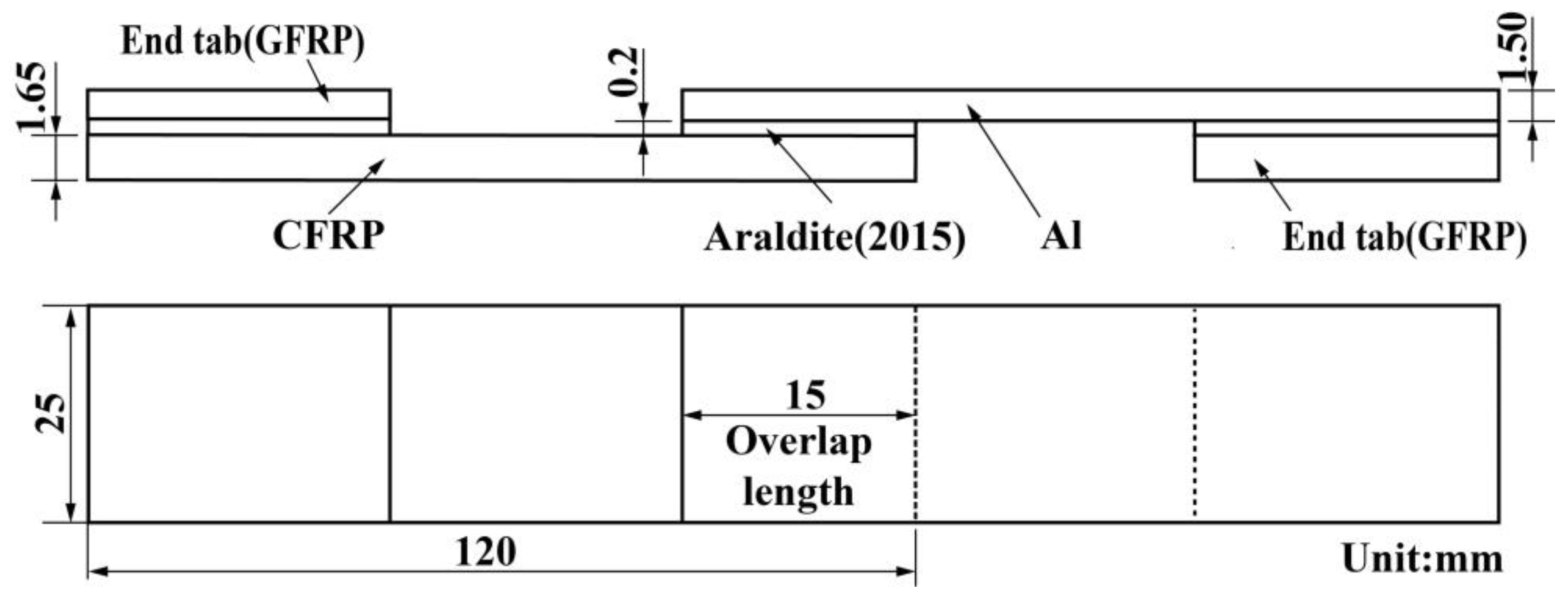
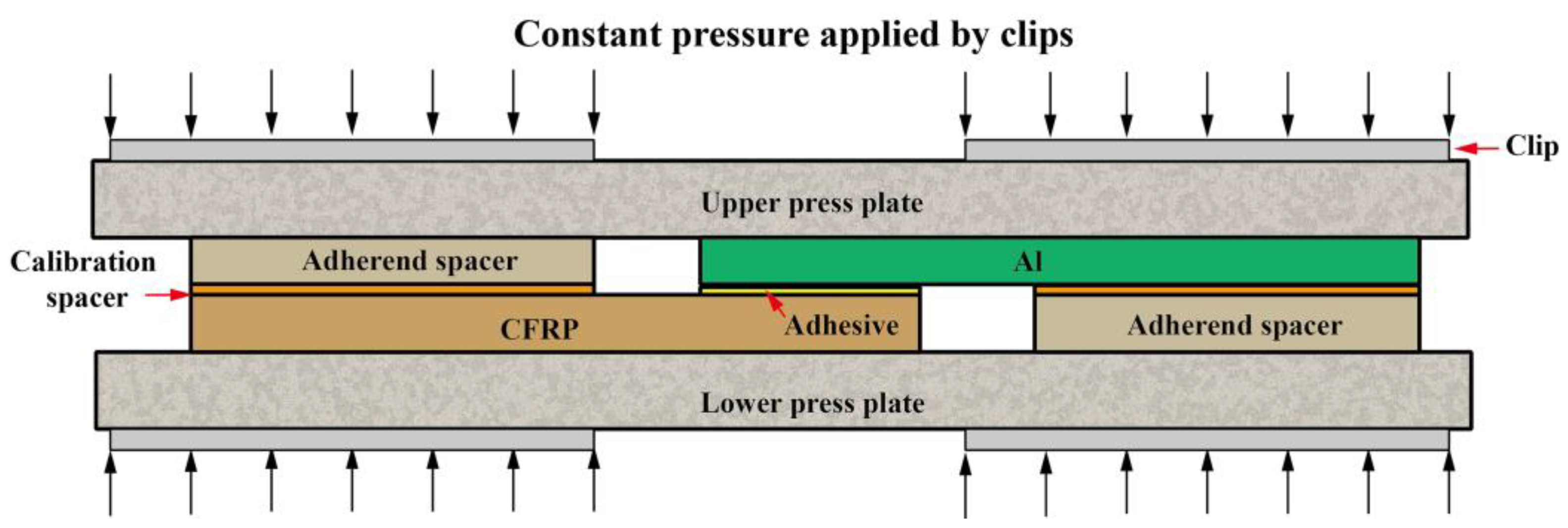
2.2. Preparation of Specimens
2.3. Test Instruments
2.3.1. Quasi-Static Tensile Test
2.3.2. Fatigue Test
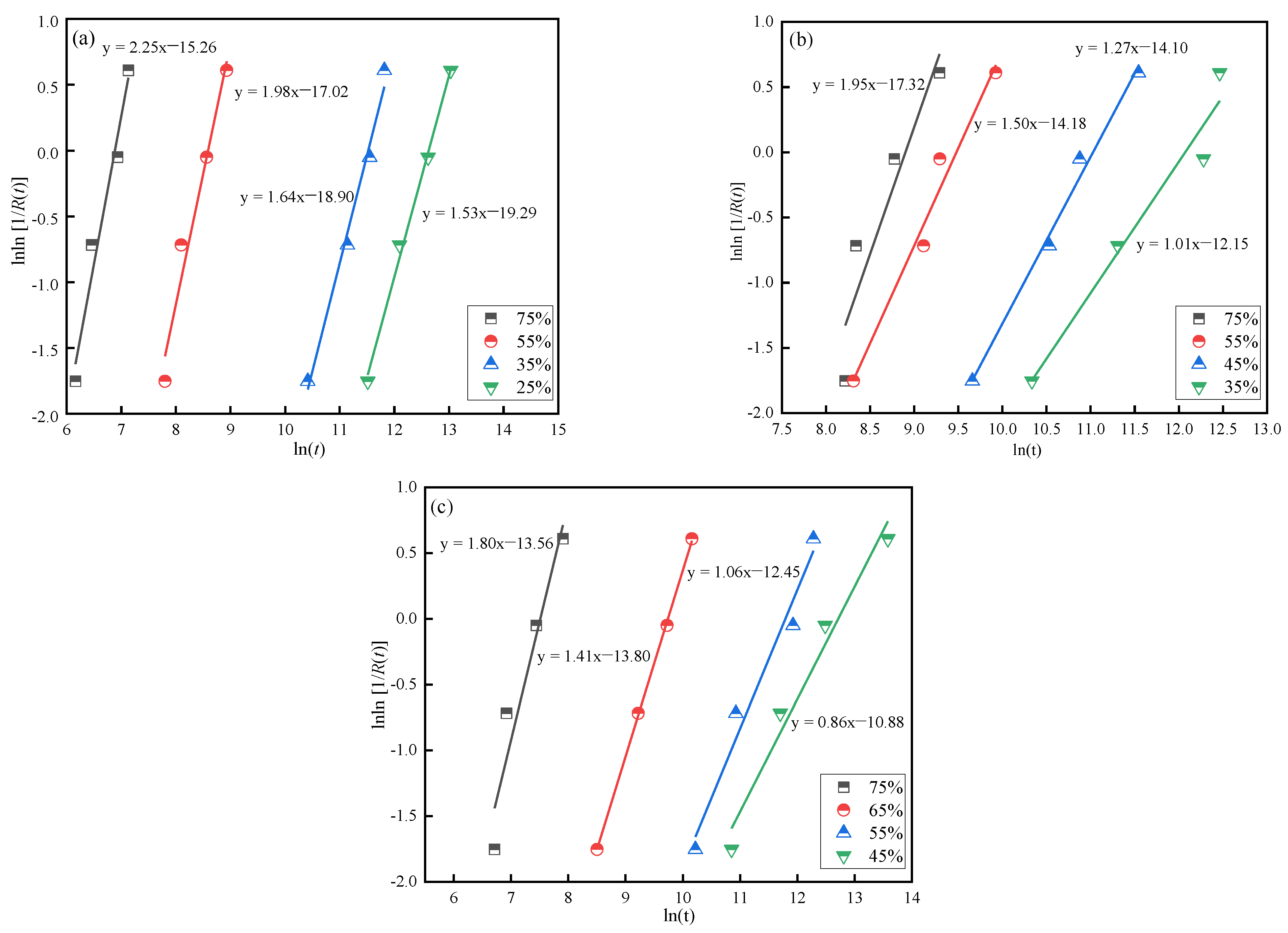
2.3.3. Fatigue Analysis
3. Results and Discussion
3.1. Quasi-Static Tensile Behaviour
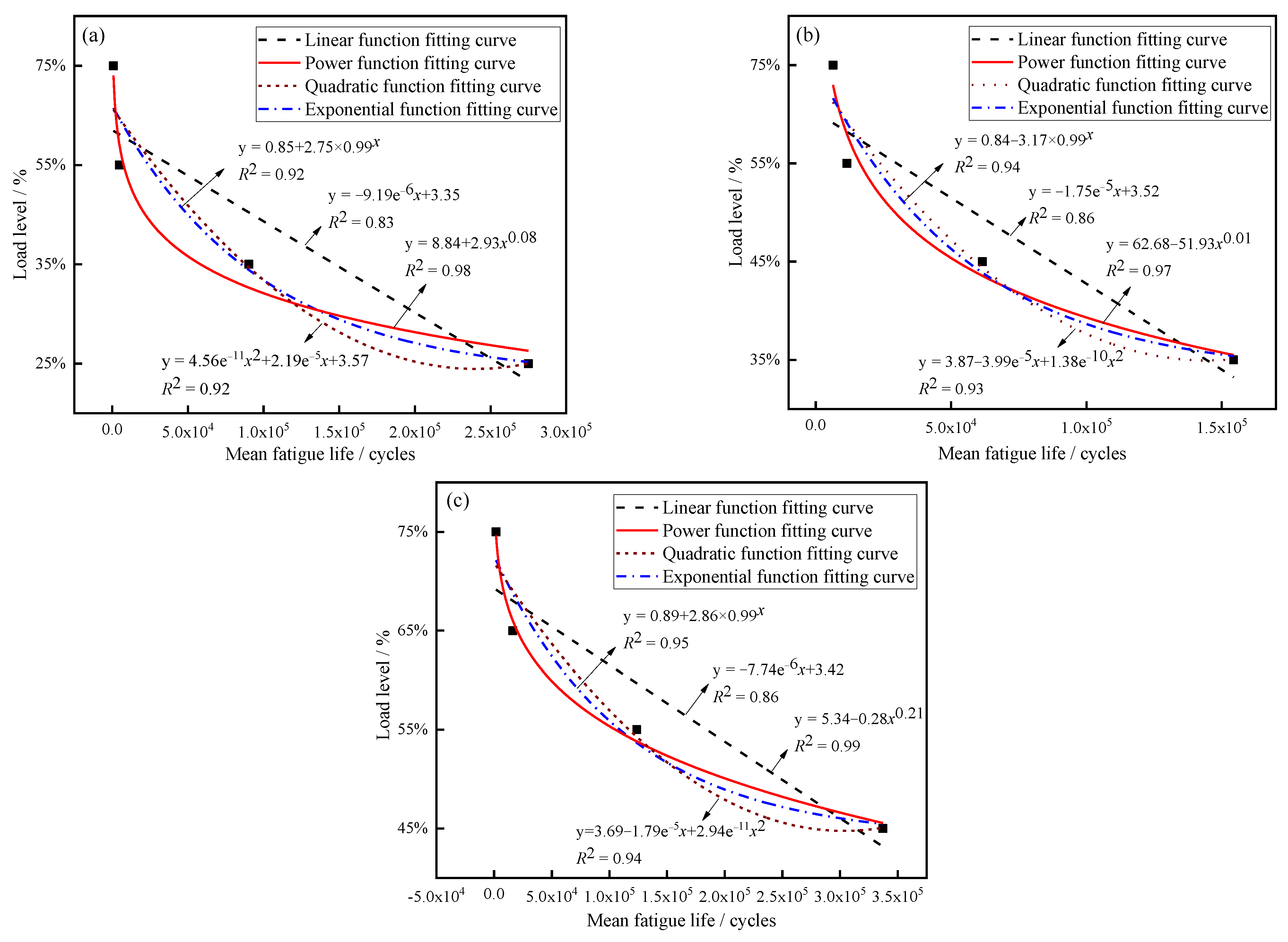
3.2. Fatigue Life
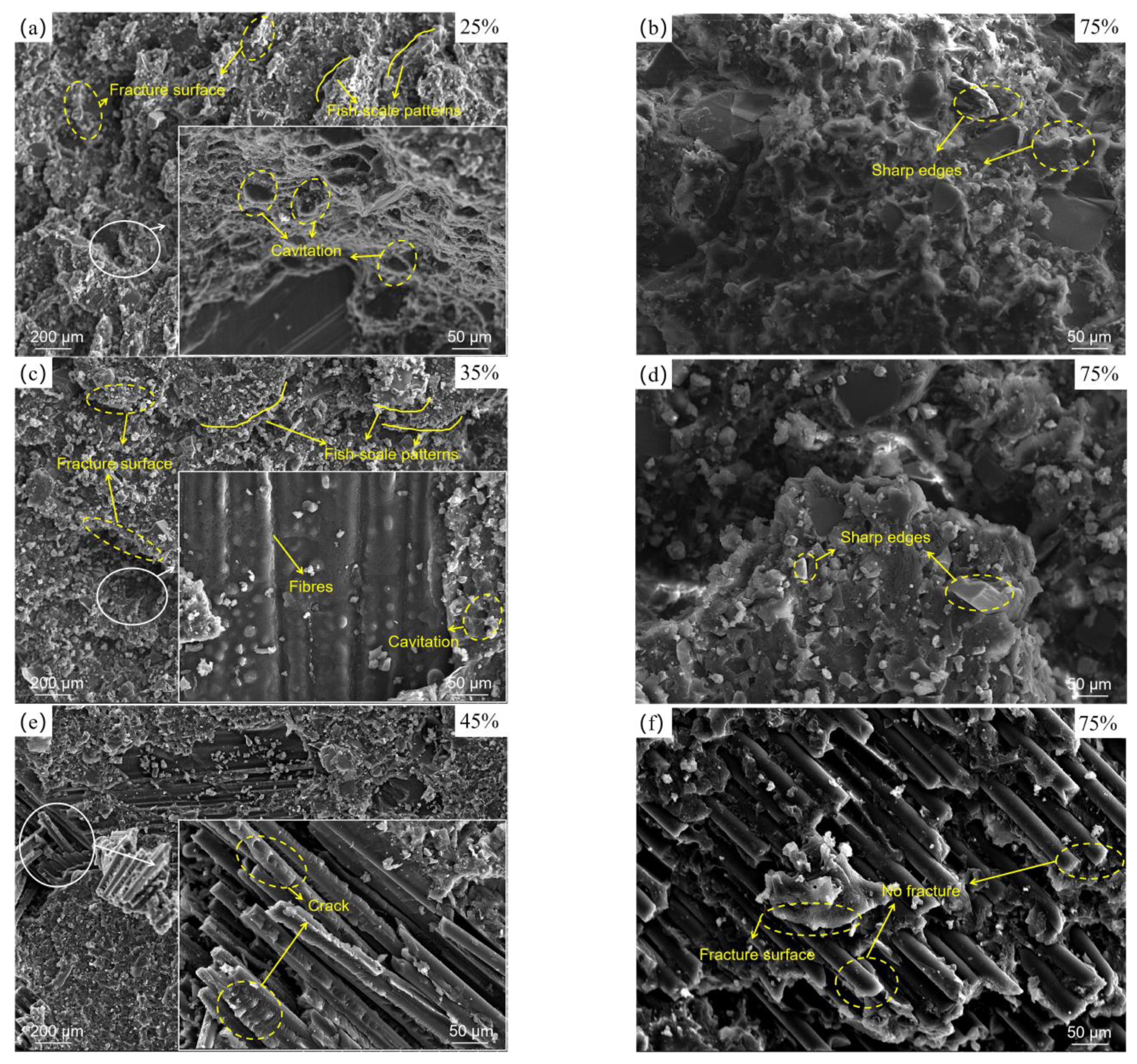
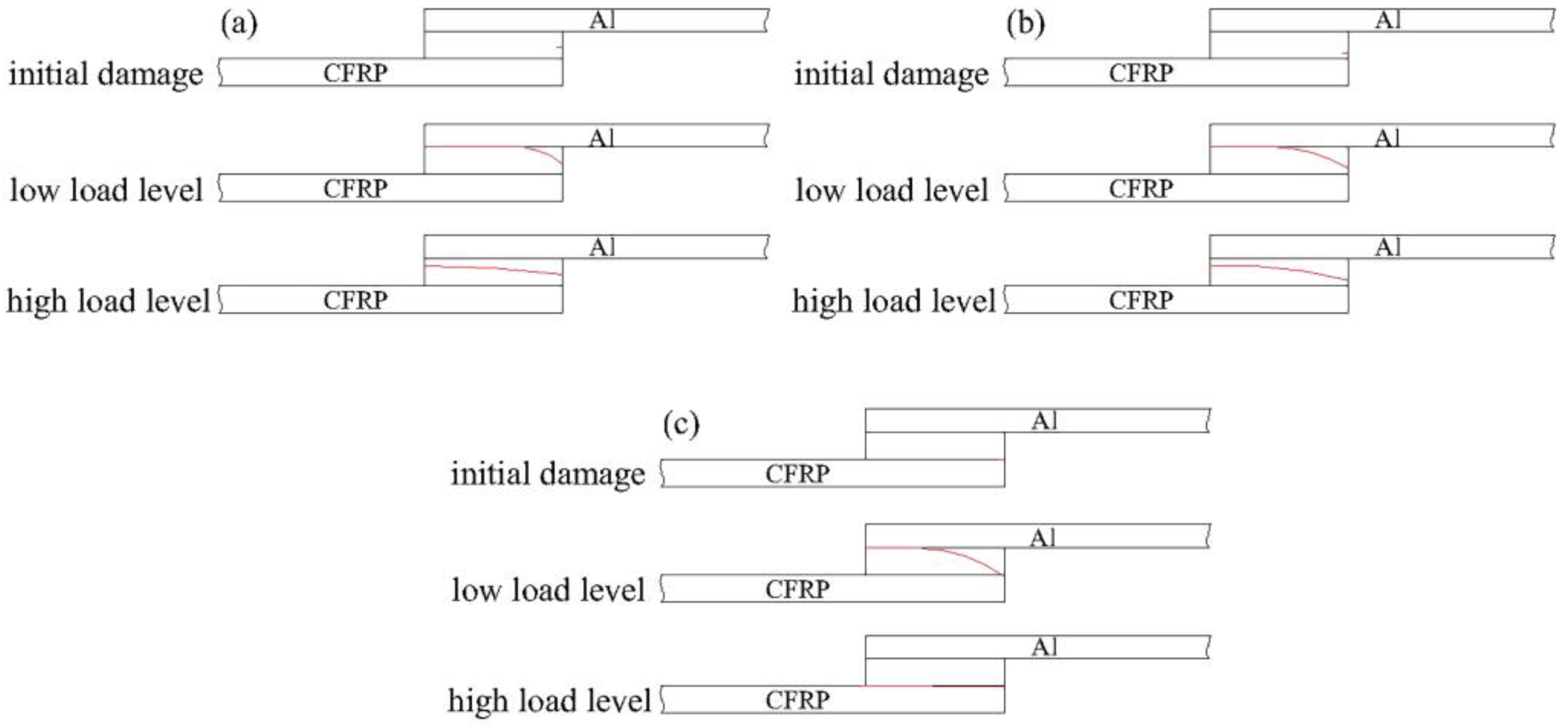
3.3. Failure Mode
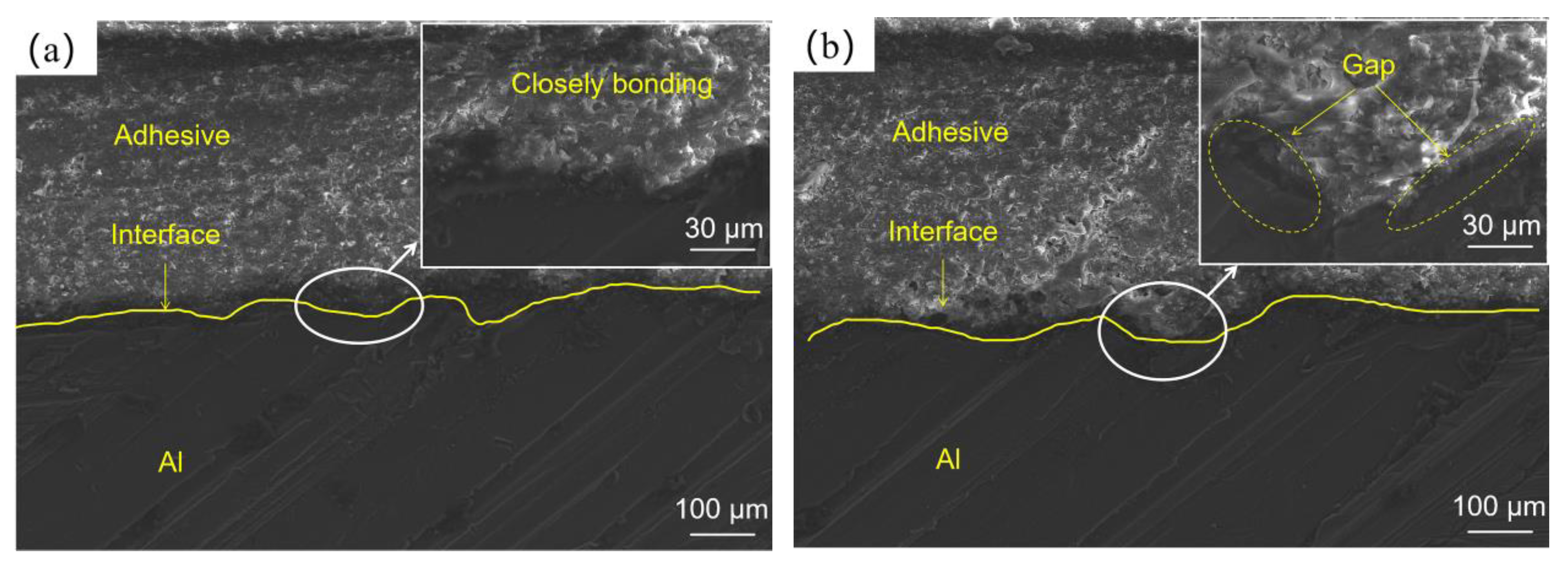
3.4. Fracture Analysis
4. Conclusions
- (1)
- Stacking sequence can influence fracture mode of a joint under quasi-static loading. The brittle fracture of J-[0/90]4s and J-[0/45/−45/90]2s occurred due to 0° fibres undertaking the main load, while the ductile fracture of J-[45/−45]4s occurred because the matrix bore the main load.
- (2)
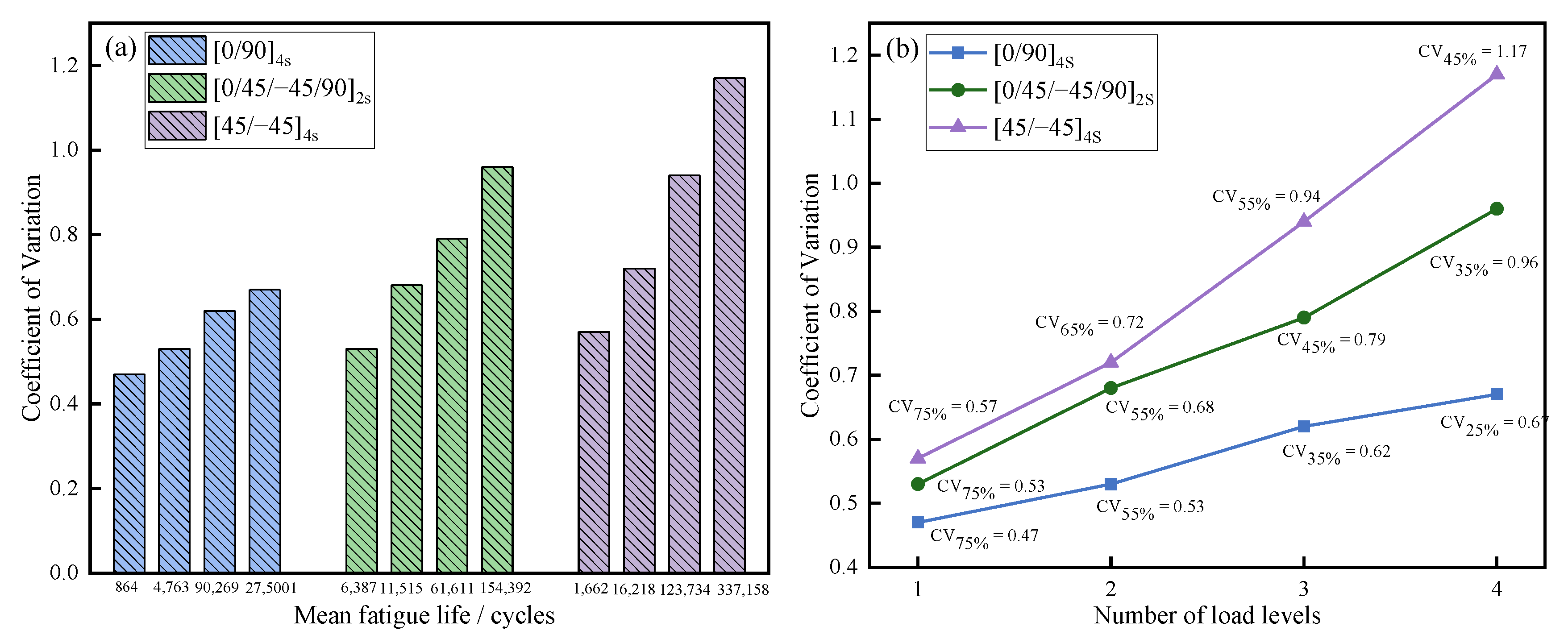
- The coefficient of variation of the joint varies depending on the stacking sequence. The stacking sequence can change the stability of the joint; the fatigue life of J-[45/−45]4s fluctuated more than that of J-[0/45/−45/90]2s and J-[0/90]4s.
- (3)
- The initial failure location of the joint changes under influence of the stacking sequence. When the proportion of 45° plies in CFRP increased, the initial failure location gradually moved from the adhesive layer towards the CFRP due to the decline of fatigue performance of CFRP.
- (4)
- Under high load levels, damage extends mainly from the initial failure location along the lap length direction and to a lesser extent in the lap thickness direction. Eventually, large-area cohesive failure occurred in joints in which the CFRP stacking sequences were [0/90]4s and [0/45/−45/90]2s, and delamination failure occurred when the CFRP stacking sequence was [45/−45]4s.
- (5)
- Under low load levels, all joints eventually suffered interfacial failure due to weakening of mechanical interlocking between the adhesive–Al interface, caused by the higher number of cycles; at this point, there was less effect of the stacking sequence on failure mode of the joint.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Luan, J.Z.; Na, J.X.; Tan, W.; Mu, W.L.; Wang, G.B.; Gao, Y. Comparative study on mechanical properties of aluminum alloy and BFRP single lap joints with hygrothermal aging. J. Adhes. 2020, 10, 918–935. [Google Scholar]
- Alam, P.; Robert, C.; Bradaigh, C.M.O. Tidal turbine blade composites-A review on the effects of hygrothermal aging on the properties of CFRP. Compos. Part B Eng. 2018, 149, 248–259. [Google Scholar] [CrossRef]
- Morgado, M.A.; Carbas, R.J.C.; Santos, D.G.d.; da Silva, L.F.M. Strength of CFRP joints reinforced with adhesive layers. Int. J. Adhes. Adhes. 2019, 97, 102475. [Google Scholar] [CrossRef]
- Alireza, D.; Saeed, R. Experimental and Numerical Study of Optimum Functionally Graded Aluminum/GFRP adhesive lap shear joints using Epoxy/CTBN. Int. J. Adhes. Adhes. 2021, 107, 102854. [Google Scholar]
- Lokman, G.; Mohammed, A.; Ceyhun, A.; Sakir, Y.; Onuralp, O.Y.; Arslan; Hakan, A.M. Optimum amount of CFRP for strengthening shear deficient reinforced concrete beams. Steel Compos. Struct. 2022, 43, 735–757. [Google Scholar]
- Aksoylu, C.; Yazman, Ş.; Özkılıç, Y.O.; Gemi, L.; Arslan, M.H. Experimental analysis of reinforced concrete shear deficient beams with circular web openings strengthened by CFRP composite. Compos. Struct. 2020, 249, 112561. [Google Scholar] [CrossRef]
- Aksoylu, C. Experimental analysis of shear deficient reinforced concrete beams strengthened by glass fiber strip composites and mechanical stitches. Steel Compos. Struct. 2021, 40, 267–285. [Google Scholar]
- Onuralp, Ö.Y.; Ceyhun, A.; Şakir, Y.; Lokman, G.; Hakan, A.M. Behavior of CFRP-strengthened RC beams with circular web openings in shear zones: Numerical study. Structures 2022, 41, 1369–1389. [Google Scholar]
- Hakan, A.M.; Şakir, Y.; Abed, H.A.; Ceyhun, A.; Onuralp, Ö.Y.; Lokman, G. Shear strengthening of reinforced concrete T-beams with anchored and non-anchored CFRP fabrics. Structures 2022, 39, 527–542. [Google Scholar]
- Gemi, L.; Aksoylu, C.; Yazman, Ş.; Özkılıç, Y.O.; Arslan, M.H. Experimental investigation of shear capacity and damage analysis of thinned end prefabricated concrete purlins strengthened by CFRP composite. Compos. Struct. 2019, 229, 111399. [Google Scholar] [CrossRef]
- Ceyhun, A. Shear strengthening of reinforced concrete beams with minimum CFRP and GFRP strips using different wrapping technics without anchoring application. Steel Compos. Struct. 2022, 44, 831–851. [Google Scholar]
- Sadeq, A.H.A.; Arife, A.; Ceyhun, A.; Musa, H.A. Strengthening of shear-critical reinforced concrete T-beams with anchored and non-anchored GFRP fabrics applications. Structures 2022, 44, 809–827. [Google Scholar]
- Onuralp, Y.A.; Sakir, Y.; Ceyhun, A.; Hakan, A.M.; Lokman, G. Numerical investigation of the parameters influencing the behavior of dapped end prefabricated concrete purlins with and without CFRP strengthening. Constr. Build. Mater. 2021, 275, 122173. [Google Scholar]
- Kgoete, F.M.; Popoola, A.P.I.; Fayomi, O.S.I. Advancement in the application of alloys and composites in the manufacture of aircraft component: A review. J. Phys. Conf. Ser. 2019, 1378, 032049. [Google Scholar] [CrossRef]
- Idris, B.; Yasir, A.; Jafar, A.; Gilles, L.; Nesar, M. Fatigue crack growth in laser-treated adhesively bonded composite joints: An experimental examination. Int. J. Adhes. Adhes. 2021, 105, 102784. [Google Scholar]
- Marques, A.C.; Mocanu, A.; Tomić, N.Z.; Balos, S.; Stammen, E.; Lundevall, A.; Abrahami, S.T.; Günther, R.; de Kok, J.M.M.; Teixeira de Freitas, S. Review on adhesives and surface treatments for structural applications: Recent developments on sustainability and implementation for metal and composite substrates. Materials 2020, 24, 5590. [Google Scholar] [CrossRef]
- Pramanik, A.; Basak, A.K.; Dong, Y.; Sarker, P.K.; Uddin, M.S.; Littlefair, G.; Dixit, A.R.; Chattopadhyaya, S. Joining of carbon fibre reinforced polymer (CFRP) composites and aluminium alloys—A review. Compos. Part A Appl. Sci. Manuf. 2017, 101, 1–29. [Google Scholar] [CrossRef]
- Banea, M.D.; Rosioara, M.; Carbas, R.J.C.; da Silva, L.F.M. Multi-material adhesive joints for automotive industry. Compos. Part B Eng. 2018, 151, 71–77. [Google Scholar] [CrossRef]
- Karataş, M.A.; Motorcu, A.R.; Gökkaya, H. Optimization of machining parameters for kerf angle and roundness error in abrasive water jet drilling of CFRP composites with different fiber orientation angles. J. Braz. Soc. Mech. Sci. Eng. 2020, 4, 173. [Google Scholar] [CrossRef]
- Jiang, L.X.; Xiao, S.N.; Dong, D.W.; Yang, B.; Chen, D.D.; Yang, G.W.; Zhu, T.; Wang, M.M. Experimental study of bonded, bolted, and hybrid braided CFRP joints with different stacking sequences and lapping patterns. Thin-Walled Struct. 2022, 177, 109408. [Google Scholar] [CrossRef]
- Ozel, A.; Yazici, B.; Akpinar, S.; Aydin, M.D.; Temiz, Ş. A study on the strength of adhesively bonded joints with different adherends. Compos. Part B Eng. 2014, 62, 167–174. [Google Scholar] [CrossRef]
- Zhang, Q.; Cheng, X.Q.; Cheng, Y.J.; Li, W.D.; Hu, R.W. Investigation of tensile behavior and influence factors of composite-to-metal 2D-scarf bonded joint. Eng. Struct. 2019, 180, 284–294. [Google Scholar] [CrossRef]
- Machado, J.J.M.; Marques, E.A.S.; Barbosa, A.Q.; da Silva, L.F.M. Effect of hygrothermal aging on the quasi-static behaviour of CFRP joints varying the overlap length. Compost. Struct. 2019, 214, 451–462. [Google Scholar] [CrossRef]
- Fernández-Cañadas, L.M.; Ivañez, I.; Saez-Sonia, S.E.J.B. Effect of adhesive thickness and overlap on the behavior of composite single-lap joints. Mech. Adv. Mater. Struct. 2019, 28, 1111–1120. [Google Scholar] [CrossRef]
- Cai, Y.; An, X.Z.; Zou, Q.C.; Fu, H.T.; Yang, X.H.; Zhang, H. Mechanical properties and failure mechanisms of composite laminates with classical fabric stacking patterns. J. Mater. Sci. 2021, 56, 11814–11827. [Google Scholar] [CrossRef]
- He, Z.K.; Luo, Q.T.; Li, Q.; Zheng, G.; Sun, G.Y. Fatigue behavior of CFRP/Al adhesive joints-Failure mechanisms study using digital image correlation (DIC) technique. Thin-Walled Struct. 2022, 174, 109075. [Google Scholar] [CrossRef]
- Federal Aviation Administration. Federal Aviation Regulations FAR 25 Airworthiness Standards: Transport Category Airplanes; U.S. Department of Transportation: Washington, DC, USA, 2017. [Google Scholar]
- Federal Aviation Administration. Advisory Circular AC 25.571-1D: Damage Tolerance and Fatigue Evaluation of Structures; U.S. Department of Transportation: Washington, DC, USA, 2010. [Google Scholar]
- Budhe, S.; Banea, M.D.; de Barros, S.; da Silva, L.F.M. An updated review of adhesively bonded joints in composite materials. Int. J. Adhes. Adhes. 2017, 72, 30–42. [Google Scholar] [CrossRef]
- Wahab, M.M.A.; Bartolomé, J.F.; Chicot, D.; Lowther, J.E. Fatigue in Adhesively Bonded Joints: A Review. ISRN Mater. Sci. 2012, 2012, 746308. [Google Scholar]
- Mariam, M.; Afendi, M.; Majid, M.S.A.; Ridzuan, M.J.M.; Gibson, A.G. Tensile and fatigue properties of single lap joints of aluminium alloy/glass fibre reinforced composites fabricated with different joining methods. Compost. Struct. 2018, 200, 647–658. [Google Scholar] [CrossRef]
- Azari, S.; Ameli, A.; Papini, M.; Spelt, J.K. Adherend thickness influence on fatigue behavior and fatigue failure prediction of adhesively bonded joints. Compos. Part A Appl. Sci. Manuf. 2013, 48, 181–191. [Google Scholar] [CrossRef]
- Kara, E.; Kurşun, A.; Haboğlu, M.R.; Enginsoy, H.M.; Aykul, H. Fatigue behavior of adhesively bonded glass fiber reinforced plastic composites with different overlap lengths. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2015, 229, 1292–1299. [Google Scholar] [CrossRef]
- Shin, K.C.; Lee, J.J. Effects of bond parameters on fatigue characteristics of a cocured double lap joint subjected to cyclic tensile loads. J. Adhes. 2010, 79, 581–596. [Google Scholar] [CrossRef]
- Hedayati, R.; Khouzani, S.G.; Jahanbakhshi, M. Investigation of debonding propagation in aluminum/composite joints under fatigue loading. J. Adhes. Sci. Technol. 2015, 29, 59–73. [Google Scholar] [CrossRef]
- Meneghetti, G.; Quaresimin, M.; Ricotta, M. Damage mechanisms in composite bonded joints under fatigue loading. Compos. Part B Eng. 2012, 43, 210–220. [Google Scholar] [CrossRef]
- Tian, T.C.; Fu, J.; Ju, Y.Z. Experimental study on failure mechanism of CFRP-to-aluminium single-lap adhesive joints under tension after out-of-plane pre-impact. J. Adhes. 2022. [Google Scholar]
- Zhao, T.; Jiang, Y. Fatigue of 7075-T651 aluminum alloy. Int. J. Fatigue 2007, 30, 834–849. [Google Scholar] [CrossRef]
- ASTM D3166; Standard Test Method for Fatigue Properties of Adhesives in Shear by Tension Loading (Metal/Metal). ASTM International: West Conshohocken, PA, USA, 2020.
- ASTM D5868-01; Standard Test Method for Lap Shear Adhesion for Fiber Reinforced Plastic (FRP) Bonding. ASTM International: West Conshohocken, PA, USA, 2014.
- Çavdar, S.; Teutenberg, D.; Meschut, G.; Wulf, A.; Hesebeck, O.; Brede, M.; Mayer, B. Stress-based fatigue life prediction of adhesively bonded hybrid hyperelastic joints under multiaxial stress conditions. Int. J. Adhes. Adhes. 2019, 97, 102483. [Google Scholar] [CrossRef]
- Xu, X.X.; Crocombe, A.D.; Smith, P.A. Fatigue Crack Growth Rates in Adhesive Joints Tested at Different Frequencies. J. Adhes. 2006, 58, 191–204. [Google Scholar] [CrossRef]
- Liu, X.L.; Zheng, G.; Luo, Q.T.; Qing, L.; Sun, G.Y. Fatigue behavior of carbon fibre reinforced plastic and aluminum single lap adhesive joints after the transverse pre-impact. Int. J. Fatigue 2021, 144, 105973. [Google Scholar] [CrossRef]
- Datla, N.V.; Ameli, A.; Azari, S.; Papini, M.; Spelt, J.K. Effects of hygrothermal aging on the fatigue behavior of two toughened epoxy adhesives. Eng. Fract. Mech. 2012, 79, 61–77. [Google Scholar] [CrossRef]
- Haidyrah, A.S.; Newkirk, J.W.; Castaño, C.H. Weibull statistical analysis of Krouse type bending fatigue of nuclear materials. J. Nucl. Mater. 2016, 470, 244–250. [Google Scholar] [CrossRef]
- Naresh, K.; Shankar, K.; Velmurugan, R. Reliability analysis of tensile strengths using Weibull distribution in glass/epoxy and carbon/epoxy composites. Compos. Part B Eng. 2018, 133, 129–144. [Google Scholar] [CrossRef]
- Hao, J.; Tong, L.; Li, G.Y.; Zhang, X.; Cui, J.J. Fatigue life assessment of electromagnetic riveted carbon fiber reinforce plastic/aluminum alloy lap joints using Weibull distribution. Int. J. Fatigue 2017, 105, 180–189. [Google Scholar]
- Mollón, V.; Bonhomme, J.; Viña, J.; Argüelles, A.; Fernández-Canteli, A. Influence of the principal tensile stresses on delamination fracture mechanisms and their associated morphology for different loading modes in carbon/epoxy composites. Compos. Part B Eng. 2012, 43, 1676–1680. [Google Scholar] [CrossRef]
- Davidson, P.; Waas, A.M. The effects of defects on the compressive response of thick carbon composites: An experimental and computational study. Compos. Struct. 2017, 176, 582–596. [Google Scholar] [CrossRef]
- Modi, V.; Singh, K.K.; Shrivastava, R. Effect of Stacking Sequence on Interlaminar Shear Strength of Multidirectional GFRP Laminates. Mater. Today 2020, 22, 2207–2214. [Google Scholar] [CrossRef]
- Gudladt, H.J.; Frömmel, S.T. Fatigue and fracture behavior of adhesive-bonded structures in the light of the surface morphology. Int. J. Adhes. Adhes. 2019, 88, 74–80. [Google Scholar] [CrossRef]
- Hull, D. Fractography, Observing, Measuring and Interpreting Fracture Surface Topography; Cambridge University Press: Cambridge, UK, 1999. [Google Scholar]
- Wei, T.; Na, J.X.; Wang, G.B.; Chen, H.L.; Meng, H. Effect of temperature on the fatigue performance and failure mechanism of a flexible adhesive butt joint. J. Adhes. 2021, 365, 1998–2028. [Google Scholar]
- Yoon, P.S.; Jong, C.W.; Hoon, C.C.; Soap, C.H. The effect of curing temperature on thermal, physical and mechanical characteristics of two types of adhesives for aerospace structures. J. Adhes. Sci. Technol. 2018, 32, 1200–1223. [Google Scholar]
- Reis, J.M.L.; Amorim, F.C.; da Silva, A.H.M.F.T.; da Costa, H.S.M. Influence of temperature on the behavior of DGEBA (bisphenol A diglycidyl ether) epoxy adhesive. Int. J. Adhes. Adhes. 2015, 58, 88–92. [Google Scholar] [CrossRef]
- Flinn, R.A.; Trojan, P.K. Engineering Materials and Their Applications; Houghton Mifflin Harcourt: Boston, MA, USA, 1995. [Google Scholar]
- Demiral, M.; Kadioglu, F. Failure behaviour of the adhesive layer and angle ply composite adherends in single lap joints: A numerical study. Int. J. Adhes. Adhes. 2018, 87, 181–190. [Google Scholar] [CrossRef]
- Fan, X.M.; Yin, X.W. Progress in research and development on matrix modification of continuous fiber-reinforced silicon carbide matrix composites. Adv. Compos. Hybrid Mater. 2018, 1, 685–695. [Google Scholar] [CrossRef]



| Parameter | Symbol | Value |
|---|---|---|
| Longitudinal tensile modulus | E11/MPa | 121,000 |
| Transverse tensile modulus | E22/MPa | 8600 |
| Shear modulus | G12/MPa | 3450 |
| Poisson’s ratio | μ12 | 0.301 |
| Density | ρ/kg·m−3 | 1570 |
| Parameter | Symbol | Value |
|---|---|---|
| Young’s modulus | E/MPa | 71,700 |
| Poisson’s ratio | μ | 0.32 |
| Density | ρ/kg·m−3 | 3000 |
| Specimen Full Name | Specimen ID |
|---|---|
| The joint with CFRP adherend stacking sequences of [0/90]4s | J-[0/90]4s |
| The joint with CFRP adherend stacking sequences of [0/45/−45/90]2s | J-[0/45/−45/90]2s |
| The joint with CFRP adherend stacking sequences of [45/−45]4s | J-[45/−45]4s |
| Specimen ID | Failure Load | Failure Displacement |
|---|---|---|
| J-[0/90]4s | 5.93 kN | 1.13 mm |
| J-[0/45/−45/90]2s | 4.40 kN | 1.47 mm |
| J-[45/−45]4s | 3.52 kN | 2.19 mm |
| Stacking Sequence | Load Level | Fatigue Life | lnln [1/R(t)] | ln(t) |
|---|---|---|---|---|
| [0/90]4s | 100% (5.93 kN) | 1 | - | - |
| 75% (4.45 kN) | 474 | −1.753 | 6.161 | |
| 638 | −0.717 | 6.458 | ||
| 1026 | −0.050 | 6.933 | ||
| 1248 | 0.609 | 7.129 | ||
| 55% (3.26 kN) | 2442 | −1.753 | 7.801 | |
| 3283 | −0.717 | 8.097 | ||
| 5235 | −0.050 | 8.563 | ||
| 7562 | 0.609 | 8.931 | ||
| 35% (2.08 kN) | 33,364 | −1.753 | 10.415 | |
| 68,903 | −0.717 | 11.140 | ||
| 103,672 | −0.050 | 11.549 | ||
| 135,573 | 0.609 | 11.817 | ||
| 25% (1.48 kN) | 99,998 | −1.753 | 11.513 | |
| 179,375 | −0.717 | 12.097 | ||
| 252,329 | −0.050 | 12.620 | ||
| 457,897 | 0.609 | 13.034 | ||
| 15% (0.89 kN) | >1,000,000 | - | - | |
| [0/45/−45/90]2s | 100% (4.40 kN) | 1 | ||
| 75% (3.30 kN) | 3706 | −1.753 | 8.218 | |
| 4184 | −0.717 | 8.339 | ||
| 6464 | −0.050 | 8.774 | ||
| 10,801 | 0.609 | 9.287 | ||
| 55% (2.42 kN) | 4072 | −1.753 | 8.312 | |
| 9015 | −0.717 | 9.107 | ||
| 10,837 | −0.050 | 9.291 | ||
| 20,441 | 0.609 | 9.925 | ||
| 45% (1.98 kN) | 15,661 | −1.753 | 9.659 | |
| 37,542 | −0.717 | 10.533 | ||
| 52,921 | -0.050 | 10.877 | ||
| 103,162 | 0.609 | 11.544 | ||
| 35% (1.54 kN) | 30,780 | −1.753 | 10.335 | |
| 81,592 | −0.717 | 11.309 | ||
| 215,581 | −0.050 | 12.281 | ||
| 258,906 | 0.609 | 12.574 | ||
| 25% (1.10 kN) | >1,000,000 | |||
| 100% (3.52 kN) | 1 | |||
| [45/−45]4s | 75% (2.64 kN) | 824 | −1.753 | 6.714 |
| 1015 | −0.717 | 6.923 | ||
| 1711 | −0.050 | 7.445 | ||
| 2716 | 0.609 | 7.907 | ||
| 65% (2.29 kN) | 4928 | −1.753 | 8.503 | |
| 10,119 | −0.717 | 9.222 | ||
| 16,734 | −0.050 | 9.725 | ||
| 25,827 | 0.609 | 10.159 | ||
| 55% (1.94 kN) | 27,448 | −1.753 | 10.220 | |
| 55,589 | −0.717 | 10.926 | ||
| 150,917 | −0.050 | 11.924 | ||
| 215,542 | 0.609 | 12.281 | ||
| 45% (1.58 kN) | 51,592 | −1.753 | 10.851 | |
| 120,667 | −0.717 | 11.701 | ||
| 264,656 | −0.050 | 12.486 | ||
| 789,365 | 0.609 | 13.579 | ||
| 35% (1.23 kN) | >1,000,000 |
| Stacking Sequence | Load Level | α | β | MTTF | CV |
|---|---|---|---|---|---|
| [0/90]4s | 75% | 2.25 | 975.56 | 864 | 0.47 |
| 55% | 1.98 | 5372.48 | 4763 | 0.53 | |
| 35% | 1.64 | 100,859.46 | 90,269 | 0.62 | |
| 25% | 1.53 | 305,218.04 | 275,001 | 0.67 | |
| [0/45/−45/90]2s | 75% | 1.95 | 7201.55 | 6387 | 0.53 |
| 55% | 1.5 | 12,750.60 | 11,515 | 0.68 | |
| 45% | 1.27 | 66,327.65 | 61,611 | 0.79 | |
| 35% | 1.01 | 151,856.77 | 154,392 | 0.96 | |
| [45/−45]4s | 75% | 1.8 | 1869.33 | 1662 | 0.57 |
| 65% | 1.41 | 17,804.99 | 16,218 | 0.72 | |
| 55% | 1.06 | 126,157.07 | 123,734 | 0.94 | |
| 45% | 0.86 | 312,126.17 | 337,158 | 1.17 |
| Specimen ID | Load Level | Failure Mode |
|---|---|---|
| J-[0/90]4s | 25% | cohesive failure (8%) + interfacial failure (92%) |
| 35% | cohesive failure (6%) + interfacial failure (94%) | |
| 55% | cohesive failure (46%) + interfacial failure (54%) | |
| 75% | cohesive failure (97%) + interfacial failure (3%) | |
| 100% | cohesive failure (100%) | |
| J-[0/45/−45/90]2s | 35% | cohesive failure (20%) + interfacial failure (80%) |
| 45% | cohesive failure (34%) + interfacial failure (66%) | |
| 55% | cohesive failure (62%) + interfacial failure (38%) | |
| 75% | cohesive failure (85%) + interfacial failure (15%) | |
| 100% | cohesive failure (98%) + slight fibre failure (2%) | |
| J-[45/−45]4s | 45% | cohesive failure (23%) + interfacial failure (77%) |
| 55% | cohesive failure (26%) + interfacial failure (74%) | |
| 65% | cohesive failure (49%) + interfacial failure (51%) | |
| 75% | delamination failure (100%) | |
| 100% | delamination failure (100%) |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zou, T.; Ju, Y.; Guan, Y.; Fu, J. Effect of Stacking Sequence on Fatigue Performance of CFRP–Al Single-Lap Adhesive Joints: Experimental Study. Polymers 2022, 14, 5088. https://doi.org/10.3390/polym14235088
Zou T, Ju Y, Guan Y, Fu J. Effect of Stacking Sequence on Fatigue Performance of CFRP–Al Single-Lap Adhesive Joints: Experimental Study. Polymers. 2022; 14(23):5088. https://doi.org/10.3390/polym14235088
Chicago/Turabian StyleZou, Tianchun, Yuezhang Ju, Yuxi Guan, and Ji Fu. 2022. "Effect of Stacking Sequence on Fatigue Performance of CFRP–Al Single-Lap Adhesive Joints: Experimental Study" Polymers 14, no. 23: 5088. https://doi.org/10.3390/polym14235088
APA StyleZou, T., Ju, Y., Guan, Y., & Fu, J. (2022). Effect of Stacking Sequence on Fatigue Performance of CFRP–Al Single-Lap Adhesive Joints: Experimental Study. Polymers, 14(23), 5088. https://doi.org/10.3390/polym14235088





