Fire Resistance Performance of Steel–Polymer Prefabricated Composite Floors Using Standard Fire Tests
Abstract
:1. Introduction
2. Modeling for Standard Fire Tests
2.1. Test Setup
2.2. Test Specimens
2.3. Failure Criteria
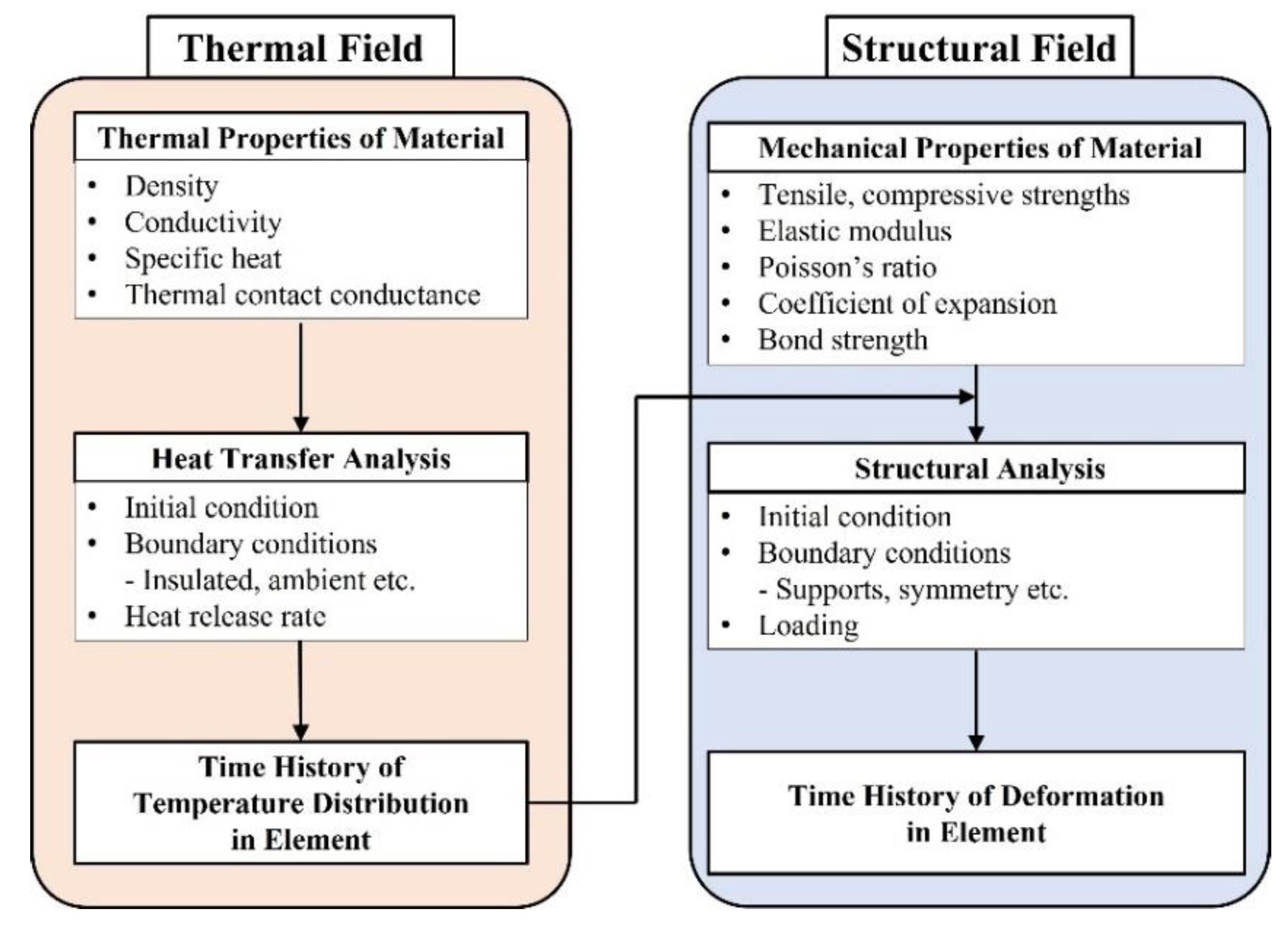
3. Finite Element Analysis
3.1. Analysis Plan
3.2. Thermal Properties
3.3. Mechanical Properties
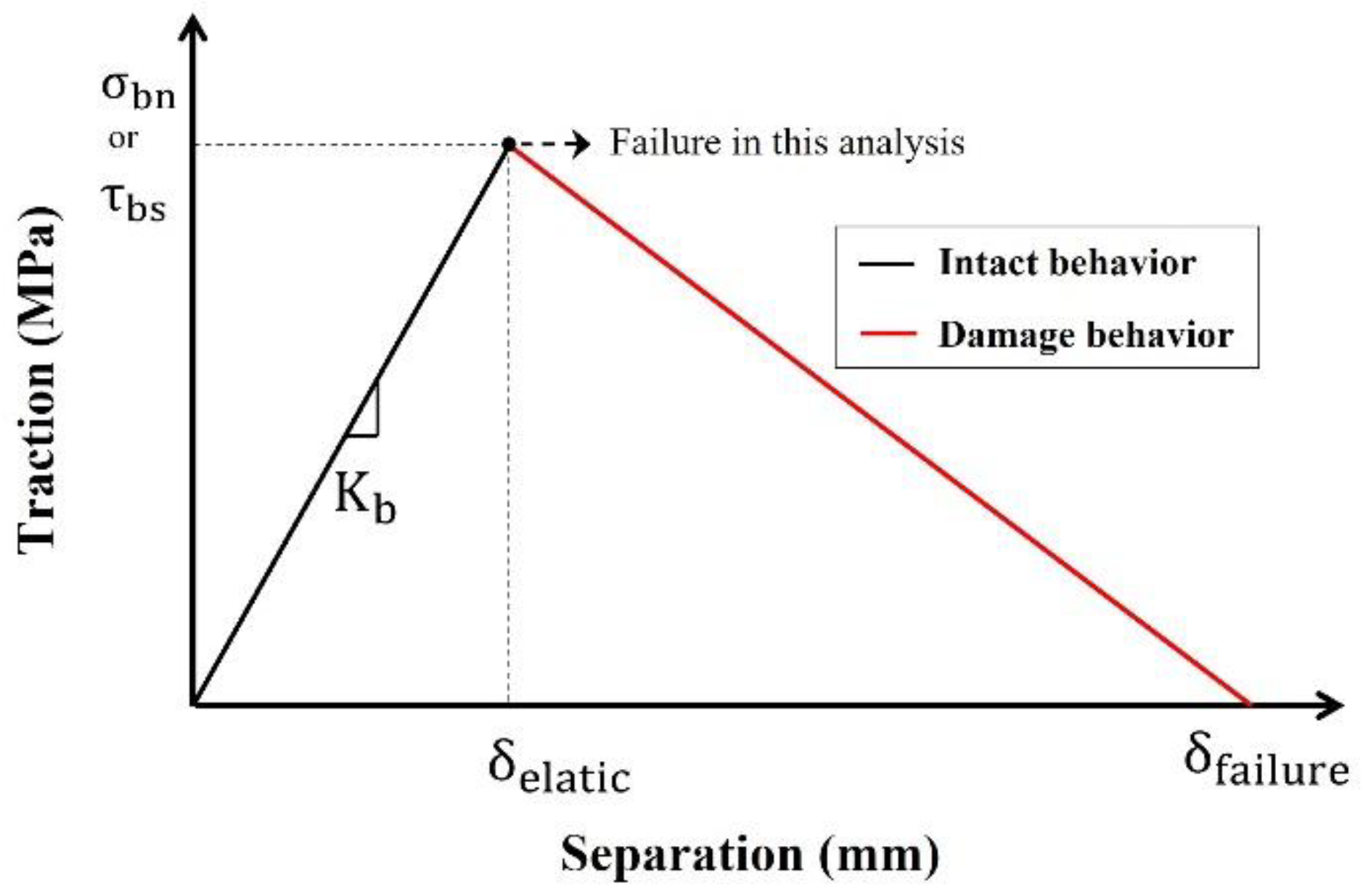
3.4. Interfacial Properties
3.5. Validation
4. Fire Resistance Performance
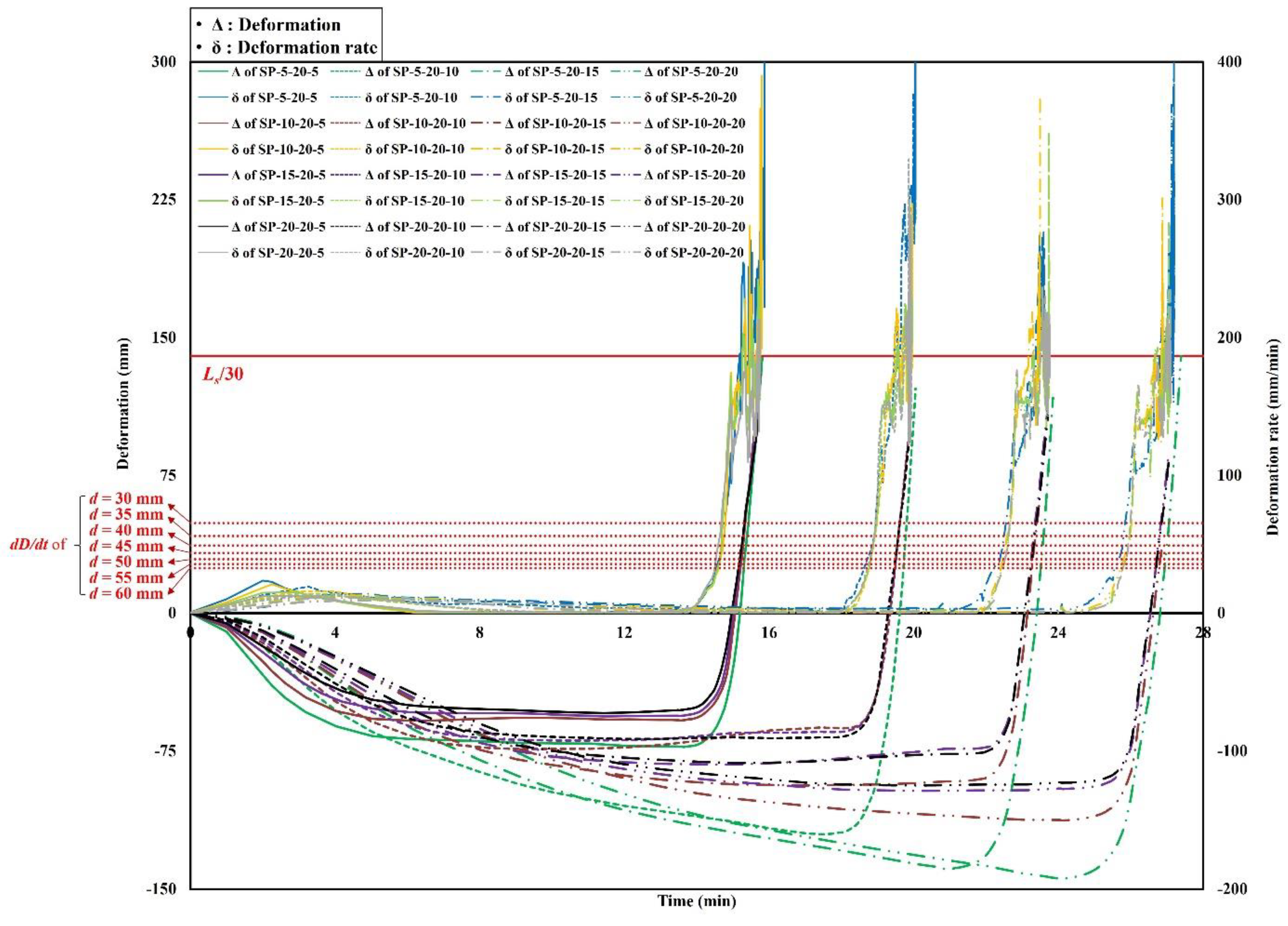
4.1. Analysis Results
4.2. Discussions
5. Conclusions
- (1)
- The top steel plate thickness showed negligible effects on the stability ratings of steel–polymer prefabricated composite floors under standard fire tests, whereas the bottom steel plate thickness exhibited a linear relationship with the stability ratings. In addition, thicker polymers resulted in lower stability ratings due to polymer expansion at high temperatures;
- (2)
- An estimated stability rating was proposed for steel–polymer prefabricated composite floors under standard fire tests. It is expressed in terms of the thickness of the bottom steel plates (dbs) and polymers (dp) with specific ranges of 5 mm ≤ dts, dbs ≤ 20 mm, and 20 mm ≤ dp ≤ 60 mm, as follows:
- (3)
- On the basis of previous studies, the fire resistance performance of steel–polymer prefabricated composite floors under standard fire tests was proposed using Equation (8), which is written in terms of the thicknesses of the top and bottom steel plates and polymers. The fire resistance performance was determined as the minimum value between the insulation and stability ratings.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Buchanan, A.H.; Abu, A.K. Structural Design for Fire Safety, 2nd ed.; WILEY: Hoboken, NJ, USA, 2017. [Google Scholar]
- ISO 834-1; Fire-Resistance Tests–Elements of Building Construction–Part 1: General Requirements. International Organization for Standardizations: Geneva, Switzerland, 1999.
- ISO 834-5; Fire-Resistance Tests–Elements of Building Construction–Part 5: Specific Requirements for Loadbearing Horizontal Separating Elements. International Organization for Standardizations: Geneva, Switzerland, 2000.
- KS F 2257-1; Methods of Fire Resistance Test for Elements of Building Construction-General Requirements. Korean Agency for Technology and Standards: Seoul, Korea, 2019.
- KS F 2257-5; Methods of Fire Resistance Test for Elements of Building Construction-Specific Requirements for Loadbearing Horizontal Separating Elements. Korean Agency for Technology and Standards: Seoul, Korea, 2019.
- Takagi, J.; Deierlein, G.G. Strength design criteria for steel members at elevated temperatures. J. Constr. Stee. Res. 2007, 63, 1036–1050. [Google Scholar] [CrossRef]
- Rackauskaite, E.; Kotsovinos, P.; Jeffers, A.; Rein, G. Computational analysis of thermal and structural failure criteria of a multi-storey steel frame exposed to fire. Eng. Struct. 2019, 180, 524–543. [Google Scholar] [CrossRef]
- Cajka, R.; Mateckova, P. Fire resistance of ceiling slab concreted in Trapezoidal sheet. Procedia. Eng. 2013, 65, 393–396. [Google Scholar] [CrossRef] [Green Version]
- Jiang, J.; Pintar, A.; Weigand, J.M.; Main, J.A.; Sadek, F. Improved calculation method for insulation-based fire resistance of composite slabs. Fire. Safety J. 2019, 105, 144–153. [Google Scholar] [CrossRef]
- Khetata, S.M.; Piloto, P.A.; Gavilán, A.B. Fire resistance of composite non-load bearing light steel framing walls. J. Fire. Sci. 2020, 38, 136–155. [Google Scholar] [CrossRef]
- Frangi, A.; Knobloch, M.; Fontana, M. Fire design of timber slabs made of hollow core elements. Eng. Struct. 2009, 31, 150–157. [Google Scholar] [CrossRef]
- Frangi, A.; Knobloch, M.; Fontana, M. Fire design of timber-concrete composite slabs with screwed connections. J. Struct. Eng. 2010, 136, 219–228. [Google Scholar] [CrossRef]
- Romero, M.L.; Cajot, L.G.; Conan, Y.; Braun, M. Fire design methods for slim-floor structures. Steel. Constr. 2015, 8, 102–109. [Google Scholar] [CrossRef]
- Frangi, A.; Fontana, M.; Hugi, E.; Jübstl, R. Experimental analysis of cross-laminated timber panels in fire. Fire Safety J. 2009, 44, 1078–1087. [Google Scholar] [CrossRef]
- Menis, A.; Fragiacomo, M.; Clemente, I. Fire resistance of unprotected cross-laminated timber floor panels: Parametric study and simplified design. Fire Safety J. 2019, 107, 104–113. [Google Scholar] [CrossRef]
- Ryu, J.; Kim, Y.Y.; Park, M.W.; Yoon, S.W.; Lee, C.H.; Ju, Y.K. Experimental and numerical investigations of steel-polymer hybrid floor panels subjected to three-point bending. Eng. Struct. 2018, 175, 467–482. [Google Scholar] [CrossRef]
- Lee, J.H.; Park, M.J.; Yoon, S.W. Floor vibration experiment and serviceability test of iFLASH system. Materials 2020, 13, 5670. [Google Scholar] [CrossRef] [PubMed]
- Park, M.J.; Yoon, S.W.; Ju, Y.K. New approaches for floor vibrations of steel-polymer sandwich floor systems. Eng. Struct. 2022, 258, 114141. [Google Scholar] [CrossRef]
- Park, M.J.; Bae, J.; Ryu, J.; Ju, Y.K. Fire resistance of hybrid floor based on small-scale furnace tests and energy-based time equivalent approach. Int. J. Steel. Struct. 2020, 20, 1811–1821. [Google Scholar] [CrossRef]
- Park, M.J.; Min, J.K.; Bae, J.; Ju, Y.K. Thermal contact conductance-based thermal behavior analytical model for a hybrid floor at elevated temperatures. Materials 2020, 13, 4257. [Google Scholar] [CrossRef]
- Park, M.J.; Bae, J.; Ryu, J.; Ju, Y.K. Fire design equation for steel-polymer composite floors in thermal fields via finite element analysis. Materials 2020, 13, 5573. [Google Scholar] [CrossRef]
- Park, M.J.; Min, J.K. Insulation design for circular spacers of steel-polymer prefabricated floor under fire. J. Arch. Inst. Korea 2021, 37, 227–234. (In Korean) [Google Scholar]
- Park, M.J. Structural Fire Behavior of Steel-Polymer Hybrid Floor for Disaster Prevention. Ph.D. Thesis, Korea University, Seoul, Korea, 2020. [Google Scholar]
- Park, M.J.; Ju, Y.K. Finite element model for the steel-polymer composite floor filled with phase-change amorphous polymers at elevated temperatures. Constr. Build. Mater. 2022, 319, 126059. [Google Scholar] [CrossRef]
- Ryan, J.V.; Robertson, A.F. Proposed criteria for defining load failure of beams, floors, and roof constructions during fire tests. J. Res. Natl. Bur. Stand. Sect. C. Eng. Instrum. 1959, 63C, 121–124. [Google Scholar] [CrossRef]
- Franssen, J.M. SAFIR: A thermal/structural program for modeling structures under fire. Eng. J.-Am. Inst. Steel. Constr. 2005, 42, 143–158. [Google Scholar]
- Nigro, E.; Cefarelli, G.; Bilotta, A.; Manfredi, G.; Cosenza, E. Fire resistance of concrete slabs reinfored with FRP bars. Part II: Experimental results and numerical simulations on the thermal field. Compos. Pt. B-Eng. 2011, 42, 1751–1763. [Google Scholar] [CrossRef]
- BS EN 1993-1-2:2005; BS. Eurocode 3: Design of Steel Structures–Part 1-2: General Rules–Structural Fire Design. British Standard: London, UK, 2005.
- Papanastasiou, P.; Sarris, E. Cohesive zone models. In Porous Rock Fracture Mechanics: With Application to Hydraulic Fracturing, Drilling and Structural Engineering; Shojaei, A.K., Shao, J., Eds.; Woodhead Publishing: Sawston, UK, 2017; pp. 119–144. [Google Scholar]


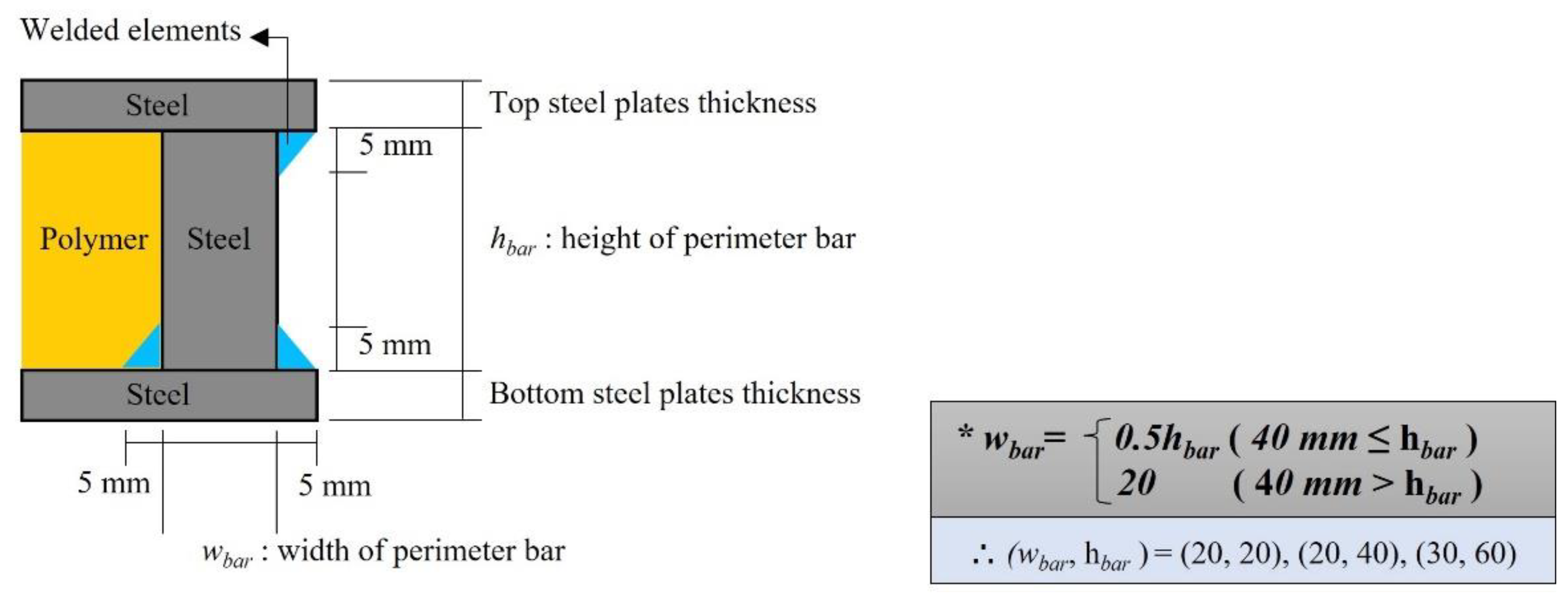



| Specimens | dts | dp | dbs | d | hbar | wbar |
|---|---|---|---|---|---|---|
| SP-5-20-5 | 5 | 20 | 5 | 30 | 20 | 20 |
| SP-10-20-5 | 10 | 35 | ||||
| SP-15-20-5 | 15 | 40 | ||||
| SP-20-20-5 | 20 | 45 | ||||
| SP-5-20-10 | 5 | 10 | 35 | |||
| SP-10-20-10 | 10 | 40 | ||||
| SP-15-20-10 | 15 | 45 | ||||
| SP-20-20-10 | 20 | 50 | ||||
| SP-5-20-15 | 5 | 15 | 40 | |||
| SP-10-20-15 | 10 | 45 | ||||
| SP-15-20-15 | 15 | 50 | ||||
| SP-20-20-15 | 20 | 55 | ||||
| SP-5-20-20 | 5 | 20 | 45 | |||
| SP-10-20-20 | 10 | 50 | ||||
| SP-15-20-20 | 15 | 55 | ||||
| SP-20-20-20 | 20 | 60 | ||||
| SP-5-40-5 | 5 | 40 | 5 | 50 | 40 | 20 |
| SP-10-40-5 | 10 | 55 | ||||
| SP-15-40-5 | 15 | 60 | ||||
| SP-20-40-5 | 20 | 65 | ||||
| SP-5-40-10 | 5 | 10 | 55 | |||
| SP-10-40-10 | 10 | 60 | ||||
| SP-15-40-10 | 15 | 65 | ||||
| SP-20-40-10 | 20 | 70 | ||||
| SP-5-40-15 | 5 | 15 | 60 | |||
| SP-10-40-15 | 10 | 65 | ||||
| SP-15-40-15 | 15 | 70 | ||||
| SP-20-40-15 | 20 | 75 | ||||
| SP-5-40-20 | 5 | 20 | 65 | |||
| SP-10-40-20 | 10 | 70 | ||||
| SP-15-40-20 | 15 | 75 | ||||
| SP-20-40-20 | 20 | 80 | ||||
| SP-5-60-5 | 5 | 60 | 5 | 70 | 60 | 30 |
| SP-10-60-5 | 10 | 75 | ||||
| SP-15-60-5 | 15 | 80 | ||||
| SP-20-60-5 | 20 | 85 | ||||
| SP-5-60-10 | 5 | 10 | 75 | |||
| SP-10-60-10 | 10 | 80 | ||||
| SP-15-60-10 | 15 | 85 | ||||
| SP-20-60-10 | 20 | 90 | ||||
| SP-5-60-15 | 5 | 15 | 80 | |||
| SP-10-60-15 | 10 | 85 | ||||
| SP-15-60-15 | 15 | 90 | ||||
| SP-20-60-15 | 20 | 95 | ||||
| SP-5-60-20 | 5 | 20 | 85 | |||
| SP-10-60-20 | 10 | 90 | ||||
| SP-15-60-20 | 15 | 95 | ||||
| SP-20-60-20 | 20 | 100 |
| Phase-Change Temperatures | Lower Bound (°C) | Upper Bound (°C) | Selected Temperatures (°C) |
|---|---|---|---|
| Glass transition (Tg) | 260 | 260 | 260 |
| Softening (Ts) | 280 | 310 | 280 |
| Melting (Tm) | 310 | 370 | 310 |
| Charring (Tc) | 370 | 420 | 370 |
| Gasification (Tgas) | 420 | 500 | 420 |
| Temperature (°C) | Strength (MPa) | Elastic Modulus (MPa) | Poisson’s Ratio |
|---|---|---|---|
| 20 | 25.9 | 1050 | 0.33 |
| 100 | 0.76 | 13.2 | 0.33 |
| 200 | 0.38 | 9.72 | 0.33 |
| 260 | 0.20 | 8.78 | 0.33 |
| 420 | 0.04 | 0.04 | 0.00 |
| 1200 | 0.00 | 0.00 | 0.00 |
| Steel and Steel | Steel and Polymers | |
|---|---|---|
| Friction Coefficient | Phase Changes of Polymers | Friction Coefficients |
| 0.3 | Solid state | 0.3 |
| Liquefied state | 0.1 | |
| Charred state | 0.1 | |
| Gasified state | 0.02 | |
| Normal Bond Strength (MPa) | Shear Bond Strength (MPa) | |
|---|---|---|
| Maximum | 8.94 | 3.9 |
| Average | 4.78 | 2.5 |
| Minimum (selected) | 1.08 | 0.8 |
| dp 20 mm | dts (mm) | 5 | 10 | 15 | 20 | ||||||||||||
| dbs (mm) | 5 | 10 | 15 | 20 | 5 | 10 | 15 | 20 | 5 | 10 | 15 | 20 | 5 | 10 | 15 | 20 | |
| Stability (min) | 15 | 20 | 23 | 27 | 15 | 20 | 23 | 27 | 15 | 20 | 23 | 27 | 15 | 20 | 23 | 27 | |
| dp 40 mm | dts (mm) | 5 | 10 | 15 | 20 | ||||||||||||
| dbs (mm) | 5 | 10 | 15 | 20 | 5 | 10 | 15 | 20 | 5 | 10 | 15 | 20 | 5 | 10 | 15 | 20 | |
| Stability (min) | 13 | 17 | 21 | 25 | 13 | 17 | 21 | 25 | 13 | 17 | 21 | 25 | 13 | 17 | 21 | 25 | |
| dp 60 mm | dts (mm) | 5 | 10 | 15 | 20 | ||||||||||||
| dbs (mm) | 5 | 10 | 15 | 20 | 5 | 10 | 15 | 20 | 5 | 10 | 15 | 20 | 5 | 10 | 15 | 20 | |
| Stability (min) | 13 | 17 | 21 | 25 | 13 | 17 | 21 | 25 | 13 | 17 | 21 | 25 | 13 | 17 | 21 | 25 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Park, M.J.; Alemayehu, R.W.; Ju, Y.K. Fire Resistance Performance of Steel–Polymer Prefabricated Composite Floors Using Standard Fire Tests. Polymers 2022, 14, 1488. https://doi.org/10.3390/polym14071488
Park MJ, Alemayehu RW, Ju YK. Fire Resistance Performance of Steel–Polymer Prefabricated Composite Floors Using Standard Fire Tests. Polymers. 2022; 14(7):1488. https://doi.org/10.3390/polym14071488
Chicago/Turabian StylePark, Min Jae, Robel Wondimu Alemayehu, and Young K. Ju. 2022. "Fire Resistance Performance of Steel–Polymer Prefabricated Composite Floors Using Standard Fire Tests" Polymers 14, no. 7: 1488. https://doi.org/10.3390/polym14071488
APA StylePark, M. J., Alemayehu, R. W., & Ju, Y. K. (2022). Fire Resistance Performance of Steel–Polymer Prefabricated Composite Floors Using Standard Fire Tests. Polymers, 14(7), 1488. https://doi.org/10.3390/polym14071488







