Free-Radical Propagation Rate Coefficients of Diethyl Itaconate and Di-n-Propyl Itaconate Obtained via PLP–SEC
Abstract
:1. Introduction
2. Materials and Methods
3. Results
3.1. Prediction of Arrhenius Parameters for DEI
3.2. PLP Experiments
3.2.1. Diethyl Itaconate
3.2.2. Di-n-Propyl Itaconate
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
Appendix A
Formulae for Calculating the Individual Entropy Components
Appendix B
Structures of the Reactants, Transition State and Product on UHF/6-31g(d) Level of Theory
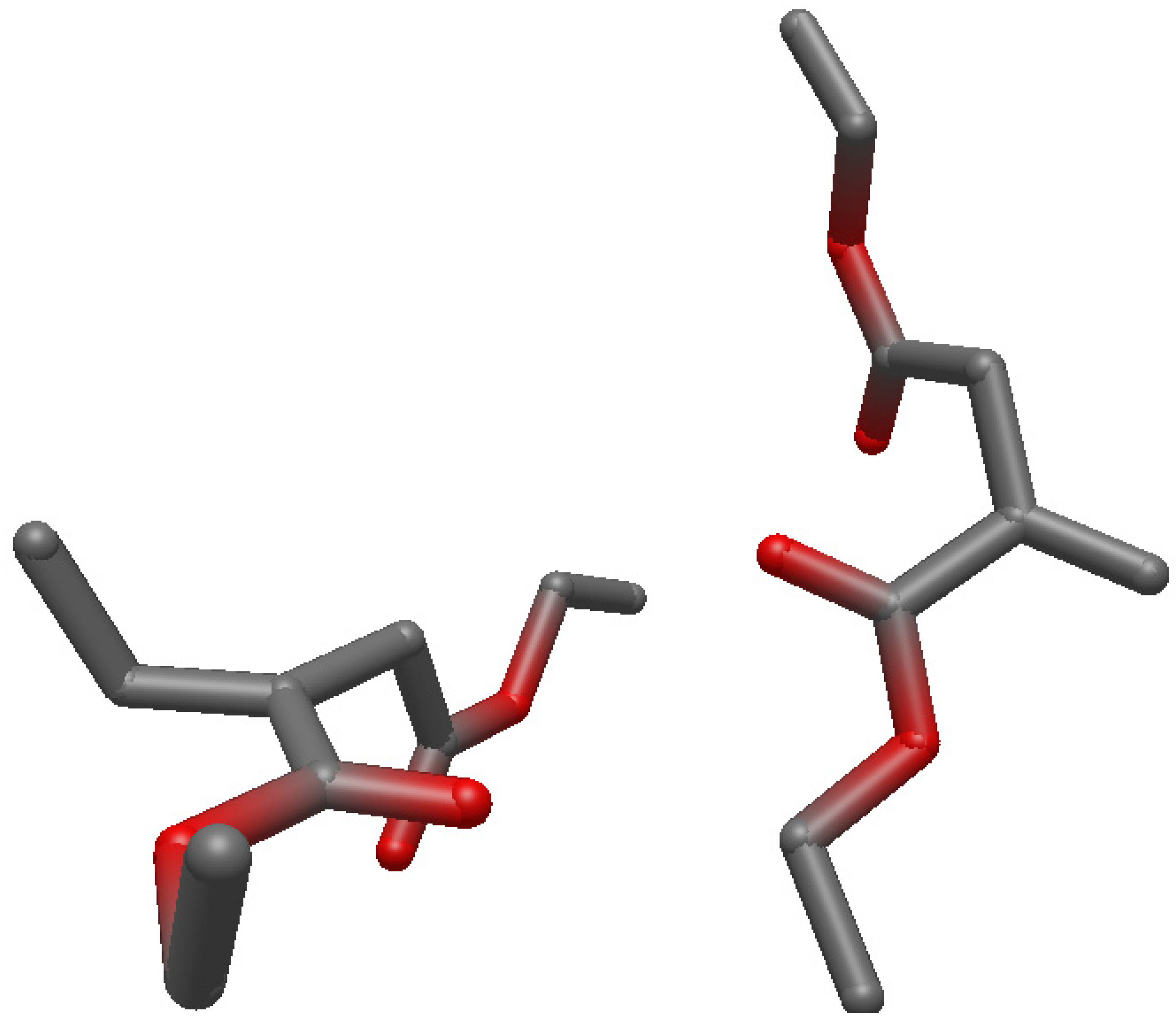

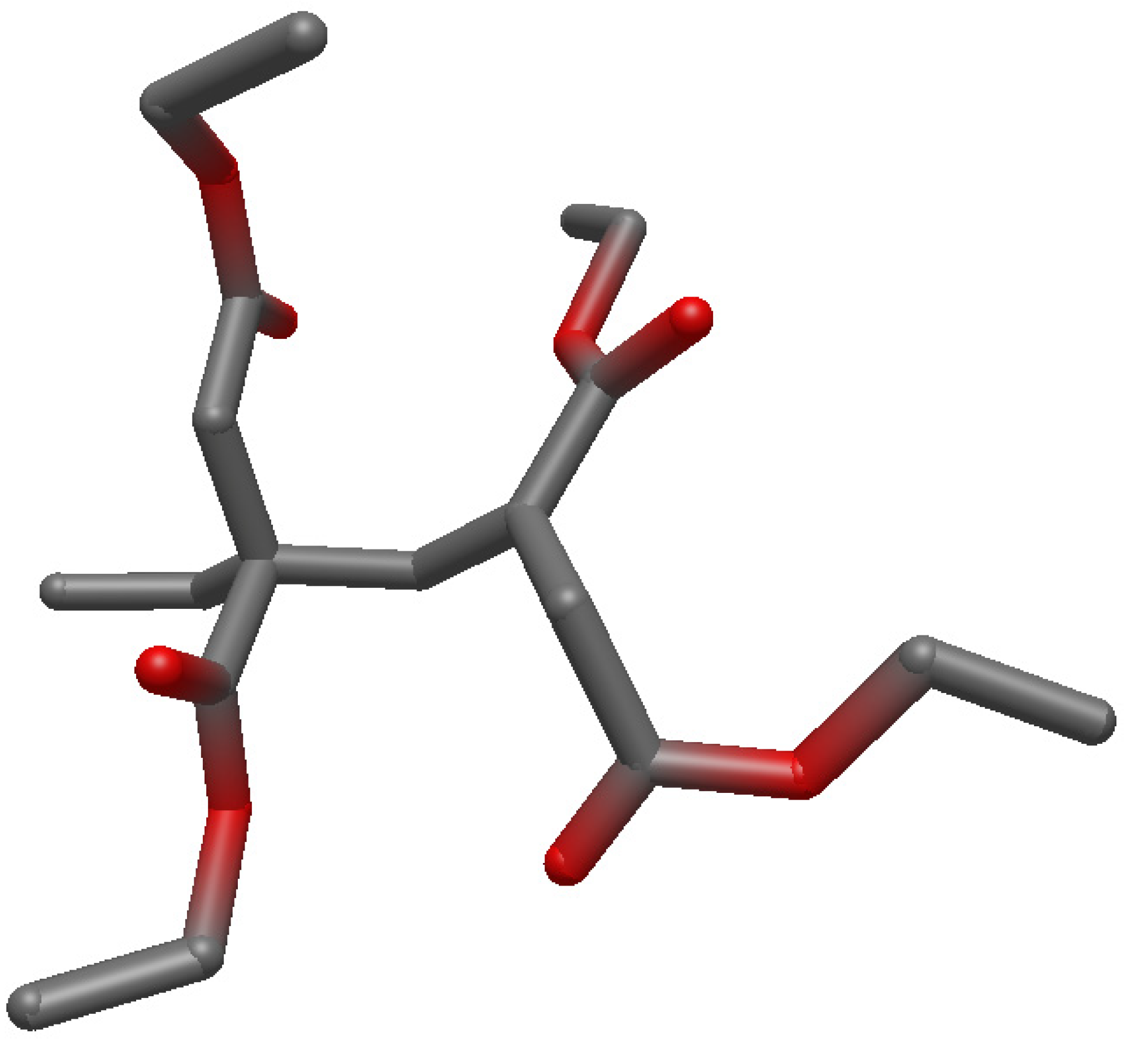
References
- Panic, V.V.; Seslija, S.I.; Popovic, I.G.; Spasojevic, V.D.; Popovic, A.R.; Nikolic, V.B.; Spasojevic, P.M. Simple One-Pot Synthesis of Fully Biobased Unsaturated Polyester Resins Based on Itaconic Acid. Biomacromolecules 2017, 18, 3881–3891. [Google Scholar] [CrossRef] [PubMed]
- Dai, Z.; Yang, Z.; Chen, Z.; Zhao, Z.; Lou, Y.; Zhang, Y.; Liu, T.; Fu, F.; Fu, Y.; Liu, X. Fully Biobased Composites of an Itaconic Acid Derived Unsaturated Polyester Reinforced with Cotton Fabrics. ACS Sustain. Chem. Eng. 2018, 6, 15056–15063. [Google Scholar] [CrossRef]
- Wierckx, N.; Agrimi, G.; Lübeck, P.S.; Steiger, M.G.; Mira, N.P.; Punt, P.J. Metabolic specialization in itaconic acid production: A tale of two fungi. COBIOT 2020, 62, 153–159. [Google Scholar] [CrossRef]
- Zhao, M.; Lu, X.; Zong, H.; Li, J.; Zhuge, B. Itaconic acid production in microorganisms. Biotechnol. Lett. 2018, 40, 455–464. [Google Scholar] [CrossRef]
- Steiger, M.G.; Blumhoff, M.L.; Mattanovich, D.; Sauer, M. Biochemistry of microbial itaconic acid production. Front. Biomol. 2013, 4, 23. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Yee, L.H.; Coote, M.L.; Chaplin, R.P.; Davis, T.P. Determination of propagation rate coefficients for an α-substituted acrylic ester: Pulsed laser polymerization of dimethyl itaconate. J. Polym. Sci. A Polym. Chem. 2000, 38, 2192–2200. [Google Scholar] [CrossRef]
- Szablan, Z.; Stenzel, M.H.; Davis, T.P.; Barner, L.; Barner-Kowollik, C. Depropagation Kinetics of Sterically Demanding Monomers: A Pulsed Laser Size Exclusion Chromatography Study. Macromolecules 2005, 38, 5944–5954. [Google Scholar] [CrossRef]
- Vana, P.; Yee, L.H.; Davis, T.P. Multipulse Initiation in Pulsed Laser and Quenched Instationary Polymerization: Determination of the Propagation and Termination Rate Coefficients for Dicyclohexyl Itaconate Polymerization. Macromolecules 2002, 35, 3008–3016. [Google Scholar] [CrossRef]
- Sato, T.; Inui, S.; Tanaka, H.; Ota, T.; Kamachi, M.; Tanaka, K. Kinetic and ESR studies on the radical polymerization of Di-n-butyl itaconate in benzene. J. Polym. Sci. A Polym. Chem. 1987, 25, 637–652. [Google Scholar] [CrossRef]
- Sato, T.; Takahashi, Y.; Seno, M.; Nakamura, H.; Tanaka, H.; Ota, T. Effect of alkyl groups on the rate constants of propagation and termination in the radical polymerization of dialkyl itaconates. Die Makromol. Chem. 1991, 192, 2909–2914. [Google Scholar] [CrossRef]
- Otsu, T.; Yamagishi, K.; Yoshioka, M. Determination of absolute rate constants for radical polymerization of dialkyl itaconates with various ester groups by electron spin resonance spectroscopy. Macromolecules 1992, 25, 2713–2716. [Google Scholar] [CrossRef]
- Otsu, T.; Yamagishi, K.; Matsumoto, A.; Yoshioka, M.; Watanabe, H. Effect of .alpha.- and .beta.-ester alkyl groups on the propagation and termination rate constants for radical polymerization of dialkyl itaconates. Macromolecules 1993, 26, 3026–3029. [Google Scholar] [CrossRef]
- Sato, T.; Hirose, Y.; Seno, M.; Tanaka, H.; Uchiumi, N.; Matsumoto, M. Kinetic and ESR studies on radical polymerization. Radical polymerization of diisopropyl itaconate. Eur. Polym. J. 1994, 30, 347–352. [Google Scholar] [CrossRef]
- Becker, H. Organikum: Organisch-Chemisches Grundpraktikum; Wiley-VCH: Weinheim, Germany, 2001. [Google Scholar]
- Veličković, J.; Vasović, S. KUHN-MARK-HOUWINK-SAKURADA relations and unperturbed dimensions of poly(di-n-alkyl itaconates). Die Makromol. Chem. 1972, 153, 207–218. [Google Scholar] [CrossRef]
- Pracht, P.; Bohle, F.; Grimme, S. Automated exploration of the low-energy chemical space with fast quantum chemical methods. Phys. Chem. Chem. Phys. 2020, 22, 7169–7192. [Google Scholar] [CrossRef] [PubMed]
- Neese, F. The ORCA program system. Wiley Interdiscip. Rev. Comput. Mol. Sci. 2012, 2, 73–78. [Google Scholar] [CrossRef]
- Neese, F. Software update: The ORCA program system, version 4.0. Wiley Interdiscip. Rev. Comput. Mol. Sci. 2018, 8, e1327. [Google Scholar] [CrossRef]
- Beuermann, S.; Harrisson, S.; Hutchinson, R.A.; Junkers, T.; Russell, G.T. Update and critical reanalysis of IUPAC benchmark propagation rate coefficient data. Polym. Chem. 2022, 13, 1891–1900. [Google Scholar] [CrossRef]
- Beuermann, S.; Buback, M. Rate coefficients of free-radical polymerization deduced from pulsed laser experiments. Prog. Polym. Sci. 2002, 27, 191–254. [Google Scholar] [CrossRef]
- Coote, M.L. Computational Quantum Chemistry For Free-Radical Polymerization. In Encyclopedia of Polymer Science and Technology, 4th ed.; Mark, H.F., Ed.; Wiley-VCH: Weinheim, Germany, 2006. [Google Scholar] [CrossRef]
- Edeleva, M.; Van Steenberge, P.M.H.; Sabbe, K.M.; D’hooge, D.R. Connecting Gas-Phase Computational Chemistry to Condensed Phase Kinetic Modeling: The State-of-the-Art. Polymers 2021, 13, 3027. [Google Scholar] [CrossRef]
- Scott, A.P.; Radom, L. Harmonic Vibrational Frequencies: An Evaluation of Hartree−Fock, Møller−Plesset, Quadratic Configuration Interaction, Density Functional Theory, and Semiempirical Scale Factors. J. Phys. Chem. 1996, 100, 16502–16513. [Google Scholar] [CrossRef]
- Kesharwani, M.K.; Brauer, B.; Martin, J.M.L. Frequency and Zero-Point Vibrational Energy Scale Factors for Double-Hybrid Density Functionals (and Other Selected Methods): Can Anharmonic Force Fields Be Avoided? J. Phys. Chem. A 2015, 119, 1701–1714. [Google Scholar] [CrossRef]
- Nitschke, A.; Riemann, L.; Kollenbach, L.; Braun, V.; Buback, M.; Vana, P. Investigation into the Kinetics of n-Pentyl Methacrylate Radical Polymerization. Macromol. Chem. Phys. 2020, 221, 1900345. [Google Scholar] [CrossRef]
- Olaj, O.F.; Bitai, I.; Hinkelmann, F. The laser-flash-initiated polymerization as a tool of evaluating (individual) kinetic constants of free-radical polymerization, 2. The direct determination of the rate of constant of chain propagation. Die Makromol. Chem. 1987, 188, 1689–1702. [Google Scholar] [CrossRef]
- Olaj, O.F.; Vana, P.; Zoder, M.; Kornherr, A.; Zifferer, G. Is the rate constant of chain propagation kp in radical polymerization really chain-length independent? Macromol. Rapid Commun. 2000, 21, 913–920. [Google Scholar] [CrossRef]
- Olaj, O.F.; Vana, P.; Zoder, M. Chain Length Dependent Propagation Rate Coefficient kp in Pulsed-Laser Polymerization: Variation with Temperature in the Bulk Polymerization of Styrene and Methyl Methacrylate. Macromolecules 2002, 35, 1208–1214. [Google Scholar] [CrossRef]
- Olaj, O.F.; Zoder, M.; Vana, P.; Kornherr, A.; Schnöll-Bitai, I.; Zifferer, G. Chain Length Dependence of Chain Propagation Revisited. Macromolecules 2005, 38, 1944–1948. [Google Scholar] [CrossRef]
- Nikitin, A.N.; Dušička, E.; Lacík, I.; Hutchinson, R.A. Chain-length dependence of the propagation rate coefficient for methyl acrylate polymerization at 25 °C investigated by the PLP-SEC method. Polym. Chem. 2022, 13, 3053–3062. [Google Scholar] [CrossRef]
- Gridnev, A.A.; Ittel, S.D. Dependence of Free-Radical Propagation Rate Constants on the Degree of Polymerization. Macromolecules 1996, 29, 5864–5874. [Google Scholar] [CrossRef]
- Heuts, J.P.A.; Russell, G.T. The nature of the chain-length dependence of the propagation rate coefficient and its effect on the kinetics of free-radical polymerization. 1. Small-molecule studies. Eur. Polym. J. 2006, 42, 3–20. [Google Scholar] [CrossRef]
- Willemse, R.X.E.; Staal, B.B.P.; van Herk, A.M.; Pierik, S.C.J.; Klumperman, B. Application of Matrix-Assisted Laser Desorption Ionization Time-of-Flight Mass Spectrometry in Pulsed Laser Polymerization. Chain-Length-Dependent Propagation Rate Coefficients at High Molecular Weight: An Artifact Caused by Band Broadening in Size Exclusion Chromatography? Macromolecules 2003, 36, 9797–9803. [Google Scholar] [CrossRef]
- Beuermann, S. Requirements Associated with Studies into a Chain-Length Dependence of Propagation Rate Coefficients via PLP−SEC Experiments. Macromolecules 2002, 35, 9300–9305. [Google Scholar] [CrossRef]
- Haehnel, A.P.; Schneider-Baumann, M.; Hiltebrandt, K.U.; Misske, A.M.; Barner-Kowollik, C. Global Trends for kp? Expanding the Frontier of Ester Side Chain Topography in Acrylates and Methacrylates. Macromolecules 2013, 46, 15–28. [Google Scholar] [CrossRef]


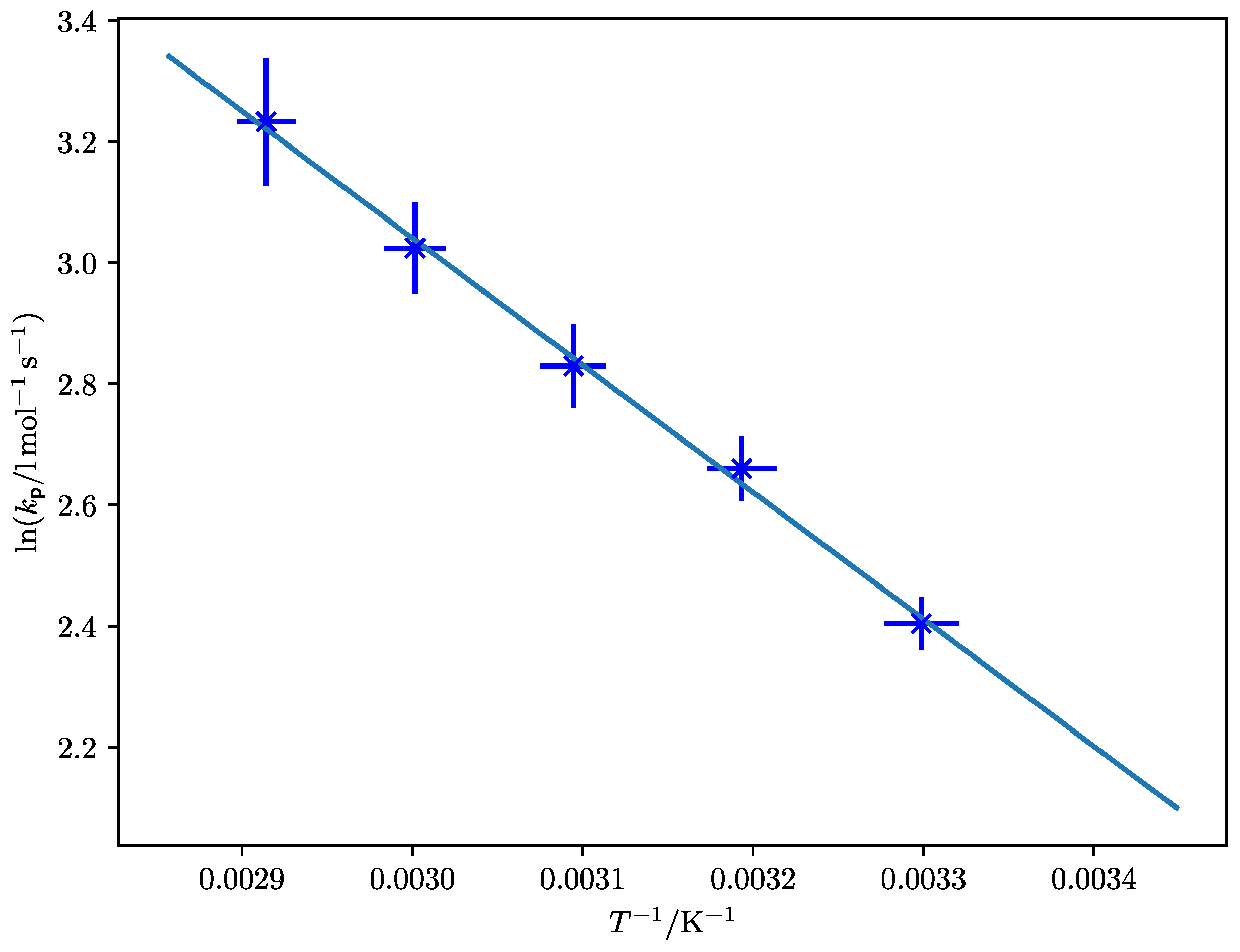

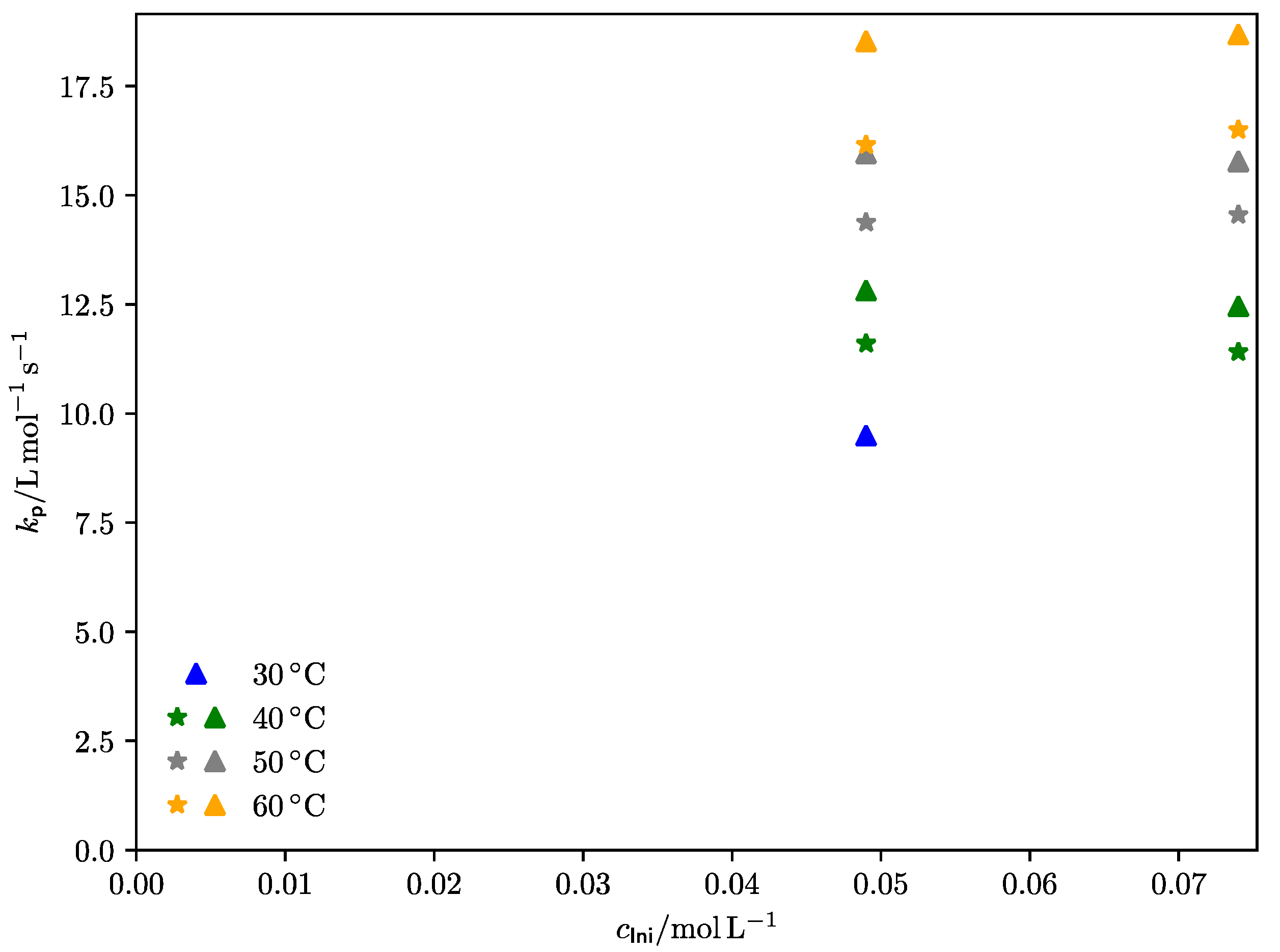


| Method | E0 [kJ·mol–1] | A [L·mol–1·s–1] | EA [kJ·mol–1] |
|---|---|---|---|
| UHF/6-31G(d) | 12.7 | 22.6 | |
| UHF/6-31G(d) scaled | 12.7 | 22.3 | |
| B3LYP/def2-TZVP | 13.8 | 22.7 | |
| B3LYP/def2-TZVP scaled | 13.8 | 22.7 |
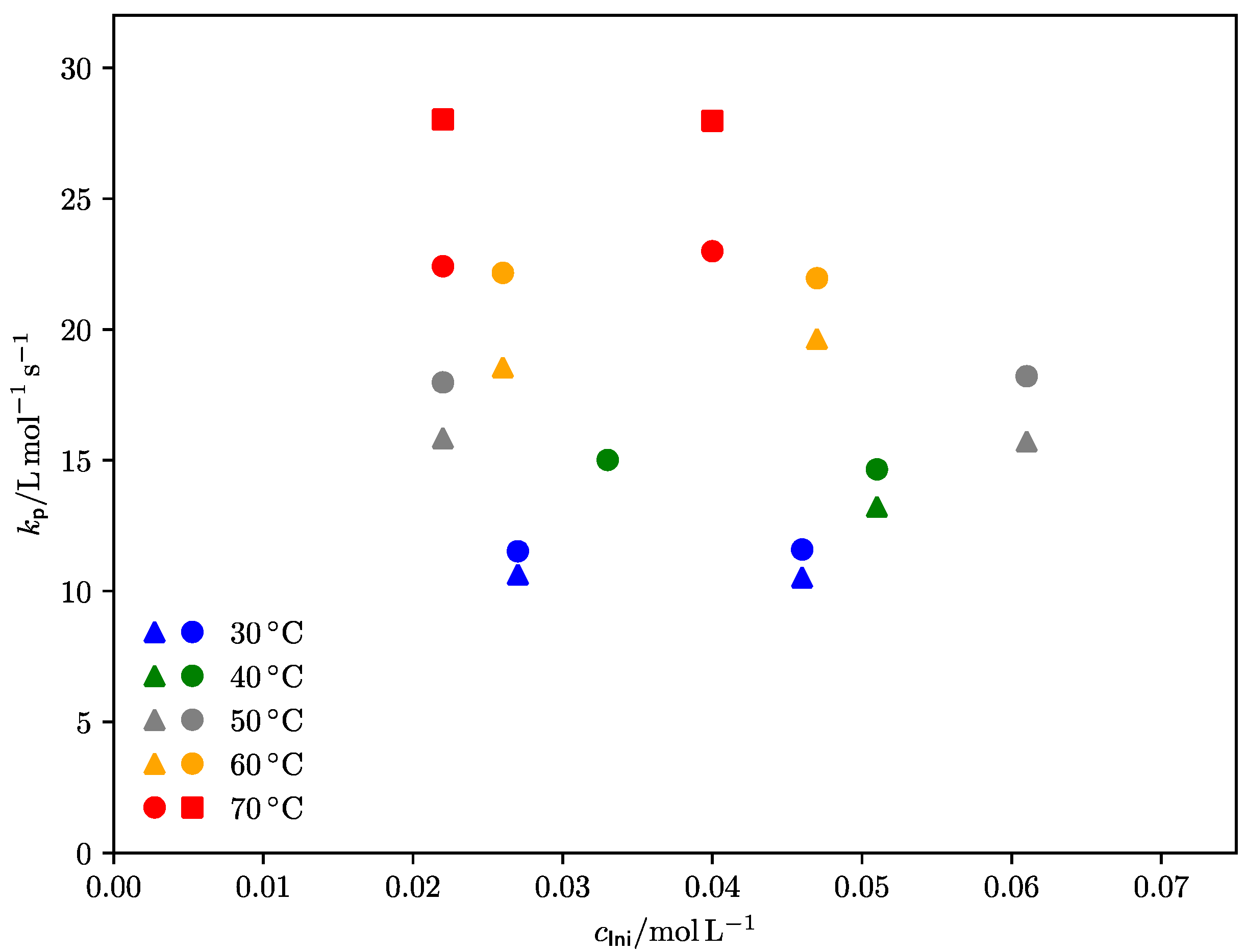
| cIni [mol·L–1] | T/K | npulses | ν [Hz] | Minf1 [g·mol–1] | Minf2 [g·mol–1] | Minf3 [g·mol–1] | kp [L·mol–1·s–1] |
|---|---|---|---|---|---|---|---|
| 0.022 | 343.15 | 2000 | 1 | 25,400 | 42,800 | - | 22.4 |
| 0.022 | 343.15 | 2000 | 2 | 15,400 | 27,700 | - | 28.0 |
| 0.040 | 343.15 | 2000 | 1 | 26,600 | 42,800 | - | 23.0 |
| 0.040 | 343.15 | 2000 | 2 | 15,200 | 28,000 | - | 28.0 |
| 0.026 | 333.15 | 1000 | 0.5 | 43,000 | 73,800 | 108,800 | 18.5 |
| 0.026 | 333.15 | 4000 | 1 | 25,100 | 44,900 | 65,600 | 22.2 |
| 0.047 | 333.15 | 2400 | 0.5 | 44,700 | 79,100 | 116,500 | 19.7 |
| 0.047 | 333.15 | 4000 | 1 | 23,600 | 44,500 | - | 22.0 |
| 0.022 | 323.15 | 2000 | 1 | 20,000 | 36,400 | 54,300 | 18.0 |
| 0.022 | 323.15 | 2000 | 0.5 | 35,700 | 63,100 | 96,100 | 15.9 |
| 0.061 | 323.15 | 2000 | 1 | 19,780 | 36,400 | - | 18.2 |
| 0.061 | 323.15 | 2000 | 0.5 | 35,100 | 61,120 | - | 15.7 |
| 0.033 | 313.15 | 2000 | 1 | 16,320 | 30,050 | - | 15.0 |
| 0.051 | 313.15 | 2000 | 1 | 15,700 | 29,800 | - | 14.7 |
| 0.051 | 313.15 | 2000 | 0.5 | 29,100 | 52,300 | - | 13.2 |
| 0.027 | 303.15 | 2000 | 1 | 12,510 | 23,310 | 35,790 | 11.5 |
| 0.027 | 303.15 | 2000 | 0.5 | 23,500 | 42,900 | 65,000 | 10.6 |
| 0.046 | 303.15 | 2000 | 1 | 12,490 | 23,420 | - | 11.6 |
| 0.046 | 303.15 | 2000 | 0.5 | 23,100 | 42,900 | 64,200 | 10.5 |
| A [L·mol–1·s–1] | EA [kJ·mol–1] |
|---|---|
| (1.1 ± 0.3) · 104 | 17.5 ± 0.6 |
| cIni [mol·L–1] | T/K | ν [Hz] | Minf1 [g·mol–1] | Minf2 [g·mol–1] | Minf3 [g·mol–1] | kp [L·mol–1·s–1] |
|---|---|---|---|---|---|---|
| 0.074 | 333.15 | 0.25 | 70,800 | 129,100 | - | 16.5 |
| 0.049 | 333.15 | 0.25 | 70,200 | 127,900 | 193,900 | 16.2 |
| 0.074 | 333.15 | 0.5 | 41,300 | 72,800 | 111,900 | 18.7 |
| 0.049 | 333.15 | 0.5 | 41,100 | 72,500 | 110,000 | 18.5 |
| 0.074 | 323.15 | 0.25 | 61,800 | 115,200 | - | 14.6 |
| 0.049 | 323.15 | 0.25 | 61,200 | 113,500 | - | 14.4 |
| 0.074 | 323.15 | 0.5 | 33,930 | 61,600 | - | 15.8 |
| 0.049 | 323.15 | 0.5 | 35,000 | 61,000 | - | 16.0 |
| 0.074 | 313.15 | 0.25 | 47,200 | 92,800 | - | 11.4 |
| 0.049 | 313.15 | 0.25 | 50,500 | 93,400 | 136,800 | 11.6 |
| 0.074 | 313.15 | 0.5 | 26,200 | 49,800 | - | 12.5 |
| 0.049 | 313.15 | 0.5 | 28,400 | 48,400 | - | 12.8 |
| 0.049 | 303.15 | 0.5 | 19,400 | 39,100 | - | 9.5 |
| 0.074 | 293.15 | 0.25 | 12,100 | 22,400 | 32,700 | 2.8 |
| 0.049 | 293.15 | 0.25 | 12,400 | 20,700 | 32,100 | 2.7 |
| 0.074 | 293.15 | 0.5 | 16,100 | 29,600 | - | 7.5 |
| 0.049 | 293.15 | 0.5 | 14,300 | 30,100 | - | 7.1 |
| A [L·mol–1·s–1] | EA [kJ·mol–1] |
|---|---|
| (1.0 ± 0.5) · 104 | 17.5 ± 1.2 |
| Substance | A [105 L·mol–1·s–1] | EA [kJ·mol–1] |
|---|---|---|
| DMI [6] | 2.2 | 24.9 |
| DMI [7] | 7.3 | 27.8 |
| DEI (this work) | 0.11 | 17.5 |
| DnPI (this work) | 0.10 | 17.6 |
| DnBI [7] | 0.33 | 21.3 |
| DCHI [7] | 0.99 | 26.5 |
| DCHI [8] | 0.17 | 22.0 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Meyer, E.; Weege, T.; Vana, P. Free-Radical Propagation Rate Coefficients of Diethyl Itaconate and Di-n-Propyl Itaconate Obtained via PLP–SEC. Polymers 2023, 15, 1345. https://doi.org/10.3390/polym15061345
Meyer E, Weege T, Vana P. Free-Radical Propagation Rate Coefficients of Diethyl Itaconate and Di-n-Propyl Itaconate Obtained via PLP–SEC. Polymers. 2023; 15(6):1345. https://doi.org/10.3390/polym15061345
Chicago/Turabian StyleMeyer, Enno, Tobias Weege, and Philipp Vana. 2023. "Free-Radical Propagation Rate Coefficients of Diethyl Itaconate and Di-n-Propyl Itaconate Obtained via PLP–SEC" Polymers 15, no. 6: 1345. https://doi.org/10.3390/polym15061345
APA StyleMeyer, E., Weege, T., & Vana, P. (2023). Free-Radical Propagation Rate Coefficients of Diethyl Itaconate and Di-n-Propyl Itaconate Obtained via PLP–SEC. Polymers, 15(6), 1345. https://doi.org/10.3390/polym15061345










