A Novel In-Line Measurement and Analysis Method of Bubble Growth-Dependent Strain and Deformation Rates during Foaming
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Rheological Characterization
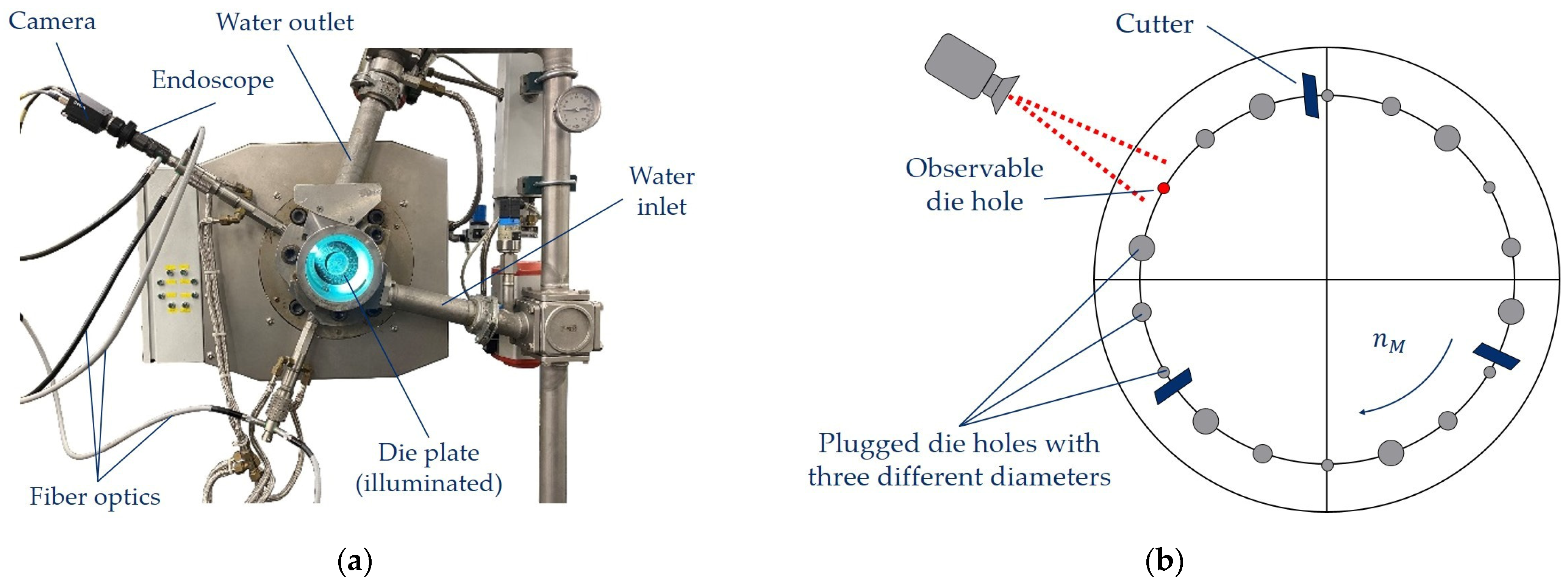
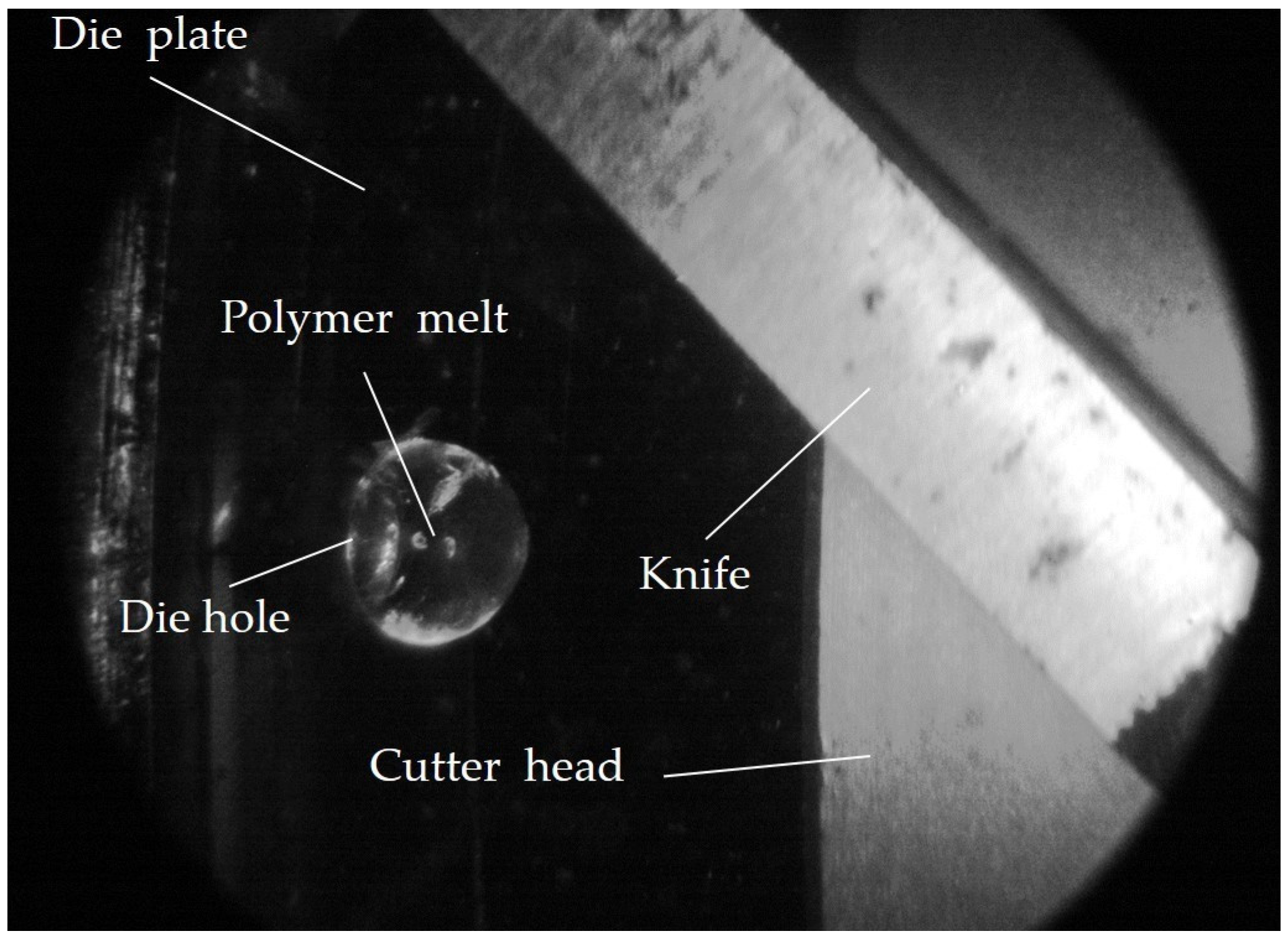
2.3. In-Line Observation Method within the Underwater Pelletizing Process
2.4. Extrusion and Underwater Pelletizing Process Parameters
2.5. Analysis of the Initial Expansion and Deformation Behavior at the Bubble Wall
3. Results and Discussion
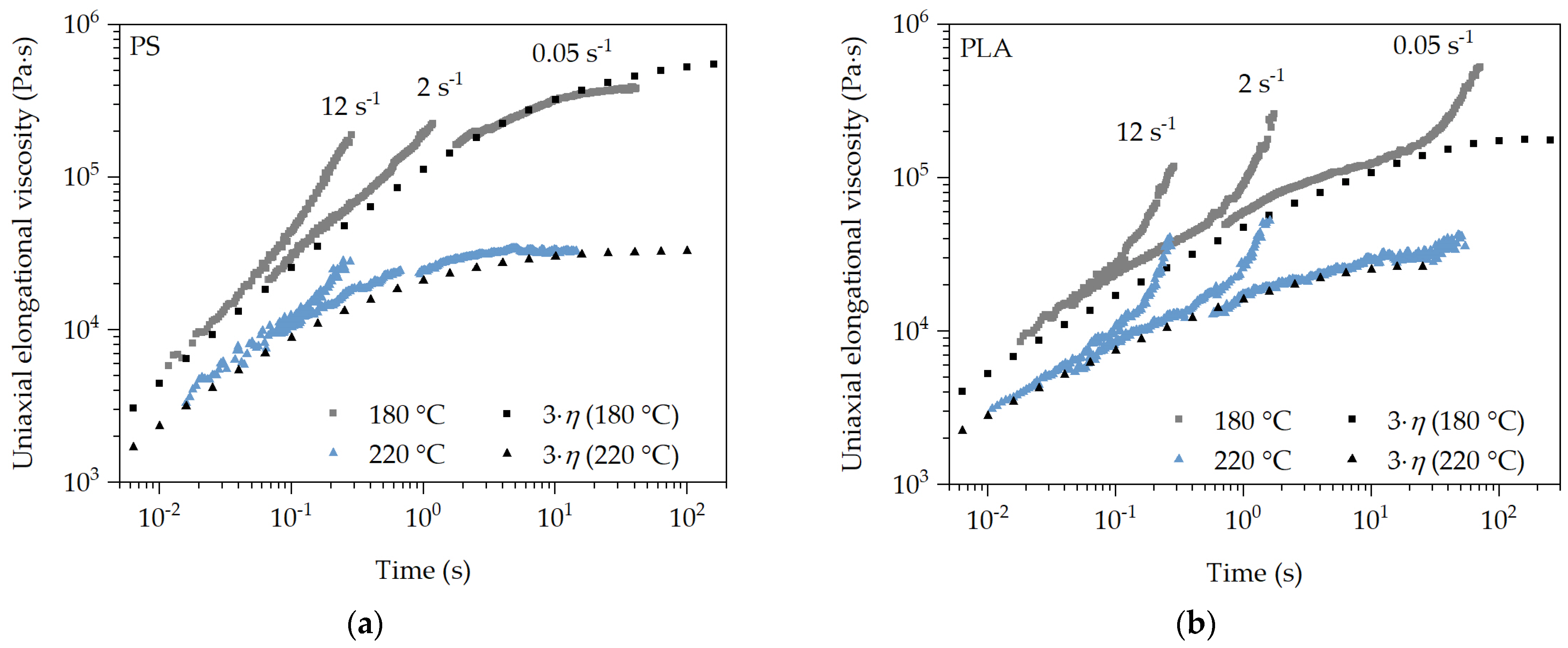
3.1. Transient Uniaxial Elongational Viscosity of PS and PLA
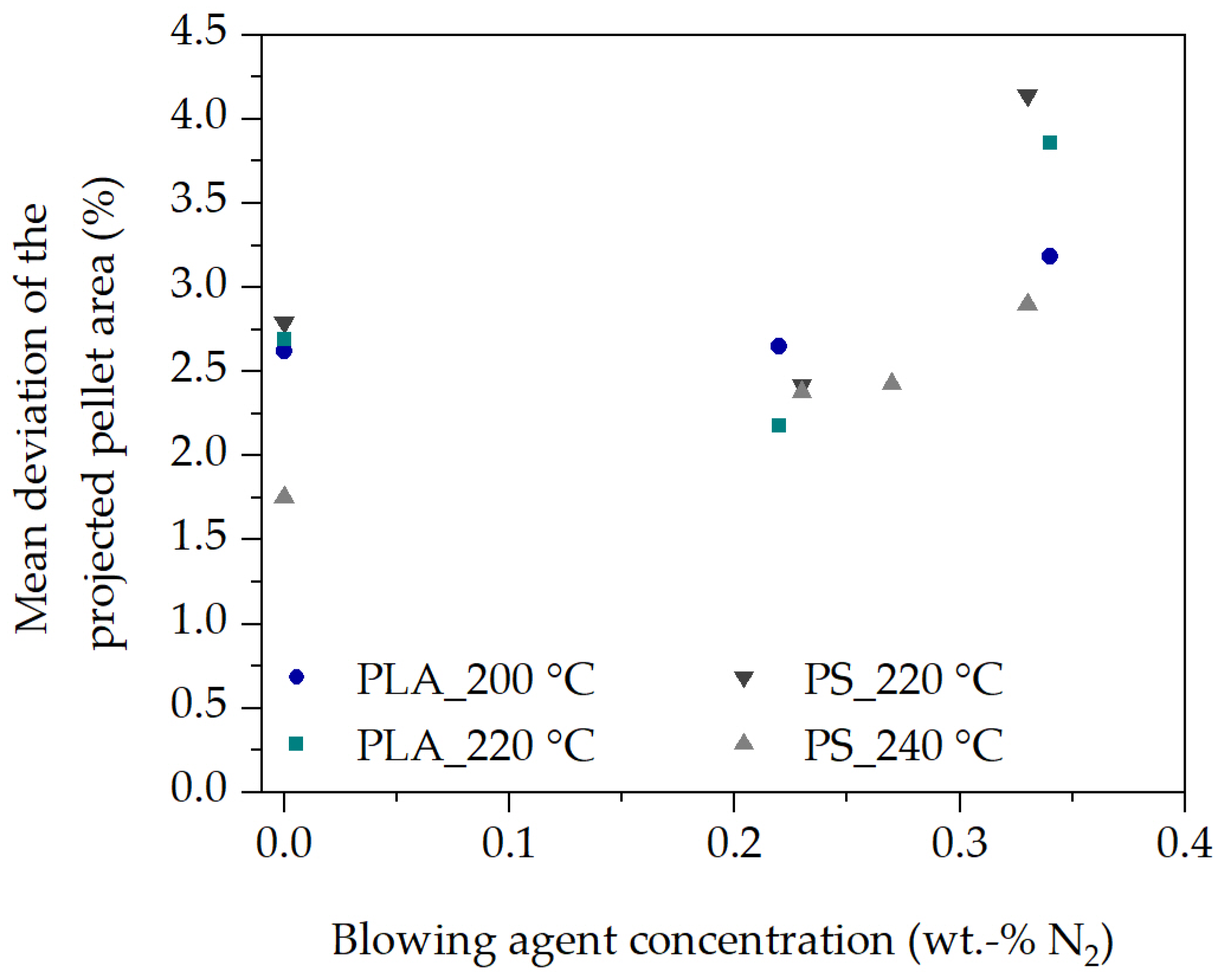
3.2. In-Line Observation Method and Evaluation of Its Accuracy and Reproducibility
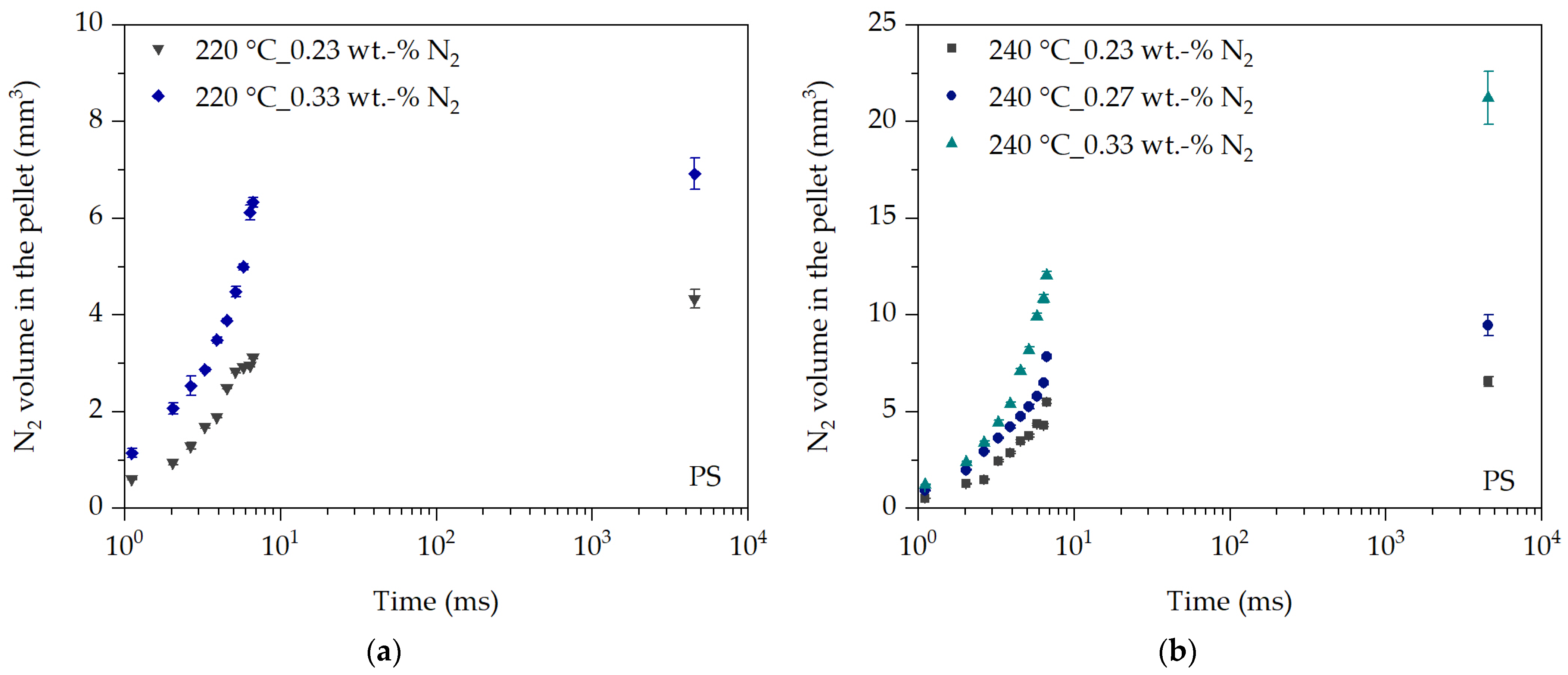
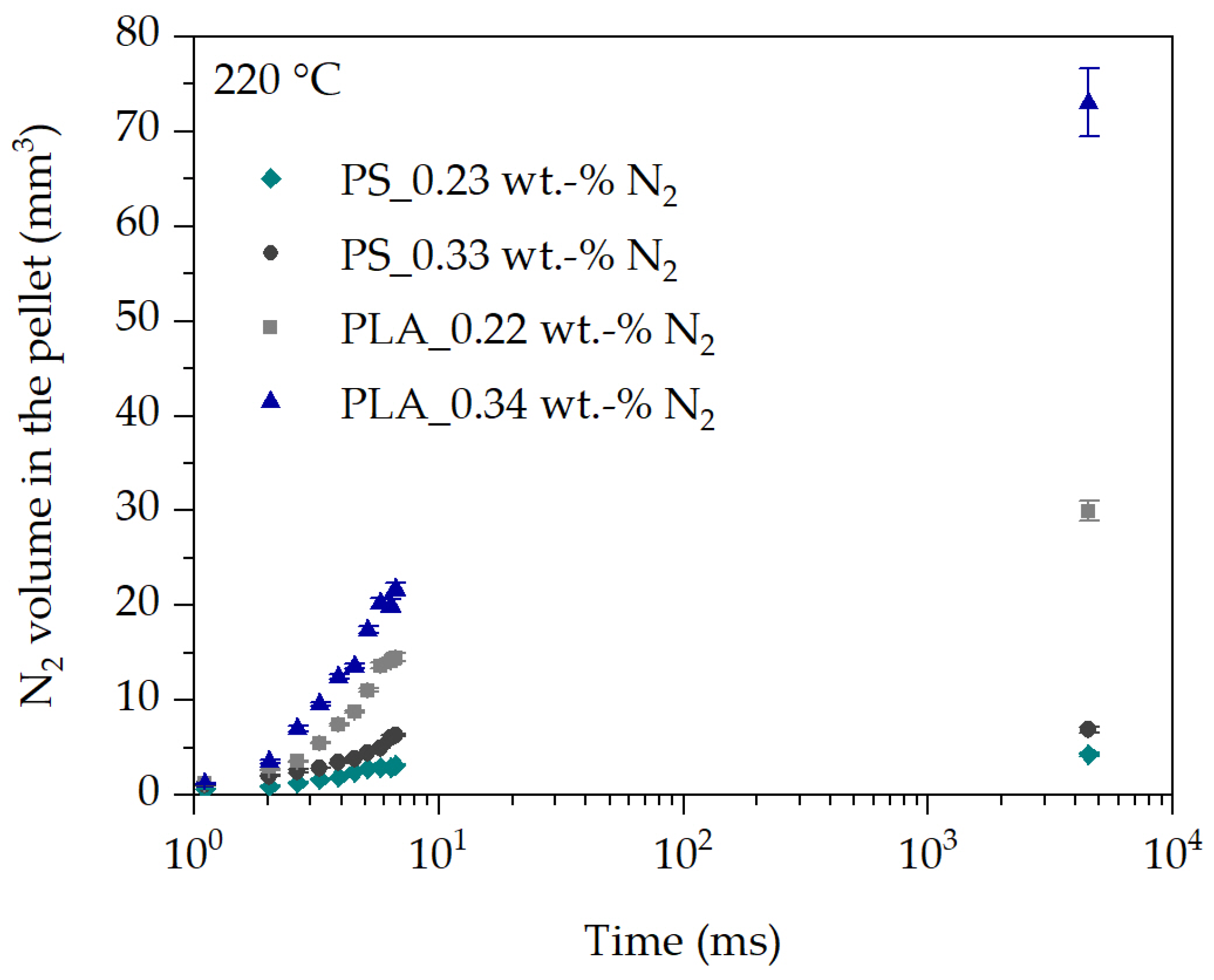
3.3. Analysis of the Initial Expansion Behavior of the Blowing Agent during Foaming
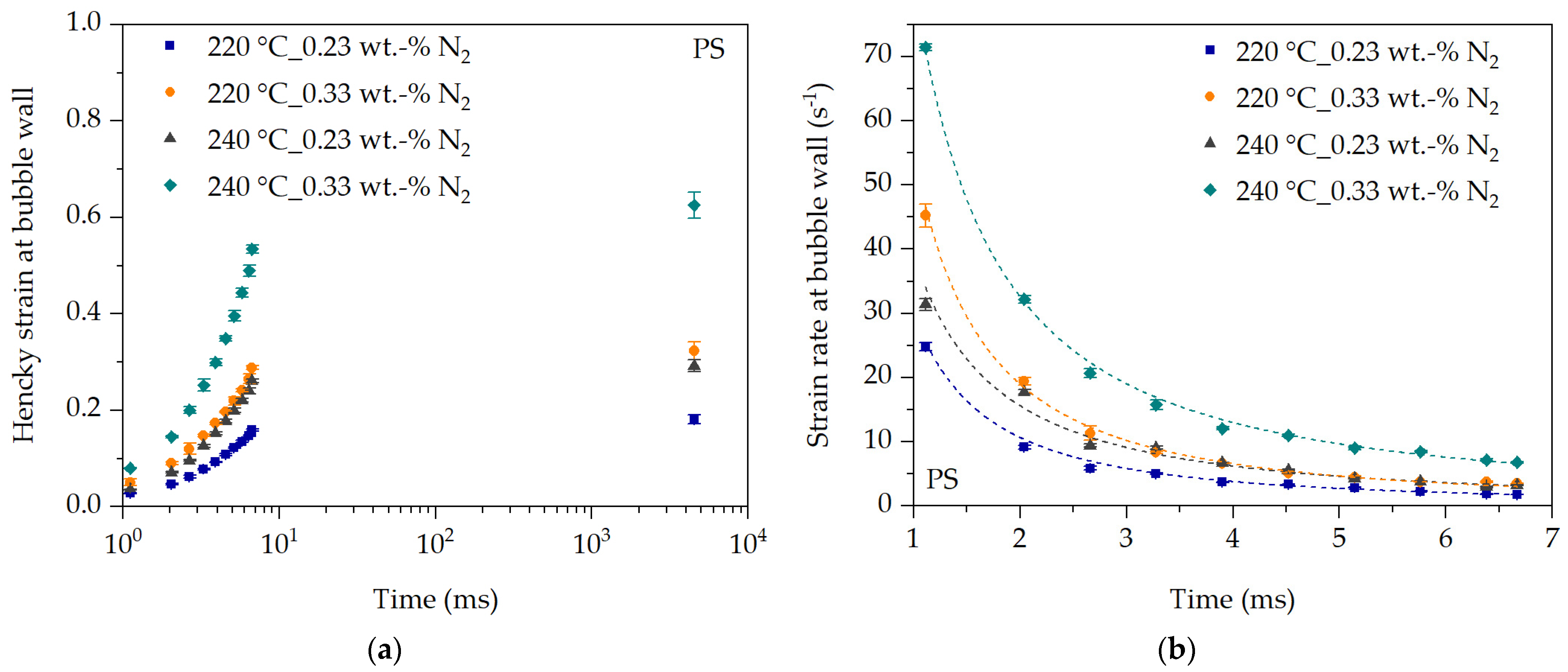
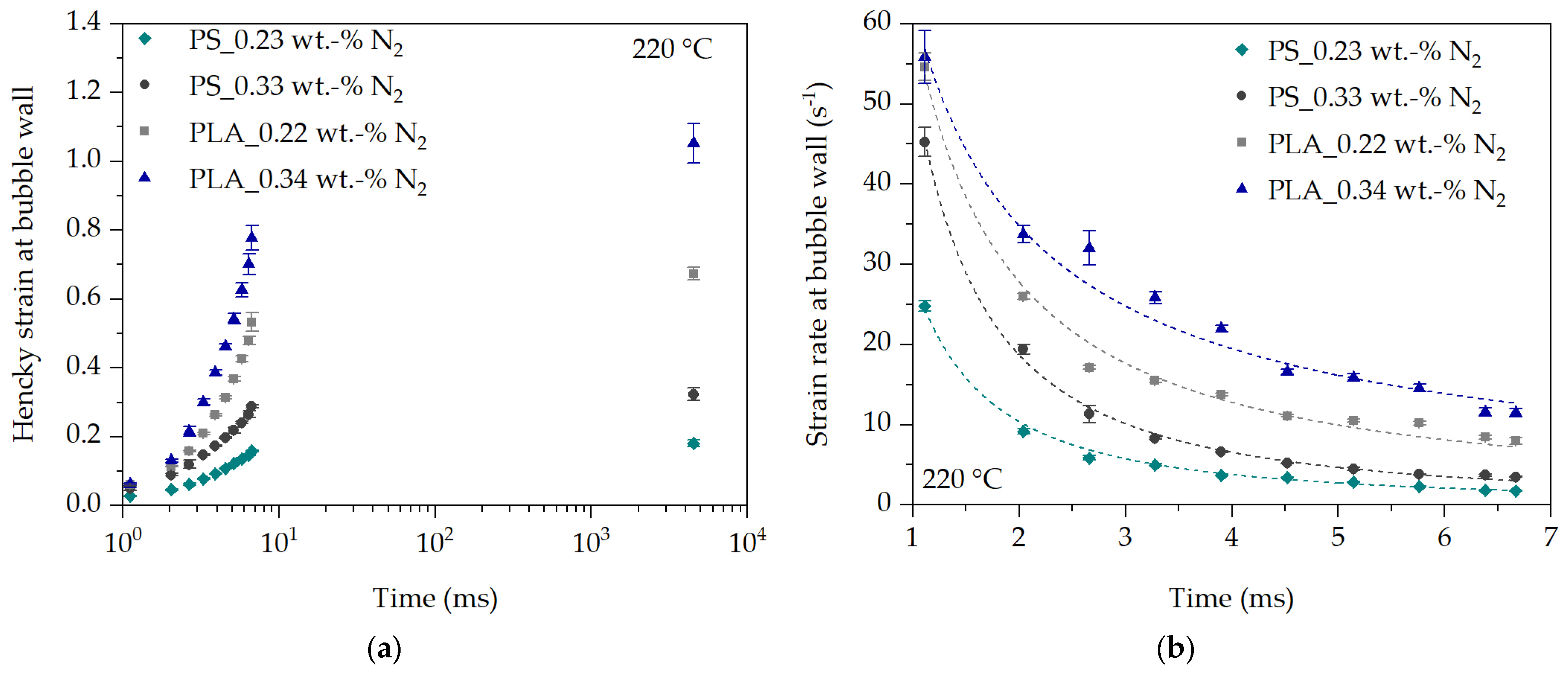
3.4. Deformation Analysis at the Bubble Wall during Bubble Growth
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Weber, H.; De Grave, I.; Röhrl, E.; Altstädt, V. Foamed Plastics. Ullmann’s Encycl. Ind. Chem. 2016, 1–54. [Google Scholar] [CrossRef]
- Bonten, C. Plastics Technology. Introduction and Fundamentals; Hanser: Munich, Germany, 2019; ISBN 978-1-56990-768-9. [Google Scholar]
- Klempner, D.; Sendijarevic, V. Handbook of Polymeric Foams and Foam Technology; Hanser: Munich, Germany, 2004; ISBN 3446218319. [Google Scholar]
- Braun, A. Verfahrensentwicklung von Physikalisch Geschäumten Polypropylenplatten für den Einsatz als Kernmaterial von Sandwichverbunden. Ph.D. Thesis, Montanuniversität Leoben, Leoben, Austria, 2011. [Google Scholar]
- Stange, J. Einfluss Rheologischer Eigenschaften auf das Schäumverhalten von Polypropylenen Unterschiedlicher Molekularer Struktur. Ph.D. Thesis, University of Erlangen-Nürnberg, Erlangen, Germany, 2006. [Google Scholar]
- Park, C.B.; Suh, N.P. Filamentary extrusion of microcellular polymers using a rapid decompressive element. Polym. Eng. Sci. 1996, 36, 34–48. [Google Scholar] [CrossRef]
- Lee, S.T.; Ramesh, N.S. Polymeric Foams: Mechanisms and Materials; CRC Press: Boca Raton, FL, USA, 2004; ISBN 9780203506141. [Google Scholar]
- Taki, K. Experimental and numerical studies on the effects of pressure release rate on number density of bubbles and bubble growth in a polymeric foaming process. Chem. Eng. Sci. 2008, 63, 3643–3653. [Google Scholar] [CrossRef]
- Throne, J.L. Thermoplastic Foam Extrusion: An Introduction; Hanser: Munich, Germany, 2004; ISBN 3446228489. [Google Scholar]
- Schaible, T. Inline-Charakterisierung und Vorhersage der Viskosität Sowie des Initialen Blasenwachstumsverhaltens Beim Schäumen von Treibmittelbeladenen Kunststoffschmelzen. Ph.D. Thesis, University of Stuttgart, Stuttgart, Germany, 2022. [Google Scholar]
- Ramesh, N.S.; Lee, S.T. Blowing Agent Effect on Extensional Viscosity Calculated from Fiber Spinning Method for Foam Processing. J. Cell. Plast. 2000, 36, 374–385. [Google Scholar] [CrossRef]
- Wang, J. Rheology of Foaming Polymers and Its Influence on Microcellular Processing. Ph.D. Thesis, University of Toronto, Toronto, ON, Canada, 2009. [Google Scholar]
- Zhang, Y.; Xin, C.; Li, X.; Waqas, M.; He, Y. Bubble growth model and its influencing factors in a polymer melt under nonisothermal conditions. J. Appl. Polym. Sci. 2019, 136, 47210. [Google Scholar] [CrossRef]
- Ataei, M.; Shaayegan, V.; Wang, C.; Costa, F.; Han, S.; Park, C.B.; Bussmann, M. Numerical analysis of the effect of the local variation of viscosity on bubble growth and deformation in polymer foaming. J. Rheol. 2019, 63, 895–903. [Google Scholar] [CrossRef]
- Schaible, T.; Bonten, C. In-line measurement and modeling of temperature, pressure, and blowing agent dependent viscosity of polymer melts. Appl. Rheol. 2022, 32, 69–82. [Google Scholar] [CrossRef]
- Doi, M.; Edwards, S.F. Dynamics of concentrated polymer systems. Part 2—Molecular motion under flow. J. Chem. Soc. Faraday Trans. 2 1978, 74, 1802–1817. [Google Scholar] [CrossRef]
- Rolón-Garrido, V.H. The molecular stress function (MSF) model in rheology. Rheol. Acta 2014, 53, 663–700. [Google Scholar] [CrossRef]
- Wagner, M.H. Zur Netzwerktheorie von Polymer-Schmelzen. Rheol. Acta 1979, 18, 33–50. [Google Scholar] [CrossRef]
- Schaible, T.; Bonten, C. Elongational viscosity analysis and modeling of a modified polylactide for foaming applications. AIP Conf. Proc. 2023, 2607, 80002. [Google Scholar] [CrossRef]
- Dealy, J.M.; Wang, J. Melt Rheology and Its Applications in the Plastics Industry, 2nd ed.; Springer: Dordrecht, The Netherlands, 2013; ISBN 9789400763951. [Google Scholar]
- Shaayegan, V.; Wang, C.; Costa, F.; Han, S.; Park, C.B. Effect of the melt compressibility and the pressure drop rate on the cell-nucleation behavior in foam injection molding with mold opening. Eur. Polym. J. 2017, 92, 314–325. [Google Scholar] [CrossRef]
- Villamizar, C.A.; Han, C.D. Studies on structural foam processing II. Bubble dynamics in foam injection molding. Polym. Eng. Sci. 1978, 18, 699–710. [Google Scholar] [CrossRef]
- Han, C.D.; Yoo, H.J. Studies on structural foam processing. IV. Bubble growth during mold filling. Polym. Eng. Sci. 1981, 21, 518–533. [Google Scholar] [CrossRef]
- Lee, C.H.; Lee, K.-J.; Jeong, H.G.; Kim, S.W. Growth of gas bubbles in the foam extrusion process. Adv. Polym. Technol. 2000, 19, 97–112. [Google Scholar] [CrossRef]
- Emami, M.; Thompson, M.R.; Vlachopoulos, J. Bubble nucleation in nonpressurized polymer foaming systems. Polym. Eng. Sci. 2014, 54, 1201–1210. [Google Scholar] [CrossRef]
- Ramesh, N.S.; Rasmussen, D.H.; Campbell, G.A. Numerical and experimental studies of bubble growth during the microcellular foaming process. Polym. Eng. Sci. 1991, 31, 1657–1664. [Google Scholar] [CrossRef]
- Tuladhar, T.R.; Mackley, M.R. Experimental observations and modelling relating to foaming and bubble growth from pentane loaded polystyrene melts. Chem. Eng. Sci. 2004, 59, 5997–6014. [Google Scholar] [CrossRef]
- Yao, S.; Chen, Y.; Ling, Y.; Hu, D.; Xi, Z.; Zhao, L. Analysis of Bubble Growth in Supercritical CO2 Extrusion Foaming Polyethylene Terephthalate Process Based on Dynamic Flow Simulation. Polymers 2021, 13, 2799. [Google Scholar] [CrossRef]
- Xu, L.; Huang, Z.; Yang, Y.; Jiang, B.; Sun, J.; Wang, J.; Yang, Y. Effects of temperature on nucleation and collapse of volatile bubbles in the high density polyethylene melt. Polym. Test. 2021, 93, 106932. [Google Scholar] [CrossRef]
- Kropp, D. Extrusion Thermoplastischer Schäume mit Alternativen Treibmitteln. Ph.D. Thesis, RWTH Aachen, Aachen, Germany, 1999. [Google Scholar]
- Murillo Castellón, S. In-Situ-Modifikation von Polylactiden Mittels Reaktiver Extrusion zur Herstellung von Bio-Schäumen. Ph.D. Thesis, University of Stuttgart, Stuttgart, Germany, 2020. [Google Scholar]
- Sentmanat, M.L. Miniature universal testing platform: From extensional melt rheology to solid-state deformation behavior. Rheol. Acta 2004, 43, 657–669. [Google Scholar] [CrossRef]
- Geiger, K.; Grünschloss, E. Analytische Betrachtung zum Granulatbildungsprozess bei der Unterwassergranulierung. In 20. Stuttgarter Kunststoff-Kolloquium 2007, 1st ed.; Fritz, H.G., Eisenbach, C.D., Eds.; Universität Stuttgart: Stuttgart, Germany, 2007; ISBN 978-3-00-020846-1. [Google Scholar]
- Park, C.B.; Baldwin, D.F.; Suh, N.P. Effect of the pressure drop rate on cell nucleation in continuous processing of microcellular polymers. Polym. Eng. Sci. 1995, 35, 432–440. [Google Scholar] [CrossRef]
- Grünschloss, E.; Geiger, K. Visuelle Analyse des Schneidvorgangs bei der Unterwassergranulierung. In 20. Stuttgarter Kunststoff-Kolloquium 2007, 1st ed.; Fritz, H.G., Eisenbach, C.D., Eds.; Universität Stuttgart: Stuttgart, Germany, 2007; ISBN 978-3-00-020846-1. [Google Scholar]
- Kast, O.; Musialek, M.; Geiger, K.; Bonten, C. Influences on particle shape in underwater pelletizing processes. AIP Conf. Proc. 2014, 1593, 20–23. [Google Scholar] [CrossRef]
- Kast, O.; Geiger, K.; Grünschloss, E.; Bonten, C. Analysis of pellet shaping kinetics at the die opening in underwater pelletizing processes. Polym. Eng. Sci. 2015, 55, 1170–1176. [Google Scholar] [CrossRef]
- Altenbach, H. Kontinuumsmechanik. In Einführung in die Materialunabhängigen und Materialabhängigen Gleichungen; Springer: Berlin/Heidelberg, Germany, 2015; ISBN 978-3-662-47069-5. [Google Scholar]
- Lombardi, L.; Tammaro, D. Effect of polymer swell in extrusion foaming of low-density polyethylene. Phys. Fluids 2021, 33, 33104. [Google Scholar] [CrossRef]
- Rólon Garrido, V.H. Molecular Structure and Constitutive Modelling of Polymer Melts. Ph.D. Thesis, TU Berlin, Berlin, Germany, 2007. [Google Scholar]
- Wagner, M.H.; Bastian, H.; Hachmann, P.; Meissner, J.; Kurzbeck, S.; Münstedt, H.; Langouche, F. The strain-hardening behaviour of linear and long-chain-branched polyolefin melts in extensional flows. Rheol. Acta 2000, 39, 97–109. [Google Scholar] [CrossRef]
- Vasvári, G.; Haimhoffer, Á.; Horváth, L.; Budai, I.; Trencsényi, G.; Béresová, M.; Dobó-Nagy, C.; Váradi, J.; Bácskay, I.; Ujhelyi, Z.; et al. Development and Characterisation of Gastroretentive Solid Dosage Form Based on Melt Foaming. AAPS PharmSciTech 2019, 20, 290. [Google Scholar] [CrossRef]




| Polymer Mass Flow Rate (kg/h) | N2 Mass Flow Rate (kg/h) | Mass Fraction (wt.-% N2) | |
|---|---|---|---|
| PS | 29.8 | 0.0; 0.07 and 0.1 | 0.0; 0.23 and 0.33 |
| PLA | 35.7 | 0.0; 0.08 and 0.12 | 0.0; 0.22 and 0.34 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Schaible, T.; Bonten, C. A Novel In-Line Measurement and Analysis Method of Bubble Growth-Dependent Strain and Deformation Rates during Foaming. Polymers 2024, 16, 277. https://doi.org/10.3390/polym16020277
Schaible T, Bonten C. A Novel In-Line Measurement and Analysis Method of Bubble Growth-Dependent Strain and Deformation Rates during Foaming. Polymers. 2024; 16(2):277. https://doi.org/10.3390/polym16020277
Chicago/Turabian StyleSchaible, Tobias, and Christian Bonten. 2024. "A Novel In-Line Measurement and Analysis Method of Bubble Growth-Dependent Strain and Deformation Rates during Foaming" Polymers 16, no. 2: 277. https://doi.org/10.3390/polym16020277
APA StyleSchaible, T., & Bonten, C. (2024). A Novel In-Line Measurement and Analysis Method of Bubble Growth-Dependent Strain and Deformation Rates during Foaming. Polymers, 16(2), 277. https://doi.org/10.3390/polym16020277






