Performance of Combined Woven Roving and Mat Glass-Fiber Reinforced Polymer Composites Under Absorption Tower Lifting Loads
Abstract
:1. Introduction
2. Structural Evaluation Process of the Hoisting Operation Using a Computational Tool
2.1. System Components
2.1.1. Dimensions of the Absorption Tower Sections
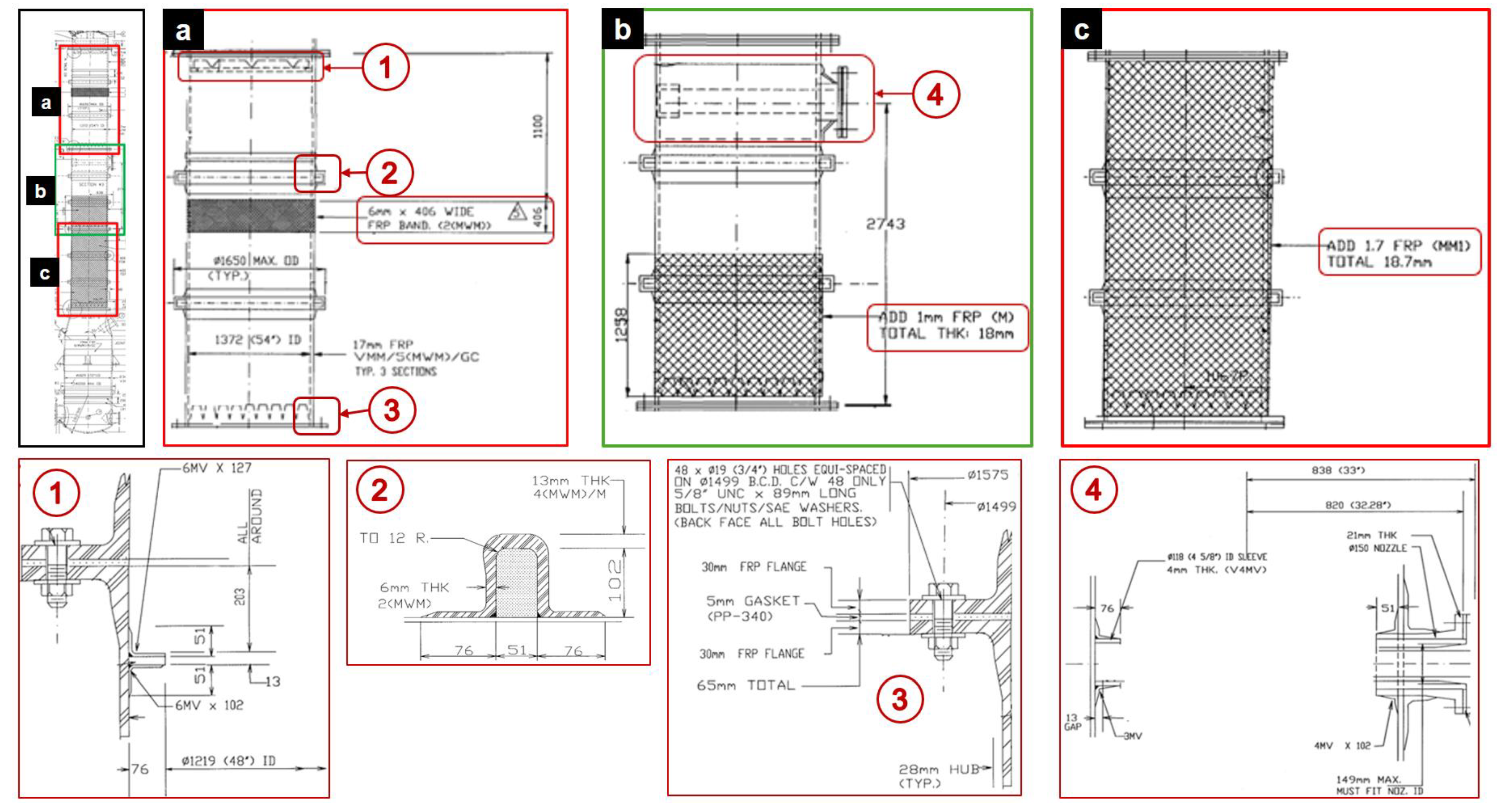
- Each section has a length of 3048 mm, an inside diameter of 1372 mm, a minimum wall thickness of 17 mm, and an outside flange diameter of 1575 mm.
- The upper and lower flanges of each section have 48 equidistant 19 mm diameter holes with a bolt circle diameter of 1499 mm and a wall thickness of 30 mm.
- Each of the GFRP tower sections has 38 mm rings located in the lower areas. The lower ring supports 5000 kg of ceramic packing.
- All sections have two structural reinforcements of variable thickness from 6 to 13 mm.
- Section (1): It has a reinforcement band 406 mm long and 6 mm thick, located 1100 mm from the upper edge. In addition, it has a 10 mm thick inner ring that supports a demister of the absorption tower.
- Section (2): It has two nozzles, an inner nozzle with a diameter of 118 mm and a thickness of 4 mm and a through nozzle with a diameter of 150 mm and a thickness of 21 mm, which support the liquid distributor. These nozzles are attached to the section by bandages, with the inner bandages being 4 mm thick and the outer bandage 27 mm thick. Additionally, this section has a 1 mm thick, 1258 mm long overlay on the bottom flange.
- Section (3): This section has a 1.7 mm thick coating on its shell.
2.1.2. Fibers and Laminate
2.1.3. Woven Roving and Mat Glass-Fiber Reinforced Polymer Composite
2.2. Critical Conditions and Parameters for the Lifting Maneuver
2.2.1. Loading Conditions
2.2.2. Constraints and Supports
2.2.3. Environmental Conditions
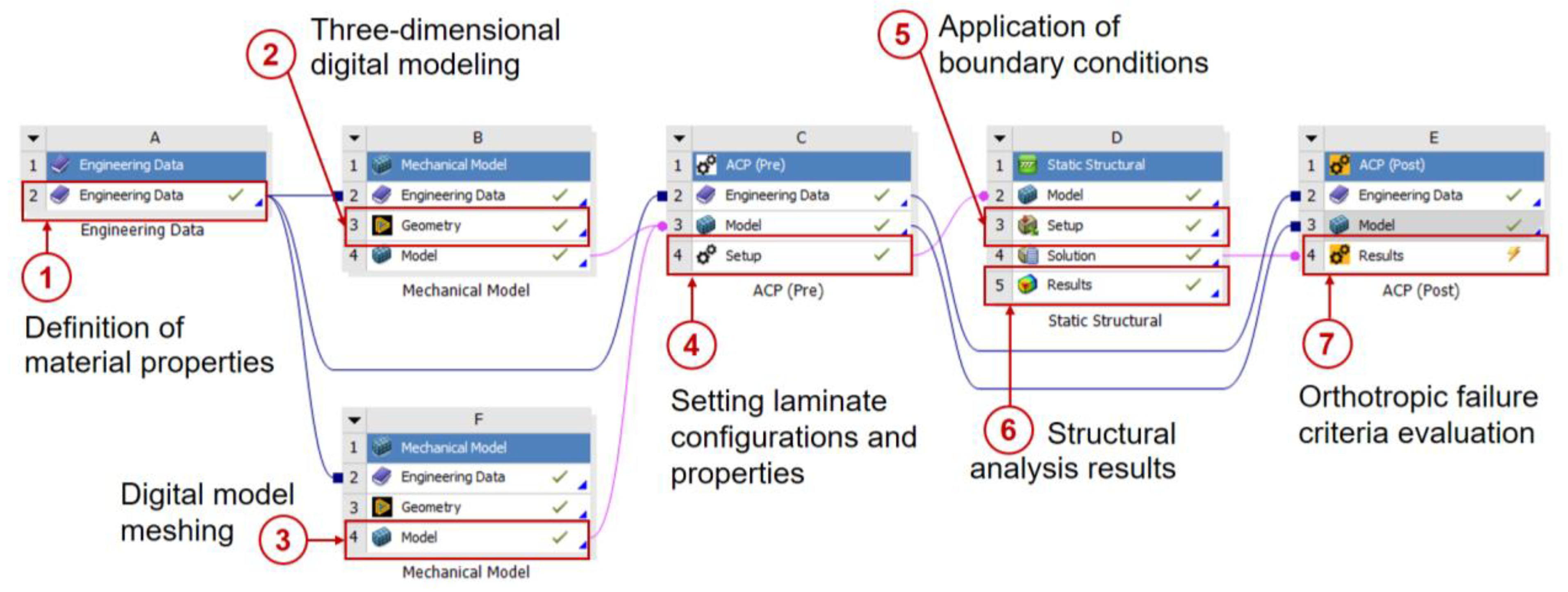
2.3. Generation of Virtual Models of the Absorption Tower
- Engineering Data: In this module, the mechanical properties of the different materials to be used in the simulation are entered. Parameters such as the modulus of elasticity, Poisson’s ratio, and density, among others, are defined here to ensure that the analysis reflects the real characteristics of the materials.
- Mechanical Model: In this module, the geometries of the tower sections are imported and defined. Subsequently, an appropriate meshing is applied to the needs of the analysis, which allows discretizing the geometry for its structural evaluation.
- ACP (Pre): This module is used to define the properties of the laminates in the imported geometries. The laminate layups are managed and the properties of the composite materials are assigned to the different tower sections.
- Static Structural: In this module, the boundary conditions are applied and the loads corresponding to the tower sections are introduced. A static analysis is performed to evaluate how the materials respond to the applied loading conditions.
- ACP (Post): This module allows a detailed analysis of the failure criteria for composite materials. Simulation results are examined to determine the behavior of the composite material under the applied loading conditions, identifying possible failure points.
Conversion of Technical Drawings to Digital Models Using Autodesk Inventor
- The base section has a height of 3048 mm, with an inside diameter of 1372 mm and an outside flange diameter of 1575 mm. In addition, it includes a hole circle with a radius of 749.5 mm in each flange. Each hole circle has 48 holes each with a radius of 9.5 mm. The base section also features a 13 mm notch radius at its flanged edges.
- In the lower zone of the base section, there is a cut in the shell at 203 mm from the lower edge, which represents an overthickness. Additionally, in the upper zone, the same cut is present in relation to the upper edge, except in Section 1, where it varies at 106 mm in the upper zone. At 51 mm from the lower edge of the base section is the support ring for the ceramic packing, which has an inner radius of 635 mm and an outer radius of 686 mm.
- The typical reinforcement has the shape of a circular band with a radius of 686 mm and a height of 203 mm. In its middle zone, it has a projection with an outer radius of 788 mm and a height of 51 mm. In addition, the corners of the reinforcement are rounded with a notch radius of 12 mm.
- Section (1): This section features a 406 mm wide structural band located 1100 mm from the top edge. In addition, this section has an upper support ring for the demister, located 203 mm from the top edge. This ring has an inner radius of 610 mm and an outer radius of 686 mm (Figure 4a).
- Section (2): This section has a manway with a diameter of 96 mm and a length of 72 mm. The manway is attached to the shell section by an outer and inner mounting flange. The outer flange has an inner radius of 96 mm, an outer radius of 172 mm and an elevation of 21 mm. The inner flange has an inner radius of 96 mm, an outer radius of 127 mm and an elevation of 51 mm. In addition, this section has an interior flange with an inner radius of 63 mm, outer radius of 110 mm, and a length of 76 mm (Figure 4b).
- Section (3): This section does not present differentiating details with respect to the base model; in fact, the base model is based on this section (Figure 4c).
2.4. Modeling the Laminate of the Gas Absorption Tower Sections
2.5. Mesh Sensitivity Analysis of Tower Sections and Components in Structural Analysis
2.6. Modeling Loading Conditions of the Lifting Operation
- Vertical lifting: For the case of vertical lifting, the first condition considered was the fastening of the tower by bolts, which will go in their respective bolt circle of the upper flange. Therefore, the ANSYS Fixed Support function was used, which fixes these holes. Additionally, a force corresponding to 5000 kg of packing ceramics, equivalent to 50 kN, was applied on the ceramic packing support ring with a direction on the Y-axis, coinciding with the direction of gravity acceleration. In Figure 6a, the loading conditions for the study of vertical lifting are shown.
- Horizontal lifting: For the case of horizontal lifting, the absorption tower sections were considered to be bolted at their two flanges. Vertical fixed support was used in the holes of both flanges. The gravity force was applied in the X direction. Finally, different ceramic packing was modeled as a distributed load of 50 kN for three different cases: case 1 loaded in 50% of the shell, case 2 in 25%, and case 3 in 12.5% of the shell inner surface.
3. Analysis of Results and Discussion
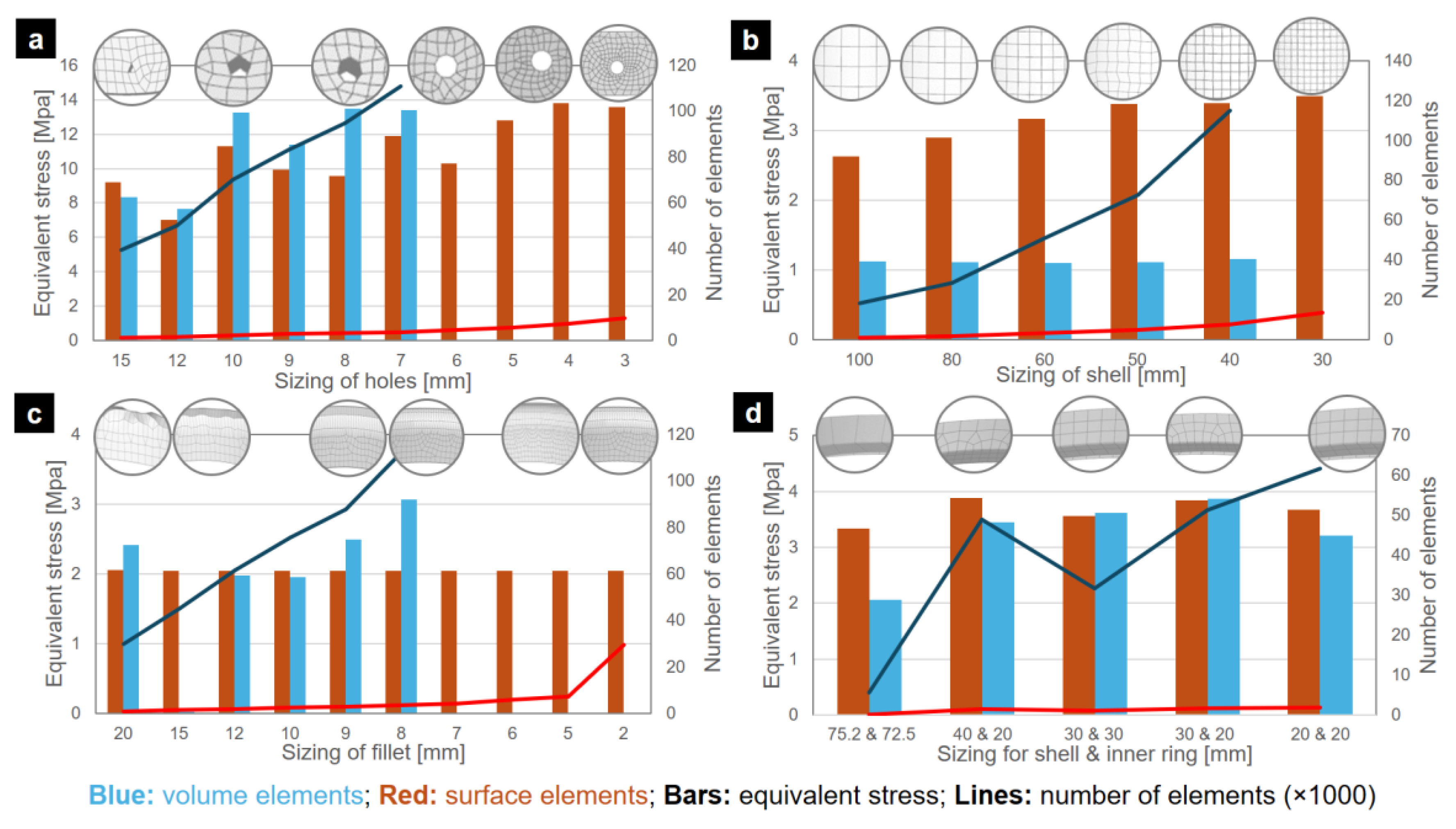
3.1. Sensitivity Analysis of Mesh: Effect of Mesh Size on Tower Stress Field Convergence
- For flange edges with holes, a sizing was assigned to the holes. The volumetric geometry tends to reach an equivalent stress of 13.37 MPa with a quantity of 94 thousand elements or higher, using a sizing of 8 mm. In surface geometry, it is possible to reach a similar value of 12.79 MPa with only 5675 elements, equivalent to a sizing of 5 mm.
- For tower shells, a general sizing is applied to this zone. For the volumetric geometry, although a smaller sizing will be assigned, its stress value did not vary significantly, remaining around 1.1 MPa, even considering that the number of elements was increased to 115 thousand elements. In the case of surface geometry, the equivalent stress varied from 2.63 MPa to 3.49 MPa. The case with the largest number of elements had only 13,536 elements for a 30 mm sizing, considering that its predecessor, the 40 mm sizing, has only 7668 elements.
- For notches, a sizing was assigned specifically to the notch curvature. In the volumetric geometry, over 87 thousand elements in the case of 9 mm sizing, an increase in the equivalent stress was observed, going from 1.95 MPa with 10 mm sizing to 3.06 MPa with 8 mm sizing, reaching a quantity of 112 thousand elements. On the other hand, the surface geometry was maintained with an average of 2 MPa and the number of elements remained under 8 thousand until the sizing of 5 mm.
- For the joint between the support ring of ceramic packing and the shell, a sizing was applied to the ring and to the corresponding shell zone, evaluating several combinations. For the volumetric case, the values tend to be around 3.6 MPa, and the number of elements varies depending on the combination of sizing in the ring and the shell, always exceeding 31 thousand elements for the cases with results similar to 3.6 MPa. For the surface geometry case, it is possible to obtain results similar to those of the volume geometry with the default ANSYS sizing configuration, which is 75.2 mm, achieving 3.34 MPa with only 176 elements.
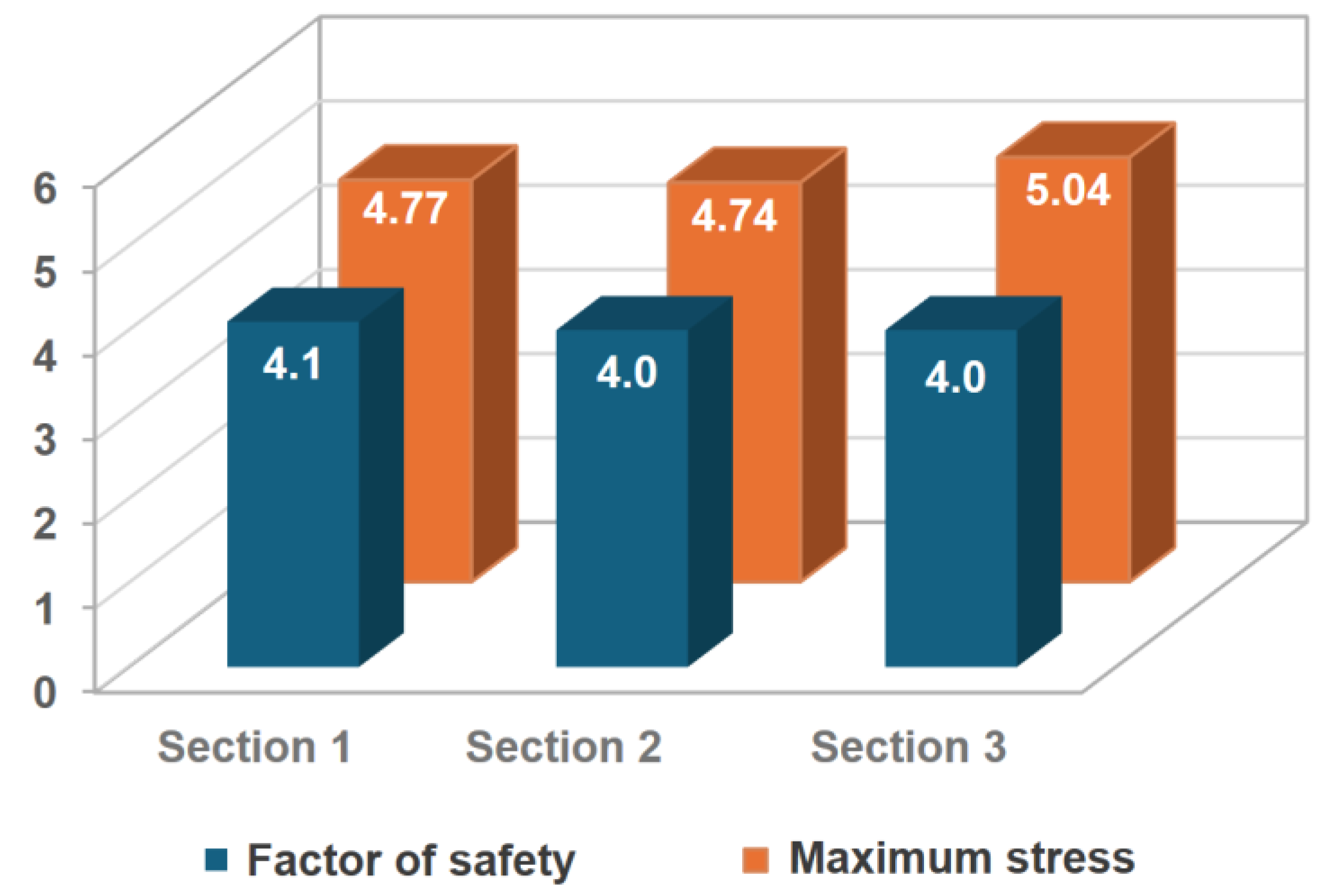
3.2. Stress Resistance and Damage of Tower Sections during Vertical Hoisting
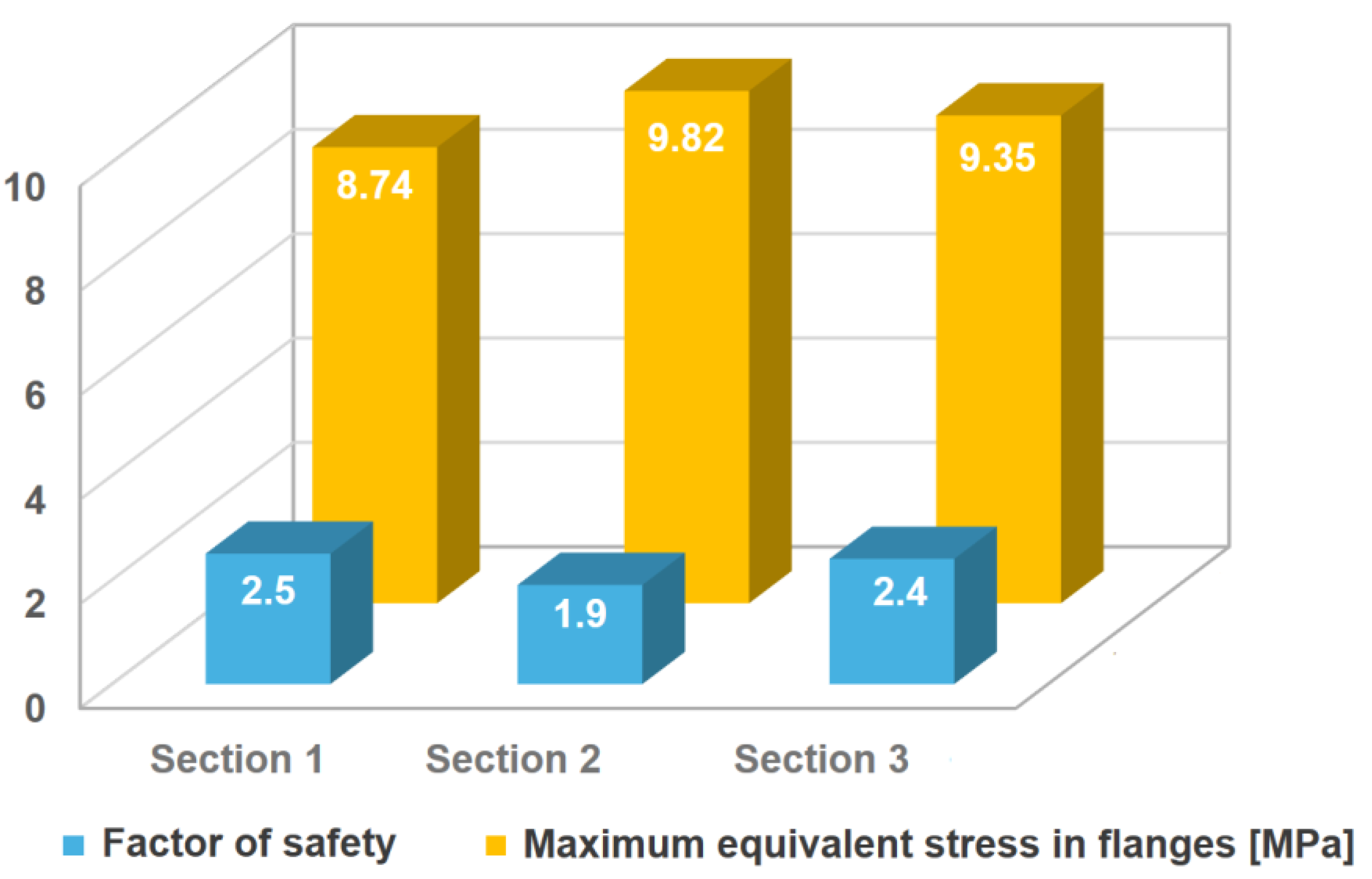
3.3. GFRP Tower Response to Horizontal Hoisting: Influence of Ceramic Packing Weight
- Case 1: An evenly distributed load resulted in a maximum stress of 4.66 MPa and a factor of safety of 4.2.
- Case 2: A more concentrated load distribution led to a higher maximum stress of 7.71 MPa and a reduced factor of safety of 2.8.
- Case 3: The most unfavorable scenario, with packing concentrated over 12.5% of the shell, yielded the highest stress of 8.74 MPa and a factor of safety of 2.5.
4. Conclusions
- ♦
- Surface geometry models can achieve comparable stress results to more computationally intensive volumetric models, but with significantly fewer elements, reducing computational resources required.
- ♦
- The analysis of the lifting operations for the FRP tanks demonstrated factors of safety ranging from 1.9 to 2.5 for horizontal lifting and a factor of safety of 4.0 for vertical lifting, providing a sufficient margin for safe execution.
- ♦
- However, low safety factors were observed in certain scenarios, especially for horizontal lifting with concentrated packing weight, necessitating a comprehensive assessment considering industry standards, dynamic loads, potential material degradation, and mitigation strategies to ensure long-term structural integrity.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Wang, Q.; Liu, X.; Peng, K. Study on Shearing Behavior of Circular Concrete-Filled CFRP (Carbon Fiber-Reinforced Plastics)-Steel Tube. Polymers 2022, 14, 3350. [Google Scholar] [CrossRef] [PubMed]
- Tuninetti, V.; Medina, C.; Salas, A.; Valdivia, I.; Fernández, E.; Meléndrez, M.; Pincheira, G.; Flores, P. Fiber-Reinforced Composite Materials; Synthesis Lectures on Mechanical Engineering; Springer Nature: Cham, Switzerland, 2023; ISBN 978-3-031-32557-1. [Google Scholar] [CrossRef]
- Bar, M.; Alagirusamy, R.; Das, A.; Ouagne, P. Low velocity impact response of flax/polypropylene hybrid roving based woven fabric composites: Where does it stand with respect to GRPC? Polym. Test. 2020, 89, 106565. [Google Scholar] [CrossRef]
- Chang, H.-K.; Lu, L.-M.; Liao, H.-C.; Yu, W.-D.; Lim, Z.-Y. Real-Time Safety Warning System for Lifting Operations in Construction Sites. In Proceedings of the 3rd IEEE International Conference on Electronic Communications, Internet of Things and Big Data 2023, Taichung, Taiwan, 14–16 April 2023; p. 3. [Google Scholar] [CrossRef]
- Rashid, B.; Sadeq, A.; Ebraheem, M.; Mohammed, A.R. Mechanical properties of hybrid woven roving and chopped strand mat glass fabric reinforced polyester composites. Mater. Res. Express 2019, 6, 105208. [Google Scholar] [CrossRef]
- Zhou, Z.; Meng, L.; Zeng, F.; Guan, S.; Sun, J.; Tafsirojjaman, T. Experimental Study and Discrete Analysis of Compressive Properties of Glass Fiber-Reinforced Polymer (GFRP) Bars. Polymers 2023, 15, 2651. [Google Scholar] [CrossRef]
- Prabhakar, M.M.; Rajini, N.; Ayrilmis, N.; Mayandi, K.; Siengchin, S.; Senthilkumar, K.; Karthikeyan, S.; Ismail, S.O. An overview of burst, buckling, durability and corrosion analysis of lightweight FRP composite pipes and their applicability. Compos. Struct. 2019, 230, 111419. [Google Scholar] [CrossRef]
- Tu, J.; Xie, H.; Gao, K. Prediction of the Long-Term Performance and Durability of GFRP Bars under the Combined Effect of a Sustained Load and Severe Environments. Materials 2020, 13, 2341. [Google Scholar] [CrossRef]
- Zhu, J.; Deng, Y.; Chen, P.; Wang, G.; Min, H.; Fang, W. Prediction of Long-Term Tensile Properties of Glass Fiber Reinforced Composites under Acid-Base and Salt Environments. Polymers 2022, 14, 3031. [Google Scholar] [CrossRef]
- Liang, R.; Hota, G. Development and evaluation of load-bearing fiber reinforced polymer composite panel systems with tongue and groove joints. Sustain. Struct. 2021, 1, 000008. [Google Scholar] [CrossRef]
- Al-Zahrani, M.M. Generational Advancements in the Transverse Shear Strength Retention of Glass Fiber-Reinforced Polymer Bars in Alkaline and Acidic Environments. Polymers 2024, 16, 2712. [Google Scholar] [CrossRef]
- Xian, G.; Bai, Y.; Zhou, P.; Wang, J.; Li, C.; Dong, S.; Guo, R.; Tian, J.; Li, J.; Zhong, J.; et al. Long-term properties evolution and life prediction of glass fiber reinforced thermoplastic bending bars exposed in concrete alkaline environment. J. Build. Eng. 2024, 91, 109641. [Google Scholar] [CrossRef]
- Vafadar, A.K.; Bouzid, A.-H.; Ngô, A.D. Analytical modeling of FRP hubbed flanges. Int. J. Press. Vessel. Pip. 2020, 188, 104247. [Google Scholar] [CrossRef]
- Shi, W.; Zhang, Z. Elastostatic properties for flange-bolted joints. Int. J. Press. Vessel. Pip. 2023, 205, 104966. [Google Scholar] [CrossRef]
- Nerilli, F.; Marino, M.; Vairo, G. A Numerical Failure Analysis of Multi-bolted Joints in FRP Laminates Based on Basalt Fibers. Procedia Eng. 2015, 109, 492–506. [Google Scholar] [CrossRef]
- Zhao, D.; Zhang, J.; Ye, Z.; Fei, J.; Li, J. Effects of scale and strain rate on the tensile behaviors of large-rupture-strain fiber-reinforced polymers (LRS-FRP). Constr. Build. Mater. 2023, 380, 131267. [Google Scholar] [CrossRef]
- You, Y.-J.; Park, Y.-H.; Kim, H.-Y.; Park, J.-S. Hybrid effect on tensile properties of FRP rods with various material compositions. Compos. Struct. 2007, 80, 117–122. [Google Scholar] [CrossRef]
- Xiang, S.; Cheng, B.; Wang, J.; Li, D.; Yan, X. Experimental and numerical investigation on failure behavior of hybrid bonded/bolted GFRP single-lap joints under static shear loading. Eng. Fail. Anal. 2024, 158, 107969. [Google Scholar] [CrossRef]
- Xiang, S.; Cheng, B.; Wang, J.; Li, D.; Li, S.; Yan, X. Investigation on fatigue behavior of GFRP hybrid bonded/bolted joint under shear loading based on nondestructive monitoring test and numerical modeling. Eng. Struct. 2024, 308, 117998. [Google Scholar] [CrossRef]
- Feo, L.; Marra, G.; Mosallam, A.S. Stress analysis of multi-bolted joints for FRP pultruded composite structures. Compos. Struct. 2012, 94, 3769–3780. [Google Scholar] [CrossRef]
- Frketic, J.; Dickens, T.; Ramakrishnan, S. Automated manufacturing and processing of fiber-reinforced polymer (FRP) composites: An additive review of contemporary and modern techniques for advanced materials manufacturing. Addit. Manuf. 2017, 14, 69–86. [Google Scholar] [CrossRef]
- Peck, J.A.; Jones, R.A.; Pang, S.-S.; Li, G.; Smith, B.H. UV-cured FRP joint thickness effect on coupled composite pipes. Compos. Struct. 2007, 80, 290–297. [Google Scholar] [CrossRef]
- Zavvar, E.; Henneberg, J.; Soares, C.G. Stress concentration factors in FRP-reinforced tubular DKT joints under axial loads. Mar. Struct. 2023, 90, 103429. [Google Scholar] [CrossRef]
- Cintra, G.G.; Cardoso, D.C.; Vieira, J.D.; Keller, T. Experimental investigation on the moment-rotation performance of pultruded FRP web-flange junctions. Compos. Part B Eng. 2021, 222, 109087. [Google Scholar] [CrossRef]
- Yanes-Armas, S.; de Castro, J.; Keller, T. Rotational stiffness of web-flange junctions of pultruded GFRP decks. Eng. Struct. 2017, 140, 373–389. [Google Scholar] [CrossRef]
- Valdivia, I.; Canales, C.; Tuninetti, V.; Flores, P.; Medina, C. Numerical Prediction of Failure in Unidirectional Fiber Reinforced Composite. Int. J. Appl. Mech. 2021, 13, 2150073. [Google Scholar] [CrossRef]
- Ben Khalifa, A.; Zidi, M.; Abdelwahed, L. Mechanical characterization of glass/vinylester ±55° filament wound pipes by acoustic emission under axial monotonic loading. Comptes Rendus Mec. 2012, 340, 453–460. [Google Scholar] [CrossRef]
- Almeida, J.H.S.; Ribeiro, M.L.; Tita, V.; Faria, H.; Marques, A.T.; Amico, S.C. Progressive failure analysis of filament wound composite tubes under internal pressure. In Proceedings of the International Conference on Composite Materials, Copenhagen, Denmark, 19–24 July 2015. [Google Scholar]



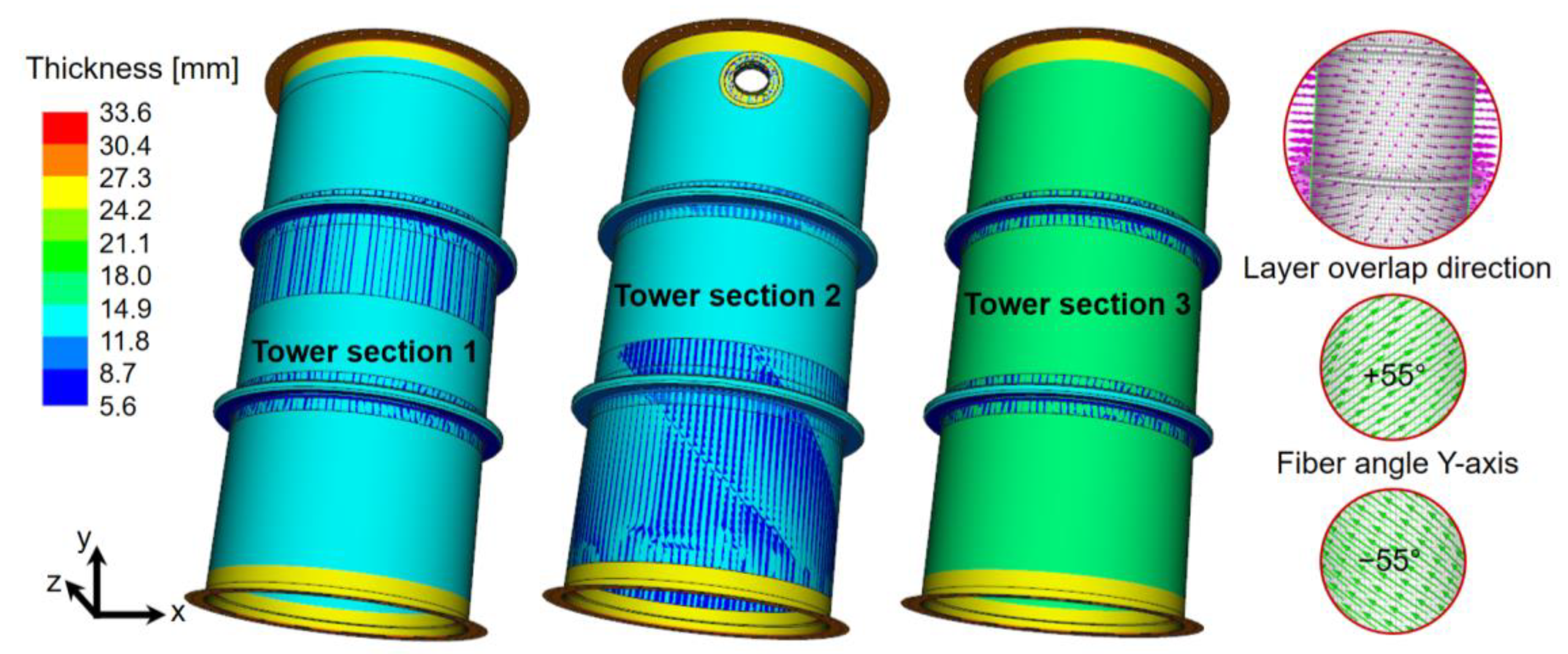




| Section Area | Section (1) | Section (2) | Section (3) | Thickness (mm) |
|---|---|---|---|---|
| Upper and lower flange | 10 MWM | 10 MWM | 10 MWM | 28 |
| Top and bottom trim | 9 MWM | 9 MWM | 9 MWM | 25.2 |
| Tower shell | 5 MWM | 5 MWM | 5 MWM + 2 M | 14 (sections (1) and (2)) 16 (section (3)) |
| Additional layers to the shell | 1 M (from bottom edge to 1258 mm to top edge) | 2 M | 1 | |
| Structural reinforcements: inner radius and joint to 788 mm radius | 2 MWM | 2 MWM | 2 MWM | 5.6 |
| Structural reinforcements: 788 mm radius only | 4 MWM + M | 4 MWM + M | 4 MWM + M | 12.2 |
| Structural band | MWM | - | - | 2.8 |
| Shell over-thickness | - | 1 M | 2 M | 1 M |
| Pass-through nozzle | - | 7 MWM | - | 19.6 |
| Inner nozzle | - | 3 M | - | 8.4 |
| External splice | - | 9 MWM | - | 25.2 |
| Internal splices | - | 4 M | - | 11.2 |
| GFRP Fiber Type | ||||
|---|---|---|---|---|
| Property | Direction | Units | Woven Roving | Mat |
| Density | [kg/m3] | 1850 | 1508.6 | |
| Tensile strength | x | [MPa] | 487.1 | 106.03 |
| y | 487.1 | 106.03 | ||
| z | 7.7 | 31 | ||
| Compressive strength | x | −292.3 | −141.3 | |
| y | −292.3 | −141.3 | ||
| z | −41.2 | −100 | ||
| Shear strength | xy | 49.6 | 71.3 | |
| yz | 35 | 35 | ||
| xz | 35 | 35 | ||
| Young’s modulus | x | 24,355 | 7069 | |
| y | 24,355 | 7069 | ||
| z | 5154.6 | 5268 | ||
| Shear modulus | xy | 1895 | 2663 | |
| yz | 1532 | 2152 | ||
| xz | 1532 | 2152 | ||
| Poisson’s ratio | xy | Unitless | 0.3011 | 0.3269 |
| yz | 0.28 | 0.28 | ||
| xz | 0.28 | 0.28 | ||
| Refinement Zone | Sizing (mm) |
|---|---|
| Hole circle | 5 |
| Fillet | 9 |
| Ring and shell joints | 30 & 30 |
| Shell | 40 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tuninetti, V.; Mariqueo, M. Performance of Combined Woven Roving and Mat Glass-Fiber Reinforced Polymer Composites Under Absorption Tower Lifting Loads. Polymers 2024, 16, 2937. https://doi.org/10.3390/polym16202937
Tuninetti V, Mariqueo M. Performance of Combined Woven Roving and Mat Glass-Fiber Reinforced Polymer Composites Under Absorption Tower Lifting Loads. Polymers. 2024; 16(20):2937. https://doi.org/10.3390/polym16202937
Chicago/Turabian StyleTuninetti, Víctor, and Matías Mariqueo. 2024. "Performance of Combined Woven Roving and Mat Glass-Fiber Reinforced Polymer Composites Under Absorption Tower Lifting Loads" Polymers 16, no. 20: 2937. https://doi.org/10.3390/polym16202937
APA StyleTuninetti, V., & Mariqueo, M. (2024). Performance of Combined Woven Roving and Mat Glass-Fiber Reinforced Polymer Composites Under Absorption Tower Lifting Loads. Polymers, 16(20), 2937. https://doi.org/10.3390/polym16202937





