Effects of Xerophytic Vegetation-Salix on Soil Water Redistribution in Semiarid Region
Abstract
:1. Introduction
2. Methods and Materials
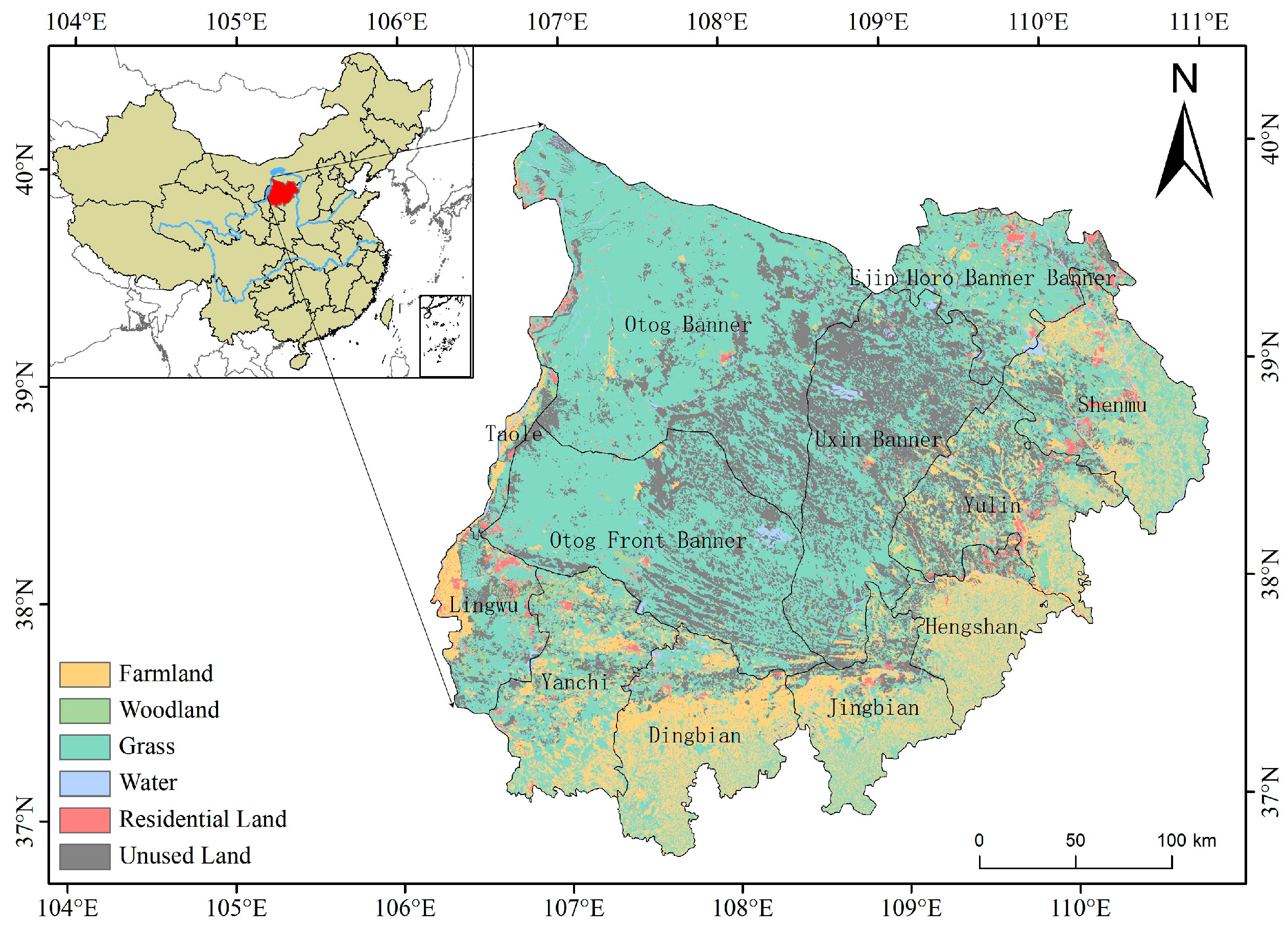
2.1. Study Area
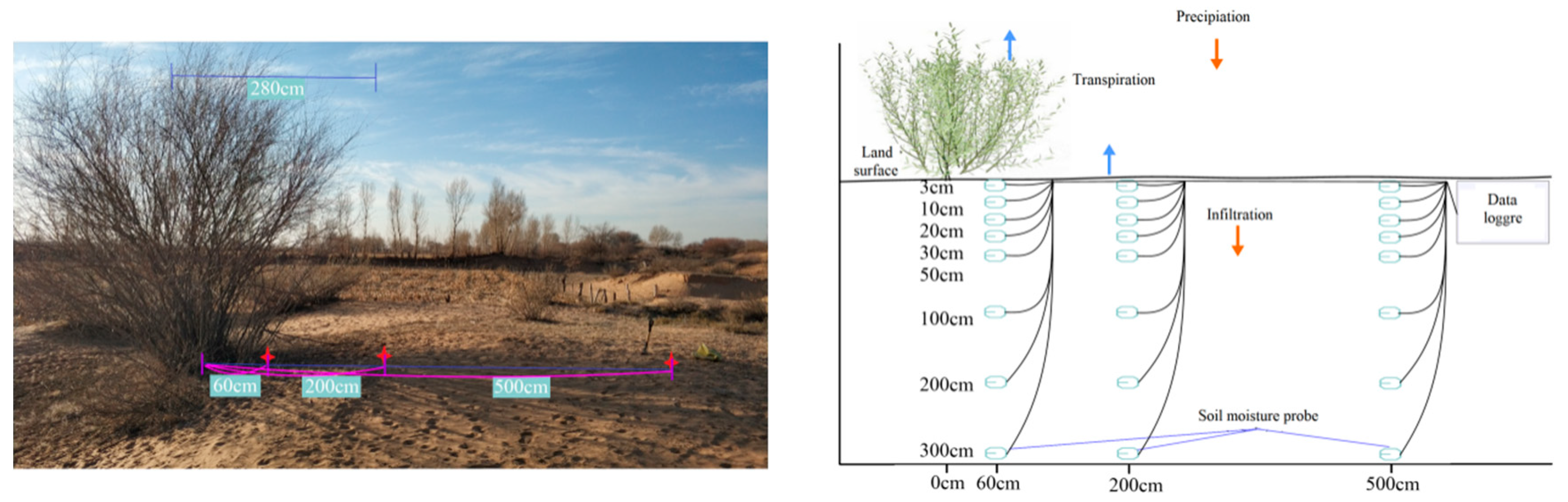
2.2. Field Monitoring Experiment
2.3. Determination of Soil Hydraulic Parameters
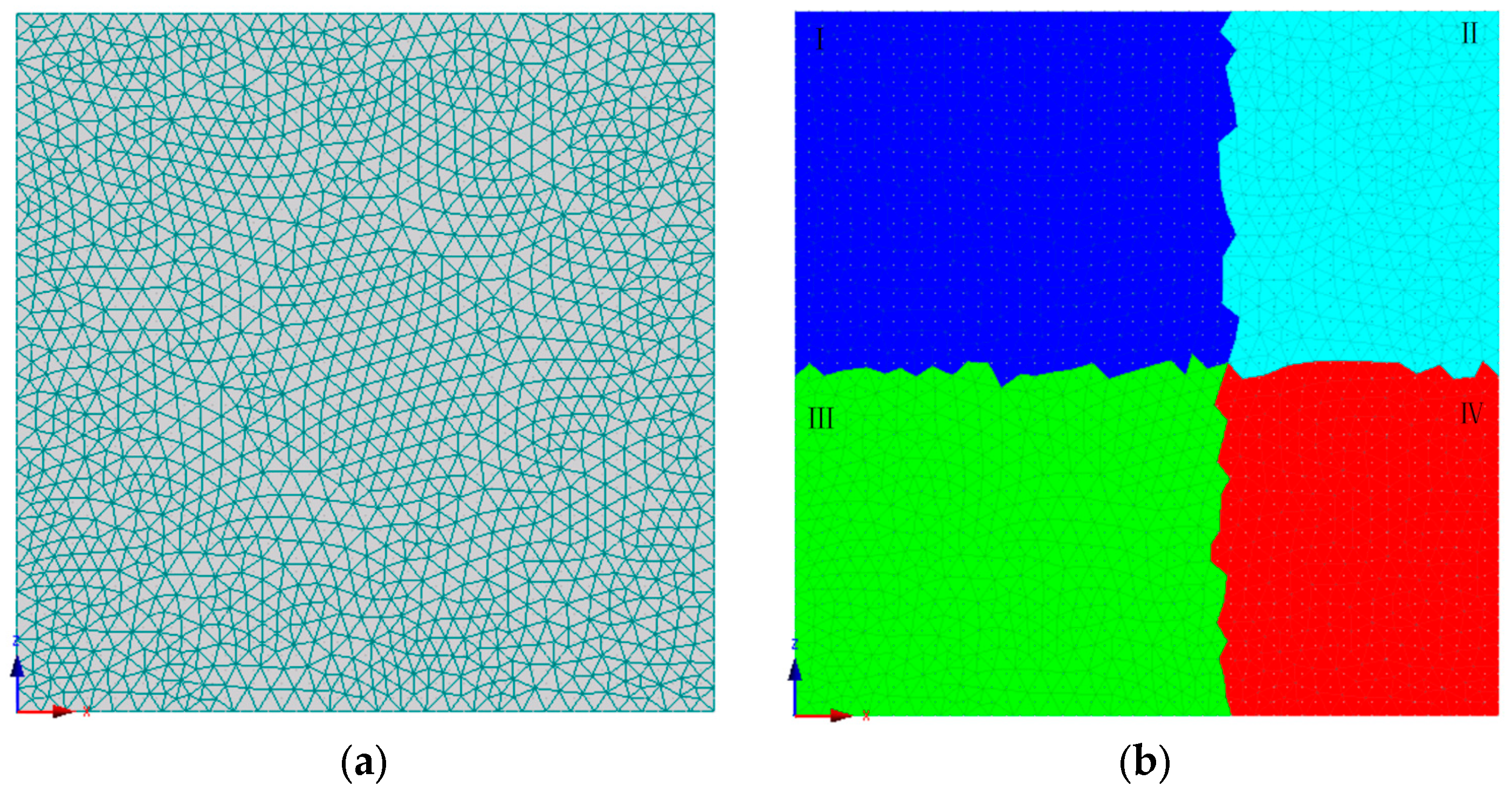
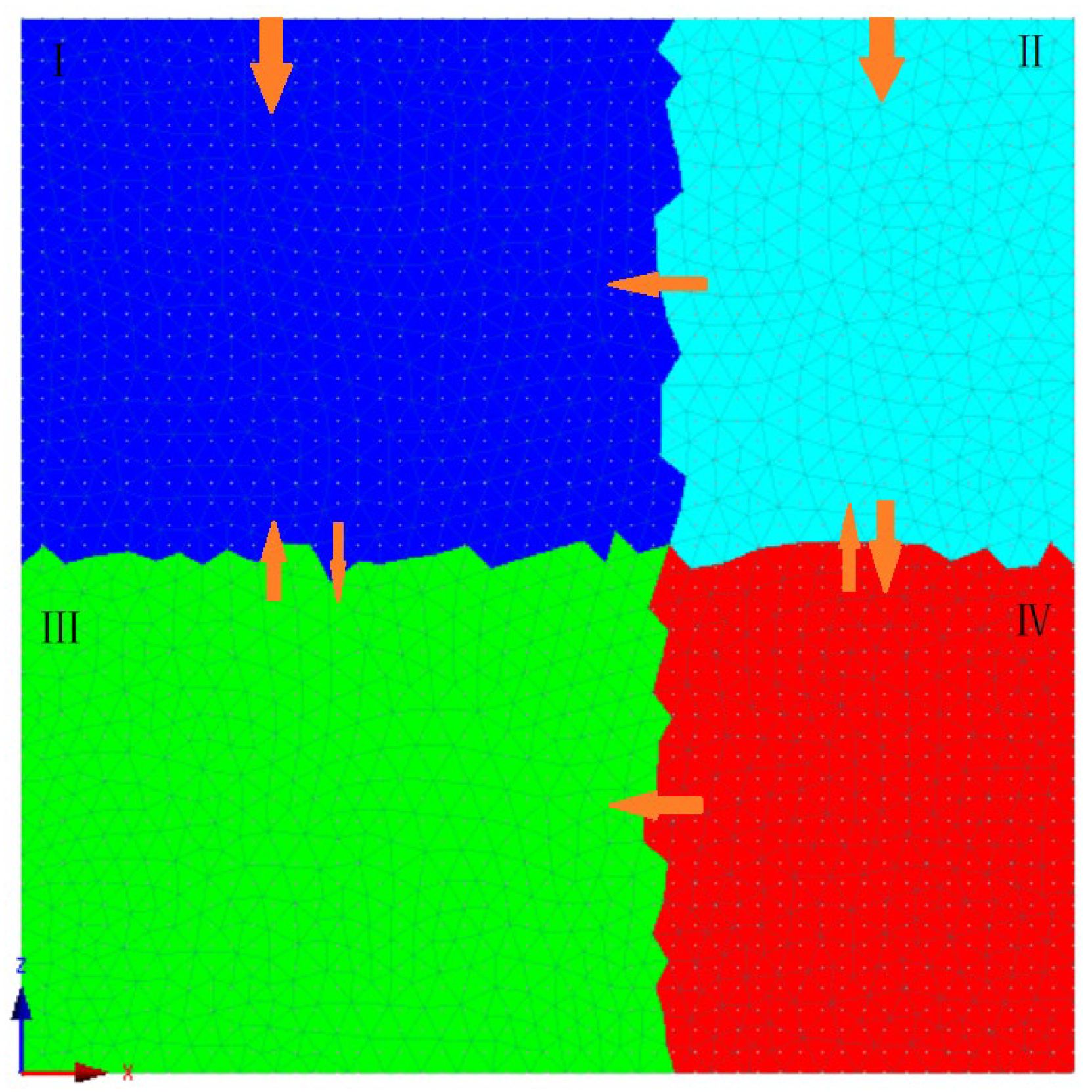
2.4. Modeling
2.5. Water Budget
3. Results and Discussion
3.1. Identification of the Model
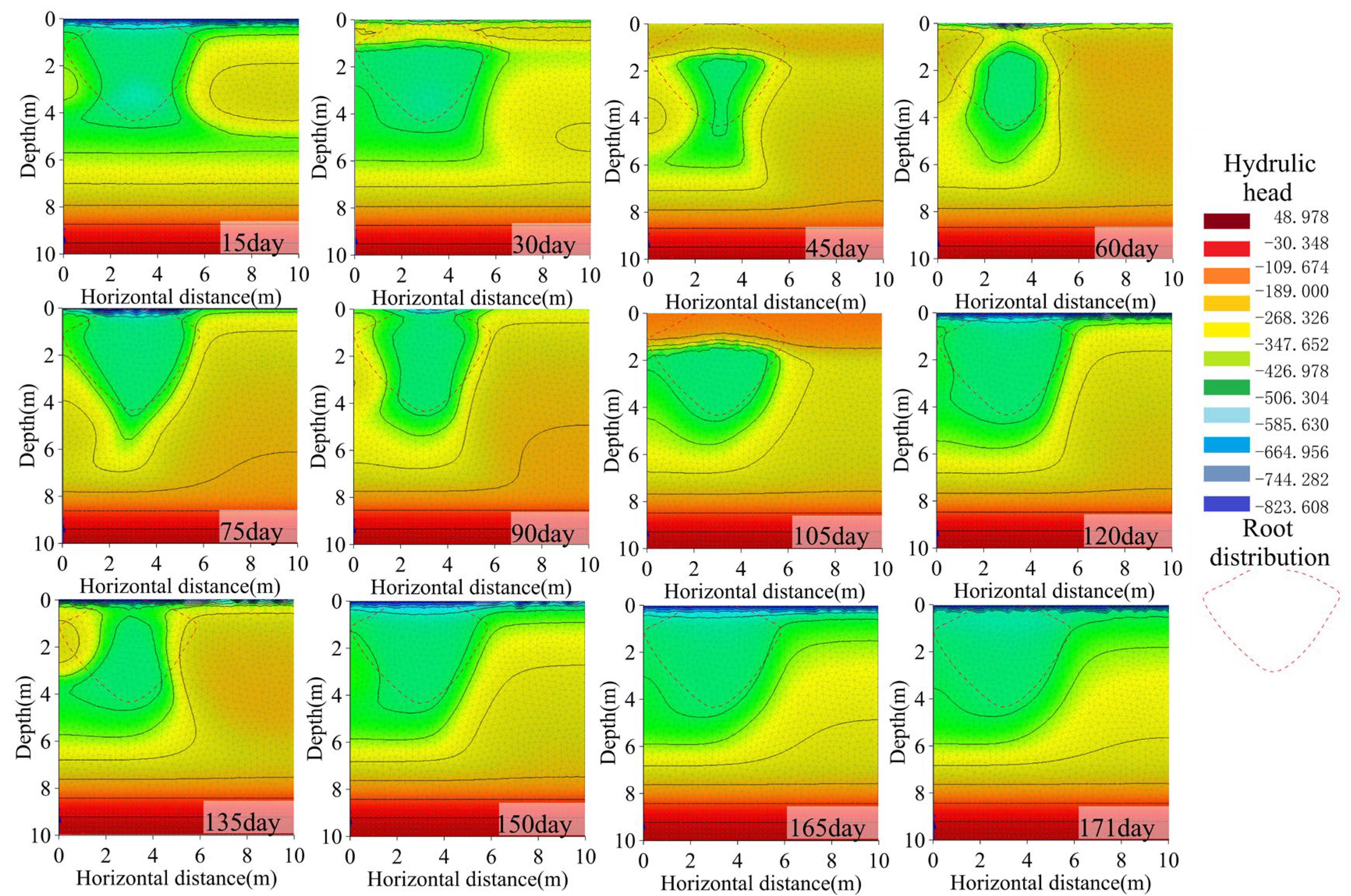
3.2. Distribution of Soil Water in Bare Land and Vegetated Land
3.3. Effects of Root Water Uptake on Water Budget
3.4. Reasonable Estimation of Vegetation Replanting Interval
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Philip, J.R. Plant water relations: Some physical aspects. Annu. Rev. Plant Physiol. 1966, 17, 245–268. [Google Scholar] [CrossRef]
- Kang, S.; Liu, X.; Xiong, Y. Soil-Plant-Atmosphere Continuum Theory; Water Resources and Electric Power Press: Beijing, China, 1994. [Google Scholar]
- Yang, J.; Li, B.; Li, Y.; Ma, Y.; Hong, B. Preliminary study on the effect of diving on SPAC system in shallow groundwater deep area. J. Hydraul. Eng. 1999, 7, 27–32. (In Chinese) [Google Scholar] [CrossRef]
- Oki, T.; Kanae, S. Global hydrological cycles and world water resources. Science 2006, 313, 1068–1072. [Google Scholar] [CrossRef] [PubMed]
- Chen, L.; Huang, Z.; Gong, J.; Fu, B.; Huang, Y. The effect of land cover/vegetation on soil water dynamic in the hilly area of the loess plateau, China. Catena 2007, 70, 200–208. [Google Scholar] [CrossRef]
- Yu, X.; Huang, Y.; Li, E.; Li, X.; Guo, W. Effects of rainfall and vegetation to soil water input and output processes in the mu us sandy land, northwest China. Catena 2018, 161, 96–103. [Google Scholar] [CrossRef]
- Jiménez-Martínez, J.; Skaggs, T.; van Genuchten, M.; Candela, L. A root zone modelling approach to estimating groundwater recharge from irrigated areas. J. Hydrol. 2009, 367, 138–149. [Google Scholar] [CrossRef]
- Zhu, Y.; Ren, L.; Skaggs, T.H.; Lü, H.; Yu, Z.; Wu, Y.; Fang, X. Simulation of Populus euphratica root uptake of groundwater in an arid woodland of the ejina basin, China. Hydrol. Process. 2010, 23, 2460–2469. [Google Scholar] [CrossRef]
- Baroni, G.; Ortuani, B.; Facchi, A.; Gandolfi, C. The role of vegetation and soil properties on the spatio-temporal variability of the surface soil moisture in a maize-cropped field. J. Hydrol. 2013, 489, 148–159. [Google Scholar] [CrossRef]
- Zhao, Y.; Zhou, Y.; Wang, H. Spatial heterogeneity of soil water under introduction to desert steppe shrub in eastern Ningxia. Chin. J. Appl. Ecol. 2018, 29, 3577–3586. (In Chinese) [Google Scholar] [CrossRef]
- Liu, B.; Ren, J.; Shao, M.; Jia, X. Study on soil desiccation process of artificial shrub vegetation in northern Loess Plateau. Acta Ecol. Sin. 2020, 40, 3795–3803. [Google Scholar] [CrossRef]
- Li, X.; Zhang, Z.; Liu, Y.; Li, X.; Yang, H. Ecological Hydrology Basis of Ecological Reconstruction and Restoration in Sandy Areas of China; Science Press: Beijing, China, 2016. [Google Scholar]
- Huang, T.; Pang, Z. Estimating groundwater recharge following land-use change using chloride mass balance of soil profiles: A case study at Guyuan and Xifeng in the Loess Plateau of China. Hydrogeol. J. 2010, 19, 177–186. [Google Scholar] [CrossRef]
- Gates, J.B.; Scanlon, B.R.; Mu, X.; Zhang, L. Impacts of soil conservation on groundwater recharge in the semi-arid Loess Plateau, China. Hydrogeol. J. 2011, 19, 865–875. [Google Scholar] [CrossRef]
- Allen, A.; Chapman, D. Impacts of afforestation on groundwater resources and quality. Hydrogeol. J. 2001, 9, 390–400. [Google Scholar] [CrossRef]
- Gao, Z.; Zhang, L.; Cheng, L.; Zhang, X.; Cowan, T.; Cai, W.; Brutsaert, W. Groundwater storage trends in the Loess Plateau of China estimated from streamflow records. J. Hydrol. 2015, 530, 281–290. [Google Scholar] [CrossRef]
- Banks, E.W.; Brunner, P.; Simmons, C.T. Vegetation controls on variably saturated processes between surface water and groundwater and their impact on the state of connection. Water Resour. Res. 2011, 47, 178–186. [Google Scholar] [CrossRef]
- Zhao, M.; Wang, W.; Wang, Z.; Chen, L.; Ma, Z.; Wang, Q. Water use of Salix in the variably unsaturated zone of a semiarid desert region based on in-situ observation. J. Hydrol. 2020, 591, 125579. [Google Scholar] [CrossRef]
- Allison, G.B.; Gee, G.W.; Tyler, S.W. Vadose-zone techniques for estimating groundwater recharge in arid and semiarid regions. Soil Sci. Soc. Am. J. 1994, 58, 6–14. [Google Scholar] [CrossRef]
- Gee, G.W.; Hillel, D. Groundwater recharge in arid regions: Review and critique of estimation methods. Hydrol. Process. 1988, 2, 255–266. [Google Scholar] [CrossRef]
- Yang, Y.; Sun, H.; Hu, K.; Geng, H. Soil water characteristics of slope land with different vegetation types in water source area of Middle Route of South-to-North Water Transfer: A case study of Zougou small watershed in Ningshan County, southern Slope of Qinling Mountains. Resour. Environ. Yangtze Basin 2008, 17, 212–216. (In Chinese) [Google Scholar] [CrossRef]
- Sun, Z.; Zhou, Q.; Luo, Y.; Wei, X.; Yu, J. Effects of vegetation coverage on soil moisture in yellow soil runoff plot. J. Water Resour. Water Eng. 2019, 30, 39–46. (In Chinese) [Google Scholar] [CrossRef]
- Fries, A.; Silva, K.; Pucha-Cofrep, F.; Oñate-Valdivieso, F.; Ochoa-Cueva, P. Water Balance and Soil Moisture Deficit of Different Vegetation Units under Semiarid Conditions in the Andes of Southern Ecuador. Climate 2020, 8, 30–38. [Google Scholar] [CrossRef]
- Farley, K.A.; Jobbágy, E.G.; Jackson, R.B. Effects of afforestation on water yield: A global synthesis with implications for policy. Glob. Chang. Biol. 2005, 11, 1565–1576. [Google Scholar] [CrossRef]
- Xue, Y.; Jin, X.; Zhu, X. Variation characteristics of evapotranspiration and verification of water balance method in Ningxia along the Yellow River Economic Zone. Hydrogeol. Eng. Geol. 2017, 44, 27–32. [Google Scholar] [CrossRef]
- Fang, W.; Lu, N.; Liu, J.; Jiao, L.; Zhang, Y.; Wang, M.; Fu, B. Canopy transpiration and stand water balance between two contrasting hydrological years in three typical shrub communities on the semiarid Loess Plateau of China. Ecohydrology 2019, 12, e2064. [Google Scholar] [CrossRef]
- Launiainen, S.; Guan, M.; Salmivaara, A.; Kieloaho, A.-J. Modeling boreal forest evapotranspiration and water balance at stand and catchment scales: A spatial approach. Hydrol. Earth Syst. Sci. 2019, 23, 3457–3480. [Google Scholar] [CrossRef]
- Zhang, C. Hydrodynamic and thermodynamic processes during evaporation from bare soils. Evaporation 2014. [Google Scholar] [CrossRef]
- Gerten, D.; Schaphoff, S.; Haberlandt, U.; Lucht, W.; Sitch, S. Terrestrial vegetation and water balance—Hydrological evaluation of a dynamic global vegetation model. J. Hydrol. 2003, 286, 249–270. [Google Scholar] [CrossRef]
- Granier, A.; Biron, P.; Lemoine, D. Water balance, transpiration and canopy conductance in two beech stands. Agric. For. Meteorol. 2000, 100, 291–308. [Google Scholar] [CrossRef]
- Li, H.; Shen, W.; Zou, C.; Jiang, J.; Fu, L.; She, G. Spatio-temporal variability of soil moisture and its effect on vegetation in a desertified aeolian riparian ecotone on the Tibetan Plateau, China. J. Hydrol. 2013, 479, 215–225. [Google Scholar] [CrossRef]
- Ge, J.; Pitman, A.J.; Guo, W.; Zan, B.; Fu, C. Impact of revegetation of the Loess Plateau of China on the regional growing season water balance. Hydrol. Earth Syst. Sci. 2020, 24, 515–533. [Google Scholar] [CrossRef]
- Wang, L.; Zhang, Q.; Bai, L.; Ma, A.; Zhang, H.; Li, L.; Zhang, J.; Fu, G.; Dong, Q. Effects of three artificial vegetation types on soil particle composition and carbon sequestration in Mu Us Sandy Land. Res. Soil Water Conserv. 2020, 27, 88–94. (In Chinese) [Google Scholar] [CrossRef]
- Jian, S.; Zhao, C.; Fang, S.; Yu, K. Effects of different vegetation restoration on soil water storage and water balance in the Chinese Loess Plateau. Agric. For. Meteorol. 2015, 206, 85–96. [Google Scholar] [CrossRef]
- Zhang, Z.; Wang, W.; Gong, C.; Zhao, M.; Franssen, H.-J.H.; Brunner, P. Salix psammophila afforestations can cause a decline of the water table, prevent groundwater recharge and reduce effective infiltration. Sci. Total. Environ. 2021, 780, 146336. [Google Scholar] [CrossRef]
- Wang, W.; Zhang, Z.; Yin, L.; Duan, L.; Huang, J. Topical Collection: Groundwater recharge and discharge in arid and semi-arid areas of China. Hydrogeol. J. 2021, 29, 521–524. [Google Scholar] [CrossRef]
- Moro, M.; Pugnaire, F.; Haase, P.; Puigdefábregas, J. Mechanisms of interaction between a leguminous shrub and its understorey in a semi-arid environment. Ecography 1997, 20, 175–184. [Google Scholar] [CrossRef]
- Madrigal, J.; García-Rodriguez, J.A.; Julian, R.; Puerto, A.; Fernández-Santos, B. Exploring the influence of shrubs on herbaceous communities in a Mediterranean climatic context of two spatial scales. Plant Ecol. 2008, 195, 225–234. [Google Scholar] [CrossRef]
- Zuo, X.; Zhao, H.; Zhao, X.; Zhang, T.; Guo, Y.; Wang, S.; Drake, S. Spatial pattern and heterogeneity of soil properties in sand dunes under grazing and restoration in Horqin Sandy Land, northern China. Soil Till. Res. 2008, 99, 202–212. [Google Scholar] [CrossRef]
- Mauchamp, A.; Janeau, J. Water funnelling by the crown of Flourensia cernua, a Chihuahuan Desert shrub. J. Arid. Environ. 1993, 25, 299–306. [Google Scholar] [CrossRef]
- Bhark, E.W.; Small, E.E. Association between plant canopies and the spatial patterns of infiltration in shrubland and grassland of the chihuahuan desert, New Mexico. Ecosystems 2003, 6, 185–196. [Google Scholar] [CrossRef]
- Rietkerk, M.; van de Koppel, J. Regular pattern formation in real ecosystems. Trends Ecol. Evol. 2008, 23, 169–175. [Google Scholar] [CrossRef]
- Dunkerley, D. Measuring interception loss and canopy storage in dryland vegetation: A brief review and evaluation of available research strategies. Hydrol. Process. 2015, 14, 669–678. [Google Scholar] [CrossRef]
- Zhao, C.; Zhang, Y.; Qin, S.; Lai, Z.; Liu, J.; Fa, K. NPP and its distribution pattern of three typical psammophytic shrubs. Beijing For. Univ. 2014, 36, 62–67. (In Chinese) [Google Scholar] [CrossRef]
- Liu, S.; Xu, G.; Li, Y.; Wu, X.; Liu, J.; Mi, X. Response of five sandy shrub species to groundwater depth change. Acta Ecol. Sin. 2021, 41, 615–625. (In Chinese) [Google Scholar] [CrossRef]



| Grain Composition | Dry Density (g·cm−3) | Porosity (cm3·cm−3) | Name | ||
|---|---|---|---|---|---|
| 0.5–0.25 | 0.25–0.075 | 0.075–0.05 | |||
| 5.7 | 88.7 | 5.6 | 1.45 | 0.37 | silty-fine sand |
| Item | θr [cm3·cm−3] | θs [cm3·cm−3] | α [cm−1] | n [-] | Ks [cm·day−1] | l [-] |
|---|---|---|---|---|---|---|
| Aeolian sand | 0.023 | 0.32 | 0.036 | 2.56 | 560 | 0.5 |
| Soil Layers | Depth (cm) | α [cm−1] | n [-] | Ks [cm·day−1] |
|---|---|---|---|---|
| 1 | 0–60 | 0.0110 | 1.440 | 450.0 |
| 2 | 60–120 | 0.0366 | 1.611 | 660.0 |
| 3 | 120–200 | 0.0199 | 3.230 | 760.0 |
| 4 | 200–280 | 0.1551 | 4.617 | 410.0 |
| 5 | 280–400 | 0.0170 | 2.800 | 760.0 |
| Depth (cm) | ||||||||
|---|---|---|---|---|---|---|---|---|
| 3 | 10 | 20 | 30 | 50 | 100 | 200 | 300 | |
| ME | 0.017 | 0.013 | 0.011 | 0.012 | 0.009 | 0.018 | 0.014 | 0.007 |
| RMSE | 0.022 | 0.016 | 0.014 | 0.014 | 0.011 | 0.020 | 0.019 | 0.009 |
| MAE | 0.235 | 0.111 | 0.109 | 0.103 | 0.075 | 0.173 | 0.100 | 0.026 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, M.; Wang, Q. Effects of Xerophytic Vegetation-Salix on Soil Water Redistribution in Semiarid Region. Agronomy 2024, 14, 2200. https://doi.org/10.3390/agronomy14102200
Zhao M, Wang Q. Effects of Xerophytic Vegetation-Salix on Soil Water Redistribution in Semiarid Region. Agronomy. 2024; 14(10):2200. https://doi.org/10.3390/agronomy14102200
Chicago/Turabian StyleZhao, Ming, and Qiangmin Wang. 2024. "Effects of Xerophytic Vegetation-Salix on Soil Water Redistribution in Semiarid Region" Agronomy 14, no. 10: 2200. https://doi.org/10.3390/agronomy14102200
APA StyleZhao, M., & Wang, Q. (2024). Effects of Xerophytic Vegetation-Salix on Soil Water Redistribution in Semiarid Region. Agronomy, 14(10), 2200. https://doi.org/10.3390/agronomy14102200





