Gravity Wave Propagation from the Stratosphere into the Mesosphere Studied with Lidar, Meteor Radar, and TIMED/SABER
Abstract
:1. Introduction
2. Instrumentation and Observation Results
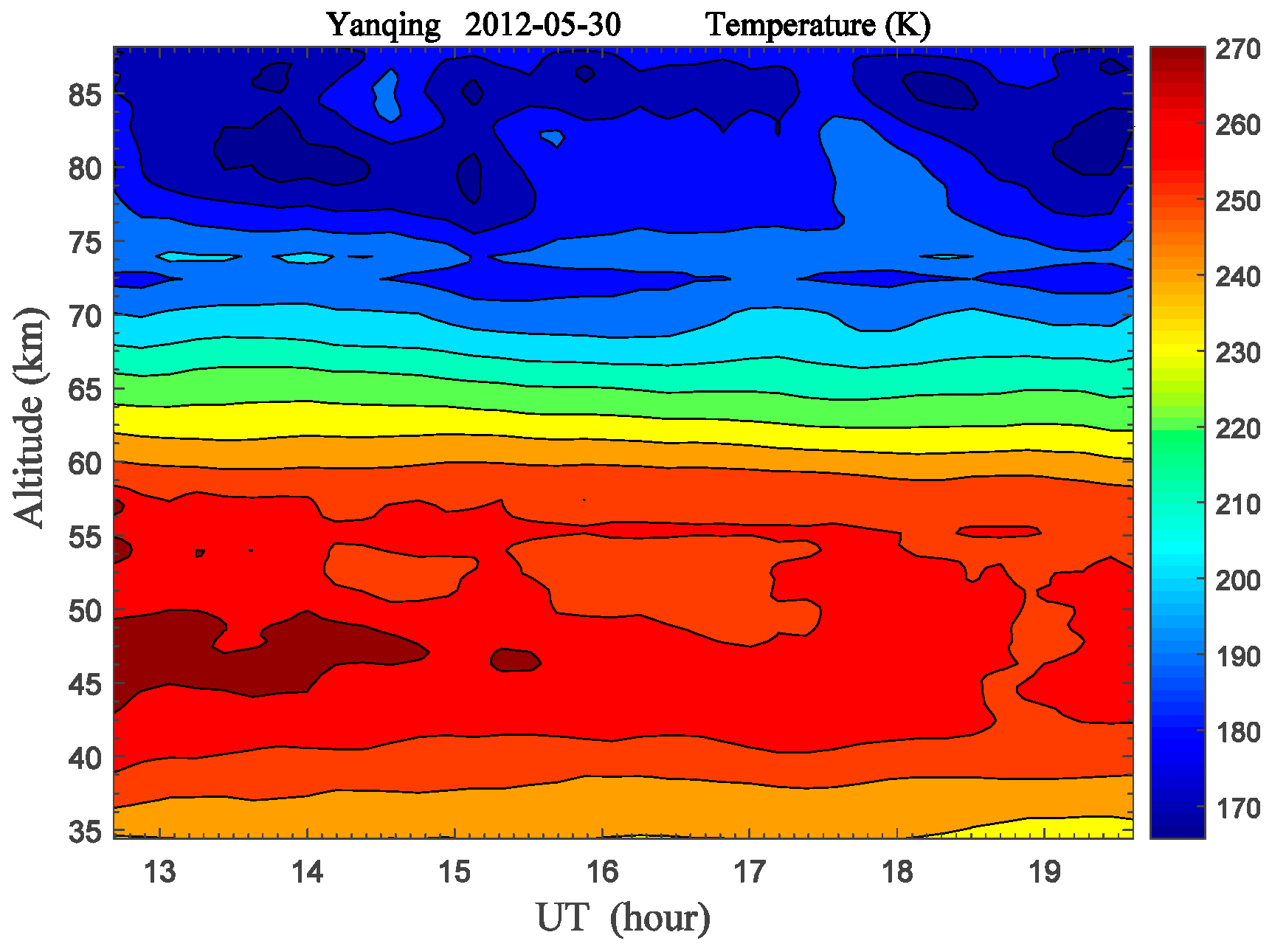
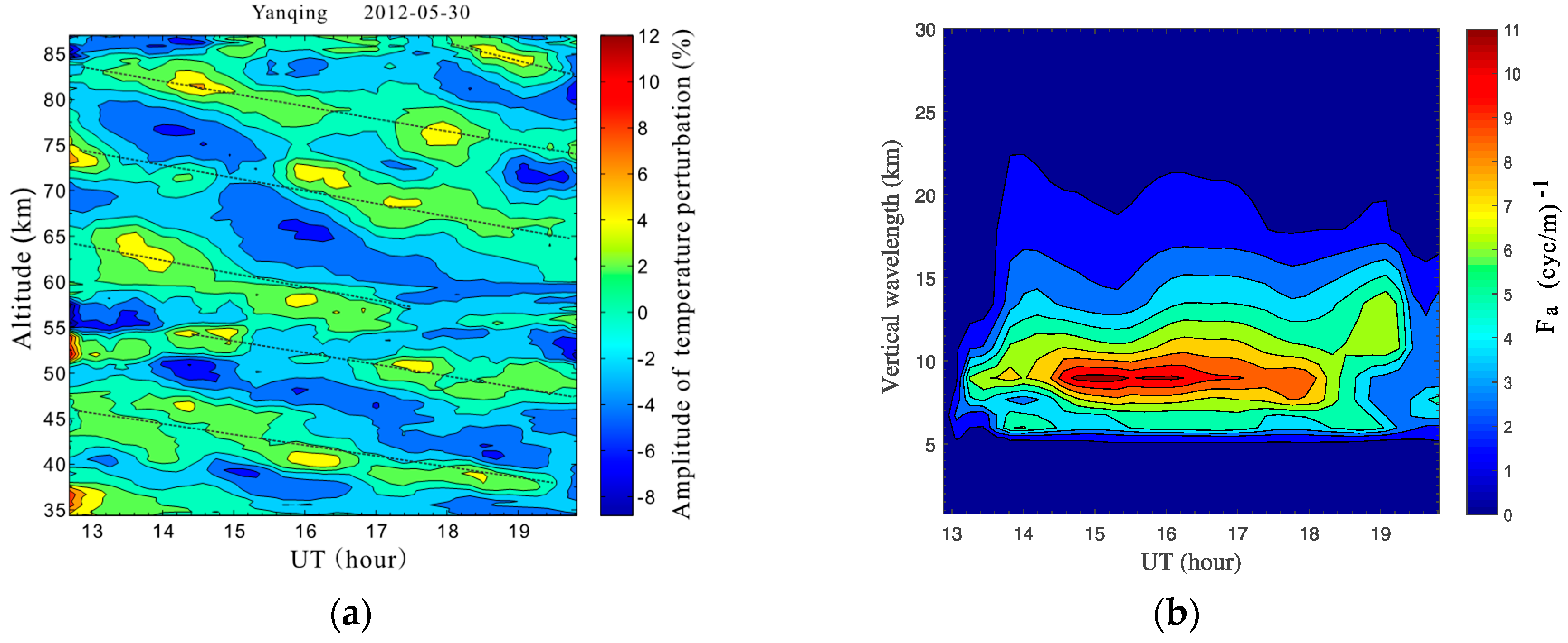
2.1. Lidar-Observed Atmospheric Temperature Structure and Wave Propagation
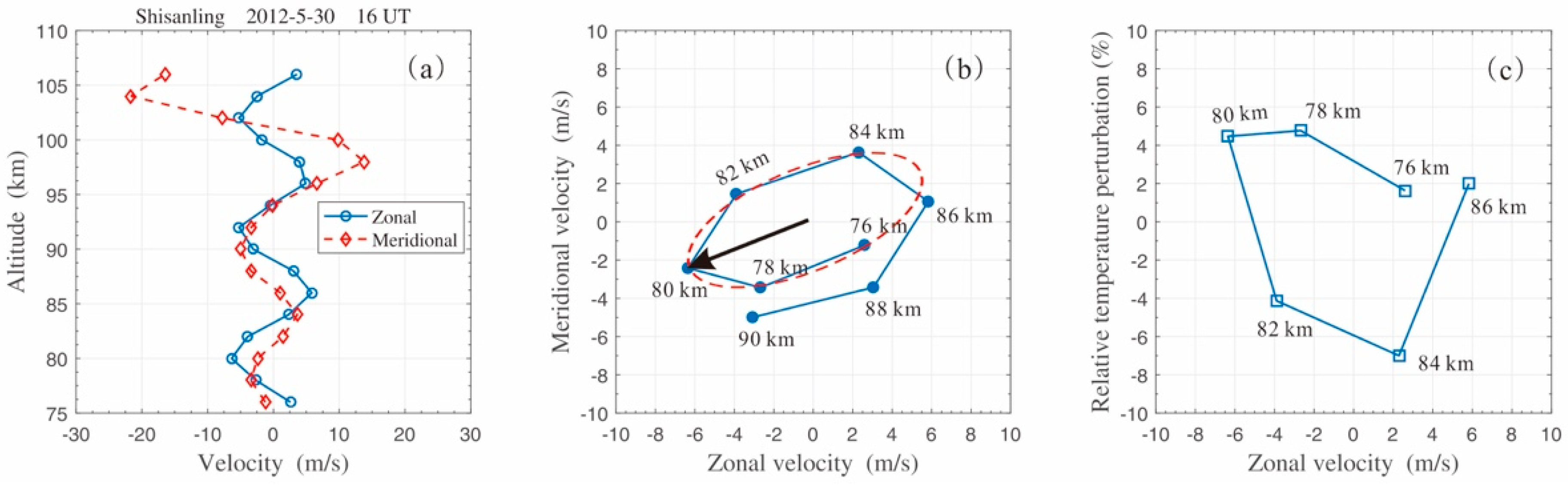
2.2. Background Wind and Wave Propagation Observed by Meteor Radar
2.3. Wave Structures Observed by TIMED/SABER
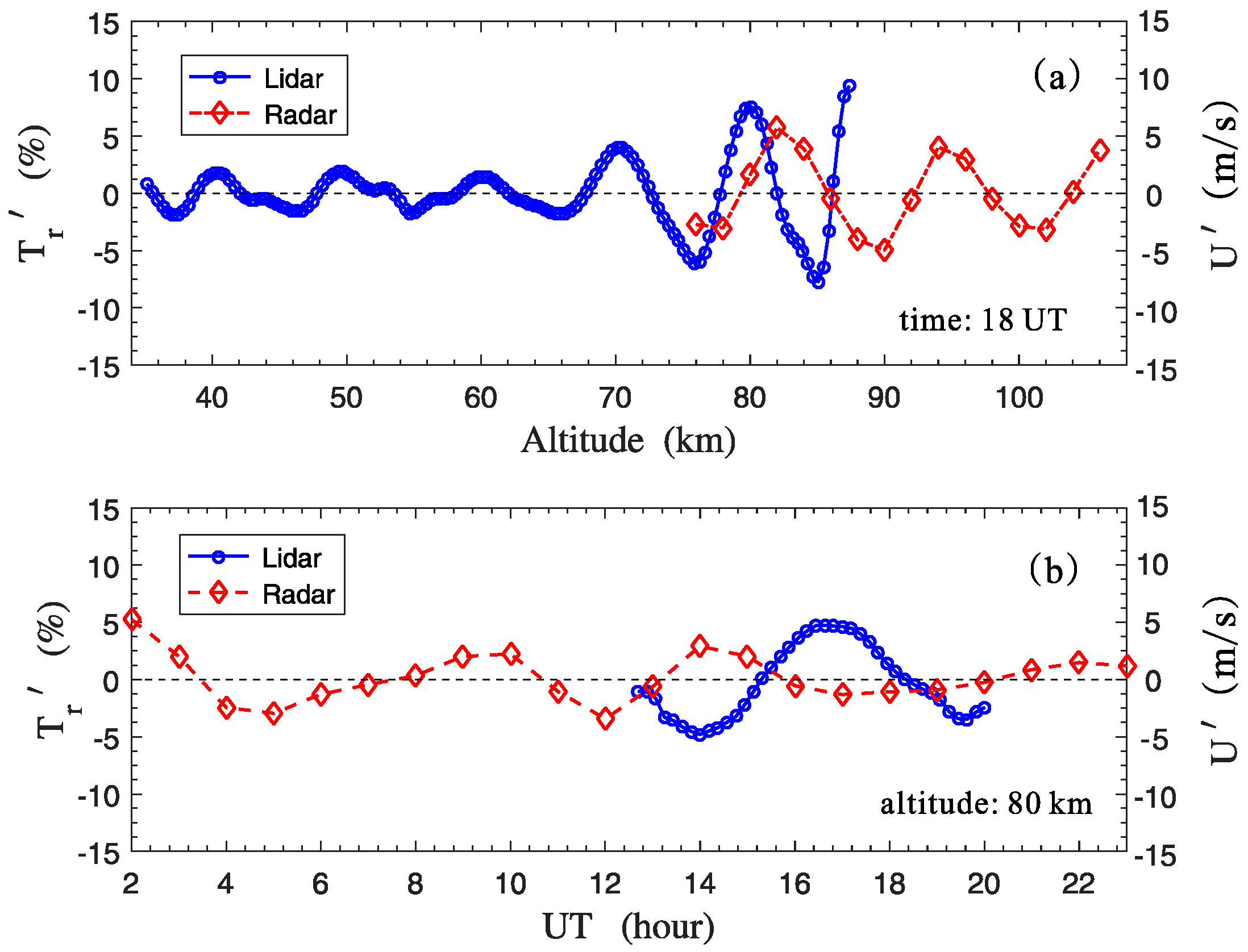
3. Comparisons and Discussion
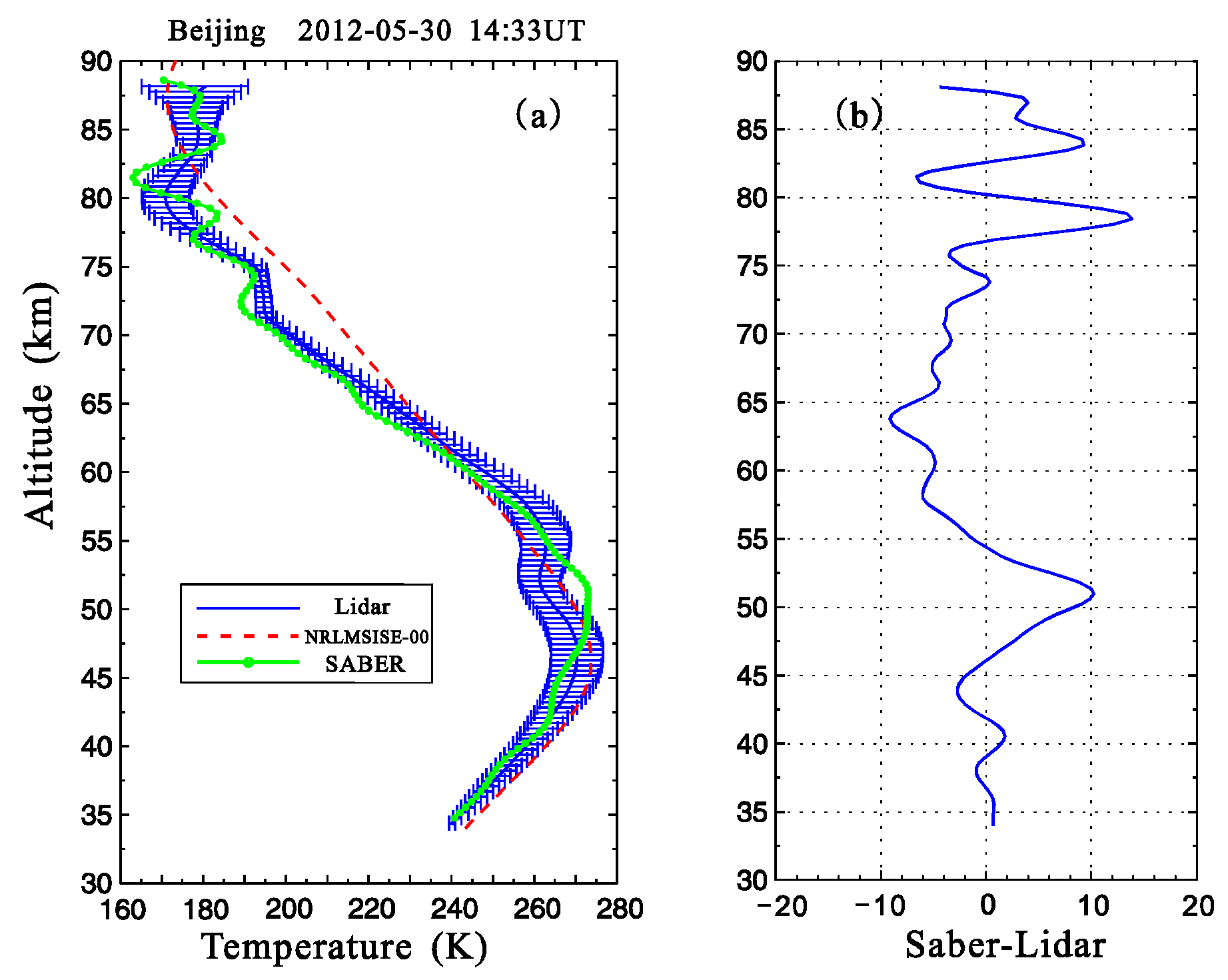
3.1. Temperature Measurement Comparison
- The atmospheric temperature profile was measured by lidar at Yanqing (40.5° N, 116° E) using a vertical detection method, and the temperature profile was measured by SABER using the limb-scanning measurement technique when the TIMED satellite was over Beijing (40.406° N, 105.605° E) at 1433 UT; and
- There are some temporal differences between the two measurements. To improve the accuracy, the sampling period was set to 25 min for the lidar measurement, but it was 36 s for the TIMED/SABER measurement.
3.2. Phase Relations between the Temperature and Wind Perturbations
- The spatial and temporal resolution of meteor radar measurements are 2 km and 1 h, respectively. Too much uncertainty probably exists in the wind data;
- The horizontal distance is ~40 km between the two observing locations, and there were some objective phase differences in the observed wave propagations.
3.3. Atmospheric Gravity Wave Properties
3.3.1. Wavelengths
3.3.2. Wave Periods and Propagation Direction
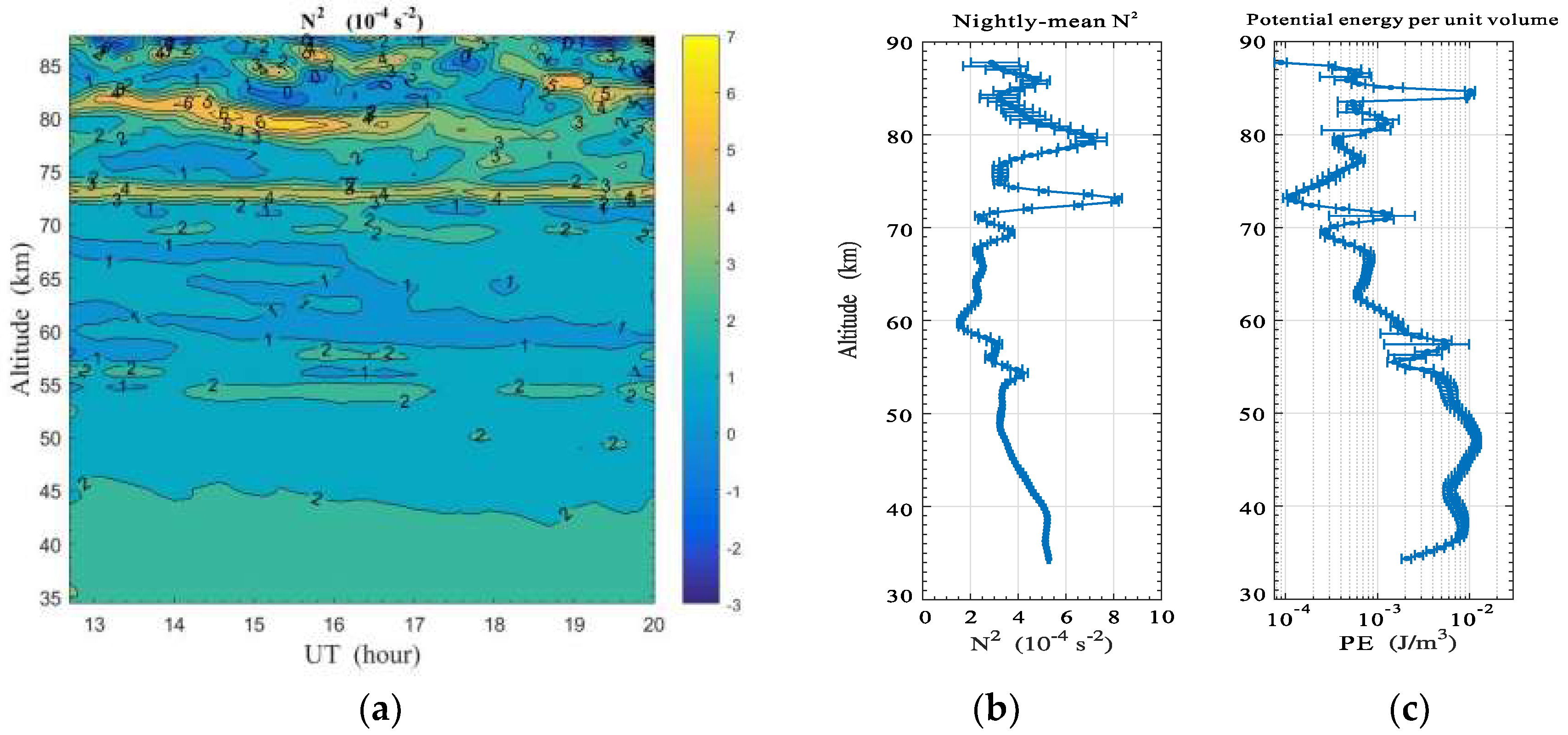
3.3.3. Static Stability
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Fritts, D.C.; Alexander, M.J. Gravity wave dynamics and effects in the middle atmosphere. Rev. Geophys. 2003, 41, 1003. [Google Scholar] [CrossRef]
- Hines, C.O. Internal atmospheric gravity waves at ionospheric heights. Can. J. Phys. 1960, 38, 1441–1481. [Google Scholar] [CrossRef]
- Hines, C.O. The saturation of gravity waves in the middle atmosphere, II. Development of Doppler–spread theory. J. Atmos. Sci. 1991, 48, 136Cl379. [Google Scholar] [CrossRef]
- Gardner, C.S. Diffusive filtering theory of gravity wave spectra in the atmosphere. J. Geophys. Res. 1994, 99, 20601–20622. [Google Scholar] [CrossRef]
- Yang, G.; Clemesha, B.; Batista, P.; Simonich, D. Gravity wave parameters and their seasonal variations derived from Na lidar observations at 23oS. J. Geophys. Res. 2006, 111, D21107. [Google Scholar] [CrossRef]
- Chanin, M.L.; Hauchecorne, A. Lidar observation of gravity and tidal waves in the stratosphere and mesosphere. J. Geophys. Res. 1981, 86, 9715–9721. [Google Scholar] [CrossRef]
- Sivakumar, V.; Rao, P.B.; Bencherif, H. Lidar observations of middle atmospheric gravity wave activity over a low-latitude site (Gadanki, 13.5° N, 79.2° E). Ann. Geophys. 2006, 24, 823–834. [Google Scholar] [CrossRef] [Green Version]
- Li, T.; She, C.-Y.; Liu, H.-L.; Leblanc, T.; McDermid, I.S. Sodium lidar-observed strong inertia-gravity wave activities in the mesopause region over Fort Collins, Colorado (41° N, 105° W). J. Geophys. Res. 2007, 112, D22104. [Google Scholar] [CrossRef]
- Gong, S.; Yang, G.; Dou, X.; Xu, J.; Chen, C.; Gong, S. Statistical study of atmospheric gravity waves in the mesopause region observed by a lidar chain in eastern China. J. Geophys. Res. Atmos. 2015, 120, 7619–7634. [Google Scholar] [CrossRef] [Green Version]
- Suzuki, S.; Nakamura, T.; Ejiri, M.K.; Tsutsumi, M.; Shiokawa, K.; Kawahara, T.D. Simultaneous airglow, lidar, and radar measurements of mesospheric gravity waves over Japan. J. Geophys. Res. 2010, 115, D24113. [Google Scholar] [CrossRef]
- Li, Q.; Xu, J.; Yue, J.; Yuan, W.; Liu, X. Statistical characteristics of gravity wave activities observed by an OH airglow imager at Xinglong, in northern China. Ann. Geophys. 2011, 29, 1401–1410. [Google Scholar] [CrossRef] [Green Version]
- Gavrilov, N.M.; Fukao, S.; Nakamura, T.; Tsuda, T. Statistical analysis of gravity waves observed with the middle and upper atmosphere radar in the middle atmosphere: 1. Method and general characteristics. J. Geophys. Res. 1996, 101, 29511–29521. [Google Scholar] [CrossRef]
- Xiong, J.; Wan, W.; Ning, B.; Liu, L. Gravity waves in the mesosphere observed with Wuhan meteor radar: A preliminary result. Adv. Space Res. 2003, 32, 831–836. [Google Scholar] [CrossRef]
- Preusse, P.; Dörnbrack, A.; Eckermann, S.D.; Riese, M.; Schaeler, B.; Bacmeister, J.T.; Broutman, D.; Crossmann, K.U. Space based measurements of stratospheric mountain waves by CRISTA: 1. Sensitivity, analysis method and a case study. J. Geophys. Res. 2002, 107, 8178. [Google Scholar] [CrossRef]
- John, S.R.; Kumar, K.K. TIMED/SABER observations of global gravity wave climatology and their interannual variability from stratosphere to mesosphere lower thermosphere. Clim. Dyn. 2012, 39, 1489–1505. [Google Scholar] [CrossRef]
- Liu, X.; Yue, J.; Xu, J.; Garcia, R.R.; Russell, J.M.; Mlynczak, M., III; Mlynczak, M.; Wu, D.L.; Nakamura, T. Variations of global gravity waves derived from 14 years of SABER temperature observations. J. Geophys. Res. Atmos. 2017, 122, 6231–6249. [Google Scholar] [CrossRef]
- Nielsen, K.; Taylor, M.J.; Pautet, P.-D.; Fritts, D.C.; Mitchell, N.; Beldon, C.; Williams, B.P.; Singer, W.; Schmidlin, F.J.; Goldberg, R.A. Propagation of short–period gravity waves at high–latitudes during the MaCWAVE winter campaign. Ann. Geophys. 2006, 24, 1227–1243. [Google Scholar] [CrossRef]
- Ejiri, M.K.; Taylor, M.J.; Nakamura, T.; Franke, S.J. Critical level interaction of a gravity wave with background winds driven by a large–scale wave perturbation. J. Geophys. Res. Atmos. 2009, 114, D18117. [Google Scholar] [CrossRef]
- Suzuki, S.; Lubken, F.J.; Baumgarten, G.; Kaifler, N.; Eixmann, R.; Williams, B.P.; Nakamura, T. Vertical propagation of a mesoscale gravity wave from the lower to the upper atmosphere. J. Atmos. Sol. Terr. Phys. 2013, 97, 29–36. [Google Scholar] [CrossRef]
- Senft, D.C.; Gardner, C.S. Seasonal variability of gravity wave activity and spectra in the mesopause region at Urbana. J. Geophys. Res. 1991, 96, 17229–17264. [Google Scholar] [CrossRef]
- Chen, C.; Chu, X.; Zhao, J.; Roberts, B.R.; Yu, Z.; Fong, W.; Lu, X.; Smith, J.A. Lidar observations of persistent gravity waves with periods of 3–10 h in the Antarctic middle and upper atmosphere at McMurdo (77.83° S, 166.67° E). J. Geophys. Res. Space Physics 2016, 121, 1483–1502. [Google Scholar] [CrossRef]
- Rauthe, M.; Gerding, M.; Höffner, J.; Lübken, F.-J. Lidar temperature measurements of gravity waves over Kühlungsborn (54oN) from 1 to 105 km: A winter-summer comparison. J. Geophys. Res. 2006, 111, D24108. [Google Scholar] [CrossRef]
- Lu, X.; Liu, A.Z.; Swenson, G.R.; Li, T.; Leblanc, T.; McDermid, I.S. Gravity wave propagation and dissipation from the stratosphere to the lower thermosphere. J. Geophys. Res. 2009, 114, D11101. [Google Scholar] [CrossRef]
- Baumgarten, G.; Fiedler, J.; Hildebrand, J.; Lübken, F.-J. Inertia gravity wave in the stratosphere and mesosphere observed by Doppler wind and temperature lidar. Geophys. Res. Lett. 2015, 42, 10929–10936. [Google Scholar] [CrossRef]
- Jia, M.; Xue, X.; Du, X.; Tang, Y.; Yu, C.; Wu, J.; Xu, J.; Yang, G.; Ning, B.; Hoffmann, L. A case study of a mesoscale gravity wave in the MLT region using simultaneous multi-instruments in Beijing. J. Atmos. Sol. Terr. Phys. 2016, 140, 1–9. [Google Scholar] [CrossRef]
- Gong, S.; Yang, G.; Xu, J.; Wang, J.; Gong, W.; Guan, S.; Gong, W.; Fu, J. Statistical characteristics of atmospheric gravity wave in the mesopause region observed with a sodium lidar at Beijing, China. J. Atmos. Sol. Terr. Phys. 2013, 97, 143–151. [Google Scholar] [CrossRef]
- Yu, Y.; Wan, W.; Ning, B.; Liu, L.; Wang, Z.; Hu, L.H.; Ren, Z. Tidal wind mapping from observations of a meteor radar chain in December 2011. J. Geophys. Res. Atmos. 2013, 118, 2321–2332. [Google Scholar] [CrossRef] [Green Version]
- Russell, J.M.; Mlynczak, M.G., III; Gordley, L.L.; Tansock, J.; Esplin, R. An overview of the SABER experiment and preliminary calibration results. Proc. SPIE 1999, 3756, 277–288. [Google Scholar]
- Remsberg, E.E.; Marshall, B.T.; Garcia-Comas, M.; Krueger, D.; Lingenfelser, G.S.; Martin-Torres, J.; Mlynczak, M.G.; Russell, J.M., III; Smith, A.K.; Zhao, Y.; et al. Assessment of the quality of the version 1.07 temperature-versus-pressure profiles of the middle atmosphere from TIMED/SABER. J. Geophys. Res. 2008, 113, D17101. [Google Scholar] [CrossRef]
- Ferrare, R.A.; McGee, T.J.; Whiteman, D.; Burries, J.; Owens, M.; Butler, J.; Barnes, R.A.; Schmidlin, F.; Komhyr, W.; Wang, P.H.; McCormick, M.P.; et al. Lidar measurements of stratospheric temperature during STOIC. J. Geophys. Res. 1995, 100, 9303–9312. [Google Scholar] [CrossRef]
- Yue, C.; Yang, G.; Wang, J.; Guan, S.; Du, L.; Cheng, X.; Yang, Y. Lidar observations of the middle atmospheric thermal structure over north China and comparisons with TIMED/SABER. J. Atmos. Sol. Terr. Phys. 2014, 120, 80–87. [Google Scholar] [CrossRef]
- Alpers, M.; Eixmann, R.; Fricke-Begemann, C.; Gerding, M.; Höffner, J. Temperature lidar measurements from 1 to 105 km altitude using resonance, Rayleigh, and Rotational Raman scattering. Atmos. Chem. Phys. 2004, 4, 793–800. [Google Scholar] [CrossRef] [Green Version]
- Guan, S.; Yang, G.; Chang, Q.; Cheng, X.; Yang, Y.; Gong, S.; Wang, J. New methods of data calibration for high power aperture lidar. Opt. Express 2013, 21, 7768–7785. [Google Scholar] [CrossRef] [PubMed]
- Dörnbrack, A.; Gisinger, S.; Kaifler, B. On the Interpretation of Gravity Wave Measurements by Ground-Based Lidars. Atmosphere 2017, 8, 49. [Google Scholar] [CrossRef]
- Baumgarten, K.; Gerding, M.; Lübken, F.-J. Seasonal variation of gravity wave parameters using different filter methods with daylight lidar measurements at midlatitudes. J. Geophys. Res. Atmos. 2017, 122, 2683–2695. [Google Scholar] [CrossRef]
- Ehard, B.; Kaifler, B.; Kaifler, N.; Rapp, M. Evaluation of methods for gravity wave extraction from middle-atmospheric lidar temperature measurements. Atmos. Meas. Tech. 2015, 8, 4645–4655. [Google Scholar] [CrossRef] [Green Version]
- Yang, J.; Xiao, C.; Hu, X.; Xu, Q. Seasonal variations of wind tides in mesosphere and lower thermosphere over Langfang (39.4° N, 116.7° E), China. Prog. Geophys. 2017, 32, 1501–1509. [Google Scholar] [CrossRef]
- Yuan, T.; She, C.Y.; Hagan, M.E.; Williams, B.P.; Li, T.; Arnold, K.; Kawahara, T.D.; Acott, P.E.; Vance, J.D.; Krueger, D.; et al. Seasonal variation of diurnal perturbations in mesopause region temperature, zonal, and meridional winds above Fort Collins, Colorado (40.6° N, 105.1° W). J. Geophys. Res. 2006, 111. [Google Scholar] [CrossRef]
- Tsuda, T.; Kato, S.; Yokol, T.; Inoue, T.; Yamamoto, M.; VanZandt, T.E.; Fukao, S.; Sato, T. Gravity waves in the mesosphere observed with the middle and upper atmosphere radar. Radio Sci. 1990, 26, 1005–1018. [Google Scholar] [CrossRef]
- Nakamura, T.; Tsuda, T.; Yamamoto, M.; Fukao, S.; Kato, S. Characteristics of gravity waves in the mesosphere observed with the middle and upper atmosphere radar, 1 & 2. J. Geophys. Res. 1993, 98, 8899–8923. [Google Scholar]
- Hu, X.; Liu, A.Z.; Gardner, C.S.; Swenson, G.R. Characteristics of quasi–monochromatic gravity waves observed with Na lidar in the mesopause region at Starfire Optical Range, NM. Geophys. Res. Lett. 2002, 29, 2169. [Google Scholar] [CrossRef]
- Fetzer, E.J.; Gille, J.C. Gravity wave variances in LIMS temperatures. Part I. Variability and comparison with background winds. J. Atmos. Sci. 1994, 51, 2461–2483. [Google Scholar] [CrossRef]
- Preusse, P.; Eckermann, S.D.; Ern, M.; Oberheide, J.; Picard, R.H.; Roble, R.G.; Riese, M.; Russell, J.M., III; Mlynczak, M.G. Global ray tracing simulations of the SABER gravity wave climatology. J. Geophys. Res. 2009, 114, D08126. [Google Scholar] [CrossRef]
- Yamashita, C.; England, S.L.; Immel, T.J.; Chang, L.C. Gravity wave variations during elevated stratopause events using SABER observations. J. Geophys. Res. Atmos. 2013, 118, 5287–5303. [Google Scholar] [CrossRef] [Green Version]
- Liu, X.; Yue, J.; Xu, J.; Wang, L.; Yuan, W.; Russell, J.M., III; Hervig, M.E. Gravity wave variations in the polar stratosphere and mesosphere from SOFIE/AIM temperature observations. J. Geophys. Res. Atmos. 2014, 119, 7368–7381. [Google Scholar] [CrossRef] [Green Version]
- Thurairajah, B.; Bailey, S.M.; Cullens, C.Y.; Hervig, M.E.; Russell, J.M., III. Gravity wave activity during recent stratospheric sudden warming events from SOFIE temperature measurements. J. Geophys. Res. Atmos. 2014, 119, 8091–8103. [Google Scholar] [CrossRef] [Green Version]
- Torrence, C.; Compo, G.P. A practical guide to wavelet analysis. Bull. Am. Meteorol. Soc. 1998, 79, 61–78. [Google Scholar] [CrossRef]




| Instruments | Altitude Range Covered | Wavelength | Wave Period | Propagation Direction |
|---|---|---|---|---|
| Lidar | 35–86 km | upward | ||
| Meteor radar | 76–106 km | * | * | upward; southwest |
| TIMED/SABER | 30–86 km | -- | -- |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gong, S.; Yang, G.; Xu, J.; Liu, X.; Li, Q. Gravity Wave Propagation from the Stratosphere into the Mesosphere Studied with Lidar, Meteor Radar, and TIMED/SABER. Atmosphere 2019, 10, 81. https://doi.org/10.3390/atmos10020081
Gong S, Yang G, Xu J, Liu X, Li Q. Gravity Wave Propagation from the Stratosphere into the Mesosphere Studied with Lidar, Meteor Radar, and TIMED/SABER. Atmosphere. 2019; 10(2):81. https://doi.org/10.3390/atmos10020081
Chicago/Turabian StyleGong, Shaohua, Guotao Yang, Jiyao Xu, Xiao Liu, and Qinzeng Li. 2019. "Gravity Wave Propagation from the Stratosphere into the Mesosphere Studied with Lidar, Meteor Radar, and TIMED/SABER" Atmosphere 10, no. 2: 81. https://doi.org/10.3390/atmos10020081
APA StyleGong, S., Yang, G., Xu, J., Liu, X., & Li, Q. (2019). Gravity Wave Propagation from the Stratosphere into the Mesosphere Studied with Lidar, Meteor Radar, and TIMED/SABER. Atmosphere, 10(2), 81. https://doi.org/10.3390/atmos10020081




