Significance of Absorbing Fraction of Coating on Absorption Enhancement of Partially Coated Black Carbon Aerosols

Abstract

Black carbon (BC), particularly internally mixed and aged BC, exerts a significant influence on the environment and climate. Black carbon coated by non-absorbing materials shows an enhancement of BC absorption, whereas absorptive coatings on BC can reduce the BC absorption enhancement. In this paper we use the multiple-sphere T-matrix method to accurately model the influence of the absorbing volume fraction of absorbing coatings on the reduction of the absorption enhancement of partially coated BC. The reduction of the absorption enhancement due to the absorbing coating exhibited a strong sensitivity to the absorbing volume fraction of the coating, and no reduction of BC absorption enhancement was seen for BC particles with non-absorbing coatings. We found that coatings with higher absorbing volume fraction, greater coated volume fraction of BC, higher shell/core ratio, and larger coated BC particle size caused stronger reductions of the BC absorption enhancement, whereas the impact of the BC’s fractal dimension was negligible. Moreover, the sensitivity of the reduction of absorption enhancement resulting from the ratio of the absorbing coating shell to the BC core increased for coatings with higher absorbing volume fractions, higher coated volume fractions of BC, or larger particle sizes, although this effect was weaker than the sensitivities to size distribution, absorbing volume fraction of coating, and coated volume fraction of BC. Reductions in the absorption enhancements resulting from the absorbing coating for partially coated BC with various size distributions typically varied in the range of 0.0–0.24 for thin coatings with shell/core ratio of 1.5 and between 0.0 and 0.43 for thick coatings with shell/core ratio of 2.7. In addition, we propose an empirical formula relating the reduction of BC absorption enhancement to the absorbing volume fraction of the coating, which could inform a quantitative understanding and further applications. Our study indicates the significance of the absorbing volume fraction of coatings on the absorption properties of BC.

1. Introduction

Aerosols in ambient air can interact with atmospheric radiation, and influence the climate and synoptic weather through the process of radiative transfer [1,2,3,4,5]. Among various aerosol constituents, carbonaceous aerosols (e.g., black carbon, BC) exert a profound influence on climate change and global energy balance due to the intense absorption and scattering of radiation [6]. As a byproduct of the incomplete combustion of fossil fuels, biomass burning, and biofuels, BC aerosols contribute significant positive radiative forcing at the top of the atmosphere and serve as a strong anthropogenic warming agent [7]. At present, the understanding of BC’s radiative properties is still limited due to the aggregates’ complex microphysics (e.g., morphology, mixing state), which make BC and organics some of the largest uncertainties in the assessment of aerosol radiative forcing [8].

Fresh BC particulates are commonly hydrophobic and externally mixed, whereas they become internally mixed with other aerosol constituents due to aging processes [9,10]. It is reported that this aging process occurs on a timescale from a few minutes to several days [11,12] and is even faster in polluted atmospheric environments [13,14]. During the aging process, BC can have water-soluble coating components, including condensations of nitrate, organics, and sulfate [15,16]; coagulations with preexisting aerosol species [17,18]; and heterogeneous reactions with gaseous oxidants [19,20]. Among BC coatings, an absorbing coating (named brown carbon) is a type of organic matter that can absorb radiation in ultraviolet and visible spectral regions [21]. Coatings on BC can significantly change BC’s microphysical, optical, and radiative properties, which have been presented in multiple studies [22]. Coated BC tends to be a compact aggregate compared to fresh chain-like structures, and large aggregate fractal dimensions close to 3.0 have been observed [23]. The optics of BC (e.g., absorption) are altered after coating, and positive radiative forcing may be caused compared to the conditions of external mixing [24]. A few recent review papers have nicely summarized and highlighted the importance BC coating materials and structures and the associated radiative effects on BC absorption [25,26,27].

Field experiments have indicated that a non-absorbing coating internally mixed with BC can significantly enhance BC absorption, although weak BC absorption enhancements have also been observed [28]. It has been reported that the mass ratio of non-BC components to BC within particles determines BC absorption enhancement, whereas no absorption enhancement has been reported for ratios lower than 1.5 [29]. Chakrabarty and Heinson [30] propose a scaling law to relate absorption enhancements to mass cross sections of absorption. A wavelength-dependent BC absorption enhancement with a value near 1.6 at near-IR wavelength was observed by You et al. [31]. Fresh BC from combustion showed a BC absorption enhancement of 1.4 whilst it reached about 3.0 for aged BC over a rural area in north China [32]. A study in Jinan, China reported an average BC absorption enhancement of 2.07, with diurnal variation patterns showing low and high values in the morning and afternoon, respectively [33]. In addition to field observations, numerical investigations related to the absorption of coated BC have also been carried out. Coated BC absorption impacted by aggregate morphology and internal mixing with the discrete dipole approximation is studied in Scarnato et al. [34]. Dong et al. [35] simulated aged BC with irregular sulfate coating and numerically investigated the absorption of coated BC as influenced by its morphology. He et al. [36] developed a model for BC aging to simulate the absorption enhancements of BC at different monodisperse sizes, and found that BC absorption enhancements varied with time. Black carbon absorption enhancements resulting from non-absorbing coatings influenced by detailed particle microphysics have been reported, and the significance of the coated volume fraction of BC on the determination of BC absorption enhancement has been emphasized [37,38].

While the absorption enhancement of BC with non-absorptive coatings has become clearer, the influence of absorbing coatings on BC absorption enhancement is still an open question. Some recent ambient experiments evaluating absorption enhancement have tried to eliminate both inorganic and organic coatings on BC particles; high absorption enhancement values of approximately 3.0 could be observed [32,33]. Nonetheless, these measured absorption enhancements may be overestimated, as organic coatings may include brown carbon [39], and the absorbing coating contributes to the overall absorption properties of coated BC aerosols. Luo et al. [40] numerically studied the impact of brown coatings on the absorption enhancement of fully encapsulated BC particles. The observations showed that aged BC aerosols commonly had fully coated, externally attached, and partially coated morphologies [41,42]. Although partial coating is also a significant mixing state of aged BC in the ambient atmosphere, studies on the absorption enhancement of partially coated BC as influenced by absorbing coatings are generally limited. Meanwhile, the coatings of BC may be a mixture of both non-absorbing and absorbing species, and the impact of these mixed coatings on BC absorption enhancement still needs more investigation.

Here, the absorption properties of BC particles partially coated with mixed coatings, and the reductions of absorption enhancement due to absorbing coatings, are systematically investigated based on current understandings. The absorption of coated BC aggregates is exactly calculated using a multiple-sphere T-matrix method (MSTM). The aim is to study the effect of the absorbing volume fraction of coatings on the reduction of absorption enhancement of partially coated BC due to the absorbing coating, which hopefully benefits our understanding of this subject and informs further applications in radiative transfer and aerosol–climate models.

2. Materials and Methods

Fresh BC aerosols generally show loose cluster-like aggregate structures with many small spherical monomers with similar sizes [43]. The fractal aggregate model has been successfully employed to describe these BC geometries on the basis of a mathematical scaling law following [44]:

$$N={\mathrm{k}}_0{\left(\frac{R_g}{a}\right)}^{D_f},$$

(1)

$$R_g^{}=\sqrt{\frac{1}{N}{\displaystyle \sum _{i=1}^N{r}_i^2}},$$

(2)

where the fractal prefactor of an aggregate (${\mathrm{k}}_0$), monomer number ($N$), fractal dimension ($D_f$), and monomer radius ($a$) construct its structure. $r_i$ is the distance between the center of mass and the ith monomer, while $R_g$ is the radius of gyration, representing the aggregate’s total spatial size. Black carbon aerosols can be coated with other species (e.g., organics, sulfate) after being emitted into the atmosphere, and become aged quickly [13]. After being coated, chain-like BC structures can collapse into compact aggregates, and their radiative properties may be substantially altered [23]. It has been documented that the fractal dimensions of coated BC aggregates can be near 3, while fresh BC generally shows fractal dimensions less than 2 [23]. The mixing-state observations show that coated BC particles mainly exhibit three morphologies: fully coated, externally attached, and partially coated [41,42]. The coated volume fraction of BC ($F$) is used to describe the morphologies of coated BC, and it is defined as:

$$F=\frac{V_{BC within coating}}{V_{BC}},$$

(3)

where $V_{BC within coating}$ indicates the volume of BC within a given coating and $V_{BC}$ is the overall volume, including the BC both in and outside its coating [38]. Based on this microphysical parameter, $F=0$, $0<F<1$, and $F=1$ correspond to externally attached, partially coated, and fully coated BC aerosols, respectively.

This study followed the BC model developed by Zhang et al. [38], and spherical coatings were considered for partially coated BC aggregates with no overlapping of monomers, as portrayed in Figure 1. It should be noted that some monomers of BC were slightly moved to avoid overlaps whereas the inner and outer parts of the aggregate were still in point contact. We considered shell/core ratios $D_P/D_c$ (volume equivalent diameter of a particle divided by diameter of the BC core) in the range of 1.1–2.7 based on observations in other works [45,46]. Black carbon aggregates were generated with a tunable algorithm of monomer-cluster aggregation developed by Skorupski et al. [47]. The ${\mathrm{k}}_0$ of BC aerosols was assumed to be 1.2 [44], and $D_f$ values were considered as 1.8 and 2.8, describing loose and compact morphologies of BC, respectively [48]. This study followed the method in Zhang et al. [38] and considered an $N$ of 200, as BC particles are mostly observed in accumulation mode.

Table 1. Microphysical parameters for coated BC particles.

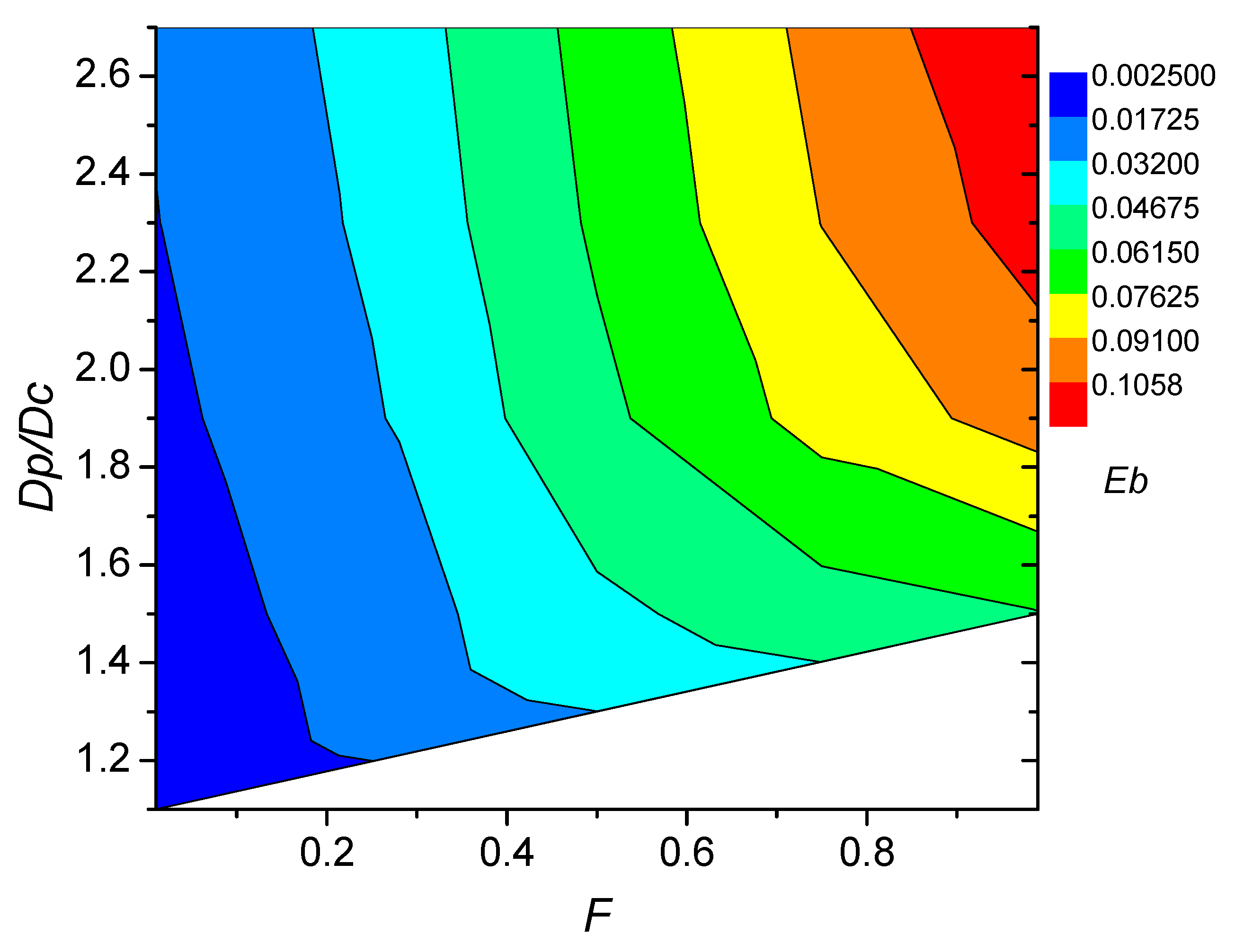
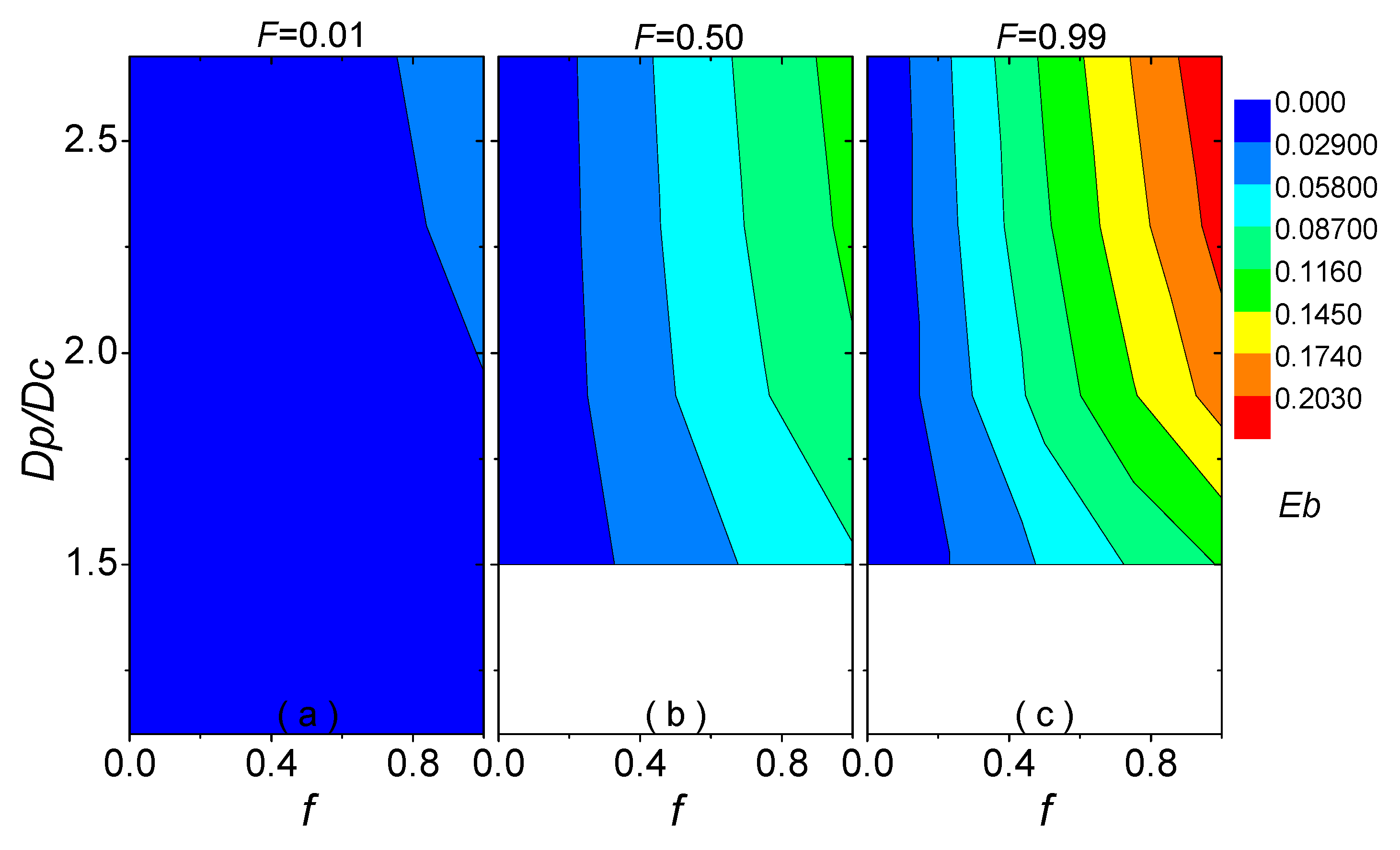
Parameters		Used Values
Df of BC		1.8, 2.8
Dp/Dc		1.1, 1.5, 1.9, 2.3, 2.7
F		0.01, 0.25, 0.5, 0.75, 0.99
Size distribution	rg, μm	0.075 (0.05–0.15)
	σg	1.59

summarizes the BC microphysical parameters used in the study, which were considered as representative on the basis of observations, and the physical meanings of the abbreviated parameters are listed in

Table 2. Abbreviated parameters for coated BC particles.

Abbreviated Parameters	Meaning
Df	Fractal dimension
Dp/Dc	Shell/core ratio
F	Coated volume fraction of BC
f	Absorbing volume fraction of coating

.

Given the constructed model of aged BC, we applied the MSTM [49] to calculate the random-orientation absorption properties of BC. This study considered the ensembles of coated BC with a lognormal size distribution in the form of

$$\mathrm{n}(r)=\frac{1}{\sqrt{2\pi }r\ln ({\sigma }_g)}\exp \left[-{\left(\frac{\ln (r)-\ln (r_g)}{\sqrt{2}\ln ({\sigma }_g)}\right)}^2\right],$$

(4)

where $r_g$ denotes the geometric radius of coated BC, while ${\sigma }_g$ is the standard deviation. We considered an $r_g$ of 0.075 μm [50] and a ${\sigma }_g$ of 1.59 [51,52]. The absorption cross section ($C_{abs}$) of coated BC with this size distribution was obtained using

$$\langle C_{abs}\rangle ={\displaystyle {\int }_{r_{\min }}^{r_{\max }}{C}_{abs}(r)}n(r)d(r).$$

(5)

The radius was considered in a range from 0.05 μm to 0.5 μm, and a linearly equidistant radius interval with value of 0.005 μm was used for averaging.

We modeled BC aggregates coated by organics, and organic coatings in ambient air may contain absorbing organics in addition to non-absorbing coatings. The absorbing volume fraction of coating ($f$) was used to characterize the volume percentages of absorbing organics in the whole coatings, and it is calculated as:

$$f=\frac{V_{absorbing}}{V_{absorbing}+V_{non\text{-}absorbing}},$$

(6)

where $V_{absorbing}$ indicates the volume of absorbing coating, and $V_{non-absorbing}$ denotes the volume of non-absorptive coating. We utilized an effective refractive index for the coatings of organics that included both absorbing and non-absorbing components determined by $f$. The popular method of volume-weighted average was used to determine the effective refractive indices of this internally mixed coating, as it produced acceptable absorption results of coated BC particles in the accumulation mode [53]. We investigated the absorption properties of coated BC at 550 nm wavelength, and the refractive indices of absorbing organics, non-absorbing organics, and BC are $1.55-0.03i$ [54], $1.55-0i$ [55], and $1.85-0.71i$ [56], respectively.

3. Results and Discussion

3.1. Blocking Effect of Absorbing Coatings on BC Absorption Enhancement

To investigate the effect of absorbing coatings on BC absorption enhancement, we considered the impacts of the scattering and absorption of absorbing coatings separately and its scattering acting as non-absorbing coatings on BC [52]. We compared the sum of the absorptions of absorbing coatings and BC with non-absorbing coatings to the absorption of BC with absorbing coatings alone. The results of partially coated BC aerosols with a coating absorbing volume fraction of 0.5 are illustrated in Figure 2. The absorptions of partially coated BC particles were averaged using the aforementioned size distribution with $r_g=0.075$ μm and ${\sigma }_g=1.59$. As shown in Figure 2a–e, the absorption cross sections $C_{abs}$ of partially coated BC with an absorbing volume fraction of coating $f$ of 0.5 were highly sensitive to the shell/core ratio $D_p/D_c$ and coated volume fraction of BC $F$, and slightly sensitive to the BC fractal dimension. The $C_{abs}$ of partially coated BC significantly decreased with increasing $D_p/D_c$, while it increased with increasing $F$. This indicates that a decrease of $D_p/D_c$ or an increase of $F$ would cause enhanced BC absorption. Zhang et al. indicated that absorption enhancements of aged BC are significantly influenced by particle microphysics such as $F$ and $D_p/D_c$ [37,38]. Figure 2a–e also indicates that the absorption of coated BC with an $f$ of 0.5 was lower than the sum of the absorptions of its absorbing coating and BC coated with related non-absorbing materials. This implies that the absorbing coating exerts a blocking effect on BC absorption enhancement, and weakening the overall particle absorption.

The absorption differences between coated BC with $f$ of 0.5 and the sum of the absorptions of its absorbing coating and BC with a related non-absorbing coating are illustrated in Figure 2f–j. It is obvious that their absorption differences were sensitive to shell/core ratio, coated volume fraction of BC, and BC fractal dimension. The differences of absorption caused by different BC fractal dimensions were generally small, and the differences became smaller at larger $D_f$. The absorption differences were strongly sensitive to $F$ and $D_p/D_c$, indicating high sensitivities of the blocking effect induced by the absorbing coating to the shell/core ratio and the coated volume fraction of BC. With increased $F$ or decreased $D_p/D_c$, the absorption of coated BC with an $f$ of 0.5 increasingly differed from the sum of absorptions of absorbing coating and BC coated with non-absorbing materials. Similar to BC absorption enhancement, we use the following equation to quantitatively express the blocking effect provided by an absorbing coating:

$$E_b=-\frac{\langle C_{abs\_\mathrm{c}oated}\rangle -\langle C_{abs\_absorbing}\rangle -\langle C_{abs\_nonabsorbing}\rangle }{\langle C_{abs\_bare}\rangle },$$

(7)

where $\langle C_{abs\_coated}\rangle$, $\langle C_{abs\_absorbing}\rangle$, $\langle C_{abs\_nonabsorbing}\rangle$, and $\langle C_{abs\_bare}\rangle$ are the absorptions of BC with an absorbing coating, the absorbing shell itself, BC coated with a non-absorbing coating, and bare BC, respectively [40]. The $E_b$ indicates the reduction of BC absorption enhancement due to the blocking effect induced by an absorbing coating. An $E_b$ of zero implies no reduction in absorption enhancement, while there is full reduction (i.e., no absorption enhancement) if $E_b$ is equal to −1.0. Our study models more realistic BC geometries, whereas core/shell models consider that spheres may shield part of the absorbing mass and thus reduce the observed absorption cross sections [26].

Figure 3 illustrates results regarding the $E_b$ partially coated BC with the aforementioned size distribution for different shell/core ratio and coated volume fraction of BC, where BC aggregates with a $D_f$ of 2.8 and $f$ of 0.5 are considered. Figure 3 clearly shows that the $E_b$ of partially coated BC was highly sensitive to $F$ and $D_p/D_c$, and had a large variation. For $F$ near 0.0, the $E_b$ of coated BC with $f$ of 0.5 exhibited weak variations with changing $D_p/D_c$ in the range of 0.003–0.019. As $F$ increased, the $E_b$ of coated BC was more sensitive to $D_p/D_c$ and its value became larger. With $F$ close to 1.0, the $E_b$ of coated BC with an $f$ of 0.5 showed large values between 0.06 and 0.12. For fixed $f$ and BC $D_f$, the $E_b$ increased as $F$ or $D_p/D_c$ increased. This indicates that a higher percentage of BC coated with a coating or a higher volume of coating leads to greater reductions in the absorption enhancement resulting from the blocking effect of the absorbing coating. Generally, these reductions were strongly sensitive to shell/core ratio and coated volume fraction of BC. Meanwhile, with increasing coated volume fraction of BC, the sensitivity to shell/core ratio increased.

3.2. Influence of Coating Absorbing Volume Fraction on Blocking Effect

Figure 4 depicts the $E_b$ of partially coated BC as functions of $D_p/D_c$ and $f$ for different $F$. The aforementioned size distribution with $r_g=0.075$μm and ${\sigma }_g=1.59$, and a BC $D_f$ of 2.8 are considered. The $E_b$ was strongly sensitive to $f$, and its sensitivity to $f$ was higher than that to $D_p/D_c$. The $E_b$ increased as $f$ increased, indicating that a higher coating absorption more strongly reduces the BC absorption enhancement with a stronger blocking effect. For BC with $f=0.0$, the $E_b$ of partially coated BC was zero, implying the absence of a blocking effect. Nevertheless, as $f$ increased to 1.0, the $E_b$ increased significantly and exhibited values of 0.01–0.04, 0.08–0.13, and 0.12–0.23 for partially coated BC with $F$ of 0.01, 0.5, and 0.99, respectively. For $F$ values of 0.01, 0.5, and 0.99, the $E_b$ values of thinly coated BC with $D_p/D_c=1.5$ were 0.0–0.02, 0.0–0.08, and 0.0–0.12, respectively, whereas for thick coatings, the $E_b$ of coated BC became more sensitive to $f$ with wider variations. Moreover, for a fixed $D_p/D_c$, the $E_b$ of partially coated BC was more sensitive to $f$ at higher $F$ values. Meanwhile, for a fixed $F$, the $E_b$ of partially coated BC showed stronger sensitivity to $D_p/D_c$ at higher $f$.

To compare the $E_b$ sensitivities to $f$ with those to $F$, Figure 5 shows the $E_b$ of partially coated BC ($D_f=2.8$) with the aforementioned size distribution as functions of $f$ and $F$ for different shell/core ratios. For $D_P/D_c$ values of 1.5, 1.9, 2.3, and 2.7, the $E_b$ values of partially coated BC with all possible coating structures were in the ranges of 0.0–0.12, 0.0–0.19, 0.0–0.21, and 0.0–0.23, respectively. It is obvious that the sensitivity of the $E_b$ to $f$ was similar to that to $F$. For a fixed $F$, the sensitivity of the $E_b$ to $f$ became higher as $D_p/D_c$ increased. Furthermore, for a fixed $D_p/D_c$ value, the sensitivity of the $E_b$ of partially coated BC to $F$ was higher at higher $f$. To summarize the results displayed in Figure 4 and Figure 5, the reductions in the absorption enhancements of partially coated BC as a result of the blocking effect of coatings were very sensitive to $f$, $F$, and $D_p/D_c$, and the sensitivities to $f$ and $F$ were stronger than those to $D_p/D_c$. Meanwhile, the sensitivity of the reduction of absorption enhancement of partially coated BC to $D_p/D_c$ became stronger as $f$ and $F$ increased.

The results discussed above consider coated BC with a fixed particle size distribution, and the impact of size distribution on the reductions in the absorption enhancement of partially coated BC due to the blocking effect is given in the following. Figure 6 illustrates the $E_b$ of partially coated BC with various particle size distributions and $f$ values for different $F$. We assumed coated BC particles following lognormal distributions with $r_g$ between 0.05 and 0.15 μm and ${\sigma }_g$ of 1.59. A BC fractal dimension of 2.8 and two shell/core ratios (1.5 and 2.7, representing thin and thick coatings, respectively) were considered. The $E_b$ of BC with an absorbing coating increased as $r_g$ increased, indicating that large particles of coated BC enhance the blocking effect due to the strong absorption of the coating. Meanwhile, the sensitivity of $E_b$ to $r_g$ was similar to its sensitivity to $f$. For fixed $F$ and $D_p/D_c$, large $f$ or $r_g$ led to high $E_b$, and partially coated BC with $f=0.0$ showed no blocking effect at all evaluated size distributions. With increasing $F$, the $E_b$ showed increased sensitivity to size distribution, and thinly coated BC with $D_p/D_c=1.5$ exhibited $E_b$ values in the ranges of 0.0–0.03, 0.0–0.16, and 0.0–0.24 for $F$ of 0.01, 0.5, and 0.99, respectively. The $E_b$ became more sensitive to particle size distribution for larger $D_p/D_c$, and wide $E_b$ variations in the ranges of 0.0–0.07, 0.0–0.24, and 0.0–0.43 were seen for thickly coated BC ($D_p/D_c=2.7$) with $F$ of 0.01, 0.5, and 0.99, respectively. In general, the $E_b$ of partially coated BC was sensitive to size distribution, and its sensitivity became stronger with increasing values of $F$, $D_p/D_c$, or $f$.

3.3. Empirical Formula for Blocking Effect Influenced by Absorbing Volume Fraction of Coating

It is possible to establish the relation between $E_b$ and $f$ on the basis of the sensitivity analyses of the reduction of the absorption enhancements of partially coated BC due to the blocking effect, as influenced by $f$ and other microphysical parameters. The BC fractal dimension was not considered, as it showed a negligible impact on the $E_b$ of partially coated BC in the analyses. To facilitate practical applications, we considered a log-normal size distribution with $r_g=0.075 \mu \mathrm{m}$ and ${\sigma }_g=1.59$, which is intensively used in aerosol–climate models, and an empirical formula was assumed with the expression:

$$E_b=E_{b0}+k_{1}f\times F+k_{2}f\times F\times D_p/D_c,$$

(8)

where k₁ and k₂ are coefficients and indicate the significances of related influences on the $E_b$ of coated BC. These coefficients can be fitted with the 95% confidence range, and the $E_b$ of partially coated BC can be expressed as:

$$E_b=0.00817+0.00495f\times F+0.0877f\times F\times D_p/D_c.$$

(9)

The fitting coefficients were obtained with the lowest root-mean-square relative errors for all $E_b$ ($R^2=0.97$). The influences of $f$ and other particle microphysical parameters on $E_b$ were evidently affirmed by fitting the coefficients in Equation (9) for quantitative understanding, and large fitting coefficients indicate significant effects on $E_b$. To show the capability of the empirical formula in predicting the $E_b$ of partially coated BC, Figure 7 illustrates absolute $E_b$ differences between predictions obtained by Equation (9) and accurate numerical simulations. The predicted $E_b$ results agree well with accurate simulations, with differences all within 0.03. This suggests that the simple empirical formula we proposed generates rather accurate estimations of $E_b$ for partially coated BC with representative size distributions, giving confidence on the accuracy of the empirical formula.

4. Conclusions

This study used an inhomogeneous model for the study of the absorption properties of partially coated BC particles computed using the MSTM, investigating the effect of the coating absorbing volume fraction on the reduction of BC absorption enhancement induced by blocking effects. Our results indicate that this reduction in BC absorption enhancement is strongly sensitive to the absorbing volume fraction of coating, and that there is no blocking effect for BC with a non-absorbing coating. Stronger blocking effects were seen for partially coated BC with a higher coating absorbing volume fraction, higher shell/core ratio, larger coated volume fraction of BC, or larger particle size of coated BC, whereas the fractal dimension of BC had a minor impact on blocking effect. The reductions of the absorption enhancements of partially coated BC with various size distributions typically varied in the range of 0.0–0.24 for thin coatings ($D_p/D_c=1.5$) and in the range of 0.0–0.43 for thick coatings ($D_p/D_c=2.7$). Furthermore, the sensitivities of the reductions of BC absorption enhancement to the absorbing volume fraction of coating, size distribution, and coated volume fraction of BC were higher than the sensitivity to shell/core ratio. The sensitivity to shell/core ratio became stronger for higher coated volume fraction of BC, higher coating absorbing volume fraction, or larger particle size. Additionally, we presented an empirical formula relating $E_b$ to $f$, and it predicted the $E_b$ of partially coated BC well, with differences less than 0.03 for a typical log-normal size distribution with $r_g=0.075 \mu \mathrm{m}$ and ${\sigma }_g=1.59$. Overall, this study highlights the importance of the coating absorbing volume fraction on the reduction of BC absorption enhancement due to blocking effects, and compares the sensitivities of the blocking effect to particle microphysical parameters. With significant influences on BC absorption properties—particularly a reduction of BC absorption enhancement due to blocking effects—there is an urgent need to consider absorbing coatings in BC observations and radiative simulations.