Turbulence in Large-Scale Two-Dimensional Balanced Hard Sphere Gas Flow
Abstract
:1. Introduction
2. The Vlasov-Type Equation with External Acceleration
2.1. Preservation of the Rényi Divergences
2.2. Joint Two-Particle Marginal Distribution of the Gibbs State
2.3. Transport Equation for a Single Particle
2.4. A Closure for the Single Particle Transport Equation
3. The Two-Dimensional Moment Equations in the Presence of Gravity
3.1. The Velocity Moment Equations
3.2. Approximation for the Vertically Integrated Mean Field Potential
4. Numerical Simulations
4.1. Density of the Air-Like Hard Sphere Gas
4.2. Two Simulated Scenarios of a Balanced Flow
- Inertial jet. In an inertial jet, the pressure is constant throughout the domain. Observe that, in our equations, the Coriolis acceleration of Earth’s rotation is not present, which corresponds roughly to the equatorial region. Thus, such inertial flow describes a special case of geostrophic flow near the equator.
- Cyclostrophic vortex. In a cyclostrophic vortex, the centripetal force, acting on a rapidly rotating gas, is balanced by the pressure gradient, and the velocity is orthogonal to both. Again, given the absence of the Coriolis acceleration, this scenario corresponds to a large-scale, rapidly rotating flow, where the Coriolis acceleration is negligible in comparison with the centripetal acceleration—that is, a fully developed tropical cyclone.
4.3. Computation of Initial and Boundary Conditions
- First, we specify the velocity of the flow in the domain, and on those boundaries where the Dirichlet boundary condition is specified. The way in which we specify the velocity is entirely scenario-dependent; in the inertial jet scenario, the initial velocity field forms a jet stream, while, in the cyclostrophic vortex scenario, the initial velocity field forms a rotating flow with an appropriate radial profile.
- Once is defined, we compute the kinetic temperature of the flow using the Bernoulli law for a compressible gas:where the background temperature of the gas is set to K—that is, the approximate vertically averaged temperature of air throughout the troposphere. Observe, however, that in the present setting, the gas is two-dimensional, and, therefore, the effective adiabatic exponent .
- Once and are defined, we specify the pressure (which automatically yields the density via ). Below, we study two scenarios: an inertial jet, and a cyclostrophic vortex. In the inertial jet scenario, we set the pressure to a constant value ,where kg/m, which constitutes the approximate mass of the air column per unit area of the Earth’s surface. In the cyclostrophic vortex scenario, the pressure gradient is balanced by the centripetal force:where is the coordinate of the point relative to the center of rotation, is the angular velocity of rotation, and the latter identity is valid for a planar rotation (that is, when ). Here, the velocity is orthogonal to both and , that is,Upon substitution, this leads toAssuming, in turn, that , and do not depend on the angle, and only depend on , we denote , , and arrive at the following explicit formula for :Above, in the integration formula, we assume that vanishes sufficiently far away from the center of rotation. Moreover, in practice, one can simplify the integration by presuming that varies weakly in comparison to under the integral, and factor the kinetic temperature out of the integration (as we do further below).
4.4. Numerical Methods and Software
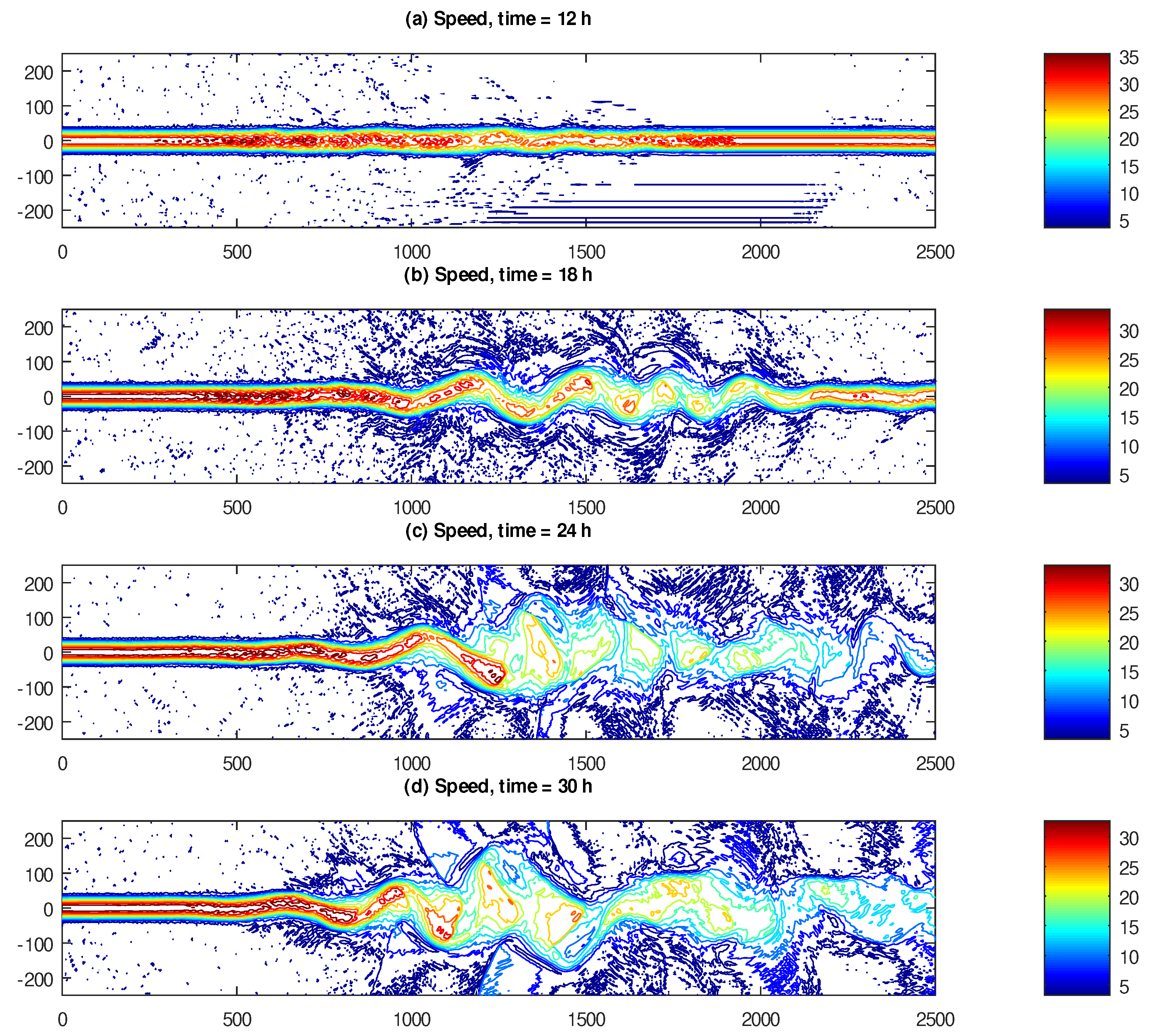
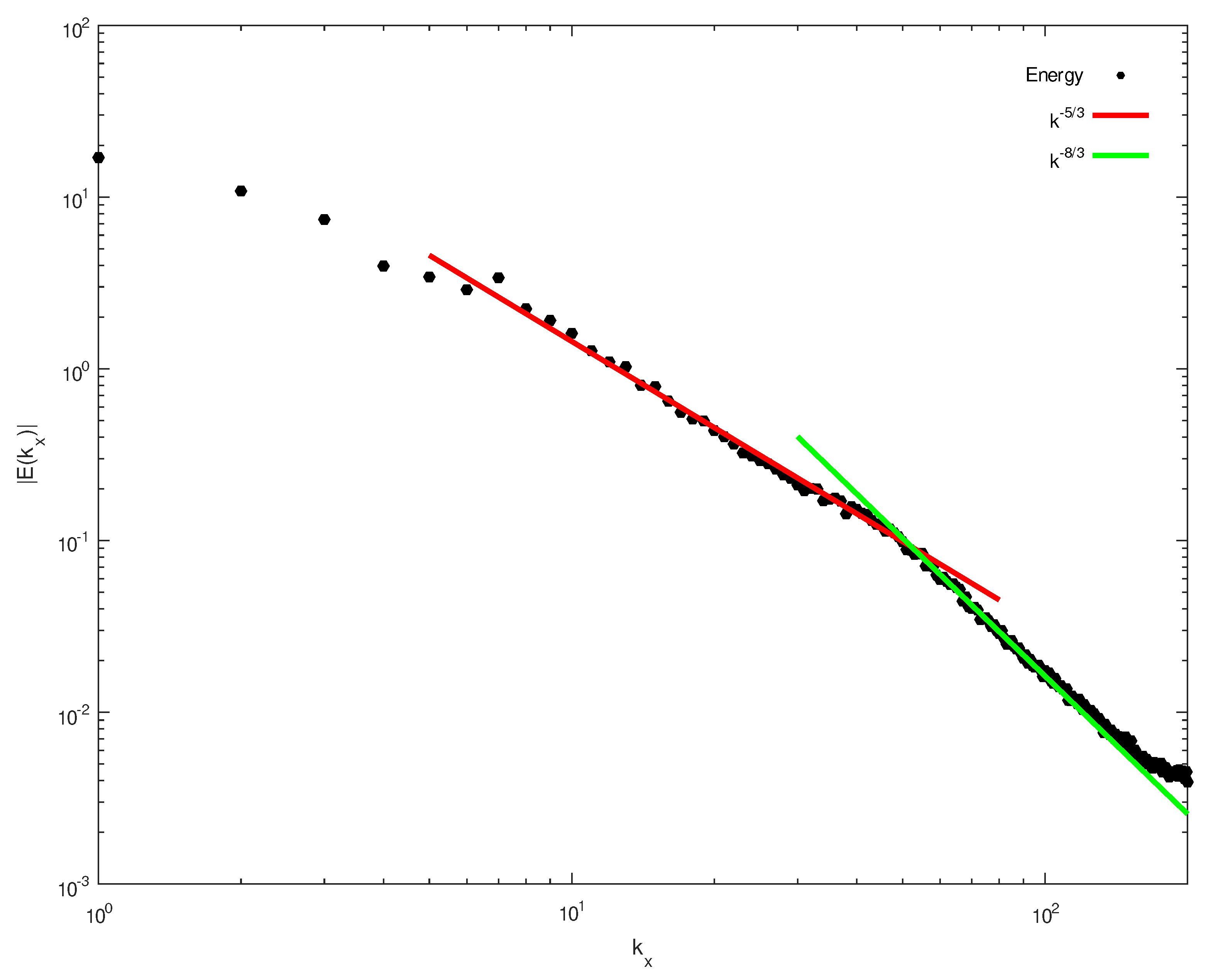
4.5. Numerical Simulation of an Inertial Jet
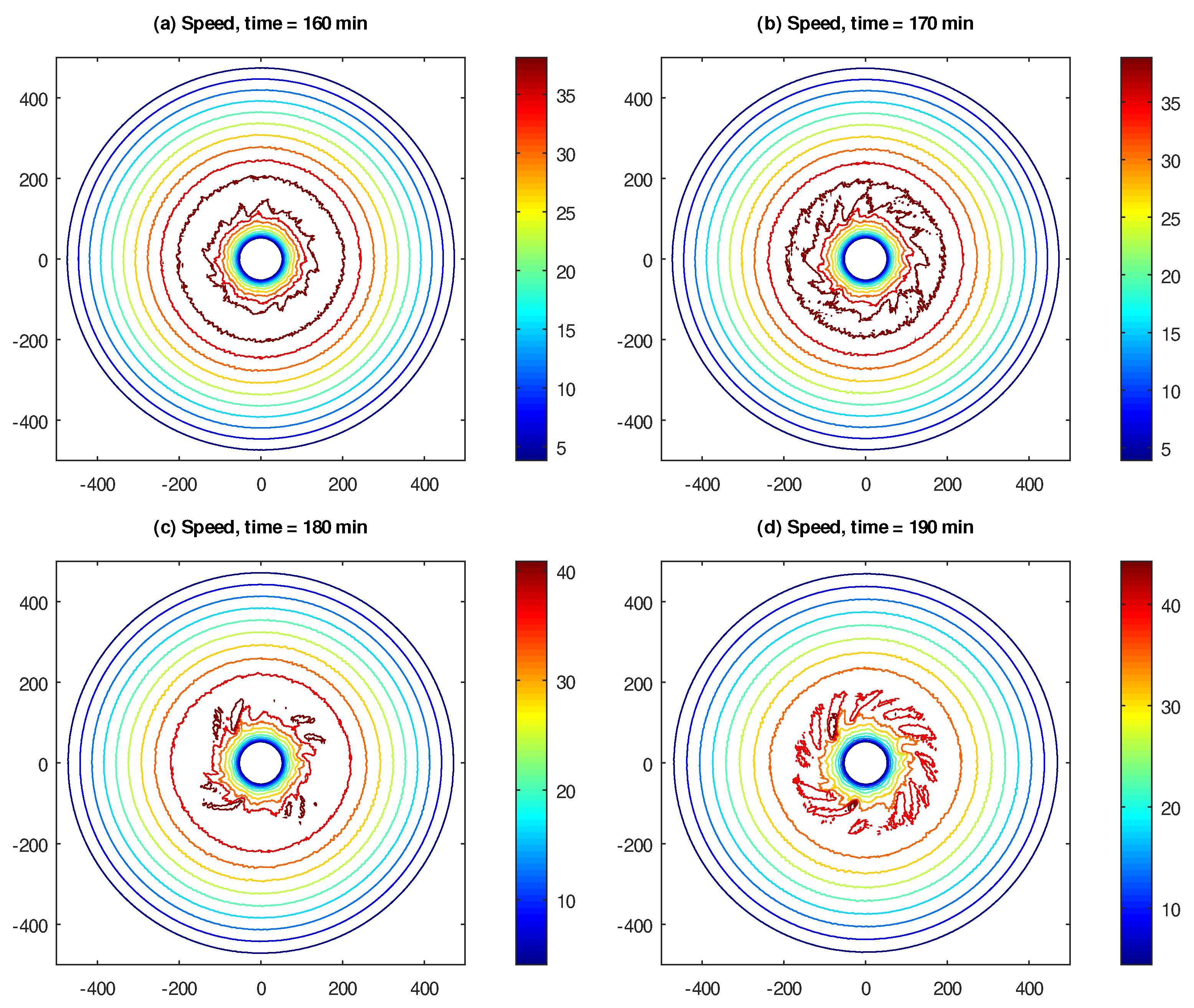
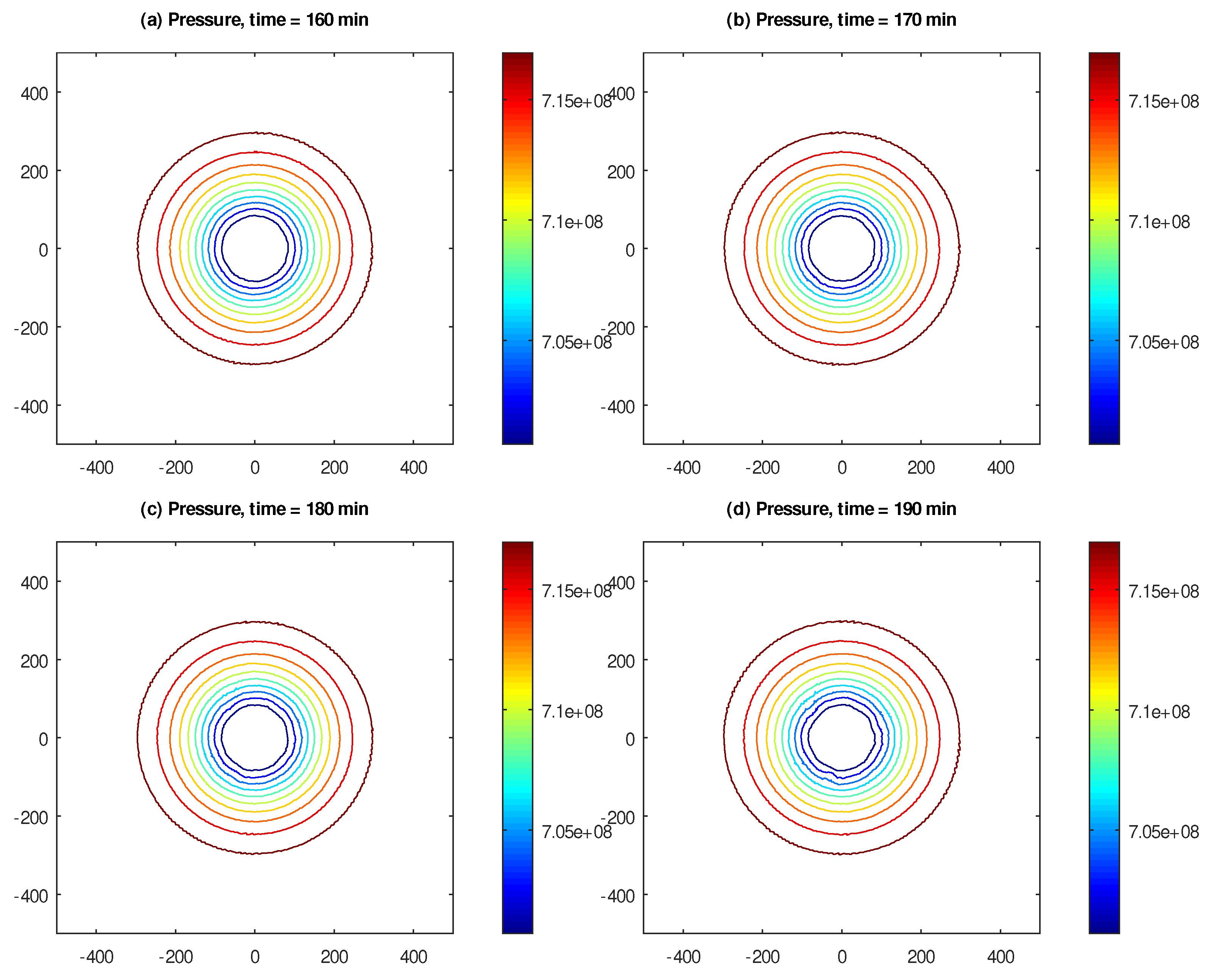
4.6. Numerical Simulation of a Cyclostrophic Vortex
5. Summary
Funding
Data Availability Statement
Conflicts of Interest
References
- Reynolds, O. An Experimental Investigation of the Circumstances which Determine whether the Motion of Water shall be Direct or Sinuous, and of the Law of Resistance in Parallel Channels. Philos. Trans. R. Soc. Lond. 1883, 35, 84–99. [Google Scholar]
- Kolmogorov, A. Local Structure of Turbulence in an Incompressible Fluid at Very High Reynolds Numbers. Dokl. Akad. Nauk SSSR 1941, 30, 299–303. [Google Scholar] [CrossRef]
- Kolmogorov, A. Decay of Isotropic Turbulence in an Incompressible Viscous Fluid. Dokl. Akad. Nauk SSSR 1941, 31, 538–541. [Google Scholar]
- Kolmogorov, A. Energy Dissipation in Locally Isotropic Turbulence. Dokl. Akad. Nauk SSSR 1941, 32, 19–21. [Google Scholar]
- Obukhov, A. On the Distribution of Energy in the Spectrum of a Turbulent Flow. Izv. Akad. Nauk. SSSR Seriya Geogr. Geofiz. 1941, 5, 453–466. [Google Scholar]
- Obukhov, A. Structure of the Temperature Field in Turbulent Flow. Izv. Akad. Nauk. SSSR Seriya Geogr. Geofiz. 1949, 13, 58–69. [Google Scholar]
- Obukhov, A. Some Specific Features of Atmospheric Turbulence. J. Geophys. Res. 1962, 67, 3011–3014. [Google Scholar] [CrossRef]
- Nastrom, G.; Gage, K. A Climatology of Atmospheric Wavenumber Spectra of Wind and Temperature Observed by Commercial Aircraft. J. Atmos. Sci. 1985, 42, 950–960. [Google Scholar] [CrossRef] [Green Version]
- Abramov, R. Macroscopic Turbulent Flow via Hard Sphere Potential. AIP Adv. 2021, 11, 085210. [Google Scholar] [CrossRef]
- Bogoliubov, N. Kinetic Equations. J. Exp. Theor. Phys. 1946, 16, 691–702. [Google Scholar]
- Born, M.; Green, H. A General Kinetic Theory of Liquids I: The Molecular Distribution Functions. Proc. R. Soc. Lond. Ser. A Math. Phys. Sci. 1946, 188, 10–18. [Google Scholar]
- Kirkwood, J. The Statistical Mechanical Theory of Transport Processes I: General Theory. J. Chem. Phys. 1946, 14, 180–201. [Google Scholar] [CrossRef]
- Vlasov, A. On Vibration Properties of Electron Gas. J. Exp. Theor. Phys. 1938, 8, 291. [Google Scholar]
- Batchelor, G. An Introduction to Fluid Dynamics; Cambridge University Press: New York, NY, USA, 2000. [Google Scholar]
- Golse, F. The Boltzmann Equation and Its Hydrodynamic Limits. In Handbook of Differential Equations: Evolutionary Equations; Elsevier: Amsterdam, The Netherlands, 2005; Volume 2, Chapter 3; pp. 159–301. [Google Scholar]
- Abramov, R. Formation of Turbulence via an Interaction Potential. arXiv 2021, arXiv:2011.10042. [Google Scholar]
- Rényi, A. On Measures of Entropy and Information. In Proceedings of the Fourth Berkeley Symposium on Mathematical Statistics and Probability, Volume 1: Contributions to the Theory of Statistics; University of California Press: Berkeley, CA, USA, 1961; pp. 547–561. [Google Scholar]
- Kullback, S.; Leibler, R. On Information and Sufficiency. Ann. Math. Stat. 1951, 22, 79–86. [Google Scholar] [CrossRef]
- Abramov, R. The Random Gas of Hard Spheres. J 2019, 2, 162–205. [Google Scholar] [CrossRef] [Green Version]
- Cercignani, C. The Boltzmann Equation and Its Applications. In Applied Mathematical Sciences; Springer: New York, NY, USA, 1988; Volume 67. [Google Scholar]
- Chapman, S.; Cowling, T. The Mathematical Theory of Non-Uniform Gases, 3rd ed.; Cambridge Mathematical Library, Cambridge University Press: New York, NY, USA, 1991. [Google Scholar]
- Grad, H. On the Kinetic Theory of Rarefied Gases. Commun. Pure Appl. Math. 1949, 2, 331–407. [Google Scholar] [CrossRef]
- Cercignani, C. Theory and Application of the Boltzmann Equation; Elsevier Science: New York, NY, USA, 1975. [Google Scholar]
- Hirschfelder, J.; Curtiss, C.; Bird, R. The Molecular Theory of Gases and Liquids; Wiley: Hoboken, NJ, USA, 1964. [Google Scholar]
- Jones, F. Interpolation Formulas for Viscosity of Six Gases: Air, Nitrogen, Carbon Dioxide, Helium, Argon and Oxygen; NBS Technical Note 1186; National Bureau of Standards: Washington, DC, USA, 1984. [Google Scholar]
- Weller, H.; Tabor, G.; Jasak, H.; Fureby, C. A Tensorial Approach to Computational Continuum Mechanics Using Object-Oriented Techniques. Comput. Phys. 1998, 12, 620–631. [Google Scholar] [CrossRef]
- Greenshields, C.; Weller, H.; Gasparini, L.; Reese, J. Implementation of Semi-Discrete, Non-Staggered Central Schemes in a Colocated, Polyhedral, Finite Volume Framework, for High-Speed Viscous Flows. Int. J. Numer. Methods Fluids 2010, 63, 1–21. [Google Scholar] [CrossRef] [Green Version]
- Kurganov, A.; Tadmor, E. New High-Resolution Central Schemes for Nonlinear Conservation Laws and Convection–Diffusion Equations. J. Comput. Phys. 2001, 160, 241–282. [Google Scholar] [CrossRef] [Green Version]
- Van Leer, B. Towards the Ultimate Conservative Difference Scheme, II: Monotonicity and Conservation Combined in a Second Order Scheme. J. Comput. Phys. 1974, 17, 361–370. [Google Scholar] [CrossRef]
- McCalpin, J.; Haidvogel, D. Phenomenology of the Low-Frequency Variability in a Reduced-Gravity, Quasigeostrophic Double-Gyre Model. J. Phys. Oceanogr. 1996, 26, 739–752. [Google Scholar] [CrossRef] [Green Version]
- Reynolds, O. On the Dynamical Theory of Incompressible Viscous Fluids and the Determination of the Criterion. Philos. Trans. R. Soc. A 1895, 186, 123–164. [Google Scholar]
- Von Kármán, T. Aerodynamics; McGraw-Hill: New York, NY, USA, 1963. [Google Scholar]
- Choi, D.; Showman, A. Power Spectral Analysis of Jupiter’s Clouds and Kinetic Energy from Cassini. Icarus 2011, 216, 597–609. [Google Scholar] [CrossRef] [Green Version]
- Moriconi, M.; Migliorini, A.; Altieri, F.; Adriani, A.; Mura, A.; Orton, G.; Lunine, J.; Grassi, D.; Atreya, S.; Ingersoll, A.; et al. Turbulence Power Spectra in Regions Surrounding Jupiter’s South Polar Cyclones from Juno/JIRAM. J. Geophys. Res. Planets 2020, 125, e2019JE006096. [Google Scholar] [CrossRef]





Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Abramov, R.V. Turbulence in Large-Scale Two-Dimensional Balanced Hard Sphere Gas Flow. Atmosphere 2021, 12, 1520. https://doi.org/10.3390/atmos12111520
Abramov RV. Turbulence in Large-Scale Two-Dimensional Balanced Hard Sphere Gas Flow. Atmosphere. 2021; 12(11):1520. https://doi.org/10.3390/atmos12111520
Chicago/Turabian StyleAbramov, Rafail V. 2021. "Turbulence in Large-Scale Two-Dimensional Balanced Hard Sphere Gas Flow" Atmosphere 12, no. 11: 1520. https://doi.org/10.3390/atmos12111520
APA StyleAbramov, R. V. (2021). Turbulence in Large-Scale Two-Dimensional Balanced Hard Sphere Gas Flow. Atmosphere, 12(11), 1520. https://doi.org/10.3390/atmos12111520





